Binder Jetting 3D Printing of Magnesium Oxychloride Cement-Based Materials: Parametric Analysis of Manufacturing Factors
Abstract
:Highlight
- Controllable parameters in the binder jetting printing process were determined, and experiments were designed and performed to investigate their effect on the mechanical properties of the final products.
- The modulus of rupture (MOR) was modeled as a function of its process parameters, including particle size, amount of binder, and layer thickness.
- In an SCA-product, there are two types of interfaces: interlayer and intervoxel; blocks printed with high fluid pressure have stronger interlayer connections than those printed with low pressure, resulting in higher MOR.
Abstract
1. Introduction
1.1. Deposition of the Powder Bed
1.2. Adjusting Layer Thickness
1.3. Jetting of the Liquid Binder
2. Experimental Designs and Methods
2.1. Material
2.2. 3D-Printing
2.3. Binder Liquid Flow Rate
2.4. Tests
3. Results and Discussions
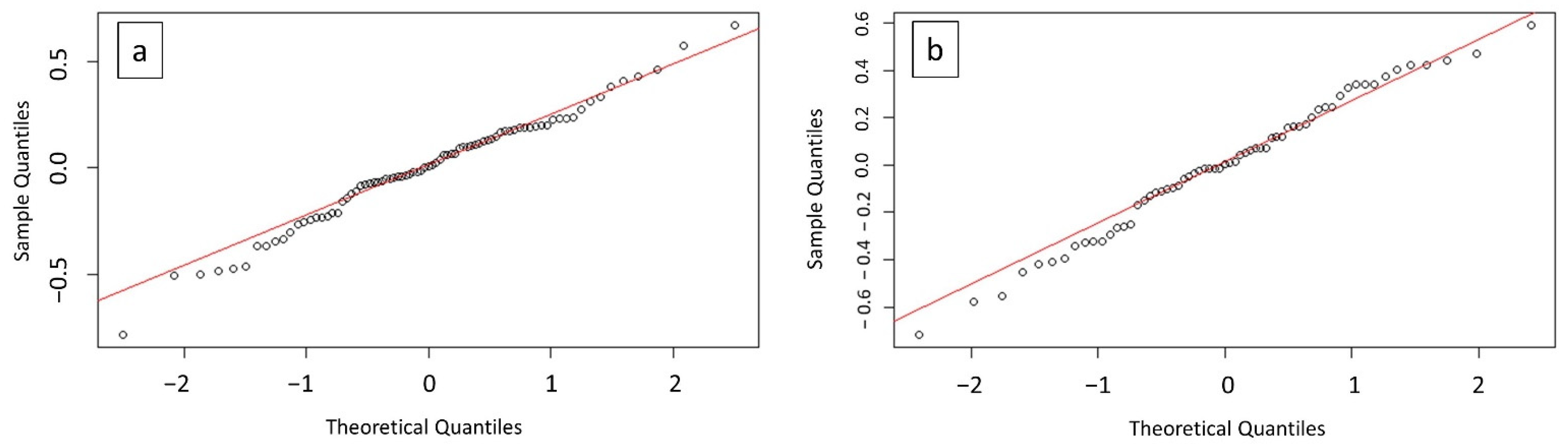
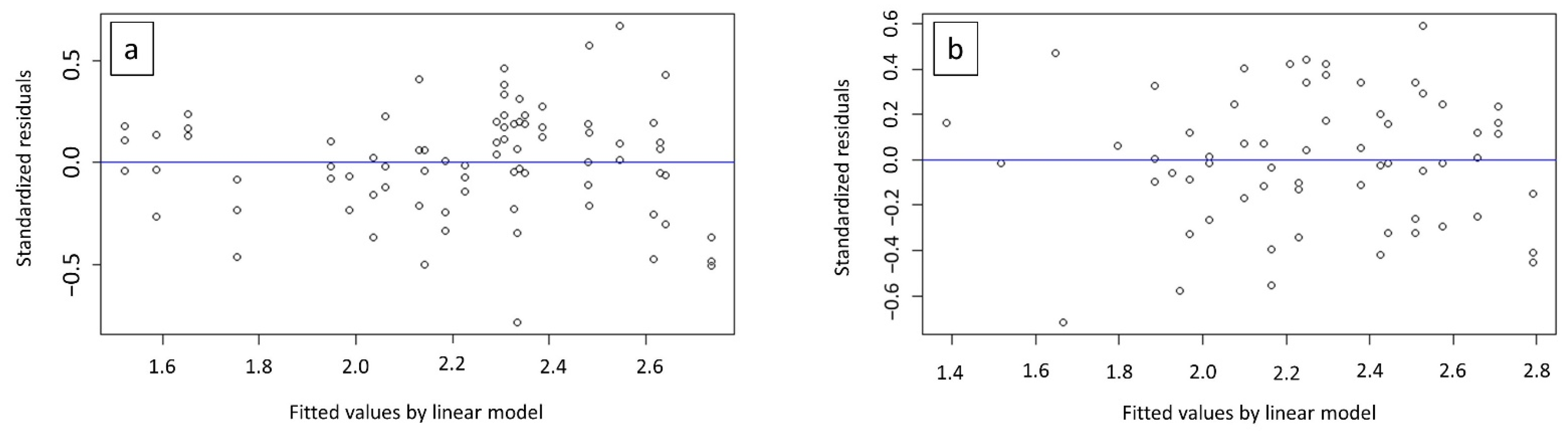
3.1. Statistical Analysis
3.2. XRD Results
3.3. Main Effect and ANOVA for Printing Inputs
3.3.1. Powder-Binder Interaction (PBI)
3.3.2. Main Effects
Effect of Particle Size and Velocity of Powder Spread
Effect of Layer Thickness and Hatch Distance
Effect of Amount of Binder
Feed Rate
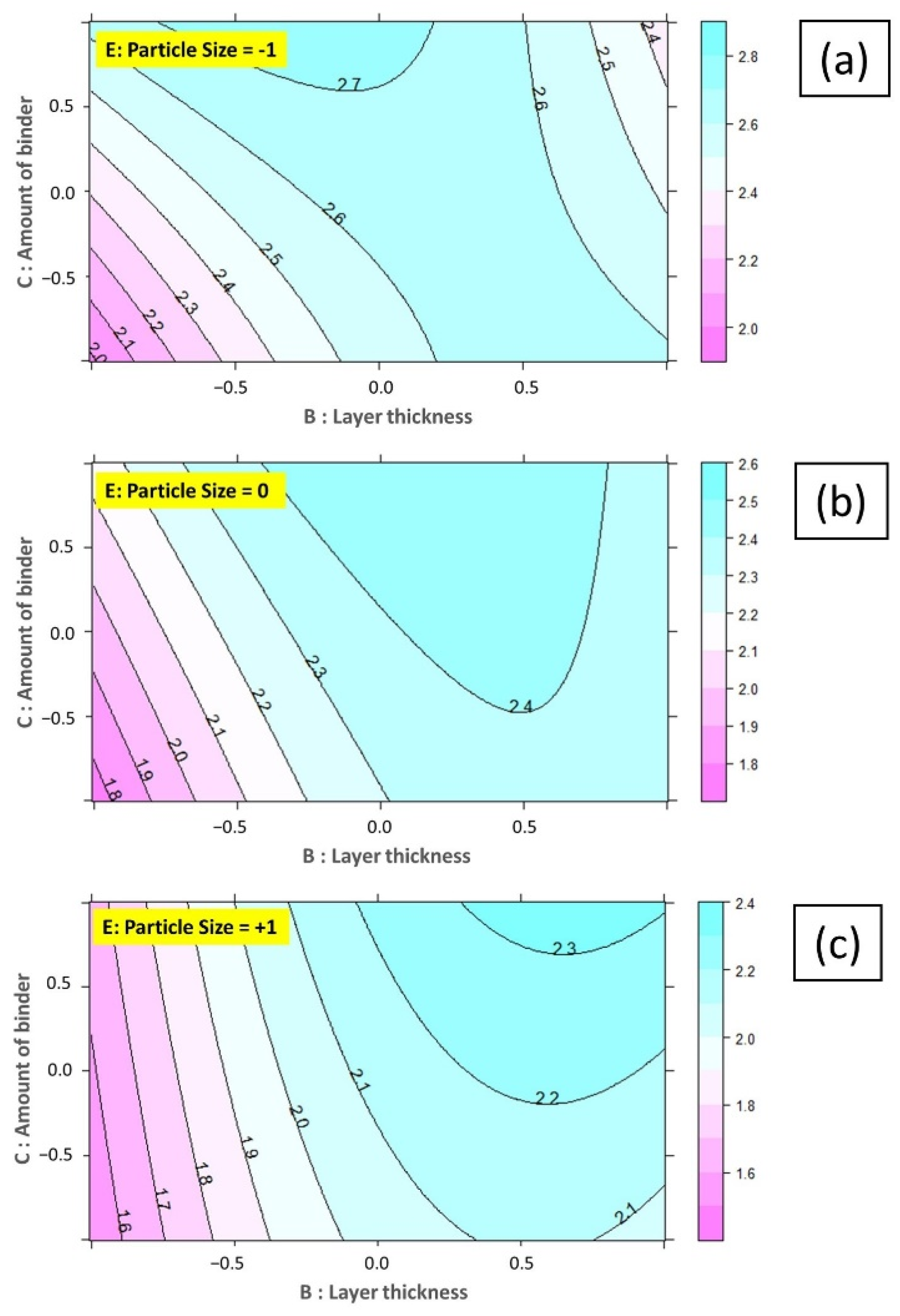
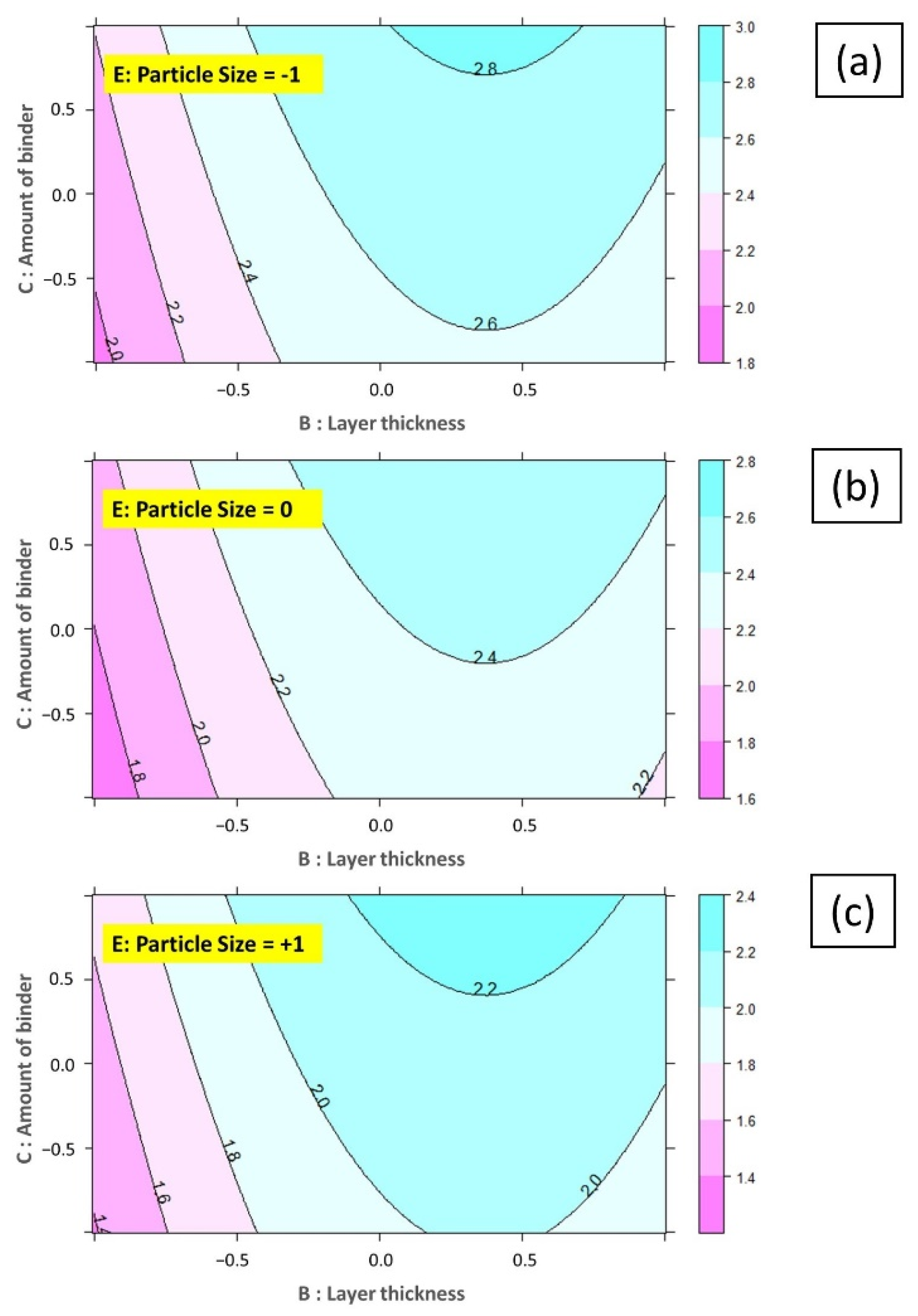
3.4. Interactions
4. Conclusions
- The modulus of rupture (MOR) was determined by the layer thickness, the amount of binder, the particle size, and the interaction between these factors. When the aim was to print better mechanical strength blocks, the feed rate and the velocity of powder spread were not significant factors. These parameters were selected freely, on the basis that the binder amount and the rate of voxel (rV) were kept constant.
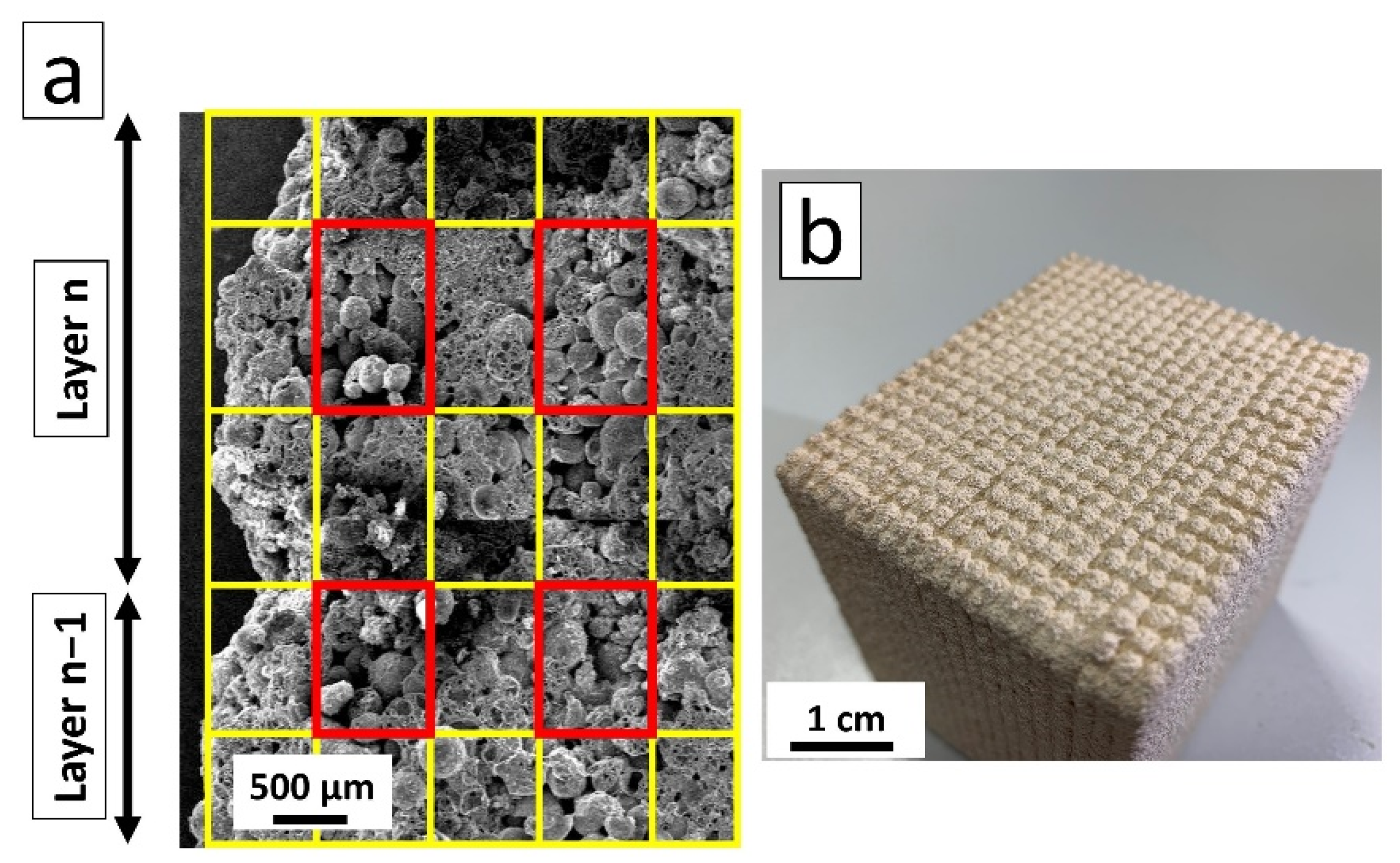
- The powder binder interaction (PBI), i.e., the interaction between jetted binder droplets and the deposited powder bed, determined the quality of the printed blocks. A sample was formed by merging rVs as single-line primitives, followed by connecting successive cross-sections; hence, inter-rV and interlayer connections were two types of interfaces in each printed component. The final products were orthotropic materials whose properties depended on the binder’s deposition direction.
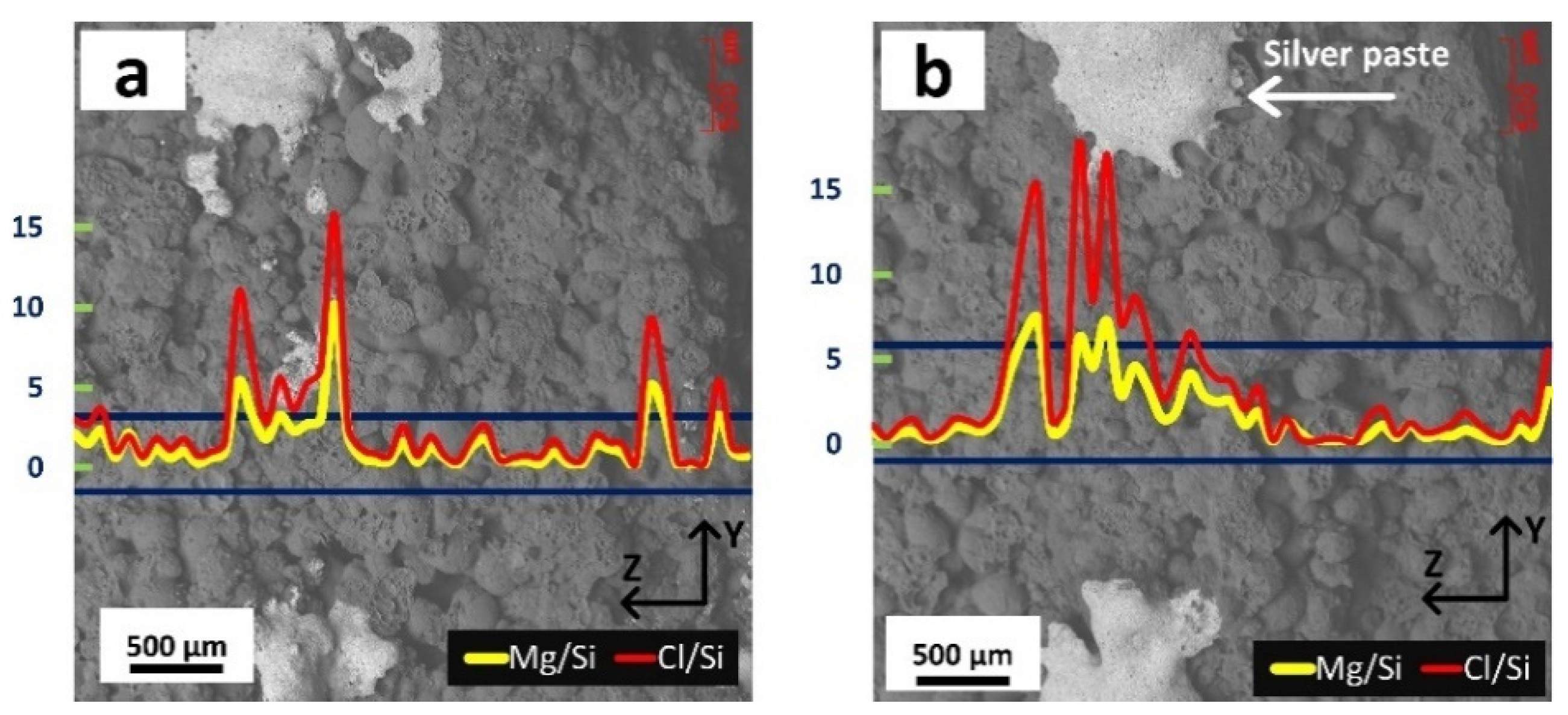
- Under high fluid pressure, single-line primitives were created through a crater formation mechanism, where the binder went deeper into the powder bed, but each layer was formed with macro porosities. At low fluid pressures, on the other hand, the spreading mechanism was dominant and the cement was distributed homogeneously throughout the printed part.
- The mechanical strength of SCA-produced products with an inorganic binder was proportional to the volume of the cementitious bonds ( among the aggregates. Finer particles generated a more compacted layer with more active surface area to form cementitious bonds. As a result, they ended up with a higher MOR. Furthermore, increasing the density of the powder bed with finer aggregates demanded higher flow rates, activating the crater mechanism and reinforcing the interlayer connections.
- In the SCA process, the AoB operated in a manner similar to that of the water-to-cement ratio in traditional concrete manufacturing. When this factor increased, the flow rate increased and, as a result, the fluid pressure rose, resulting in the formation of stronger interlayer connections.
- Statistical models were developed to predict MOR in three levels: as a function of layer thickness, the amount of binder, and particle size. The aim was to print the most robust products; hence, fine particle size combined with a high level of AoB factor, with the layer thickness maintained in mid-level, provided the best results for both Y0 and Y90 outcomes.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gibson, I.; Rosen, D.W.; Stucker, B.; Khorasani, M.; Rosen, D.; Stucker, B.; Khorasani, M. Additive Manufacturing Technologies; Springer: Cham, Switzerland, 2021; Volume 17. [Google Scholar]
- Xia, M.; Nematollahi, B.; Sanjayan, J. Printability, accuracy and strength of geopolymer made using powder-based 3D printing for construction applications. Autom. Constr. 2019, 101, 179–189. [Google Scholar] [CrossRef]
- Goh, G.L.; Dikshit, V.; Koneru, R.; Peh, Z.K.; Lu, W.; Goh, G.D.; Yeong, W.Y. Fabrication of design-optimized multifunctional safety cage with conformal circuits for drone using hybrid 3D printing technology. Int. J. Adv. Manuf. Technol. 2022, 120, 2573–2586. [Google Scholar] [CrossRef]
- Collins, M.N.; Ren, G.; Young, K.; Pina, S.; Reis, R.L.; Oliveira, J.M. Scaffold Fabrication Technologies and Structure/Function Properties in Bone Tissue Engineering. Adv. Funct. Mater. 2021, 31, 2010609. [Google Scholar] [CrossRef]
- Zheng, Y.; Hong, X.; Wang, J.; Feng, L.; Fan, T.; Guo, R.; Zhang, H. 2D Nanomaterials for Tissue Engineering and Regenerative Nanomedicines: Recent Advances and Future Challenges. Adv. Health Mater. 2021, 10, 2001743. [Google Scholar] [CrossRef]
- Keneth, E.S.; Kamyshny, A.; Totaro, M.; Beccai, L.; Magdassi, S. 3D Printing Materials for Soft Robotics. Adv. Mater. 2020, 33, e2003387. [Google Scholar] [CrossRef] [PubMed]
- Sinha, A.K.; Goh, G.L.; Yeong, W.Y.; Cai, Y. Ultra-Low-Cost, Crosstalk-Free, Fast-Responding, Wide-Sensing-Range Tactile Fingertip Sensor for Smart Gloves. Adv. Mater. Interfaces 2022, 9, 2200621. [Google Scholar] [CrossRef]
- Xia, M.; Sanjayan, J. Method of formulating geopolymer for 3D printing for construction applications. Mater. Des. 2016, 110, 382–390. [Google Scholar] [CrossRef]
- Lowke, D.; Dini, E.; Perrot, A.; Weger, D.; Gehlen, C.; Dillenburger, B. Particle-bed 3D printing in concrete construction—Possibilities and challenges. Cem. Concr. Res. 2018, 112, 50–65. [Google Scholar] [CrossRef]
- Nematollahi, B.; Xia, M.; Sanjayan, J. Current Progress of 3D Concrete Printing Technologies. In ISARC. Proceedings of the International Symposium on Automation and Robotics in Construction; IAARC Publications: Taipei, Taiwan, 2017; Volume 34. [Google Scholar]
- Xia, M.; Nematollahi, B.; Sanjayan, J. Influence of Binder Saturation Level on Compressive Strength and Dimensional Accuracy of Powder-Based 3D Printed Geopolymer. In Materials Science Forum; Trans Tech Publications: Zurich, Switzerland, 2018; Volume 939, pp. 177–183. [Google Scholar]
- Shakor, P.; Chu, S.H.; Puzatova, A.; Dini, E. Review of binder jetting 3D printing in the construction industry. Prog. Addit. Manuf. 2022, 1–27. [Google Scholar] [CrossRef]
- Shrestha, S.; Manogharan, G. Optimization of Binder Jetting Using Taguchi Method. JOM 2017, 69, 491–497. [Google Scholar] [CrossRef]
- Lowke, D.; Talke, D.; Dressler, I.; Weger, D.; Gehlen, C.; Ostertag, C.; Rael, R. Particle Bed 3D Printing by Selective Cement Activation–Applications, Material and Process Technology. Cem. Concr. Res. 2020, 134, 106077. [Google Scholar] [CrossRef]
- Pierre, A.; Perrot, A. 3D Printing by Selective Binding in a Particle Bed: Principles and Challenges. In 3D Printing of Concrete: State of the Art and Challenges of the Digital Construction Revolution; John Wiley & Sons: Hoboken, NJ, USA, 2019; pp. 73–99. [Google Scholar]
- Walling, S.A.; Provis, J.L. Magnesia-Based Cements: A Journey of 150 Years, and Cements for the Future? Chem. Rev. 2016, 116, 4170–4204. [Google Scholar] [CrossRef] [PubMed]
- Góchez, R.; Wambaugh, J.; Rochner, B.; Kitchens, C.L. Kinetic Study of the Magnesium Oxychloride Cement Cure Reaction. J. Mater. Sci. 2017, 52, 7637–7646. [Google Scholar] [CrossRef]
- Sglavo, V.M.; De Genua, F.; Conci, A.; Ceccato, R.; Cavallini, R. Influence of Curing Temperature on the Evolution of Magnesium Oxychloride Cement. J. Mater. Sci. 2011, 46, 6726–6733. [Google Scholar] [CrossRef]
- Xu, B.; Ma, H.; Hu, C.; Yang, S.; Li, Z. Influence of Curing Regimes on Mechanical Properties of Magnesium Oxychloride Cement-Based Composites. Constr. Build. Mater. 2016, 102, 613–619. [Google Scholar] [CrossRef]
- Dini, F.; Ghaffari, S.A.; Jafar, J.; Hamidreza, R.; Marjan, S. A Review of Binder Jet Process Parameters; Powder, Binder, Printing and Sintering Condition. Met. Powder Rep. 2020, 75, 95–100. [Google Scholar] [CrossRef]
- Utela, B.; Storti, D.; Anderson, R.; Ganter, M. A Review of Process Development Steps for New Material Systems in Three Dimensional Printing (3DP). J. Manuf. Processes 2008, 10, 96–104. [Google Scholar] [CrossRef]
- Lv, X.; Ye, F.; Cheng, L.; Fan, S.; Liu, Y. Binder Jetting of Ceramics: Powders, Binders, Printing Parameters, Equipment, and Post-Treatment. Ceram. Int. 2019, 45, 12609–12624. [Google Scholar] [CrossRef]
- Miyanaji, H.; Momenzadeh, N.; Yang, L. Effect of Printing Speed on Quality of Printed Parts in Binder Jetting Process. Addit. Manuf. 2018, 20, 1–10. [Google Scholar] [CrossRef]
- Mostafaei, A.; Elliott, A.M.; Barnes, J.E.; Li, F.; Tan, W.; Cramer, C.L.; Nandwana, P.; Chmielus, M. Binder Jet 3D Printing—Process Parameters, Materials, Properties, Modeling, and Challenges. Prog. Mater. Sci. 2021, 119, 100707. [Google Scholar] [CrossRef]
- Vaezi, M.; Chua, C.K. Effects of Layer Thickness and Binder Saturation Level Parameters on 3D Printing Process. Int. J. Adv. Manuf. Technol. 2011, 53, 275–284. [Google Scholar] [CrossRef]
- Farzadi, A.; Solati-Hashjin, M.; Asadi-Eydivand, M.; Abu Osman, N.A. Effect of Layer Thickness and Printing Orientation on Mechanical Properties and Dimensional Accuracy of 3D Printed Porous Samples for Bone Tissue Engineering. PLoS ONE 2014, 9, e108252. [Google Scholar] [CrossRef] [PubMed]
- Gonzalez, J.; Mireles, J.; Lin, Y.; Wicker, R. Characterization of ceramic components fabricated using binder jetting additive manufacturing technology. Ceram. Int. 2016, 42, 10559–10564. [Google Scholar] [CrossRef] [Green Version]
- Yao, A.W.; Tseng, Y. A robust process optimization for a powder type rapid prototyper. Rapid Prototyp. J. 2002, 8, 180–189. [Google Scholar] [CrossRef]
- Kumar, A.; Bai, Y.; Eklund, A.; Williams, C.B. Effects of Hot Isostatic Pressing on Copper Parts Fabricated via Binder Jet-ting. Procedia Manuf. 2017, 10, 935–944. [Google Scholar] [CrossRef]
- Derby, B. Additive Manufacture of Ceramics Components by Inkjet Printing. Engineering 2015, 1, 113–123. [Google Scholar] [CrossRef] [Green Version]
- Ingaglio, J.; Fox, J.; Naito, C.J.; Bocchini, P. Material characteristics of binder jet 3D printed hydrated CSA cement with the addition of fine aggregates. Constr. Build. Mater. 2019, 206, 494–503. [Google Scholar] [CrossRef]
- Günther, D.; Mögele, F. Additive Manufacturing of Casting Tools Using Powder-Binder- Jetting Technology. New Trends 3D Print. 2016, 53–86. [Google Scholar] [CrossRef] [Green Version]
- Ramakrishnan, R.; Griebel, B.; Volk, W.; Günther, D.; Günther, J. 3D Printing of Inorganic Sand Moulds for Casting Applica-tions. In Advanced Materials Research; Trans Tech Publications: Zurich, Switzerland, 2014; Volume 1018, pp. 441–449. [Google Scholar]
- Miyanaji, H. Binder Jetting Additive Manufacturing Process Fundamentals and the Resultant Influences on Part Quality. Ph.D. Dissertation, University of Louisville, Louisville, KY, USA, 2019. [Google Scholar] [CrossRef]
- Zhao, H.; Ye, C.; Fan, Z.; Wang, C. 3D Printing of Cao-Based Ceramic Core Using Nanozirconia Suspension as a Binder. J. Eur. Ceram. Soc. 2017, 37, 5119–5125. [Google Scholar] [CrossRef]
- Huang, S.-J.; Ye, C.-S.; Zhao, H.-P.; Fan, Z.-T. Parameters optimization of binder jetting process using modified silicate as a binder. Mater. Manuf. Process. 2019, 35, 214–220. [Google Scholar] [CrossRef]
- Lu, K.; Hiser, M.; Wu, W. Effect of particle size on three dimensional printed mesh structures. Powder Technol. 2009, 192, 178–183. [Google Scholar] [CrossRef]
- Weger, D.; Lowke, D.; Gehlen, C. 3D Printing of Concrete Structures Using the Selective Binding Method–Effect of Concrete Technology on Contour Precision and Compressive Strength. In Proceedings of the 11th fib International PhD Symposium in Civil Engineering, Tokyo, Japan, 29–31 August 2016; pp. 1–8. [Google Scholar]
- Craveiroa, F.; Duartec, J.P.; Bartoloa, H.; Bartolod, P.J. Additive Manufacturing as an Enabling Technology for Digital Construction: A Perspective on Construction 4.0. Sustain. Dev. 2019, 4, 6. [Google Scholar] [CrossRef]
- American Society for Testing and Materials. ASTM C293, Standard Test Method for Flexural Strength of Concrete Using Simple Beam with Center Point Loading; ASTM: West Conshohocken, PA, USA, 2002. [Google Scholar]
- Montgomery, D.C. Design and Analysis of Experiments; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]
- Gunst, R.F.; Mason, R.L. Fractional Factorial Design. Wiley Interdiscip. Rev. Comput. Stat. 2009, 1, 234–244. [Google Scholar] [CrossRef]
- Team RStudio. RStudio: Integrated Development Environment for R; PBC: Boston, MA, USA, 2020. [Google Scholar]
- Chandrasekaran, R.R.; Benoit, M.J.; Barrett, J.M.; Gerlich, A.P. Multi-Variable Statistical Models for Predicting Bead Geometry in Gas Metal Arc Welding. Int. J. Adv. Manuf. Technol. 2019, 105, 1573–1584. [Google Scholar] [CrossRef]
- Lutterotti, L.; Ceccato, R.; Dal Maschio, R.; Pagani, E. Quantitative Analysis of Silicate Glass in Ceramic Materials by the Rietveld Method. In Materials Science Forum; Trans Tech Publications: Aedermannsdorf, Switzerland, 1998; Volume 278, pp. 87–92. [Google Scholar]
- Lutterotti, L.; Bortolotti, M.; Ischia, G.; Lonardelli, I.; Wenk, H. Rietveld Texture Analysis from Diffraction Images. Z. Kristallogr. 2007, 26, 125–130. [Google Scholar] [CrossRef]
- Xiao, J.; Liu, H.; Ding, T. Finite Element Analysis on the Anisotropic Behavior of 3D Printed Concrete under Compression and Flexure. Addit. Manuf. 2020, 39, 101712. [Google Scholar] [CrossRef]
- Ford, C. Understanding Qq Plots. Access Date 2015, 4, 18. [Google Scholar]
- Lanzetta, M.; Sachs, E. The Line Formation with Alumina Powders in Drop on Demand Three Dimensional Printing. In PRIME 2001, 1st Int. CIRP Seminar on: PRogress in Innovative Manufacturing Engineering; Dipartimento di Ingegneria della Produzione, Università di Genova: Genoa, Italy, 2001; pp. 189–196. [Google Scholar]
- Miyanaji, H.; Zhang, S.; Yang, L. A New Physics-Based Model for Equilibrium Saturation Determination in Binder Jetting Additive Manufacturing Process. Int. J. Mach. Tools Manuf. 2018, 124, 1–11. [Google Scholar] [CrossRef]
- Emady, H.N.; Kayrak-Talay, D.; Litster, J.D. A Regime Map for Granule Formation by Drop Impact on Powder Beds. AIChE J. 2013, 59, 96–107. [Google Scholar] [CrossRef]
- Emady, H.N.; Kayrak-Talay, D.; Litster, J.D. Modeling the Granule Formation Mechanism from Single Drop Impact on a Powder Bed. J. Colloid Interface Sci. 2013, 393, 369–376. [Google Scholar] [CrossRef] [Green Version]
- Salari, F.; Bosetti, P.; Sglavo, V.M. Binder Jet 3D Printing of Magnesium Oxychloride Cement-Based Concrete: A Framework to Design the Rate of Voxel. In Key Engineering Materials; Trans Tech Publications: Zurich, Switzerland, 2022; Volume 919, pp. 3–14. [Google Scholar]
- Boyce, C.M.; Ozel, A.; Sundaresan, S. Intrusion of a Liquid Droplet into a Powder Under Gravity. Langmuir 2016, 32, 8631–8640. [Google Scholar] [CrossRef] [PubMed]
- Bai, Y.; Wall, C.; Pham, H.; Esker, A.; Williams, C.B. Characterizing Binder–Powder Interaction in Binder Jetting Additive Manufacturing via Sessile Drop Goniometry. J. Manuf. Sci. Eng. 2019, 141. [Google Scholar] [CrossRef]
- Hapgood, K.P.; Litster, J.D.; Biggs, S.R.; Howes, T. Drop Penetration into Porous Powder Beds. J. Colloid Interface Sci. 2002, 253, 353–366. [Google Scholar] [CrossRef] [PubMed]
- Wagner, J.J.; Shu, H.; Kilambi, R. Experimental Investigation of Fluid-Particle Interaction in Binder Jet 3D Printing. Preprints 2021, 2021010546. [Google Scholar]
- Mangulkar, M.; Jamkar, S. Review of Particle Packing Theories Used for Concrete Mix Proportioning. Contrib. Pap. 2013, 141. [Google Scholar]
- Mirzababaei, S.; Pasebani, S. A Review on Binder Jet Additive Manufacturing of 316L Stainless Steel. J. Manuf. Mater. Processing 2019, 3, 82. [Google Scholar] [CrossRef] [Green Version]
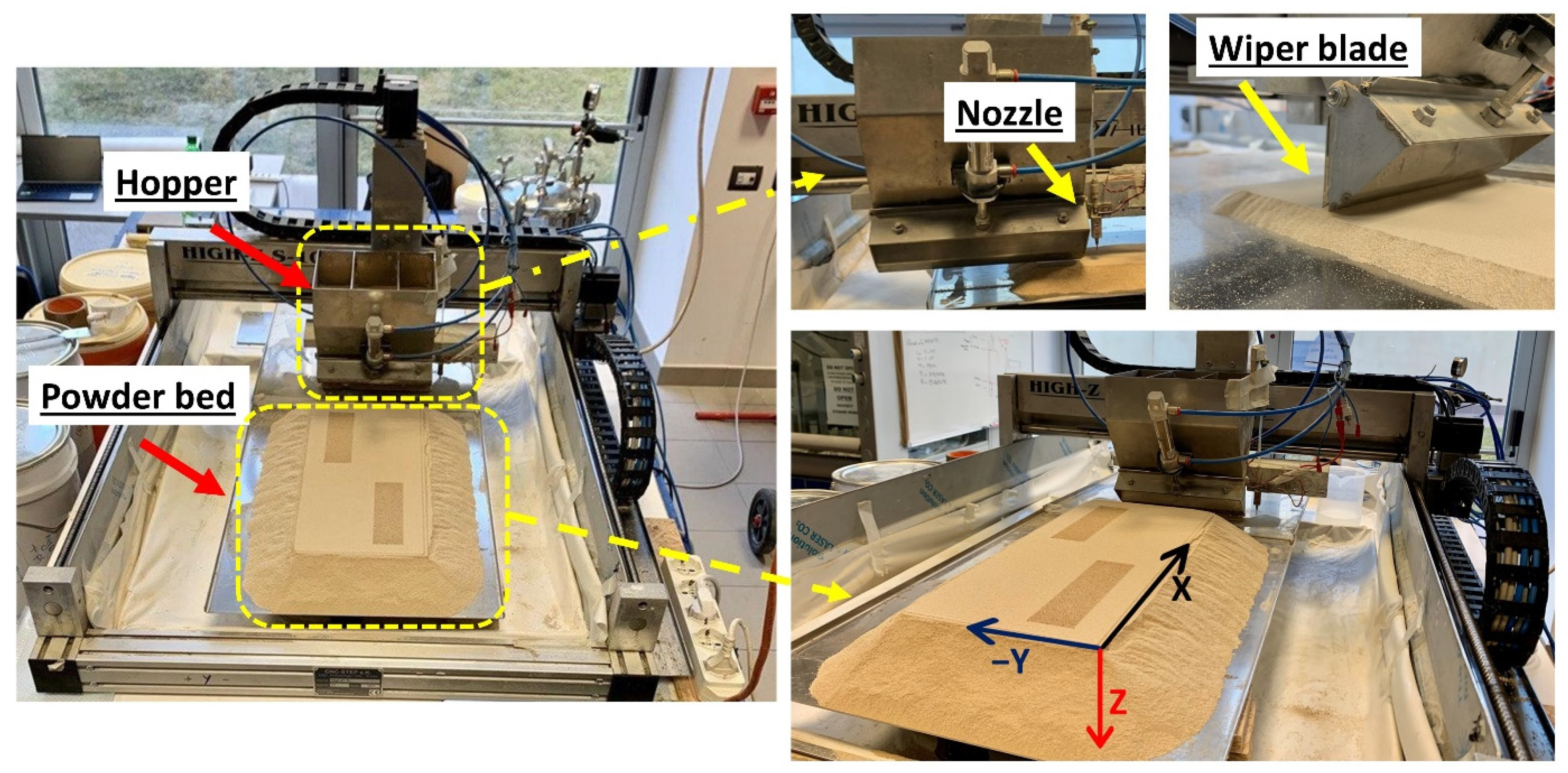
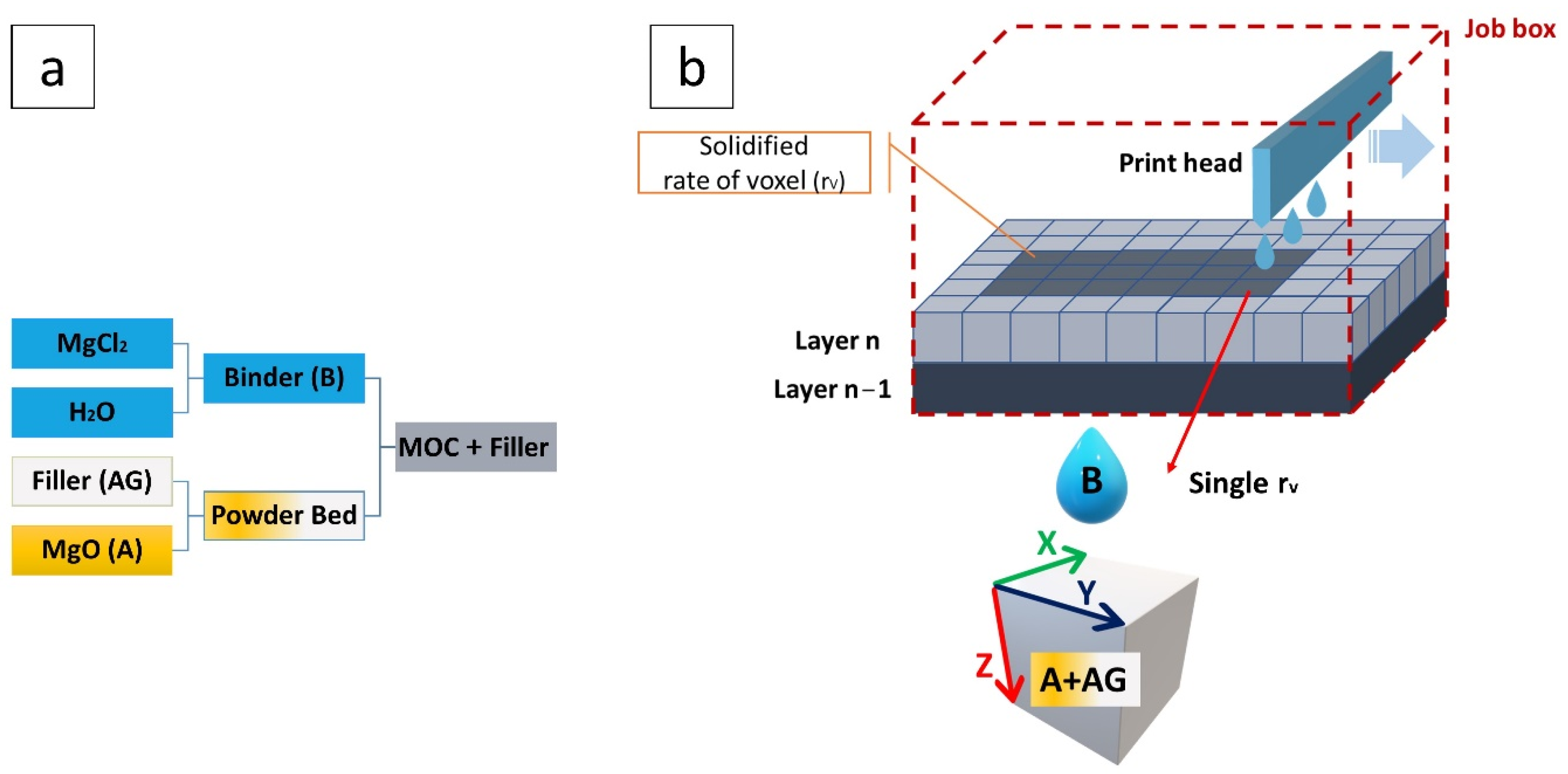










| Composition | Content (wt%) |
|---|---|
| MgCl2.6H2O | 49.5 |
| H2O | 49.5 |
| Rice starch | 1 |
| Composition | Content (wt%) |
|---|---|
| Porous glass | 60.8 |
| MgO | 36.5 |
| Methylcellulose | 1.5 |
| H3PO4 | 1.2 |
| Label | Factor | Abbreviation | Unit | Level | Description | ||
|---|---|---|---|---|---|---|---|
| Low (−1) | Center Point (0) | High (+1) | |||||
| A | Feed Rate | FR | mm/min | 7200 | 7600 | 8000 | velocity of the nozzle, installed on printer head, as it dispenses binder agent |
| B | Layer Thickness | L | mm | 2 | 2.25 | 2.5 | distance the printer head is raised at the start of a new layer |
| C | Amount of Binder Liquid | AoB | wt% | 90 | 95 | 101 | relative amount of binder |
| D | Velocity of Powder Spread | V | mm/min | 1500 | 1750 | 2000 | velocity of the hopper when spreading a layer powder bed |
| E | Particle Size | PS | mm | (0.1–0.3) | Mix * | (0.25–0.5) | grain sizes of porous glass aggregates |
| F | Hatch Distance | H | mm | 1 | 1.25 | 1.5 | distance between successive tracks |
| Run | Treatment | Factor | |||||
|---|---|---|---|---|---|---|---|
| A | B | C | D | E | F | ||
| 1 | 1 | −1 | −1 | −1 | −1 | −1 | −1 |
| 2 | 2 | −1 | −1 | −1 | −1 | −1 | −1 |
| 3 | 3 | −1 | −1 | −1 | −1 | −1 | −1 |
| 4 | 1 | 1 | −1 | −1 | −1 | −1 | 1 |
| 5 | 2 | 1 | −1 | −1 | −1 | −1 | 1 |
| 6 | 3 | 1 | −1 | −1 | −1 | −1 | 1 |
| 7 | 1 | −1 | 1 | −1 | −1 | −1 | 1 |
| 8 | 2 | −1 | 1 | −1 | −1 | −1 | 1 |
| 9 | 3 | −1 | 1 | −1 | −1 | −1 | 1 |
| 10 | 1 | 1 | 1 | −1 | −1 | −1 | −1 |
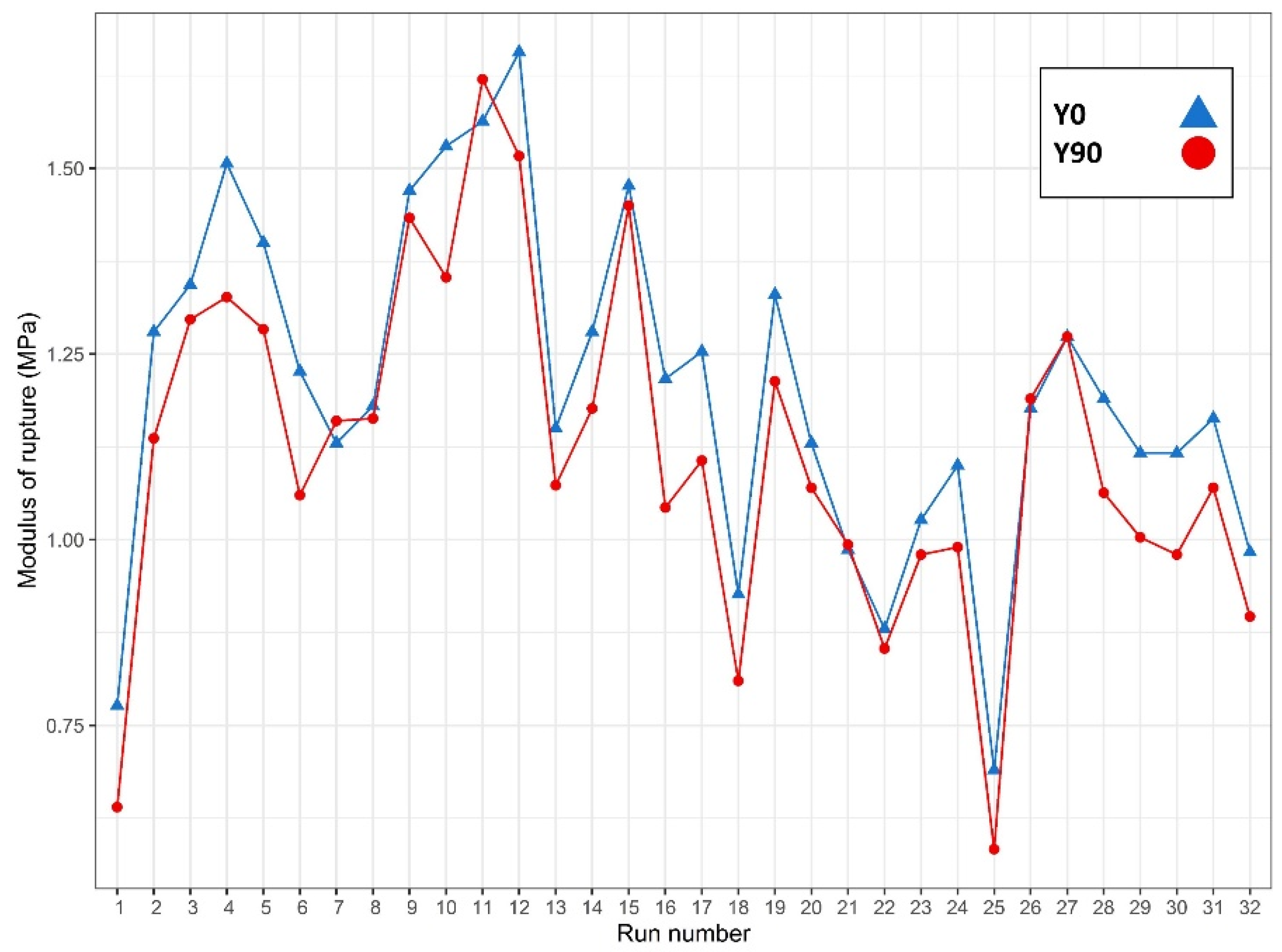
| Run Number | Printing Input Parameters * | Modulus of Rupture (MPa) | ||||||
|---|---|---|---|---|---|---|---|---|
| FR | L | AoB | V | PS | H | Y0 | Y90 | |
| 1 | 7200 | 2 | 0.9 | 1500 | P013 | 1 | 0.78 | 0.64 |
| 2 | 8000 | 2 | 0.9 | 1500 | P013 | 1.5 | 1.28 | 1.14 |
| 3 | 7200 | 2.5 | 0.9 | 1500 | P013 | 1.5 | 1.34 | 1.30 |
| 4 | 8000 | 2.5 | 0.9 | 1500 | P013 | 1 | 1.51 | 1.33 |
| 5 | 7200 | 2 | 1.01 | 1500 | P013 | 1.5 | 1.40 | 1.28 |
| 6 | 8000 | 2 | 1.01 | 1500 | P013 | 1 | 1.23 | 1.06 |
| 7 | 7200 | 2.5 | 1.01 | 1500 | P013 | 1 | 1.13 | 1.16 |
| 8 | 8000 | 2.5 | 1.01 | 1500 | P013 | 1.5 | 1.18 | 1.16 |
| 9 | 7200 | 2 | 0.9 | 2000 | P013 | 1.5 | 1.47 | 1.43 |
| 10 | 8000 | 2 | 0.9 | 2000 | P013 | 1 | 1.53 | 1.35 |
| 11 | 7200 | 2.5 | 0.9 | 2000 | P013 | 1 | 1.56 | 1.62 |
| 12 | 8000 | 2.5 | 0.9 | 2000 | P013 | 1.5 | 1.66 | 1.52 |
| 13 | 7200 | 2 | 1.01 | 2000 | P013 | 1 | 1.15 | 1.07 |
| 14 | 8000 | 2 | 1.01 | 2000 | P013 | 1.5 | 1.28 | 1.18 |
| 15 | 7200 | 2.5 | 1.01 | 2000 | P013 | 1.5 | 1.48 | 1.45 |
| 16 | 8000 | 2.5 | 1.01 | 2000 | P013 | 1 | 1.22 | 1.04 |
| 17 | 7200 | 2 | 0.9 | 1500 | P255 | 1.5 | 1.25 | 1.11 |
| 18 | 8000 | 2 | 0.9 | 1500 | P255 | 1 | 0.93 | 0.81 |
| 19 | 7200 | 2.5 | 0.9 | 1500 | P255 | 1 | 1.33 | 1.21 |
| 20 | 8000 | 2.5 | 0.9 | 1500 | P255 | 1.5 | 1.13 | 1.07 |
| 21 | 7200 | 2 | 1.01 | 1500 | P255 | 1 | 0.99 | 0.99 |
| 22 | 8000 | 2 | 1.01 | 1500 | P255 | 1.5 | 0.88 | 0.85 |
| 23 | 7200 | 2.5 | 1.01 | 1500 | P255 | 1.5 | 1.03 | 0.98 |
| 24 | 8000 | 2.5 | 1.01 | 1500 | P255 | 1 | 1.10 | 0.99 |
| 25 | 7200 | 2 | 0.9 | 2000 | P255 | 1 | 0.69 | 0.58 |
| 26 | 8000 | 2 | 0.9 | 2000 | P255 | 1.5 | 1.18 | 1.19 |
| 27 | 7200 | 2.5 | 0.9 | 2000 | P255 | 1.5 | 1.27 | 1.27 |
| 28 | 8000 | 2.5 | 0.9 | 2000 | P255 | 1 | 1.19 | 1.06 |
| 29 | 7200 | 2 | 1.01 | 2000 | P255 | 1.5 | 1.12 | 1.00 |
| 30 | 8000 | 2 | 1.01 | 2000 | P255 | 1 | 1.12 | 0.98 |
| 31 | 7200 | 2.5 | 1.01 | 2000 | P255 | 1 | 1.16 | 1.07 |
| 32 | 8000 | 2.5 | 1.01 | 2000 | P255 | 1.5 | 0.98 | 0.90 |
| Center point | 7600 | 2.25 | 1 | 1750 | Mix | 1.25 | 1.56 | 1.74 |
| Center point | 7600 | 2.25 | 1 | 1750 | Mix | 1.25 | 1.74 | 1.88 |
| Center point | 7600 | 2.25 | 1 | 1750 | Mix | 1.25 | 1.67 | 1.70 |
| Center point | 7600 | 2.25 | 1 | 1750 | Mix | 1.25 | 1.71 | 1.84 |
| Center point | 7600 | 2.25 | 1 | 1750 | Mix | 1.25 | 1.58 | 1.85 |
| Center point | 7600 | 2.25 | 1 | 1750 | Mix | 1.25 | 1.68 | 1.75 |
| Label | Factor | Abbreviation | Unit | Level | ||
|---|---|---|---|---|---|---|
| Low (−1) | Midpoint (0) | High (+1) | ||||
| B | Layer Thickness | L | mm | 1.5 | 2 | 2.5 |
| C | Amount of Binder Liquid | AoB | wt% | 90 | 95 | 101 |
| E | Particle Size | PS | mm | (0.1–0.3) | Mix | (0.25–0.5) |
| Factor | Degree of Freedom | Sum of Square | F-Value | Pr (>F) |
|---|---|---|---|---|
| B2 | 1 | 1.08 | 14.5443 | 0.0003 |
| B | 1 | 2.02 | 27.0476 | 1.7 × 10−6 |
| C | 1 | 0.48 | 6.4546 | 0.0131 |
| E | 1 | 3.52 | 47.1907 | 1.8 × 10−9 |
| B:C | 1 | 0.36 | 4.7974 | 0.0317 |
| B:E | 1 | 0.40 | 5.4034 | 0.0229 |
| B:C:E | 1 | 0.40 | 5.3312 | 0.0238 |
| Residuals | 73 | 5.45 |
| Factor | Degree of Freedom | Sum of Square | F-Value | Pr (>F) |
|---|---|---|---|---|
| B2 | 1 | 1.5 | 17.2056 | 0.0001 |
| B | 1 | 0.85 | 9.7881 | 7.8 × 10−8 |
| C | 1 | 0.72 | 8.2915 | 0.0056 |
| E | 1 | 3.29 | 37.7698 | 0.0027 |
| Residuals | 58 |
| Coefficients | Estimate |
|---|---|
| 2.38593 | |
| 0.19333 | |
| 0.09444 | |
| −0.25537 | |
| −0.09972 | |
| 0.10583 | |
| 0.12875 | |
| −0.24556 |
| Coefficients | Estimate |
|---|---|
| 2.3796 | |
| 0.2496 | |
| 0.1312 | |
| −0.2800 | |
| −0.3333 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salari, F.; Bosetti, P.; Sglavo, V.M. Binder Jetting 3D Printing of Magnesium Oxychloride Cement-Based Materials: Parametric Analysis of Manufacturing Factors. J. Manuf. Mater. Process. 2022, 6, 86. https://doi.org/10.3390/jmmp6040086
Salari F, Bosetti P, Sglavo VM. Binder Jetting 3D Printing of Magnesium Oxychloride Cement-Based Materials: Parametric Analysis of Manufacturing Factors. Journal of Manufacturing and Materials Processing. 2022; 6(4):86. https://doi.org/10.3390/jmmp6040086
Chicago/Turabian StyleSalari, Farid, Paolo Bosetti, and Vincenzo M. Sglavo. 2022. "Binder Jetting 3D Printing of Magnesium Oxychloride Cement-Based Materials: Parametric Analysis of Manufacturing Factors" Journal of Manufacturing and Materials Processing 6, no. 4: 86. https://doi.org/10.3390/jmmp6040086
APA StyleSalari, F., Bosetti, P., & Sglavo, V. M. (2022). Binder Jetting 3D Printing of Magnesium Oxychloride Cement-Based Materials: Parametric Analysis of Manufacturing Factors. Journal of Manufacturing and Materials Processing, 6(4), 86. https://doi.org/10.3390/jmmp6040086








