The Dimensional Accuracy of Thin-Walled Parts Manufactured by Laser-Powder Bed Fusion Process
Abstract
1. Introduction
2. Materials and Methods
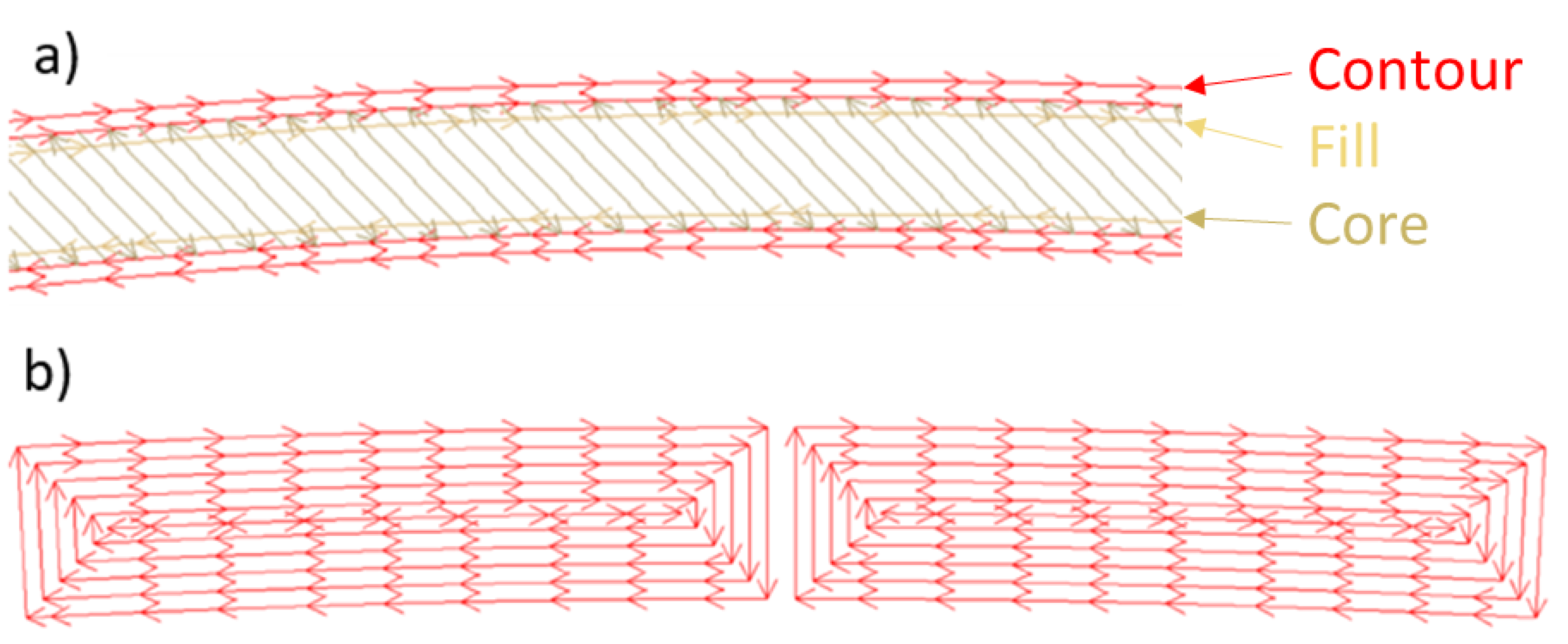
2.1. Printing Strategies
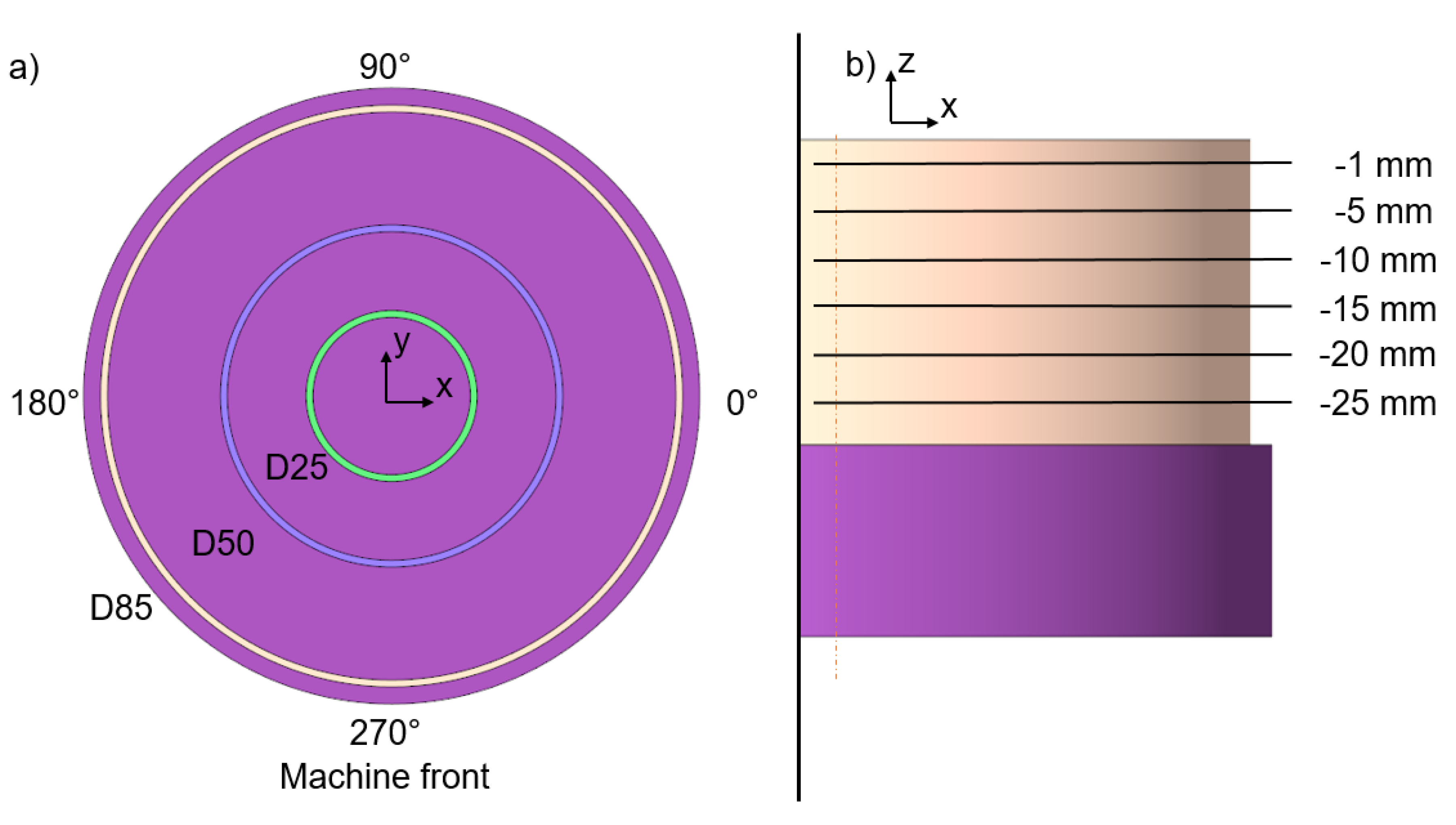
2.2. Specimens
2.3. Manufacturing Conditions
2.4. Experiments
3. Results and Discussion
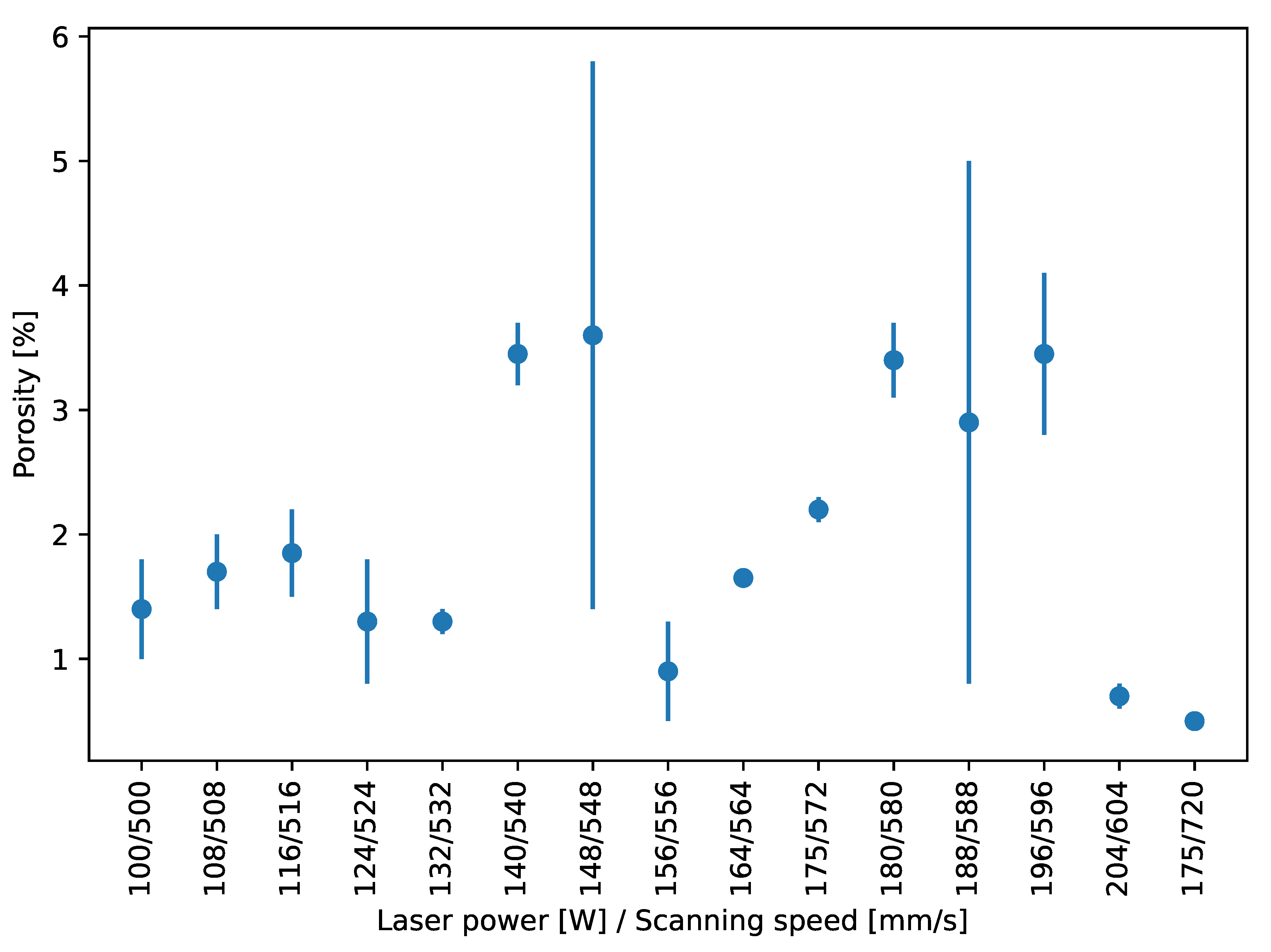
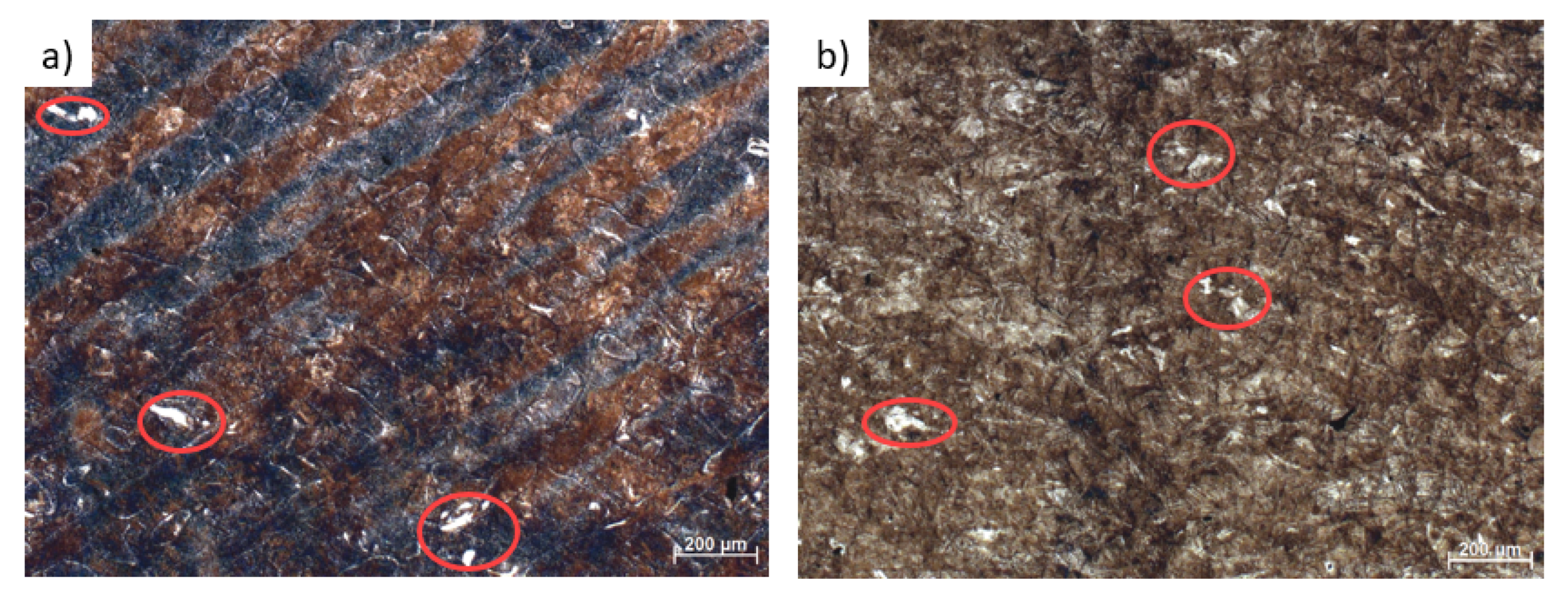
3.1. Preliminary Research
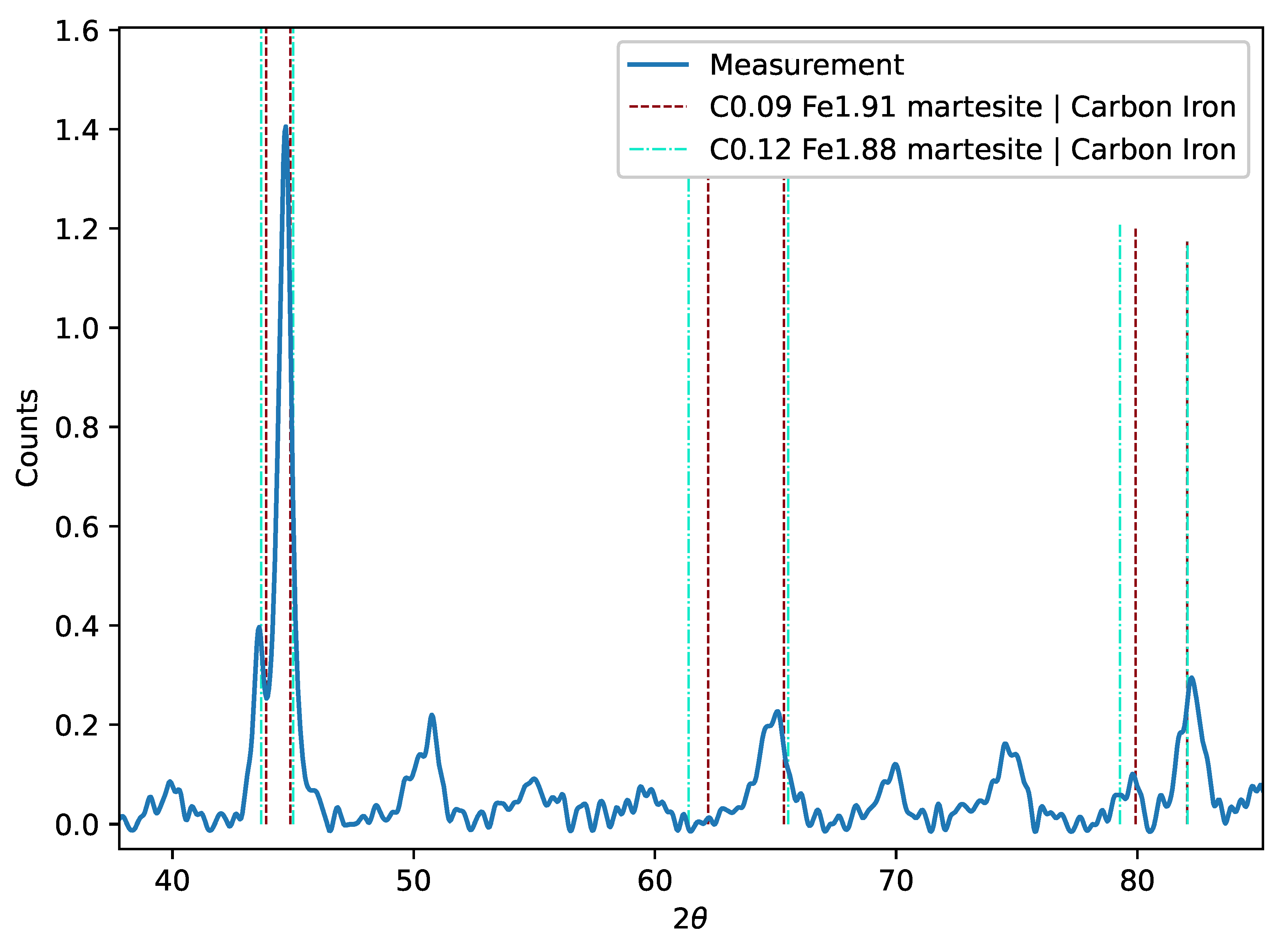
3.2. Residual Stresses
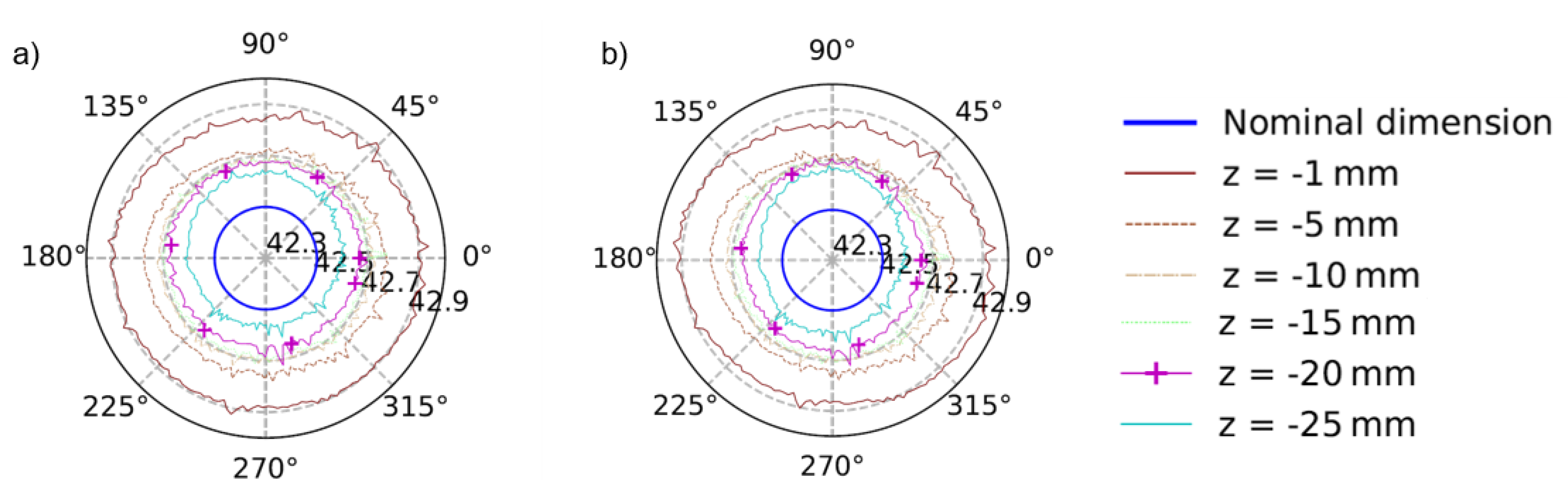
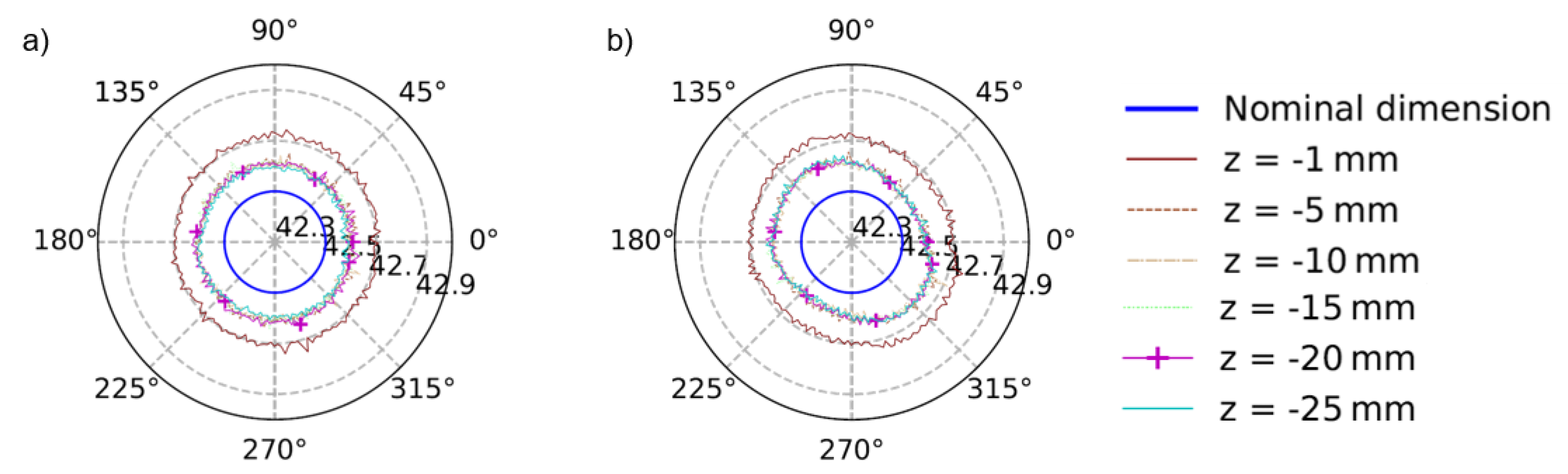
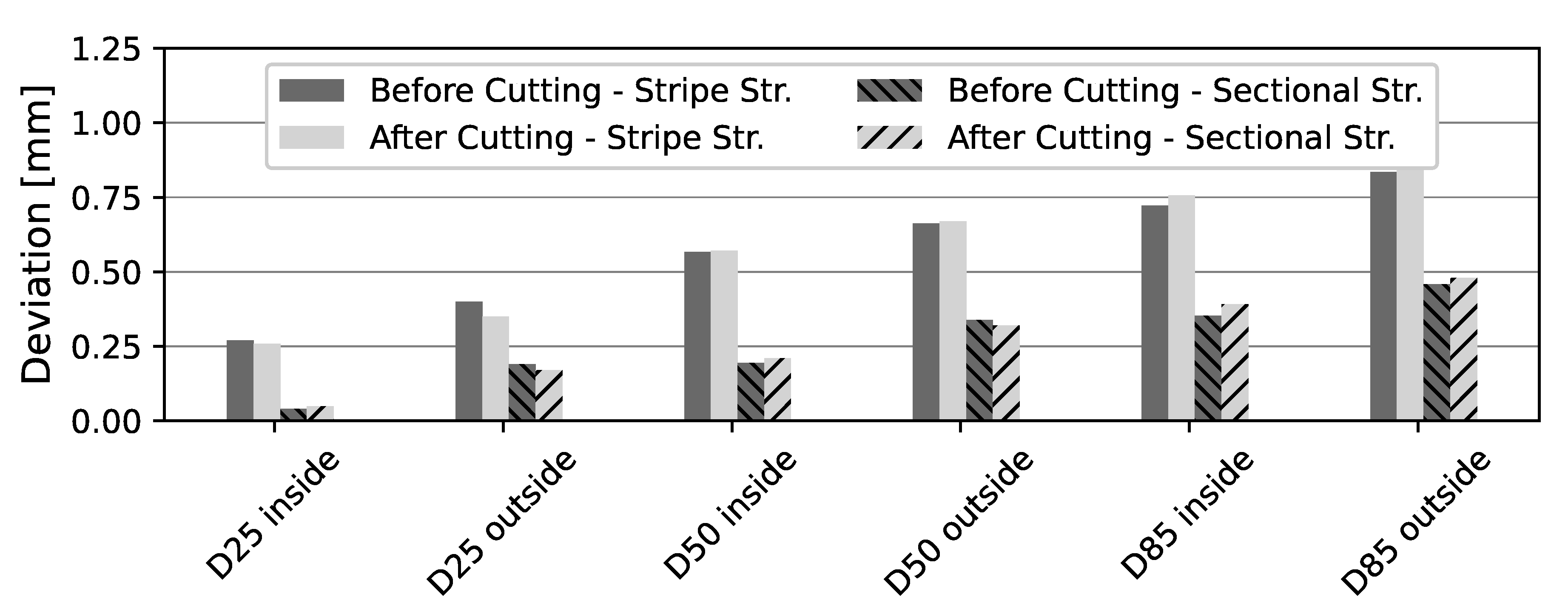
3.3. Tactile Measurements
3.4. Causes of Errors in 3D Printing
3.4.1. Oversize
3.4.2. Concave Outer Surface
3.4.3. Elliptical Shape
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| LPBF | Laser-Powder Bed Fusion |
| TGM | Temperature Gradient Mechanism |
| XRD | X-Ray Diffraction |
| ICDD | International Centre for Diffraction Data |
| DFG | Deutsche Forschungsgemeinschaft (German Research Foundation) |
References
- Wohlers, T.; Campbell, R.I.; Diegel, O.; Huff, R.; Kowen, J. Wohlers Report 2020: 3D Printing and Additive Manufacturing State of the Industry; Wohlers Associates: Fort Collins, CO, USA, 2020. [Google Scholar]
- Hitzler, L.; Merkel, M.; Hall, W.; Öchsner, A. A Review of Metal Fabricated with Laser- and Powder-Bed Based Additive Manufacturing Techniques: Process, Nomenclature, Materials, Achievable Properties, and its Utilization in the Medical Sector. Adv. Eng. Mater. 2018, 20, 1700658. [Google Scholar] [CrossRef]
- Gan, M.X.; Wong, C.H. Practical support structures for selective laser melting. J. Mater. Process. Technol. 2016, 238, 474–484. [Google Scholar] [CrossRef]
- Mazur, M.; Leary, M.; McMillan, M.; Elambasseril, J.; Brandt, M. SLM additive manufacture of H13 tool steel with conformal cooling and structural lattices. Rapid Prototyp. J. 2016, 504–518. [Google Scholar] [CrossRef]
- Yan, C.; Hao, L.; Hussein, A.; Young, P.; Raymont, D. Advanced lightweight 316L stainless steel cellular lattice structures fabricated via selective laser melting. Mater. Des. 2014, 55, 533–541. [Google Scholar] [CrossRef]
- Tomas, J.; Schubert, T.; Bernthaler, T.; Merkel, M.; Schneider, G.; Sellmer, D. Laser sintering of tungsten carbide cutter shafts with intergrated cooling channels. In Pro-AM 2018; Nanyang Technological University: Singapore, 2018; pp. 297–302. [Google Scholar] [CrossRef]
- Yadroitsev, I.; Bertrand, P.; Smurov, I. Parametric analysis of the selective laser melting process. Appl. Surf. Sci. 2007, 8064–8069. [Google Scholar] [CrossRef]
- Lee, P.H.; Chung, H.; Lee, S.W.; Yoo, J.; Ko, J. Review: Dimensional Accuracy in Additive Manufacturing Processes. In Proceedings of the ASME 9th International Manufacturing Science and Engineering Conference—2014, Detroit, MI, USA, 9–13 June 2014; Ragai, I., Ed.; ASME: New York, NY, USA, 2014. [Google Scholar] [CrossRef]
- Kruth, J.P.; Deckers, J.; Yasa, E.; Wauthlé, R. Assessing and comparing influencing factors of residual stresses in selective laser melting using a novel analysis method. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2012, 226, 980–991. [Google Scholar] [CrossRef]
- Mercelis, P.; Kruth, J.P. Residual stresses in selective laser sintering and selective laser melting. Rapid Prototyp. J. 2006, 254–265. [Google Scholar] [CrossRef]
- Alkahari, M.R.; Furumoto, T.; Ueda, T.; Hosokawa, A.; Tanaka, R.; Abdul Aziz, M.S. Thermal Conductivity of Metal Powder and Consolidated Material Fabricated via Selective Laser Melting. Key Eng. Mater. 2012, 523–524, 244–249. [Google Scholar] [CrossRef]
- Eigenmann, B.; Macherauch, E. Röntgenographische Untersuchung von Spannungszuständen in Werkstoffen. Mater. Und Werkst. 1995, 148–160. [Google Scholar] [CrossRef]
- Ali, H.; Ghadbeigi, H.; Mumtaz, K. Effect of scanning strategies on residual stress and mechanical properties of Selective Laser Melted Ti6Al4V. Mater. Sci. Eng. A 2018, 175–187. [Google Scholar] [CrossRef]
- Kruth, J.P.; Froyen, L.; van Vaerenbergh, J.; Mercelis, P.; Rombouts, M.; Lauwers, B. Selective laser melting of iron-based powder. J. Mater. Process. Technol. 2004, 616–622. [Google Scholar] [CrossRef]
- Berger, U.; Merkel, M.; Liebisch, A. The Influence of Preheating on Laser Beam Melting. In Pro-AM 2016; Research Publishing: Singapore, 2016; pp. 306–311. [Google Scholar]
- Mertens, R.; Vrancken, B.; Holmstock, N.; Kinds, Y.; Kruth, J.P.; van Humbeeck, J. Influence of Powder Bed Preheating on Microstructure and Mechanical Properties of H13 Tool Steel SLM Parts. Phys. Procedia 2016, 882–890. [Google Scholar] [CrossRef]
- Zhang, K.; Wang, S.; Liu, W.; Long, R. Effects of substrate preheating on the thin-wall part built by laser metal deposition shaping. Appl. Surf. Sci. 2014, 839–855. [Google Scholar] [CrossRef]
- VDI-2221. Blatt 2. VDI-Gesellschaft Entwicklung Konstruktion Vertrieb. Methodik zum Entwickeln und Konstruieren technischer Systeme und Produkte Qualifizierung Qualitätssicherung und Nachbearbeitung, Mai; VDI-Verlag: Berlin/Heidelberg, Germany, 1993. [Google Scholar]
- Cheng, B.; Shrestha, S.; Chou, K. Stress and deformation evaluations of scanning strategy effect in selective laser melting. Addit. Manuf. 2016, 12, 240–251. [Google Scholar] [CrossRef]
- Dunbar, A.; Denlinger, E.; Heigel, J.; Michaleris, P.; Guerrier, P.; Martukanitz, R.; Simpson, T. Development of experimental method for in situ distortion and temperature measurements during the laser powder bed fusion additive manufacturing process. Addit. Manuf. 2016, 12, 25–30. [Google Scholar] [CrossRef]
- Yu, W.; Sing, S.L.; Chua, C.K.; Tian, X. Influence of re-melting on surface roughness and porosity of AlSi10Mg parts fabricated by selective laser melting. J. Alloys Compd. 2019, 792, 574–581. [Google Scholar] [CrossRef]
- Yadroitsev, I.; Thivillon, L.; Bertrand, P.; Smurov, I. Strategy of manufacturing components with designed internal structure by selective laser melting of metallic powder. Appl. Surf. Sci. 2007, 254, 980–983. [Google Scholar] [CrossRef]
- VDI-3405. Blatt 2 VDI-Gesellschaft Produktion und Logistik (GPL)—Additive Fertigungsverfahren Strahlschmelzen Metallischer Bauteile; Verlag des Vereins Deutscher Ingenieure: Düsseldorf, Germany, August 2013. [Google Scholar]
- Steinhauser, M.; Sert, E.; Hitzler, L.; Öchsner, A.; Merkel, M. Fatigue Behavior of the Additively Manufactured Tool Steel H13 after Surface Treatment using Different Post-Processing Methods. Pract. Metallogr. 2020, 57, 140–167. [Google Scholar] [CrossRef]
- Meiners, W. Direktes Selektives Laser Sintern Einkomponentiger Metallischer Werkstoffe. Ph.D. Thesis, RWTH Aachen University, Aachen, Germany, 15 April 1999. [Google Scholar]
- Gu, D.D.; Meiners, W.; Wissenbach, K.; Poprawe, R. Laser additive manufacturing of metallic components: Materials, processes and mechanisms. Int. Mater. Rev. 2012, 133–164. [Google Scholar] [CrossRef]
- Hitzler, L.; Hirsch, J.; Heine, B.; Merkel, M.; Hall, W.; Öchsner, A. On the Anisotropic Mechanical Properties of Selective Laser-Melted Stainless Steel. Materials 2017, 10, 1136. [Google Scholar] [CrossRef] [PubMed]
| Fe | C | Cr | Mn | Mo | Ni + Cu | P | S | Si | V |
|---|---|---|---|---|---|---|---|---|---|
| Bal. | 0.32–0.45 | 4.75–5.50 | 0.20–0.60 | 1.10–1.75 | 0.75 | 0.03 | 0.03 | 0.80–1.25 | 0.80–1.20 |
| Laser Power [W] | Scan Speed [mm/s] | Hatch Distance [mm] | Energy Density [J/mm3] | |
|---|---|---|---|---|
| Contour | 100 | 400 | ||
| Fill | 150 | 450 | ||
| Core | 175 | 720 | ||
| Layer thickness of 30 μm | ||||
| Mounting plate temperature 200 °C | ||||
| Yield Strength [MPa] | Tensile Strength [MPa] | Elongation at Fracture [%] | |
|---|---|---|---|
| As built | |||
| As built | |||
| As built soft annealed | |||
| As built soft annealed |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tomas, J.; Hitzler, L.; Köller, M.; von Kobylinski, J.; Sedlmajer, M.; Werner, E.; Merkel, M. The Dimensional Accuracy of Thin-Walled Parts Manufactured by Laser-Powder Bed Fusion Process. J. Manuf. Mater. Process. 2020, 4, 91. https://doi.org/10.3390/jmmp4030091
Tomas J, Hitzler L, Köller M, von Kobylinski J, Sedlmajer M, Werner E, Merkel M. The Dimensional Accuracy of Thin-Walled Parts Manufactured by Laser-Powder Bed Fusion Process. Journal of Manufacturing and Materials Processing. 2020; 4(3):91. https://doi.org/10.3390/jmmp4030091
Chicago/Turabian StyleTomas, Josef, Leonhard Hitzler, Marco Köller, Jonas von Kobylinski, Michael Sedlmajer, Ewald Werner, and Markus Merkel. 2020. "The Dimensional Accuracy of Thin-Walled Parts Manufactured by Laser-Powder Bed Fusion Process" Journal of Manufacturing and Materials Processing 4, no. 3: 91. https://doi.org/10.3390/jmmp4030091
APA StyleTomas, J., Hitzler, L., Köller, M., von Kobylinski, J., Sedlmajer, M., Werner, E., & Merkel, M. (2020). The Dimensional Accuracy of Thin-Walled Parts Manufactured by Laser-Powder Bed Fusion Process. Journal of Manufacturing and Materials Processing, 4(3), 91. https://doi.org/10.3390/jmmp4030091






