Aerodynamic Hinge Moment Characteristics of Pitch-Regulated Mechanism for Mars Rotorcraft: Investigation and Experiments
Abstract
1. Introduction
2. Materials and Methods
2.1. Numerical Method and Governing Equations
2.2. Grid Partitioning and Sensitivity Analysis
3. Results
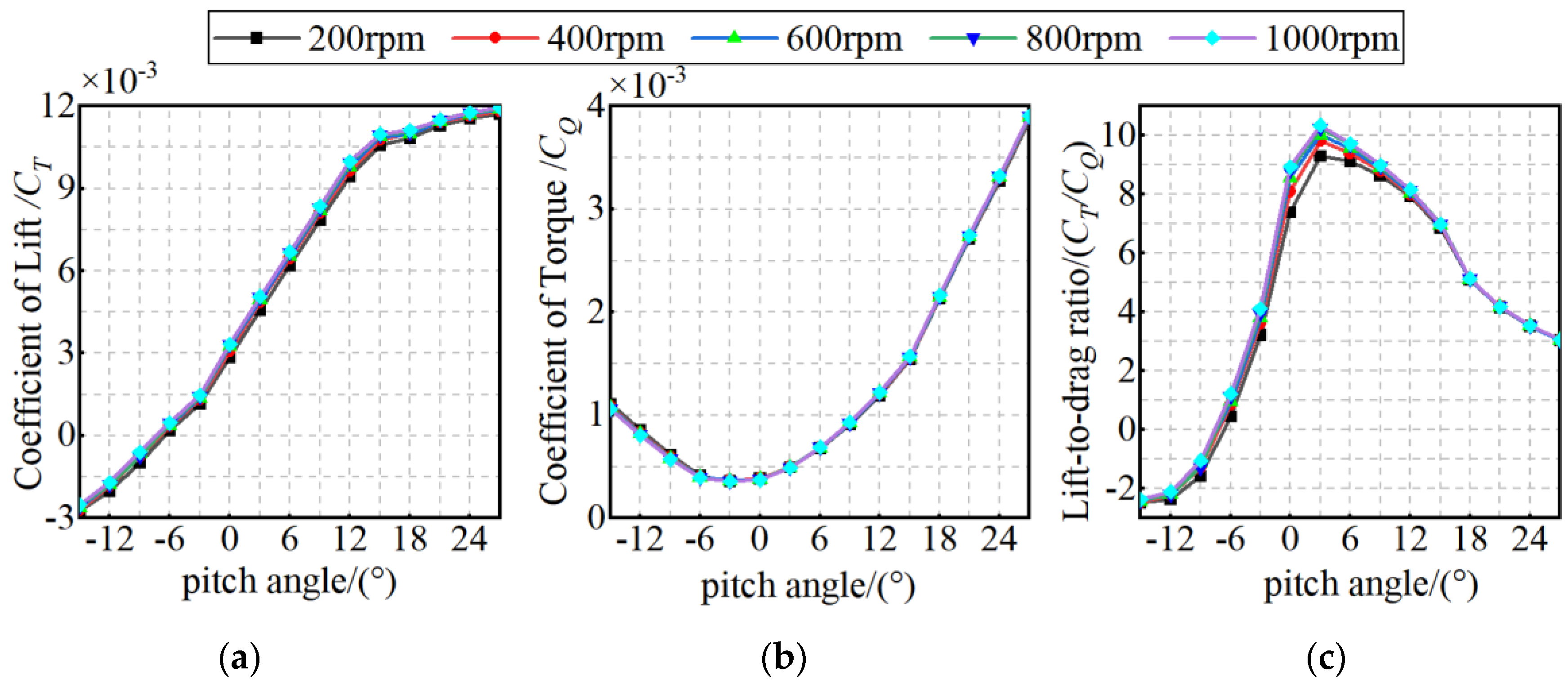
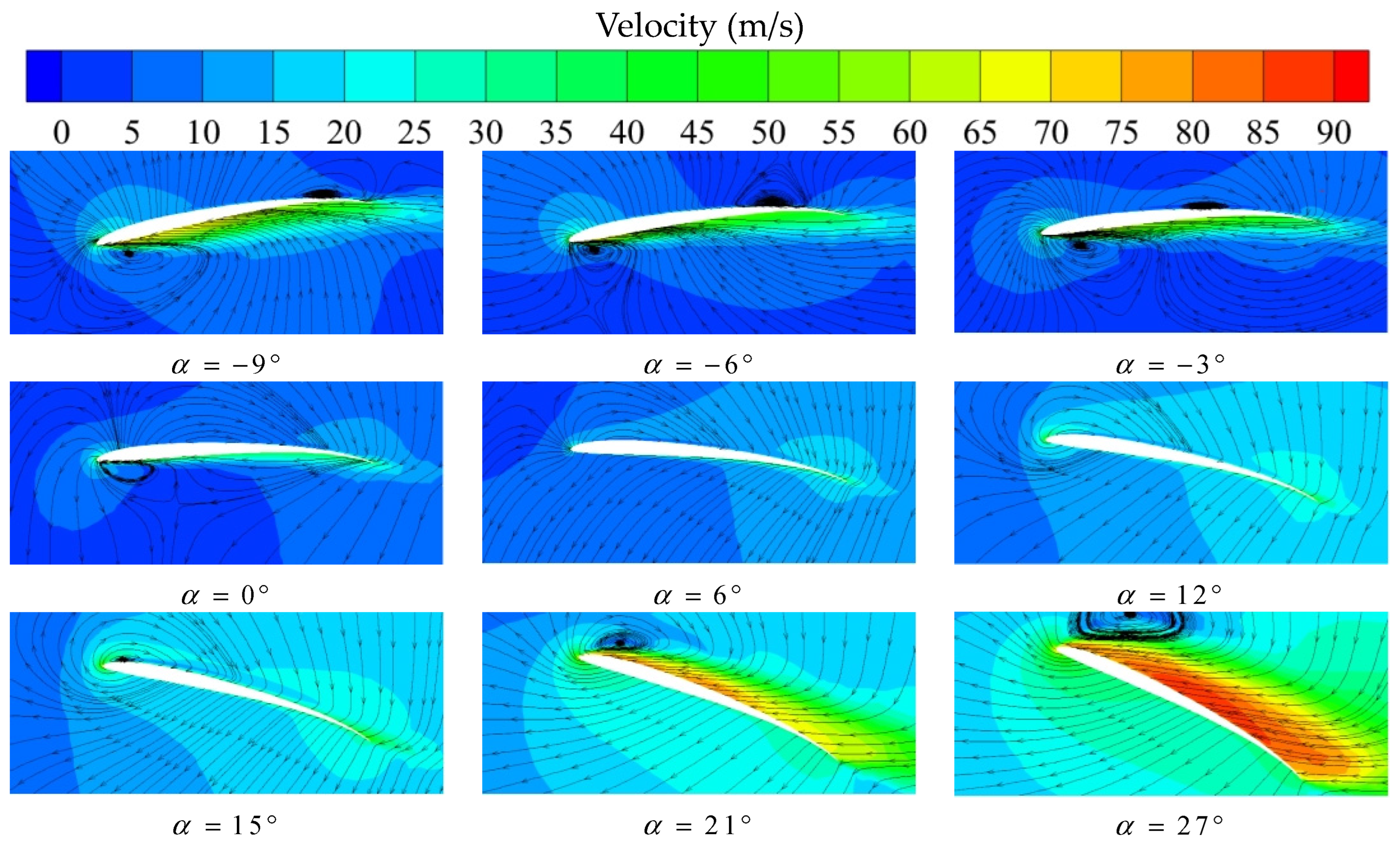
3.1. Aerodynamic Characteristics Analysis
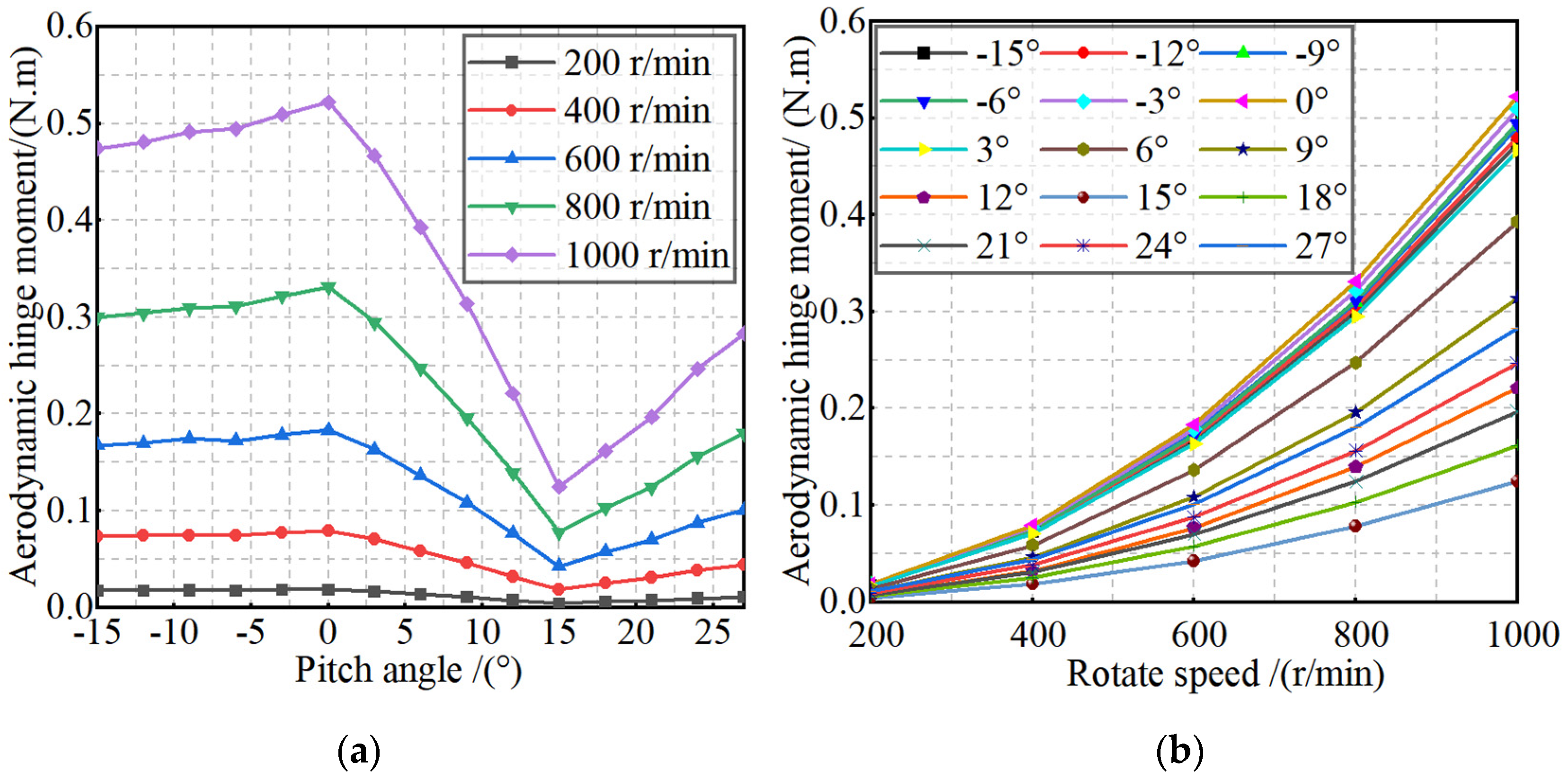
3.2. Analysis of Aerodynamic Hinge Moment Characteristics
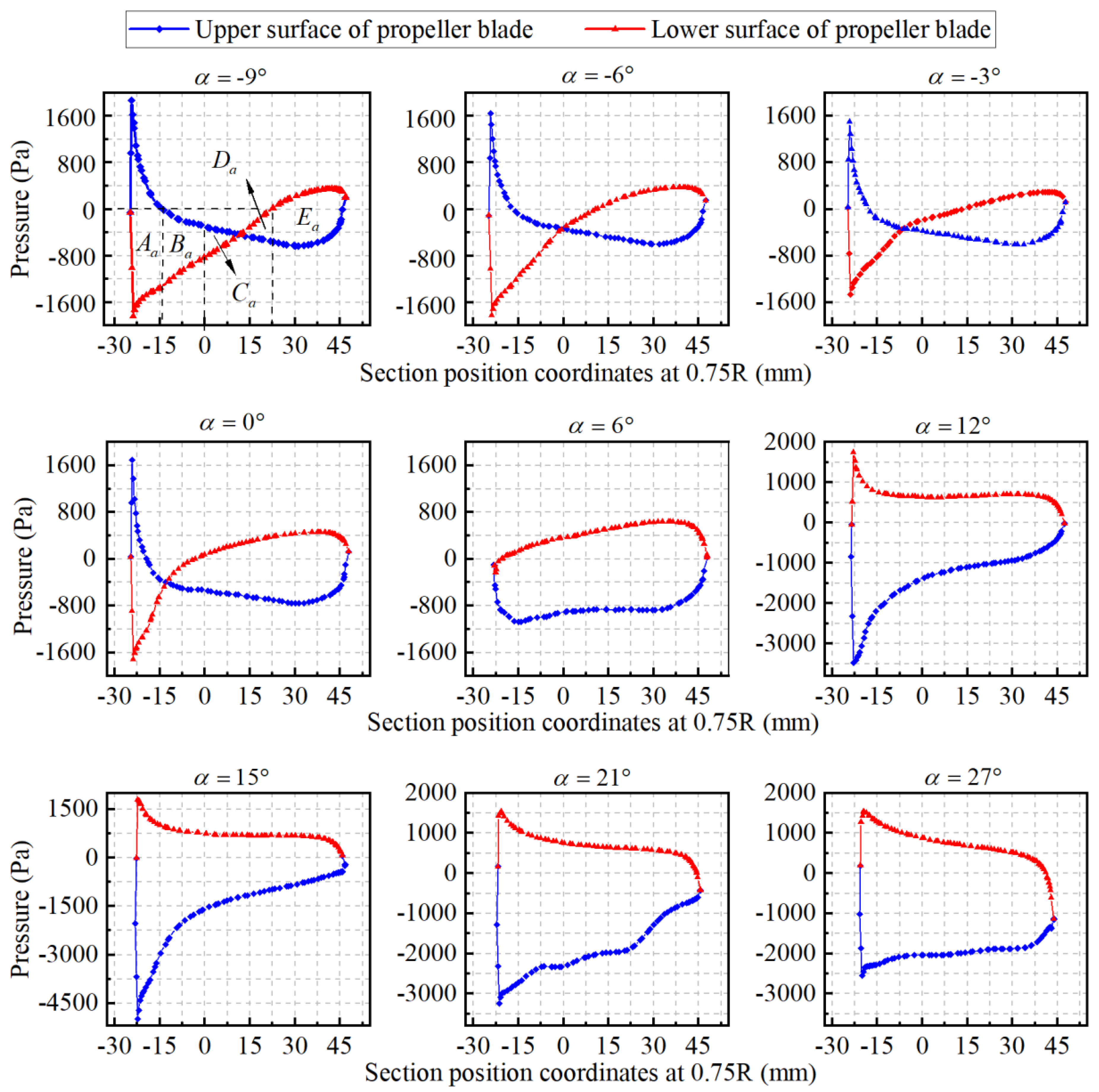
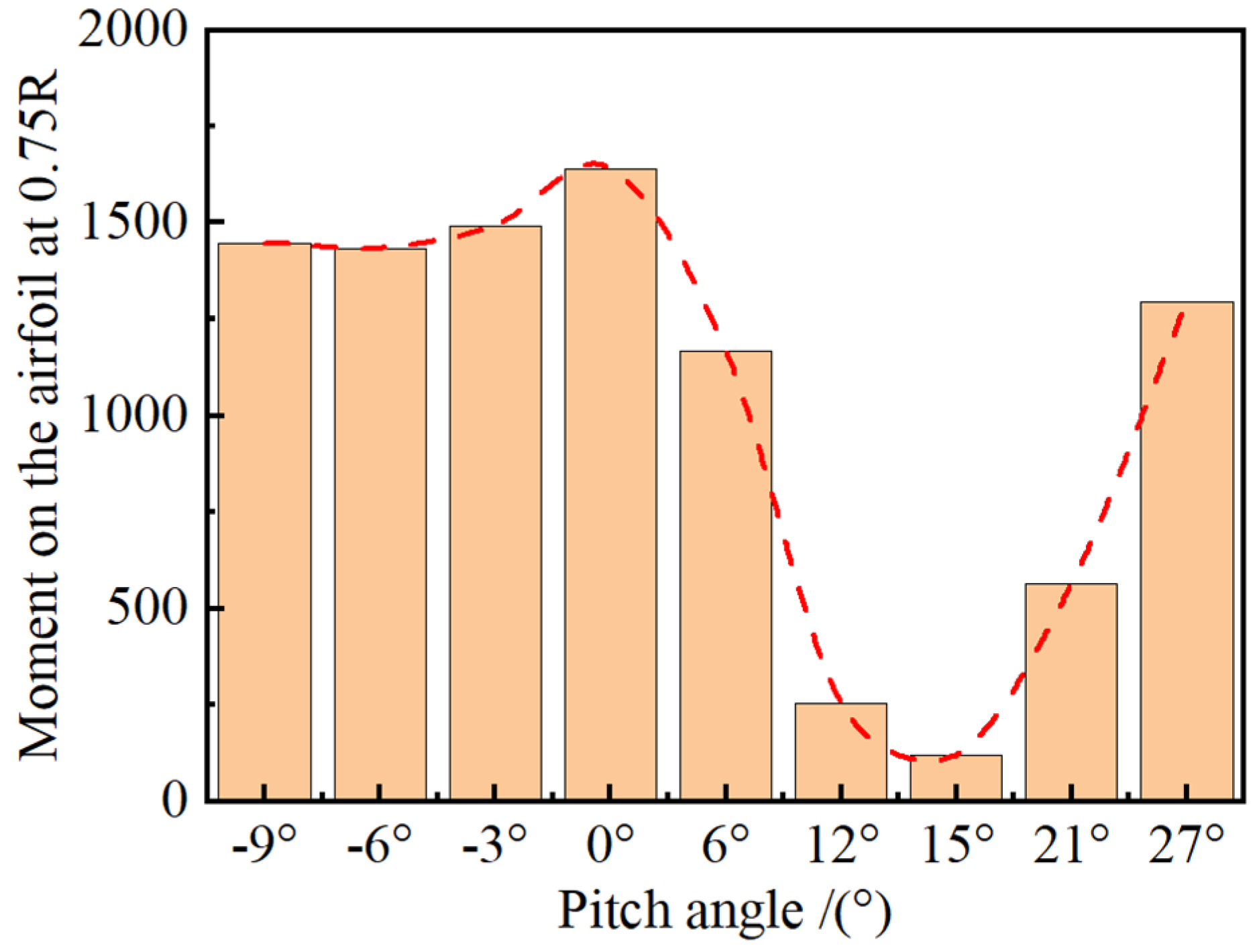
3.3. Quantitative Representation of Wing Profile Pressure
4. Experiment and Discussion
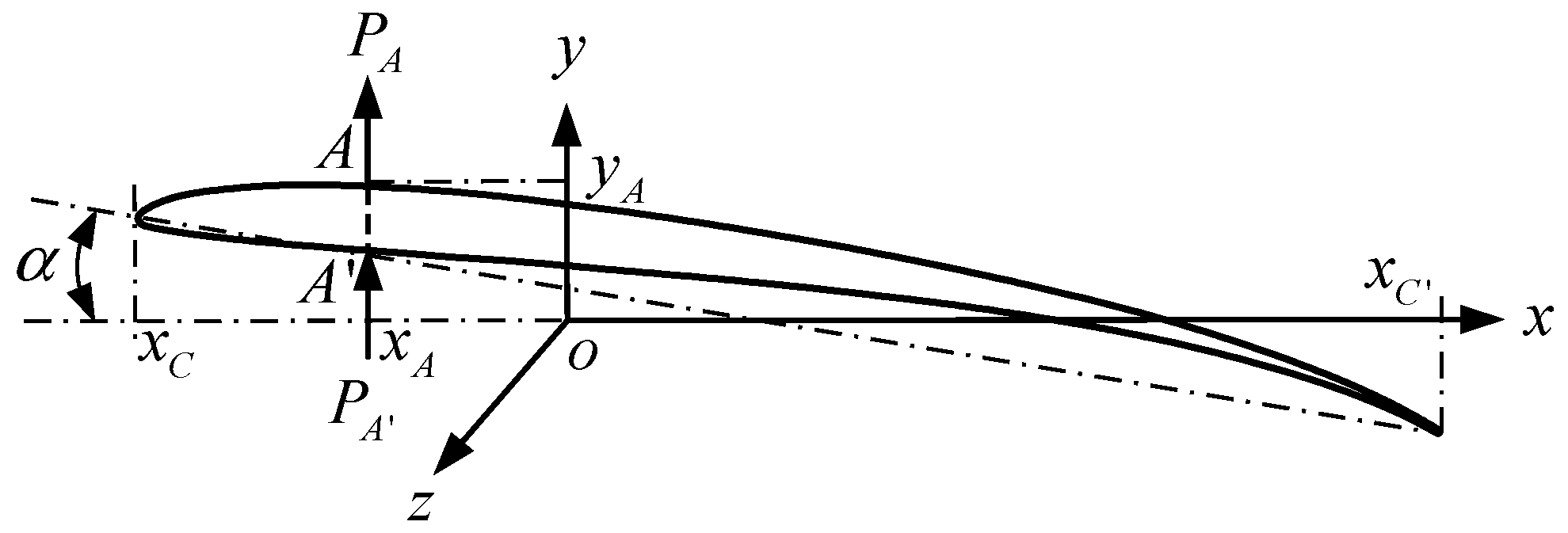
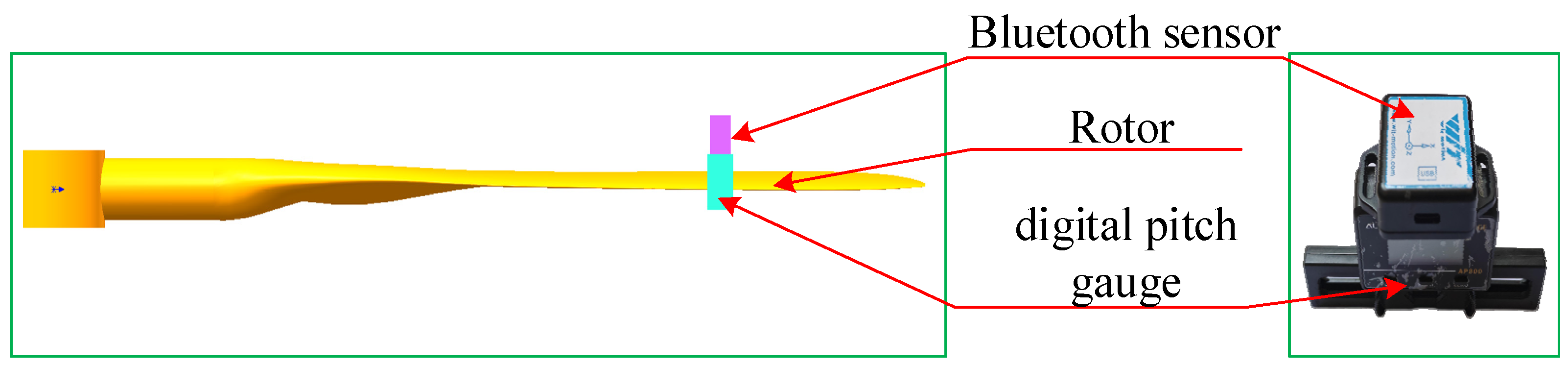
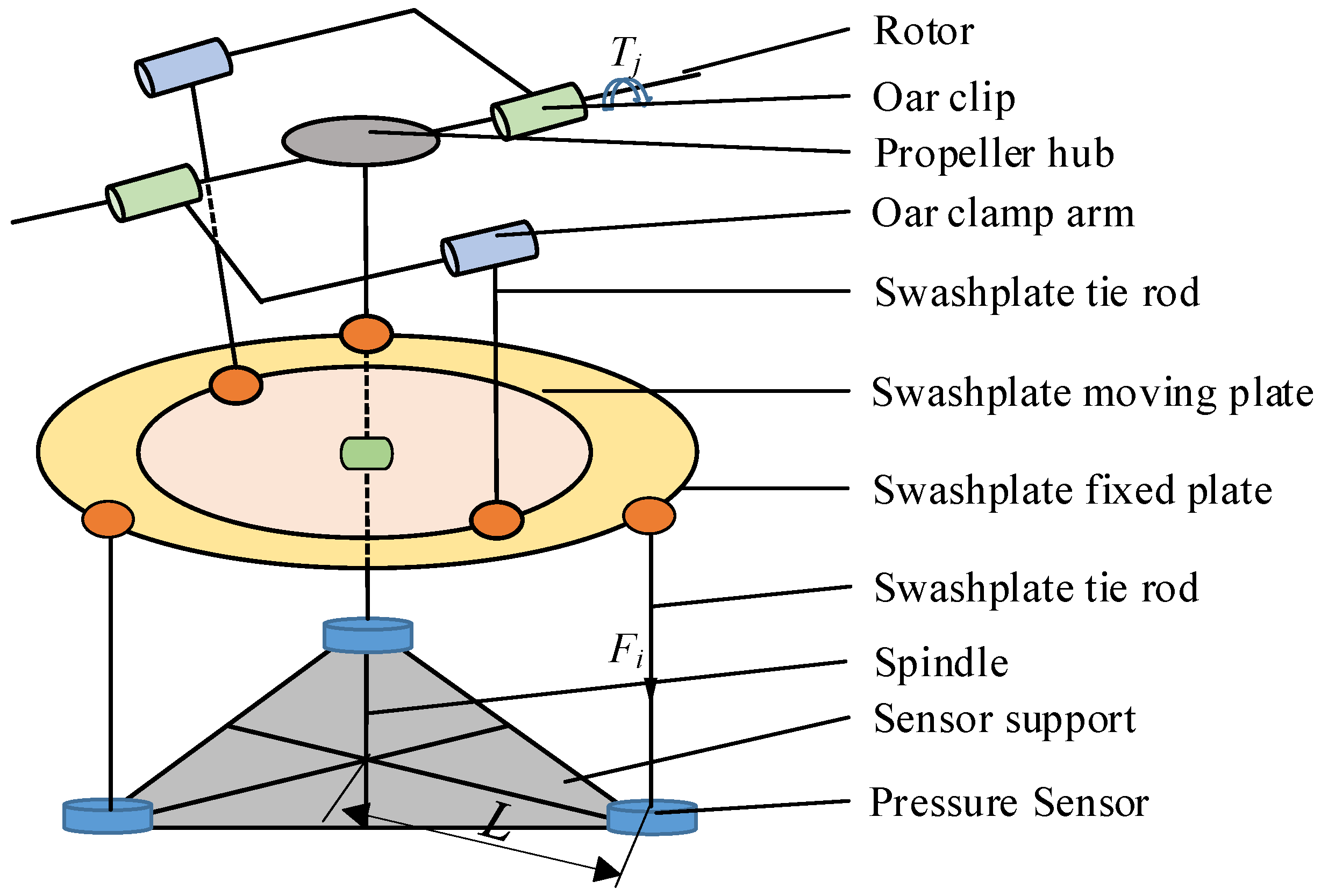
4.1. Aerodynamic Hinge Moment Test Principle
4.2. Earth/Mars Environment Experimental Comparison
4.2.1. Experiment in Earth Environment
4.2.2. Experiment in Simulated Martian Environment
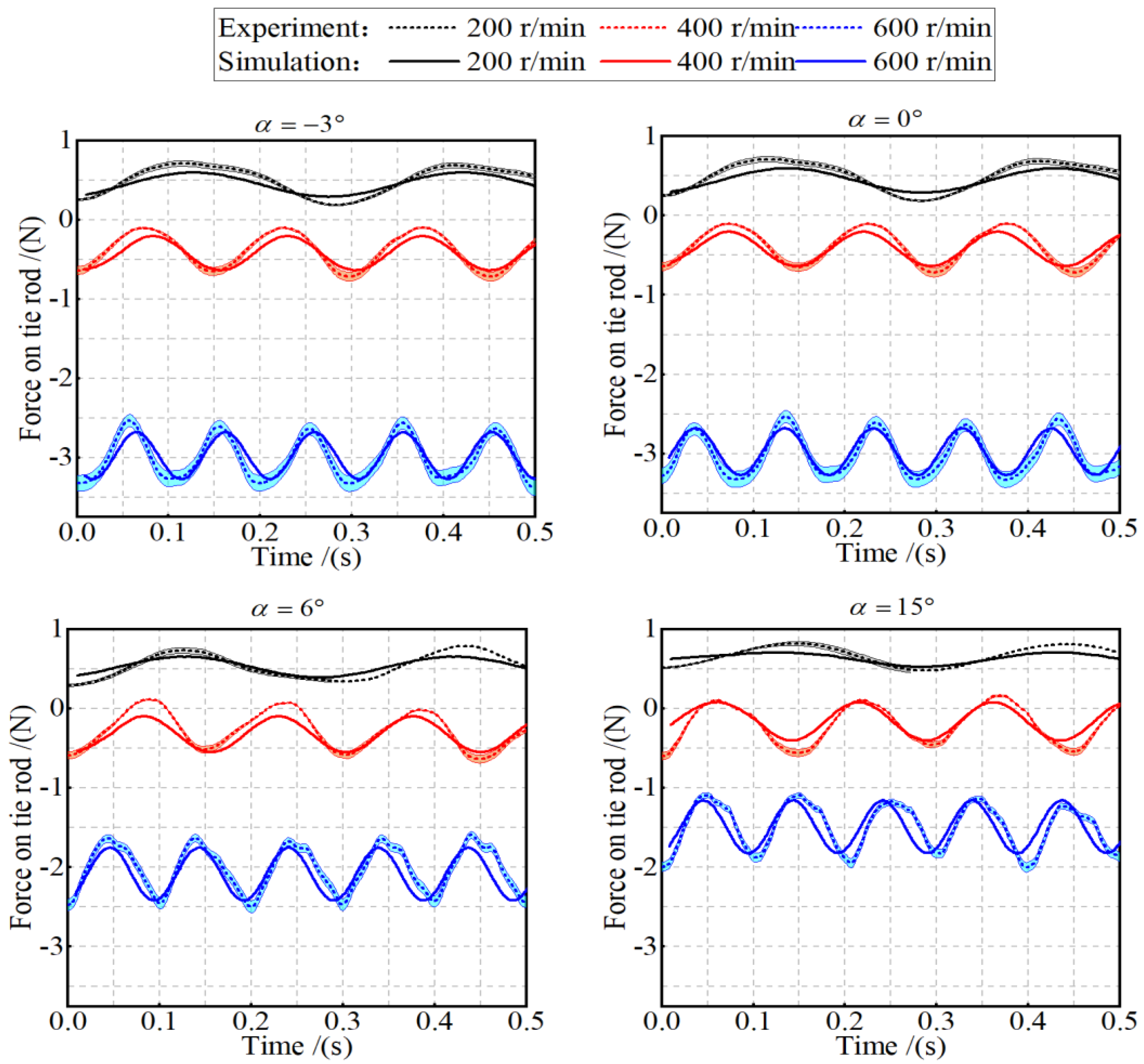
4.3. Aerodynamic Hinge Moment Testing Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, M.; Zhang, Y.; Ni, Y.; Huang, X.; Zhang, Z.; Xing, Z. Progress and Prospect in Planetary Exploration of Solar System. Chin. Space Sci. Technol. 2023, 43, 1–12. [Google Scholar] [CrossRef]
- Yu, D.; Sun, Z.; Meng, L.; Shi, D. The Development Process and Prospects for Mars Exploration. J. Deep. Space Explor. 2016, 3, 108–113. [Google Scholar] [CrossRef]
- Hall, J.; Pauken, M.; Kerzhanovich, V.; Walsh, G.; Fairbrother, D.; Shreves, C.; Lachenmeier, T. Flight Test Results for Aerially Deployed Mars Balloons. In Proceedings of the AIAA Balloon Systems Conference, Williamsburg, VA, USA, 21–24 May 2007. [Google Scholar]
- Braun, R.D.; Wright, H.S.; Croom, M.A.; Levine, J.S.; Spencer, D.A. Design of the ARES Mars Airplane and Mission Architecture. J. Spacecr. Rocket. 2006, 43, 1026–1034. [Google Scholar] [CrossRef]
- Verstraete, D.; Palmer, J.L.; Hornung, M. Preliminary Sizing Correlations for Fixed-Wing Unmanned Aerial Vehicle Characteristics. J. Aircr. 2018, 55, 715–726. [Google Scholar] [CrossRef]
- Fujita, K.; Oyama, A.; Kubo, D.; Kanazaki, M.; Nagai, H. Wind Tunnel Test for Videogrammetric Deformation Measurement of UAV for Mars Airplane Balloon Experiment-1 (MABE-1). JFCMV 2019, 07, 87–100. [Google Scholar] [CrossRef]
- Withrow, S.; Johnson, W.; Young, L.A.; Cummings, H.; Balaram, J.; Tzanetos, T. An Advanced Mars Helicopter Design. In Proceedings of the ASCEND 2020, American Virtual Event, 16–18 November 2020. [Google Scholar]
- Zhu, K.; Quan, Q.; Wang, K.; Tang, D.; Tang, B.; Dong, Y.; Wu, Q.; Deng, Z. Conceptual Design and Aerodynamic Analysis of a Mars Octocopter for Sample Collection. Acta Astronaut. 2023, 207, 10–23. [Google Scholar] [CrossRef]
- Shrestha, R.; Benedict, M.; Hrishikeshavan, V.; Chopra, I. Hover Performance of a Small-Scale Helicopter Rotor for Flying on Mars. J. Aircr. 2016, 53, 1160–1167. [Google Scholar] [CrossRef]
- Bar-Cohen, Y.; Colozza, A.; Badescu, M.; Sherrit, S.; Bao, X. Biomimetic Flying Swarm of Entomopters for Mars Extreme Terrain Science Investigations. Concepts Approaches Mars Explor. 2012, 1679, 4075. [Google Scholar]
- Patel, A.; Karlsson, S.; Lindqvist, B.; Kanellakis, C.; Agha-Mohammadi, A.-A.; Nikolakopoulos, G. Towards Energy Efficient Autonomous Exploration of Mars Lava Tube with a Martian Coaxial Quadrotor. Adv. Space Res. 2023, 71, 3837–3854. [Google Scholar] [CrossRef]
- Folsom, L.; Ono, M.; Otsu, K.; Park, H. Scalable Information-Theoretic Path Planning for a Rover-Helicopter Team in Uncertain Environments. Int. J. Adv. Robot. Syst. 2021, 18, 1729881421999587. [Google Scholar] [CrossRef]
- Grip, H.F.; Johnson, W.; Malpica, C.; Scharf, D.P.; Mandić, M.; Young, L.; Allan, B.; Mettler, B.; Martin, M.S.; Lam, J. Modeling and Identification of Hover Flight Dynamics for NASA’s Mars Helicopter. J. Guid. Control Dyn. 2020, 43, 179–194. [Google Scholar] [CrossRef]
- Zhao, P.; Gao, X.; Yan, Z.; Li, Y.; Wu, J.; Deng, Z. Investigation of Aerodynamic Performance of Coaxial Rotors for Mars Rotorcraft. In Proceedings of the 2023 IEEE International Conference on Mechatronics and Automation (ICMA), Harbin, China, 6–9 August 2023; pp. 1275–1280. [Google Scholar]
- Zhao, H.; Ding, Z.; Leng, G.; Li, J. Flight Dynamics Modeling and Analysis for a Mars Helicopter. Chin. J. Aeronaut. 2023, 36, 221–230. [Google Scholar] [CrossRef]
- Grip, H.F.; Lam, J.; Bayard, D.S.; Conway, D.T.; Singh, G.; Brockers, R.; Delaune, J.H.; Matthies, L.H.; Malpica, C.; Brown, T.L.; et al. Flight Control System for NASA’s Mars Helicopter. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019; American Institute of Aeronautics and Astronautics: San Diego, CA, USA. [Google Scholar]
- Qu, S. Design and Analysis of the Measuring Structure of Hinge Moment in the Aeroelastic Model. Master’s Thesis, Dalian University of Technology, Dalian, China, 2014. [Google Scholar]
- Xia, F. Hinge Moment and Flutter of Rotor Blade for Heavy-Lift Helicopter. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2009. [Google Scholar]
- Mohamed, A.; Abdelhady, M.; Wood, D.H. Using the Hinge Moment of a Trailing Edge Flap for Controlling Dynamic Stall Loads. Exp Fluids 2021, 62, 184. [Google Scholar] [CrossRef]
- Cabaleiro de la Hoz, C.; Fioriti, M. New Methodology for Flight Control System Sizing and Hinge Moment Estimation. Proc. Inst. Mech. Eng. Part G: J. Aerosp. Eng. 2022, 236, 2375–2390. [Google Scholar] [CrossRef]
- Sebastia, C.; Hornung, M. Numerical Analysis of Aerodynamic Flap Hinge Moment under Unsteady Flow Conditions Considering Laminar-Turbulent Transition. In Proceedings of the AIAA AVIATION 2023 Forum, San Diego, CA, USA, 12–16 June 2023. [Google Scholar]
- Xiang, L.; Shu, H.; Xu, X. Flow-Through Model Hinge Moment Test Techniques Investigation in Hypersonic Wind Tunnel. Flight Dyn. 2022, 40, 21–27. [Google Scholar] [CrossRef]
- Rose, R.; Nicholas, O.P.; Vorley, G. Flight Measurements of the Elevator and Aileron Hinge-Moment Derivatives of the Fairey Delta 2 Aircraft up to a Mach Number of 1.6 and Comparisons with Wind-Tunnel Results. 1965. Available online: https://reports.aerade.cranfield.ac.uk/bitstream/handle/1826.2/4061/arc-rm-3485.pdf?sequence=1&isAllowed=y (accessed on 20 May 2024).
- Grismer, M.; Kinsey, D.; Grismer, D. Hinge Moment Predictions Using CFD. In Proceedings of the 18th Applied Aerodynamics Conference; American Institute of Aeronautics and Astronautics, Denver, CO, USA, 14 August 2000. [Google Scholar]
- Wu, Z.; Zhu, Z.; Ding, N. Calculation of Hinge Moment of 3-D Aileron. Acta Aeronaut. Et Astronaut. Sin. 2007, 28, 519–526. [Google Scholar]
- Huang, Z.; Wang, X.; Zhang, R. Vestigation of Gap Effect on the Rudder Hinge Moment Characteristics. J. Exp. Fluid Mech. 2007, 21, 1–6. [Google Scholar]
- Hambrick, E.; Thomason, N. Conceptual Aircraft Hinge Moment Measurement System. Aerosp. Eng. 2010. [Google Scholar]
- Zhang, G.Q.; Yu, S.C.M.; Chien, A. Investigation of the Three-Dimensional Hinge Moment Characteristics Generated by the ONERA-M6 Wing with an Aileron. Adv. Mech. Eng. 2013, 5, 714168. [Google Scholar] [CrossRef]
- Xu, G.; Cheng, M. Research on Rotor Blade Hinge Moment of a Small-scale Coaxial Helicopter. J. Aerosp. Power 2010, 25, 1805–1810. [Google Scholar] [CrossRef]
- Yuan, M.; Sun, P.; Fan, F.; Liu, P.; Lin, Y. Investigation on Blade Aerodynamic Hinge Moment of Coaxial Rigid Rotor with Different Pith Axes. Aeronaut. Sci. Technol. 2018, 29, 61–66. [Google Scholar] [CrossRef]
- Meng, Q.; Ke, Z.; Wei, W.; Zhao, M.; Tu, J.; Yan, Q. Study on the Transient Dynamic Characteristics of the Pitch-Regulated Device for Coaxilcopter Under Aerodynamic Loads. In Proceedings of the ASME Turbo Expo 2023: Turbomachinery Technical Conference and Exposition, Boston, MA, USA, 26–30 June 2023. [Google Scholar]
- Grismer, D.; Grismer, M.; Simon, J.; Tinapple, J. An Experimental Investigation of Factors Influencing Hinge Moments. In Proceedings of the 18th Applied Aerodynamics Conference; American Institute of Aeronautics and Astronautics, Denver, CO, USA, 14 August 2000. [Google Scholar]
- Liu, F. Moment Acquisition and Transmission Research in the Helicopters’Airscrew Dynamic-Balancing Test Platform. Master’s Thesis, Harbin Engineering University, Harbin, China, 2007. [Google Scholar]
- Xue, L.; Wang, S. Dynamics Analysis and Modeling of Helicopter Rotor Test-Bed. J. Beijing Univ. Aeronaut. Astronaut. 2009, 35, 296–299. [Google Scholar] [CrossRef]
- Lange, c.; Ranjbaran, F.; Angeles, J.; Goritschnig, G. The Kinematics of the Swashplate Mechanism of a VTOL Unmanned Aerial Vehicle. Multibody Syst. Dyn. 1999, 3, 333–365. [Google Scholar] [CrossRef]
- Zhu, K.; Tang, D.; Quan, Q.; Lv, Y.; Shen, W.; Deng, Z. Modeling and Experimental Study on Orientation Dynamics of a Mars Rotorcraft with Swashplate Mechanism. Aerosp. Sci. Technol. 2023, 138, 108311. [Google Scholar] [CrossRef]
- Hayami, K.; Sugawara, H.; Yumino, T.; Tanabe, Y.; Kameda, M. CFD Analysis on the Performance of a Coaxial Rotor with Lift Offset at High Advance Ratios. Aerosp. Sci. Technol. 2023, 135, 108194. [Google Scholar] [CrossRef]
- Singh, P.; Venkatesan, C. Analytical Formulation Of Heave-Yaw Modes For A Coaxial Rotor Micro Aerial Vehicle. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, National Harbor, MD, USA, 13–17 January 2014. [Google Scholar]
- Smith, M.J.; Lim, J.W. An Assessment of CFD/CSD Prediction State-of-the-Art Using the HART II International Workshop Data. In Proceedings of the Annual Forum Proceedings—AHS International, Ft. Worth, TX, USA, 1–3 May 2012; Volume 1, pp. 1–41. [Google Scholar]
- Banfield, D.; Spiga, A.; Newman, C.; Forget, F.; Lemmon, M.; Lorenz, R.; Murdoch, N.; Viudez-Moreiras, D.; Pla-Garcia, J.; Garcia, R.F.; et al. The Atmosphere of Mars as Observed by InSight. Nat. Geosci. 2020, 13, 190–198. [Google Scholar] [CrossRef]
- Zhang, W.; Xu, B.; Zhang, H.; Fan, W.; Zhao, Z. Aerodynamic Performance of Propeller under Ultra-Low Reynolds Number on Mars. Trans. Beijing Inst. Technol. 2024, 44, 172–181. [Google Scholar] [CrossRef]
- Koning, W.J.F.; Johnson, W.; Allan, B.G. Generation of Mars Helicopter Rotor Model for Comprehensive Analyses. In Proceedings of the AHS Specialists’ Conference on Aeromechanics Design for Transformative Vertical Flight, San Francisco, CA, USA, 16–18 January 2018. [Google Scholar]
- Zhang, W.; Xu, B.; Zhang, H.; Xiang, C.; Fan, W.; Zhao, Z. Analysis of Aerodynamic Characteristics of Propeller Systems Based on Martian Atmospheric Environment. Drones 2023, 7, 397. [Google Scholar] [CrossRef]
- Wei, W.; Wang, R.; Xu, B.; Fan, K.; Zhao, Z.; Zhou, B. Multi-Objective Optimization of Rotor Blade Aerodynamic Performance of Aerial-Ground Platform. Trans. Beijing Inst. Technol. 2022, 42, 842–849. [Google Scholar] [CrossRef]
- Wei, W.; Tu, J.; Ke, Z.; Wang, R.; Xu, B. Analysis and Optimization of the Coupling Effect for Duct-Rotor Based on Aerodynamic Performance. Aerosp. Sci. Technol. 2024, 150, 109200. [Google Scholar] [CrossRef]
- Li, S.; Xiong, J.; Wang, X. New Helicopter Rotor Aerodynamic Characteristics and Layout Analysis. J. Nanjing Univ. Aeronaut. Astronaut. 2020, 52, 318–333. [Google Scholar] [CrossRef]
- Li, Y.; Wu, T.; Yuan, Z.; Yu, W.; Wu, X. The Influence Law of Hinge Moment Balance Structural Parameters on Lift Measurement. J. Proj. Rocket. Missiles Guid. 2022, 42, 1–4. [Google Scholar] [CrossRef]
- Xu, Y.; Qiu, J.W. Research on Influence of Contact Stress on the Hinge Moment Balance Measures. Appl. Mech. Mater. 2012, 148–149, 833–836. [Google Scholar] [CrossRef]
- Zhang, W.; Xu, B.; Xiang, C.; Yao, Z.; Zhang, H.; Fan, W.; Zhao, Z.; Hu, Y. Aerodynamic Performance Study of Propellers for Mars Atmospheric Environment. Phys. Fluids 2023, 35, 127124. [Google Scholar] [CrossRef]
- Quan, Q.; Zhao, P.; Chen, S.; Wang, D.; Li, H.; Bai, D.; Deng, Z. Experimental Evaluating Approach to a Suitable Martian Coaxial Rotorcraft Blade. In Proceedings of the 2017 IEEE International Conference on Mechatronics and Automation (ICMA), Takamatsu, Japan, 6–9 August 2017; pp. 1958–1963. [Google Scholar]
- Dull, C.; Wagner, L.; Young, L.; Johnson, W. Hover and Forward Flight Performance Modeling of the Ingenuity Mars Helicopter. In Proceedings of the Aeromechanics for Advanced Vertical Flight Technical Meeting, Transformative Vertical Flight 2022, San Jose, CA, USA, 25–27 January 2022. [Google Scholar]
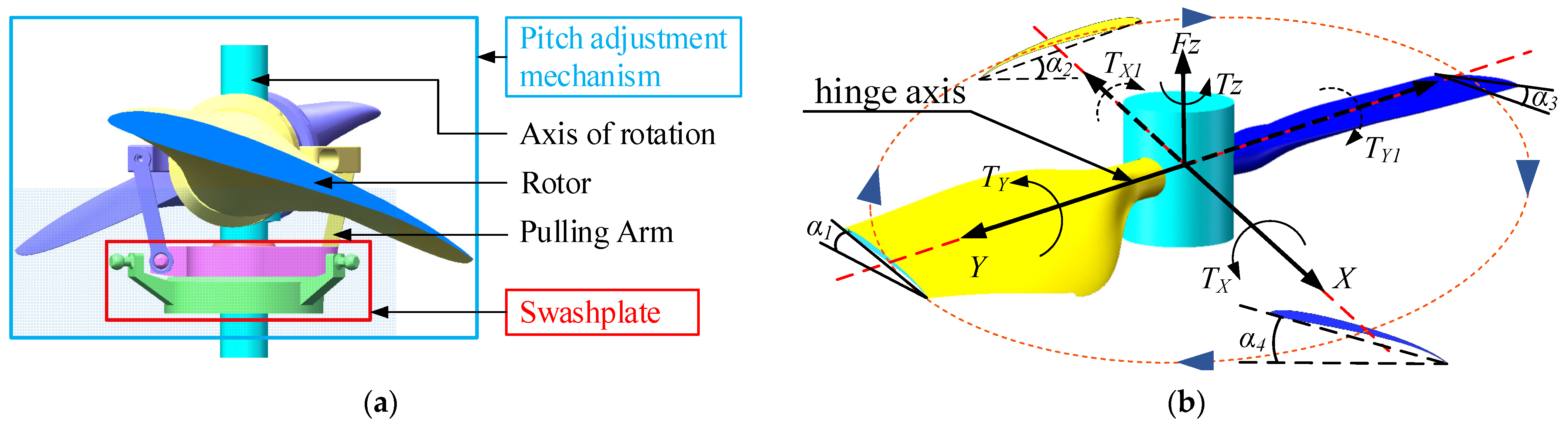

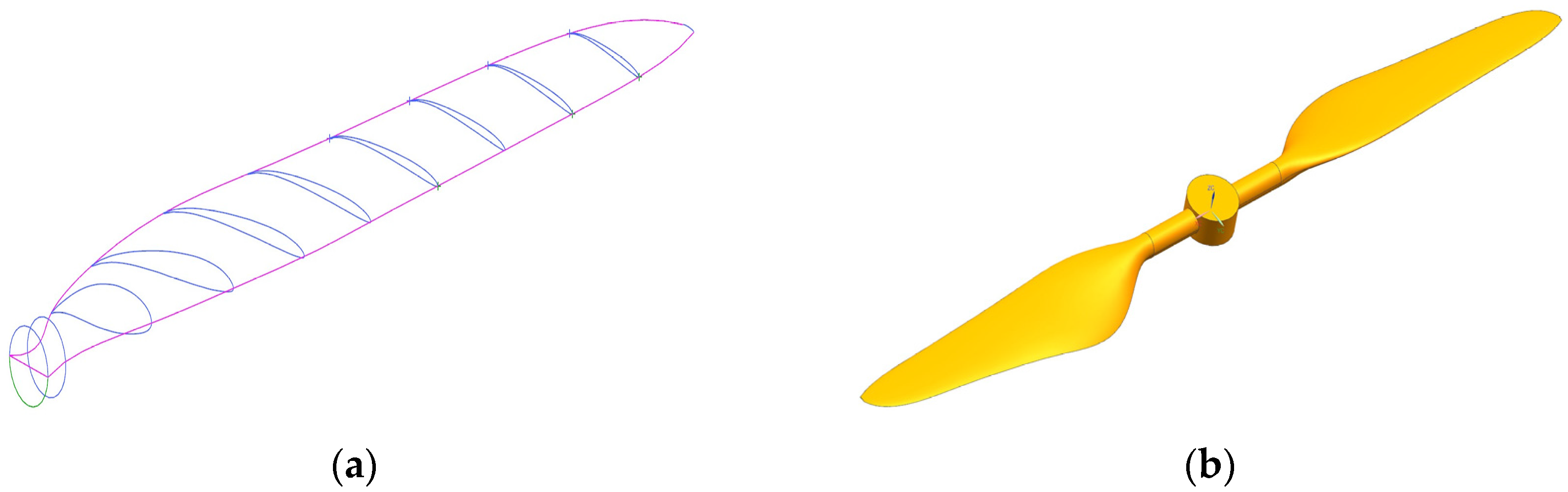
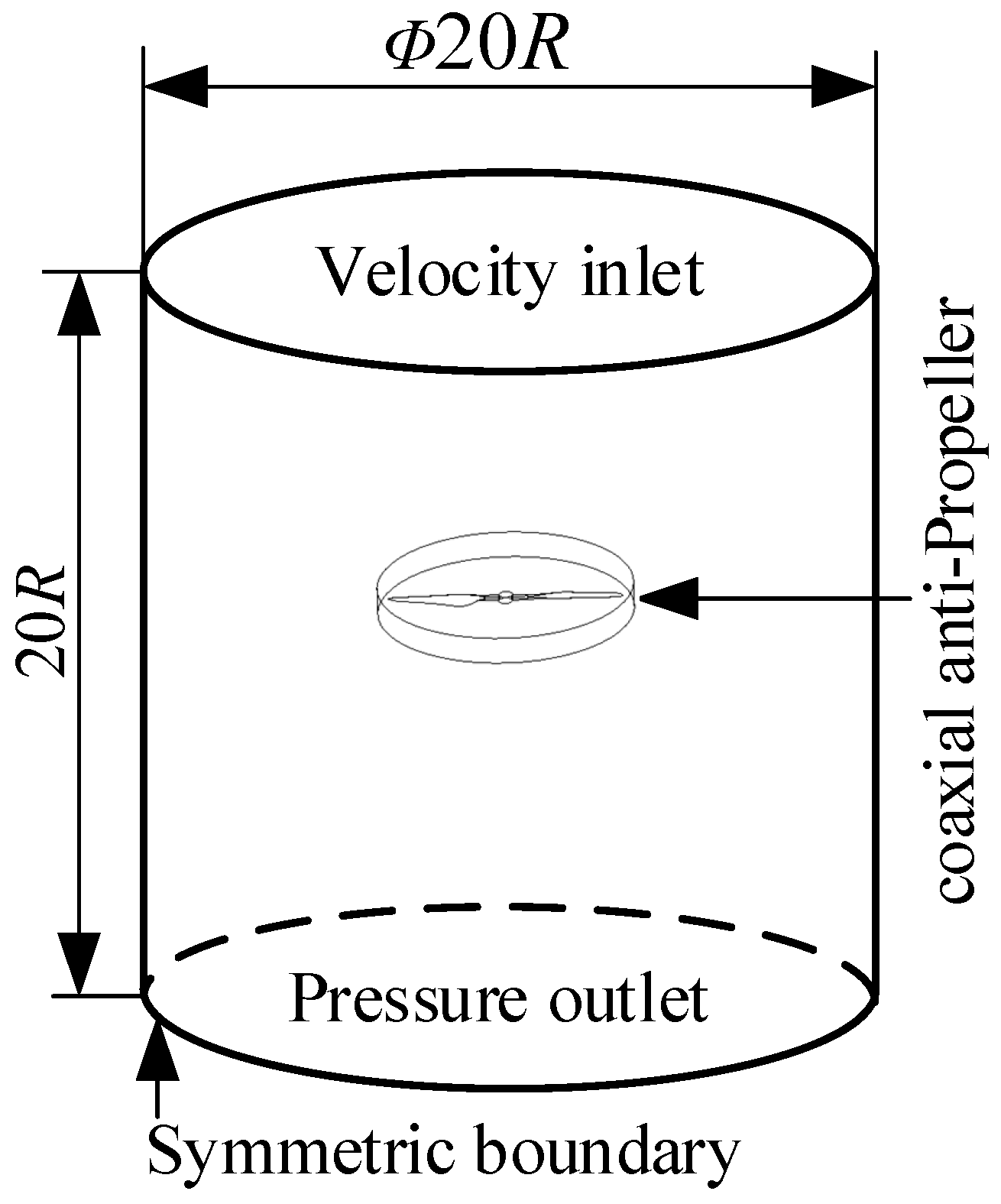



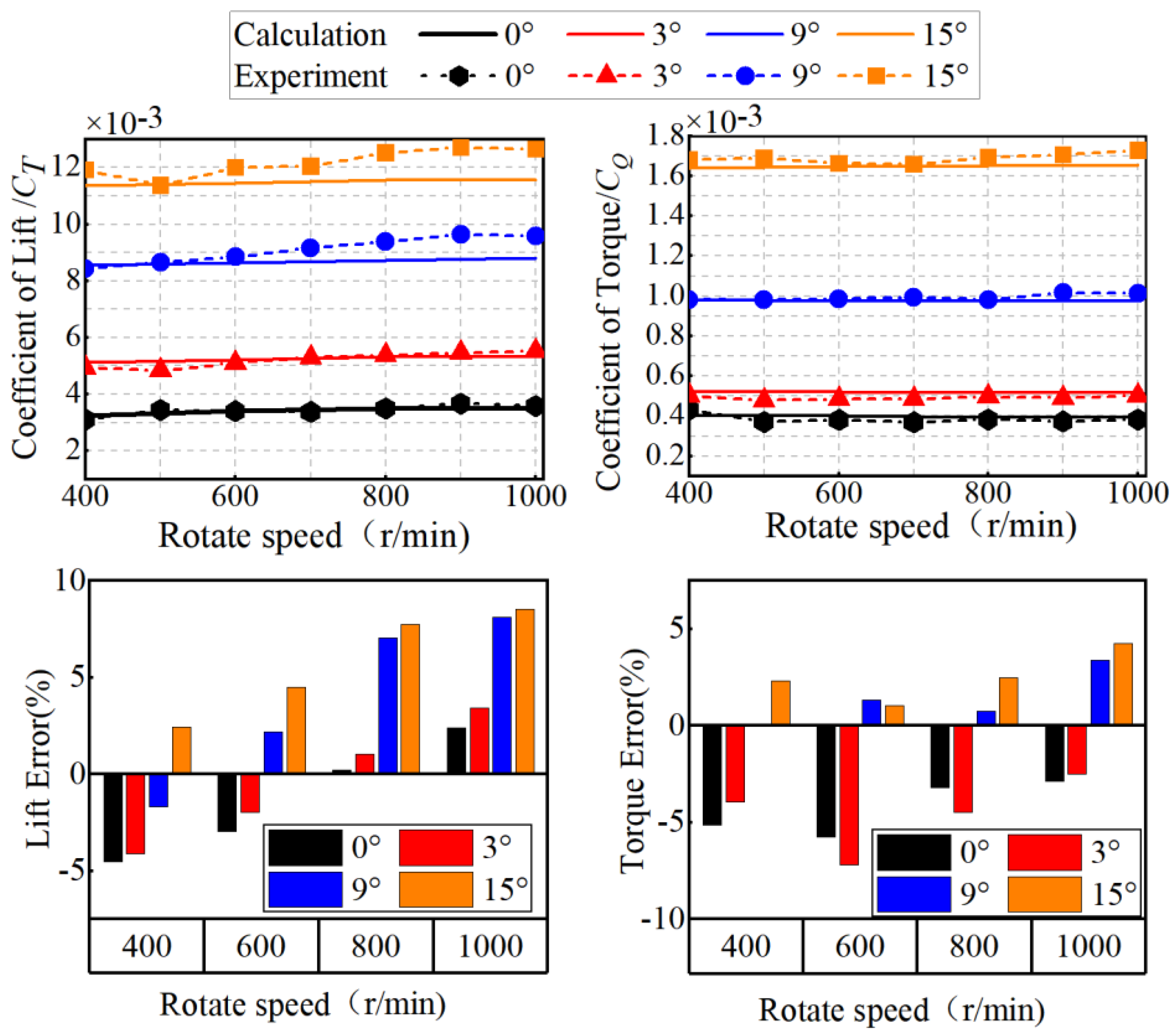
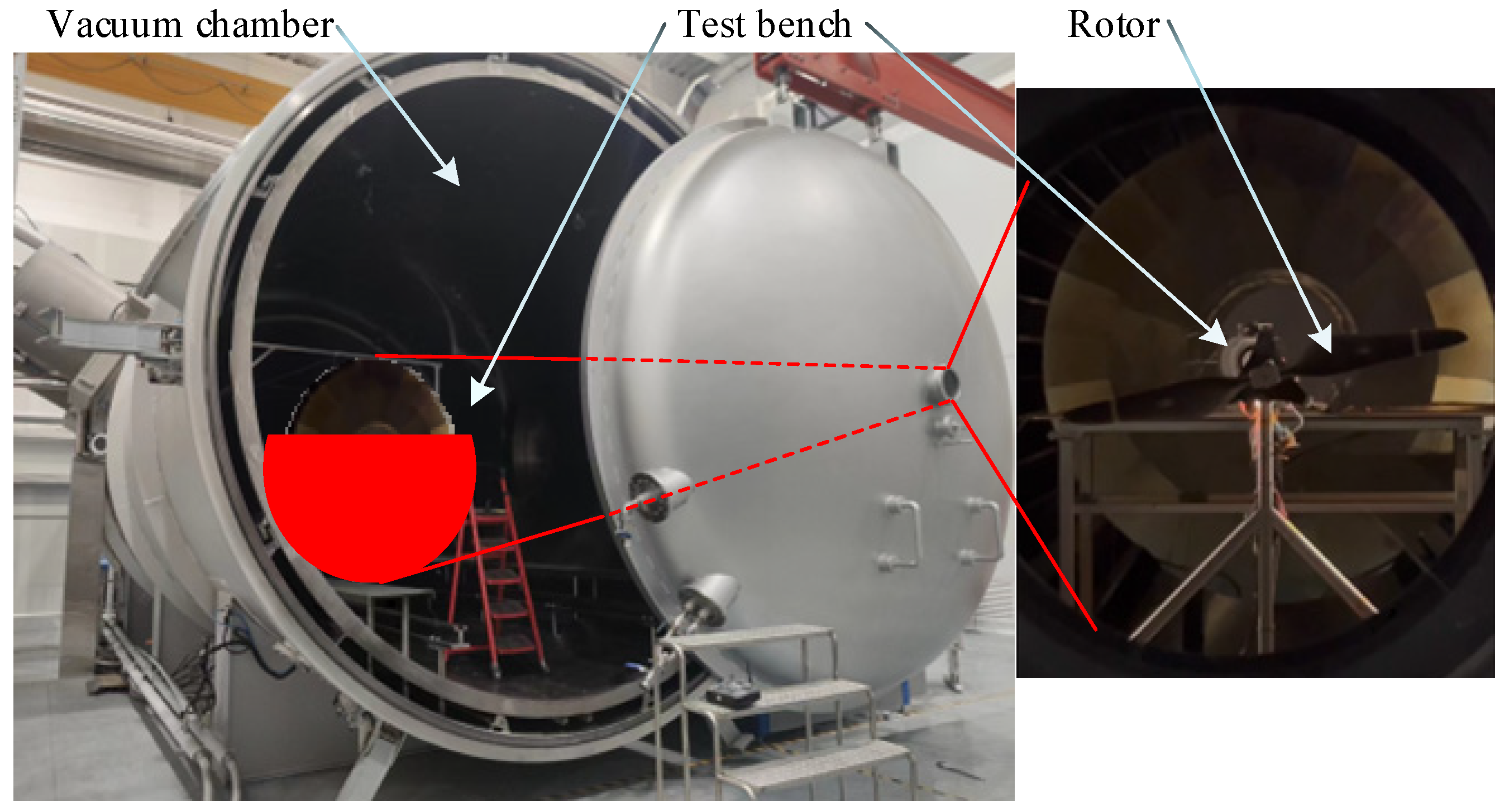
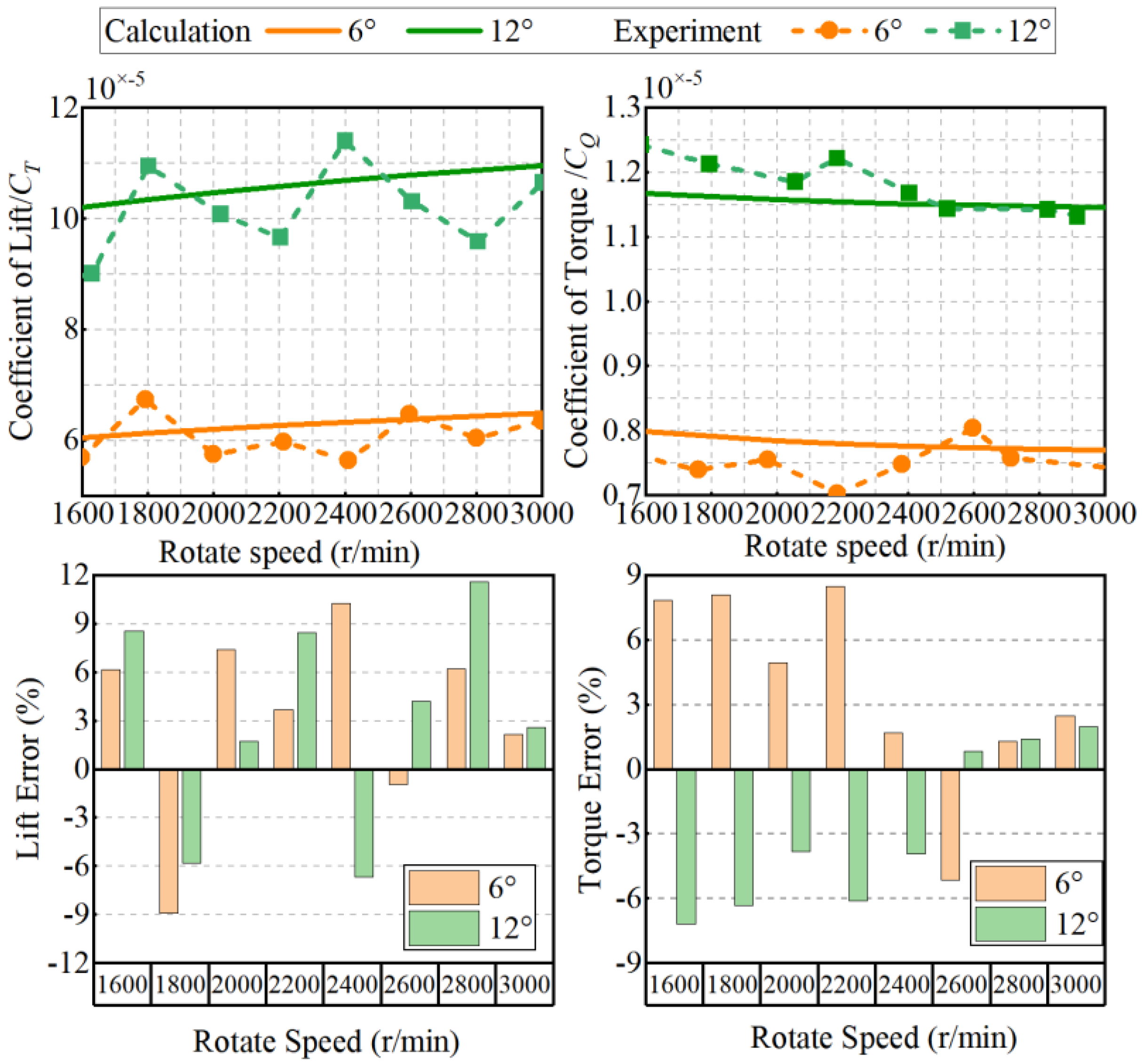
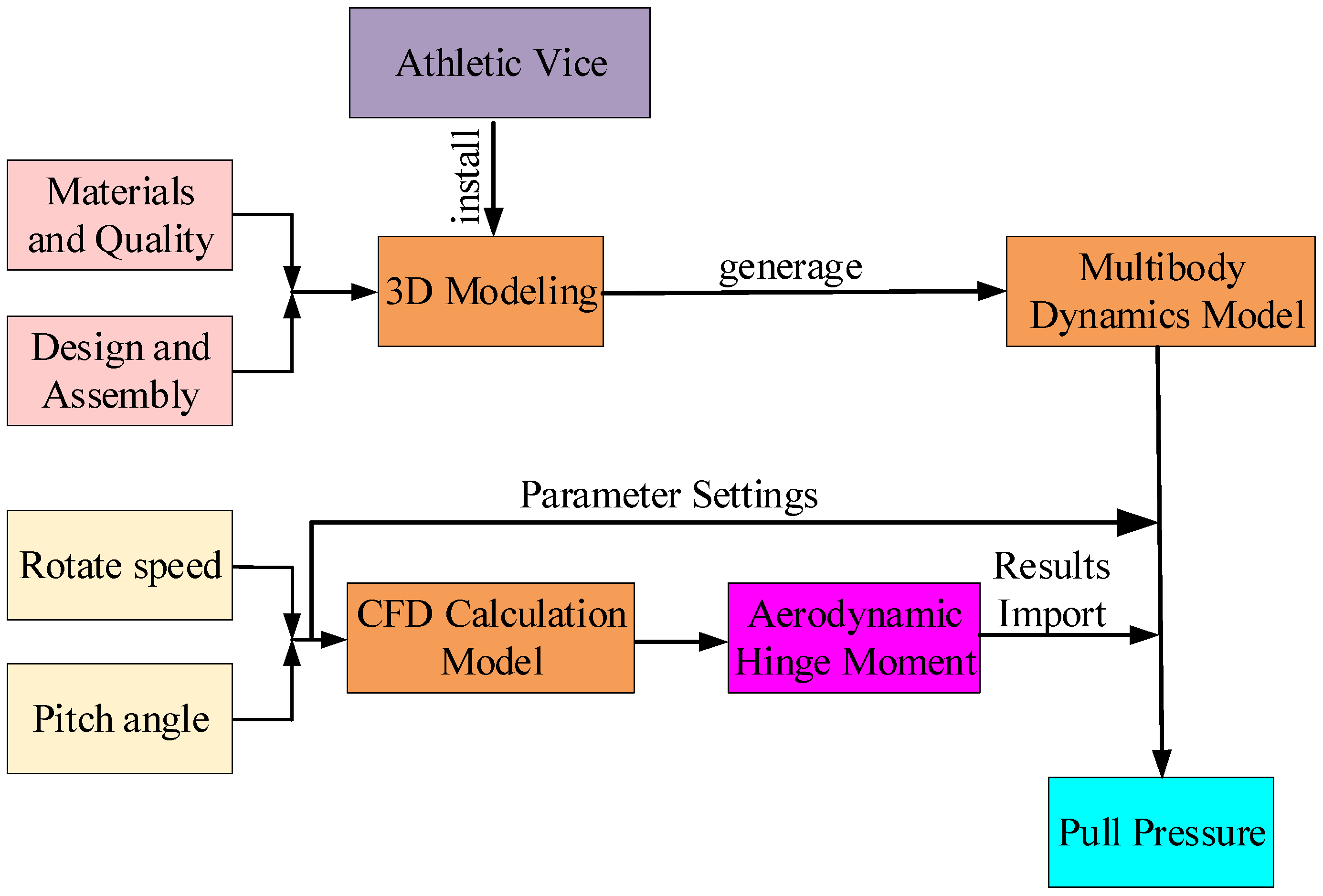

| Type of Aircraft | Model | Research Methodology | Authors | Year |
|---|---|---|---|---|
| Fixed-wing | Fairey Delta 2 | Experimental | Rose R. et al. [23] | 1965 |
| \ | Experimental | Grismer M et al. [24] | 2000 | |
| \ | Analytical and CFD | WU et al. [25] | 2007 | |
| \ | Experimental and CFD | Huang et al. [26] | 2007 | |
| NACA 64A010 | Experimental and CFD | Hambrick E et al. [27] | 2010 | |
| ONERA-M6 | CFD | Zhang et al. [28] | 2013 | |
| Rotor | \ | Analytical and CFD | Xia et al. [18] | 2009 |
| \ | Analytical and CFD | Xu et al. [29] | 2010 | |
| OA213 and OA209 | CFD | Yuan et al. [30] | 2018 | |
| CLF5605 | CFD | Meng et al. [31] | 2023 |
| Parameter Type | Parameter Name | Value or Description |
|---|---|---|
| Domain Parameters | Computational Domain Shape | Cylinder |
| Computational Domain Height | 20R (R is the rotor radius) | |
| Computational Domain Diameter | 20R | |
| Inlet Boundary | The upper surface of the computational domain is set as a velocity inlet boundary with an inlet velocity of 0. | |
| Outlet Boundary | The lower surface of the computational domain is set as a pressure outlet boundary with an outlet pressure of 101,325 Pa. | |
| Ambient Temperature | 288 K | |
| Solver Settings | Turbulent model | SST k-ω |
| Discretization algorithm | The second-order upwind scheme finite volume method | |
| RMS residual | 10−5 | |
| Rotor Parameters | Airfoil Type | clf5605 |
| Chord Length Distribution | The root chord length is 30.45 mm; the tip chord length is 8.86 mm; the chord length at 0.75R is 74.89 mm. |
| Grid Quantity (10,000) | Lift (N) | Aerodynamic Hinge Moment (N.mm) | Lift Error | Hinge Moment Error | |
|---|---|---|---|---|---|
| Stationary Domain | Rotating Domain | ||||
| 17.5 | 593 | 22.3 | 525.1 | 4.93% | 2.63% |
| 27.8 | 593 | 21.2 | 511.3 | 4.25% | 1.96% |
| 89 | 593 | 20.3 | 501.3 | 2.96% | −0.12% |
| 170 | 593 | 19.7 | 501.9 | 0 | −0.58% |
| 245 | 593 | 19.7 | 504.8 | −1.02% | −1.21% |
| 391 | 593 | 19.9 | 510.9 | \ | \ |
| 89 | 23.3 | 18.7 | 503.3 | −3.21% | 0.18% |
| 89 | 47.7 | 19.3 | 502.4 | −7.78% | 0.12% |
| 89 | 97.2 | 17.8 | 501.8 | −10.67% | −0.13% |
| 89 | 199.5 | 19.7 | 502.5 | −1.02% | −0.06% |
| 89 | 271 | 19.9 | 502.8 | −2.01% | 0.30% |
| 89 | 593 | 20.3 | 501.4 | \ | \ |
| Serial Number | Phase/(°) | Pitch Angle (Error)/(°) |
|---|---|---|
| 1 | −0.45 | 0.01 |
| 2 | −30.15 | 0.07 |
| 3 | −59.74 | 0.08 |
| 4 | −90.62 | 0.10 |
| 5 | −120.39 | 0.06 |
| 6 | −149.89 | 0.07 |
| 7 | −179.54 | 0.03 |
| 8 | 149.74 | 0.02 |
| 9 | 119.51 | 0.02 |
| 10 | 90.50 | −0.09 |
| 11 | 59.04 | −0.09 |
| 12 | 30.68 | −0.06 |
| Features | Mars | Earth | Ratio (Mars/Earth) |
|---|---|---|---|
| Acceleration of gravity (m/s2) | 3.72 | 9.80 | 38/100 |
| Atmospheric pressure (Pa) | 636 | 101,325 | 6.3/100 |
| Sound velocity (m/s) | 240 | 343 | 7/10 |
| Air density (kg/m3) | 0.0118 | 1.17 | 1/100 |
| Mean temperature (°C) | −63 | 15 | |
| Viscosity [kg/(m·s)] | 1.289 × 10−5 | 1.789 × 10−5 | 72.1/100 |
| Pitch Angle Test Situation | Pitch Angle/(°) | Rotor Aerodynamic Hinge Moment/(N.mm) | ||
|---|---|---|---|---|
| 200 r/min | 400 r/min | 600 r/min | ||
| 1 | −3° | 18.26 | 77.20 | 178.27 |
| 2 | 0° | 18.60 | 78.80 | 182.90 |
| 3 | 6° | 13.80 | 58.79 | 136.09 |
| 4 | 15° | 7.48 | 32.27 | 76.51 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, Q.; Hu, Y.; Wei, W.; Yao, Z.; Ke, Z.; Zhang, H.; Zhao, M.; Yan, Q. Aerodynamic Hinge Moment Characteristics of Pitch-Regulated Mechanism for Mars Rotorcraft: Investigation and Experiments. Drones 2024, 8, 277. https://doi.org/10.3390/drones8070277
Meng Q, Hu Y, Wei W, Yao Z, Ke Z, Zhang H, Zhao M, Yan Q. Aerodynamic Hinge Moment Characteristics of Pitch-Regulated Mechanism for Mars Rotorcraft: Investigation and Experiments. Drones. 2024; 8(7):277. https://doi.org/10.3390/drones8070277
Chicago/Turabian StyleMeng, Qingkai, Yu Hu, Wei Wei, Zhaopu Yao, Zhifang Ke, Haitao Zhang, Molei Zhao, and Qingdong Yan. 2024. "Aerodynamic Hinge Moment Characteristics of Pitch-Regulated Mechanism for Mars Rotorcraft: Investigation and Experiments" Drones 8, no. 7: 277. https://doi.org/10.3390/drones8070277
APA StyleMeng, Q., Hu, Y., Wei, W., Yao, Z., Ke, Z., Zhang, H., Zhao, M., & Yan, Q. (2024). Aerodynamic Hinge Moment Characteristics of Pitch-Regulated Mechanism for Mars Rotorcraft: Investigation and Experiments. Drones, 8(7), 277. https://doi.org/10.3390/drones8070277








