Wind Tunnel Balance Measurements of Bioinspired Tails for a Fixed Wing MAV
Abstract
1. Introduction
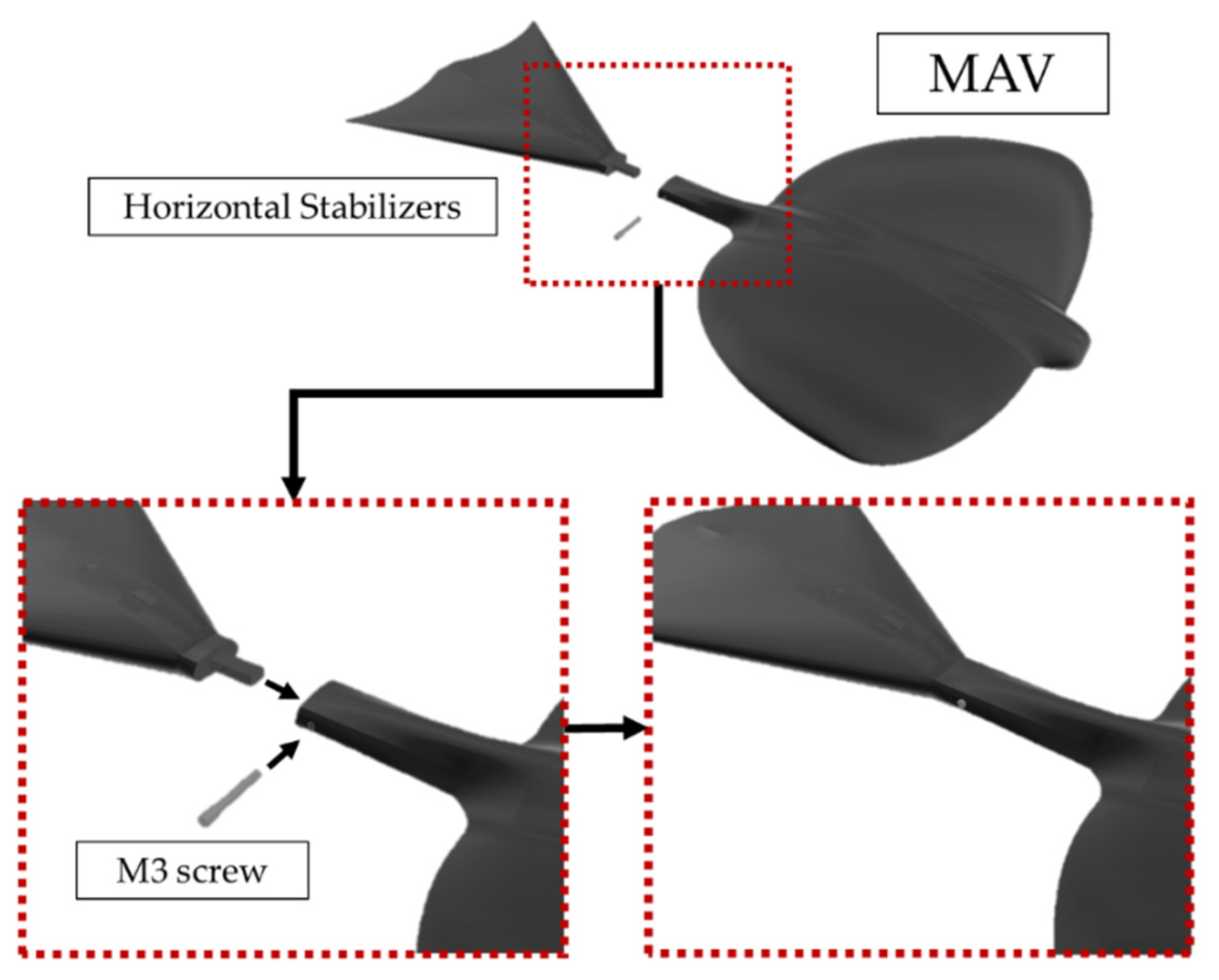
2. Bioinspired MAV
2.1. Base Model
2.2. Bioinspired Horizontal Stabilizers
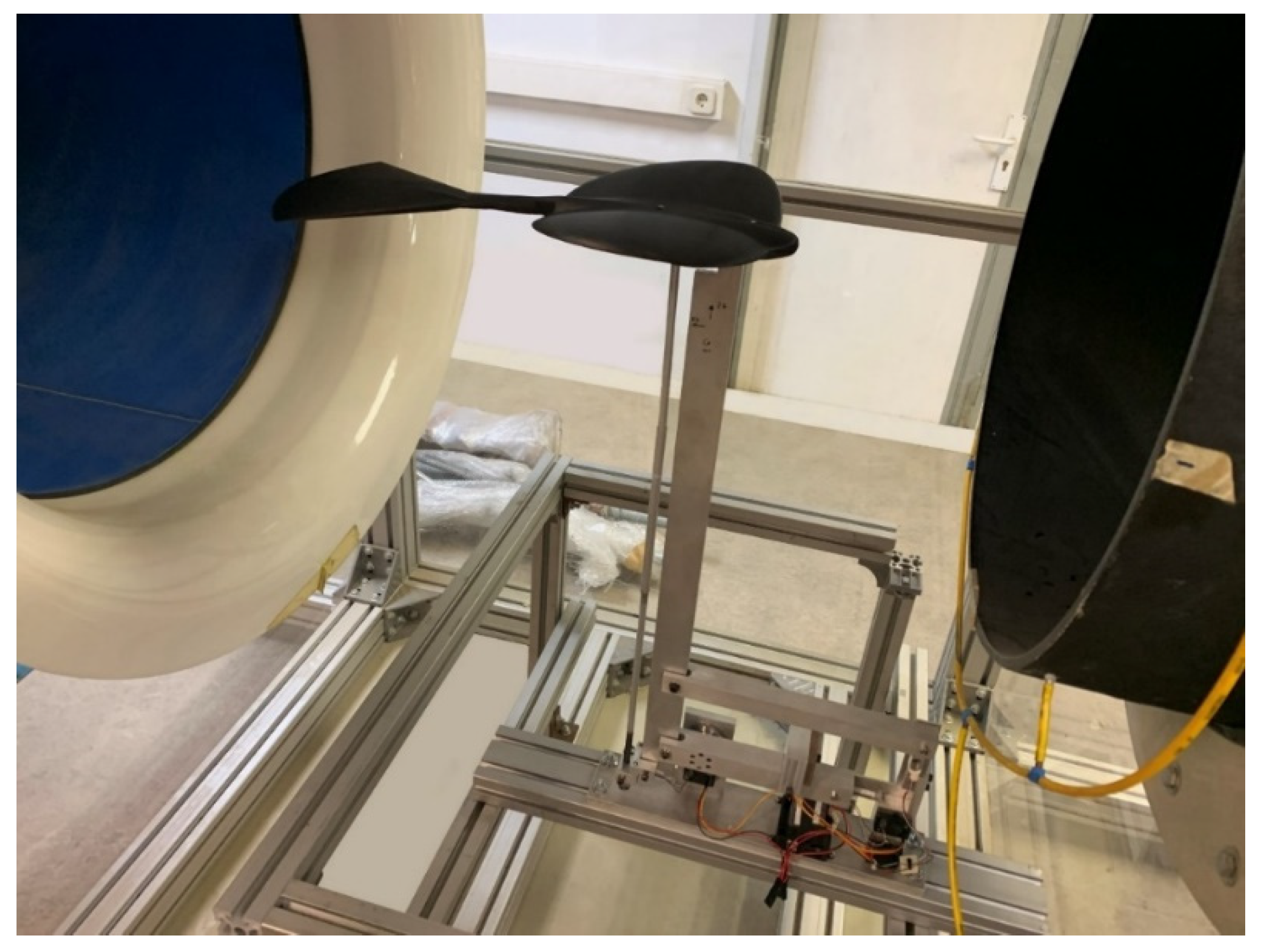
3. Experimental Set-Up
3.1. Wind Tunnel
3.2. External Balance
3.3. Testing Model
3.4. Experimental Tests
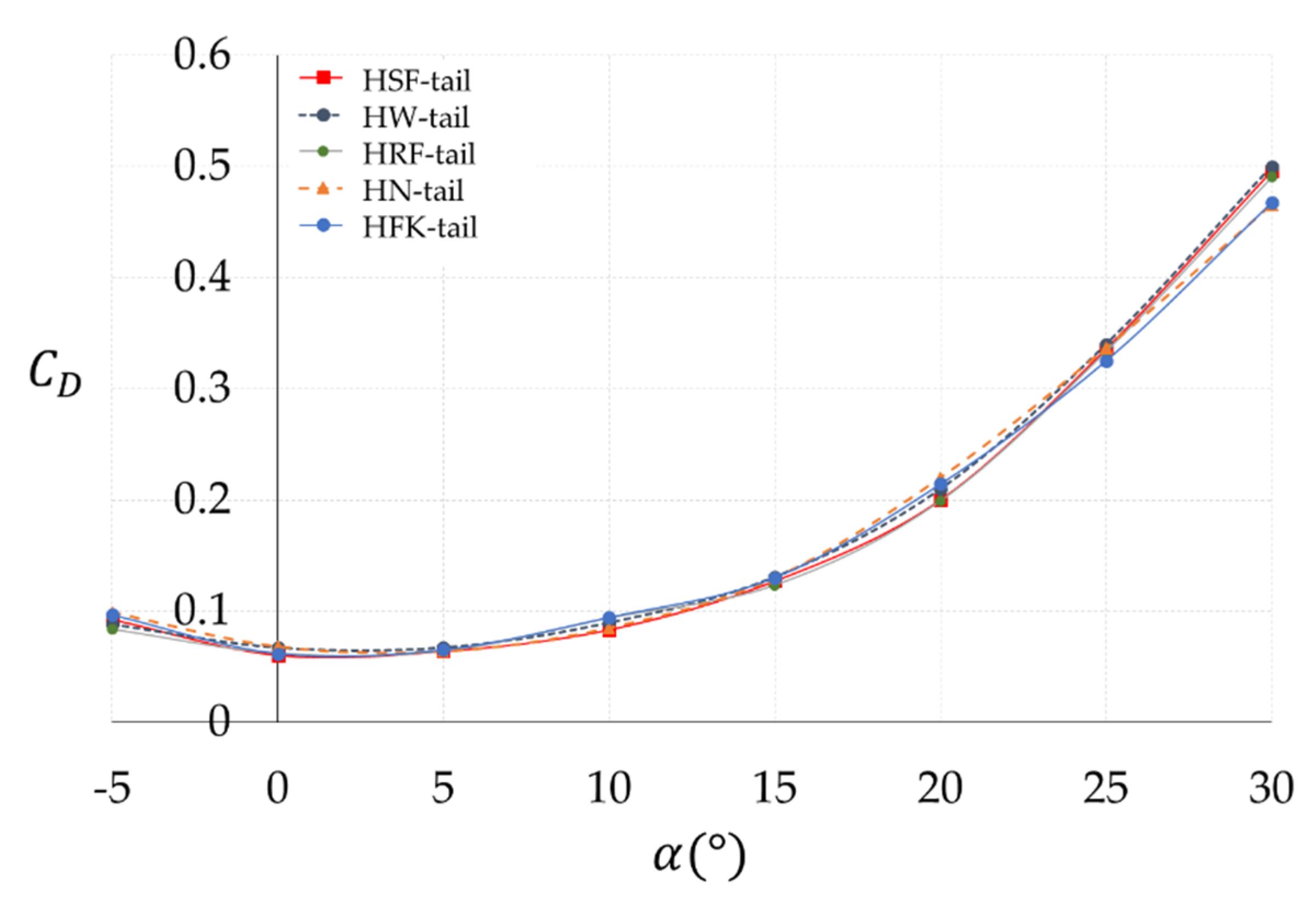
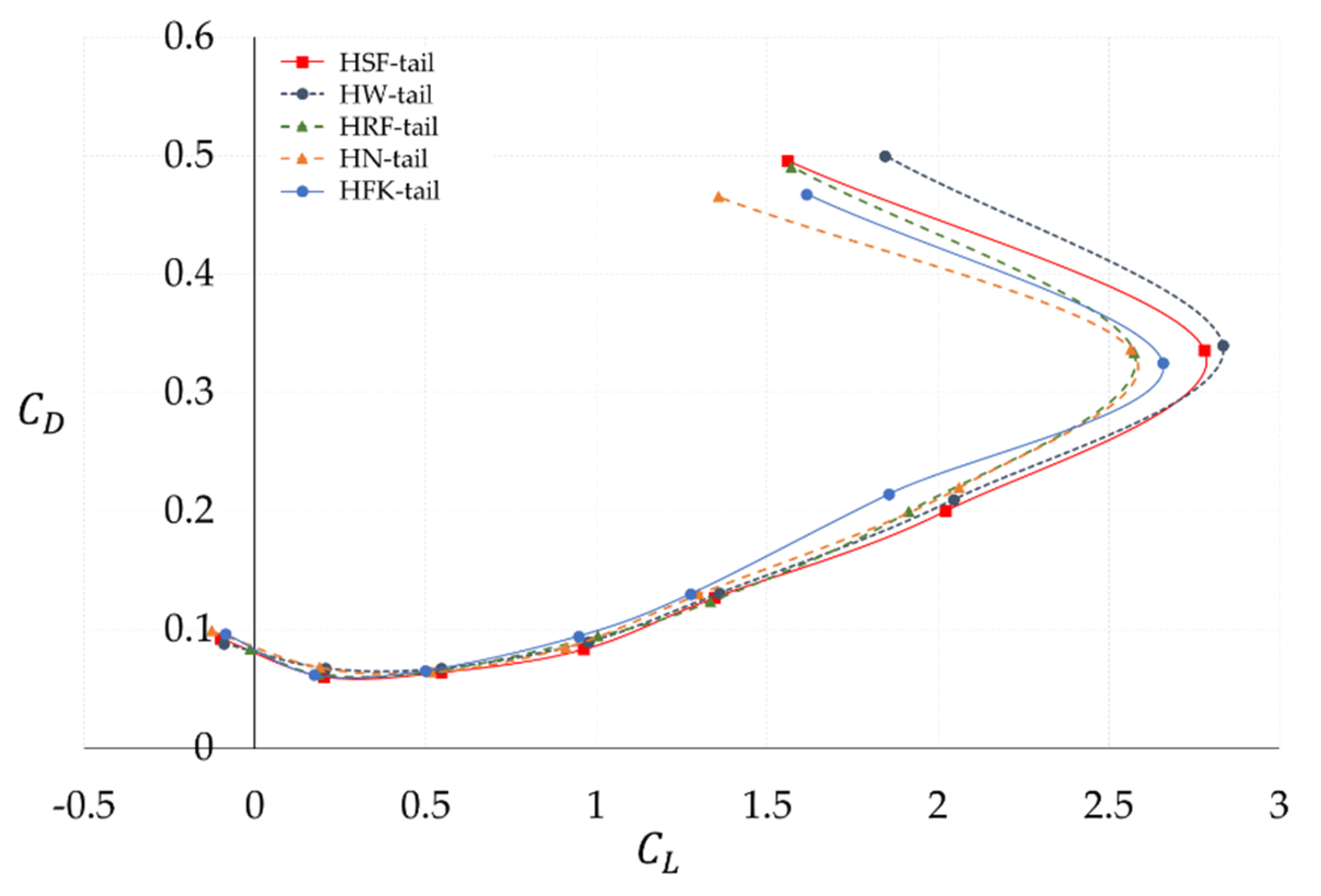
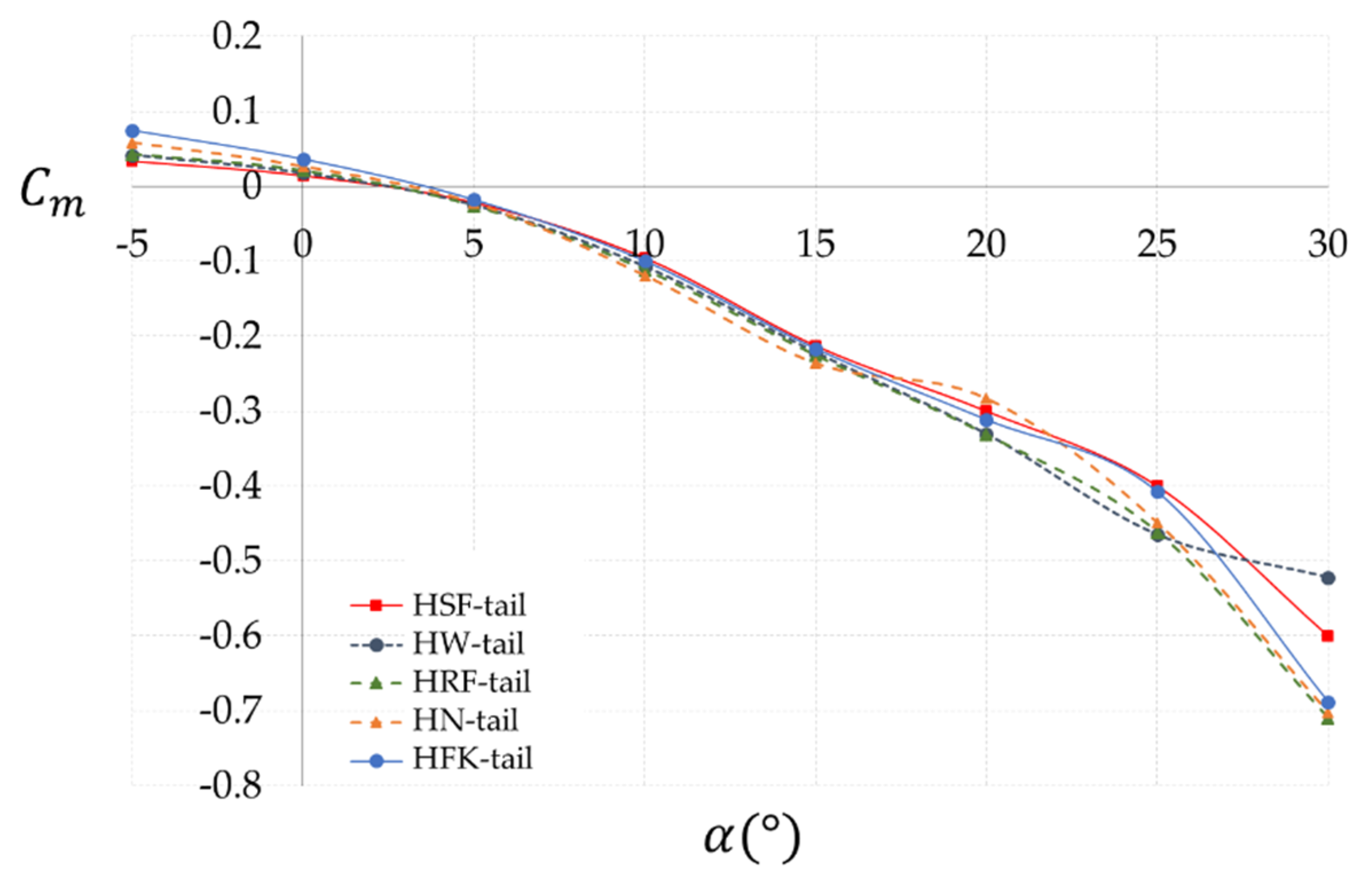
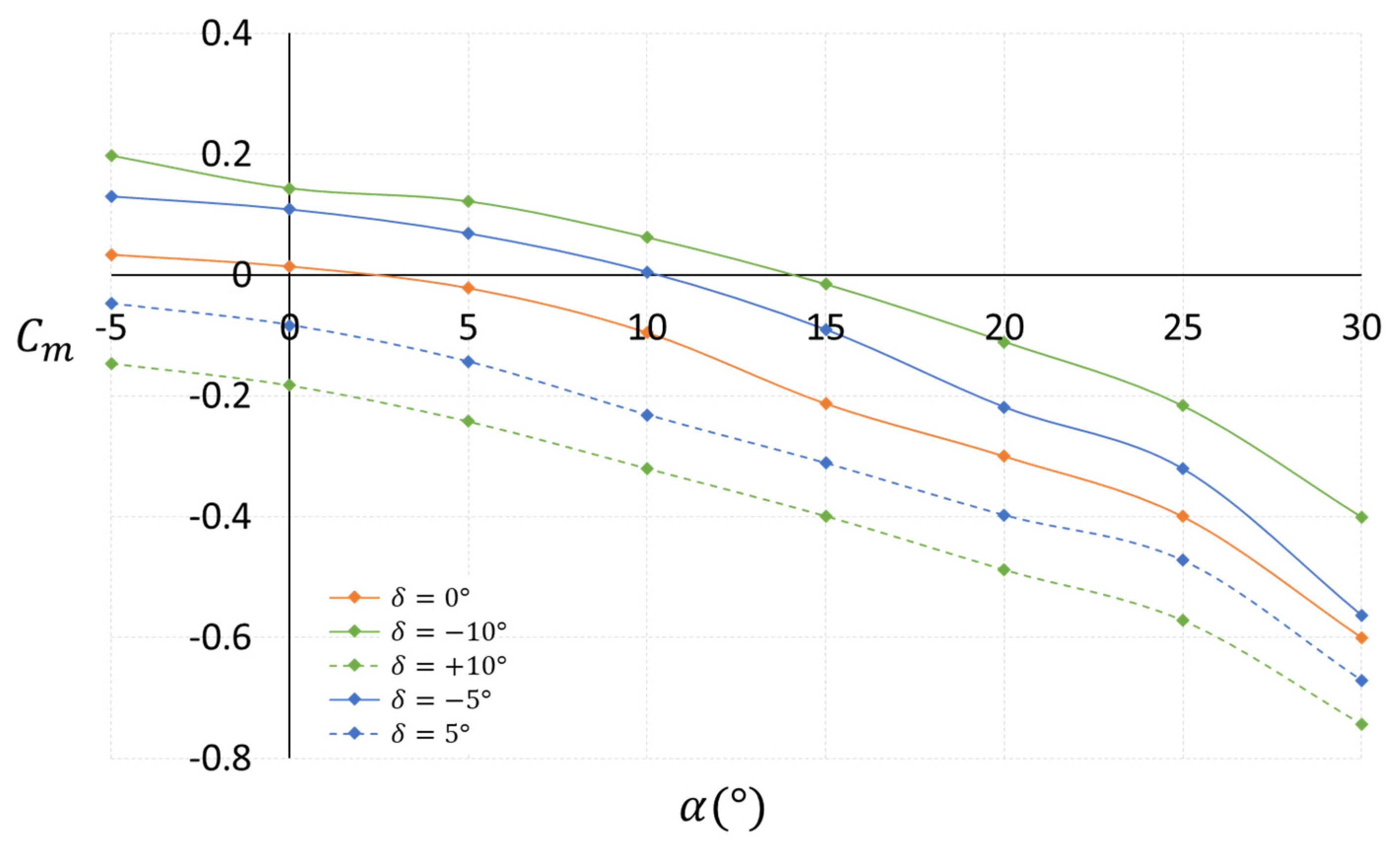
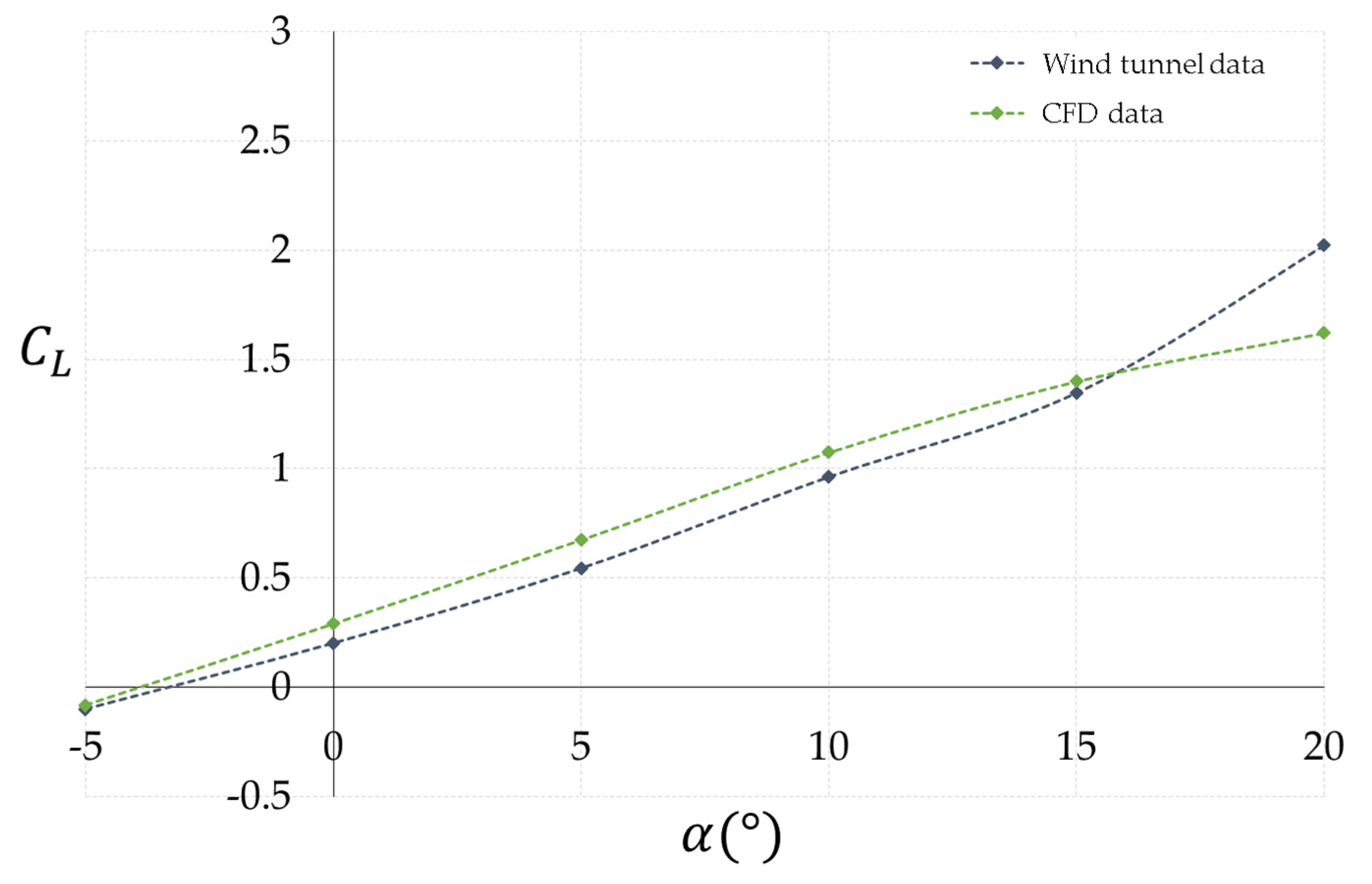
4. Analysis of the Results
4.1. Measurements with External Balance
4.2. Deflection-Horizontal Stabilizer
4.3. Experimental Validation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hassanalian, M.; Abdelkefi, A. Classifications, applications and design challenges of drones: A review. Prog. Aerosp. Sci. 2017, 91, 99–131. [Google Scholar] [CrossRef]
- Harvey, C.; Inman, D.J. Aerodynamic efficiency of gliding birds vs. comparable UAVs: A review. Bioinspiration Biomim. 2021, 16, 031001. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.; Zhou, Z.; Dudley, R.; MacKem, S.; Chuong, C.M.; Erickon, G.M.; Varricchio, D.J. An integrative approach to understanding bird origins. Science 2014, 346, 1253293. [Google Scholar] [CrossRef] [PubMed]
- Balmford, A.; Jones, I.L.; Thomas, A.L.R. On avian asymmetry: Evidence of natural selection for symmetrical tails and wings in birds. Proc. Biol. Sci. 1993, 252, 245–251. [Google Scholar] [CrossRef]
- Sachs, G. Tail effect on yaw stability in birds. J. Theor. Biol. 2007, 249, 464–472. [Google Scholar] [CrossRef] [PubMed]
- Thomas, A.R.L. On the aerodynamics of birds’ tails. Pilos. Trans. Biol. Sci. 1993, 340, 361–380. [Google Scholar] [CrossRef]
- Ltshuler, D.L.; Bahlman, J.W.; Dakin, R.; Gaede, A.H.; Goller, B.; Lentink, D.; Segre, P.S.; Skandalis, D.A. The biophysics of bird flight: Functional relationships integrate aerodynamics, morphology, kinematics, muscles, and sensors. Can. J. Zool. 2015, 93, 961–975. [Google Scholar] [CrossRef]
- Thomas, A.L.R. The flight of birds that have wings and a tail: Variable geometry expands the envelope of flight performance. J. Theor. Biol. 1996, 183, 237–245. [Google Scholar] [CrossRef]
- Ward, T.A.; Fearday, C.J.; Salami, E.; Binti Soin, N. A bibliometric review of progress in micro air vehicle research. Int. J. Micro Air Veh. 2017, 9, 146–165. [Google Scholar] [CrossRef]
- Ariyanto, M.; Setiawan, J.D.; Prabowo, T.; Haryanto, I. Design of a Low-Cost Fixed Wing UAV. In Proceedings of the 2nd International Joint Conference on Advanced Engineering and Technology (IJCAET 2017) and International Symposium on Advanced Mechanical and Power Engineering (ISAMPE 2017), Bali, Indonesia, 24–26 August 2017. [Google Scholar]
- Keennon, M.; Klingebiel, K.; Won, H. Development of the nano hummingbird: A tailless flapping wing micro air vehicle. In Proceedings of the 50th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Nashville, TN, USA, 9–12 January 2012; p. 588. [Google Scholar]
- Folkertsma, G.A.; Straatman, W. Robird A Robotic Bird of Prey. IEEE Robot. Autom. Mag. 2017, 24, 22–29. [Google Scholar] [CrossRef]
- Ajanic, E.; Feroskhan, M.; Mintchev, S.; Noca, F.; Floreano, D. Bioinspired wing and tail morphing extends drone flight capabilities. Sci. Robot. 2020, 5, eabc2897. [Google Scholar] [CrossRef] [PubMed]
- Zheng, L.; Zhou, Z.; Sun, P.; Zhang, Z.; Wang, R. A novel control mode of bionic morphing tail based on deep reinforcement learning. arXiv 2020. [Google Scholar] [CrossRef]
- Murayama, Y.; Nakata, T.; Liu, H. Aerodynamic performance of a bird-inspired morphing tail. J. Biomech. Sci. Eng. 2023, 18, 22–00340. [Google Scholar] [CrossRef]
- Chang, E.; Lentink, D. Biohybrid morphing tail aerial robot. In Proceedings of the 9th Internatinal Symposium on Adaptive Motion of Animals and Machines, Lausanne, Switzerland, 20–23 August 2019. [Google Scholar]
- Parga, J.R.; Reeder, M.F.; Leveron, T.; Blackburn, K. Experimental study of a micro air vehicle with a rotatable tail. J. Aircr. 2007, 44, 1761–1768. [Google Scholar] [CrossRef]
- Gamble, L.L.; Inman, D.J. A tale of two tails: Developing an avian inspired morphing actuator for yaw control and stability. Bioinspiration Biomim. 2018, 13, 026008. [Google Scholar] [CrossRef] [PubMed]
- Pérez Sanchez, V.; Gómez Tamm, A.E.; Savastano, E.; Arrue, B.C. Bio-Inspired Morphing Tail for Flapping-Wings Aerial Robots Using Macro Fiber Composites. Appl. Sci. 2021, 11, 2930. [Google Scholar] [CrossRef]
- Barcala-Montejano, M.A.; Rodríguez-Sevillano, A.; Crespo-Moreno, J.; Bardera Mora, R.; Silva-González, A.J. Optimized performance of a morphing micro air vehicle. In Proceedings of the 2015 International Conference on Unmanned Aircraft Systems (ICUAS), Denver, CO, USA, 9–12 June 2015; IEEE: New York, NY, USA, 2015; pp. 794–800. [Google Scholar]
- Bardera, R.; Rodríguez-Sevillano, A.A.; García-Magariño, A.; Barroso, E. Wake Measurements of a MAV with Morphing Geometry. In Proceedings of the 8th European Conference for Aeronautics and Space Sciences (EUCASS), Madrid, Spain, 1–4 July 2019. [Google Scholar]
- Barroso Barderas, E.; Bardera Mora, R.; Rodríguez-Sevillano, A.A.; Matías García, J.C. Numerical Analysis of Bioinspired Tails in a Fixed-Micro Air Vehicle. Aerospace 2023, 10, 793. [Google Scholar] [CrossRef]
- Barroso Barderas, E.; Rodríguez-Sevillano, A.A.; Barderas Mora, R.; Crespo Moreno, J.; Matías García, J.C. Design of Non-Conventional Flight Control Systems for Bioinspired Micro Air Vehicles. Drones 2022, 6, 248. [Google Scholar] [CrossRef]
- Barderas, E.B.; Bardera, R.; Rodríguez-Sevillano, Á.; Matias-Garcia, J.C.; Muñoz-Campillejo, J. Experimental Analysis of the T-Tail Vortex effect in a Bioinspired Micro Air Vehicle. In Proceedings of the AIAA Aviation Forum, Chicago, IL, USA, 27 June–1 July 2022. [Google Scholar]
- Mueller, T.J. Introduction to the Design of Fixed-Wing Micro Air Vehicles; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1982. [Google Scholar]
- Carmichael, B.H. Low Reynolds Number Airfoil Survey, Volume 1; Technical Report NASA-Cr-154803-Vol-1; National Aeronautics and Space Administration: Washington, DC, USA, 1981.











| Geometrical Features of the Base Model | Value |
|---|---|
| Fuselage length, | |
| Fuselage width, | |
| Dihedral angle, | |
| Wingspan, | |
| Wing tip chord, | |
| Wing root chord, | |
| Wing reference surface, | |
| Taper ratio, | |
| Aspect ratio, | |
| Mean aerodynamic chord, | |
| Mean geometrical chord, |
| Configurations | |||||
|---|---|---|---|---|---|
| HSF-tail | 200 | 170 | 2.10 | 0 | 0 |
| HRF-tail | 196 | 166.6 | 2.10 | −1 | 0 |
| HSF-tail | 200 | 170 | 2.10 | 0 | 0 |
| HFK-tail | 215 | 121 | 2.10 | 3 | 0 |
| HN-tail | 196 | 170 | 2.05 | −1 | 0 |
| HW-tail | 196 | 170 | 2.05 | −1 | 0 |
| Aerodynamic Component | |
|---|---|
| 1.272 | |
| 1.158 | |
| 0.723 |
| Aerodynamic Parameters | Value | Horizontal Stabilizer |
|---|---|---|
| HN-tail | ||
| HW-tail | ||
| HSF-tail | ||
| HSF-tail | ||
| HSF-tail |
| Variation | HN-Tail | HW-Tail | HSF-Tail | HRF-Tail | HFK-Tail |
|---|---|---|---|---|---|
| - | |||||
| - | |||||
| - | |||||
| - | |||||
| - |
| Efficiency | HW-Tail | HN-Tail | HSF-Tail | HRF-Tail | HFK-Tail |
|---|---|---|---|---|---|
 |  |  |  |  |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bardera, R.; Rodríguez-Sevillano, Á.A.; Barroso, E.; Matías, J.C.; Sor Mendi, S. Wind Tunnel Balance Measurements of Bioinspired Tails for a Fixed Wing MAV. Drones 2024, 8, 16. https://doi.org/10.3390/drones8010016
Bardera R, Rodríguez-Sevillano ÁA, Barroso E, Matías JC, Sor Mendi S. Wind Tunnel Balance Measurements of Bioinspired Tails for a Fixed Wing MAV. Drones. 2024; 8(1):16. https://doi.org/10.3390/drones8010016
Chicago/Turabian StyleBardera, Rafael, Ángel. A. Rodríguez-Sevillano, Estela Barroso, Juan Carlos Matías, and Suthyvann Sor Mendi. 2024. "Wind Tunnel Balance Measurements of Bioinspired Tails for a Fixed Wing MAV" Drones 8, no. 1: 16. https://doi.org/10.3390/drones8010016
APA StyleBardera, R., Rodríguez-Sevillano, Á. A., Barroso, E., Matías, J. C., & Sor Mendi, S. (2024). Wind Tunnel Balance Measurements of Bioinspired Tails for a Fixed Wing MAV. Drones, 8(1), 16. https://doi.org/10.3390/drones8010016









