Study on the Evolution Law of Overlying Strata Structure in Stope Based on “Space–Air–Ground” Integrated Monitoring Network and Discrete Element
Abstract
1. Introduction
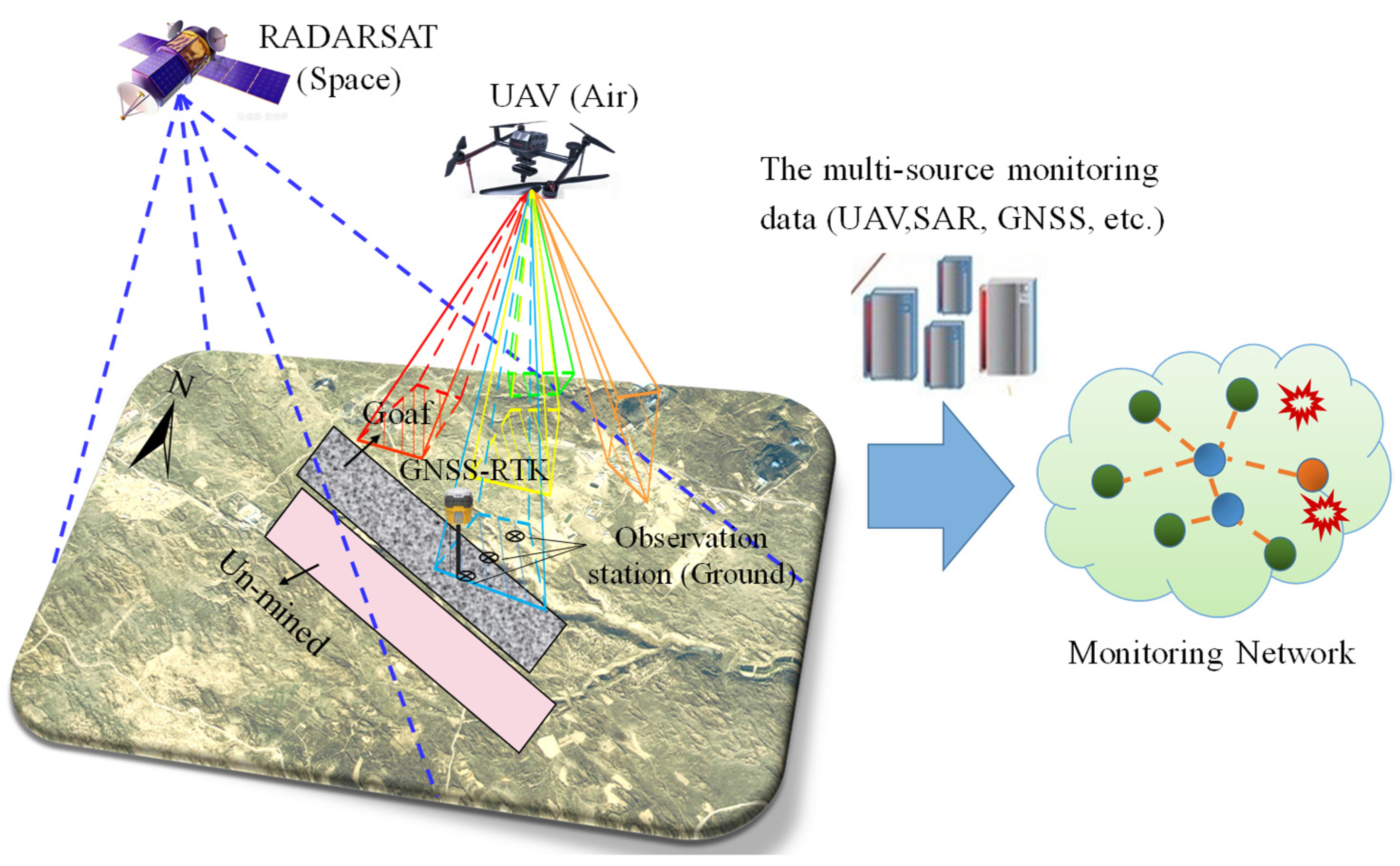
- Using the advantages of various subsidence monitoring technologies and the technical framework of the Internet of Things (IoT), a “space–air–ground” integrated collaborative monitoring network is constructed.
- The evolution law of overlying strata structure is studied based on discrete element simulations and theoretical analysis.
- A discrete element mechanical parameter inversion method based on the “space–air–ground” integrated monitoring network is proposed.
- Based on the theory of “arch–beam” structure and numerical simulations results, the overlying strata structure model of adjacent mining in the mining area is constructed and evaluated using real datasets.
2. Related Work
3. Overview of the Study Area and Datasets
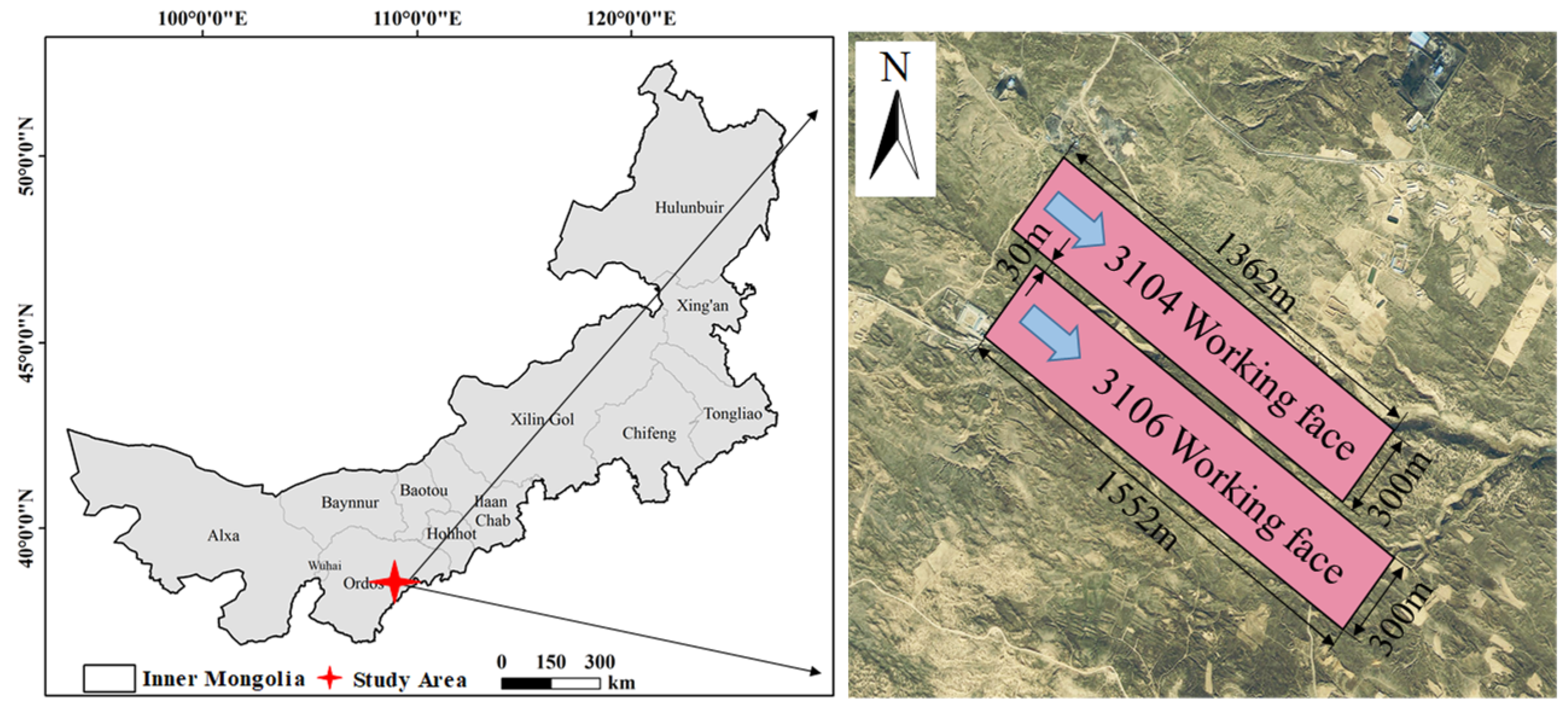
3.1. Study Area
3.2. Datasets
4. Research Methods
4.1. “Space–Air–Ground” Integrated Monitoring Network
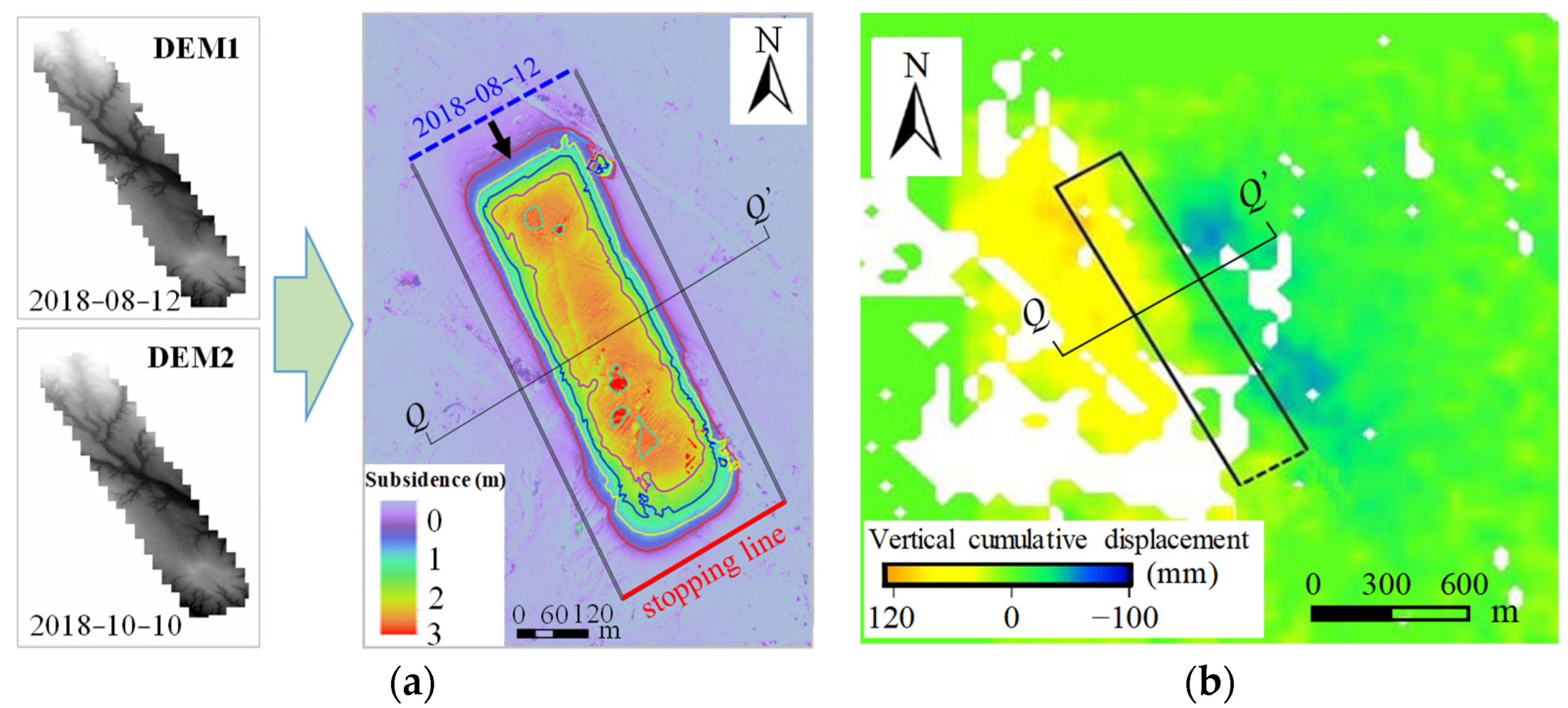
4.1.1. UAV and InSAR Solution of Subsidence Basin
4.1.2. UAV/InSAR Fusion Subsidence Basin
4.2. Discrete Element Numerical Simulation
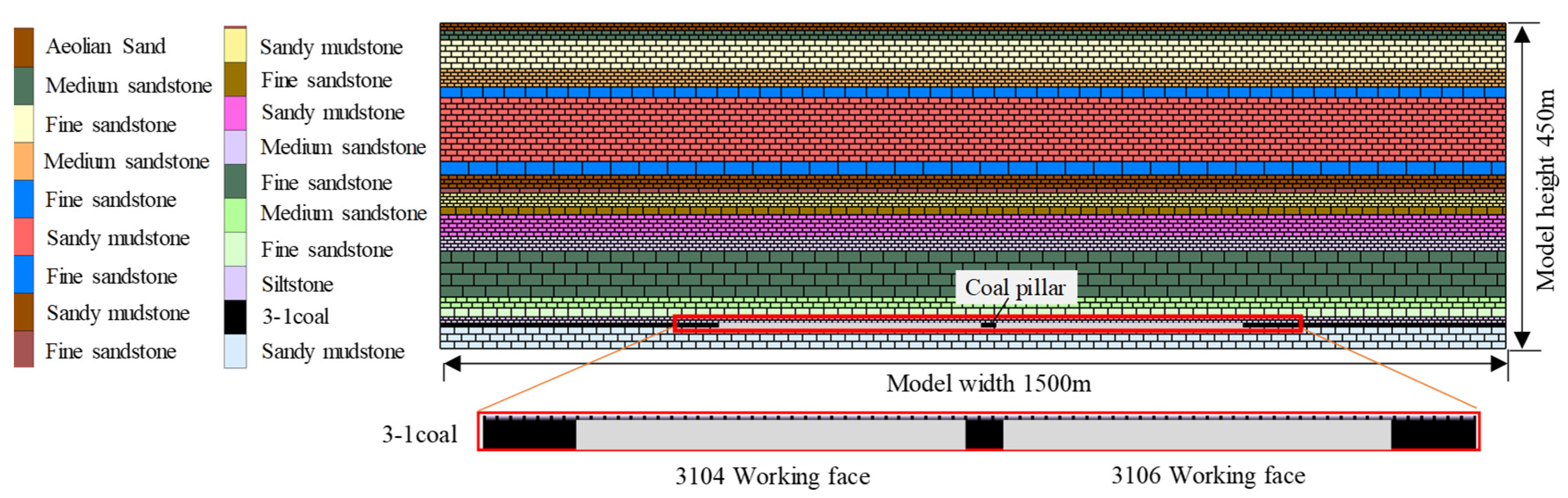
4.2.1. Numerical Model Establishment
4.2.2. Inversion of Mechanical Parameters Based on “Space–Air–Ground” Integrated Monitoring Network
5. Results and Analysis
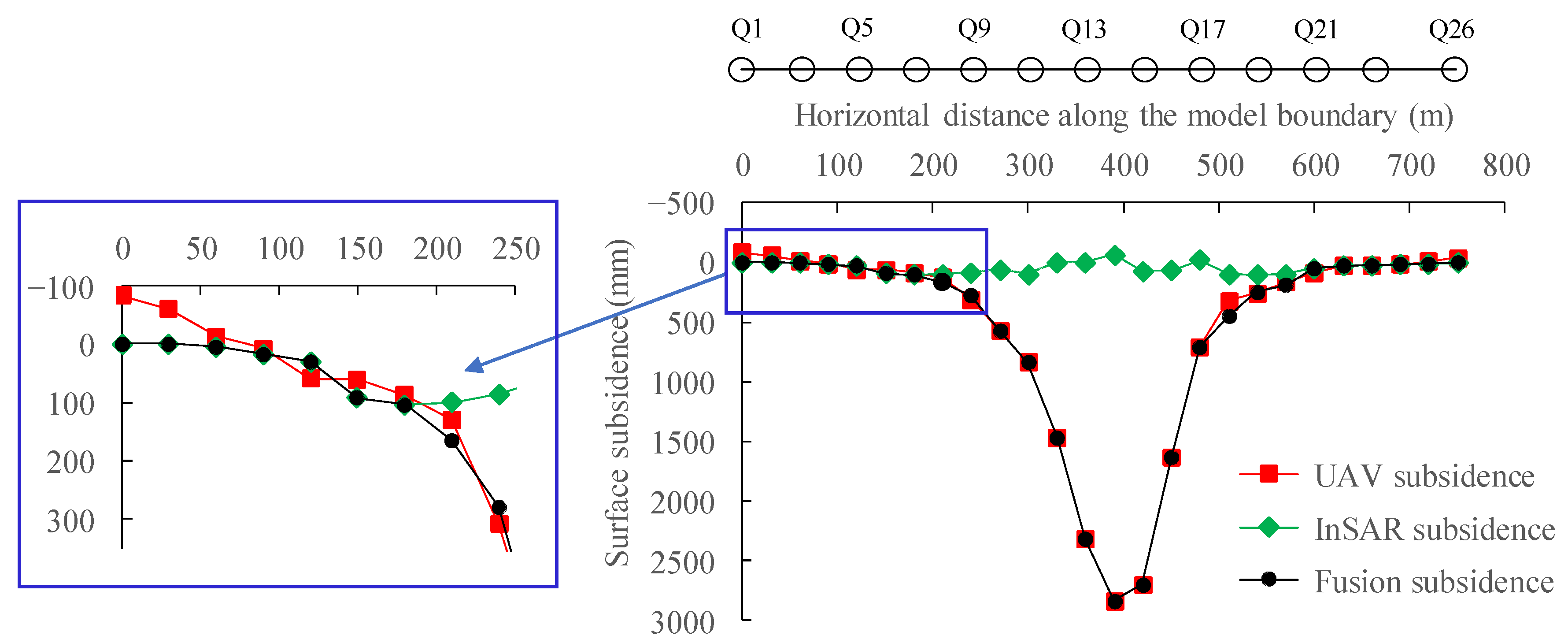
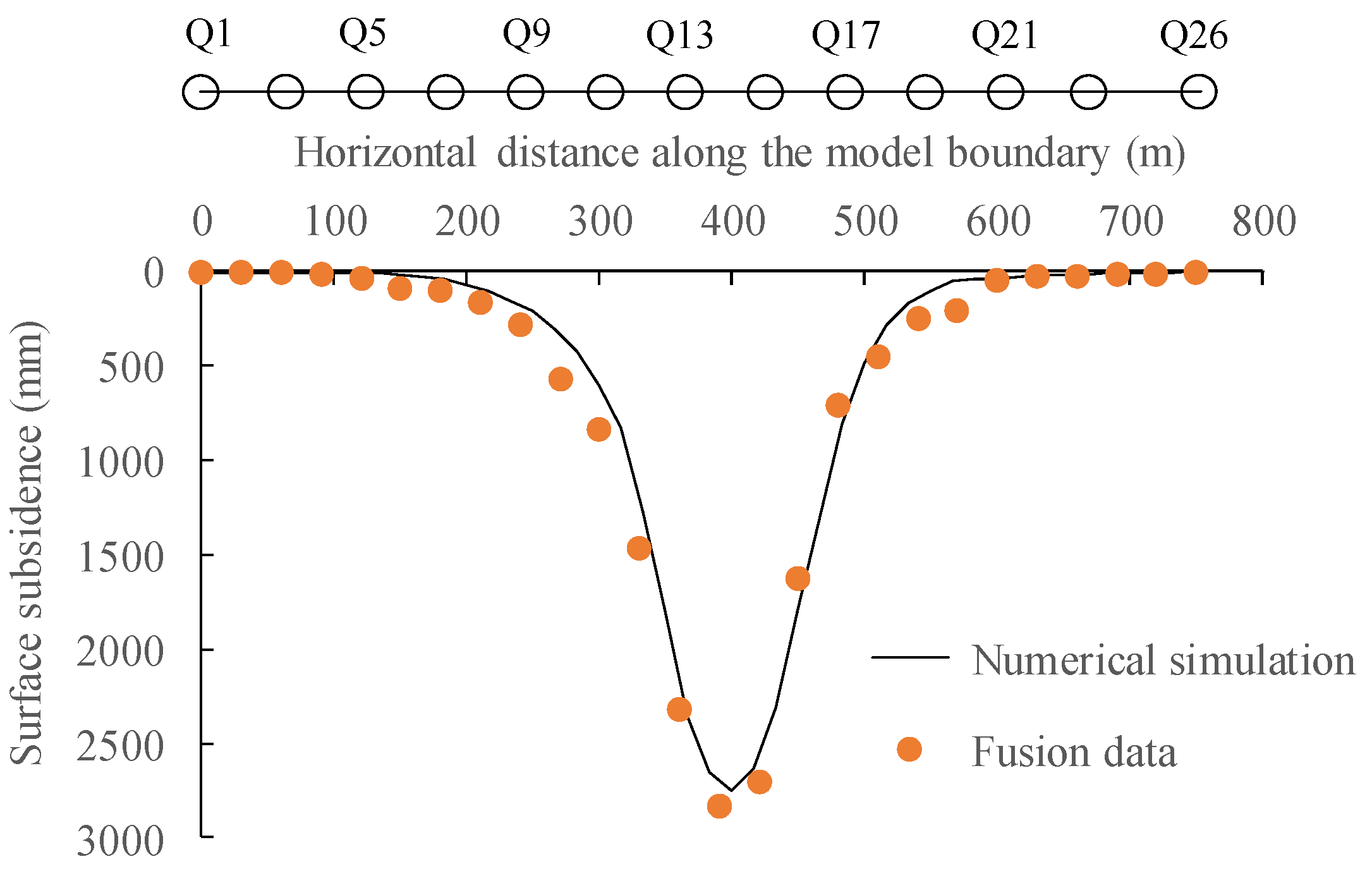
5.1. Accuracy Assessment of “Space–Air–Ground” Integrated Monitoring Network
| Point Mark | UAV/InSAR Fusion Subsidence Value (mm) | GNSS Monitoring Subsidence Value (mm) |
|---|---|---|
| Q3 | 5 | 19 |
| Q9 | 325 | 280 |
| Q14 | 2823 | 2856 |
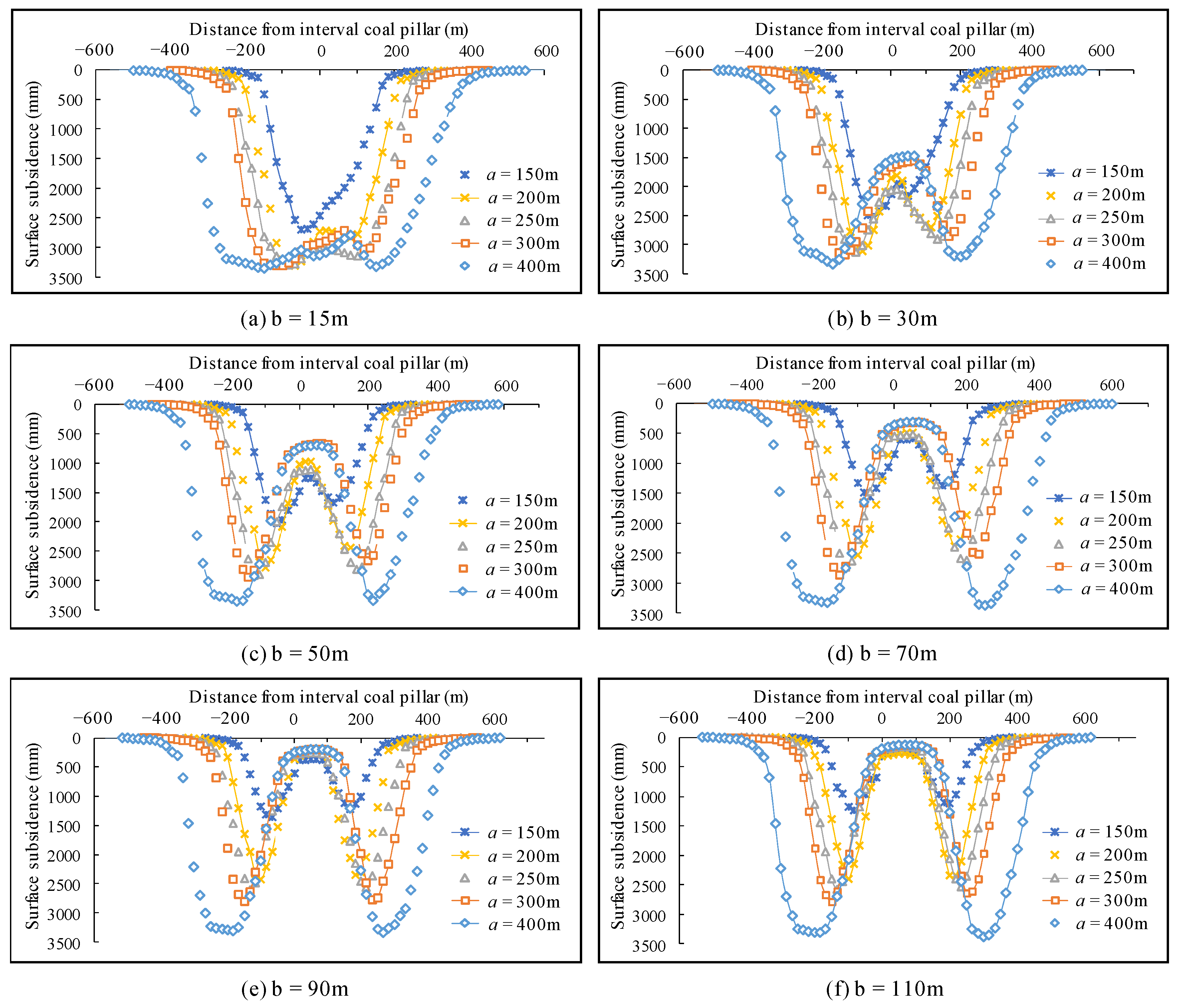
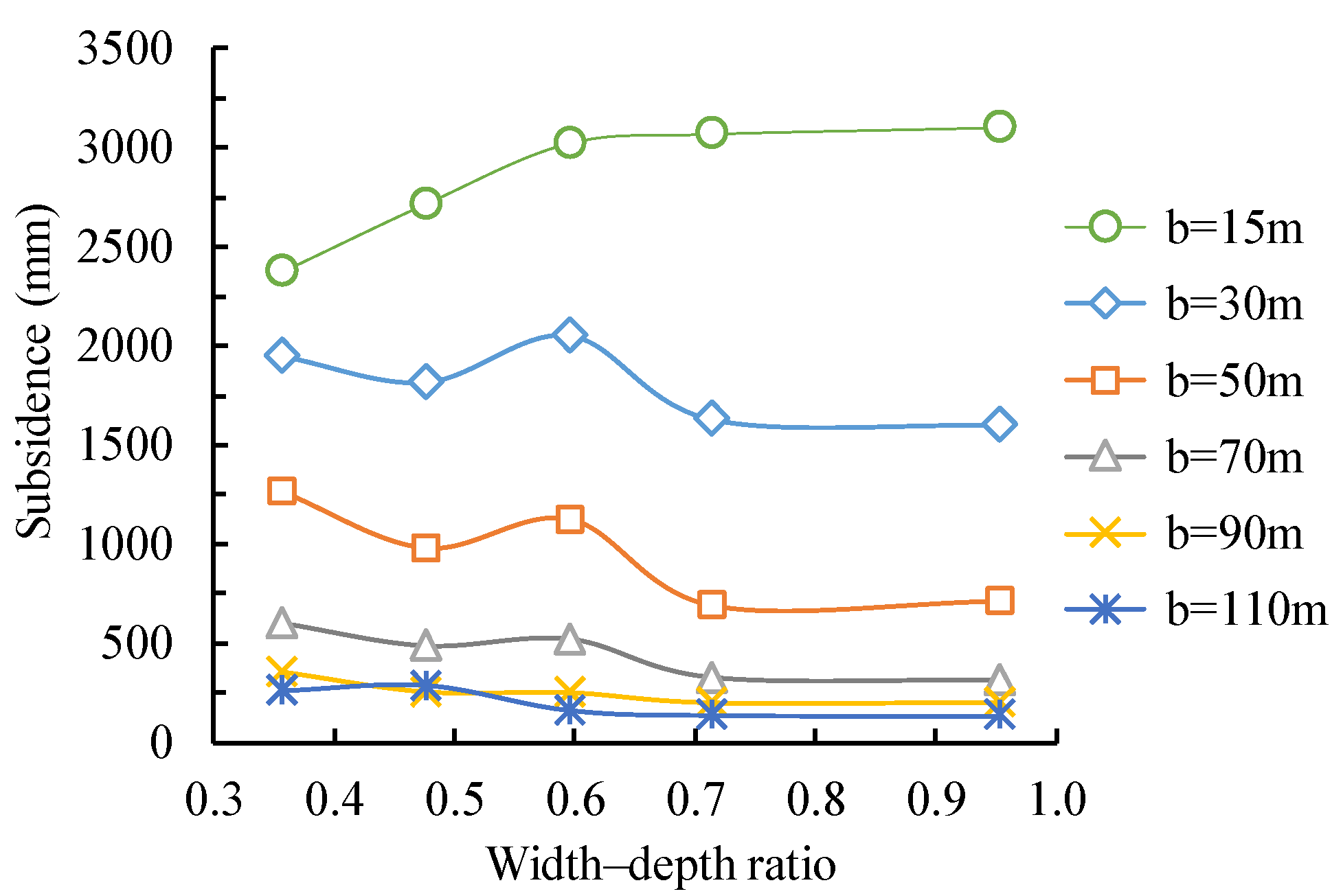
5.2. Analysis of Adjacent Mining Surface Subsidence Results
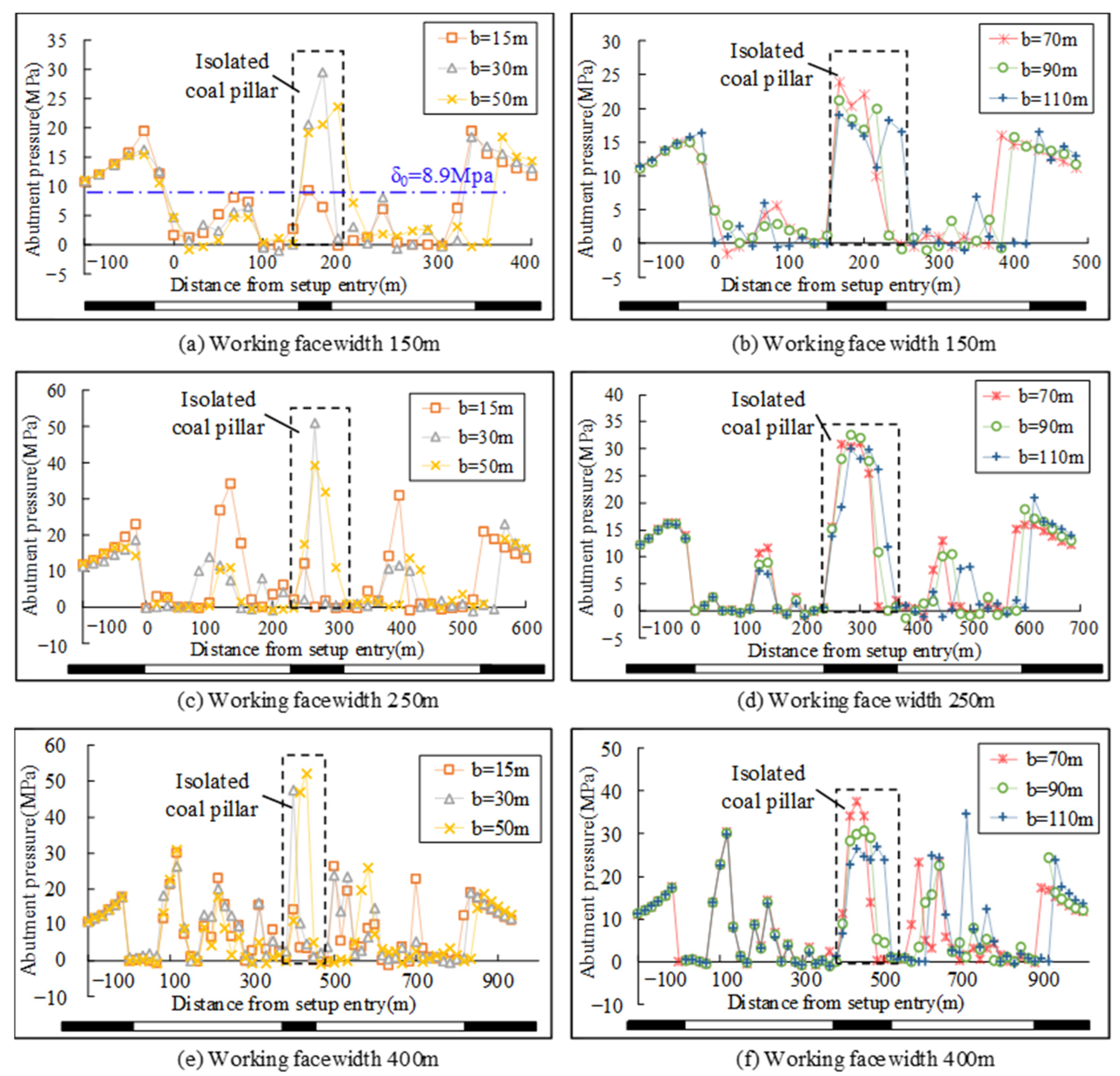
5.3. Analysis of Stress Change in Goaf and Coal Pillar during Adjacent Mining
6. Discussion
6.1. Comparison of “Space–Air–Ground” Integrated Monitoring Network with Existing Monitoring Methods
6.2. Surface Sufficiency Change and Interval Coal Pillar Stability in Adjacent Mining
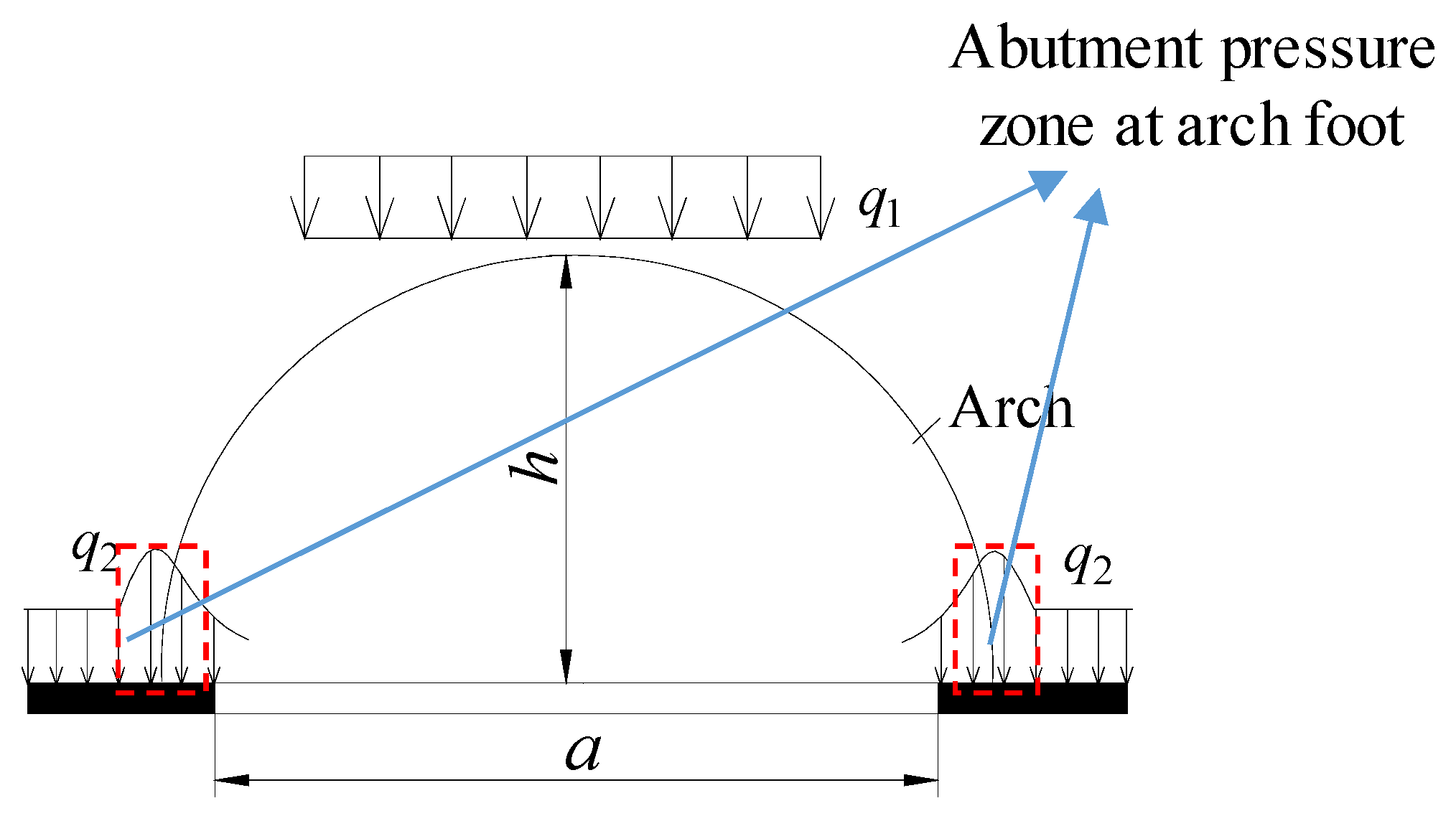
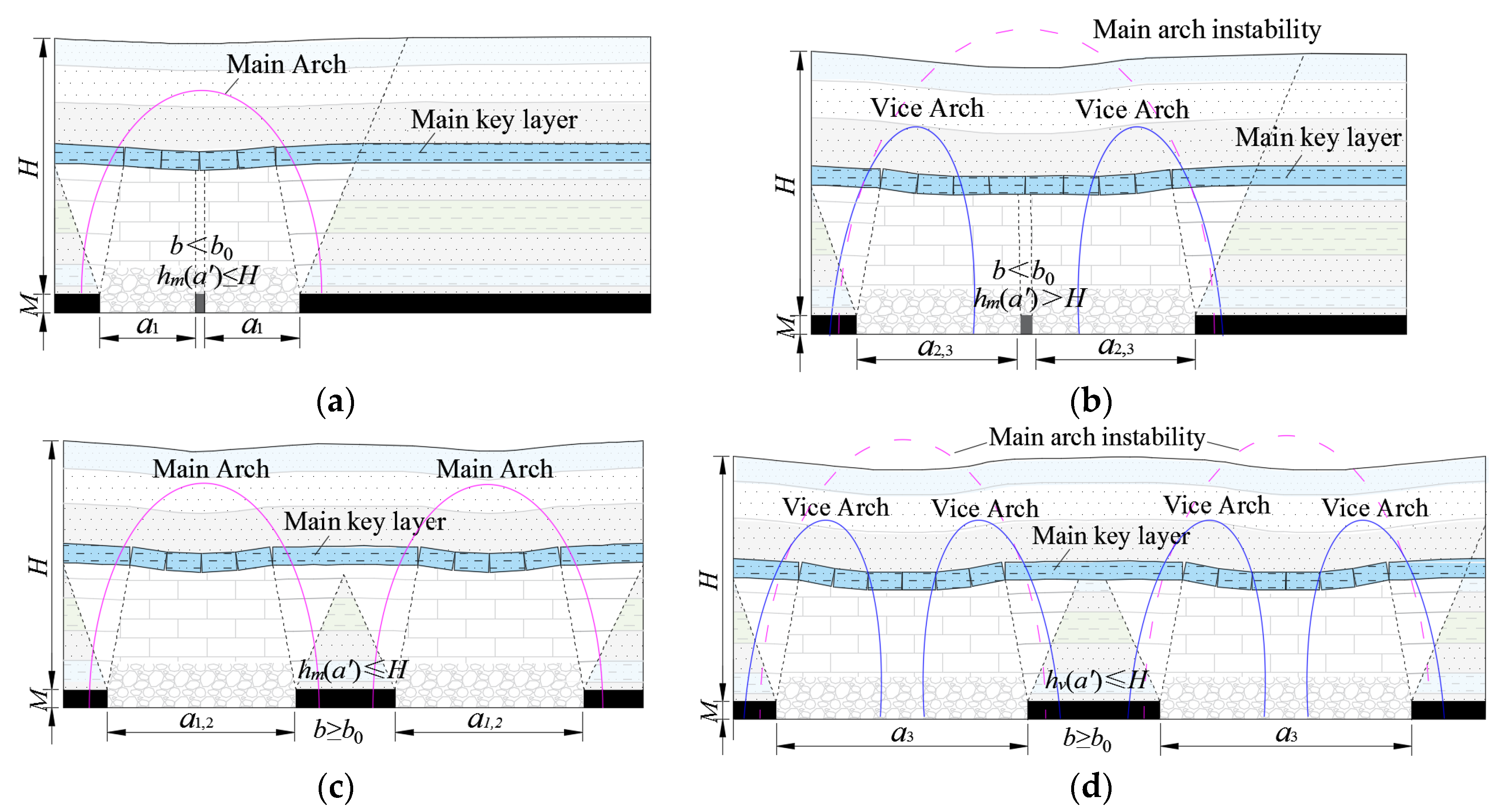
6.3. Evolution Law of Overlying Strata Structure in Adjacent Mining Face
- For mining widths a1 and a2, there is a main arch structure in the overlying strata. When the interval coal pillar width b ≥ b0, then the coal pillar is stable, the goaf on both sides is separated, and the double arch feet are formed above the coal pillar. The development height of the main rock vault on both sides does not exceed the surface, forming the structure of the double main arch + “W-shaped” beam structure, as shown in Figure 13c. At this time, if the width of the coal pillar is relatively small (b = 30 m or 50 m), then the two arch feet overlap, and the single stress peak state appears above the coal pillar, and the vertical stress is larger. When the interval coal pillar width b < b0, the coal pillar is affected by the concentrated stress, and failure occurs. The arch structure of the two working faces is connected. If the mining face width is small (a1), then the height of the arch in the adjacent mining of the working face is still less than the mining depth, that is, hm(a′) ≤ H. Furthermore, the height of the connected main arch does not exceed the ground, forming a single main arch + “V-shaped” beam structure, as shown in Figure 13a. When the width of the mining face is large (a2), and the arch height of the adjacent mining of the working face is greater than the mining depth, that is, hm(a′) > H, then the connected main rock arch exceeds the ground, and two vice rock arches are formed on both sides, forming a double vice arch + “U-shaped” beam structure, as shown in Figure 13b.
- For mining width a3, the overlying strata has a vice arch structure. When the width of the interval coal pillar b < b0, then the coal pillar is a failure, and the overlying strata structure on both sides of the working face is connected to form the structure of the double vice arch + “U-shaped” beam. At this time, there is no obvious stress concentration in the center of the goaf, and only several original rock stress recovery areas appear, as shown in Figure 13b. When the width of the interval coal pillar b ≥ b0, then the coal pillar is stable, separated from the goaf on both sides, and a double arch foot is formed above the coal pillar (the arch foot is shared when the coal pillar is small). There are vice arches on both sides of the coal wall, forming a structure of four vice arches + double “U-shaped” beams, as shown in Figure 13d.
7. Conclusions and Future Work
- Combined with the threshold of surface subsidence monitored using UAV and InSAR, a monitoring method based on “space–air–ground” network integration is proposed. The proposed method can make up for disadvantages such as the UAV not being able to effectively monitor the small deformation at the edge of the subsidence basin and the InSAR not being able to monitor the large deformation at the center of the subsidence basin. In addition, Internet of Things technology can fuse and aggregate the data. The overall mean square error of the InSAR and UAV fusion subsidence basin is 33.2 mm, and the mean square error of the discrete element numerical simulation and fusion subsidence results is 13.4 mm. Based on the monitoring network, a discrete element mechanical parameter inversion method was proposed.
- A discrete element numerical simulation model for adjacent mining in a thick coal seam working face was also established. The surface subsidence law of adjacent mining under different working face widths and interval coal pillar widths was revealed. The Bozeman function model of the surface subsidence ratio changing with the aspect ratio was inverted, . The calculation formula for the width reduction coefficient of the adjacent mining face was established, , and the critical failure width of the coal pillar was calculated as 20.5 m. Therefore, in order to ensure safety, the width of the interval coal pillar should be greater than this size when the adjacent mining face is laid in this study area.
- Based on the theory of arch and beam structure and the results of numerical simulations, the structural model of adjacent mining overlying strata with different mining widths and interval coal pillar widths in the mining area was constructed. These include a single main arch + “V-shaped” beam, double vice arch + “U-shaped” beam, double main arch + “W-shaped” beam, and four vice arch + double “U-shaped” beam.
Author Contributions
Funding
Conflicts of Interest
References
- Xu, Z.H.; Li, Q.S.; Li, X.B.; Zhang, G.J.; Yang, Y.L.; He, W.R.; Wu, X.Y. Structural evolution of overburden and surface damage caused by high-intensity mining with shallow depth. J. China Coal Soc. 2020, 45, 2728–2739. [Google Scholar]
- He, G.Q.; Yang, L.; Ling, G.D.; Jia, F.C.; Feng, D. Ming Subsidence Science; China University of Mining and Technology Press: Xuzhou, China, 1991. [Google Scholar]
- Li, S.S.; Ren, H.W. Research status and development trend of position and posture measurement technology on hydraulic support, scraper conveyor, shearer in fully-mechanized mining face. Coal Sci. Technol. 2020, 48, 218–226. [Google Scholar]
- Cho, S.; Bang, E.; Kang, I. Construction of precise digital terrain model for nonmetal open-pit mine by using unmanned aerial photograph. Econ. Environ. Geol. 2015, 48, 205–212. [Google Scholar] [CrossRef]
- Gong, C.G.; Bian, Z.F.; Bian, H.F.; Lei, S.G.; Huang, J.; Zhang, Z.A.; Guo, H.Q.; Zhang, H. Key technology of DEM model construction based on UAV and vegetation index in dump soil field. J. China Coal Soc. 2019, 44, 3849–3858. [Google Scholar]
- Cwiakala, P.; Gruszczynski, W.; Stoch, T.; Puniach, E.; Wójcik, A. UAV Applications for Determination of Land Deformations Caused by Underground Mining. Remote Sens. 2020, 12, 1733. [Google Scholar] [CrossRef]
- Ge, L.L.; Li, X.J.; Ng, A.H. UAV for mining applications: A case study at an open-cut mine and a longwall mine in New South Wales, Australia. In Proceedings of the 2016 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Beijing, China, 10–15 July 2016; pp. 5422–5425. [Google Scholar]
- Zhou, D.; Qi, L.; Zhang, D.; Zhou, B.; Guo, L. Unmanned Aerial Vehicle (UAV) Photogrammetry Technology for Dynamic Mining Subsidence Monitoring and Parameter Inversion: A Case Study in China. IEEE Access 2020, 8, 16372–16386. [Google Scholar]
- Gao, Y.G.; Zhou, D.W.; An, S.K.; Wang, L.; Zhang, D.M.; Zhan, S.Q. Study on surface subsidence in coal mining by UAV-photogrammetry monitoring technology. Coal Sci. Technol. 2022, 50, 57–65. [Google Scholar]
- Puniach, E.; Gruszczyński, W.; Ćwiąkała, P.; Matwij, W. Application of UAV-based orthomosaics for determination of horizontal displacement caused by underground mining. ISPRS J. Photogramm. Remote Sens. 2021, 174, 282–303. [Google Scholar] [CrossRef]
- Dai, S.; Wei, G.J.; Liang, B. Influence of control point number on UAV low-altitude photogrammetry and its application: A case study in subsidence monitoring of a tailing dam area in northwestern China. Chin. J. Geol. Hazard Control. 2021, 32, 113–120. [Google Scholar]
- Gabriel, A.K.; Goldstein, R.M.; Zebker, H.A. Mapping small elevation changes over large areas: Differential rader interferometry. J. Geophys. Res. 1989, 94, 9183–9191. [Google Scholar] [CrossRef]
- Carnec, C.; Massonnet, D.; King, C. Two examples of the use of SAR interferometry on displacement fields of small spatial extent. Geophys. Res. Lett. 1996, 23, 3579–3582. [Google Scholar] [CrossRef]
- Yang, Z.F.; Zhu, J.J.; Li, Z.W.; Wang, C.C.; Wang, Y.J.; Chen, G.L. Analysis of law of kinematic mining subsidence by integrating InSAR and leveling measurements. J. Cent. South Univ. 2015, 10, 3743–3751. [Google Scholar]
- Yang, Z.F. Retrieving and Predicting Mining-Induced 3-D Displacements from a Single Imaging Geometry InSAR Dataset. Geogr. Geo-Inf. Sci. 2018, 34, 125. [Google Scholar]
- Yang, Z.F.; Yi, H.W.; Zhu, J.J.; Li, Z.W.; Shu, J.M.; Liu, Q. Spatio-temporal evolution law analysis of whole mining subsidence basin based on InSAR-derived time-series deformation. Trans. Nonferrous Met. 2016, 26, 1515–1522. [Google Scholar]
- Zhang, W.; Zhang, W.S.; Zhang, D.S.; Hu, W.M.; Sun, Y.Y.; Tang, J.J. Monitoring technology of “space-surface” for activity laws of mining-induced overburden. J. China Univ. Min. Technol. 2018, 47, 1212–1223. [Google Scholar]
- Gao, Y.F. “Four-zone” model of rockmass movement and back analysis of dynamic displacement. J. China Coal Soc. 1996, 21, 51–56. [Google Scholar]
- Li, Q.S.; Zhang, Y.J.; Zhao, Y.Q.; Zhu, Y.H.; Yan, Y.G. Study on Stability Discrimination Technology of Stope Arch Structure. Sustainability 2022, 14, 17. [Google Scholar] [CrossRef]
- Wang, F.; Chen, T.; Ma, B.; Chen, D.H. Formation mechanism of stress arch during longwall mining based on key strata theory. Energy Explor. Exploit. 2022, 40, 2. [Google Scholar] [CrossRef]
- Song, Z.Q.; Liu, Y.X.; Chen, M.B.; Song, Y. Appearance of abutment pressure before and after rock beam fracture and its application. J. Shandong Min. Inst. 1984, 1, 27–39. [Google Scholar]
- Wang, J.C.; Xu, J.L.; Yang, S.L.; Wang, Z.H. Development of strata movement and its control in underground mining: In memory of 40 years of Voussoir Beam Theory proposed by Academician Minggao Qian. Coal Sci. Technol. 2023, 51, 80–94. [Google Scholar]
- Sun, Y.J.; Zuo, J.P.; Karakus, M.; Wang, J.T. Investigation of movement and damage of integral overburden during shallow coal seam mining. Int. J. Rock Mech. Min. Sci. 2019, 117, 63–75. [Google Scholar] [CrossRef]
- Wu, L.X.; Wang, J.Z.; Zhao, X.S. Strata and Surface Subsidence Control in Strip-Partial Mining Under. Buildings. J. China Univ. Min. Technol. 1994, 4, 74–85. [Google Scholar]
- Jiang, F.X. Viewpoint of Spatial Structures of Overlying Strata and Its Application in Coal Mine. J. Min. Saf. Eng. 2006, 1, 30–33. [Google Scholar]
- He, H. Research on the evolution mechanism of spatial structure of overlying strata and rock burst inducing in coal mine. J. China Coal Soc. 2012, 37, 1245–1246. [Google Scholar]
- He, H.; Dou, L.M.; Cao, A.Y.; Fan, J. Mechanisms of Mining Seismicity under Large Scale Exploitation with Multikey Strata. Shock. Vib. 2015, 2, 1–9. [Google Scholar] [CrossRef]
- Yang, H.Q.; Han, C.L.; Zhang, N.; Sun, C.; Dong, M. Stability Control of a Goaf-Side Roadway under the Mining Disturbance of an Adjacent Coal Working Face in an Underground Mine. Sustainability 2019, 11, 6398. [Google Scholar] [CrossRef]
- Yu, X.Y.; Wang, Z.S.; Yang, Y.; Mao, X.W. Numerical study on the movement rule of overburden in fully mechanized caving mining with thick depth and high mining height. J. Min. Strat. Control. Eng. 2020, 7, 1–11. [Google Scholar]
- Guilhot, D.; Hoyo, T.M.D.; Bartoli, A.; Ramakrishnan, P.; Leemans, G.; Houtepen, M.; Salzer, J.; Metzger, J.S.; Maknavicius, G. Internet-of-Things-Based Geotechnical Monitoring Boosted by Satellite InSAR Data. Remote Sens. 2021, 13, 2757. [Google Scholar] [CrossRef]
- Ojagh, S.; Cauteruccio, F.; Terracina, G.; Liang, S.H.L. Enhanced air quality prediction by edge-based spatiotemporal data preprocessing. Comput. Electr. Eng. 2021, 96, 107572. [Google Scholar] [CrossRef]
- Zeybek, M.; Şanlıoğlu, İ. Point cloud filtering on UAV based point cloud. Measurement 2019, 133, 99–111. [Google Scholar] [CrossRef]
- Serifoglu, Y.C.; Yilmaz, V.; Güngör, O. Investigating the performances of commercial and non-commercial software for ground filtering of UAV-based point clouds. Int. J. Remote Sens. 2018, 39, 5016–5042. [Google Scholar] [CrossRef]
- Baran, I.; Stewart, M.; Claessens, S. A new functional model for determining minimum and maximum detectable deformation gradient resolved by satellite radar interferometry. IEEE Trans. Geosci. Remote Sens. 2005, 4, 675–682. [Google Scholar] [CrossRef]
- Zhou, D.W.; An, S.K.; Wu, K.; Hu, Z.Q.; Diao, X.P. Key technology and application of InSAR/UAV fusion monitoring for coal mining damages. Coal Sci. Technol. 2022, 50, 121–134. [Google Scholar]
- Wang, L. Research on UDEC Numerical simulation of Upward mining in 42 Panel area of Wenjiapo mine. Coal Technol. 2021, 40, 23–25. [Google Scholar]
- Li, H.Z.; Zhao, B.C.; Guo, G.L.; Zha, J.F.; Bi, J.X. The influence of an abandoned goaf on surface subsidence in an adjacent working coal face: A prediction method. Bull. Eng. Geol. Environ. 2018, 77, 305–315. [Google Scholar] [CrossRef]
- Guo, W.B.; Deng, K.Z.; Zou, Y.F. Study on Failure and Instability of Strip Coal Pillar by Catastrophic Theory. J. China Univ. Min. Technol. 2005, 1, 80–84. [Google Scholar]
- Lou, J.F. Influence mechanism of beam-arch binary structure and strata characteristics on fracture and stress evolution of overlying strata in stope. J. Min. Saf. Eng. 2021, 38, 678–686. [Google Scholar]


| Data Source | Acquisition Date | Other Information |
|---|---|---|
| InSAR | 17 July 2018, 29 July 2018 10 August 2018, 22 August 2018 3 September 2018, 15 September 2018 27 September 2018, 9 October 2018 | Time baseline (12d) Imaging mode (IW) Spatial resolution (5 m × 20 m) |
| UAV | 19 July 2018 5 October 2018 | Sensor type (CMOS) Sensor size (23.5 × 15.6 mm (aps-c)) Spatial resolution (5 cm) |
| GNSS | 19 July 2018, 5 October 2018 | -- |
| Lithology | Thickness (m) | Density (kg·m−3) | Bulk Modulus (GPa) | Shear Modulus (GPa) | Cohesion (MPa) | Friction Angle (°) | Tensile Strength (MPa) |
|---|---|---|---|---|---|---|---|
| Aeolian sand | 10 | 1580 | 0.67 | 0.53 | 0.2 | 10 | 0.12 |
| Medium sandstone | 13 | 2200 | 5.30 | 4.80 | 2.0 | 25 | 1.80 |
| Fine sandstone | 40 | 2500 | 5.80 | 4.30 | 1.1 | 45 | 1.53 |
| Medium sandstone | 25 | 2200 | 5.30 | 4.80 | 2.0 | 25 | 1.80 |
| Fine sandstone | 15 | 2500 | 5.80 | 1.37 | 1.1 | 45 | 1.53 |
| Sandy mudstone | 88 | 2390 | 2.27 | 1.17 | 3.3 | 28 | 1.72 |
| Fine sandstone | 19 | 2500 | 5.80 | 4.30 | 1.1 | 45 | 1.53 |
| Sandy mudstone | 18 | 2390 | 2.27 | 1.17 | 3.3 | 28 | 1.72 |
| Fine sandstone | 7 | 2500 | 5.80 | 4.30 | 1.1 | 45 | 1.53 |
| Sandy mudstone | 19 | 2390 | 2.27 | 1.17 | 3.3 | 28 | 1.72 |
| Fine sandstone | 11 | 2500 | 5.80 | 4.30 | 1.1 | 45 | 1.53 |
| Sandy mudstone | 30 | 2390 | 2.27 | 1.17 | 3.3 | 28 | 1.72 |
| Medium sandstone | 20 | 2200 | 5.30 | 4.80 | 2.0 | 25 | 1.80 |
| Fine sandstone | 64 | 2500 | 5.80 | 4.30 | 1.1 | 45 | 1.53 |
| Medium sandstone | 15 | 2200 | 5.30 | 4.80 | 2.0 | 25 | 1.80 |
| Fine sandstone | 12 | 2500 | 5.80 | 4.30 | 1.1 | 45 | 1.53 |
| Siltstone | 8.8 | 2400 | 3.00 | 3.97 | 3.8 | 43 | 1.50 |
| 3-1coal | 5.2 | 1480 | 1.33 | 5.62 | 0.9 | 35 | 0.72 |
| Sandy mudstone | 30 | 2390 | 2.27 | 1.17 | 3.3 | 28 | 1.72 |
| Width of Working Face | Width of Interval Coal Pillar | Sufficiency State of Single Working Face | Coal Pillar Stability State | Number of Peak Stress | |
|---|---|---|---|---|---|
| Position of Interval Coal Pillar | Goaf | ||||
| a1 = 150 m | b = 15 m | Insufficient | Instability | 0 | 0 |
| b = 30 m | Insufficient | Stability | 1 | 0 | |
| b = 50 m, 70 m, 90 m, 110 m | Insufficient | Stability | 2 | 0 | |
| a2 = 200, 250, 300 m | b = 15 m | Insufficient | Instability | 0 | 2 |
| b = 30 m, 50 m | Insufficient | Stability | 1 | 0 | |
| b = 70 m, 90 m, 110 m | Insufficient | Stability | 2 | 0 | |
| a3 = 400 m | b = 15 m | Sufficient | Instability | 0 | 2 |
| b = 30 m, 50 m, 70 m | Sufficient | Stability | 1 | 4 | |
| b = 90 m, 110 m | Sufficient | Stability | 2 | 4 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Y.; Yan, Y.; Zhang, Y.; Zhang, W.; Kong, J.; Dai, A. Study on the Evolution Law of Overlying Strata Structure in Stope Based on “Space–Air–Ground” Integrated Monitoring Network and Discrete Element. Drones 2023, 7, 309. https://doi.org/10.3390/drones7050309
Zhu Y, Yan Y, Zhang Y, Zhang W, Kong J, Dai A. Study on the Evolution Law of Overlying Strata Structure in Stope Based on “Space–Air–Ground” Integrated Monitoring Network and Discrete Element. Drones. 2023; 7(5):309. https://doi.org/10.3390/drones7050309
Chicago/Turabian StyleZhu, Yuanhao, Yueguan Yan, Yanjun Zhang, Wanqiu Zhang, Jiayuan Kong, and Anjin Dai. 2023. "Study on the Evolution Law of Overlying Strata Structure in Stope Based on “Space–Air–Ground” Integrated Monitoring Network and Discrete Element" Drones 7, no. 5: 309. https://doi.org/10.3390/drones7050309
APA StyleZhu, Y., Yan, Y., Zhang, Y., Zhang, W., Kong, J., & Dai, A. (2023). Study on the Evolution Law of Overlying Strata Structure in Stope Based on “Space–Air–Ground” Integrated Monitoring Network and Discrete Element. Drones, 7(5), 309. https://doi.org/10.3390/drones7050309








