Tilted Triangular Springs with Constant-Force Reaction †
Abstract
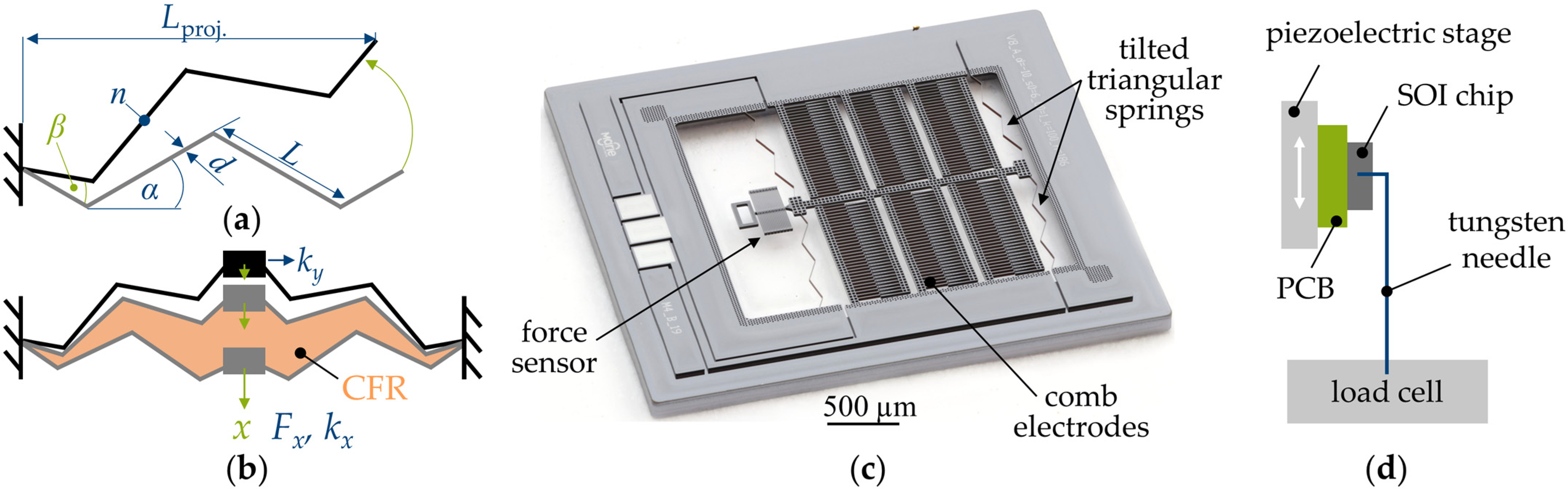
1. Introduction
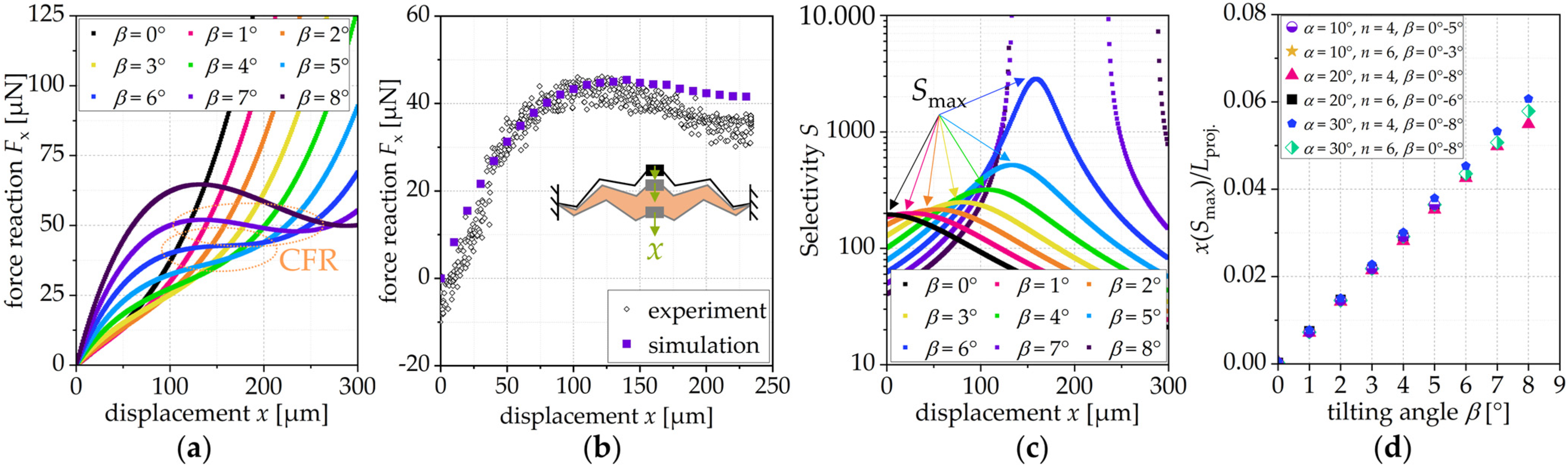
2. Materials and Methods
3. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, S.; Xu, Q. Design and simulation of a passive-type constant-force MEMS microgripper. In Proceedings of the 2017 IEEE International Conference on Robotics and Biomimetics (ROBIO), Macau, China, 5–8 December 2017; pp. 1100–1105. [Google Scholar]
- Wang, P.; Xu, Q. Design and modeling of constant-force mechanisms: A survey. Mech. Mach. Theory 2018, 119, 1–21. [Google Scholar] [CrossRef]
- Legtenberg, R.; Groeneveld, A.W.; Elwenspoek, M. Comb-drive actuators for large displacements. J. Micromech. Microeng. 1996, 6, 320. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schmitt, L.; Schmitt, P.; Hoffmann, M. Tilted Triangular Springs with Constant-Force Reaction. Proceedings 2024, 97, 222. https://doi.org/10.3390/proceedings2024097222
Schmitt L, Schmitt P, Hoffmann M. Tilted Triangular Springs with Constant-Force Reaction. Proceedings. 2024; 97(1):222. https://doi.org/10.3390/proceedings2024097222
Chicago/Turabian StyleSchmitt, Lisa, Philip Schmitt, and Martin Hoffmann. 2024. "Tilted Triangular Springs with Constant-Force Reaction" Proceedings 97, no. 1: 222. https://doi.org/10.3390/proceedings2024097222
APA StyleSchmitt, L., Schmitt, P., & Hoffmann, M. (2024). Tilted Triangular Springs with Constant-Force Reaction. Proceedings, 97(1), 222. https://doi.org/10.3390/proceedings2024097222





