Optimal Sizing of RF Integrated Inductors for Power Transfer of Implantable Biosensors ^†

Abstract

Energy recovery methods are currently receiving a very great deal of attention from the research community. Especially, in the case of implantable biosensors where wireless energy transfer has become the main technique in these applications. An implant is a medical device manufactured to replace a missing biological structure, support a damaged biological structure, or enhance an existing biological structure. Biosensors are man-made devices, in contrast to a transplant, which is a transplanted biomedical tissue. The method of energy transfer eliminates the risk of skin infection, as well as the need for invasive surgery to change the battery. In this paper, we present the efficient approach to design an optimized octagonal spiral inductor operating at a frequency of 2.4 GHz with an inductance L value of 4 nH and a maximum factor of quality Q. The principle part of this work is based on the use of a collection of methods called metaheuristics, which are approaches used to solve a wide range of optimization problems, in order to achieve a high-performance optimized design. The problem is represented by an objective function that will be implemented using a MATLAB script and then the validation of the results obtained will be performed using the advanced design system (ADS) microwave circuit simulation software.

1. Introduction

Nowadays, a lot of research is focused on the realization of integrated inductors to meet the needs and more operational for various applications: in signal processing to enter the realization of low-noise amplifiers [1], in biomedical engineering [2] including applications related to energy storage [3]. The design of such components requires specific techniques and mainly the use of three types of materials: a conductive material, a magnetic material, which in most cases is ferrite, iron-silicon alloys or nickel-silicon alloys in the case of inductance with a core and sometimes an insulator. The performance of the component depends on several factors, on the one hand on the magnetic properties of the material [4], if it is a low-frequency application we generally find magnetic conductive materials with high permeability.

In this paper the objective will be the design and optimization of an integrated inductor in complementary metal oxide semi-conductor (CMOS) technology for biosensors using the differential evolution algorithm (DE). The study consists of using the differential evolution algorithm to design a planar integrated inductor of the octagonal spiral type operating at a frequency around 2.4 GHz with a maximum quality factor and an inductance L which tends towards 4 nH, taking into account the different electrical and magnetic phenomena (the skin effect, the proximity effect…), which are reproduced in such structures.

2. Materials and Methods

2.1. Physical Model of Spiral Inductor

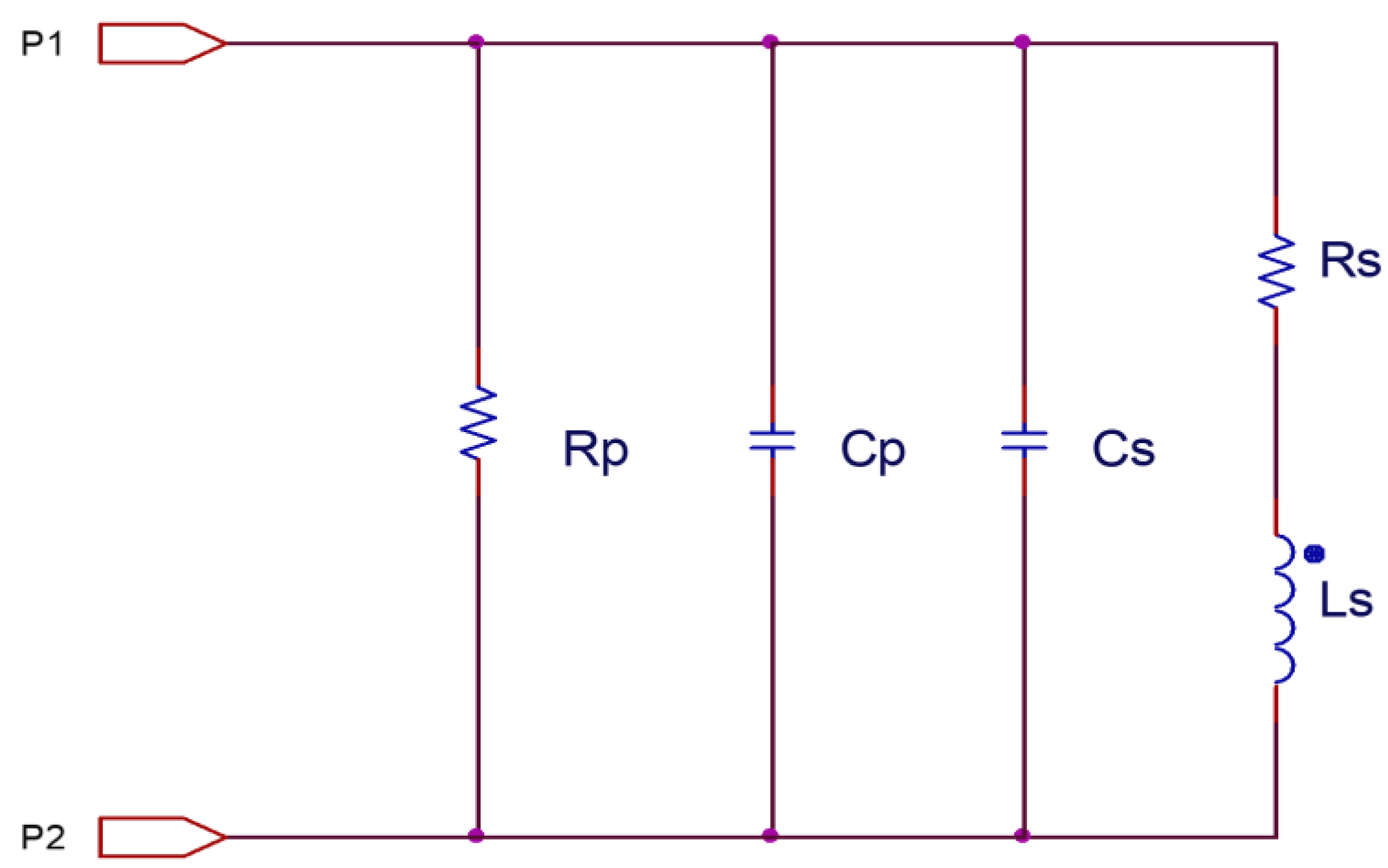
2.1.1. Equivalent Model

We have considered the equivalent circuit shown in Figure 1 to calculate the inductance value by the following equation:

$$L_S=-\frac{1}{2\pi f}Im(\frac{1}{Y_{12}})$$

(1)

The parameters of the physical model are calculated according to the geometrical parameters of the inductance by the following equations [5,6]:

• Coupling capacity between the coils:

$$C_s=n·w^2·\frac{{\epsilon }_{ox}}{t_{oxM1-M2}}$$

• Parasitic capacitance:

$$C_{ox}=\frac{1}{2}·l·w·\frac{{\epsilon }_{ox}}{t_{ox}}$$

• Capacity of substrates:

$$C_{si}=\frac{1}{2}·l·w· C_{sub}$$

• Resistance of the substrate:

$$R_{si}=\frac{2}{l·w·G_{sub}}$$

2.1.2. Inductance L_s

Several methods are presented in the literature to calculate the Ls such as wheeler’s method [7], Baryan’s method [8], Terman’s method, monomial method and others. In our simulation we will use the monomial method because it remains valid for a small number of turns. The monomial expression used to calculate the self-inductance is given by the following relation:

$$Ls=\beta ·D_{out}^{{}_{\mathsf{\alpha }1}}·W^{{}_{\mathsf{\alpha }2}}·D_{avg}^{{}_{\mathsf{\alpha }3}}· N^{{}_{\mathsf{\alpha }4}}· S^{{}_{\mathsf{\alpha }5}}$$

N is the number of turns, β, α1, α2, α3, α4 and α5 are coefficients according to the geometrical shape of the inductance studied, D_avg is calculated from inner diameter D_in of the inductance outer diameter D_out by the following relation:

$$\begin{gathered} D_{avg}=\frac{\left(D_{in}+D_{out}\right)}{2} \\ {D}_{out}=D_{in}+2·N·W+2·\left(N-1\right)·S \end{gathered}$$

The coefficients β, α1, α2, α3, α4 and α5 are given as follows in the

Table 1. Table of coefficients used in the monomial expression.

Geometry	β	α1	α2	α3	α4	α5
Octagonal	$1.33\times {10}^{-3}$	−1.21	−0.163	2.43	1.75	−0.049

:

$1.33\times {10}^{-3}$

2.1.3. Factor of Quality-Q

To calculate the quality factor Q, a parallel equivalent schema of the spiral inductance is proposed, this scheme is characterized by the parallel capacitance Cp and parallel resistance Rp. The Figure 2 below shows the proposed parallel model.

The quality factor is defined in the following equation as follows:

$$Q=\frac{\omega L_S}{R_S}·\frac{R_P}{R_P+\left[{\left(\raisebox{1ex}{$\omega L_S$}\!\left/ \!\raisebox{-1ex}{$R_S$}\right.\right)}^2+1\right]R_S}·\left[1-\frac{\left(C_P+C_S\right)R_S{}^2}{L_S}-{\omega }^2{L}_S\left(C_P+C_S\right)\right]$$

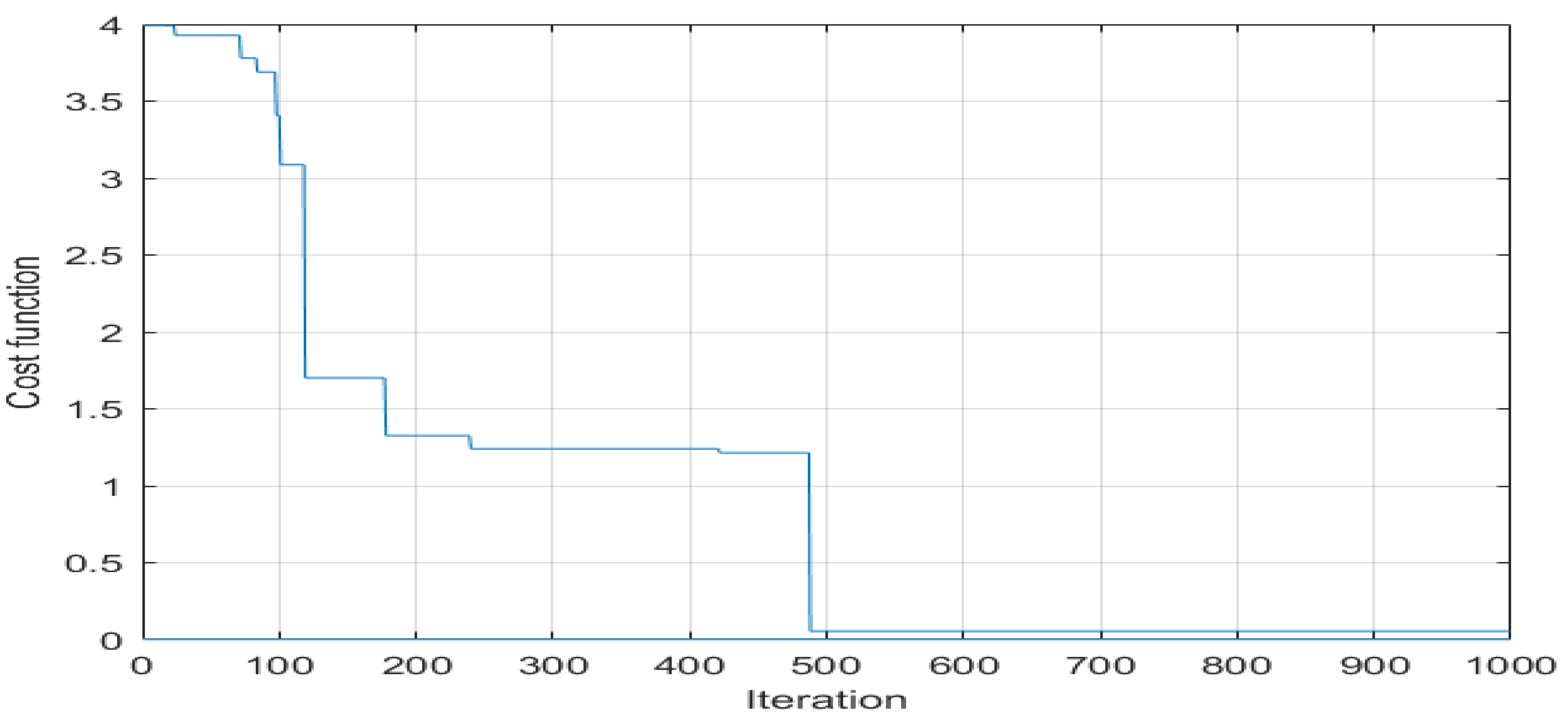
2.2. Differential Evolutionary Algorithm (DE)

Differential evolutions, or DE, has been proposed by Storn and Kenneth Price [9]. It is an algorithm inspired by genetics and evolutionary strategy, it is also based on the three operations: mutation, crossover and selection. The mutation generates new elements from certain elements randomly chooses then the crossing between the parents and the created elements and a selection operation to have only the most suitable elements in the generation.

Objective Function

We are looking to maximize the quality factor Q expressed in equation x. This equation will be considered as an objective function (OF), this amount to:

• Maximize the quality factor Q which depends on the geometrical parameters of the inductor (N, W, S);
• We will introduce the cost function Qreq = 1/Q, it represents the desired quality factor, so the problem is transformed towards a minimization of Qreq, in order to maximize the quality factor Q;
• All this will be done by the following constraints that must be satisfied:

2 ≤ N ≤ 10

20 ≤ W ≤ 100

3 ≤ S ≤ 50

3. Results

At 1000 iterations, after satisfying the stopping condition the algorithm provides the best combination of geometrical parameters corresponding to the optimal quality factor Q, the Figure 3 shows the result of the inductance optimization by the DE method. Figure 4 shows the momentum-ADS simulation results Q using the obtained optimal values by the DE-based method

The

Table 2. Parameters given by the DE algorithm.

S (µm)	w (µm)	N	Dout (µm)	Lsreq (nH)	LDE (nH)	QDE
4.56	13.62	3.21	223	4	3.95	13.46

shows the optimal parameters given by the DE algorithm:

4. Discussion

The simulation has shown very clearly that an octagonal inductance can be designed with the desired criteria with a very high percentage, in fact we have obtained a fairly high-quality factor, and the simulation value is close to the value provided by the DE algorithm, with an error that does not exceed 8%.