Effect of Rest Periods on Mechanical Ageing of Running Shoes ^†

Abstract

Running is a popular form of exercise, although runners are prone to injury from repeated impact. Running shoes can limit impact forces, but they deteriorate with use. Mechanical ageing typically involves repeatedly compressing the midsole while measuring the energy absorbed within compression cycles to assess degradation. Literature suggests mechanical aging often causes a higher rate of degradation than natural ageing. This work investigated the effect of introducing rest periods into mechanical ageing. Five shoes were mechanically aged using a sine plus dwell waveform (1.25 Hz, max. load 1.5 kN) for seven hours, equating to a simulated distance of 60 km. Three of the shoes were rested for 22 h every 20 km. The shoes aged with rest periods absorbed more energy than their unrested counterparts for the first 10 km when testing recommenced. This finding has implications for the mechanical ageing, design and recommended lifespan of running shoes.

1. Introduction

Close to a third of adults in England are thought to be obese, costing the National Health Service millions of pounds each year [1]. The UK government wants people to exercise more, as the obesity epidemic is in part due to low levels of physical activity [2]. Running is a popular form of exercise that is thought to be well suited to getting people more active, with parkrun UK claiming >1.5 million have participated in their events [3]. Poorly conditioned recreational runners are, however, prone to ‘overuse injuries’ from repeated impact at foot strike [4,5], which could act as a barrier to participation and weight loss. Most runners wear running shoes to reduce the risk of injury, but degradation of shoes with use [4] can reduce their ability to limit impact forces [6] and increase injury risk [7]. Running shoes can also affect muscle activity, influencing fatigue, comfort, work, performance [8] and the running economy [9].

Mechanical ageing is a technique used to impose and measure shoe degradation by repeatedly compressing the midsole in a test machine to mimic running [4,10,11,12,13,14,15,16]. The midsole is compressed many times, and the energy absorbed within a loading cycle is compared at set intervals related to running distances. Midsoles are typically made from closed cell foam such as Ethylene Vinyl Acetate (EVA) [17] or manufacturer-specific materials in high-end running shoes [9], and repeated buckling of cellular walls under cyclic compression can lead to gas loss and increased temperature, rendering the shoe less effective at absorbing energy. There is uncertainty as to how well mechanical ageing mimics running induced or ‘natural’ ageing. The literature suggests mechanical ageing is often, but not always [15], too ‘aggressive’ and gives more pronounced degradation than natural ageing [4,6], so there is scope for more representative testing protocols.

Recent work on mechanical ageing of shoes has used a dwell plus sine rather than the traditional continuous sine waveform [11,12,13,14] to factor in the time between foot strikes or ‘float phase’ of running. The short dwell periods (~0.5 s) allow the foam midsole to slightly recover and give less pronounced degradation that is more in line with natural ageing. These mechanical ageing protocols, however, often simulate long running distances in one test (e.g., marathon or further), whereas recreational runners tend to cover shorter distances on a given run (e.g., 5 km parkrun), with the shoes accumulating miles with each outing. As EVA can recover when rested [17], the aim of this work was to investigate the effect of introducing ‘rest periods’ into running shoe mechanical ageing protocols to simulate breaks between runs.

2. Materials and Methods

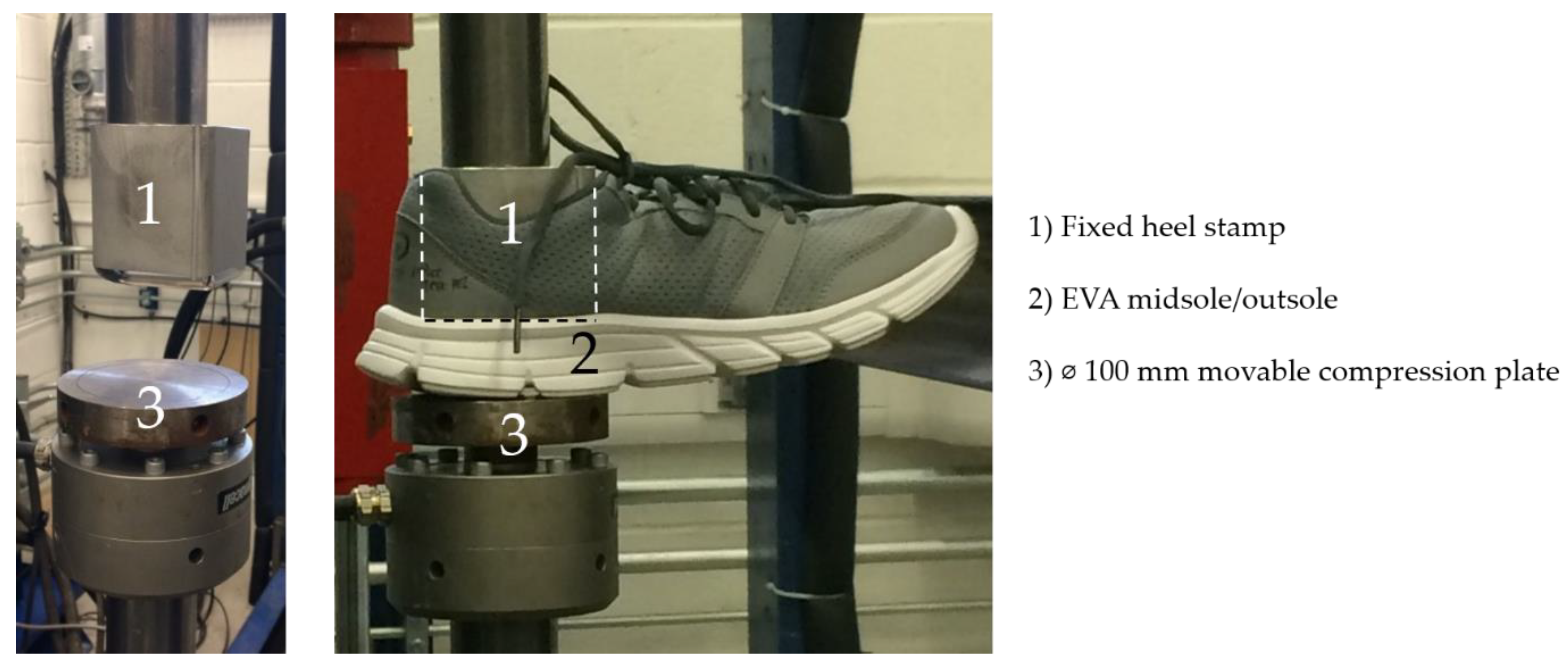
Three pairs of low cost, entry level running shoes (Kalenji Run 100 Men’s, Decathlon, size 8) were used in this research. The shoes had a one-piece EVA (stated in manufacturer specifications) midsole/outsole (~30 mm thick at heel region) and did not have a rubber outsole, as found on high-end shoes for improved grip and protection of the midsole [17]. One shoe was used for pilot testing, and results are presented for the other five. The shoes were mechanically aged in a servo hydraulic test device (Instron, fitted with 5 kN load cell and sampling at 1 kHz) to simulate heel strike running (Figure 1). The CNC machined aluminum heel stamp combined a rectangular and semi-circular section to give a basic representation of a human heel.

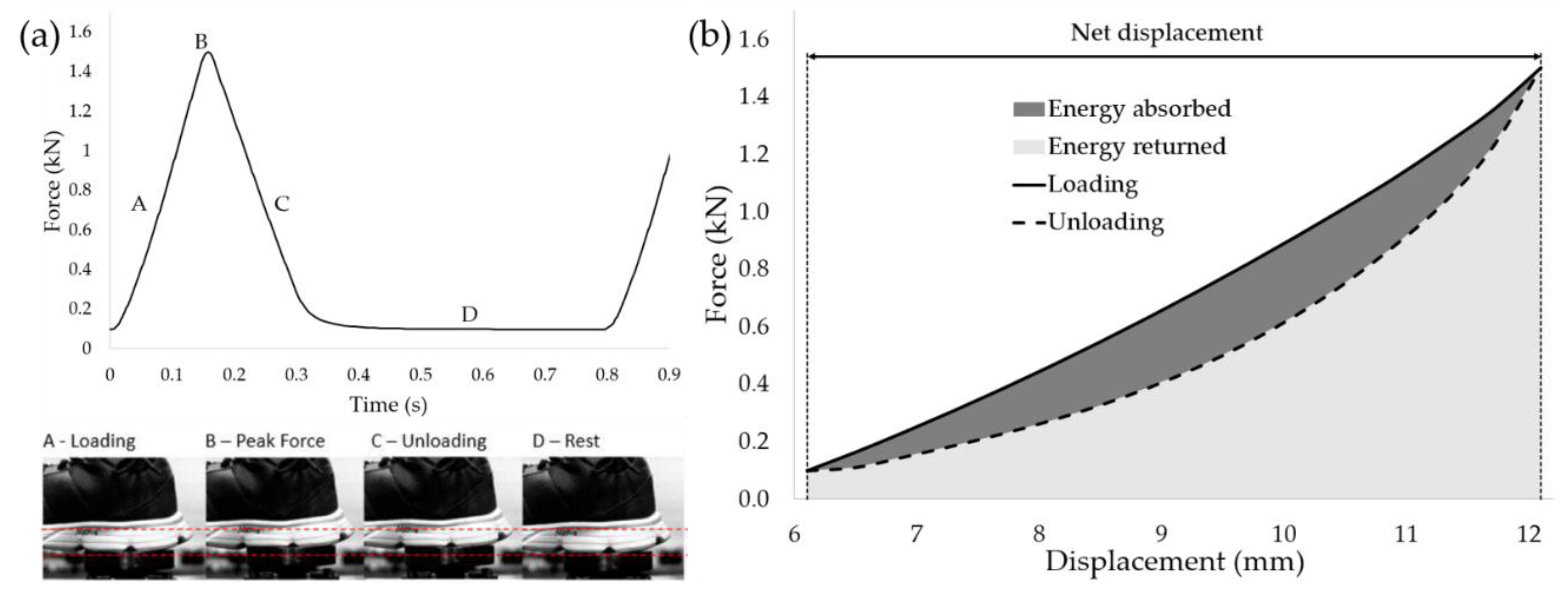
A sine plus dwell waveform was used to age the shoes (Figure 2a, Sine: loading to 1.5 kN at 10 kN/s, 0.01 s pause, unloading at 10 kN/s; dwell: 0.5 s pause), based on the work of Lippa et al. [7]. The sine wave represents the loading and unloading of the heel region of the midsole during foot strike, with the dwell representing the float phase. The maximum load of 1.5 kN was selected based on the values (~1.4 kN) used by Lippa and colleagues [11,12,13] and was assumed to approximately represent twice the body weight [17] of a typical male (~70–80 kg). A load of 100 N was applied at the start of the test and maintained during the 0.5 s dwell periods to hold the shoe in place, so the maximum load applied during each cycle was 1.4 kN.

Stride frequency was set at 1.25 Hz, giving a stride time of 0.8 s (Figure 2a). Assuming a step length (half the stride length) of 1 m (simulated speed of 2.5 m/s) [13], every 1000 cycles equated to a running distance of 2 km. The total simulated running distance was 60 km (30,000 cycles, ~7 h testing), and three of the shoes were rested for ~22 h (in device without load) every 20 km (10,000 cycles, ~2 h testing) to investigate the effect of adding rest periods to the mechanical ageing test. At 30,000, the total number of cycles for these tests was lower than other studies e.g., [15,16], but was considered sufficient to investigate the effect of rest periods. Cycles were selectively recorded with decreasing sampling frequency (i.e., increased spacing) as the rate of shoe degradation is known to decrease with simulated distance [4,10,11,12,13,14]. Every tenth cycle was recorded between one and 100 cycles, then recordings were made every 100 cycles up to 1000 cycles and then every 500 cycles. Recordings were made in groups of five cycles (e.g., cycle 998, 999, 1000, 1001, 1002) and used to obtain the mean energy absorbed at a given distance (e.g., 2 km) [13].

Data was exported to Microsoft Excel, and the energy absorbed by the shoe in a given loading cycle was calculated as the area within the force vs. displacement hysteresis loop (Figure 2b) using the trapezium rule. The energy in the hysteresis loop was normalised to the energy under the loading curve to account for any inconsistencies in the loading profile. The net displacement of the midsole during a loading cycle was calculated by subtracting the minimum (initial) from the maximum displacement [11,12,13,14] (Figure 2b). The energy absorbed by the shoe and the net displacement were calculated at the start of the test (cycles 10, 20, 30, 40, 50), at 400 m (cycles 198–202), 1 and 2 km (cycles 498–502 and 998–1002), 5 km (cycles 2498–2502) and then every 5 km (every 2500 cycles). For the shoes tested with rest periods, these distances where loading cycles were analysed were reset when testing recommenced to capture any changes shortly after the rest period. The first loading cycle was not included in the results, as observations of previous work [10,11,12,13,14] indicate its force-displacement curve is different from the others, with considerably more hysteresis.

3. Results

For shoes tested without rest periods, the energy absorbed decreased with the number of loading cycles from ~35% to 25% with a decreasing rate of change, as expected [4,11,12,13,14] (Figure 3a). Shoes tested with rest periods showed similar energy absorption to those tested without rest periods in the first ageing period (to 10,000 cycles) (Figure 2a). When testing restarted after each rest period the energy absorbed by the shoes returned to values comparable to those at the start of the test (30 cycles), and then decreased in a similar manner to the first ageing period. Clear differences can be observed in the energy absorbed by different shoes (~2% to 4%), indicating an inconsistency in the samples, with smaller variation for a test on a given shoe (standard deviation (S.D.) across five cycles < 0.25% of energy under loading curve).

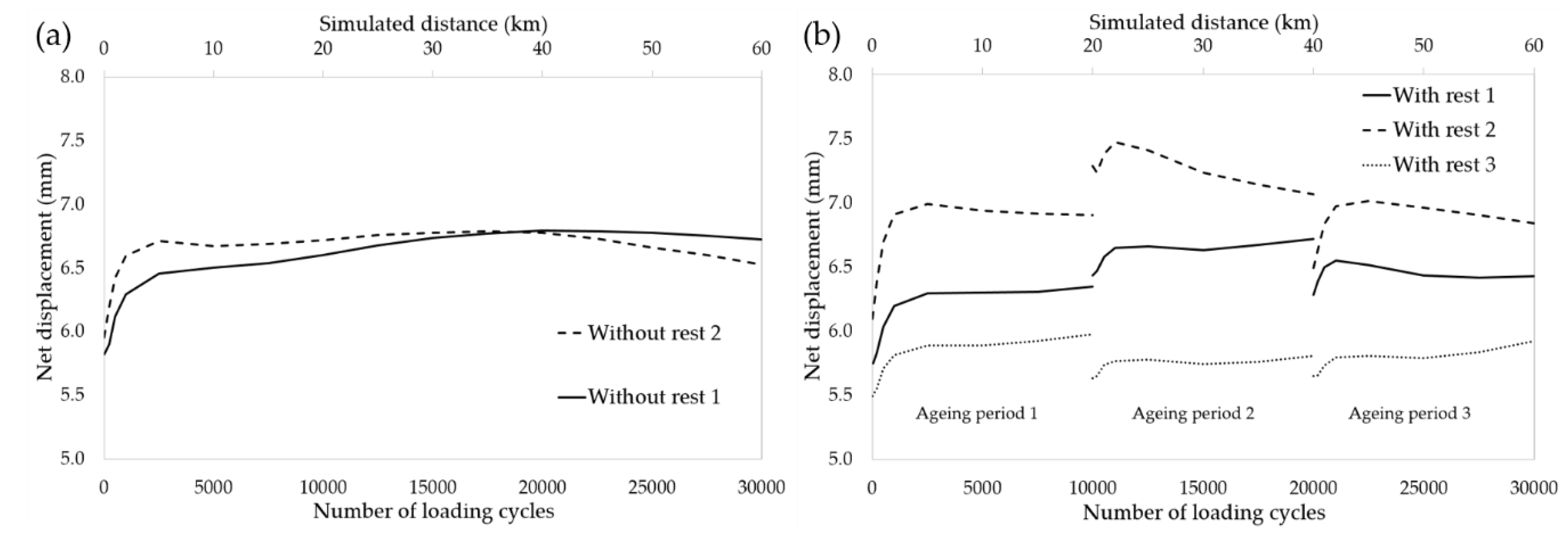
For shoes tested without rest periods, the net displacement of the shoes increased with the number of loading cycles from ~5.8 to 6.8 mm, with most of the change towards the start of the test (Figure 4a). Shoes tested with rest periods showed similar results to those without rest periods for net displacement in the first ageing period (Figure 4b). When testing recommenced after each rest period, the net displacement mimicked the trend of the first ageing period, with inconsistency as to whether the value at the start of the new ageing period was higher or lower than the value before the rest period. For four out of six cases, the initial value for net displacement after a rest period was lower than the value before the rest period. Differences can be observed between shoes for net displacement (up to ~2 mm), further indicating an inconsistency in the samples, with less variation for a test on any given shoe (S.D. across five cycles < 0.015 mm).

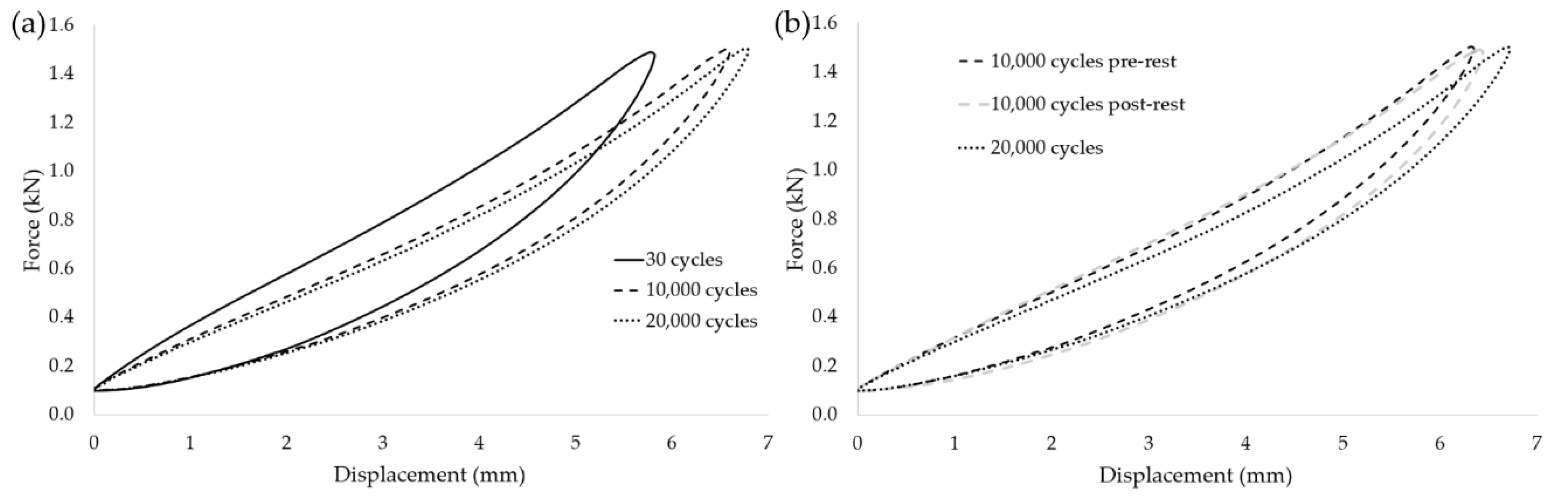
Figure 5 shows force vs. displacement curves from the ageing tests, with displacement at the start of each cycle set to zero (displacement at the start of each cycle increases with the number of cycles [11,12,13,14]) to facilitate visual comparison. For shoes tested without rest periods (Figure 5a), the net displacement increased with the number of loading cycles (corresponding to a reduction in stiffness), while the size of the hysteresis loop decreased. The difference between cycle 30 and 10,000 was clearly larger than the difference between cycle 10,000 and 20,000, as expected from Figure 3a. When comparing before and after the first rest period at 10,000 cycles (Figure 5b), in this instance the loading curves were similar but the unloading curve took a lower path after the rest period, accounting for the increased energy absorbed in Figure 3b. Prior to the next rest period at 20,000 cycles, the net displacement had increased while the size of the hysteresis loop decreased in a similar manner to the results reported in Figure 5a for 20,000 cycles.

4. Discussion

Introducing rest periods into the mechanical ageing of running shoes influenced results for energy absorption and net displacement. Shoes tested without rest periods behaved as expected [4,11,12,13,14], with the amount of energy absorbed and the net displacement changing with the number of loading cycles, with the largest changes within the first 5000 cycles. Shoes ‘recovered’ during 22-h rest periods after 10,000 and 20,000 loading cycles, initially absorbing a similar amount of energy to that at the start of the test (30 cycles) and then decreasing in a manner mimicking the first ageing period. By the end of the ageing test at 60,000 cycles (30 km), shoes tested with and without rest periods presented similar results for energy absorbed and net displacement. Clear differences were, however, observed between the shoes throughout the ageing tests (up to ~4% energy absorbed, up to ~2 mm net displacement), indicating an inconsistency in the samples.

The results may partially explain discrepancies reported between mechanical and natural ageing, where mechanical ageing is generally, but not always [15], considered to be too aggressive, resulting in more pronounced degradation [4,6]. When comparing naturally and mechanically aged shoes in a mechanical test (e.g., energy absorbed in loading cycle, impact performance as per American Society for Testing and Materials (ASTM) F1614), any delay between the participant completing their run and the mechanical test (i.e., a rest period) and any associated shoe recovery may need to be accounted for. Equally, any delays between mechanical ageing and participant testing may need to be accounted for when investigating how mechanical (or natural) ageing affects running biomechanics [14]. Future work could investigate the effect of the duration and location of rest periods within a mechanical ageing test for different shoe designs, loading profiles and longer total simulated distances that are more in line with those used by others [15,16], with loading at both the heel and forefoot to determine if the results are widely applicable. As mechanical ageing is often more aggressive than natural ageing [4,6], future work could look to determine whether rest periods affect natural ageing across a range of shoe designs.

The energy absorbed by the shoes ranged from ~1.3 to 1.6 J, which is within values presented by others during mechanical ageing (~0.5 to 2.5 J) [12,13,14]. In contrast to previous work, the net displacement of the shoes increased with the number of loading cycles. Discrepancies between studies could be due to differences in the profile and location (i.e., heel or forefoot) of the applied load, and the design (i.e., midsole material and thickness) of the shoes. While the shoes tested here were of a low cost, entry level design, the percentage of energy absorbed in a loading cycle was similar to values reported for high-end running shoes (~25% to 35% for 2 kN loading cycle) used for less than 50 km of running, but lower than a prototype shoe with a thick, compliant midsole (13%) [9]. The midsole of the prototype shoe deformed around twice as much as its thinner, stiffer counterparts (~12 vs. 6 mm at 2 kN), allowing it to store more energy, and then returned over twice as much energy due to its higher resilience [9]. Previous work has shown compliant midsoles to degrade faster than their stiffer counterparts [12]. The results indicate that the properties of entry level shoes could change within 20 km runs, influencing loading to the wearer’s lower limbs [14], with implications on performance and injury risk [7]. Future work could look to determine if the findings are applicable to other running shoes, particularly those at the high-end. Monitoring midsole foam thickness, temperature and cellular structure [17] after a period of mechanical ageing could further our understanding of how midsole foam may change and recover its properties, with implications for shoe design and material selection.

5. Conclusions

The energy absorbed by running shoes mechanically aged without rest periods decreased most rapidly in the first 5000 loading cycles (10 km). Shoes mechanically aged with rest periods partially recovered, absorbing more energy than their unrested counterparts for around the first 5000 loading cycles when testing restarted. This finding has implications for the mechanical ageing, design and recommended lifespan of entry level running shoes.