Graph Entropy Associated with Multilevel Atomic Excitation †
Abstract
:1. Introduction
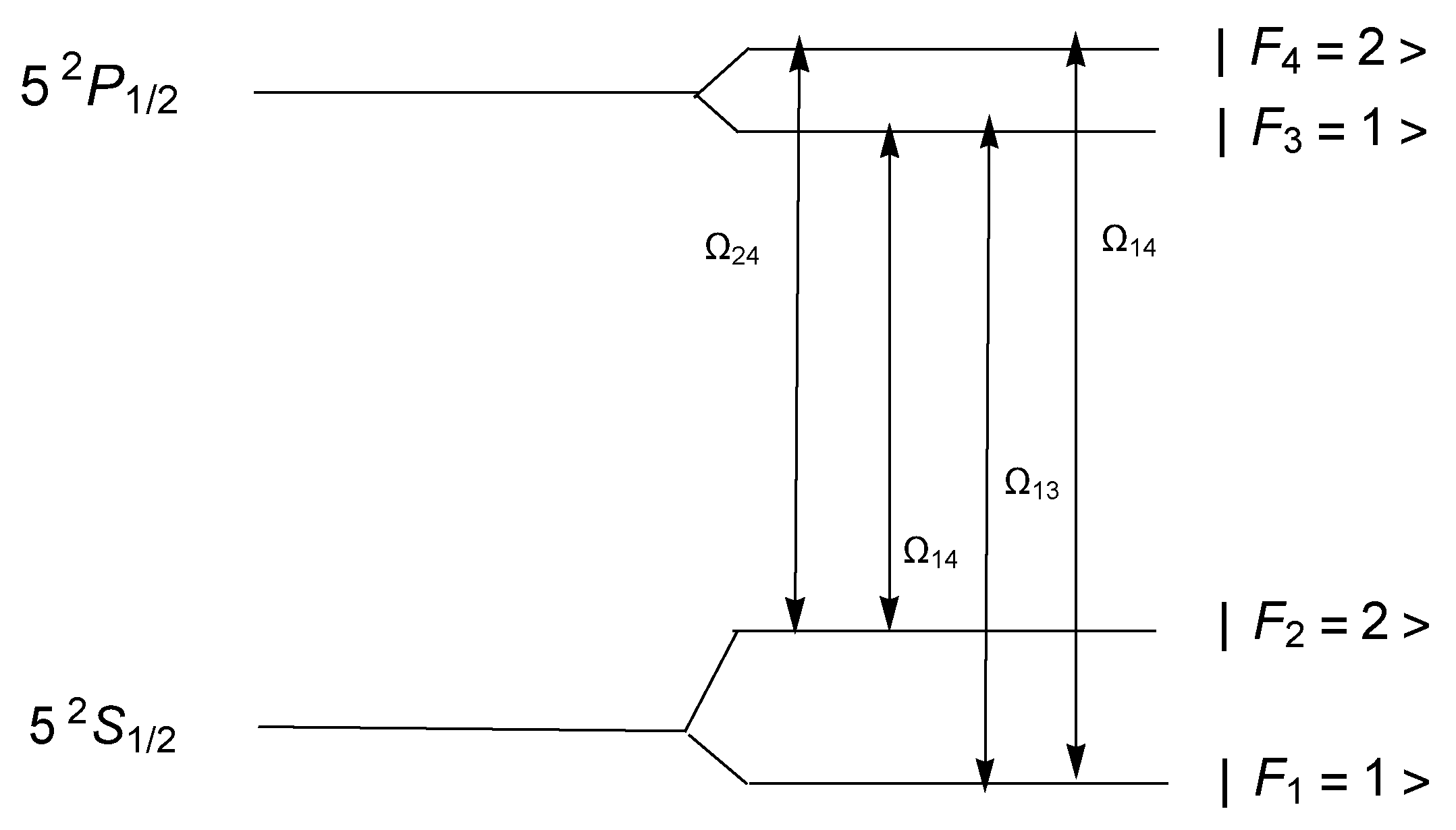
2. Theoretical Description
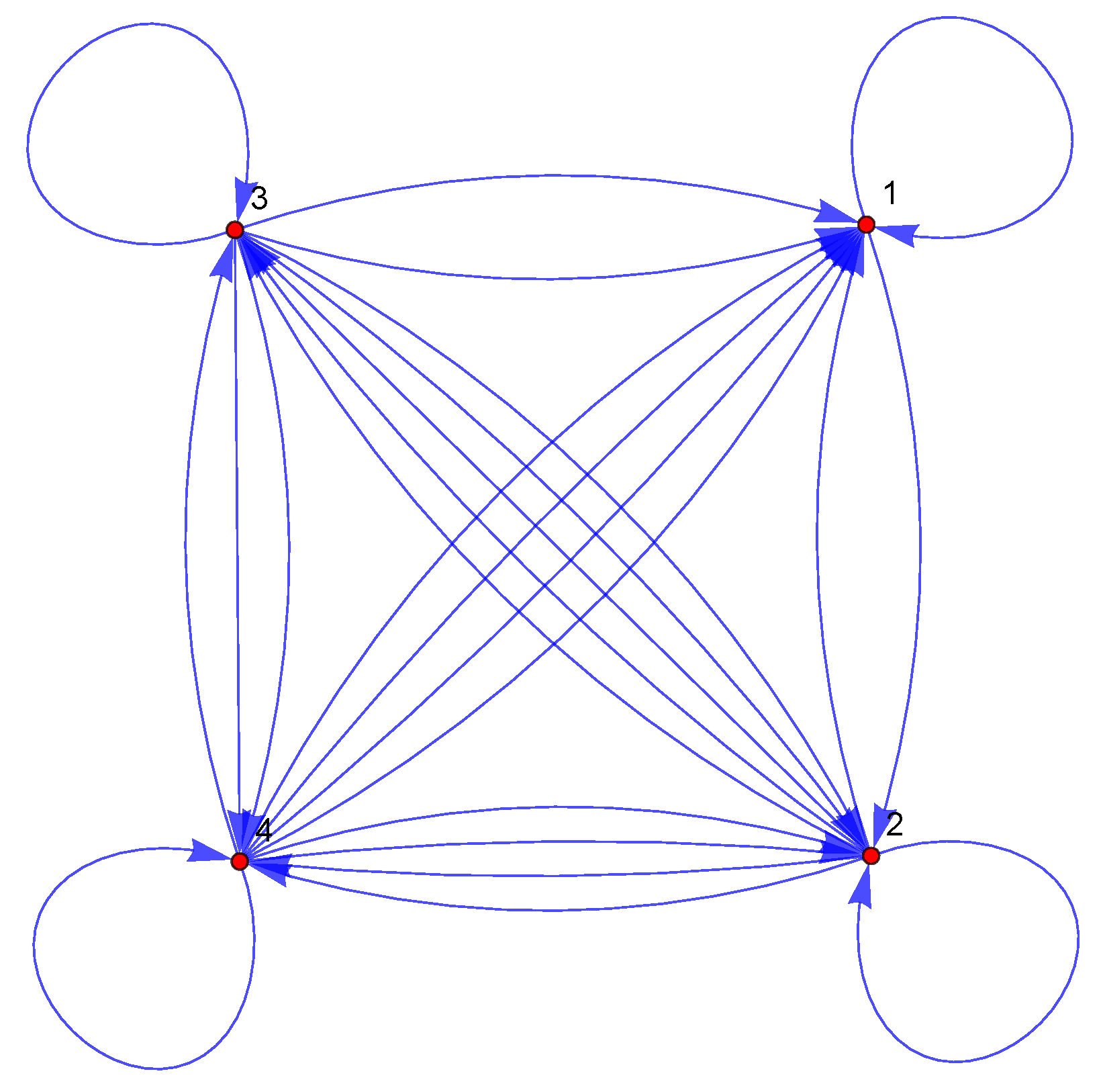
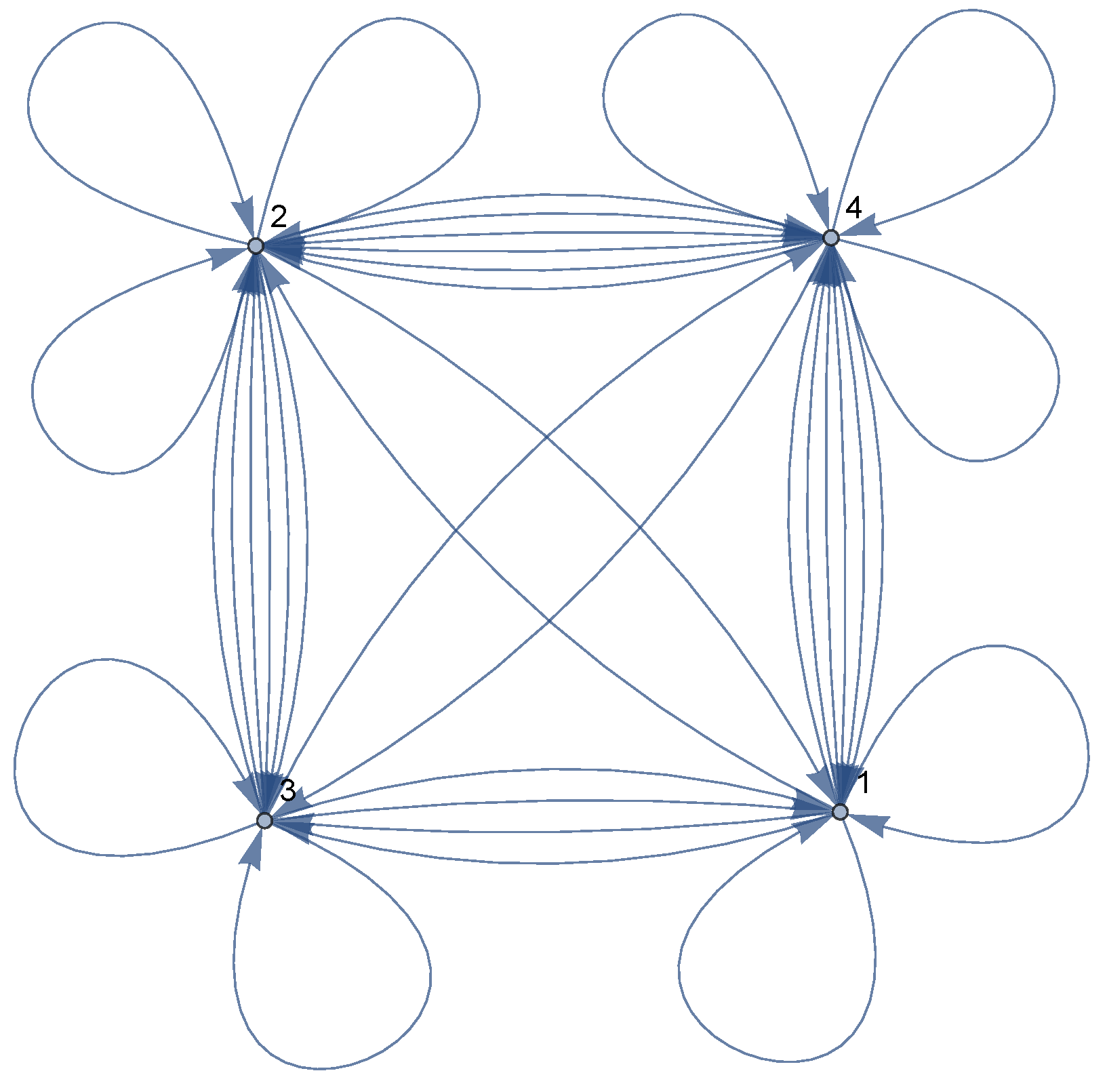
3. Graph Description
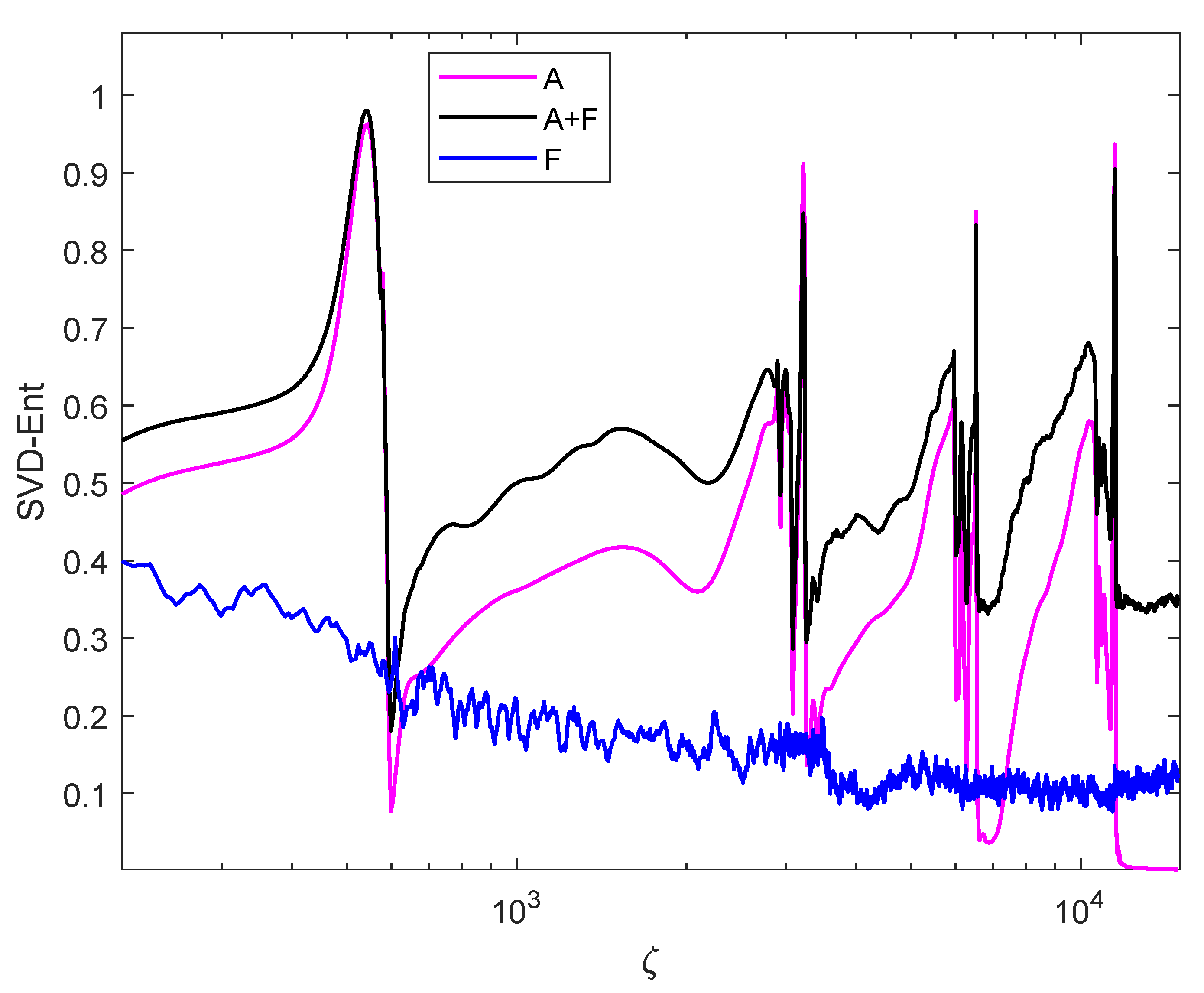
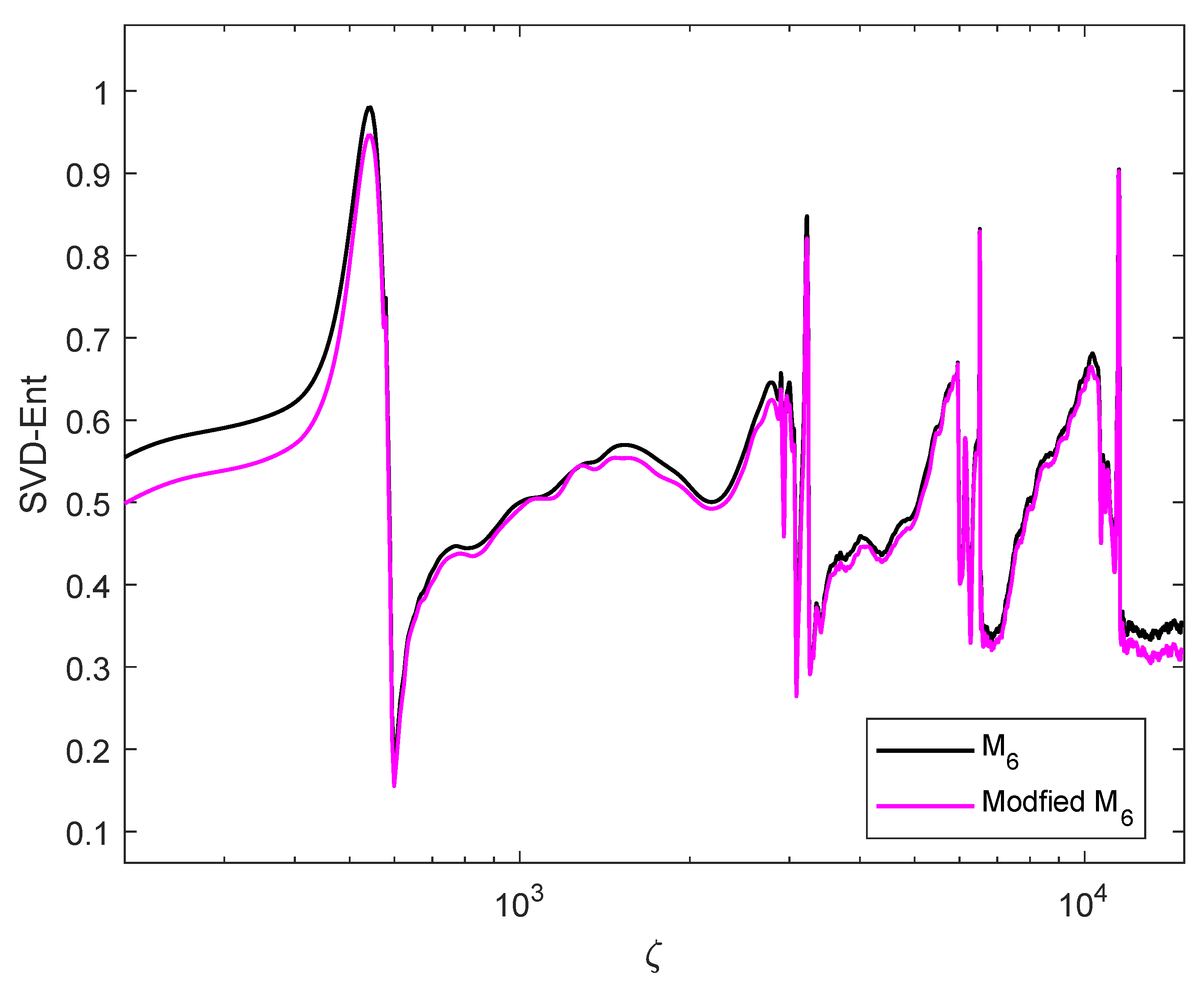
4. Graph-Entropy
5. Conclusions
Conflicts of Interest
Abbreviations
| SIT | Self-induced Transparency |
| EIT | Electromagnetically Induced Transparency |
| hf | hyperfine |
| dmc | density matrix components |
References
- McCall, S.L.; Hahn, F.L. Self-induced transparency by pulsed coherent light. Phys. Rev. Lett. 1967, 18. [Google Scholar] [CrossRef]
- Slusher, R.E.; Gibbs, H.M. Self-Induced Transparency in Atomic Rubidium. Phys. Rev. A 1972, 5, 1634. [Google Scholar] [CrossRef]
- Boller, K.J.; Imamoglu, A.; Harris, S.E. Observation of Electromagnetically Induced Transparency. Phys. Rev. Lett. 1991, 66, 2593–2596. [Google Scholar] [CrossRef] [PubMed]
- Alhasan, A.M. Entropy Associated with Local Stabilization of the Pulse Area in Multilevel Atomic Media. Act. Phys. Pol. A 2018, 133, 5. [Google Scholar] [CrossRef]
- Alhasan, A.M. Advanced soliton-train generation through drive field enhancement and multiple light storage effect in cold rubidium atoms. Eur. Phys. J. Spec. Top. 2007, 144, 277–282. [Google Scholar] [CrossRef]
- Sparavigna, A.C. Entropy in Image Analysis. Entropy 2019, 21, 502. [Google Scholar] [CrossRef] [PubMed]
- Gandi, V. Brain-Computer Interfacing for Assistive Robotics: Electroencephalograms, Recurrent Quantum Neural Networks, and User-Centric Graphical Interfaces; Academic Press, Elsevier Inc.: Amsterdam, The Netherlands, 2015. [Google Scholar] [CrossRef]
- Alhasan, A.M. Entropy Associated with Information Storage and Its Retrieval. Entropy 2015, 17, 5920–5937. [Google Scholar] [CrossRef]
- Alhasan, A.M. Short pulses propagation in multilevel atomic media. AIP Conf. Proc. 2018, 1976, 020005. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alhasan, A.M. Graph Entropy Associated with Multilevel Atomic Excitation. Proceedings 2020, 46, 9. https://doi.org/10.3390/ecea-5-06675
Alhasan AM. Graph Entropy Associated with Multilevel Atomic Excitation. Proceedings. 2020; 46(1):9. https://doi.org/10.3390/ecea-5-06675
Chicago/Turabian StyleAlhasan, Abu Mohamed. 2020. "Graph Entropy Associated with Multilevel Atomic Excitation" Proceedings 46, no. 1: 9. https://doi.org/10.3390/ecea-5-06675
APA StyleAlhasan, A. M. (2020). Graph Entropy Associated with Multilevel Atomic Excitation. Proceedings, 46(1), 9. https://doi.org/10.3390/ecea-5-06675




