Measurement of Environmental Efficiency in the Countries of the European Union with the Enhanced Data Envelopment Analysis Method (DEA) during the Period 2005–2012 †
Abstract
:1. Introduction
2. Methodology
| Inputs | Outputs Desirable | Outputs Undesirable |
|---|---|---|
| Production of Electricity from Coal | PIB Gross Domestic Product (GDP) | CO2 |
| Production of Electricity from Petroleum | Rent per Capita | CH4 |
| Production of Electricity of Nuclear Origin | ||
| Industrial Production Index | ||
| Volume of Vehicles |
2.1. Method 1
2.2. Method 2
3. Results
3.1. Method 1
3.2. Method 2
4. Discussion of Results
4.1. Statistical Study of Inputs and Outputs Data
- As for the generation of electricity from coal, the maximum value is reached in Estonia in the year 2007 while the lowest value is repeated in different countries for several years, as for example in Malta, Cyprus, Latvia and Luxembourg during the years 2005 and 2006, among others.
- For the generation of electricity from oil, the highest value is reached in Malta for several years, for example, in 2005 or in 2008. The lowest value is reached in Latvia in 2012.
- In the generation of electricity from nuclear energy, we have that the highest value is reached in 2011 in France. As for the minimum value, this is reached on several occasions in several countries although they are often repeated. For example, this value is achieved in Austria, Cyprus, Croatia, Denmark, Estonia, Greece, Ireland, Italy, Latvia, Luxembourg, Malta, Poland and Portugal in 2007 and 2008, among others.
- The maximum value of the industrial production index is given in 2010 in Estonia. However, the lowest value was also reached in Estonia in 2009.
- Regarding the volume of vehicles, the maximum value is found in Germany in 2006 and the lowest value in Malta in the year 2005.
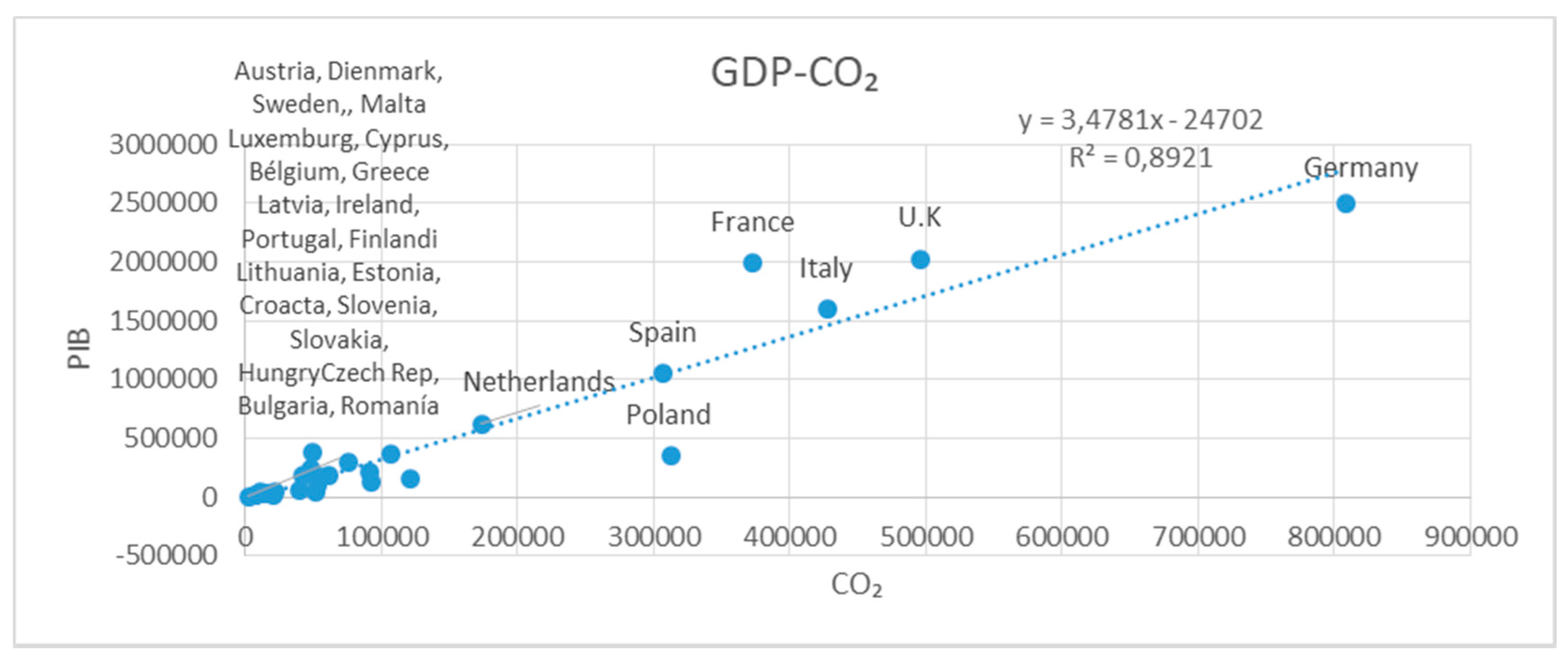
- For GDP, the highest value is reached in 2012 in Germany. The lowest value is reached in Malta in the year 2005.
- In per capita income, the highest value is reached in Luxembourg in 2011 while the lowest value is reached in 2005 in Bulgaria.
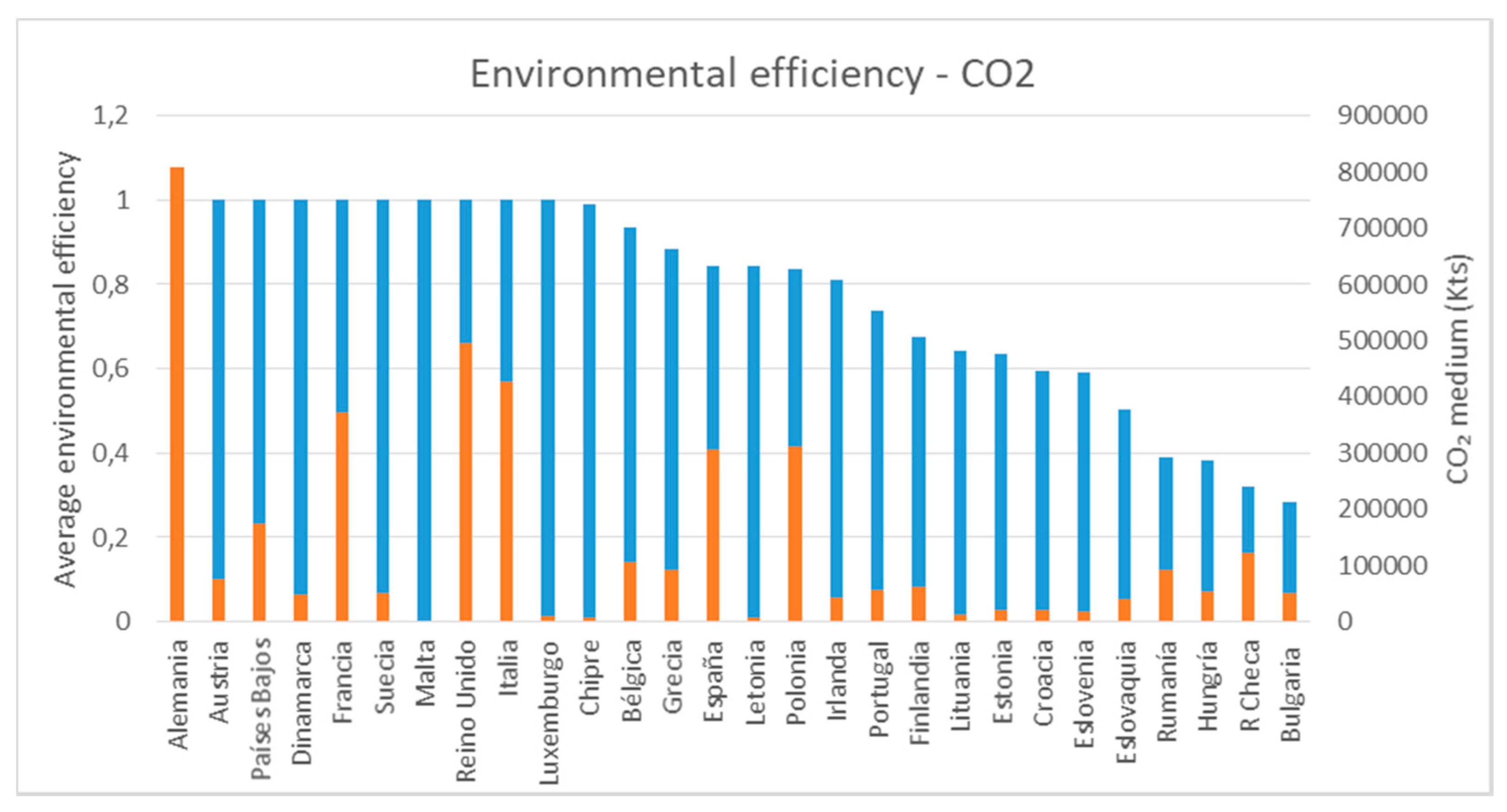
- In carbon dioxide emissions, the highest value is found in 2006 in Germany and the lowest value in Luxembourg in 2012.
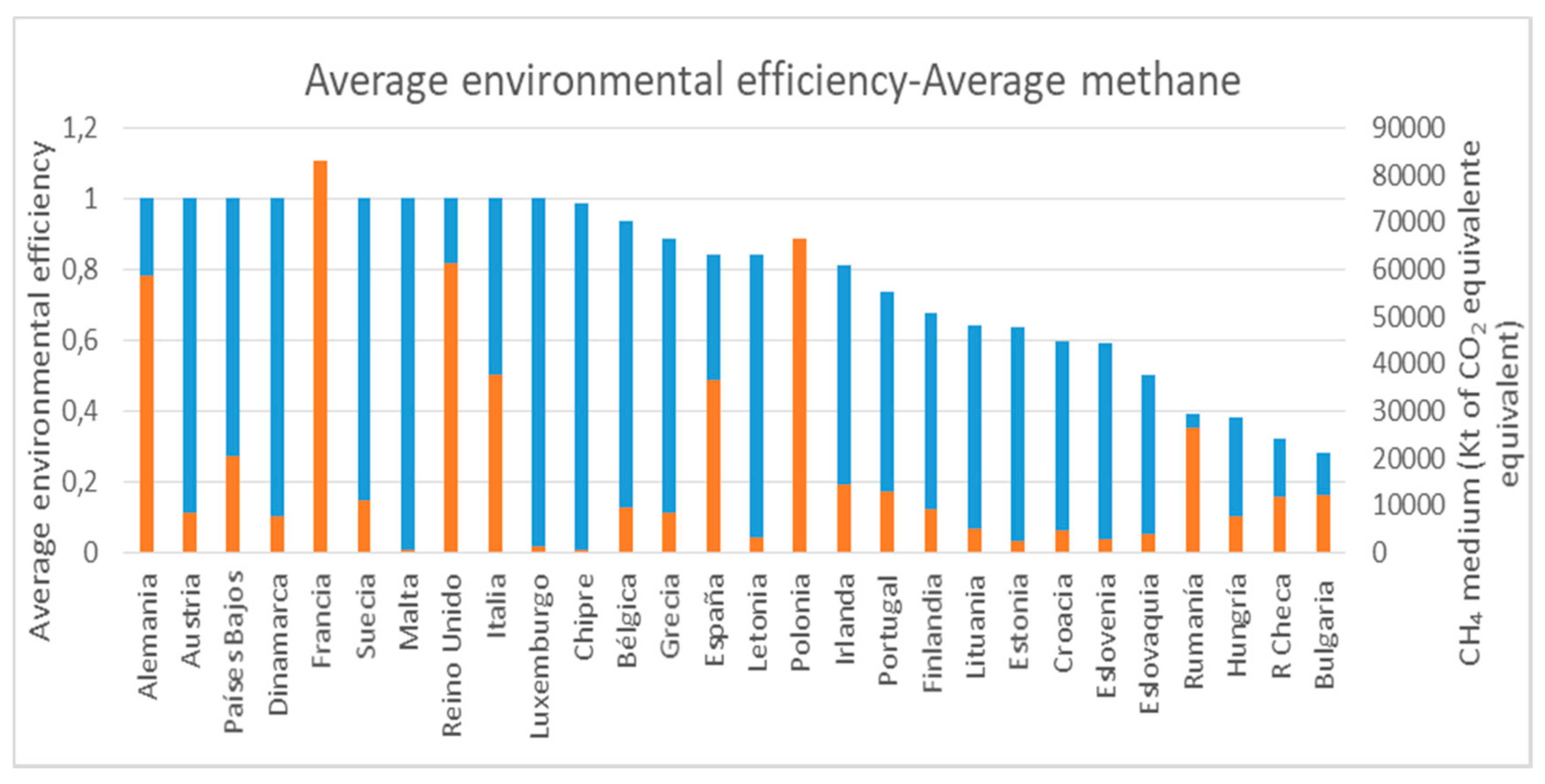
- For methane emissions, the highest value was reached in 2010 in France. However, the lowest value was reached in Luxembourg in 2012.
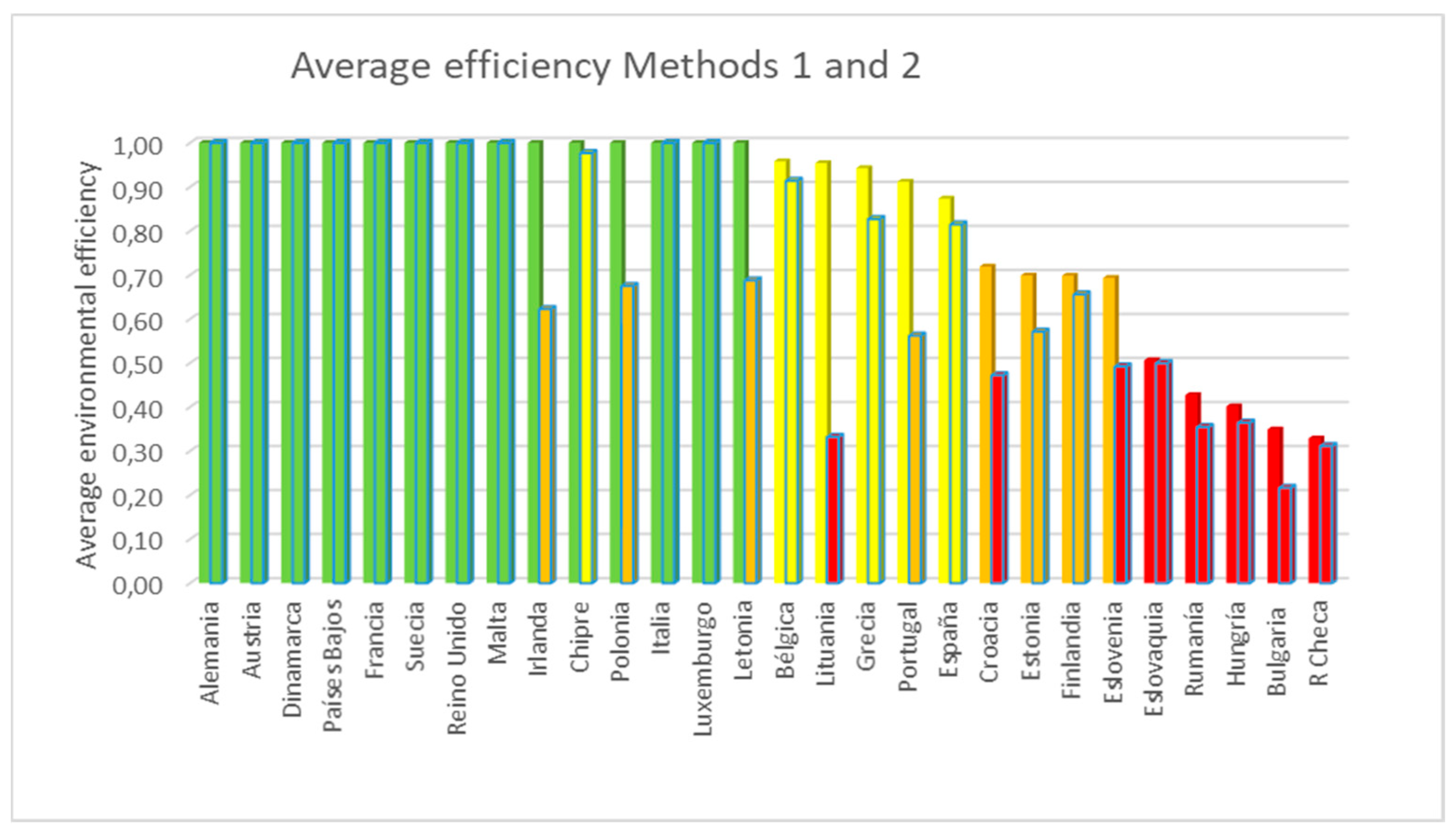
4.2. Comparison between Method 1 and Method 2
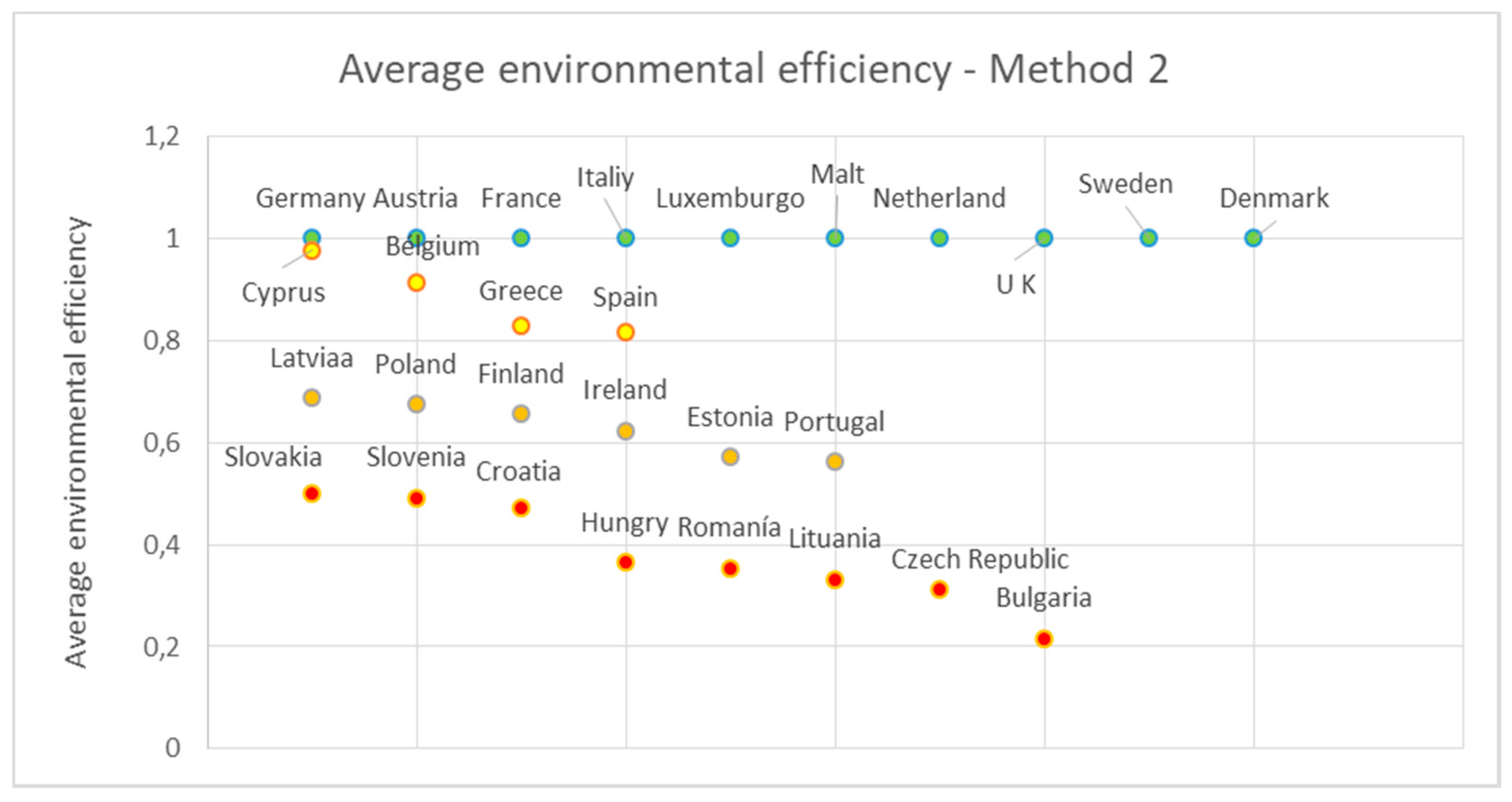
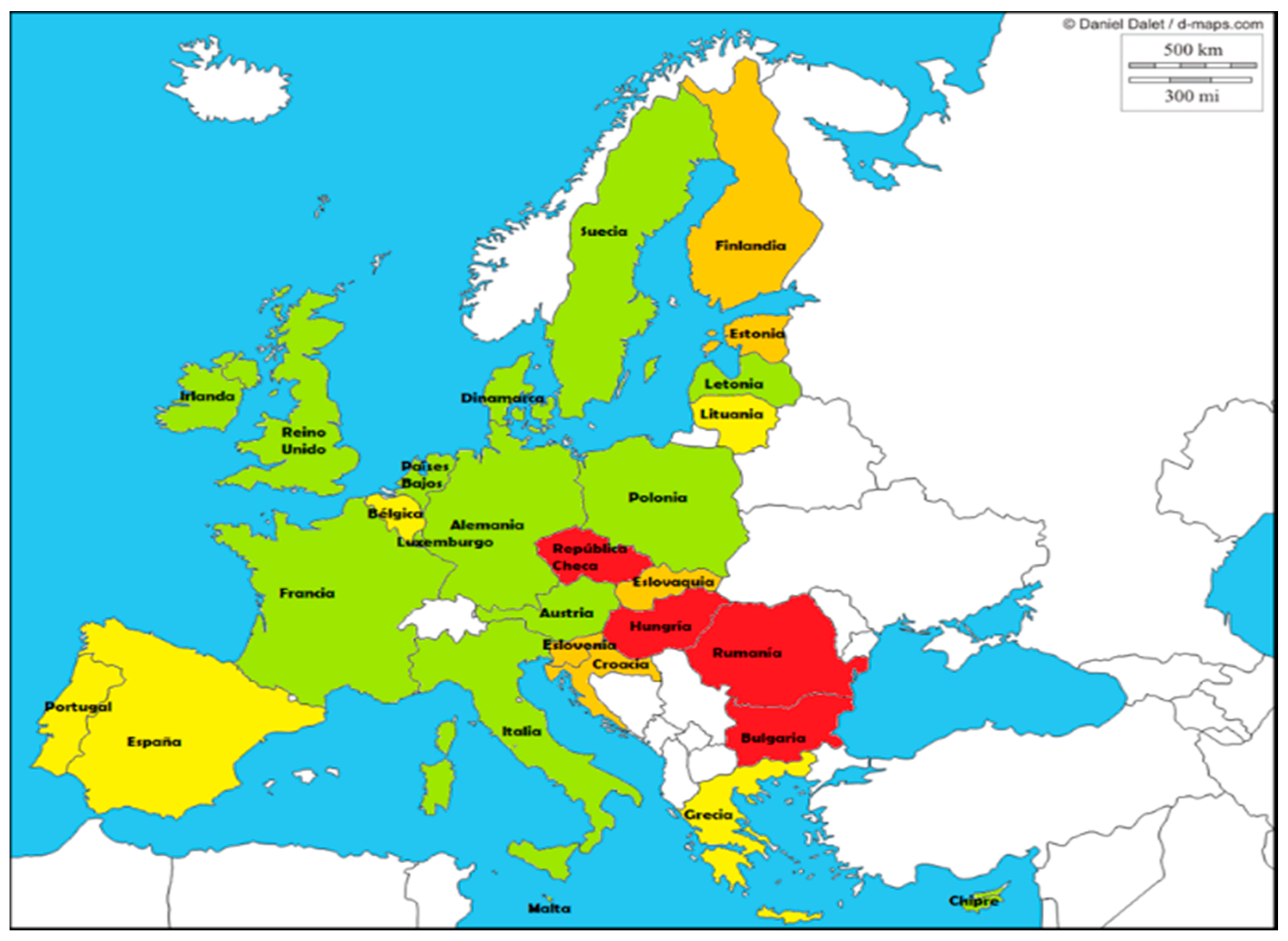
4.3. Establishment of Categories Based on Relative Environmental Efficiency Values
- Excellent environmental efficiency: for those countries with an average of between 0.9999 and 1 and which was represented with the green color.
- Good environmental efficiency: for those countries with an average between 0.8 and 0.9999 and that are represented by the color yellow.
- Average environmental efficiency: for those countries with an average between 0.5 and 0.79 and which are represented by the color orange.
- Improved environmental efficiency: for those countries with an average between 0 and 0.49 and which are represented by the color red.
- 14 countries are in the category “Excellent environmental efficiency” and are Germany, Austria, Cyprus, Denmark, France, Ireland, Italy, Latvia, Luxembourg, Malta, the Netherlands, Poland, the United Kingdom and Sweden.
- 5 countries are in the category of “Good environmental efficiency” and are Belgium, Spain, Greece, Lithuania and Portugal.
- 5 countries are in the category of “Average environmental efficiency” and are Croatia, Slovakia, Slovenia, Estonia and Finland.
- The remaining 4 countries are in the category of “Improvable environmental efficiency” and are Bulgaria, Hungary, Czech Republic and Romania.
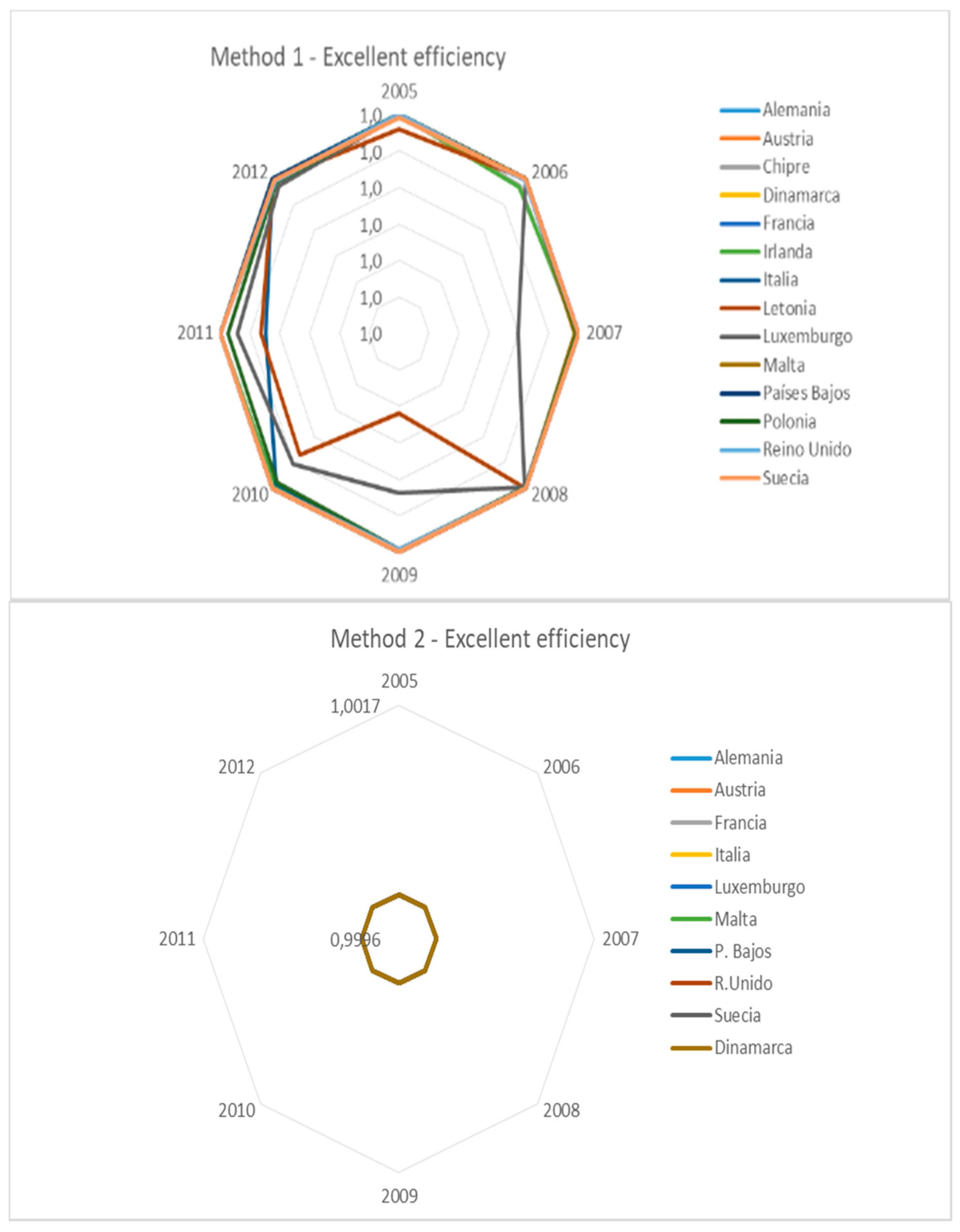
- Cyprus goes from excellent efficiency to good efficiency.
- Ireland goes from excellent efficiency to good efficiency.
- Latvia goes from excellent efficiency to average efficiency.
- Lithuania goes from good efficiency to improvable efficiency.
- Poland goes from excellent efficiency to average efficiency.
- Slovakia goes from medium efficiency to improvable efficiency.
- Slovenia goes from medium efficiency to improvable efficiency.
- Croatia goes from medium efficiency to improved efficiency.

4.4. Evolution of Environmental Efficiency for Different Countries.
- Austria maintains environmental efficiency values very close to 1 because its emissions are not as high as in other countries although the economic indicators are lower than, for example, Germany in the previous case. Despite this, it has environmental efficiency values similar to Germany because its emissions are lower since less than 20% of its electricity comes from coal and oil and its volume of vehicles is not so high. However, the situation could improve if they improve their economic indicators.
- Belgium has a very high environmental efficiency. Their emissions are not very high, which is partly due to the fact that most of the electricity they consume comes from nuclear power plants and less than 20% comes from coal and oil. The number of vehicles is high, although within our study there are countries with a greater volume of vehicles, such as Germany. Its economic indicators are not very high, but as the emissions are not either, a high environmental efficiency is maintained. A possible improvement would come if the GDP and per capita income were improved.
- Bulgaria maintains a low environmental efficiency except in the years 2010 and 2012 in which the environmental efficiency differs a lot from the trend of the other years. Bulgaria shows the lowest environmental efficiency values that have been recorded in our study. This may be due to the fact that GDP and per capita income are very low although emissions are not very high. However, the fact that economic indicators are so low is what makes environmental efficiency very low. It is also true that emissions could be reduced since there is a large volume of vehicles and almost 50% of the electricity comes from coal, although almost the entire remaining part comes from nuclear power plants. The solution for Bulgaria would be to reduce the use of coal to obtain electricity and improve economic indicators.
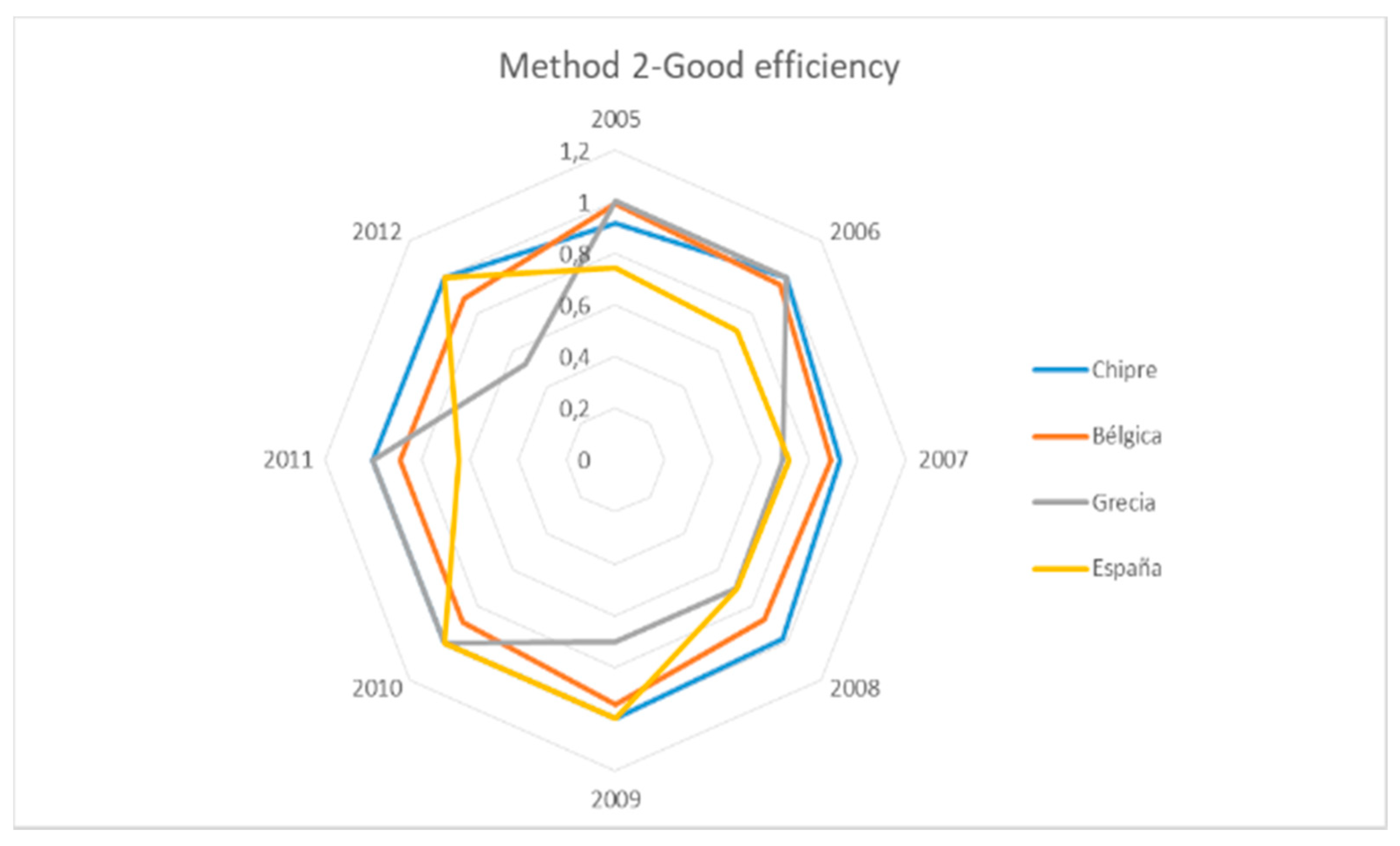
- Cyprus has environmental efficiency values very close to 1. This is due to the fact that GDP and per capita income are kept at medium values and that emissions are not very high. However, practically 100% of electricity comes from oil, so it would be very convenient to look for other more sustainable sources of energy, such as renewable energies, to improve their situation.
- Croatia maintains an average efficiency but from 2010 its environmental efficiency rises a lot and remains the same in the rest of the years of the study. Croatia shows these relatively low values of environmental efficiency because, although emissions are not too high, GDP and per capita income are not among the highest we find in the countries under study. The volume of vehicles is not excessively high and between the production of electricity from coal and oil do not reach 50% so to improve their situation should improve their economy and perhaps seek other sources of more renewable energy or bet on the nuclear energy that is practically absent in this country. As of 2010, the values of environmental efficiency are very close to 1. This may be due to the fact that the emission values are lower during these years as well as the production of electricity from oil.
- Denmark always shows very high values of environmental efficiency. These values are due to the fact that the emissions have acceptable values, in addition to the fact that the economic indicators are correct, although they are not as high as in Germany, which is the country that shows the best GDP and per capita income among the countries that make up our study. However, the production of electricity from coal is always higher than 34%, so maybe we should look for other sources of energy such as renewables or bet on nuclear energy that is absent in this country.
- Slovakia maintains a low environmental efficiency, except in the year 2005 in which environmental efficiency has a very different value from the trend followed. The emission values are below the average as well as the per capita income, but the GDP is well below the average, which may be the reason why its efficiency values are not too high. It shows values of electricity production from very high nuclear sources (always above 50%) and much higher than those from coal and oil, which is positive from the point of view of reducing emissions. However, to improve its efficiency it should improve its GDP. In addition, environmental measures could be taken such as increasing the number of electric cars, switching to renewable energies, among other measures.
- Slovenia has a greater environmental efficiency in the years 2010 and 2011 However, in the remaining years, environmental efficiency remains at medium values. In 2010 and 2011 the values of the economic indicators are slightly higher than in the rest of the years, this may be the reason why the environmental efficiency is greater in these years, while the CO2 emissions are also reduced a bit. with respect to previous years. The emission values remain below the average but also the values of the economic indicators, which means that the environmental efficiency is not higher. To improve the situation, it would be necessary to improve its economy. The production of electricity from coal and nuclear sources have similar values around 30%, so the search for other energy sources to try to reduce the use of coal could also be advantageous.
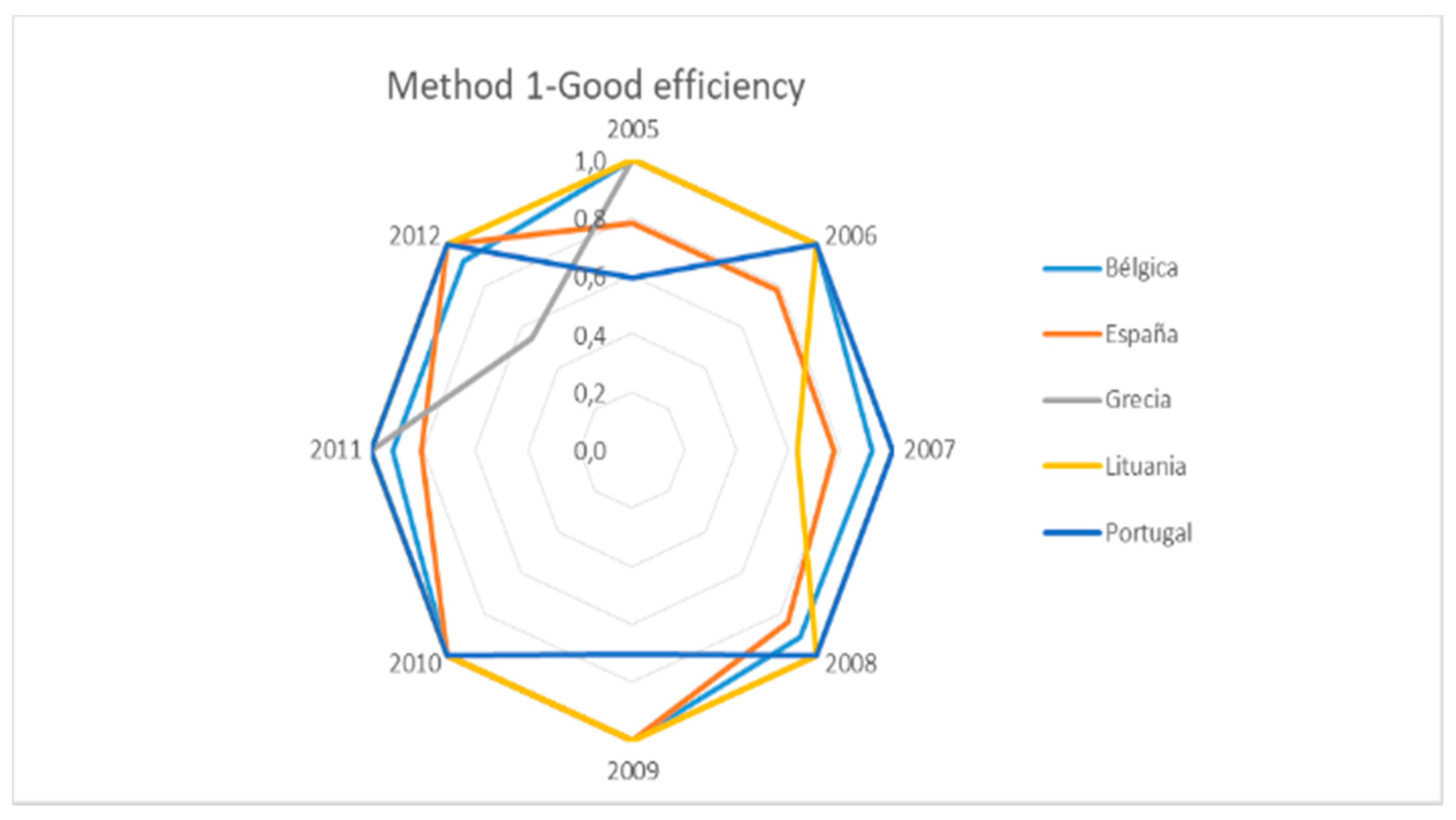
- Spain has a medium-high environmental efficiency but there are three years in which the environmental efficiency is higher and close to 1. Spain has a GDP above the average while in per capita income barely exceeds the average and in most of the cases does not even reach the average. In the emissions, the average values are exceeded. This is what makes the environmental efficiency values are not higher. In the years 2009, 2010 and 2012, the values of carbon dioxide emissions are slightly lower than in the rest of years, which may be the reason why the efficiencies are higher in those years. The solution for Spain would be to improve its per capita income and reduce emissions.
- Estonia maintains an environmental efficiency in average values but there are two years whose values of environmental efficiency go out of the followed trend and they are the year 2009 and the year 2012. Estonia has a GDP and a per capita income well below the average although, despite the fact that 90% of its electricity comes from coal, the emissions are below the average. To achieve greater efficiency, Estonia should improve its economy and bet on other sources of energy to reduce the use of coal, such as renewable energy or perhaps develop nuclear energy that is not exploited in this country.
- Finland has a high environmental efficiency in the years 2005 and 2010. In the rest of the years there are average values of efficiency. The GDP is below the average as well as the emissions but the per capita income does exceed the average. The fact that GDP is relatively low is what can make efficiency no higher. A possible solution for Finland would be to increase its GDP to have greater environmental efficiency in addition to adopting environmental measures that reduce the impact, such as renewable energy.
- France always has a very high environmental efficiency and with few variations, always being very close to 1. The emissions of carbon dioxide and methane are well above the average as well as per capita income and GDP. Although the emissions are relatively high, the GDP is also very high, which makes it have a good efficiency. France should reduce its emissions by reducing the number of vehicles (which is much higher than the average) since it hardly uses coal when most of its electricity comes from nuclear power plants (around 70%).
- In the case of Greece, the values of environmental efficiency are very high for all years except for the last one in which the environmental efficiency decreases a lot. The GDP is below the average as well as the income per capita, but the efficiency is high because the emissions are not high and are well below the average but could be improved if it were achieved to increase the GDP and the rent per capita. The generation of electricity from coal exceeds 50% and if the oil is added already exceeds 70% so that Greece should look for other sources of energy such as renewables. In 2012, the values of economic indicators are lower, which is possibly the reason why efficiency is much lower in that year.
- Hungary shows quite low environmental efficiency values. Unlike many countries, it does not show anomalous environmental efficiency values that go out of the trend that is followed in the rest of years. In Hungary, the economic indicators are below the average which means that, although the emissions are below the average, the values of environmental efficiency are at quite low values. The situation could improve if the country’s economy improved.
- Ireland, like other countries, shows very high values of environmental efficiency that hardly change. In Ireland, GDP is below the average, as opposed to income per capita, but emissions are also below the average, which is what makes their efficiency very high. For the situation to be optimal, Ireland should increase its GDP. Other environmental measures could also be taken to further improve the situation.
- In Italy, the situation is contrary to Ireland. Both countries have a very high environmental efficiency in all years, but while in Ireland it is due to low emissions, in Italy it is because GDP and per capita income are well above the average, but emissions also exceed the average. The advisable thing for Italy would be that they reduced their emissions since the volume of vehicles is very high (in fact it is very above the average).
- In the case of Latvia, efficiency remains very high and without fluctuations in the years of the study. A very positive issue is that very little energy comes from coal and oil or from nuclear sources in Latvia, so perhaps part of their electricity comes from renewable sources, which is very convenient for high environmental efficiency. GDP and per capita income are below the average but emissions are quite low, which makes efficiency high. However, for the situation to be optimal, GDP and per capita income should be increased.
- Lithuania has very high environmental efficiency values. However, in 2007, the value of environmental efficiency is very different from the others and much lower. For emissions, Lithuania shows low values (below the average) which is what possibly makes its efficiency high despite the fact that GDP and per capita income are also low and do not exceed the average. For this reason, Lithuania should improve its economy. In Lithuania little coal and oil are used to obtain electricity, so nuclear energy or renewable sources are mostly used, which is good for high environmental efficiency.
- Luxembourg always shows a very high efficiency. This is because the emissions are quite low and well below the average and also the per capita income is also above the average, but not the GDP. Energy in Luxembourg comes from coal, oil and nuclear sources, so we assume that renewable energies are well developed in this country. The situation could be even better if GDP could be increased.
- Malta maintains a high environmental efficiency during the eight years of study. It has GDP and per capita income values well below the average. However, the emissions are well below the average and are very low, which is what makes their efficiency high. On the other hand, 100% of the energy comes from oil, so to improve its situation one could opt for renewable energies, in addition to improving GDP and per capita income.
- The Netherlands has high and constant environmental efficiency values. Both GDP and per capita income are above average, as are carbon dioxide emissions, although methane emissions are quite low, which means that, together with the good situation of economic indicators, environmental efficiency be high The electricity consumed in Luxembourg does not come from coal, oil or nuclear sources so it can come from renewable sources, which would be very convenient to have a high environmental efficiency. Luxembourg should reduce its CO2 emissions to make the situation even better.
- Poland presents high environmental efficiency values. The GDP and per capita income values are below the average and CO2 emissions slightly exceed the average. However, it has methane emission values below the average, which is what can make its efficiency high. On the other hand, 90% of its electricity comes from coal, so it would be necessary to reduce this figure, perhaps with the change to renewable sources of energy. It would also be necessary to reduce CO2 emissions and increase GDP and per capita income.
- Portugal maintains a high environmental efficiency for all the years of the study except for two years, in 2005 and 2009. Its GDP and per capita income are always below the average and methane emissions are above the average. However, it does present a high efficiency because carbon dioxide emissions are very low and well below the average. Among the electricity that comes from oil and coal, they exceed 50% of the total electricity generation, so it would be advisable to invest in renewable energies, reduce methane emissions and improve GDP and per capita income.
- The United Kingdom shows very high values of environmental efficiency. Its GDP and per capita income values are above the average, in addition to its GDP is quite high, this is what makes its efficiency is high since the emissions are quite high and are above the average, so it would be advisable to reduce these emissions, for example, by reducing the volume of vehicles that is quite high in this country or, better still, replacing conventional vehicles with electric or hybrid vehicles.
- The Czech Republic shows low efficiency values and there is no data other than the trend followed. These values are due to the fact that GDP and per capita income are below the average, although emissions are slightly lower than the average, so it would be better to improve GDP and per capita income and reduce emissions. 60% of the energy comes from coal, so it would also be convenient to increase the energy coming from renewable sources.
- Romania maintains its low environmental efficiency values. However, there are two years in which the environmental efficiency is very different, in 2005 and 2010. In Romania, GDP and per capita income are well below the average and methane emissions are above the average. average, which means that, although carbon dioxide emissions are below the average, environmental efficiency is low. To improve their environmental efficiency, GDP and per capita income should be improved, in addition to reducing methane emissions.
- In the case of Sweden, environmental efficiency is always high. GDP does not exceed the average, although income per capita does, and carbon dioxide and methane emissions are quite low and do not exceed the average. This is what makes its environmental efficiency high but could still be improved if GDP were increased. There is also the fact that there is hardly any electricity produced from oil and coal, which is favorable for high environmental efficiency.
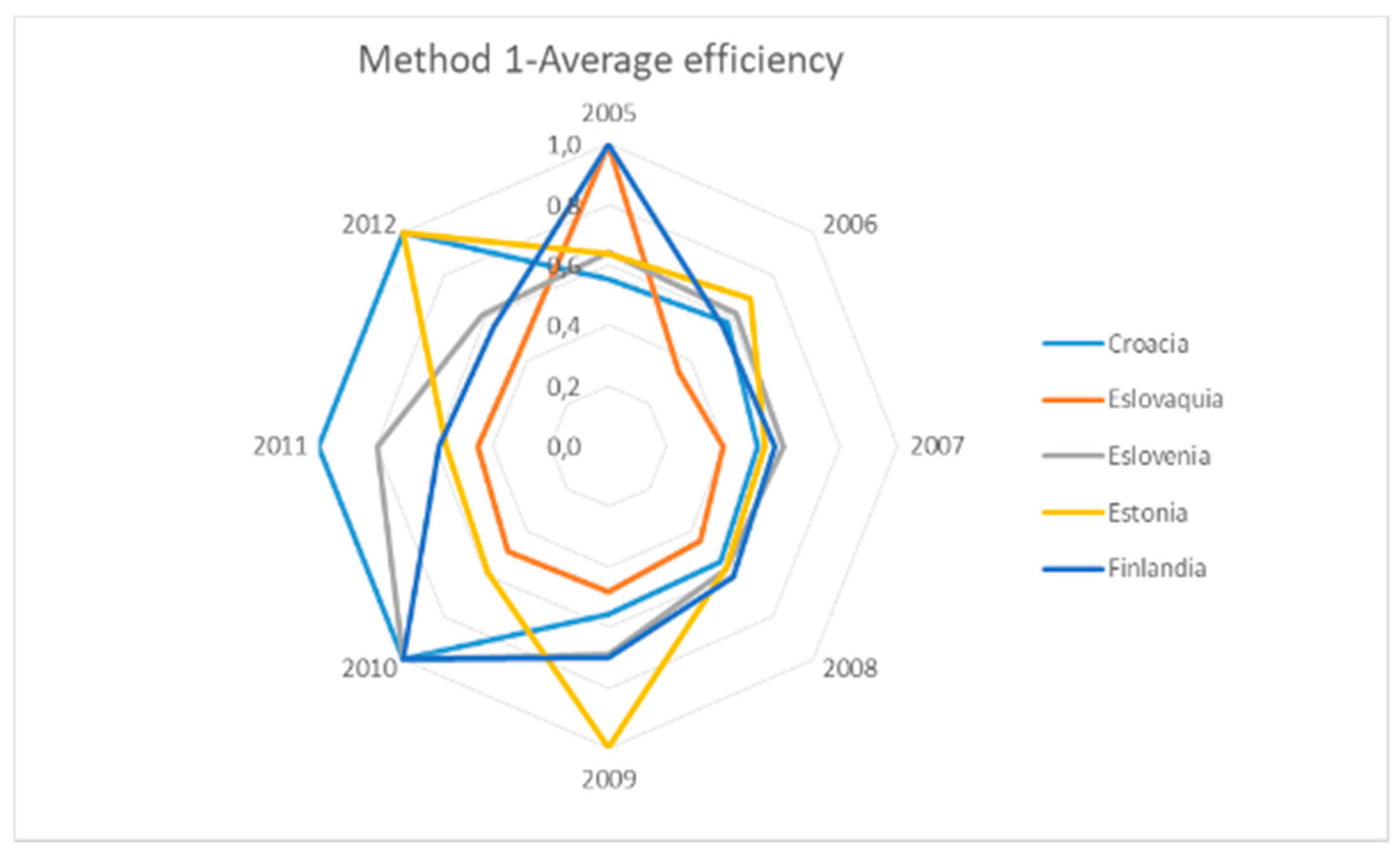
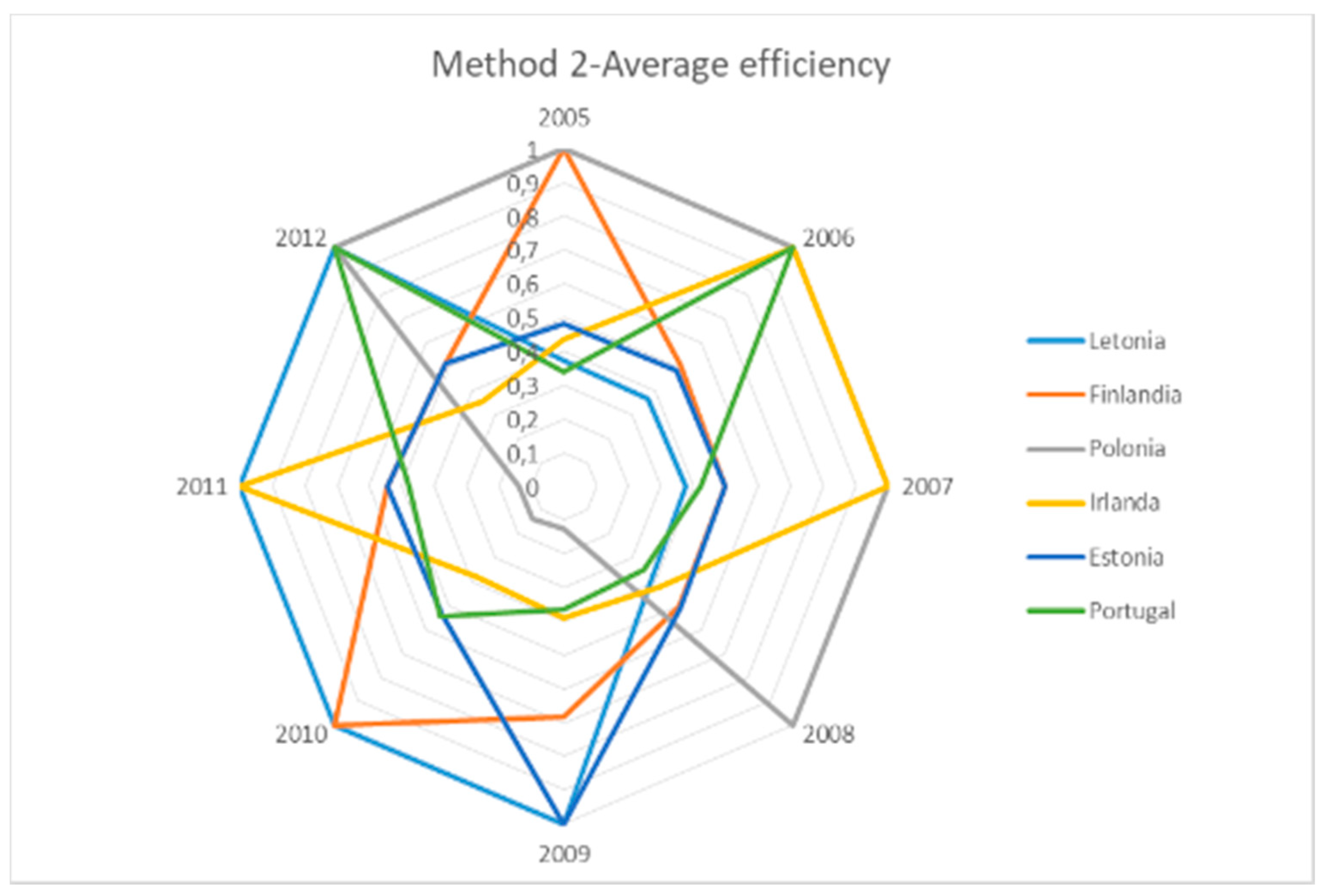
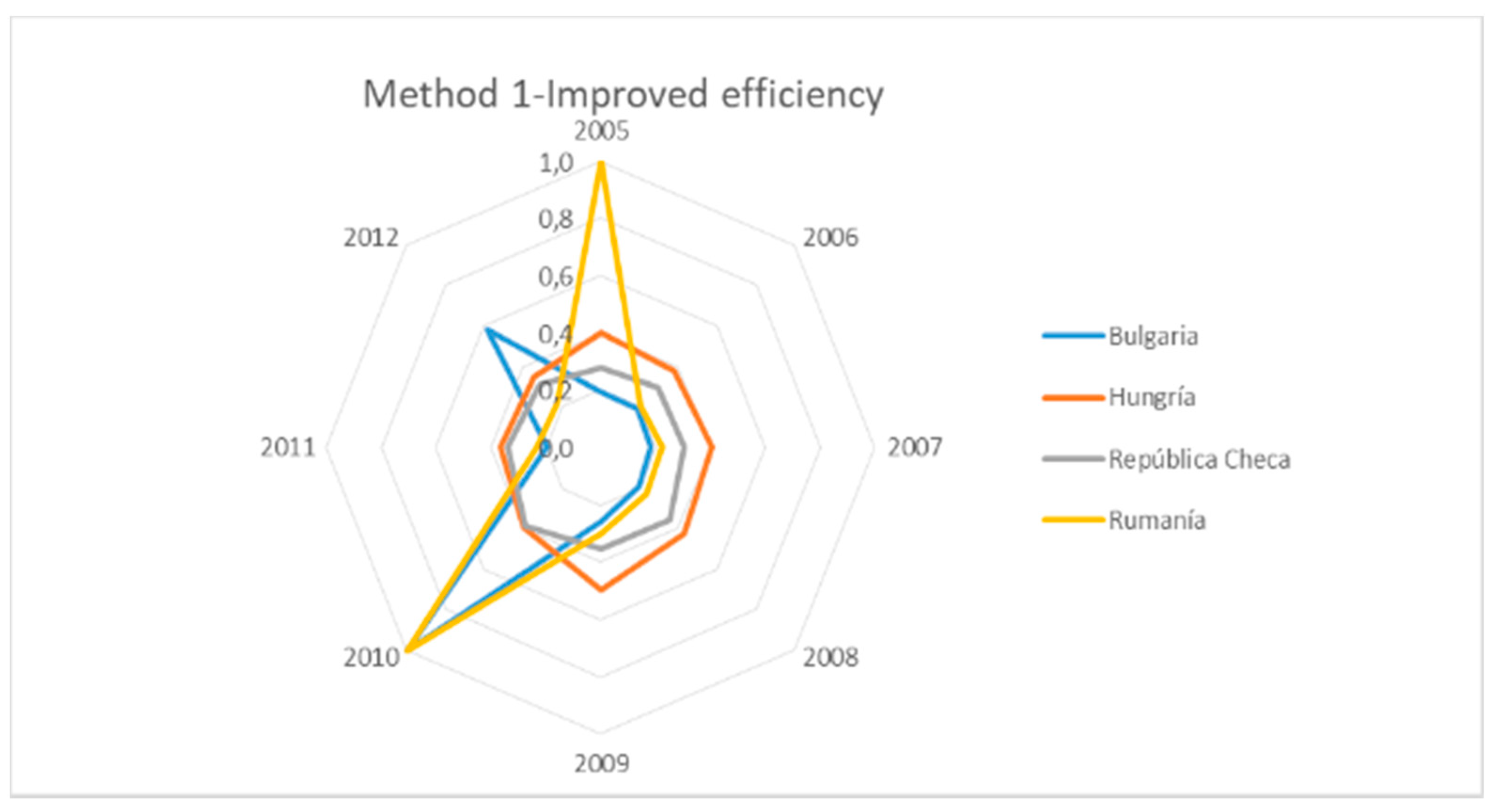
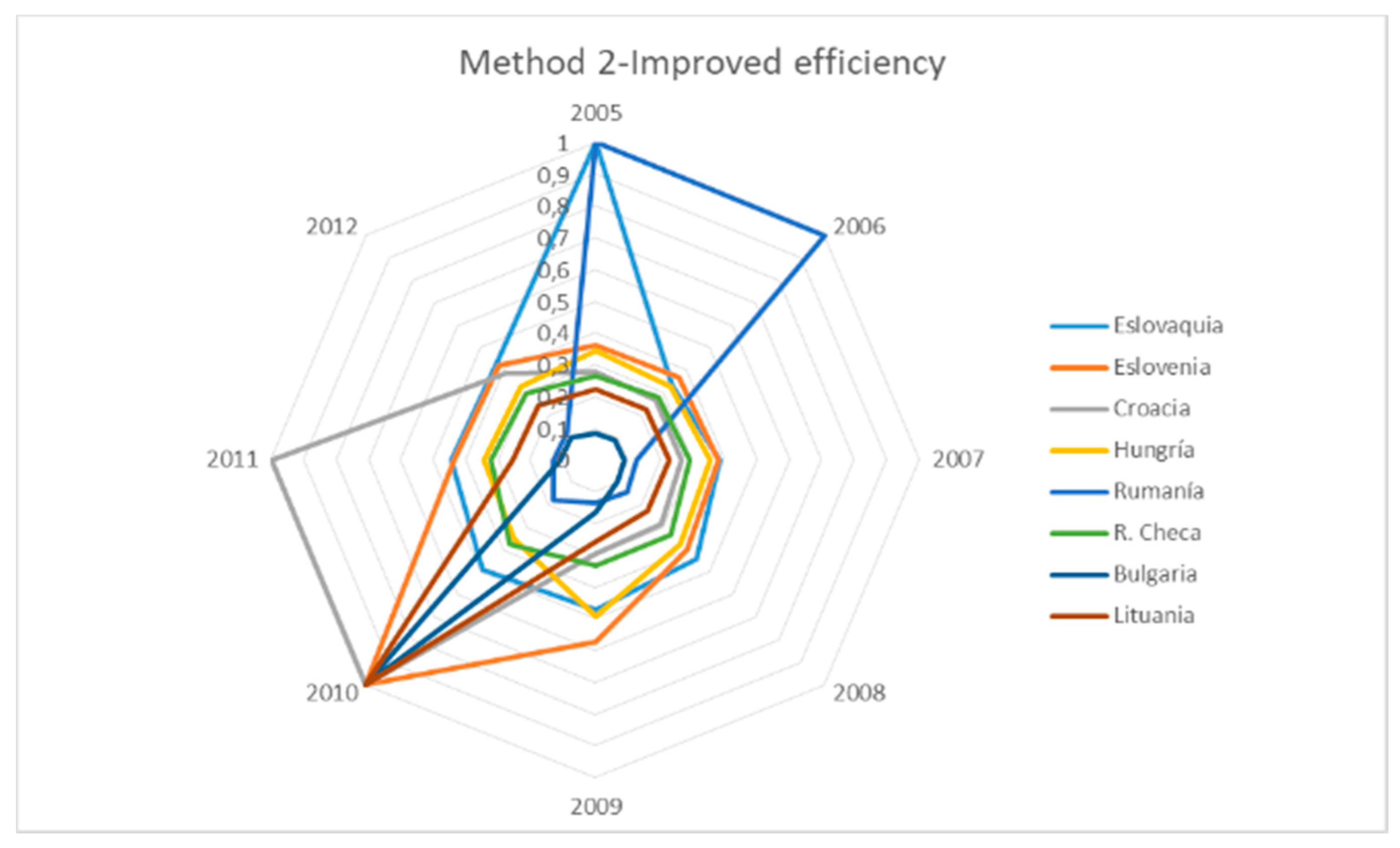
4.5. Temporal Evolution of Average Environmental Efficiency
- There are countries that also maintain a very high environmental efficiency in the 8 years but lower than the countries with excellent environmental efficiency. This would be the case of Belgium, which maintains a high environmental efficiency with slight variations.
- There are countries that follow a trend and their values of environmental efficiency fluctuate little in the eight years of the study. This would be the case, for example, of Hungary and the Czech Republic.
- We also find countries that follow a trend except in some years in which the values of environmental efficiency go out of the trend followed and are much higher than in the remaining years, even reaching an efficiency of 100%. This occurs in countries such as Bulgaria, Spain and Romania, among others (perhaps because they are countries with a more unstable economy and which are more sensitive to changes in the global economic situation) and usually also occur in specific years such as 2005 or 2011 but it is in 2010 that the largest amount of anomalous data is concentrated for the different countries with a large number of them with an efficiency close to 1 when in the remaining years they have a much lower efficiency [44].
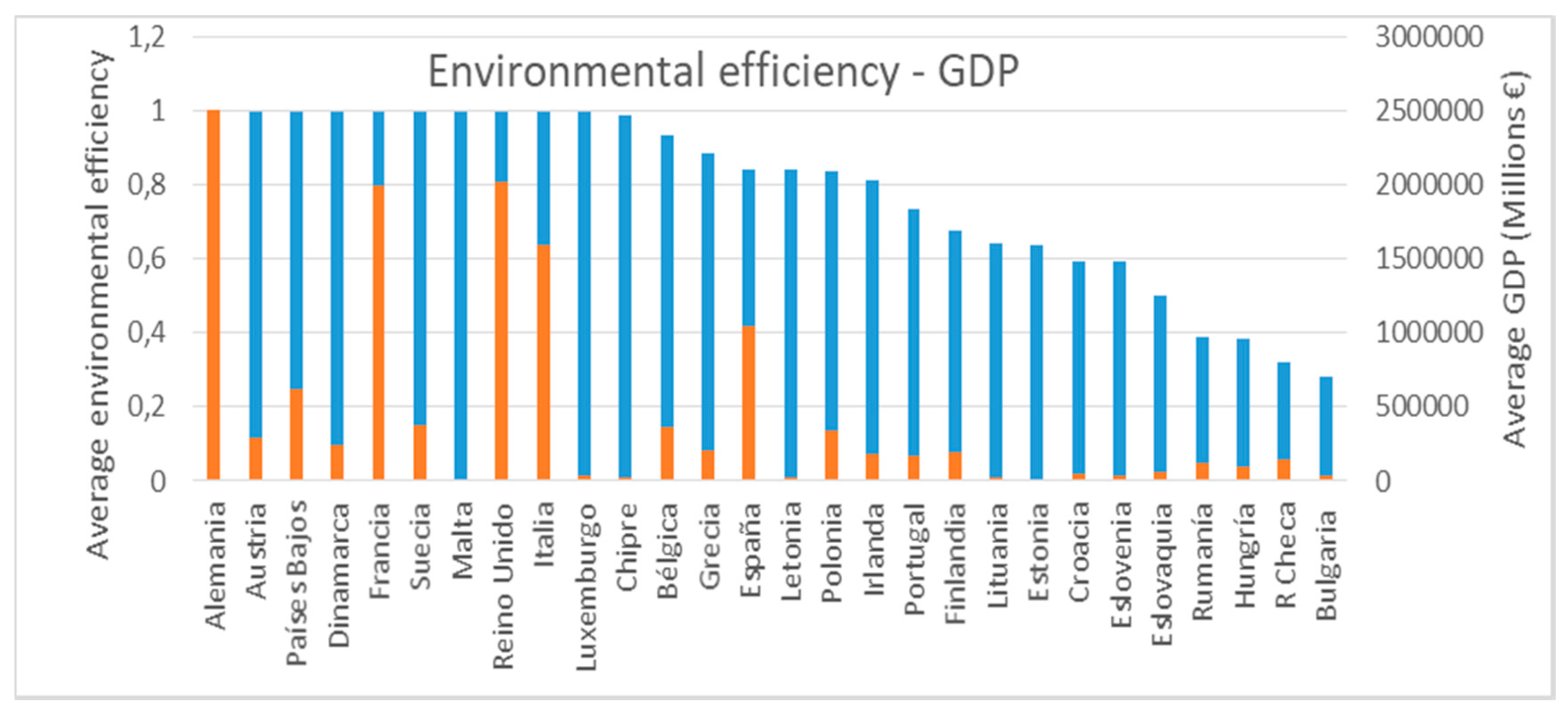
- Subsequently, we obtain the average environmental efficiency data for each of the years of the study, making the average of the environmental efficiency values of all the countries, for both Method 1 and Method 2 and then make the average between Method 1 and Method 2 to analyze the global situation in the Euro Zone. Based on the results obtained for the mean of Methods 1 and 2, it can be seen that in the eight years in which we have done the study, the values of environmental efficiency are quite high, with values that fluctuate between 0.73605 the year in which the average environmental efficiency was lower than it was in 2007 and 0.87039 the year with the highest average environmental efficiency that was 2010. This is due to the fact that in 2010 many countries show a very high environmental efficiency and higher than in the rest of the years due to the fact that in that year the emissions are somewhat lower. We have also obtained an average efficiency value for the total of the European Union from the average of the values we had obtained from the mean of Methods 1 and 2. This value is 0.7824 which is in the category of Average environmental efficiency but it is quite high. All these values are in Table 11 [45].
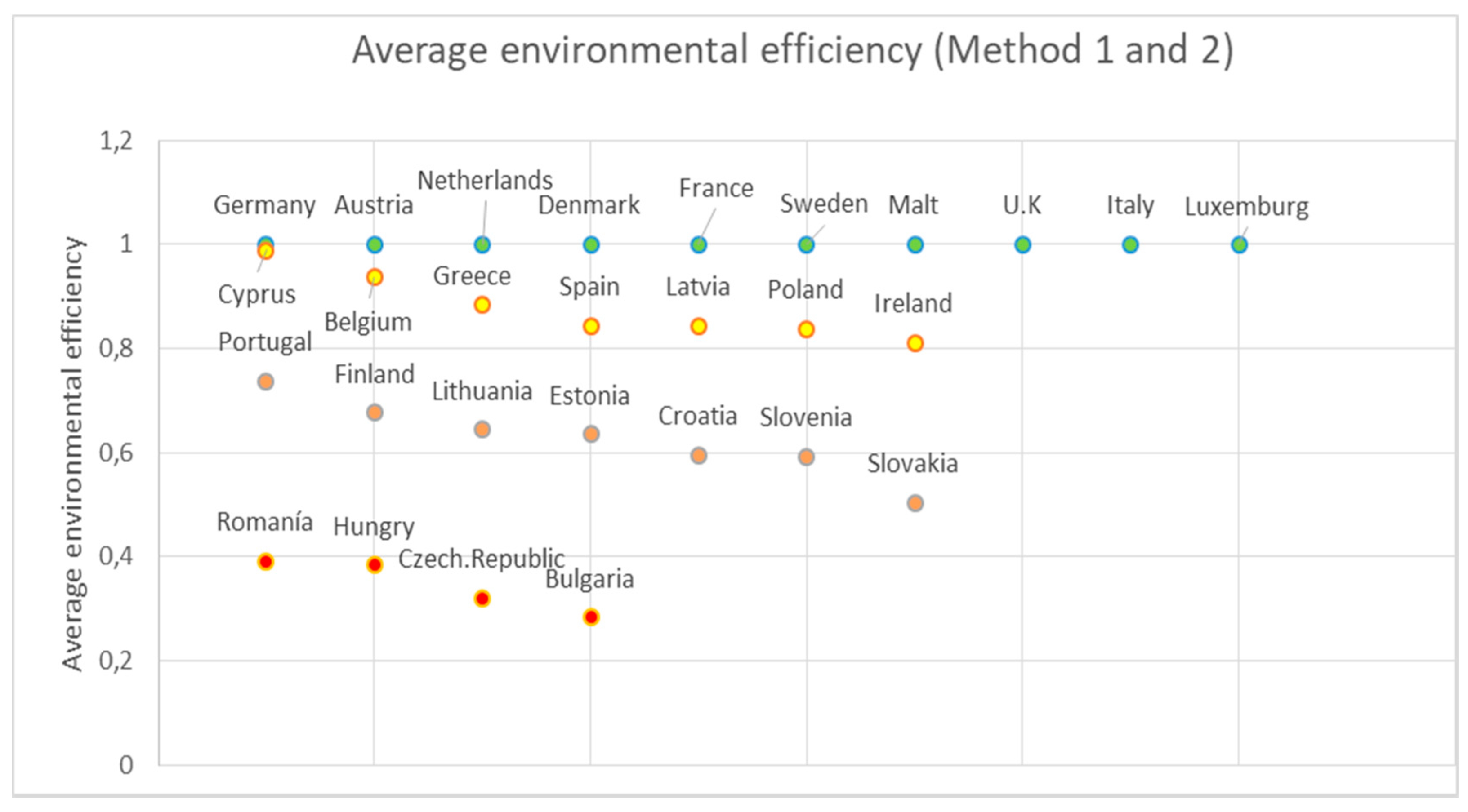
4.6. Obtaining Average Values between Method 1 and Method 2
4.7. Improved Analysis Method (MAM)
5. Conclusions
- Change to more sustainable economic models (such as the Circular Economy) to try to increase its GDP and per capita income: it would be applicable to all countries in general, although in particular to Austria, Belgium, Bulgaria, Croatia, Slovakia, Slovenia, Spain , Estonia, Greece, Hungary, Ireland, Latvia, Malta, Poland, Portugal, Czech Republic, Romania and Sweden. However, it must be pointed out that this solution is complicated to apply and depends more on economic policies.
- Reduce your carbon dioxide or methane emissions or both: Germany, Bulgaria, Spain, France, Italy, the Netherlands, Poland, Portugal, the United Kingdom, the Czech Republic and Romania. This could be achieved by using energy sources that do not come from coal or petroleum or proposing changes in the energy model of these countries that make them more sustainable and environmentally efficient.
- Increase the amount of energy from renewable sources by reducing the energy dependence derived from highly polluting fossil fuels such as coal and oil: Cyprus, Croatia, Denmark, Slovenia, Estonia, Greece, Malta, Poland, Portugal and the Czech Republic.
References
- Hansen, J.; Sato, M.; Kharecha, P.; Russell, G.; Lea, D.W.; Siddall, M. Climate change and trace gases. Philos. Trans. R. Soc. Eng. Sci. 2007, 365, 1925–1954. [Google Scholar] [CrossRef] [PubMed]
- Harlem-Brundtland, G. Our Common Future. Brundtland Commission on Environment Development Report. 1987. Available online: http://www.ecominga.uqam.ca/PDF/BIBLIOGRAPHIE/GUIDE_LECTURE_1/CMMAD-Informe-Comision-Brundtland-sobre-Medio-Ambiente-Desarrollo.pdf (accessed on 1 June 2019).
- United Nations Conference on Environment and Development. Rio Declaration on Environment and Development. 1992. Available online: http://www.unesco.org/education/pdf/RIO_S.PDF (accessed on 1 June 2019).
- United Nations Framework Convention on Climate Change (UNFCCC). Kyoto Protocol. 1997. Available online: https://unfccc.int/resource/docs/convkp/kpspan.pdf (accessed on 1 June 2019).
- United Nations Framework Convention on Climate Change (UNFCCC). Adoption of the Paris Agreement. 2015. Available online: https://unfccc.int/resource/docs/2015/cop21/spa/l09s.pdf (accessed on 1 June 2019).
- World Business Council for Sustainable Development (WBCSD). Eco-Efficiency. 1992. Available online: https://www.sciencedirect.com/topics/engineering/world-business-council-for-sustainable-development (accessed on 1 June 2019).
- Unión Europea (UE). Paquete de Medidas Sobre Clima y Energía Hasta 2020. 2007. Available online: https://ec.europa.eu/clima/policies/strategies/2020_es (accessed on 1 June 2019).
- Lundgren, T.; Zhou, W.C. Firm performance and the role of environmental management. J. Environ. Manag. 2017, 203, 330–341. [Google Scholar] [CrossRef] [PubMed]
- Charnes, A.; Cooper, W.W.; Rhodes, E. Measuring the efficiency of decisión making units. Eur. J. Oper. Res. 1978, 2, 429–444. [Google Scholar] [CrossRef]
- Cook, W.D.; Seiford, L.M. Data envelopment analysis (DEA)—Thirty years on. Elsevier. Eur. J. Oper. Res. 2009, 192, 1–17. [Google Scholar] [CrossRef]
- Cook, W.D.; Zhu, J. Data Envelopment Analysis: Modeling Operational Processes and Measuring Productivity; 2008; ISBN-10: 1434830233. [Google Scholar]
- Charnes, A.; Cooper, W.W.; Golany, B.; Seiford, L.; Stutz, J. Foundations of data envelopment analysis and Pareto-Koopmans empirical production functions. J. Econ. 1985, 30, 91–107. [Google Scholar] [CrossRef]
- Banker, R.D.; Charnes, A.; Cooper, W.W. Some models for estimating technical and scale inefficiencies in data development analysis. Manag. Sci. 1984, 30, 1078–1092. [Google Scholar] [CrossRef]
- Green, R.H.; Cook, W.; Doyle, J. A note on the additive data envelopment analysis model. J. Oper. Res. Soc. 1997, 48, 446–448. [Google Scholar] [CrossRef]
- Fare, R.; Lovell, C. Measuring the technical efficiency of production. J. Econ. Theory 1978, 19, 150–162. [Google Scholar] [CrossRef]
- Fare, R.; Lovell, C. Measuring the technical efficiency of production-Reply. J. Econ. Theory 1981, 25, 453–454. [Google Scholar] [CrossRef]
- Pastor, J.T.; Ruiz, J.l.; Sirvent, I. An enhanced DEA Russel graph efficiency measure. Eur. J. Oper. Res. 1999, 115, 596–607. [Google Scholar] [CrossRef]
- Cooper, W.W.; Park, K.S.; Yu, G. IDEA and AR-IDEA: Models for dealing with imprecise data in DEA. Manag. Sci. 1999, 45, 597–607. [Google Scholar] [CrossRef]
- De Clercq, D.; Wen, Z.; Caicedo Luis Caicedo, L.; Cao, X.; Fan, F.; Xu, R.F. Application of DEA and statistical inference to model the determinants of biomethane production efficiency: A case study in south China. Appl. Energy 2017, 205, 1231–1243. [Google Scholar] [CrossRef]
- Chen, W.; Zhou, K.L.; Yang, S.L. Evaluation of China’s electric energy efficiency under environmental constraints: A DEA cross efficiency model based on game relationship. J. Clean. Prod. 2017, 164, 38–44. [Google Scholar] [CrossRef]
- Zhang, B.; Lu, D.; He, Y.; Chiu, Y.H. The efficiencies of resource-saving and environment: A case study based on Chinese cities. Energy 2018, 150, 493–507. [Google Scholar] [CrossRef]
- Lee, P.; Park, Y.J. Eco-Efficiency Evaluation Considering Environmental Stringency. Sustainability 2017, 9, 661. [Google Scholar] [CrossRef]
- Yu, S.H.; Gao, Y.; Shiue, Y.C. A Comprehensive Evaluation of Sustainable Development Ability and Pathway for Major Cities in China. Sustainability 2017, 9, 1483. [Google Scholar] [CrossRef]
- Mardani, A.; Streimikiene, D.; Balezentis, T.; Saman, M.Z.M.; Nor, K.M.; Khoshnava, S.M. Data Envelopment Analysis in Energy and Environmental Economics: An Overview of the State-of-the-Art and Recent Development Trends. Energies 2018, 11, 2002. [Google Scholar] [CrossRef]
- Wu, X.H.; Tan, L.; Guo, J.; Wang, Y.Y.; Liu, H.; Zhu, W.W. A study of allocative efficiency of PM2.5 emission rights based on a zero sum gains data envelopment model. J. Clean. Prod. 2015, 113, 1024–1031. [Google Scholar] [CrossRef]
- Feng, C.; Wang, M. Analysis of energy efficiency and energy savings potential in China’s provincial industrial sectors. J. Clean. Prod. 2017, 164, 1531–1541. [Google Scholar] [CrossRef]
- National Statistics Institute of Spain (INE). 2018. Available online: http://www.ine.es/ (accessed on 1 June 2019).
- National Statistics Institute of Germany (Statistisches Bundesamt). 2018. Available online: https://www.destatis.de/DE/Startseite.html (accessed on 1 June 2019).
- National Institute of Statistics Statistics of France (INSEE). 2018. Available online: https://www.insee.fr/fr/statistiques (accessed on 1 June 2019).
- European Statistical Office (Eurostat, the Statistical Office of the European Communities). 2018. Available online: https://ec.europa.eu/eurostat/publications/statistical-reports (accessed on 1 June 2019).
- International Monetary Fund (IMF). 2018. Available online: http://www.imf.org/en/publications (accessed on 1 June 2019).
- World Bank. 2018. Available online: https://datos.bancomundial.org/indicador (accessed on 1 June 2019).
- World Trade Organization. 2018. Available online: https://www.wto.org/english/res_e/statis_e/statis_e.htm (accessed on 1 June 2019).
- European Central Bank (ECB). 2018. Available online: https://www.ecb.europa.eu/stats/html/index.en.html (accessed on 1 June 2019).
- Bank of Spain. 2018. Available online: https://www.bde.es/bde/es/areas/estadis/ (accessed on 1 June 2019).
- Institute National Statistical Office of Italy (Istat, L’Istituto nazionale di statistica). 2018. Available online: https://www4.istat.it/it/istituto-nazionale-di-statistica (accessed on 1 June 2019).
- Central Bank of England (Bank of England). 2018. Available online: https://www.bankofengland.co.uk/news/statistics (accessed on 1 June 2019).
- Tyteca, D. Linear programming models for the measurement of environmental performance of firms-Concepts and empirical results. J. Product. Anal. 1997, 8, 183–197. [Google Scholar] [CrossRef]
- European Economic Community. Tratado Constitutivo de la Comunidad Económica Europea. 1957. Available online: https://eur-lex.europa.eu/legal-content/ES/TXT/HTML/?uri=CELEX:11957E&from=ES (accessed on 1 June 2019).
- Claver, E.; Molina, J.F.; Tari, J.J. Quality Management and Environmental Management; Editorial Pirámide: Madrid, Spain, 2011; ISBN 978-84-368-2458-2. [Google Scholar]
- Wen, M.L.; Zhang, Q.Y.; Kang, R.; Yang, Y. Some new ranking criteria in data envelopment analysis under uncertain environment. Comput. Ind. Eng. 2017, 110, 498–504. [Google Scholar] [CrossRef]
- Yang, J.; Li, X.G.; Zhou, Z.X. A Cross-efficiency data envelopment analysis (DEA) based model for measuring environmental performance. Environ. Eng. Manag. J. 2014, 13, 1139–1146. [Google Scholar]
- Zhou, P.; Poh, K.L.; Ang, B.W. Data Envelopment Analysis for Measuring Environmental Performance. In Handbook of Operations Analytics Using Data Envelopment Analysis; Springer: Boston, MA, USA, 2016; pp. 31–49. [Google Scholar]
- European Union. A Healthy and Sustainable Environment for Generations to Come. 2014. Available online: https://publications.europa.eu/es/publication-detail/-/publication/3456359b-4cb4-4a6e-9586-6b9846931463 (accessed on 1 June 2019).
- Gen, Z.Q.; Dong, J.G.; Han, Y.M.; Zhu, Q.X. Energy and environment efficiency analysis based on an improved environment DEA cross-model: Case study of complex chemical processes. Appl. Energy 2017, 205, 465–476. [Google Scholar] [CrossRef]


| Indicator | Unity | Maximum | Mínimum | Average | Typical Deviation | |
|---|---|---|---|---|---|---|
| Inputs | Generation of Electricity from Coal | % | 95,709 | 0 | 26,918 | 25,028 |
| Generation of Electricity from Petroleum | % | 100 | 0,016 | 10,005 | 25,127 | |
| Generation of Electricity from Nuclear Energy | % | 79,512 | 0 | 18,924 | 23,105 | |
| Industrial Production Index | % | 22,842 | −23,442 | 1085 | 7421 | |
| Traffic Volume | Vehículos | 49,742,000 | 252,000 | 9,805,492,103 | 1,362,752,668 | |
| Outputs Deseables | PIB (GDP) | Millones de € | 2,758,260 | 5142 | 454236,517 | 688,213,992 |
| Rent per Cápita | € | 83,100 | 3100 | 23768,5803 | 15,550,584 | |
| Outputs Indeseables | CO2 Emissions | Kts | 844,435 | 2432 | 142,200,227 | 190,781,005 |
| CH4 Emissions | Kt de Equivalente de CO2 | 837,569 | 1,409,427 | 18,849,737 | 22,216,665 |
| 2005 | 2006 | 2007 | 2008 | 2009 | 2010 | 2011 | 2012 | |
|---|---|---|---|---|---|---|---|---|
| Germany | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Austria | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Belgium | 1 | 1 | 0.91974 | 0.90943 | 1 | 1 | 0.91808 | 0.9181 |
| Bulgaria | 0.19318 | 0.19312 | 0.18073 | 0.19388 | 0.25535 | 1 | 0.19434 | 0.58077 |
| Cyprus | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Croatia | 0.55502 | 0.57879 | 0.51608 | 0.54401 | 0.55786 | 1 | 1 | 1 |
| Denmark | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Slovakia | 1 | 0.34476 | 0.3937 | 0.44765 | 0.4817 | 0.49112 | 0.45281 | 0.43873 |
| Slovenia | 0.64194 | 0.62028 | 0.6022 | 0.57549 | 0.69106 | 1 | 0.80081 | 0.6149 |
| Spain | 0.78143 | 0.78112 | 0.77369 | 0.84181 | 1 | 1 | 0.81044 | 1 |
| Estonia | 0.63667 | 0.68943 | 0.53955 | 0.57273 | 1 | 0.5902 | 0.56159 | 1 |
| Finland | 1 | 0.56014 | 0.57224 | 0.61133 | 0.69907 | 1 | 0.58454 | 0.55831 |
| France | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Greece | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0.54241 |
| Hungary | 0.39984 | 0.38055 | 0.40296 | 0.42662 | 0.49486 | 0.39354 | 0.36761 | 0.34726 |
| Ireland | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Italy | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Latvia | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Lithuania | 1 | 1 | 0.63402 | 1 | 1 | 1 | 1 | 1 |
| Luxembourg | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Malt | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Netherlands | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Poland | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Portugal | 0.59589 | 1 | 1 | 1 | 0.70056 | 1 | 1 | 1 |
| UK | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Czech Republic | 0.28101 | 0.29438 | 0.30624 | 0.3564 | 0.35152 | 0.38802 | 0.33825 | 0.3137 |
| Romania | 1 | 0.20547 | 0.22566 | 0.23233 | 0.30254 | 1 | 0.23183 | 0.22111 |
| Sweden | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Method 1 | |
|---|---|
| Germany | 1 |
| Austria | 1 |
| Belgium | 0.95817 |
| Bulgaria | 0.34892 |
| Cyprus | 1 |
| Croatia | 0.71897 |
| Denmark | 1 |
| Slovakia | 0.50631 |
| Slovenia | 0.69333 |
| Spain | 0.87356 |
| Estonia | 0.69877 |
| Finland | 0.6982 |
| France | 1 |
| Greece | 0.9428 |
| Hungary | 0.40165 |
| Ireland | 1 |
| Italy | 1 |
| Latvia | 1 |
| Lithuania | 0.95425 |
| Luxembourg | 1 |
| Malt | 1 |
| Netherlands | 1 |
| Poland | 1 |
| Portugal | 0.91205 |
| UK | 1 |
| Czech Republic | 0.32869 |
| Romania | 0.42737 |
| Sweden | 1 |
| Method 2 | 2005 | 2006 | 2007 | 2008 | 2009 | 2010 | 2011 | 2012 |
|---|---|---|---|---|---|---|---|---|
| Germany | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Austria | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Belgium | 0.98893 | 0.95715 | 0.8916 | 0.87118 | 0.94409 | 0.88882 | 0.8871 | 0.88412 |
| Bulgaria | 0.08567 | 0.08791 | 0.09166 | 0.09607 | 0.16249 | 1 | 0.10569 | 0.10154 |
| Cyprus | 0.91388 | 1 | 0.92983 | 0.97352 | 1 | 1 | 1 | 1 |
| Croatia | 0.27692 | 0.26546 | 0.26547 | 0.28589 | 0.29653 | 1 | 1 | 0.38745 |
| Denmark | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Slovakia | 1 | 0.33362 | 0.38596 | 0.44111 | 0.47296 | 0.49098 | 0.44371 | 0.42889 |
| Slovenia | 0.36179 | 0.36746 | 0.38247 | 0.39883 | 0.57175 | 1 | 0.43572 | 0.42127 |
| Spain | 0.74073 | 0.70968 | 0.71636 | 0.70626 | 1 | 1 | 0.64551 | 1 |
| Estonia | 0.48067 | 0.48782 | 0.49582 | 0.50994 | 1 | 0.53298 | 0.54701 | 0.51749 |
| Finland | 1 | 0.5064 | 0.49674 | 0.50087 | 0.68142 | 1 | 0.54721 | 0.51542 |
| France | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Greece | 1 | 1 | 0.68915 | 0.7002 | 0.70334 | 1 | 1 | 0.52688 |
| Hungary | 0.34526 | 0.3281 | 0.35654 | 0.37236 | 0.49103 | 0.35305 | 0.34391 | 0.32601 |
| Ireland | 0.43603 | 1 | 1 | 0.41988 | 0.39115 | 0.37938 | 1 | 0.35505 |
| Italy | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Latvia | 0.3715 | 0.36776 | 0.37698 | 0.3866 | 1 | 1 | 1 | 1 |
| Lithuania | 0.22283 | 0.2231 | 0.22836 | 0.22883 | 0.25729 | 1 | 0.25265 | 0.24597 |
| Luxembourg | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Malt | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Netherlands | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Poland | 1 | 1 | 1 | 1 | 0.12434 | 0.13739 | 0.13727 | 1 |
| Portugal | 0.34108 | 1 | 0.42332 | 0.34787 | 0.36575 | 0.54239 | 0.47781 | 1 |
| UK | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Czech Republic | 0.26539 | 0.27826 | 0.29203 | 0.33118 | 0.33438 | 0.37275 | 0.32031 | 0.2987 |
| Romania | 1 | 1 | 0.12978 | 0.14137 | 0.13375 | 0.18142 | 0.12784 | 0.1234 |
| Sweden | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Méthod 2 | |
|---|---|
| Germany | 1 |
| Austria | 1 |
| Belgium | 0.91412 |
| Bulgaria | 0.21638 |
| Cyprus | 0.97715 |
| Croatia | 0.47221 |
| Denmark | 1 |
| Slovakia | 0.49965 |
| Slovenia | 0.49241 |
| Spain | 0.81481 |
| Estonia | 0.57147 |
| Finland | 0.65601 |
| France | 1 |
| Greece | 0.82744 |
| Hungary | 0.36453 |
| Ireland | 0.62268 |
| Italy | 1 |
| Letonia | 0.68785 |
| Lituania | 0.33238 |
| Luxemburgo | 1 |
| Malta | 1 |
| Países Bajos | 1 |
| Polonia | 0.67487 |
| Portugal | 0.56228 |
| Reino Unido | 1 |
| República Checa | 0.31162 |
| Rumanía | 0.35469 |
| Suecia | 1 |
| 2005 | 2006 | 2007 | 2008 | |||||
|---|---|---|---|---|---|---|---|---|
| Method 1 | Method 2 | Method 1 | Method 2 | Method 1 | Method 2 | Method 1 | Method 2 | |
| Germany | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Austria | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Belgium | 1 | 0.98893 | 1 | 0.95715 | 0.91974 | 0.8916 | 0.90943 | 0.87118 |
| Bulgaria | 0.19318 | 0.08567 | 0.19312 | 0.08791 | 0.18073 | 0.09166 | 0.19388 | 0.09607 |
| Cyprus | 1 | 0.91388 | 1 | 1 | 1 | 0.92983 | 1 | 0.97352 |
| Croatia | 0.55502 | 0.27692 | 0.57879 | 0.26546 | 0.51608 | 0.26547 | 0.54401 | 0.28589 |
| Denmark | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Slovakia | 1 | 1 | 0.34476 | 0.33362 | 0.3937 | 0.38596 | 0.44765 | 0.44111 |
| Slovenia | 0.64194 | 0.36179 | 0.62028 | 0.36746 | 0.6022 | 0.38247 | 0.57549 | 0.39883 |
| Spain | 0.78143 | 0.74073 | 0.78112 | 0.70968 | 0.77369 | 0.71636 | 0.84181 | 0.70626 |
| Estonia | 0.63667 | 0.48067 | 0.68943 | 0.48782 | 0.53955 | 0.49582 | 0.57273 | 0.50994 |
| Finland | 1 | 1 | 0.56014 | 0.5064 | 0.57224 | 0.49674 | 0.61133 | 0.50087 |
| France | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Greece | 1 | 1 | 1 | 1 | 1 | 0.68915 | 1 | 0.7002 |
| Hungary | 0.39984 | 0.34526 | 0.38055 | 0.3281 | 0.40296 | 0.35654 | 0.42662 | 0.37236 |
| Ireland | 1 | 0.43603 | 1 | 1 | 1 | 1 | 1 | 0.41988 |
| Italy | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Latvia | 1 | 0.3715 | 1 | 0.36776 | 1 | 0.37698 | 1 | 0.3866 |
| Lithuania | 1 | 0.22283 | 1 | 0.2231 | 0.63402 | 0.22836 | 1 | 0.22883 |
| Luxembourg | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Malt | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Netherlands | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Poland | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Portugal | 0.59589 | 0.34108 | 1 | 1 | 1 | 0.42332 | 1 | 0.34787 |
| UK | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Czech Republic | 0.28101 | 0.26539 | 0.29438 | 0.27826 | 0.30624 | 0.29203 | 0.3564 | 0.33118 |
| Romania | 1 | 1 | 0.20547 | 1 | 0.22566 | 0.12978 | 0.23233 | 0.14137 |
| Sweden | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 2009 | 2010 | 2011 | 2012 | |||||
|---|---|---|---|---|---|---|---|---|
| Method 1 | Method 2 | Method 1 | Method 2 | Method 1 | Method 2 | Method 1 | Method 2 | |
| Germany | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Austria | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Belgium | 1 | 0.94409 | 1 | 0.88882 | 0.91808 | 0.8871 | 0.90943 | 0.88412 |
| Bulgaria | 0.25535 | 0.16249 | 1 | 1 | 0.19434 | 0.10569 | 0.19388 | 0.10154 |
| Cyprus | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Croatia | 0.55786 | 0.29653 | 1 | 1 | 1 | 1 | 0.54401 | 0.38745 |
| Denmark | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Slovakia | 0.4817 | 0.47296 | 0.49112 | 0.49098 | 0.45281 | 0.44371 | 0.44765 | 0.42889 |
| Slovenia | 0.69106 | 0.57175 | 1 | 1 | 0.80081 | 0.43572 | 0.57549 | 0.42127 |
| Spain | 1 | 1 | 1 | 1 | 0.81044 | 0.64551 | 0.84181 | 1 |
| Estonia | 1 | 1 | 0.5902 | 0.53298 | 0.56159 | 0.54701 | 0.57273 | 0.51749 |
| Finland | 0.69907 | 0.68142 | 1 | 1 | 0.58454 | 0.54721 | 0.61133 | 0.51542 |
| France | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Greece | 1 | 0.70334 | 1 | 1 | 1 | 1 | 1 | 0.52688 |
| Hungary | 0.49486 | 0.49103 | 0.39354 | 0.35305 | 0.36761 | 0.34391 | 0.42662 | 0.32601 |
| Ireland | 1 | 0.39115 | 1 | 0.37938 | 1 | 1 | 1 | 0.35505 |
| Italy | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Latvia | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Lithuania | 1 | 0.25729 | 1 | 1 | 1 | 0.25265 | 1 | 0.24597 |
| Luxembourg | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Malt | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Netherlands | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Poland | 1 | 0.12434 | 1 | 0.13739 | 1 | 0.13727 | 1 | 1 |
| Portugal | 0.70056 | 0.36575 | 1 | 0.54239 | 1 | 0.47781 | 1 | 1 |
| UK | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Czech Republic | 0.35152 | 0.33438 | 0.38802 | 0.37275 | 0.33825 | 0.32031 | 0.3564 | 0.2987 |
| Romania | 0.30254 | 0.13375 | 1 | 0.18142 | 0.23183 | 0.12784 | 0.23233 | 0.1234 |
| Sweden | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Method 1 | Method 2 | |
|---|---|---|
| Germany | 1 | 1 |
| Austria | 1 | 1 |
| Belgium | 0.95817 | 0.91412 |
| Bulgaria | 0.34892 | 0.21638 |
| Cyprus | 1 | 0.97715 |
| Croatia | 0.71897 | 0.47221 |
| Denmark | 1 | 1 |
| Slovakia | 0.50631 | 0.49965 |
| Slovenia | 0.69333 | 0.49241 |
| Spain | 0.87356 | 0.81481 |
| Estonia | 0.69877 | 0.57147 |
| Finland | 0.6982 | 0.65601 |
| France | 1 | 1 |
| Greece | 0.9428 | 0.82744 |
| Hungary | 0.40165 | 0.36453 |
| Ireland | 1 | 0.62268 |
| Italy | 1 | 1 |
| Latvia | 1 | 0.68785 |
| Lithuania | 0.95425 | 0.33238 |
| Luxembourg | 1 | 1 |
| Malt | 1 | 1 |
| Netherlands | 1 | 1 |
| Poland | 1 | 0.67487 |
| Portugal | 0.91205 | 0.56228 |
| UK | 1 | 1 |
| Czech Republic | 0.32869 | 0.31162 |
| Romania | 0.42737 | 0.35469 |
| Sweden | 1 | 1 |
| Method 2 | Method 1 | ||
|---|---|---|---|
| Germany | 1 | Germany | 1 |
| Austria | 1 | Austria | 1 |
| France | 1 | Denmark | 1 |
| Italy | 1 | Netherland | 1 |
| Luxemburg | 1 | France | 1 |
| Malt | 1 | Sweden | 1 |
| Netherland | 1 | UK | 1 |
| UK | 1 | Malt | 1 |
| Sweden | 1 | Ireland | 1 |
| Denmark | 1 | Cyprus | 1 |
| Cyprus | 0.97715 | Poland | 1 |
| Bélgium | 0.91412 | Italy | 1 |
| Greece | 0.82744 | Luxemburg | 1 |
| Spain | 0.81481 | Latvia | 1 |
| Letonia | 0.68785 | Bélgiium | 0.95817 |
| Polond | 0.67487 | Lithuania | 0.95425 |
| Finland | 0.65601 | Greece | 0.9428 |
| Ireland | 0.62268 | Portugal | 0.91205 |
| Estonia | 0.57147 | Spain | 0.87356 |
| Portugal | 0.56228 | Croatia | 0.71897 |
| Slovakia | 0.49965 | Estonia | 0.69877 |
| Slovenia | 0.49241 | Finland | 0.6982 |
| Croatia | 0.47221 | Slovenia | 0.69333 |
| Hungry | 0.36453 | Slovakia | 0.50631 |
| Romanía | 0.35469 | Romanía | 0.42727 |
| Lithuania | 0.33238 | Hungry | 0.40165 |
| Czech Republic | 0.31162 | Bulgaria | 0.34892 |
| Bulgaria | 0.21638 | Czech Republic | 0.32869 |
| Method 1 | Méethod 2 | Average | Total Average European Union | ||
|---|---|---|---|---|---|
| 2005 | 0.86017851 | 0.74395426 | 0.80206639 | 0.78242436 | |
| 2006 | 0.80886046 | 0.74688476 | 0.77787261 | ||
| 2007 | 0.78810251 | 0.68400509 | 0.7360538 | ||
| 2008 | 0.81113359 | 0.6682874 | 0.7397105 | ||
| 2009 | 0.84051984 | 0.71179776 | 0.7761588 | ||
| 2010 | 0.92367475 | 0.81711428 | 0.87039451 | ||
| 2011 | 0.83072642 | 0.72399294 | 0.77735968 | ||
| 2012 | 0.84054784 | 0.71900935 | 0.7797786 | ||
| Method 1 | Method 2 | Average | |
|---|---|---|---|
| Germany | 1 | 1 | 1 |
| Austria | 1 | 1 | 1 |
| Netherland | 1 | 1 | 1 |
| Denmark | 1 | 1 | 1 |
| France | 1 | 1 | 1 |
| Sweden | 1 | 1 | 1 |
| Malt | 1 | 1 | 1 |
| UK | 1 | 1 | 1 |
| Italy | 1 | 1 | 1 |
| Luxemburg | 1 | 1 | 1 |
| Cyprus | 1 | 0.977155 | 0.9885775 |
| Belgium | 0.95817106 | 0.91412867 | 0.93614987 |
| Greece | 0.94280217 | 0.82744886 | 0.88512552 |
| Espain | 0.87356326 | 0.81481902 | 0.84419114 |
| Latvia | 1 | 0.68785791 | 0.84392896 |
| Poland | 1 | 0.67487704 | 0.83743852 |
| Ireland | 1 | 0.62268811 | 0.81134406 |
| Portugal | 0.91205631 | 0.56228108 | 0.7371687 |
| Finland | 0.69820809 | 0.65601299 | 0.67711054 |
| Lithuania | 0.95425344 | 0.33238138 | 0.64331741 |
| Estonia | 0.69877384 | 0.57147078 | 0.63512231 |
| Croatia | 0.71897334 | 0.47221884 | 0.59559609 |
| Slovenia | 0.69333977 | 0.49241602 | 0.5928779 |
| Slovakia | 0.50631401 | 0.49965621 | 0.50298511 |
| Romanía | 0.42737165 | 0.35469841 | 0.39103503 |
| Hungry | 0.40165806 | 0.36453795 | 0.38309801 |
| Czech.Rep | 0.32869324 | 0.31162983 | 0.32016153 |
| Bulgaria | 0.34892545 | 0.21638236 | 0.2826539 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hermoso-Orzáez, M.J.; García-Alguacil, M.; Terrados-Cepeda, J.; Brito, P. Measurement of Environmental Efficiency in the Countries of the European Union with the Enhanced Data Envelopment Analysis Method (DEA) during the Period 2005–2012. Proceedings 2019, 38, 20. https://doi.org/10.3390/proceedings2019038020
Hermoso-Orzáez MJ, García-Alguacil M, Terrados-Cepeda J, Brito P. Measurement of Environmental Efficiency in the Countries of the European Union with the Enhanced Data Envelopment Analysis Method (DEA) during the Period 2005–2012. Proceedings. 2019; 38(1):20. https://doi.org/10.3390/proceedings2019038020
Chicago/Turabian StyleHermoso-Orzáez, Manuel Jesús, Miriam García-Alguacil, Julio Terrados-Cepeda, and Paulo Brito. 2019. "Measurement of Environmental Efficiency in the Countries of the European Union with the Enhanced Data Envelopment Analysis Method (DEA) during the Period 2005–2012" Proceedings 38, no. 1: 20. https://doi.org/10.3390/proceedings2019038020
APA StyleHermoso-Orzáez, M. J., García-Alguacil, M., Terrados-Cepeda, J., & Brito, P. (2019). Measurement of Environmental Efficiency in the Countries of the European Union with the Enhanced Data Envelopment Analysis Method (DEA) during the Period 2005–2012. Proceedings, 38(1), 20. https://doi.org/10.3390/proceedings2019038020







