Estimating Flight Characteristics of Anomalous Unidentified Aerial Vehicles in the 2004 Nimitz Encounter †
Abstract
:1. Introduction
2. Nimitz Encounters (2004)
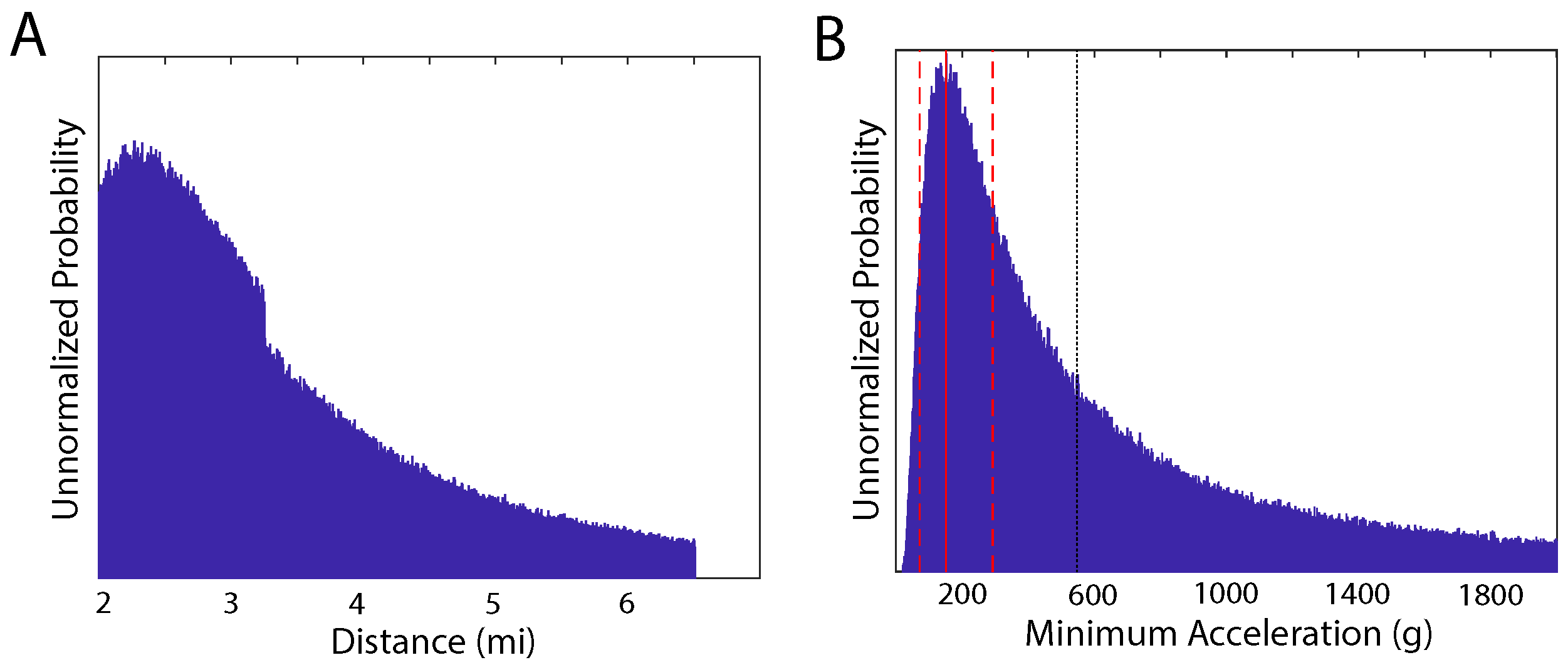
2.1. Senior Chief Operations Specialist Kevin Day (RADAR)
2.2. Commander David Fravor (PILOT)
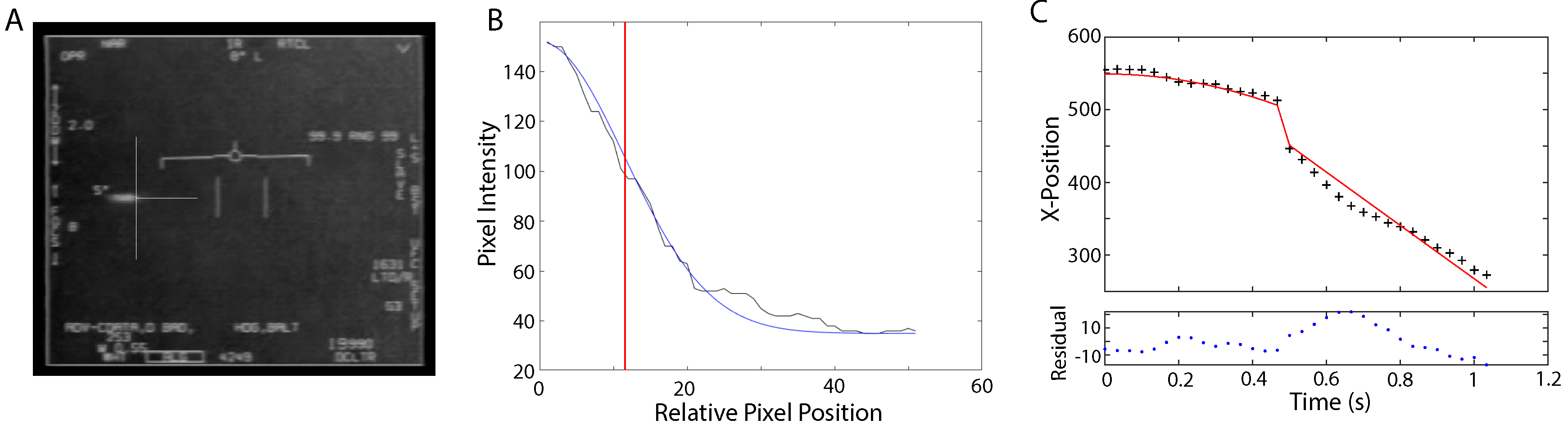
2.3. ATFLIR Video
3. Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Vallee, J.; Aubeck, C. Wonders in the Sky: Unexplained Aerial Objects from Antiquity to Modern Times; Penguin: New York, NY, USA, 2010. [Google Scholar]
- Unidentified Flying Objects and Air Force Project Blue Book. Available online: https://web.archive.org/web/20030624053806/http://www.af.mil/factsheets/factsheet.asp?fsID=188 (accessed on 9 June 2019).
- CEFAA. Comité de Estudios de Fenómenos Aéreos Anómalos. Available online: http://www.cefaa.gob.cl/ (accessed on 27 July 2019).
- Elizondo, L. The imminent change of an old paradigm: The U.S. government’s involvement in UAPs, AATIP, and TTSA. In Proceedings of the Anomalous Aerospace Phenomena Conference (AAPC 2019) Presentation, Huntsville, AL, USA, 15–17 March 2019. [Google Scholar]
- Cooper, H.; Blumenthal, R.; Kean, L. Glowing auras and “black money”: The Pentagon’s mysterious U.F.O. program. The New York Times.
- Stieb, M. Navy pilots were seeing UFOs on an almost daily basis in 2014 and 2015: Report. New York Magazine, 2019. Available online: http://nymag.com/intelligencer/2019/05/navy-pilots-are-seeing-ufos-on-an-almost-daily-basis-report.html(accessed on 24 July 2019).
- Rogoway, T. Recent UFO Encounters with Navy pilots occurred constantly across multiple squadrons. The Drive, 2019. Available online: https://www.thedrive.com/the-war-zone/28627/recent-ufo-encounters-with-navy-pilots-occurred-constantly-across-multiple-squadrons(accessed on 24 July 2019).
- Monzon, I. Tech CEOs want to capture UFOs and reverse engineer them. International Business Times, 2019. Available online: https://www.ibtimes.com/tech-ceos-want-capture-ufos-reverse-engineer-them-2803920,(accessed on 24 July 2019).
- Hynek, J.A. The UFO Experience: A Scientific Inquiry; Henry Regnery: Chicago, IL, USA, 1972. [Google Scholar]
- Hill, P.R. Unconventional Flying Objects: A Scientific Analysis; Hampton Roads Publishing Co.: Charlottesville, VA, USA, 1995. [Google Scholar]
- Sturrock, P.A. The UFO Enigma: A New Review of the Physical Evidence; Aspect: New York, NY, USA, 2000. [Google Scholar]
- Knuth, K.H. Are we alone? The question is worthy of serious scientific study. The Conversation. 2018. Available online: https://theconversation.com/are-we-alone-the-question-is-worthy-of-serious-scientific-study-98843 (accessed on 24 July 2018).
- Colombano, S.P. New Assumptions to Guide SETI Research. 2018. Available online: https://ntrs.nasa.gov/archive/nasa/casi.ntrs.nasa.gov/20180001925.pdf (accessed on 24 July 2018).
- Powell, R.; Reali, P.; Thompson, T.; Beall, M.; Kimzey, D.; Cates, L.; Hoffman, R. A Forensic Analysis of Navy Carrier Strike Group Eleven’s Encounter with an Anomalous aerial Vehicle. 2019. Available online: https://www.explorescu.org/post/nimitz_strike_group_2004 (accessed on 9 July 2018).
- Day, K. (U.S. Navy (ret.)). Private Communication, 2019. [Google Scholar]
- Palo Verde Nuclear Generating Station. Available online: https://en.wikipedia.org/wiki/Palo_Verde_Nuclear_Generating_Station (accessed on 8 Augest 2018).
- Skilling, J. Nested sampling for general Bayesian computation. Bayesian Anal. 2006, 1, 833–859. [Google Scholar] [CrossRef]
- Sivia, D.S.; Skilling, J. Data Analysis. A Bayesian Tutorial, second ed.; Oxford University Press: Oxford, 2006. [Google Scholar]
- 2004 Nimitz Pilot Report. 2017. Available online: https://thevault.tothestarsacademy.com/nimitz-report (accessed on 7 October 2018).
- Knuth, K.H.; Powell, R.M.; Reali, P.A. Estimating Flight Characteristics of Anomalous Unidentified Aerial Vehicles. Entropy 2019, 21, 939. [Google Scholar] [CrossRef]
- Eiband, A.M. Human Tolerance to Rapidly Applied Accelerations: A Summary of the Literature. 1959. Available online: https://ntrs.nasa.gov/archive/nasa/casi.ntrs.nasa.gov/19980228043.pdf (accessed on 27 July 2019).
- Kent, J. F-35 Lightning II News. 2010. Available online: http://www.f-16.net/f-35-news-article4113.html (accessed on 27 July 2019).
- Army-Technology.com. Crotale NG Short Range Air Defence System. Available online: https://www.army-technology.com/projects/crotale/ (accessed on 27 July 2019).
- Wright, J. Searches for technosignatures: The state of the profession. arXiv 2019, arXiv:1907.07832. [Google Scholar]
- Bracewell, R. Communications from superior galactic communities. Nature 1960, 186, 670–671. [Google Scholar] [CrossRef]
- Bracewell, R. Interstellar probes. In Interstellar Communication: Scientific Perspectives; Ponnamperuma, C., Cameron, A.G.W., Eds.; Houghton-Mifflin: Boston, MA, USA, 1974; pp. 141–167. [Google Scholar]
- Freitas, R.A., Jr. The search for extraterrestrial artifacts (SETA). J. Br. Interplanet. Soc. 1983, 36, 501–506. [Google Scholar] [CrossRef]
- Tough, A.; Lemarchand, G. Searching for extraterrestrial technologies within our solar system. In Symposium-International Astronomical Union; Cambridge University Press: Cambridge, UK, 2004; Volume 213, pp. 487–490. [Google Scholar]
- Haqq-Misra, J.; Kopparapu, R. On the likelihood of non-terrestrial artifacts in the Solar System. Acta Astronaut. 2012, 72, 15–20. [Google Scholar] [CrossRef]
- Kecskes, C. Observation of asteroids for searching extraterrestrial artifacts. In Asteroids; Badescu, V., Ed.; Springer: Berlin/Heidelberg, Germany, 2013; pp. 633–644. [Google Scholar]
- Haines, R.F. Aviation Safety in America: A Previously Neglected Factor; NARCAP TR 01-2000; National Aviation Reporting Center on Anomalous Phenomena (NARCAP). 2000. Available online: http://www.noufors.com/Documents/narcap.pdf (accessed on 27 July 2019).
- Bender, B.P. Senators Get Classified Briefing on UFO Sightings. Available online: https://www.politico.com/story/2019/06/19/warner-classified-briefing-ufos-1544273 (accessed on 27 July 2019).
- Golgowski, N.H. Congress Briefed on Classified UFO Sightings as Threat to Aviator Safety, Navy Says. Available online: https://www.huffpost.com/entry/navy-briefs-congress-ufos_n_5d0baf79e4b06ad4d25cf1be (accessed on 27 July 2019).
- Lutz, E.V.F. Congress Is Taking the UFO Threat Seriously. Available online: https://www.vanityfair.com/news/2019/06/congress-is-taking-the-ufo-threat-seriously (accessed on 27 July 2019).

| Model | logZ | LogL | ||||
|---|---|---|---|---|---|---|
| Model 1 | – | |||||
| Model 2 | ||||||
| Model 3 | – | |||||
| Model 4 |
| Case | Detection Modalities | Kinematic Model | Figure | Min. Acceleration |
|---|---|---|---|---|
| Day | R | (1) | Figure 1B | 5370 |
| Fravor | R,Vs | (7) | Figure 2C | 150 |
| ATFLIR | R,Vs,IR | (11) | Figure 3C |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Knuth, K.H.; Powell, R.M.; Reali, a.P.A. Estimating Flight Characteristics of Anomalous Unidentified Aerial Vehicles in the 2004 Nimitz Encounter. Proceedings 2019, 33, 26. https://doi.org/10.3390/proceedings2019033026
Knuth KH, Powell RM, Reali aPA. Estimating Flight Characteristics of Anomalous Unidentified Aerial Vehicles in the 2004 Nimitz Encounter. Proceedings. 2019; 33(1):26. https://doi.org/10.3390/proceedings2019033026
Chicago/Turabian StyleKnuth, Kevin H., Robert M. Powell, and and Peter A. Reali. 2019. "Estimating Flight Characteristics of Anomalous Unidentified Aerial Vehicles in the 2004 Nimitz Encounter" Proceedings 33, no. 1: 26. https://doi.org/10.3390/proceedings2019033026
APA StyleKnuth, K. H., Powell, R. M., & Reali, a. P. A. (2019). Estimating Flight Characteristics of Anomalous Unidentified Aerial Vehicles in the 2004 Nimitz Encounter. Proceedings, 33(1), 26. https://doi.org/10.3390/proceedings2019033026






