Applying a Two-Layer Quadrupole Model for Quantitative Thermography at Overload-Induced Delaminations in GFRP †
Abstract
:1. Introduction
| Thermal and Material Property | Value (Room Temperature) | Measurement Method [8] |
|---|---|---|
| thermal diffusivity (in-depth) | calculated | |
| thermal conductivity (in-depth) | hot disk | |
| heat capacity | adiabatic calorimeter | |
| density | dimensions and weight |
2. Materials and Methods
2.1. Specimen
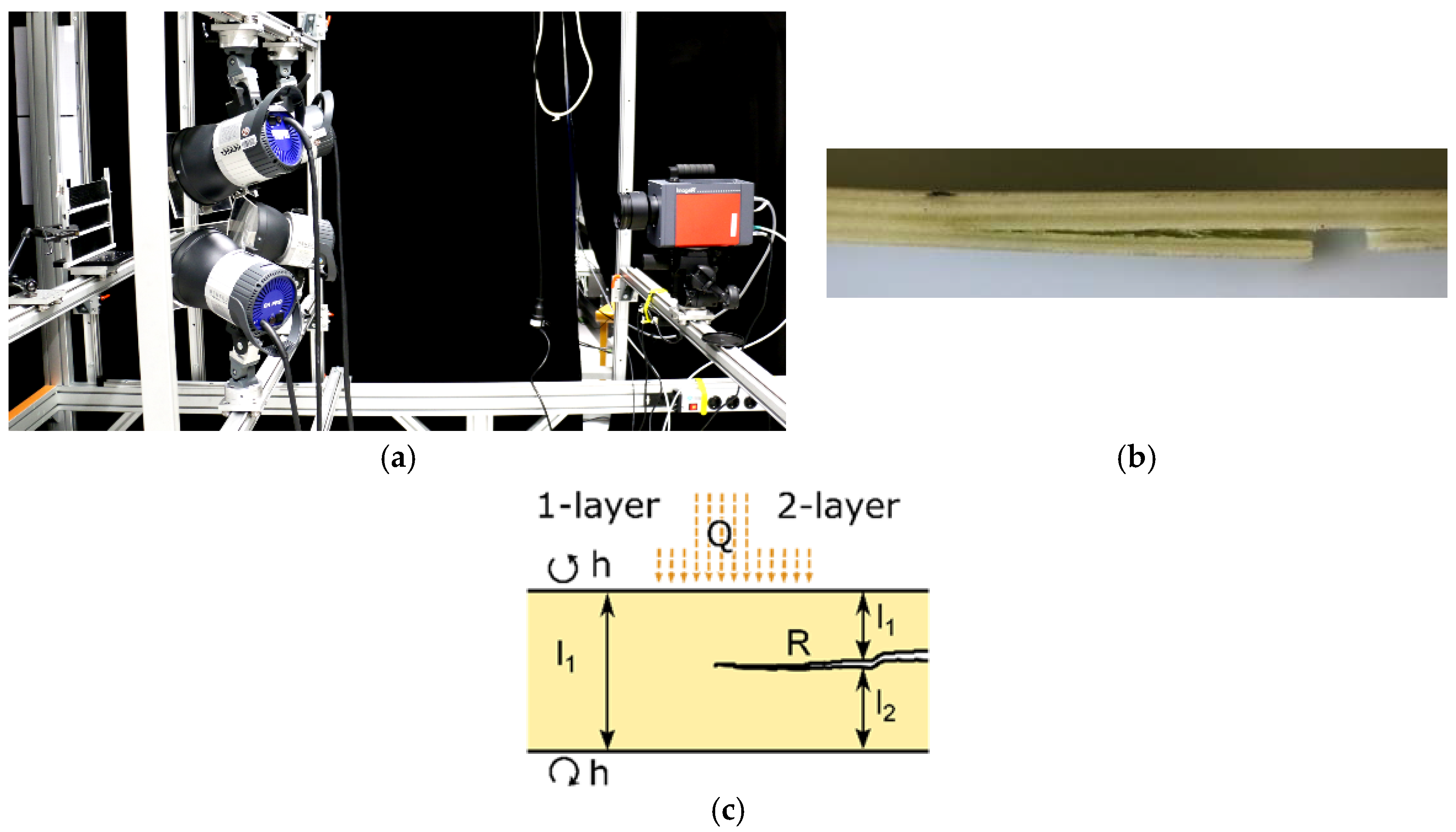
2.2. Thermographic Setup and Evaluation of Data
3. Results and Discussion
4. Conclusions
Funding
Conflicts of Interest
References
- Ibarra-Castanedo, C.; Maldague, X. Pulsed phase thermography reviewed. QIRT. J. 2004, 1, 47–70. [Google Scholar] [CrossRef]
- Shepard, S.M.; Lhota, J.R.; Rubadeux, B.A.; Wang, D.; Ahmed, T. Reconstruction and enhancement of active thermographic image sequences. Opt. Eng. 2003, 42, 1337–1342. [Google Scholar] [CrossRef]
- Oswald-Tranta, B. Comparison of time and frequency behavior in TSR and PPT evaluation. In Proceedings of the SPIE Commercial + Scientific Sensing and Imaging 2016, Baltimore, MY, USA, 17–21 April 2016; pp. 1–13. [Google Scholar]
- Balageas, D.; Roche, J.-M. Taking into account heat losses in front-face pulse IR thermography experiment for thermal diffusivity identification. In Proceedings of the 14th Conf. QIRT, Berlin, Germany, 25–29 June 2018. [Google Scholar]
- Maillet, D.; André, S.; Batsale, J.-C. Thermal Quadrupoles; Wiley: Hoboken, NJ, USA, 2000. [Google Scholar]
- Müller, J.P.; Krankenhagen, R. Optimizing thermographic testing of thick GFRP plates by assessing the real energy absorbed within the material. Comp. Struct. 2019, 215, 60–68. [Google Scholar] [CrossRef]
- Feuillet, V.; Ibos, L.; Fois, M.; Dumoulin, J.; Candau, Y. Defect detection and characterization in composite materials using square pulse thermography coupled with singular value decomposition analysis and thermal quadrupole modeling. NDT E Int. 2012, 51, 58–67. [Google Scholar] [CrossRef]
- Gower, M. EMRP Project ENG 57, Final Report. Available online: https://www.euramet.org/research-innovation/search-research-projects/details/?eurametCtcp_project_show%5Bproject%5D=1266 (accessed on 27 May 2019).
- Maierhofer, C.; Krankenhagen, R.; Röllig, M.; Rehmer, B.; Gower, M.; Baker, G.; Lodeiro, M.; Aktas, A.; Monte, C.; Adibekyan, A.; et al. Defect characterisation of tensile loaded CFRP and GFRP laminates used in energy applications by means of infrared thermography. QIRT. J. 2018, 15, 17–36. [Google Scholar] [CrossRef]
- Krankenhagen, R.; Worzewski, T.; Maierhofer, C. Cooling-down of thermal thick probes after flash excitation—A measure for the real energy density? Infrared Phys. Techn. 2015, 72, 258–265. [Google Scholar] [CrossRef]
- Maldague, X.; Marinetti, S. Pulse phase infrared thermography. J. Appl. Phys. 1996, 79, 2694. [Google Scholar] [CrossRef]
- Bernegger, R.; Altenburg, S.J.; Röllig, M.; Maierhofer, C. Applicability of a 1D analytical model for pulse thermography of laterally heterogeneous semitransparent materials. Int. J. Thermophys 2018, 39, 1–17. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Müller, J.P.; Dell’Avvocato, G.; Krankenhagen, R. Applying a Two-Layer Quadrupole Model for Quantitative Thermography at Overload-Induced Delaminations in GFRP. Proceedings 2019, 27, 40. https://doi.org/10.3390/proceedings2019027040
Müller JP, Dell’Avvocato G, Krankenhagen R. Applying a Two-Layer Quadrupole Model for Quantitative Thermography at Overload-Induced Delaminations in GFRP. Proceedings. 2019; 27(1):40. https://doi.org/10.3390/proceedings2019027040
Chicago/Turabian StyleMüller, Jan P., Giuseppe Dell’Avvocato, and Rainer Krankenhagen. 2019. "Applying a Two-Layer Quadrupole Model for Quantitative Thermography at Overload-Induced Delaminations in GFRP" Proceedings 27, no. 1: 40. https://doi.org/10.3390/proceedings2019027040
APA StyleMüller, J. P., Dell’Avvocato, G., & Krankenhagen, R. (2019). Applying a Two-Layer Quadrupole Model for Quantitative Thermography at Overload-Induced Delaminations in GFRP. Proceedings, 27(1), 40. https://doi.org/10.3390/proceedings2019027040




