Investigation on Non-Linear Vibration Response of Cantilevered Thin Plates with Crack Using Electronic Speckle Pattern Interferometry †
Abstract
:1. Introduction
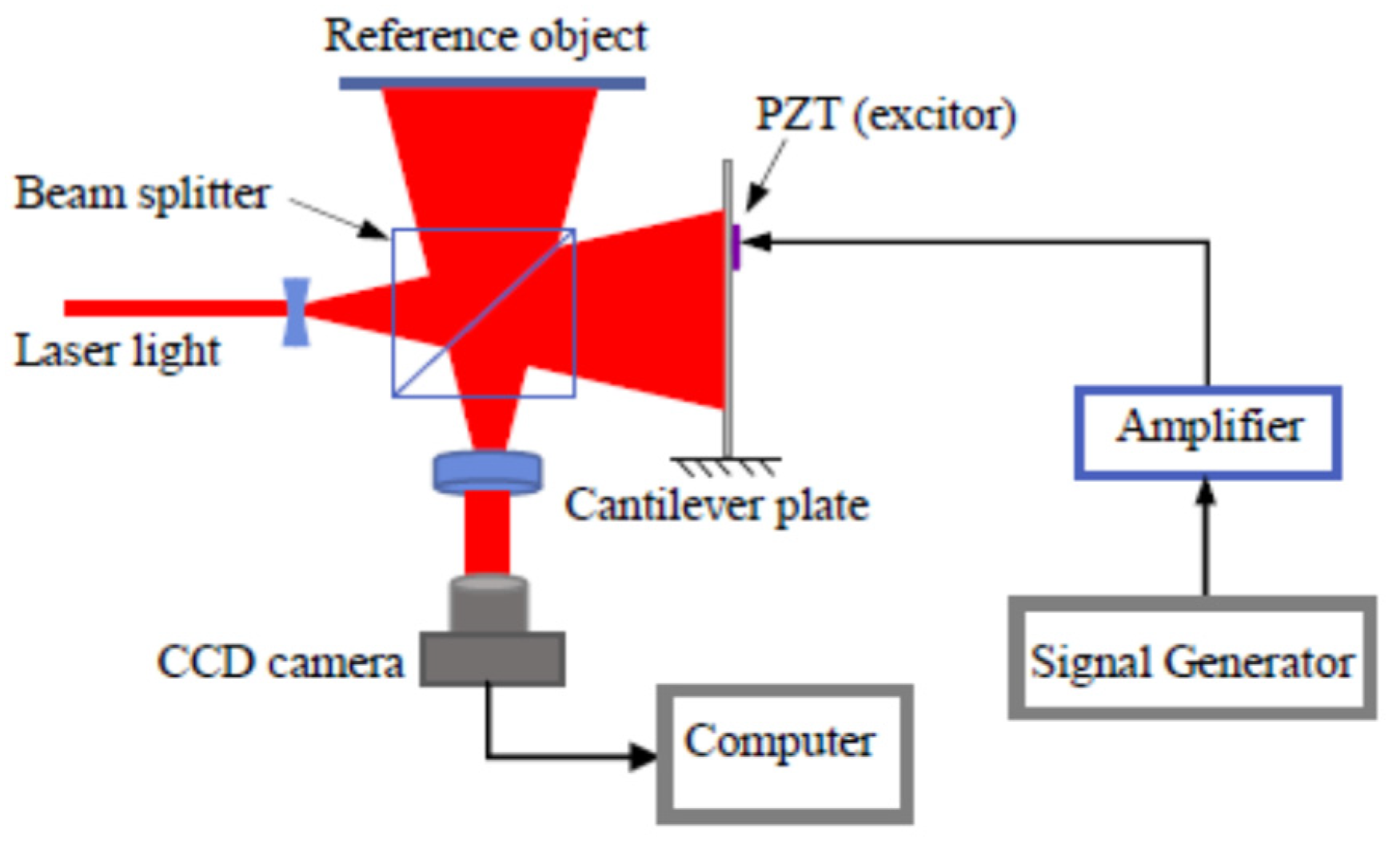
2. Experimental Work and Mode Analysis
2.1. Specimens Preparation and Numerical Model
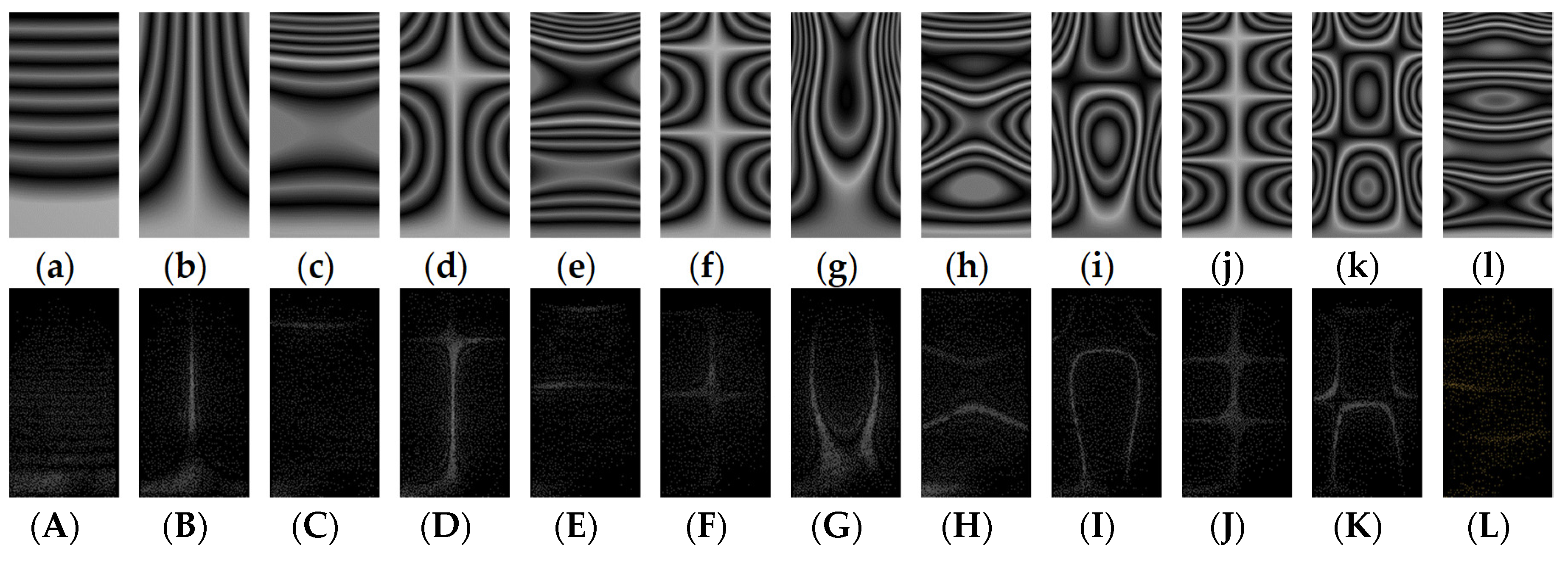
2.2. Natural Frequencies and Mode Shapes
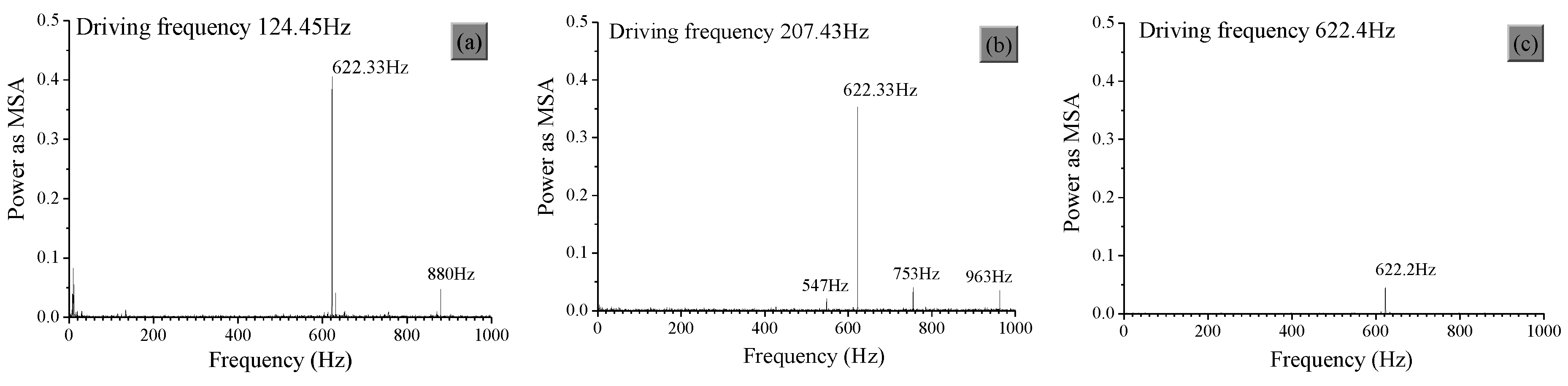
2.3. Nonlinear Vibrating Response of the Intact Plate
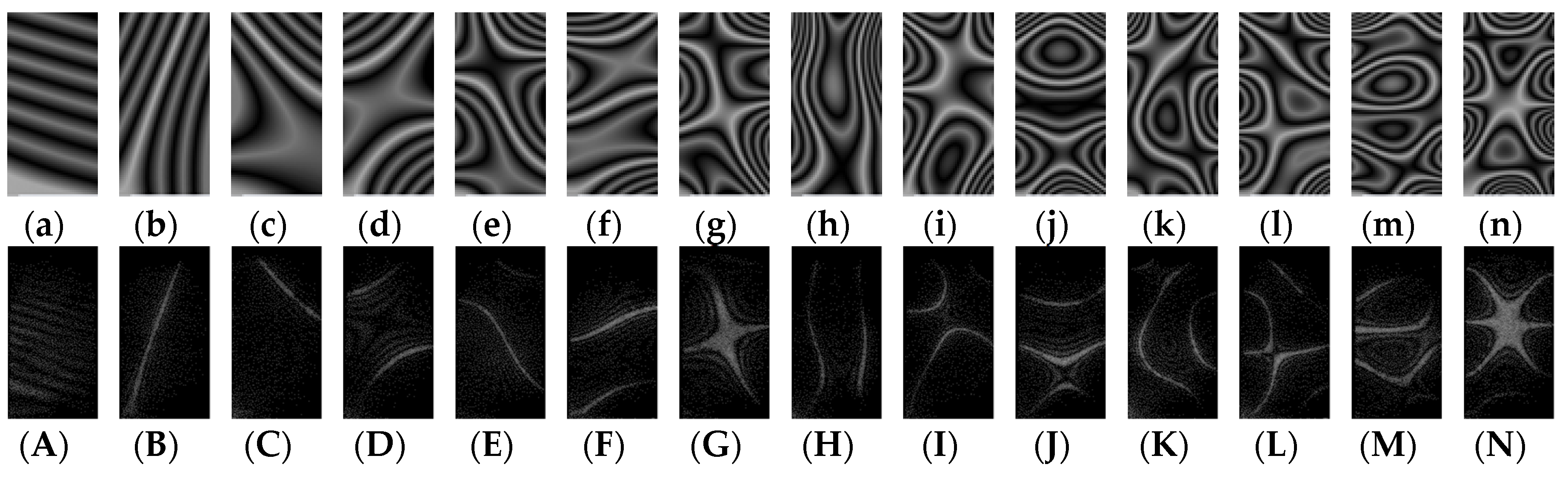
2.4. Mode Shapes Vary with the Length of Root-Slit Variation
3. Concluding Remarks
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Dimarogonas, A.D. Vibration of cracked structures: A state of the art review. Eng. Fract. Mech. 1996, 55, 831–857. [Google Scholar] [CrossRef]
- Qiu, Z.C.; Wu, H.X.; Ye, C.D. Acceleration sensors based modal identification and active vibration control of flexible smart cantilever plate. Aerosp. Sci. Technol. 2009, 13, 277–290. [Google Scholar] [CrossRef]
- Lynn, P.P.; Kumbasar, N. Free vibration of thin rectangular plates having narrow cracks with simply supported edges. Develop. Mech. 1967, 4, 911–928. [Google Scholar]
- Stahl, B.; Keer, L.M. Vibration and stability of cracked rectangular plates. Int. J. Solids Struct. 1972, 8, 69–91. [Google Scholar] [CrossRef]
- Wu, G.Y.; Shih, Y.S. Dynamic instability of rectangular plate with an edge crack. Comput. Struct. 2005, 84, 1–10. [Google Scholar] [CrossRef]
- Huang, C.S.; Leissa, A.W.; Li, R.S. Accurate vibration analysis of thick, cracked rectangular plates. J. Sound Vib. 2011, 330, 2079–2093. [Google Scholar] [CrossRef]
- Saito, A.; Castanier, M.P.; Pierre, C. Estimation and veering analysis of nonlinear resonant frequencies of cracked plates. J. Sound Vib. 2009, 326, 725–739. [Google Scholar] [CrossRef]
- Andreaus, U.; Baragatti, P. Experimental damage detection of cracked beams by using nonlinear characteristics of forced response. Mech. Syst. Signal Process. 2012, 31, 382–404. [Google Scholar] [CrossRef]
- Sundaresan, M.J.; Ghoshal, A.; Li, J.; Schulz, M.J.; Pai, P.F.; Chung, J.H. Experimental damage detection on a wing panel using vibration deflection shapes. Struct. Health Monit. 2003, 2, 243–256. [Google Scholar] [CrossRef]
- Maruyama, K.; Ichinomiya, O. Experimental study of free vibration of clamped rectangular plates with straight narrow slits. JSME Int. J. Ser. III 1989, 32, 187–193. [Google Scholar] [CrossRef]
- Wang, W.C.; Hwang, C.H.; Lin, S.Y. Vibration measurement by the time-averaged electronic speckle pattern interferometry methods. Appl. Opt. 1996, 35, 4502–4509. [Google Scholar] [CrossRef] [PubMed]

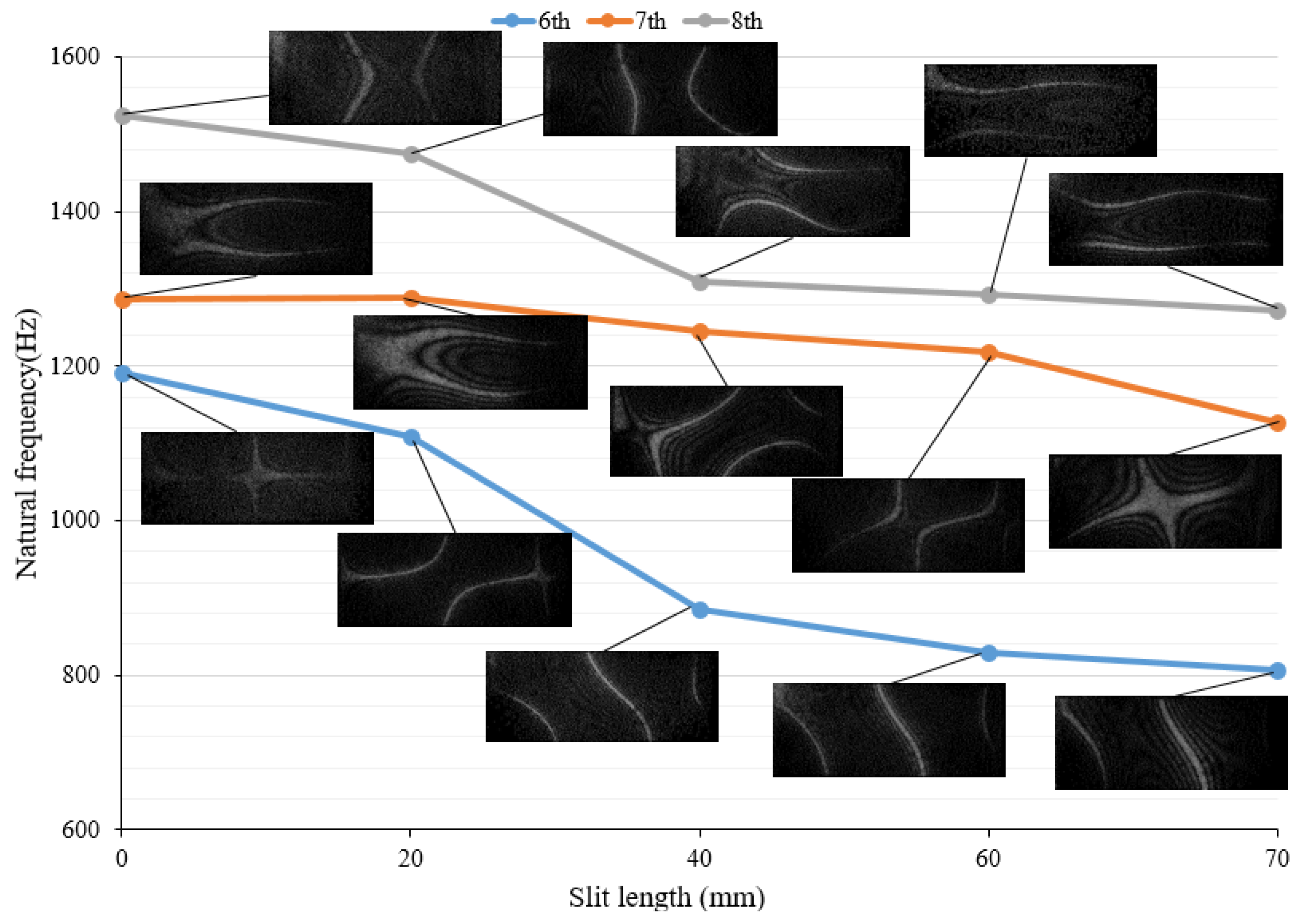
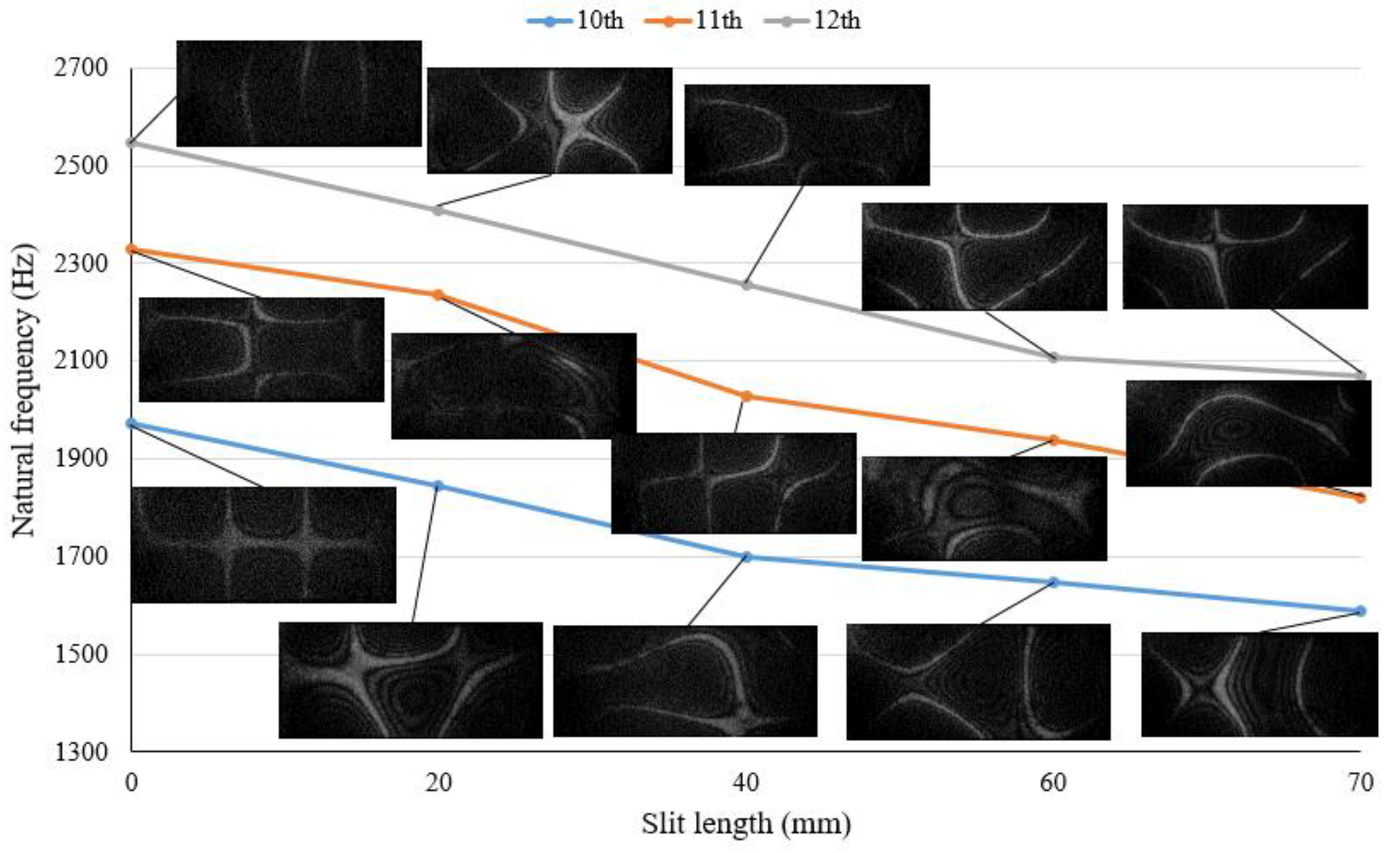
| Mode | Specimen 1 | Specimen 2 | Specimen 3 | Specimen 4 | Specimen 5 | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| FEM | ESPI | FEM | ESPI | FEM | ESPI | FEM | ESPI | FEM | ESPI | |
| 1 | 44.176 | 44.2 | 42.698 | 42.3 | 38.359 | 37.2 | 31.802 | 29.9 | 25.662 | 23.4 |
| 2 | 191.79 | 189.6 | 182.52 | 178.9 | 159.33 | 152.4 | 122.07 | 119.0 | 98.036 | 93.0 |
| 3 | 274.96 | 276.6 | 266.34 | 265.3 | 249.52 | 244.6 | 229.20 | 228.0 | 216.11 | 210.8 |
| 4 | 623.69 | 621.4 | 589.87 | 585.7 | 489.99 | 473.5 | 385.16 | 383.9 | 350.88 | 348.1 |
| 5 | 771.77 | 777.8 | 748.34 | 747.1 | 703.39 | 688.4 | 659.81 | 658.0 | 632.32 | 619.7 |
| 6 | 1195.0 | 1195.0 | 1113.4 | 1107.8 | 898.68 | 886.3 | 815.589 | 828.6 | 797.70 | 806.1 |
| 7 | 1265.2 | 1287.9 | 1258.1 | 1289.0 | 1251.0 | 1245.6 | 1218.6 | 1218.9 | 1142.4 | 1127.2 |
| 8 | 1527.8 | 1524.8 | 1480.1 | 1475.2 | 1314.5 | 1309.0 | 1258.9 | 1292.8 | 1251.4 | 1271.6 |
| 9 | 1690.4 | 1696.8 | 1614.9 | 1628.1 | 1529.8 | 1523.6 | 1474.0 | 1490.1 | 1424.4 | 1435.7 |
| 10 | 1968.4 | 1973.2 | 1844.5 | 1844.6 | 1717.2 | 1700.1 | 1631.6 | 1647 | 1579.5 | 1588.9 |
| 11 | 2352.0 | 2329.4 | 2233.1 | 2234.2 | 2051.7 | 2027.5 | 1926.1 | 1939 | 1822.3 | 1821.7 |
| 12 | 2546.2 | 2548.7 | 2413.5 | 2410.0 | 2294.3 | 2256.5 | 2099.3 | 2106.3 | 2069.9 | 2068.3 |
| 13 | 2554.2 | 2580.8 | 2521.1 | 2512.6 | ||||||
| 14 | 2662.1 | 2692 | 2571.9 | 2602.7 | ||||||
| 15 | 3026.7 | 3048 | 3010.2 | 3011 | ||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tao, N.; Ma, Y.; Jiang, H.; Dai, M.; Yang, F. Investigation on Non-Linear Vibration Response of Cantilevered Thin Plates with Crack Using Electronic Speckle Pattern Interferometry. Proceedings 2018, 2, 539. https://doi.org/10.3390/ICEM18-05456
Tao N, Ma Y, Jiang H, Dai M, Yang F. Investigation on Non-Linear Vibration Response of Cantilevered Thin Plates with Crack Using Electronic Speckle Pattern Interferometry. Proceedings. 2018; 2(8):539. https://doi.org/10.3390/ICEM18-05456
Chicago/Turabian StyleTao, Nan, Yinhang Ma, Hanyang Jiang, Meiling Dai, and Fujun Yang. 2018. "Investigation on Non-Linear Vibration Response of Cantilevered Thin Plates with Crack Using Electronic Speckle Pattern Interferometry" Proceedings 2, no. 8: 539. https://doi.org/10.3390/ICEM18-05456
APA StyleTao, N., Ma, Y., Jiang, H., Dai, M., & Yang, F. (2018). Investigation on Non-Linear Vibration Response of Cantilevered Thin Plates with Crack Using Electronic Speckle Pattern Interferometry. Proceedings, 2(8), 539. https://doi.org/10.3390/ICEM18-05456




