Quasi-Static and Dynamic Testing of Annealed Float Glass †
Abstract
:1. Introduction
2. Material and Methods
2.1. Glass Material
2.2. Digital Image Correlation
2.3. SIMLab Shock Tube Facility
3. Experiments
3.1. Four-Point Bending Tests
3.2. Quasi-Static Pressure Tests
3.3. Shock Tube Tests
4. Strength Prediction Modelling
4.1. Model Basis and Procedure
|
|
|
| ||
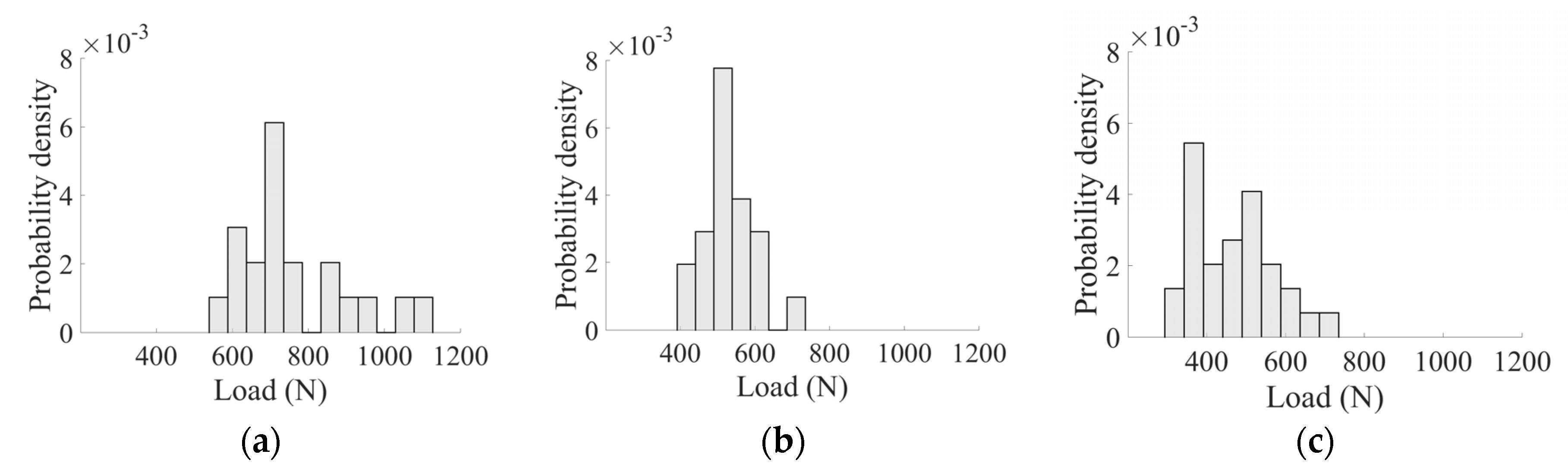
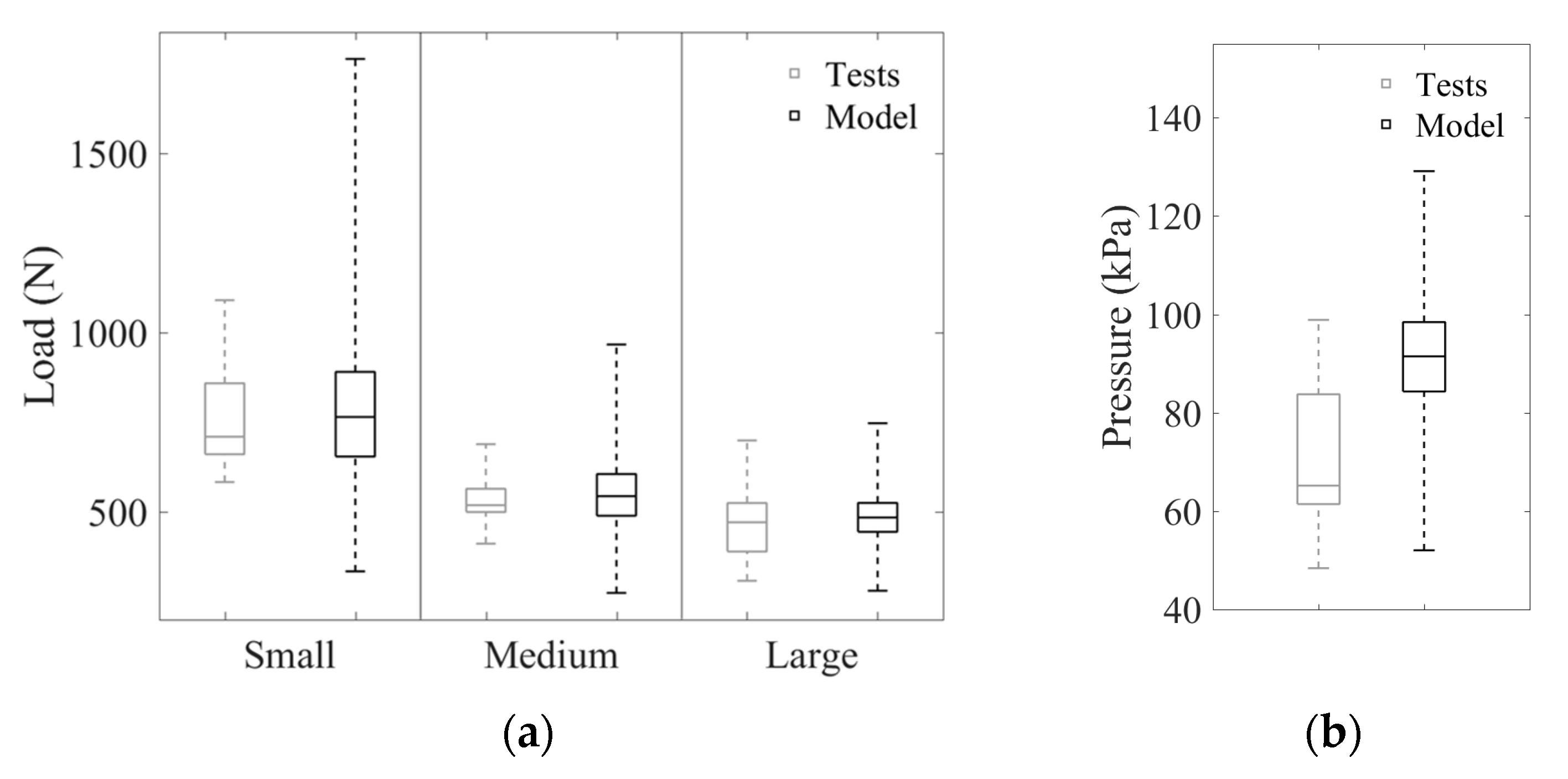
4.2. Model Results
|
|
|
5. Concluding Remarks
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Shariat, S.; Mallonee, S.; Stephens-Stidham, S. Summary of Reportable Injuries in Oklahoma: Oklahoma City Bombing Injuries. 1998. Available online: https://www.ok.gov/health2/documents/OKC_Bombing.pdf (accessed on 13 July 2018).
- Beason, W.L.; Morgan, J.R. Glass failure prediction model. J. Struct. Eng. 1984, 110, 197–212. [Google Scholar] [CrossRef]
- Wachtman, J.B.; Cannon, W.R.; Matthewson, M.J. Mechanical Properties of Ceramics, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Larcher, M.; Solomos, G.; Casadei, F.; Gebbeken, N. Experimental and numerical investigations of laminated glass subjected to blast loading. Int. J. Impact Eng. 2012, 39, 42–50. [Google Scholar] [CrossRef]
- Aune, V.; Fagerholt, E.; Langseth, M.; Børvik, T. A shock tube facility to generate blast loading on structures. Int. J. Prot. Struct. 2016, 7, 340–366. [Google Scholar] [CrossRef]
- Osnes, K.; Børvik, T.; Hopperstad, O.S. Shock tube testing and strength modelling of annealed float glass. 2018; in press. [Google Scholar]
- Yankelevsky, D.Z. Strength prediction of annealed glass plates—A new model. Eng. Struct. 2014, 79, 244–255. [Google Scholar] [CrossRef]
- Newman, J.; Raju, I. An empirical stress-intensity factor equation for the surface crack. Eng. Fract. Mech. 1981, 15, 185–192. [Google Scholar] [CrossRef]
- Fagerholt, E. Field Measurements in Mechanical Testing Using Close-Range Photogrammetry and Digital Image Analysis. Ph.D. Thesis, Norwegian University of Science and Technology (NTNU), Trondheim, Norway, 2012. [Google Scholar]
- Dassault Systemes Simulia Corporation. Abaqus, Version 6.14; Dassault Systemes Simulia Corporation: Providence, RI, USA, 2014. [Google Scholar]
- Anstis, G.; Chantikul, P.; Lawn, B.R.; Marshall, D. A critical evaluation of indentation techniques for measuring fracture toughness: I, Direct crack measurements. J. Am. Ceram. Soc. 1981, 64, 533–538. [Google Scholar] [CrossRef]


| Test | Test | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| A-01 | 53.0 | 1.21 | 4.52 | 15.8 | C-01 | 73.4 | 1.29 | 5.89 | 21.5 |
| A-02 | 51.6 | 1.38 | 5.06 | 14.7 | C-02 | 73.2 | 1.33 | 6.48 | 22.3 |
| B-01 | 63.7 | 0.88 | 3.23 | 24.5 | B-05 | 63.3 | 1.00 | 4.29 | 21.8 |
| B-02 | 64.6 | 1.25 | 5.40 | 18.8 | B-06 | 62.9 | 0.92 | 3.68 | 19.3 |
| B-03 | 65.5 | 1.46 | 5.64 | 17.3 | B-07 | 64.0 | 1.33 | 5.77 | 19.0 |
| B-04 | 62.5 | 5.44 | B-08 | 62.9 | 5.96 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Osnes, K.; Hopperstad, O.S.; Børvik, T. Quasi-Static and Dynamic Testing of Annealed Float Glass. Proceedings 2018, 2, 495. https://doi.org/10.3390/ICEM18-05412
Osnes K, Hopperstad OS, Børvik T. Quasi-Static and Dynamic Testing of Annealed Float Glass. Proceedings. 2018; 2(8):495. https://doi.org/10.3390/ICEM18-05412
Chicago/Turabian StyleOsnes, Karoline, Odd Sture Hopperstad, and Tore Børvik. 2018. "Quasi-Static and Dynamic Testing of Annealed Float Glass" Proceedings 2, no. 8: 495. https://doi.org/10.3390/ICEM18-05412
APA StyleOsnes, K., Hopperstad, O. S., & Børvik, T. (2018). Quasi-Static and Dynamic Testing of Annealed Float Glass. Proceedings, 2(8), 495. https://doi.org/10.3390/ICEM18-05412




