Investigation of 3D TRC’s by Means of Three Point Bending Tests on Short Beam Specimens †
Abstract
:1. Introduction
2. Materials and Methods
2.1. Material Selection
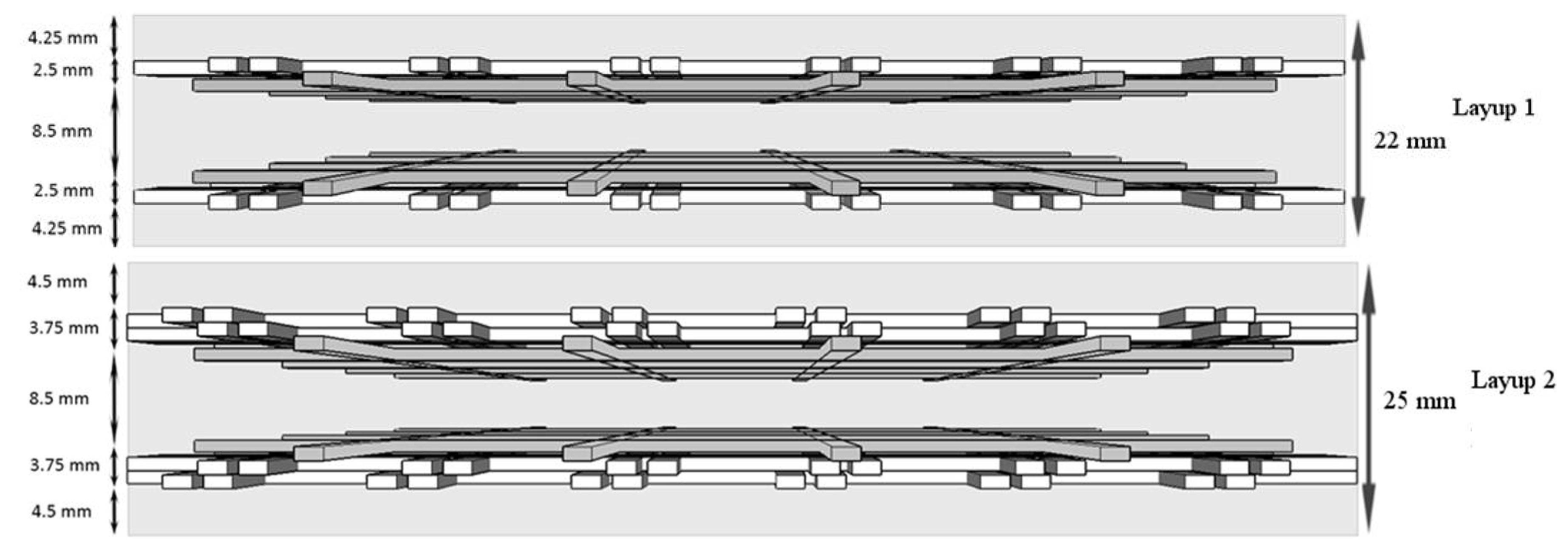
2.2. Considered Fibre Textile Layups
2.3. Specimen Manufacturing Process
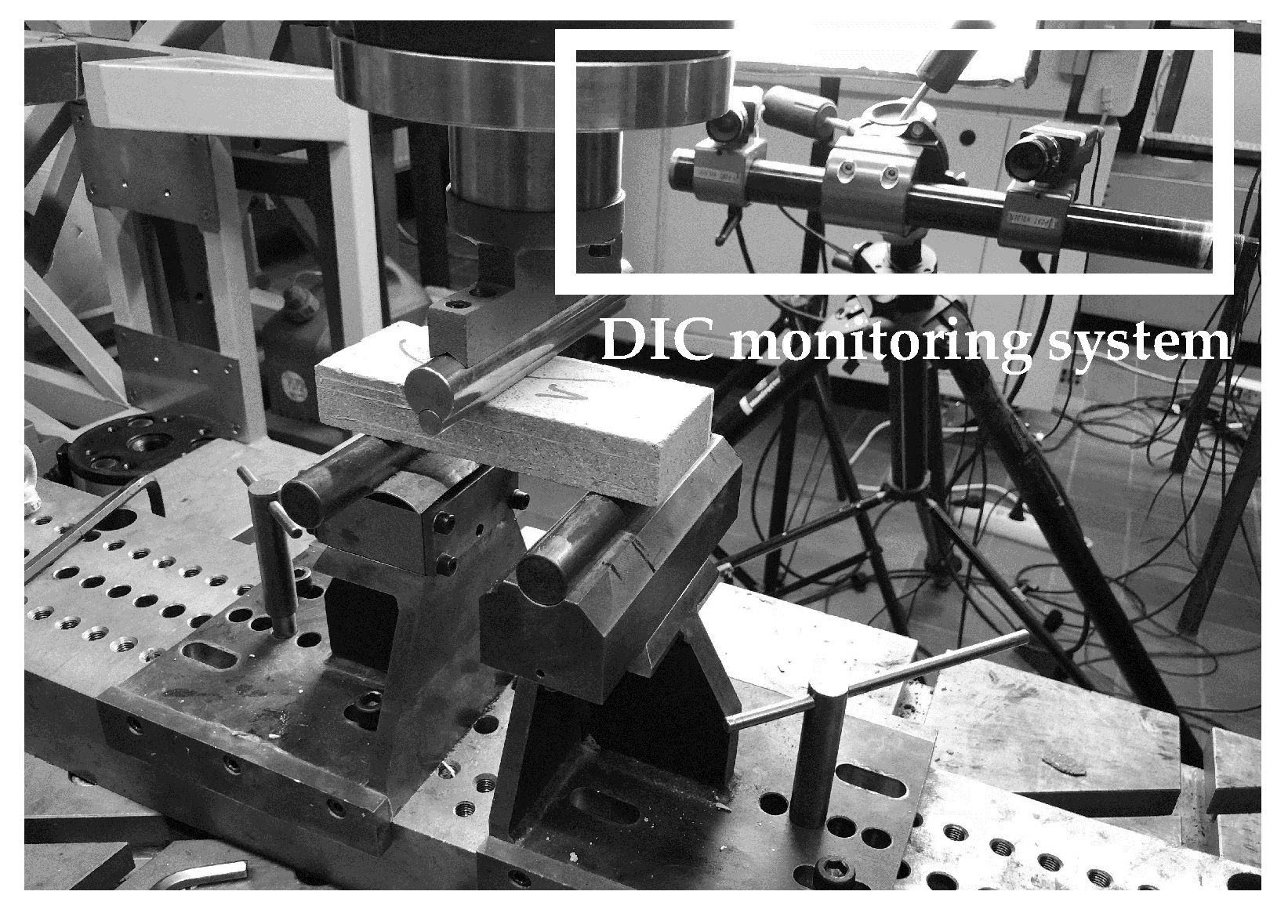
2.4. Three-Point Bending Test Setup
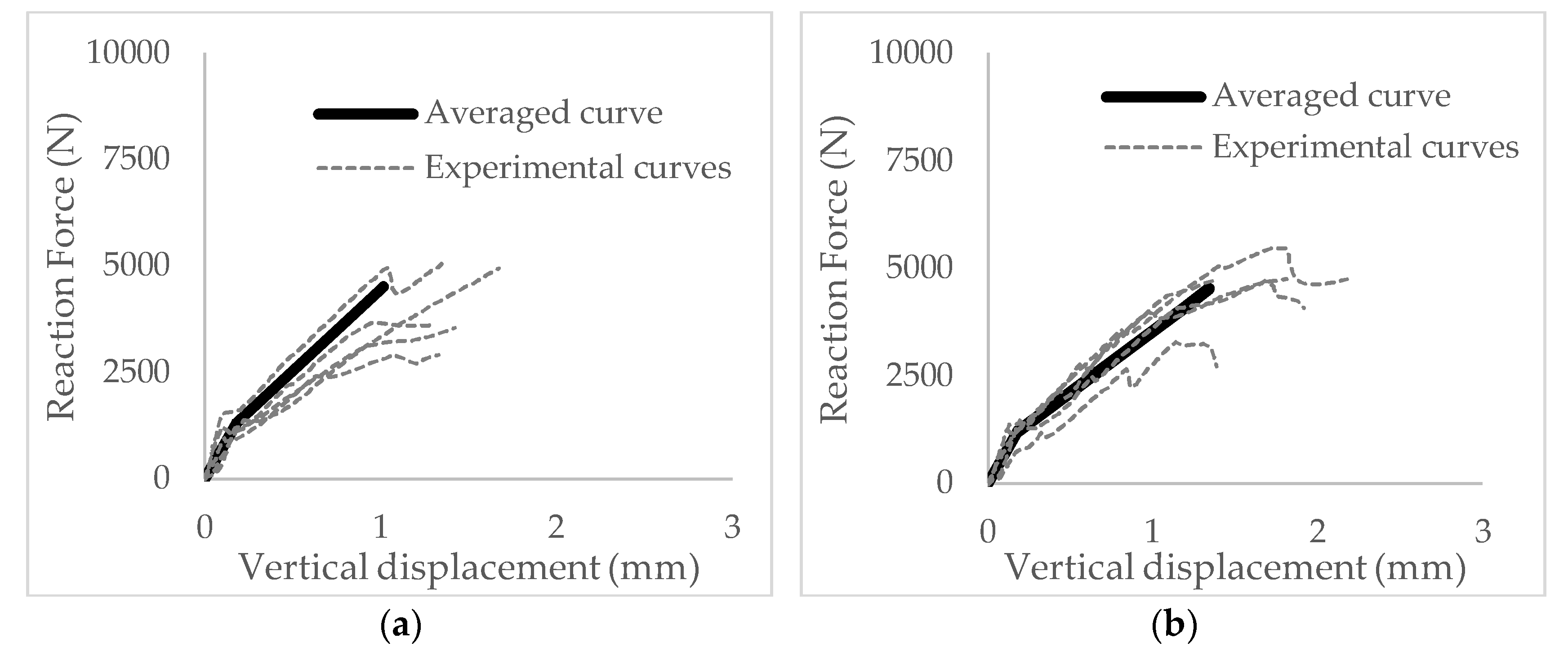
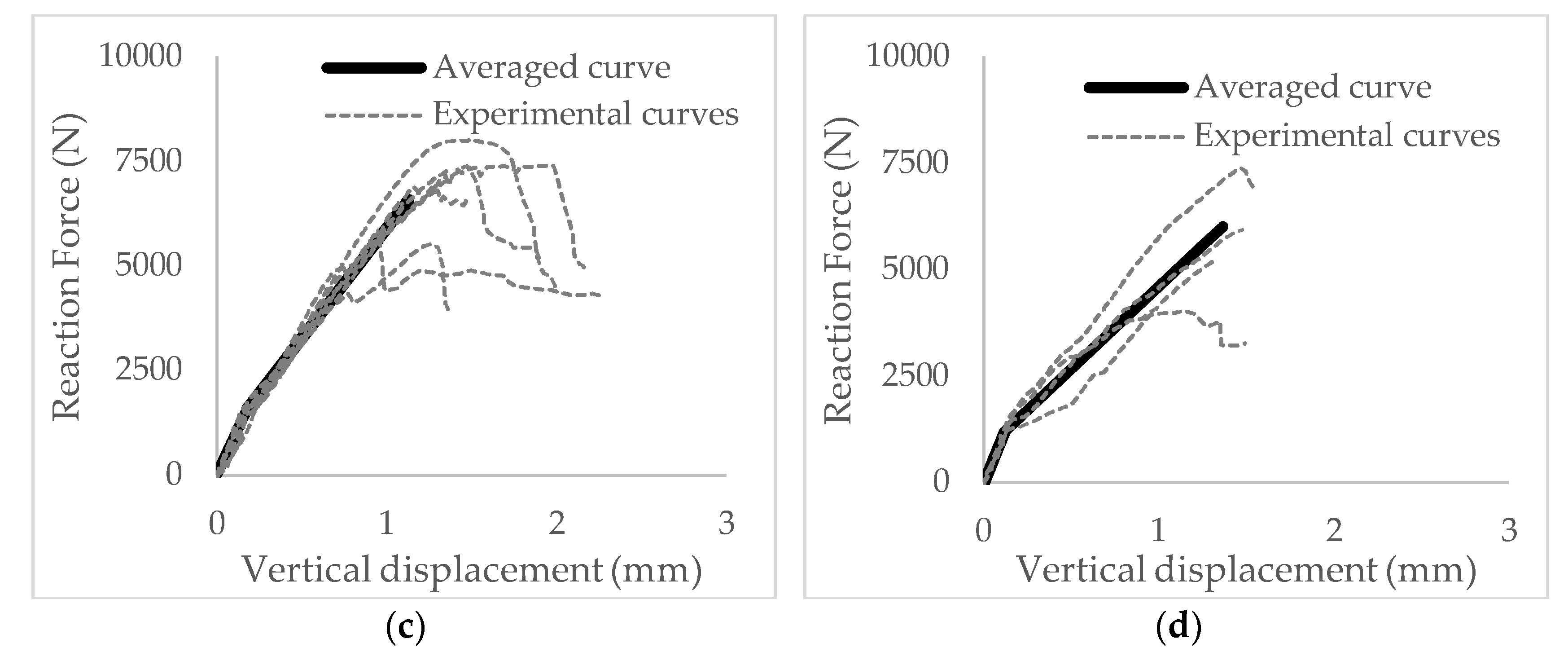
3. Results and Discussion
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Brameshuber, W. Textile Reinforced Concrete—State-of-the-Art Report of RILEM TC 201-TRC, 1st ed.; RILEM: Aachen, Germany, 2006. [Google Scholar]
- Bentur, A.; Mindess, S. Fibre Reinforced Cementitious Composites; Modern Concrete Technology Series; Taylor & Francis: New York, NY, USA, 2007. [Google Scholar]
- Aveston, J.; Cooper, G.A.; Kelly, A. Single and multiple fracture In Properties of Fibre Composites; National Physical Laboratory: Teddington, UK, 1971; pp. 15–24. [Google Scholar]
- Triantafillou, T. Textile Fibre Composites in Civil Engineering, 1st ed.; Woodhead Publishing: Sawston, UK, 2016. [Google Scholar]
- Sharei, E.; Scholzen, A.; Hegger, J.; Chudoba, R. Structural behavior of a lightweight, textile-reinforced concrete barrel vault shell. Compos. Struct. 2017, 171, 505–514. [Google Scholar] [CrossRef]
- Scholzen, A.; Chudoba, R.; Hegger, J. Thin-walled shell structures made of textile-reinforced concrete Part I: Structural design and construction. Concr. Struct. 2015, 16, 106–114. [Google Scholar] [CrossRef]
- Verwimp, E.; Tysmans, T.; Mollaert, M.; Berg, S. Experimental and numerical buckling analysis of a thin TRC dome. Thin-Walled Struct. 2015, 94, 89–97.
- Roye, A.; Gries, T. 3-D Textiles for Advanced Cement Based Matrix Reinforcement. J. Ind. Text. 2007, 37, 163–173. [Google Scholar] [CrossRef]
- Gurkan, P. 3D Woven Fabrics. In Woven Fabrics; InTech: London, UK, 2012; pp. 91–121. [Google Scholar]
- El Kadi, M.; Verbruggen, S.; Vervloet, J.; De Munck, M.; Wastiels, J.; Tysmans, T. Experimental investigation and benchmarking of 3D textile reinforced cementitious composites. In Proceedings of the 4th International Conference on Strain-Hardening Cement-Based Composites, Dresden, Germany, 18–20 September 2017; pp. 1–8. [Google Scholar]
- Peled, A.; Haik, R.; Sasi, E.A. Influence of three-dimensional (3D) fabric orientation on flexural properties of cement-based composites. Cem. Concr. Compos. 2017, 80, 1–9. [Google Scholar]
- Sutton, M.A.; Orteu, J.-J.; Schreier, H.W. Image Correlation for Shape, Motion and Deformation Measurements; Springer Science + Business Media: Berlin, Germany, 2009. [Google Scholar]
- Sika, Sikagrout 217, Note de Produit. Available online: https://fra.sika.com/fr/solutions-produits/marche- construction/voirie/produits-systemes/scellement-calage-grandes-dimensions.html (accessed on 7 November 2017).

| Aggregate Size (mm) | Compressive Strength (28d) MPa | Modulus of Rupture (28d) MPa | Young’s Modulus (GPa) |
|---|---|---|---|
| 0–1.6 | 55 | 12 | 9 |
| Fibre Textile | Material | Mesh Size (mm × mm) | Coated Density (g/m²) | Uncoated Density% (g/m²) |
|---|---|---|---|---|
| 3D | AR-Glass | 22.5 × 22.5 | 616 | 536 |
| 2D | AR-Glass | 17.5 × 17.5 | 653 | 568 |
| Parameter/Layup | Layup 1, Connected | Layup 1, Cut Through | Layup 2, Connected | Layup 2, Cut Through |
|---|---|---|---|---|
| fc (N) | 1317.9 | 1219.8 | 1578.0 | 1195.0 |
| dc (mm) | 0.18 | 0.18 | 0.18 | 0.12 |
| ff (N) | 4510.4 | 4525.5 | 6580.0 | 6013.1 |
| df (mm) | 1.02 | 1.34 | 1.13 | 1.37 |
| Epost_cracking (N/mm) | 3800.60 | 2849.74 | 5265.26 | 3854.48 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kadi, M.E.; Verbruggen, S.; Vervloet, J.; Munck, M.D.; Wastiels, J.; Hemelrijck, D.V.; Tysmans, T. Investigation of 3D TRC’s by Means of Three Point Bending Tests on Short Beam Specimens. Proceedings 2018, 2, 397. https://doi.org/10.3390/ICEM18-05227
Kadi ME, Verbruggen S, Vervloet J, Munck MD, Wastiels J, Hemelrijck DV, Tysmans T. Investigation of 3D TRC’s by Means of Three Point Bending Tests on Short Beam Specimens. Proceedings. 2018; 2(8):397. https://doi.org/10.3390/ICEM18-05227
Chicago/Turabian StyleKadi, Michael El, Svetlana Verbruggen, Jolien Vervloet, Matthias De Munck, Jan Wastiels, Danny Van Hemelrijck, and Tine Tysmans. 2018. "Investigation of 3D TRC’s by Means of Three Point Bending Tests on Short Beam Specimens" Proceedings 2, no. 8: 397. https://doi.org/10.3390/ICEM18-05227
APA StyleKadi, M. E., Verbruggen, S., Vervloet, J., Munck, M. D., Wastiels, J., Hemelrijck, D. V., & Tysmans, T. (2018). Investigation of 3D TRC’s by Means of Three Point Bending Tests on Short Beam Specimens. Proceedings, 2(8), 397. https://doi.org/10.3390/ICEM18-05227






