Abstract
Support interference in wind tunnel testing is an unavoidable effect. It is difficult to measure the aerodynamic force acting on a model such as a ball owing to this effect []. A magnetic suspension and balance system (MSBS) suspends the model without any mechanical supports by using magnetic force, and at the same time, can measure the aerodynamic force acting on the model. The 1-m MSBS, located at the Institute of Fluid Science, Tohoku University, is the world’s largest MSBS. It has a 1-m-wide octagonal cross section. A sphere is taken as the experimental object, and the results of the aerodynamic force acting on it are presented. The diameter of the sphere is 150 mm, and its blockage ratio is 2.1%. The experiment was conducted at Reynolds numbers ranging from 0.5 × 105 to 4.7 × 105. It clearly shows the drag crisis at approximately Re = 3.7 × 105, and the fluctuation of the sphere abruptly increase around this region.
1. Introduction
In a wind tunnel test, mechanical support methods such as strings, struts, and wires are used for supporting a model in the test section. The use of mechanical supports causes a problem called support interference that changes the flow field around the model. One of the devices used for avoiding support interference is a magnetic suspension and balance system (MSBS). This is a device that can magnetically support a model on a test section by using the interaction between a magnet in the model and the magnetic field generated by the coils surrounding the test section. The magnetic force acting on the suspended model is evaluated by the current passing through the coils. Then, the aerodynamic force is obtained from the motion equation of the model. This implies that the MSBS has a balance function. Owing to these characteristics, the MSBS is suitable for measurement of aerodynamic forces acting on bluff bodies such as a sphere, extremely elongated objects such as a spear, and complicated shape models that are difficult to support. Research on the flow field around a ball elucidates the mechanism of the ball’s behavior in ball games such as baseball, soccer, tennis, table tennis, and golf. Therefore, it contributes toward developing a ball that enhances the enjoyment of the game and devising an efficient practice method. In addition, an MSBS can possibly contribute to diverse fields such as application to space return capsule technology. The world’s first MSBS was built at ONERA in France in 1957. It was applied to a supersonic wind tunnel with a Mach number 1 to 3 with an 8.5 × 8.5 cm test section. The MSBS had five-degrees-of-freedom control. To date, many MSBSs have been built at MIT, University of Southampton, AEDC/NASA Langley, and other institutes. In Japan too, JAXA built a 10 cm × 10 cm MSBS in 1987, and a 60-cm-wide MSBS was put to practical use around 2000. MSBSs are being developed even in institutions other than JAXA []. At the Institute of Fluid Science (IFS), Tohoku University, an MSBS (1-m MSBS) with a 1.01-m-wide test section, which is the largest in the world, was introduced in 2015. The world’s largest test section contributes to a reduction in blockage ratio and a relatively high Reynolds number. Furthermore, an ideal wind tunnel test environment with low turbulence intensity and no support interference can be implemented by installing it in a low-turbulence wind tunnel []. It is also registered as a shared facility for the entire university, and it is widely used by users from both inside and outside the university. In this paper, we introduce the outline of the 1-m MSBS developed by IFS and present the test results of the drag acting on a sphere obtained using the 1-m MSBS.
2. MSBS Principle
The MSBS generates a magnetic force by applying a magnetic field generated by an electromagnetic coil arranged around the measuring part to a permanent magnet inserted inside a model of nonmagnetic material. The MSBS supports the model in the test section by antagonizing its magnetic force to gravity and aerodynamic force acting on the model. When a permanent magnet with a magnetic moment M [Wbm] is placed in the magnetic field of strength H [AT/m], the force acting on the magnet can be expressed as follows.
where F is the force (N), N is the moment (Nm), and the subscript magnet denotes the magnetic force. From these equations, it is understood that we can control the magnetic force by controlling the magnetic field intensity H. When the model mass, including the mass of the magnet, is represented by m and the moment of inertia around the center of gravity of the model is represented by I [Nm], the equation of motion of the model is as follows.
Here, the subscript aero represents the aerodynamic force and gravity represents gravity. Further, v is the velocity vector of the center of gravity (m/s) of the model, and ω is the angular velocity vector around the center of gravity of the model (rad/s). When these equations are time-averaged for a long time when keeping the model in space, the left-hand sides of Equations (3) and (4) become zero. Then, the time-averaged aerodynamic force can be evaluated easily from the time-averaged magnetic force. Please refer to [,] for further details.
3. Outline of 1-m MSBS
As shown in Figure 1, the 1-m MSBS has a regular octagonal cross section with a test section having a length of 3 m and a width of 1.01 m. Ten coils are arranged to surround the test section, and the function of each coil is listed in Table 1. A power amplifier is attached to each coil, and it can pass current up to 150 A. In addition, as the measuring section is large, the coils are far apart from each other. Therefore, the magnetic field is generated efficiently by forming a magnetic circuit by connecting coils # 1 to # 4 and # 5 to # 8 with the yoke. The position and attitude of the model are captured by five- or six-line sensor cameras using LED light sources and optical systems, and the current in each coil is controlled by a PI controller and a double phase advancer, as shown in Figure 2. The reading frequency of the line sensor camera is 1250 Hz, and by appropriately rearranging the optical system according to the model, it is possible to measure the position and attitude of the model in a non-contact manner with high speed and high accuracy. At the 1-m MSBS, a model with a large permanent magnet (main magnet) is used for the test. A model installed with only a main magnet has five-degrees-of-freedom control, except for the rolls. For six-degrees-of-freedom control, small magnets called auxiliary magnets are installed in addition to the main magnet. The adjustment of the magnetic force for controlling the position and attitude of the model is carried out by controlling the currents flowing through the 10 coils. The method of controlling the currents is described below. First, when considering the x-axis, the magnetic force in the x-axis direction is obtained from expression (1) as follows.
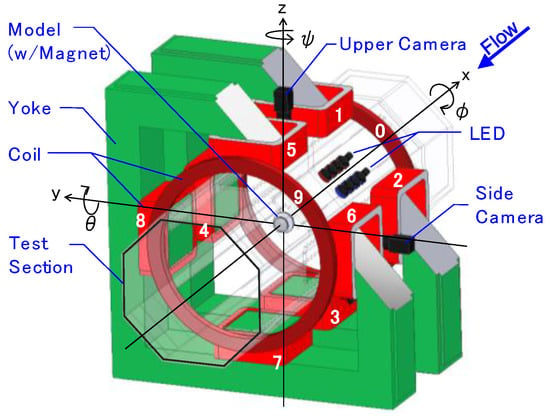
Figure 1.
Schematic view of 1-m MSBS.

Table 1.
Function of coils in 1-m MSBS.

Figure 2.
Diagram of 1-m MSBS.
When the magnetic moment of the main magnet is set to coincide with the center axis of the model, the magnetic moment has a component only in the x-direction when the central axis of the model coincides with the x-axis of the measurement part. Then, Equation (5) is expressed as follows.
Here,
Because the 1-m MSBS is designed such that hxx is almost constant near the center of the test section, it is possible to control Fx by Idrag only. The other magnetic forces can be similarly controlled by the control currents, as shown in Table 1.
The wind tunnel test is performed by setting the 1-m MSBS to the low-turbulence wind tunnel. The maximum flow speed is 65 m/s, and the mainstream turbulence intensity is less than 0.06% in the range from 5 m/s to the maximum flow speed.
4. Measurement of Sphere Drag using 1-m MSBS
4.1. Purpose of the Test
Drag measurement of a sphere, which is a representative shape of bluff bodies, has been conducted in the past, and the critical Reynolds number at which a sudden reduction in drag coefficient (drag crisis) occurs is well known. Even in ball games, a ball speed that crosses the critical Reynolds number is often observed, and it can be stated that its influence on the competition result is not negligible. Drag measurement of a sphere in the critical Reynolds number range without support interference has been reported by JAXA’s 60-cm MSBS [], but there are not many reports. In addition, according to the document, large aerodynamic force fluctuations are observed in the critical Reynolds number range. Therefore, this test was conducted to provide the measurement results of the aerodynamic force acting on a sphere in the critical Reynolds number range and to examine the support performance of the 1-m MSBS.
4.2. Apparatus and Model
The quality of uniform flow inside the test section of the 1-m MSBS installed in the low-turbulence wind tunnel of the IFS was evaluated. It was confirmed that the effect of buoyancy can be neglected in the drag measurement of the sphere. Five-degrees-of-freedom control, except for the rolls, was adopted when magnetically supporting the axisymmetric model in the 1-m MSBS. The model used in the 1-m MSBS must be made of a nonmagnetic material and have a characteristic point; a strong contrast due to white and black that can be measured by a line sensor camera. Therefore, if the magnet is fixed inside and can be measured with a line sensor camera, the 1-m MSBS can support the model with the outer skin of the sports equipment. The sphere model used in this test is shown in Figure 3. The diameter is 150 mm, and the blockage ratio is 2.1%. Incidentally, the corrections of blockage effect were not applied to the obtained results. The sphere surface was polished with sandpaper of grade # 1200 or more. However, as it fell several times during the experiment, its surface was slightly roughened, although it was corrected. In addition, the model consists of three parts, the center part (brass) the front and rear parts (transparent acrylic resin), and its joint part is at 50.7° and 129.3° from the x-axis of the model, and there were remain slight steps. The difference in the linear expansion coefficient between the center part and the front and rear parts is ~5 μm/K. The maximum change in flow temperature during the test was 1.1°C. A cylindrical neodymium magnet with a diameter of 100 mm and a length of 100 mm was inserted inside the model, as shown in Figure 3. The total weight of the model was ~11 kg. The magnetic force resisting the drag depends on the magnetic field formed by the air-core coil arranged at the front and rear of the measuring part. Therefore, it is necessary to obtain the relationship between the current flowing in the air-core coil and the magnetic force in advance. For this reason, a known force was applied along the same direction as the drag force, instead of the drag force, and the relation between the current flowing in the air-core coil and the magnetic force was investigated (drag calibration). As shown in Figure 4, a linear relationship was confirmed, and for a Reynolds number of 0.1 × 106 or more, the error in evaluating the average drag is less than 1.1%.
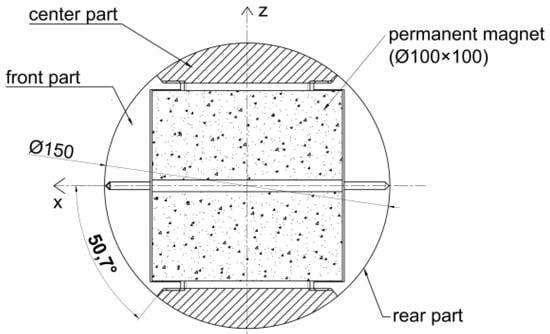
Figure 3.
Sphere model.
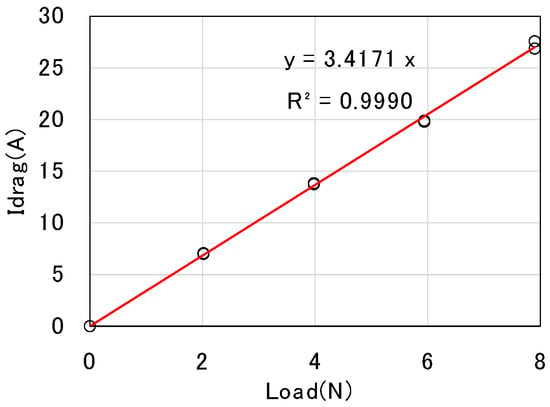
Figure 4.
Drag calibration result.
4.3. Test Contents
In the test, the position and attitude of the model and the current flowing through each coil were measured 8191 times at 0.8-ms intervals at each flow speed between 5 m/s and 50 m/s. At the same time, the uniform flow was also measured. A reciprocating rotation around the x-axis of the model was observed; the maximum amplitude was less than ~10° and its period was very long of ~6 s. The drag of the model was evaluated based on the drag calibration test results obtained from the average value of the 8191 air-core coil current commands. These average aerodynamic forces can be regarded as time-average values of ~6.5 s. In addition, the MSBS has the ability to measure unsteady aerodynamic forces. This is based on the basic theorem of kinematics that the sum of the magnetic force and aerodynamic force acting on the model is always equal to the inertial force of the model. Thus, if the inertial force of the model can be accurately evaluated, the aerodynamic force acting on the model can be accurately evaluated in an unsteady state. However, as acceleration is evaluated from the position of the model, the accuracy of evaluating the inertial force of the model decreases remarkably, and it remains to the extent that the unsteady force in the low frequency range can be estimated. In this test, we attempted to evaluate the unsteady aerodynamic forces less than 10 Hz.
4.4. Results and Discussion
Figure 5 shows the position of the model at flow speeds of 10 m/s and 37 m/s. Because the measurement results of the position and attitude of the model contain some noise, they are filtered using a secondary Butterworth-type low-pass filter with a cut-off frequency of 10 Hz. The position fluctuation is less than 0.1 mm when the flow speed is 10 m/s. However, at a flow speed of 37 m/s, which is close to the critical Reynolds number, the position fluctuations of the model are close to 1 mm, indicating a drastic change in the aerodynamic force. Furthermore, the position fluctuation of the model converges at a flow speed of 49 m/s, corresponding to the supercritical range.

Figure 5.
Position fluctuations at two flow speeds: (a) 10 m/s, (b) 37 m/s.
The change in the drag coefficient accompanying the Reynolds number change is shown in Figure 6 together with the measurement values from JAXA [] and Achenbach (1972). In the subcritical Reynolds number range, the drag coefficient obtained in the test is around 0.48, and it is slightly smaller than that of JAXA’s drag coefficient of ~0.49. However, considering that the blockage ratio is small, both these values are considered to be the same. Moreover, considering that both the critical Reynolds number and mainstream turbulence intensity are slightly lower in this test, it is reasonable to consider the influence of the difference in surface roughness, including the connecting part. In this test, the drag coefficient in the supercritical region decreased to a minimum of 0.17 with the increase in Reynolds number and then increased to approximately 0.19. However, as per JAXA’s results, the drag coefficient remained constant at ~0.19 even if the Reynolds number increased. This difference is due to the difference in the transition process pointed out in Reference []. In addition, the aerodynamic coefficient vector projection on a plane perpendicular to the uniform flow direction viewed from behind the models is shown in Figure 6. Around the critical Reynolds number, the aerodynamic force projections on a plane perpendicular to the uniform flow are much larger, and it turns out that they rotate around the wind axis. This phenomenon is similar to that pointed out in reference []. Further, there is a report that the vortex oscillates slowly and rotationally owing to the aerodynamic force caused by the horseshoe vortex generated at the rear of the sphere [].
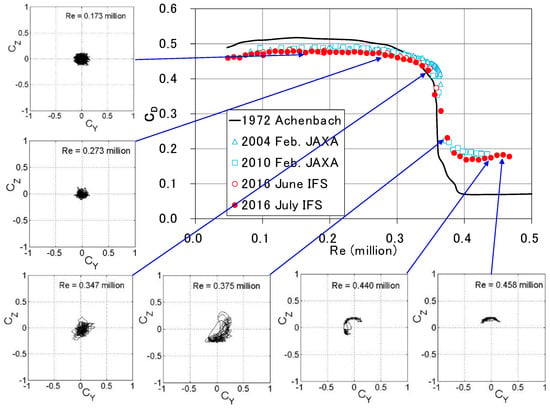
Figure 6.
Drag coefficient vs Reynolds number and lateral force coefficient as seen from behind the sphere.
5. Concluding Remarks
In this paper, the 1-m MSBS developed by IFS was outlined, and as a test example, we introduced the drag measurement of a sphere in the critical Reynolds number range. Drag crisis was observed at Re 3.7 × 105 and large aerodynamic fluctuations, especially in the lateral direction, were observed in the critical Reynolds number range. Therefore, using the 1-m MSBS, we can perform steady and unsteady aerodynamic force measurements without support interference. In addition to the sphere, athletics spear, simple car models (Ahmed body), and standard winged models (AGARD-B) have already been tested using the 1-m MSBS. The 1-m MSBS has the potential to make a significant contribution to the field of fluid science and sports engineering.
Acknowledgments
This work was supported by JSPS KAKENHI Grant Number JP16K06065.
References
- Hoerner, S. Tests of Spheres with Reference to Reynolds Number, Turbulence, and Surface Roughness; NACA-TM-777; National Advisory Committee for Aeronautics: Langley, VA, USA, 1935. [Google Scholar]
- Sawada, H. Wind Tunnels with Magnetic Suspension and Balance System. NAGARE 2014, 33, 267–272. (In Japanese) [Google Scholar]
- Kohama, Y.; Kobayashi, R.; Ito, H. Tohoku University Low-Turbulence Wind Tunnel. In Proceedings of the AIAA 17th Aerospace Ground Testing Conference, Nashville, TN, USA, 6–8 July 1992. [Google Scholar]
- Sawada, H. A Magnetic Suspension and Balance System for Wind Tunnels. Inst. Fluid Sci. Tohoku Univ. 2012, 24, 1–12. [Google Scholar]
- Sawada, H.; Suda, S. Study on aerodynamic force acting on a sphere with and without boundary layer trips around the critical Reynolds number with a magnetic suspension and balance system. Exp. Fluids 2011, 50, 271–284. [Google Scholar] [CrossRef]
- Taneda, S. Visual observations of the flow past a sphere at Reynolds numbers between 104 and 106. J. Fluid Mech. 1978, 85, 187–192. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).