The Influence of the Phase Difference between the Crank Angle of the Pilot and that of the Stoker on the Drag Acting on a Tandem Bike †
Abstract
:1. Introduction
2. Methods
2.1. Full-Scale Model
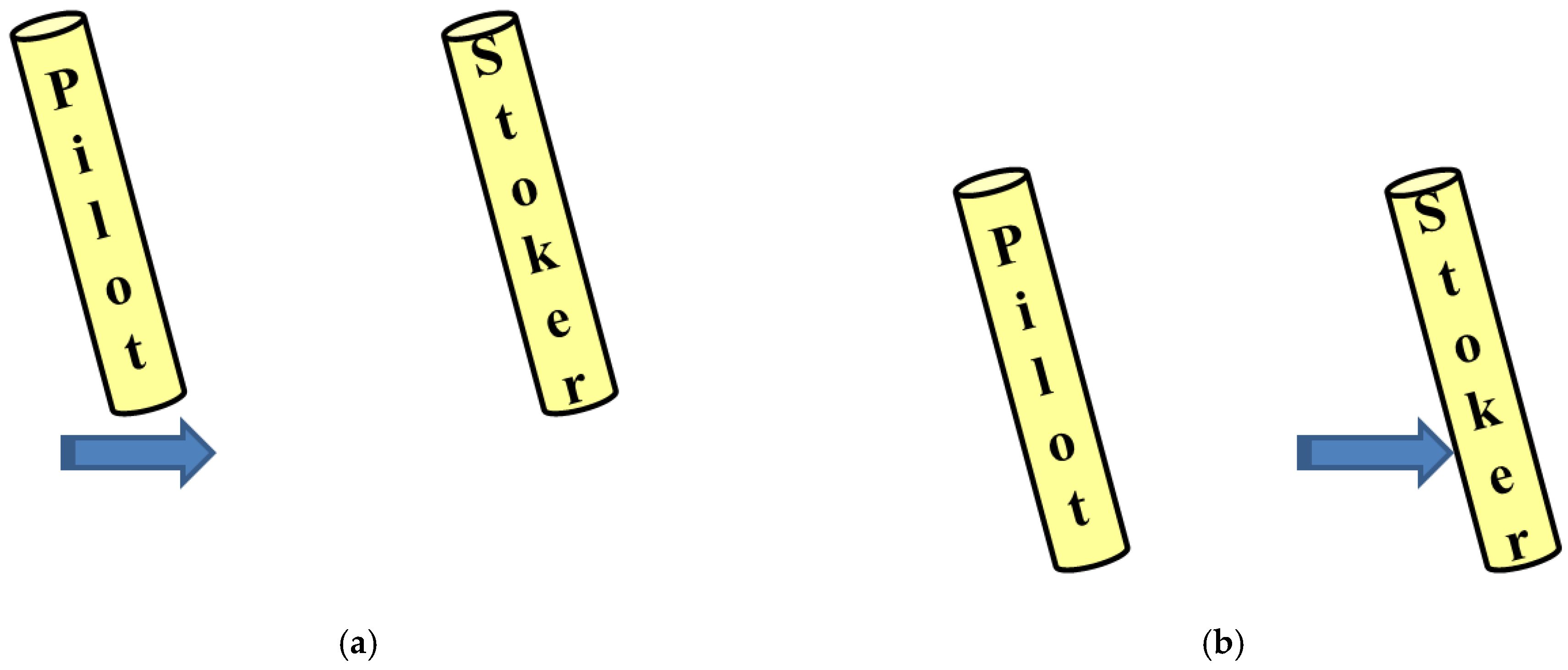
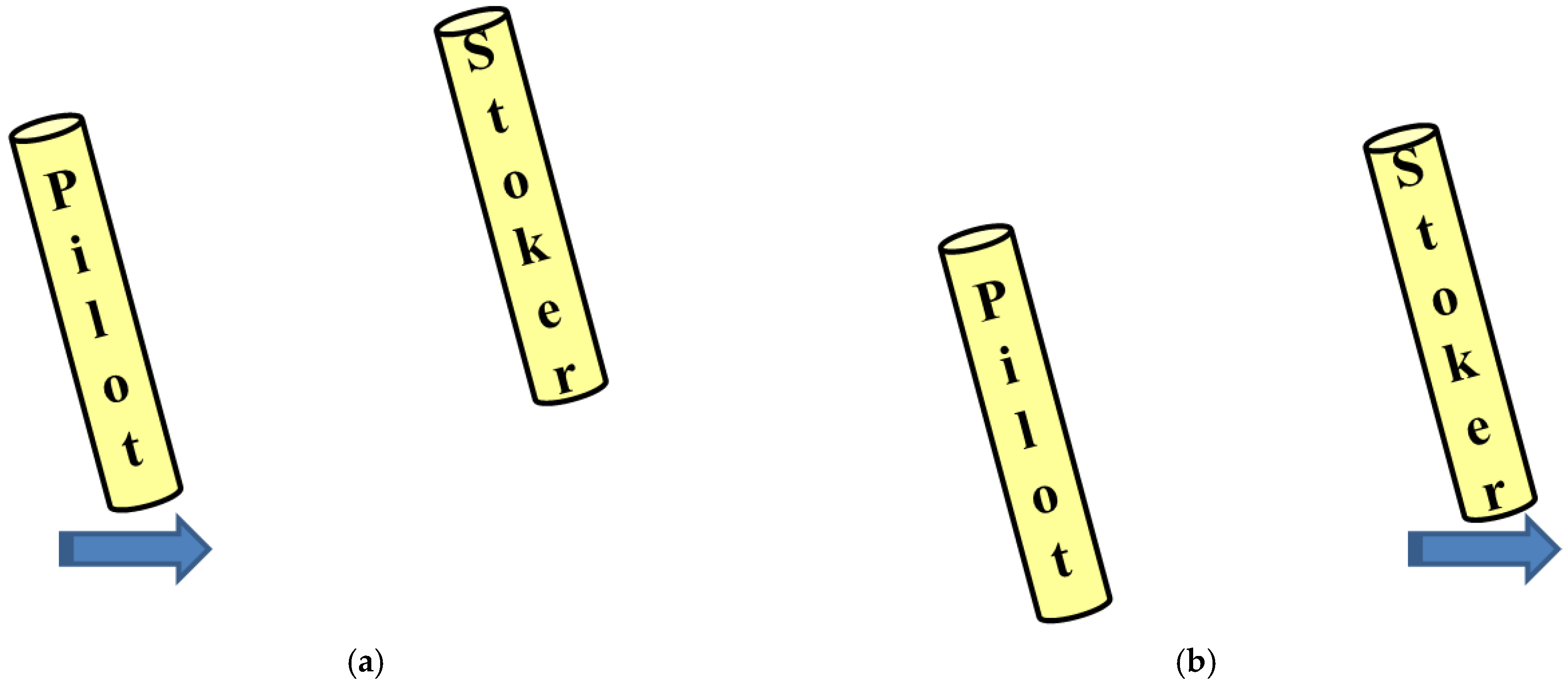
2.2. Two Oscillating Cylinders in the Tandem Arrangement
3. Results and Discussions
Drag Measurements
4. Conclusions
Acknowledgments
References
- Barry, N.; Burton, D.; Sheridan, J.; Thompson, M.; Brown, N.A.T. An analysis of the wake of pedalling cyclists in a tandem Formation. Procedia Eng. 2016, 147, 7–12. [Google Scholar] [CrossRef]
- Crouch, T.N.; Burton, D.; Brown, N.A.T.; Thompson, M.C.; Sheridan, J. Flow topology in the wake of a cyclist and its effect on aerodynamic drag. J. Fluid Mech. 2014, 748, 5–35. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Seo, K.; Eda, S. The Influence of the Phase Difference between the Crank Angle of the Pilot and that of the Stoker on the Drag Acting on a Tandem Bike. Proceedings 2018, 2, 209. https://doi.org/10.3390/proceedings2060209
Seo K, Eda S. The Influence of the Phase Difference between the Crank Angle of the Pilot and that of the Stoker on the Drag Acting on a Tandem Bike. Proceedings. 2018; 2(6):209. https://doi.org/10.3390/proceedings2060209
Chicago/Turabian StyleSeo, Kazuya, and Satoshi Eda. 2018. "The Influence of the Phase Difference between the Crank Angle of the Pilot and that of the Stoker on the Drag Acting on a Tandem Bike" Proceedings 2, no. 6: 209. https://doi.org/10.3390/proceedings2060209
APA StyleSeo, K., & Eda, S. (2018). The Influence of the Phase Difference between the Crank Angle of the Pilot and that of the Stoker on the Drag Acting on a Tandem Bike. Proceedings, 2(6), 209. https://doi.org/10.3390/proceedings2060209





