Kerr Effect and Dielectric Anisotropy in Series of Rare-Earth LC Complexes with Systematically Changing Counterions Structure †
Abstract
1. Introduction
2. Methods
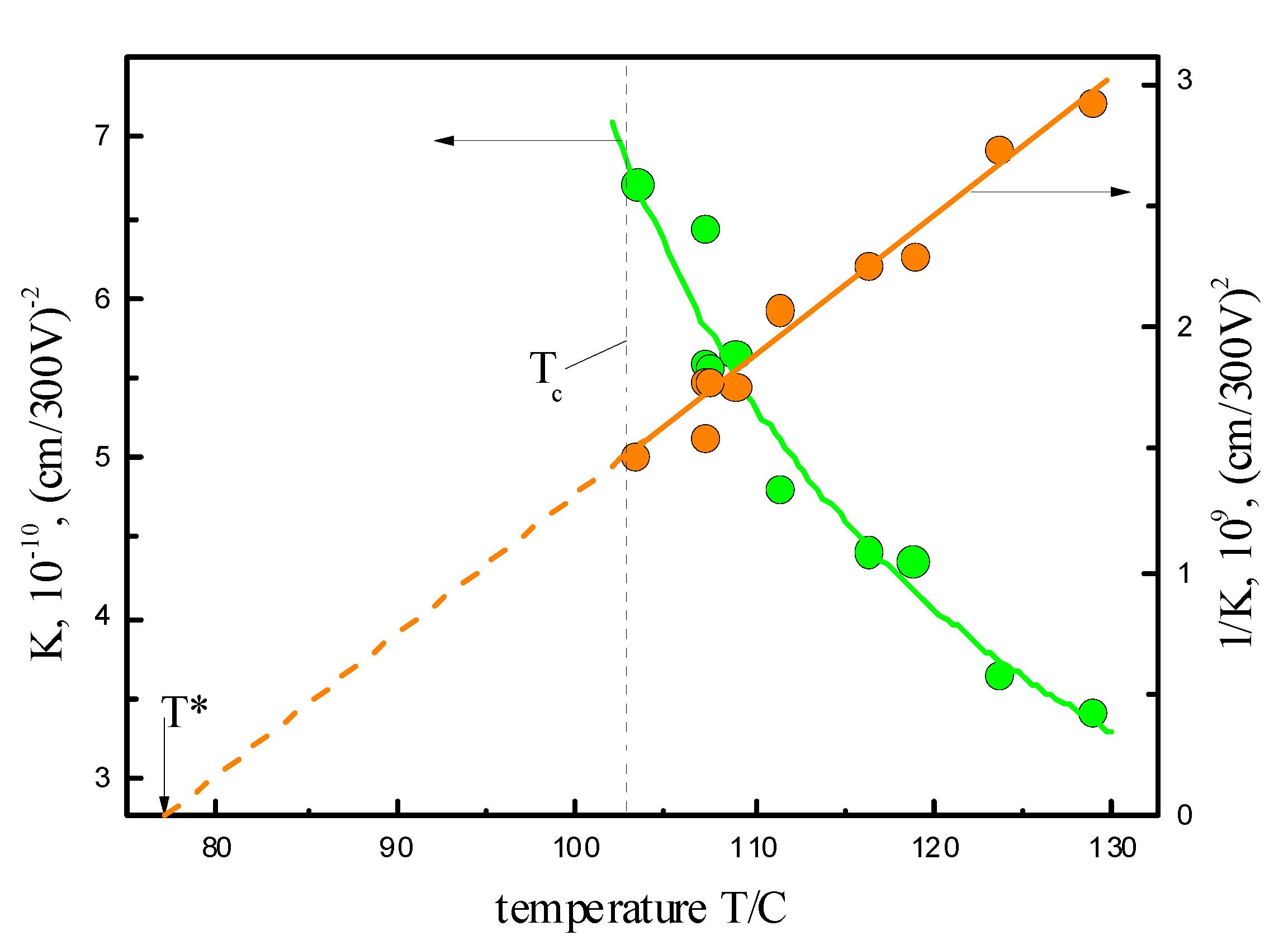
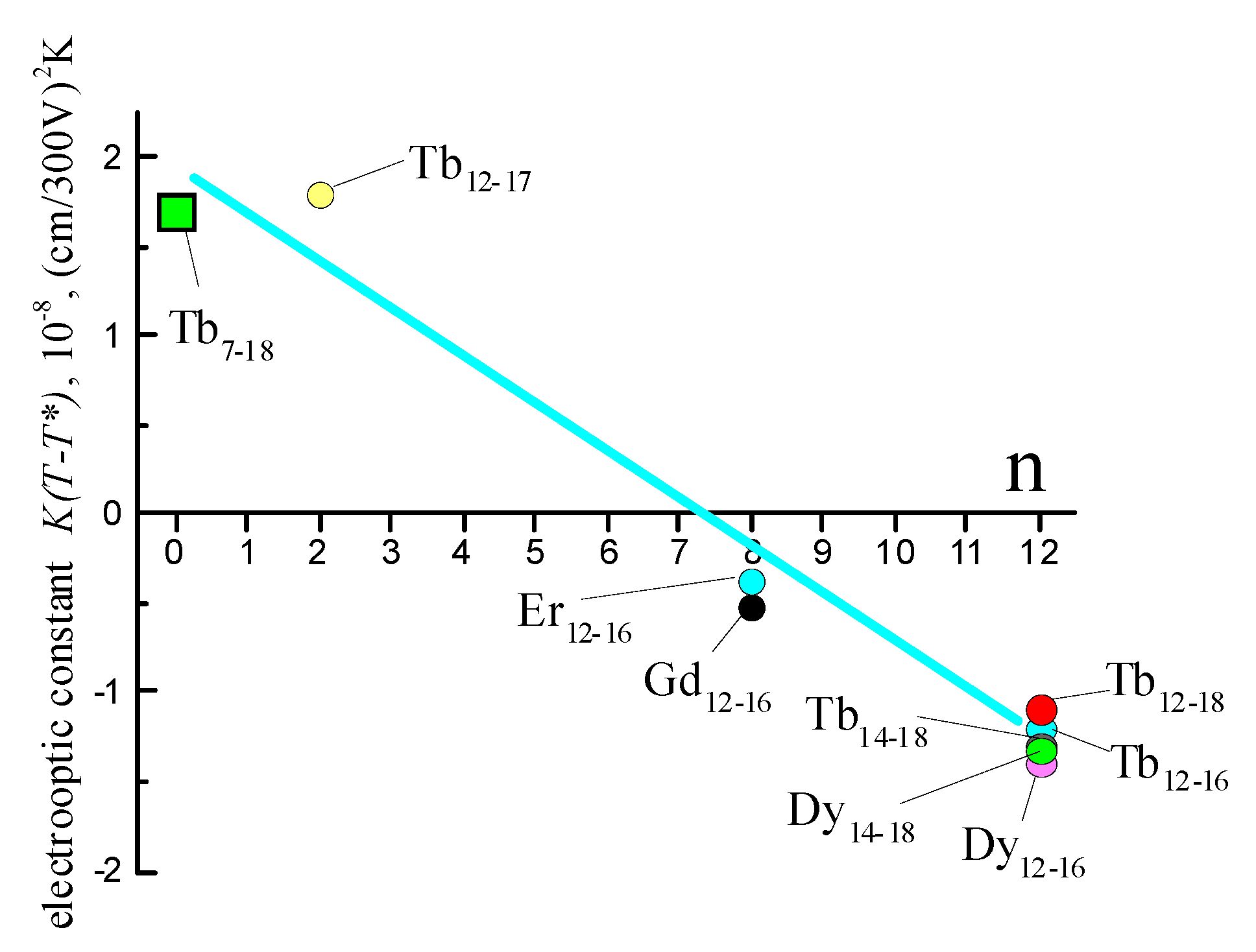
3. Results and Discussion

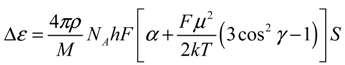
Author Contributions
Acknowledgments
Conflicts of Interest
References
- De Gennes, P.G. Short range order effects in the isotropic phase of nematics and cholesterics. Mol. Cryst. Liq. Cryst. 1971, 12, 193–214. [Google Scholar] [CrossRef]
- Coles, H.J. Laser and electric field induced birefringence studies on the cyanobiphenyl homologues. Mol. Cryst. Liq. Cryst. 1978, 49, 67–74. [Google Scholar] [CrossRef]
- Yamamoto, R.; Ishihara, S.; Hayakawa, S.; Morimoto, K. The Kerr constants and relaxation times in the isotropic phase of nematic homologous series. Phys. Lett. 1978, 69A, 276–278. [Google Scholar] [CrossRef]
- Tsvetkov, N.V.; Rjumtsev, E.I. Kerr-effect in the isotropic liquid phase of nematogens. Mol. Cryst. Liq. Cryst. 1986, 133, 125–134. [Google Scholar] [CrossRef]
- Ryumtsev, E.I.; Polushin, S.G.; Tarasenko, K.N.; Kovshik, A.P. Equilibrium and dynamic electrooptical properties of nematic and isotropic phases of 4-n-alkoxy-4′-cyanobiphenyls. Zhurnal Fizicheskoi Khimii 1995, 69, 940–943. [Google Scholar]
- Serrano, J.L. Metallomesogenes, Synthesis, Properties and Applications; VCH: New York, NY, USA, 1996. [Google Scholar] [CrossRef]
- Binnemans, K.; Gorller-Walrand, C. Lanthanide-containing liquid crystals and surfactants. Chem. Rev. 2002, 102, 2303–2345. [Google Scholar] [CrossRef]
- Galyametdinov, Y.G.; Haase, W.; Malykhina, L.; Prosvirin, A.; Bikchantaev, I.; Rakmatullin, A.; Binnemans, K. Synthesis, mesomorphism, and unusual magnetic behaviour of lanthanide complexes with perfluorinated counterions. J. Chem. Eur. 2001, 7, 99–105. [Google Scholar] [CrossRef]
- Galyametdinov, Y.G.; Haase, W.; Goderis, B.; Moors, D.; Driesen, K.; Van Deun, R.; Binnemans, K. Magnetic alignment study of rare-earth-containing liquid crystals. J. Phys. Chem. B 2007, 111, 13881–13885. [Google Scholar] [CrossRef][Green Version]
- Bünzly, J.C.; Piguet, C. Taking advantage of luminescent lanthanide ions. Chem. Soc. Rev. 2005, 34, 1048–1077. [Google Scholar] [CrossRef]
- Eliseeva, S.V.; Bünzli, J.C. Lanthanide luminescence for functional materials and bio-sciences. Chem. Soc. Rev. 2010, 39, 189–227. [Google Scholar] [CrossRef]
- Van Deun, R.А.; Parac-Vogt, T.N.; Van Hecke, K.; Van Meervelt, L.; Binnemans, K.; Guillon, D.; Donnio, B. Liquid-crystalline azines formed by the rare-earth promoted decomposition of hydrazide «habbe» ligands: Structural and thermal properties. J. Mater. Chem. 2003, 13, 1639–1645. [Google Scholar] [CrossRef]
- Cardinaels, T.; Driesen, K.; Parac-Vogt, T.N.; Heinrich, B.; Bourgogne, C.; Guillon, D.; Donnio, B.; Binnemans, K. Design of high coordination number metallomesogens by decoupling of the complex-forming and mesogenic groups: Nematic and lamello-columnar mesophases. Chem. Mater. 2005, 17, 6589–6598. [Google Scholar] [CrossRef]
- Galyametdinov, Y.G.; Knyazev, A.A.; Dzhabarov, V.I.; Cardinaels, T.; Driesen, K.; Gorller-Walrand, C.; Binnemans, K. Polarized luminescence from aligned samples of nematogenic lanthanide complexes. Adv. Mater. 2008, 20, 252257. [Google Scholar] [CrossRef]
- Dzhabarov, V.I.; Knyazev, A.A.; Strelkov, M.V.; Molostova, E.Y.; Schustov, V.A.; Haase, W.; Galyametdinov, Y.G. Tris(β-diketonates) lanthanum nematic adducts. Liq. Cryst. 2010, 37, 285–291. [Google Scholar] [CrossRef]
- Kovshik, A.P.; Krainyukov, E.S.; Kovshik, S.A.; Knyazev, A.A.; Galyametdinov, Y.G.; Ryumtsev, E.I. Optical anisotropy of liquid_crystal lanthanide complexes. Opt. Spectrosc. 2014, 116, 56–61. [Google Scholar] [CrossRef]
- Dobrun, L.A.; Sakhatskii, A.S.; Kovshik, A.P.; Ryumtsev, E.I.; Knyazev, A.A.; Galyametdinov, Y.G. Dielectric properties of an ytterbium_based nematic liquid_crystal complex. JETP Lett. 2014, 99, 133–135. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Liquid Crystal; Cambridge University Press: Cambridge, UK, 1977. [Google Scholar]
- Polushin, S.G.; Rogozhin, V.B.; Ryumtsev, E.I.; Galyametdinov, Y.G. Electrooptical properties of mesogenic rare earth complexes in isotropic melts. Dokl. Phys. Chem. 2014, 455, 64–66. [Google Scholar] [CrossRef]
- Polushin, S.G.; Rogozhin, V.B.; Ryumtsev, E.I.; Lezov, A.V. The Kerr effect in the vicinity of the transition from the isotropic to smectic-A phase. Russ. J. Phys. Chem. A 2006, 80, 1016–1020. [Google Scholar] [CrossRef]
- Dunmur, D.A.; Tomes, A.E. The Pretransitional Kerr effect in 4-n-pentyl-4′-cyanobiphyenyl. Mol. Cryst. Liq. Cryst. 1981, 76, 231–240. [Google Scholar] [CrossRef]
- Maier, W.; Meier, G. Eine einfache theorie der dielektrischen eigenschaften homogen orientierter kristallinglussiger phasen des nematischen typs. Z. Naturforsch. 1961, 16A, 262–267. [Google Scholar] [CrossRef]
- Ryumtsev, E.I.; Rotinyan, T.A.; Kovshik, A.P.; Tsvetkov, V.N. Dispersion of electric birefringence in isotropic phase of nematic liquid crystals. Sov. Phys. Crystallogr. 1982, 27, 1143–1147. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zakhryapa, A.; Rogozhin, V.; Lezova, I.; Polushina, G.; Polushin, S. Kerr Effect and Dielectric Anisotropy in Series of Rare-Earth LC Complexes with Systematically Changing Counterions Structure. Proceedings 2018, 2, 1123. https://doi.org/10.3390/IECC_2018-05258
Zakhryapa A, Rogozhin V, Lezova I, Polushina G, Polushin S. Kerr Effect and Dielectric Anisotropy in Series of Rare-Earth LC Complexes with Systematically Changing Counterions Structure. Proceedings. 2018; 2(14):1123. https://doi.org/10.3390/IECC_2018-05258
Chicago/Turabian StyleZakhryapa, Artyom, Viacheslav Rogozhin, Irina Lezova, Galina Polushina, and Sergey Polushin. 2018. "Kerr Effect and Dielectric Anisotropy in Series of Rare-Earth LC Complexes with Systematically Changing Counterions Structure" Proceedings 2, no. 14: 1123. https://doi.org/10.3390/IECC_2018-05258
APA StyleZakhryapa, A., Rogozhin, V., Lezova, I., Polushina, G., & Polushin, S. (2018). Kerr Effect and Dielectric Anisotropy in Series of Rare-Earth LC Complexes with Systematically Changing Counterions Structure. Proceedings, 2(14), 1123. https://doi.org/10.3390/IECC_2018-05258




