Piezoelectric Sensors for Lamb Waves’ Direction of Arrival (DoA) Estimation †
Abstract
:1. Introduction
2. Propagation of Uncertainty in DoA Estimation
3. The Radon Transform as Sensor Design Tool
 , and in case of a non-dispersive propagation, i.e., k0(ω) = ω/vg, we have that:
, and in case of a non-dispersive propagation, i.e., k0(ω) = ω/vg, we have that:- (1).
- The frequency responses VP1(ω) e VP2(ω) are equal a part from a scaling factor and a linear phase-shift in θ. Then, it is possible to use the cross-correlation method for estimating the DoA.
- (2).
- Antitransforming according to Fourier, vP1(t) e vP2(t) are equal except for a scale factor and a linear time-shift in θ, obtaining the desired target: Δt = (ρ0 + αθ)/vg.
Sensor Directivity Analysis and Synthesis
 . The shape synthesis procedure exploits the fact that the Radon transform can be inverted with suitable algorithms. Then, defined the geometry of the piezo patch P1 (ϕ1(x, y)) (e.g., a conventional disk), computed the Radon transform R1θ(ρ) of the shape ϕ1(x, y), the desired Radon transform of the shape ϕ2(x, y) is
. The shape synthesis procedure exploits the fact that the Radon transform can be inverted with suitable algorithms. Then, defined the geometry of the piezo patch P1 (ϕ1(x, y)) (e.g., a conventional disk), computed the Radon transform R1θ(ρ) of the shape ϕ1(x, y), the desired Radon transform of the shape ϕ2(x, y) is
 . In this way, in case of non-dispersive propagation, i.e., k0(ω) = ω/vg, vP1(t) and vP3(t) are shifted by a constant Δt13 = ρ3/vg. This relation clearly highlights how to estimate vg from Δt13. To obtain the aforementioned relationship between the directivity functions, it is sufficient to impose a relation between the Radon transforms of the shape functions ϕ1 and ϕ3 as follows:
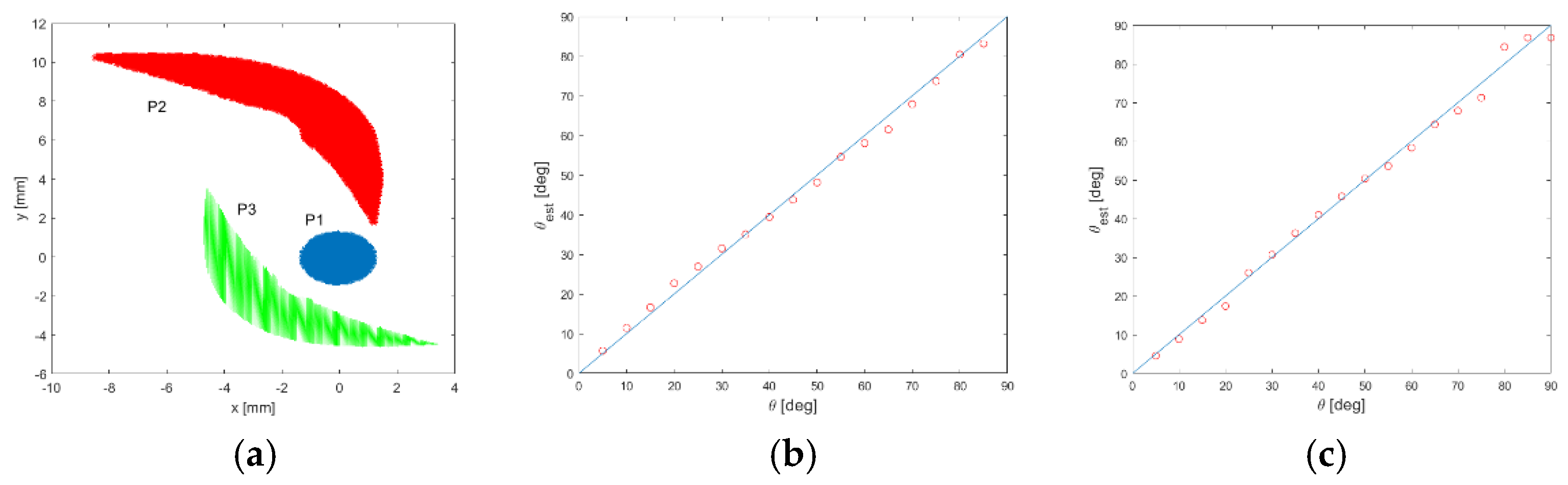
. In this way, in case of non-dispersive propagation, i.e., k0(ω) = ω/vg, vP1(t) and vP3(t) are shifted by a constant Δt13 = ρ3/vg. This relation clearly highlights how to estimate vg from Δt13. To obtain the aforementioned relationship between the directivity functions, it is sufficient to impose a relation between the Radon transforms of the shape functions ϕ1 and ϕ3 as follows: 4. Numerical Validation
5. Conclusions
References
- Kundu, T.; Das, S.; Jata, K. Detection of the point of impact on a stiffened plate by the acoustic emission technique. Smart Mater. Struct. 2009, 18, 035006. [Google Scholar] [CrossRef]
- De Marchi, L.; Testoni, N.; Marzani, A. Spiral-shaped piezoelectric sensors for Lamb waves direction of arrival (DoA) estimation. Smart Mater. Struct. 2018, 27, 045016. [Google Scholar] [CrossRef]
- Senesi, M.; Ruzzene, M. A frequency selective acoustic transducer for directional lamb wave sensing. J. Acoust. Soc. Am. 2011, 130, 1899–1907. [Google Scholar] [CrossRef] [PubMed]
- Gaskill, J.D. Linear Systems, Fourier Transforms, and Optics; Wiley: New York, NY, USA, 1978. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marchi, L.D.; Dibiase, M.; Testoni, N. Piezoelectric Sensors for Lamb Waves’ Direction of Arrival (DoA) Estimation. Proceedings 2018, 2, 806. https://doi.org/10.3390/proceedings2130806
Marchi LD, Dibiase M, Testoni N. Piezoelectric Sensors for Lamb Waves’ Direction of Arrival (DoA) Estimation. Proceedings. 2018; 2(13):806. https://doi.org/10.3390/proceedings2130806
Chicago/Turabian StyleMarchi, Luca De, Marco Dibiase, and Nicola Testoni. 2018. "Piezoelectric Sensors for Lamb Waves’ Direction of Arrival (DoA) Estimation" Proceedings 2, no. 13: 806. https://doi.org/10.3390/proceedings2130806
APA StyleMarchi, L. D., Dibiase, M., & Testoni, N. (2018). Piezoelectric Sensors for Lamb Waves’ Direction of Arrival (DoA) Estimation. Proceedings, 2(13), 806. https://doi.org/10.3390/proceedings2130806





