Fuzzy Linear Regression of Rainfall-Altitude Relationship †
Abstract
:1. Introduction
2. Mathematical Model
2.1. Model of Tzimopoulos et al. (2006)
2.1.1. Generalities
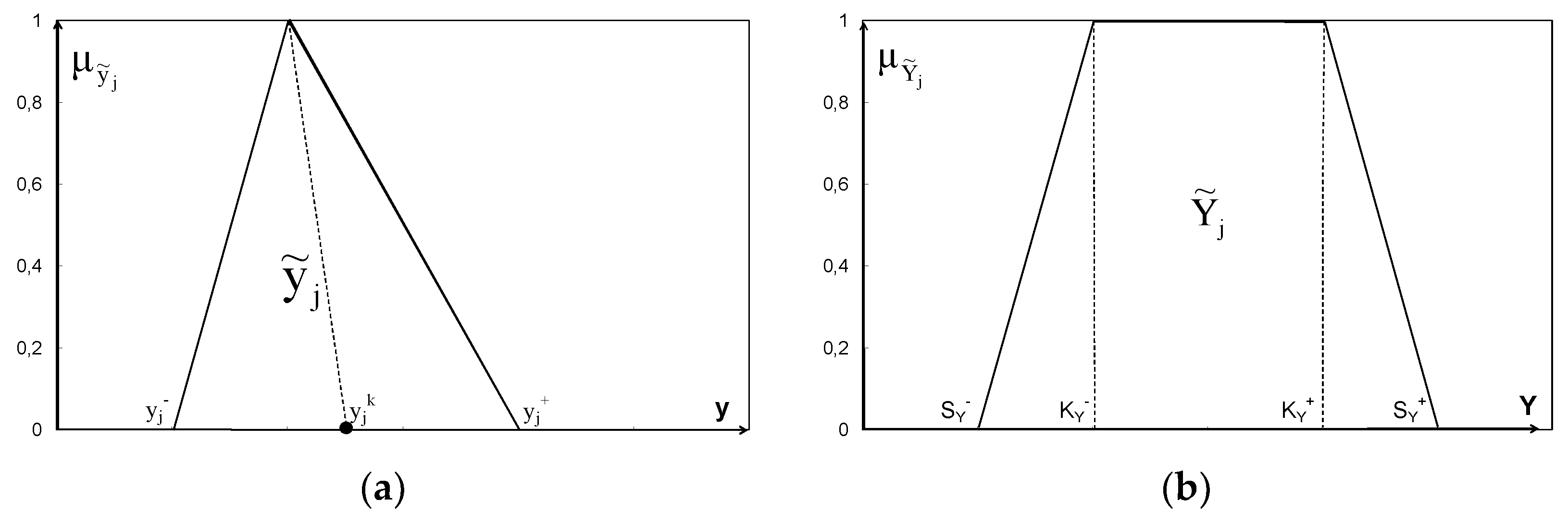
2.1.2. Step 1
2.1.3. Step 2
3. Applications
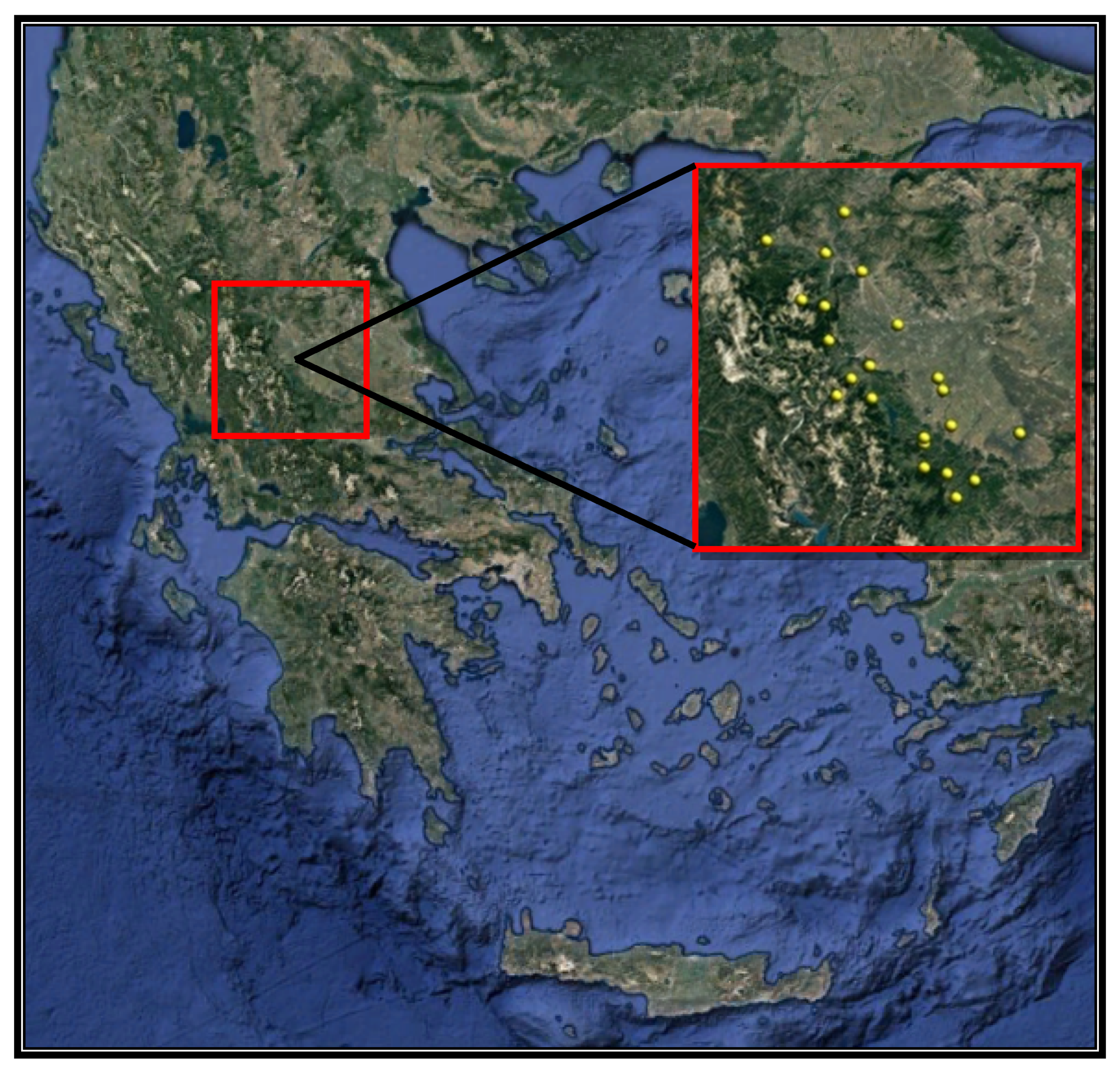
3.1. Generalities
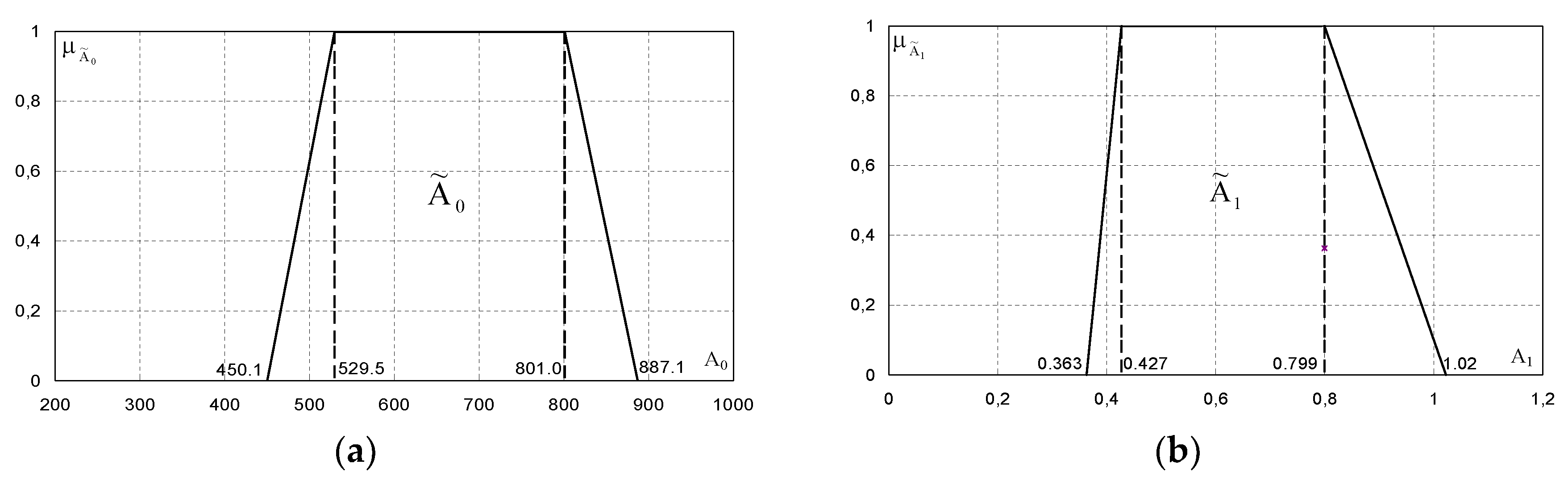
3.2. Step 1
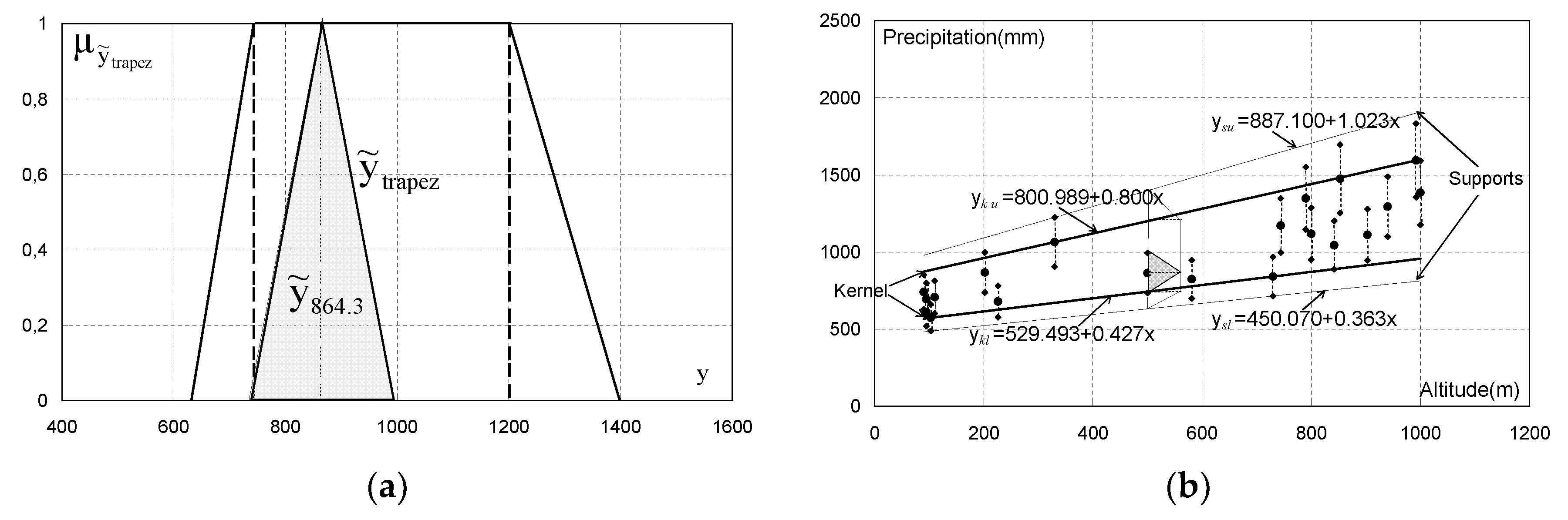
3.3. Step 2
4. Comments-Conclusions
References
- Zadeh, L.A. Fuzzy Sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Savic, D.A.; Pedrycz, W. Evaluation of fuzzy linear regression models. Fuzzy Sets Syst. 1991, 39, 51–63. [Google Scholar] [CrossRef]
- Papadopoulos, B.; Sirpi, M. Similarities in Fuzzy Regression Models. J. Optim. Theory Appl. 1999, 102, 373–383. [Google Scholar] [CrossRef]
- Papadopoulos, B.; Sirpi, M. Similarities and Distances in Fuzzy Regression Models. Soft Comput. 2004, 8, 556–561. [Google Scholar] [CrossRef]
- Redden, D.T.; Woodall, W.H. Further examination of fuzzy linear regression. Fuzzy Sets Syst. 1996, 79, 203–211. [Google Scholar] [CrossRef]
- Shapiro, A.F.; Berry-Stölzle, T.R.; Koissi, M.-C. The Fuzziness in Regression Models. ARC2009_Shapiro_06 PDF. 2009; p. 6.
- Tanaka, H.; Uejima, S.; Asai, K. Linear regression analysis with fuzzy models. IEEE Trans. Syst. Man Cybern. 1982, 12, 903–907. [Google Scholar]
- Tanaka, H. Fuzzy data analysis by possibilistic linear models. Fuzzy Sets Syst. 1987, 24, 363–375. [Google Scholar] [CrossRef]
- Tanaka, H.; Watada, J. Possibilistic linear systems and their application to the linear regression model. Fuzzy Sets Syst. 1988, 27, 275–289. [Google Scholar] [CrossRef]
- Tanaka, H.; Hayashi, I.; Watada, J. Possibilistic linear regression analysis for fuzzy data. EJOR 1989, 40, 389–396. [Google Scholar] [CrossRef]
- Tanaka, H.; Ishibuchi, H. Identification of possibilistic linear systems by quadratic membership functions of fuzzy parameters. Fuzzy Sets Syst. 1991, 41, 145–160. [Google Scholar] [CrossRef]
- Tzimopoulos, C.; Papadopoulos, Β. Fuzzy Logic with Application in Engineering; Ziti: Thessaloniki, Greece, 2013; 627p. (In Greek) [Google Scholar]
- Tzimopoulos, C.; Papadopoulos, K.; Papadopoulos, B. Fuzzy Regression with Applications in Hydrology. IJEIT 2016, 5, 69–75. [Google Scholar]
- Bisserier, A. Une approche Paramétrique de la Régression Linéaire Floue-Formalisation par Intervalles. Ph.D. Thesis, Université de Savoie, Chambéry, France, 2010. [Google Scholar]
- Bisserier, A.; Boukezzoula, R.; Galichet, S. Linear Fuzzy Regression Using Trapezoidal Fuzzy Intervals. In Proceedings of the IPMU’08, Torremolinos, Spain, 22–27 June 2008; pp. 181–188. [Google Scholar]
- Bisserier, A.; Boukezzoula, R.; Galichet, S. Linear Fuzzy Regression Using Trapezoidal Fuzzy Intervals. JUS 2010, 4, 59–72. [Google Scholar]
- Bisserier, A.; Boukezzoula, R.; Galichet, S. A revisited approach to linear fuzzy regression using trapezoidal fuzzy intervals. Inf. Sci. 2010, 180, 3653–3673. [Google Scholar] [CrossRef]
- Bisserier, A.; Boukezzoula, R.; Galichet, S. Linear Fuzzy Regression Using Trapezoidal Fuzzy Intervals. In Foundations of Reasoning under Uncertainty; Bouchon-Meunier, B., Magdalena, L., Ojeda-Aciego, M., Verdegay, J.L., Yager, R., Eds.; Springer: Berlin, Germany, 2010; Volume 249, pp. 2–22. [Google Scholar]
- Charfeddine, S. Optimisation de l’ offre d’ une Compagnie Aérienne en Environnement Incertain. Thèse de Docteur; Université de Toulouse: Toulouse, France, 2004. [Google Scholar]
- Charfeddine, S.; Mora-Camino, F.; De Coligny, M. Fuzzy linear regression: Application to the estimation of air transport demand. In Proceedings of the International Conference on Fuzzy Sets and Soft Computing in Ecomomics and Finance, Saint-Petersburg, Russia, 17–20 June 2004; pp. 350–359. [Google Scholar]
- Charfeddine, S.; Zbidi, K.; Mora-Camino, F. Fuzzy Regression Analysis using Trapezoidal Fuzzy Numbers. In Proceedings of the EUSFLAT—LFA 2005, Barcelona, Spain, 7–9 September 2005; pp. 1213–1218. [Google Scholar]
- Ganesan, K.; Veeramani, P. Fuzzy Linear Programs with Trapezoidal Fuzzy Numbers. Ann. Oper. Res. 2006, 143, 305–315. [Google Scholar] [CrossRef]
- Kheirfam, B.; Verdegay, J.L. The dual simplex method and sensitivity analysis for fuzzy linear programming with symmetric trapezoidal numbers. Fuzzy Optim. Decis. Mak. 2013, 12, 171–189. [Google Scholar] [CrossRef]
- Kumar, A.; Kaur, J. A New Method for Solving Fuzzy Linear Programs with Trapezoidal Fuzzy Numbers. J. Fuzzy Set Valued Anal. 2011, 3, 103–118. [Google Scholar] [CrossRef]
- Fung, R.Y.K.; Chen, Y.; Tang, J. Estimating the functional relationships for quality function deployment under uncertainties. Fuzzy Sets Syst. 2006, 157, 98–120. [Google Scholar] [CrossRef]
| M. Stations | Kallifonio | Grammatiko | Karditsomag. | Karditsa | Trikala | Kalamapaka | Mouza. Ypex | Rahoula | Meg_Ker_Ypex | Agiofyllo |
|---|---|---|---|---|---|---|---|---|---|---|
| X (m) | 90 | 95 | 95 | 103 | 110 | 202 | 226 | 330 | 500 | 581 |
| Y (mm) | 741 | 611 | 694 | 573 | 707 | 867 | 679 | 1065 | 864 | 823 |
| e = 0.15Y | 111,11071 | 91,66502743 | 104,089286 | 86,02214 | 106,06393 | 130,10625 | 101,859934 | 159,726 | 129,6461372 | 123,495 |
| M. Stations | Loutr._Ypex | Amarantos | Moloha | Bathylakkos | Malakasio | Brontero | Rentina | Chrisomilia | Argithea | Kerasia |
|---|---|---|---|---|---|---|---|---|---|---|
| X (m) | 730 | 744 | 790 | 800 | 842 | 853 | 903 | 940 | 992 | 1000 |
| Y (mm) | 841 | 1172 | 1348 | 1118 | 1045 | 1475 | 1112 | 1294 | 1594 | 1384,9 |
| e = 0.15Y | 126,18743 | 175,8080864 | 202,244286 | 167,6779 | 156,731302 | 221,27336 | 166,768142 | 194,169 | 239,1214286 | 207,7294 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tzimopoulos, C.; Evangelides, C.; Vrekos, C.; Samarinas, N. Fuzzy Linear Regression of Rainfall-Altitude Relationship. Proceedings 2018, 2, 636. https://doi.org/10.3390/proceedings2110636
Tzimopoulos C, Evangelides C, Vrekos C, Samarinas N. Fuzzy Linear Regression of Rainfall-Altitude Relationship. Proceedings. 2018; 2(11):636. https://doi.org/10.3390/proceedings2110636
Chicago/Turabian StyleTzimopoulos, Christos, Christos Evangelides, Christos Vrekos, and Nikiforos Samarinas. 2018. "Fuzzy Linear Regression of Rainfall-Altitude Relationship" Proceedings 2, no. 11: 636. https://doi.org/10.3390/proceedings2110636
APA StyleTzimopoulos, C., Evangelides, C., Vrekos, C., & Samarinas, N. (2018). Fuzzy Linear Regression of Rainfall-Altitude Relationship. Proceedings, 2(11), 636. https://doi.org/10.3390/proceedings2110636






