Experiments and Numerical Analysis of Flow in an Open Channel with Gravel Bed †
Abstract
1. Introduction
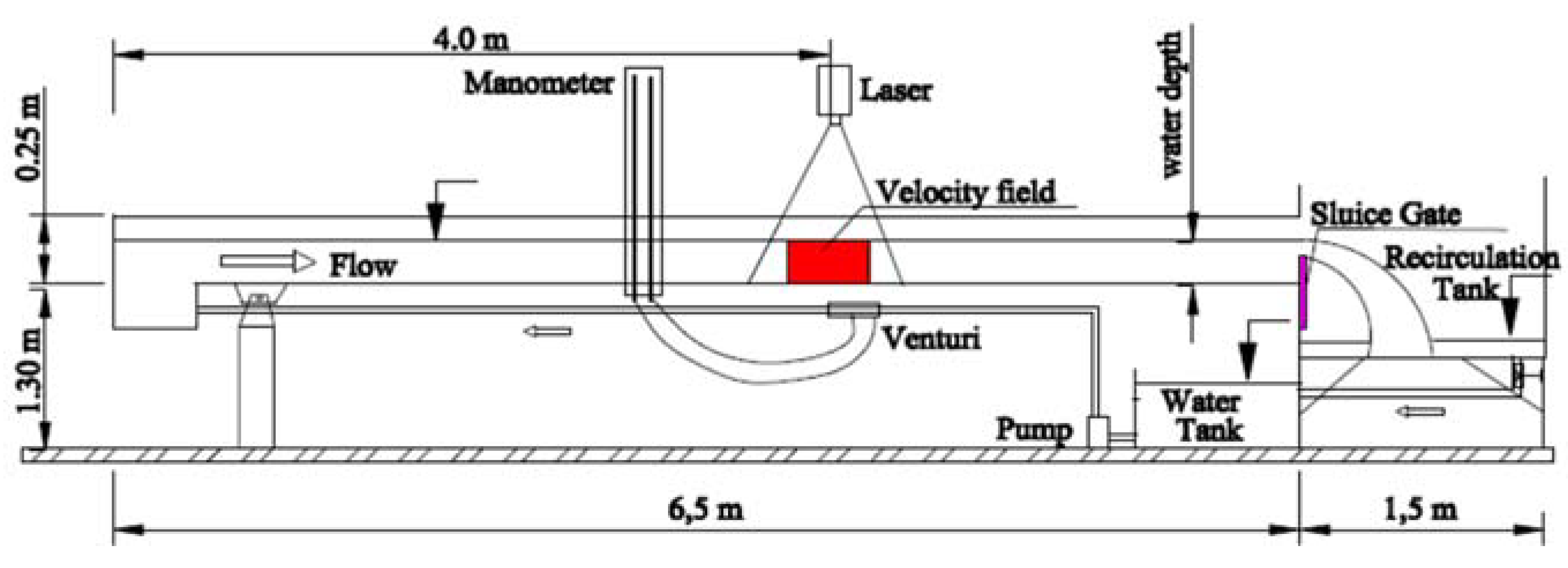
2. Experimental Procedure—Measurements
3. Mathematical Model and Numerical Simulation
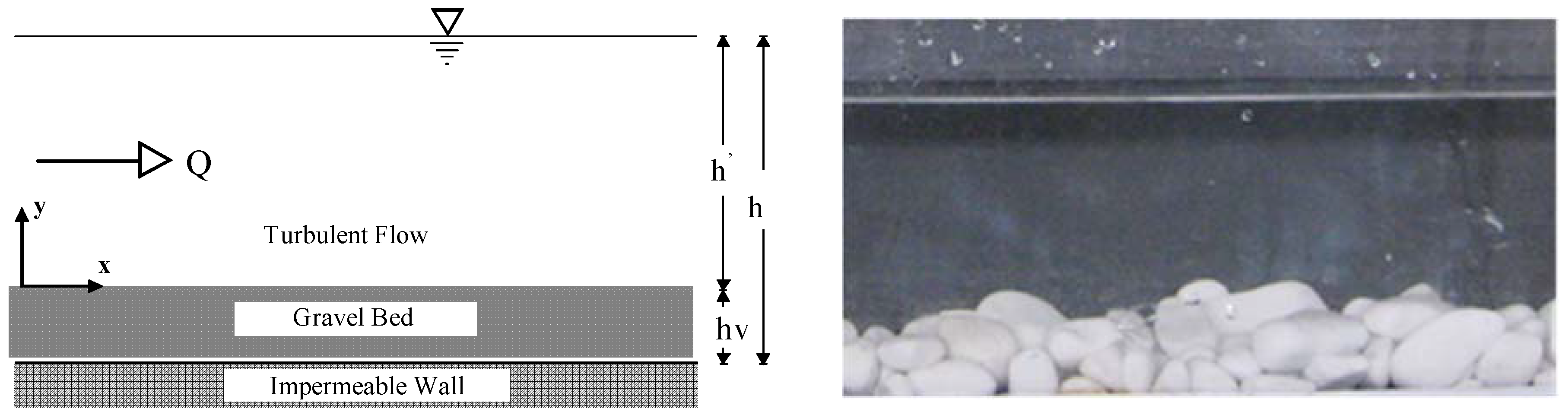
3.1. Mathematical Model
3.2. Boundary Conditions and Mesh Design
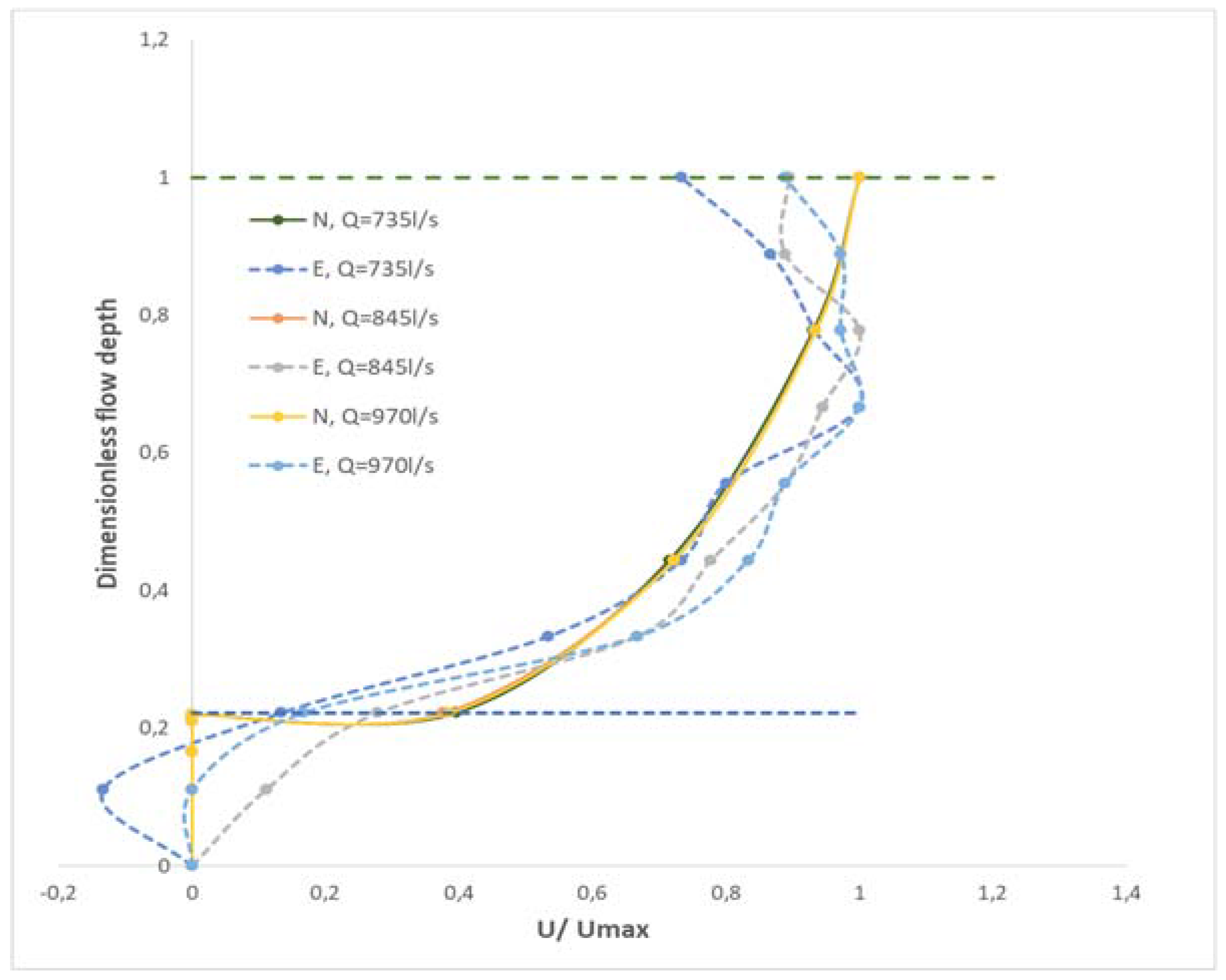
4. Results
5. Conclusions
Author Contributions
References
- Schalchi, U. The clogging of coarse gravel river beds by fine sediment. Hydrobiologia 1992, 235, 189–197. [Google Scholar] [CrossRef]
- Brunke, M.; Gonser, T. The ecological significance of exchange processes between rivers and groundwater. Freshw. Biol. 1997, 37, 1–33. [Google Scholar] [CrossRef]
- Keramaris, E. Comparison between different porous bed (porous filter and rods bundle) in open channels. J. Porous Media 2010, 13, 183–193. [Google Scholar] [CrossRef]
- Pechlivanidis, G.; Keramaris, E.; Pechlivanidis, I. Experimental Study of the Effects of Permeable Bed (Grass Vegetation and Gravel Bed) on the Turbulent Flow Using Particle Image Velocimetry. J. Turbul. 2015, 16, 1–16. [Google Scholar] [CrossRef]
- Keramaris, E. Effects of inclined impermeable bed on turbulent characteristics of the flow using particle image velocimetry. J. Turbul. 2015, 16, 540–554. [Google Scholar] [CrossRef]
- Keramaris, E.; Pechlivanidis, G. The influence of transition from vegetation to gravel bed and vice versa in open channel using the PIV method. Water Utility J. 2015, 10, 37–43. [Google Scholar]
- Keramaris, E.; Pechlivanidis, G.; Pechlivanidis, I. The different impact of a half-separated gravel and vegetated bed in open channels. Environ. Process. 2015, 2, 123–132. [Google Scholar] [CrossRef][Green Version]
- Guo, J.; Zhang, J. Velocity distribution in laminar and turbulent vegetated flow. J. Hydraul. Res. 2016, 54, 117–130. [Google Scholar] [CrossRef]
- Voermans, J.J.; Ghisalberti, M.; Ivey, G.N. The variation of flow and turbulence across the sediment-water interface. J. Fluid Mech. 2017, 824, 413–437. [Google Scholar] [CrossRef]
- Klopstra, D.; Bameveld, H.J.; Van Noortwijk, J.M.; Van Velzen, E.H. Analytical model of hydraulic roughness of submerged vegetation. Proceedings of the 27th IAHR Congress, San Francisco, CA, USA, 10–15 August 1997; American Society of Civil Engineers: New York, NY, USA, 1997; pp. 775–780. [Google Scholar]
- Meijer, D.G.; Van Velzen, E.H. Prototype-scale flume experiments on hydraulic roughness of submerged vegetation. In Proceedings of the 28th IAHR Congress, Graz, Austria, 22–27 August 1999; Institute of Hydraulics and Hydrology, Technical University of Graz: Graz, Austria, 1999; p. 221. [Google Scholar]
- Lopez, F.; Garcia, M. Mean flow and turbulence structure of open channel flow through non-emergent vegetation. J. Hydraul. Eng. 2001, 127, 392–402. [Google Scholar] [CrossRef]
- Defina, A.; Bixio, A. Mean flow and turbulence in vegetated open channel flow. Water Resour. Res. 2005, 41, 1–12. [Google Scholar] [CrossRef]
- Wilson, C.A.M.E.; Yagci, O.; Rauch, H.P.; Olsen, N.R.B. 3D numerical modeling of a willow vegetated river/floodplain system. J. Hydrol. 2006, 327, 13–21. [Google Scholar] [CrossRef]
- Keramaris, E.; Pechlivanidis, G.; Kasiteropoulou, D.; Michalolias, N.; Liakopoulos, A. Experimental and numerical analysis in open channel turbulent flow. Procedia Eng. J. 2016, 162, 381–387. [Google Scholar] [CrossRef][Green Version]
- Keramaris, E.; Kasiteropoulou, D.; Liakopoulos, A.; Michalolias, N.; Pechlivanidis, G. A study of flow in open channels with vegetation: Experiments and numerical models. Spec. Top. Rev. Porous Media 2017, 8, 109–129. [Google Scholar] [CrossRef]
- Keramaris, E.; Pechlivanidis, G.; Pechlivanidis, I.; Samaras, G. The impact of lateral walls on the velocity profile in an open channel using the PIV method. In Proceedings of the 13th International Conference on Environmental Science and Technology, Athens, Greece, 5–7 September 2013. [Google Scholar]
- Liakopoulos, A. Fluid Mechanics; Tziolas Publication: Thessaloniki, Greece, 2010. (In Greek) [Google Scholar]
- Liakopoulos, A. Explicit Representations of the Complete Velocity Profile in a Turbulent Boundary Layer. AIAA J. 1984, 22, 844–846. [Google Scholar] [CrossRef]
- George, W.K.; Castillo, L. Zero-pressure-gradient turbulent boundary layer. Appl. Mech. Rev. 1997, 50, 689–729. [Google Scholar] [CrossRef]
- Lumley, J.L. Stochastic Tools in Turbulence; Academic: New York, NY, USA, 1971; pp. 54–82. [Google Scholar]
- Sirovich, L. Turbulence and the Dynamics of Coherent Structures, Parts I–III. Q. Appl. Math. 1987, 45, 561–590. [Google Scholar] [CrossRef]
- Cipolla, K.; Liakopoulos, A.; Rockwell, D.O. Quantitative Imaging in Proper Orthogonal Decomposition of Flow Past a Delta Wing. AIAA J. 1998, 36, 1247–1255. [Google Scholar] [CrossRef]
- Kim, Y.; Rockwell, D.; Liakopoulos, A. Quantitative flow interpretation of vortex buffeting on an aircraft tail via proper orthogonal decomposition (POD). AIAA J. 2005, 43, 550–559. [Google Scholar] [CrossRef]

| Discharge (L/s) | h (cm) | Umean (m/s) Exp/Num | Re Exp/Num | Differences (%) | Number of Nodes | Number of Tetrahedra |
|---|---|---|---|---|---|---|
| 0.735 | 7 | 0.145/0.136 | 10,150/9520 | 6.20 | 19,658 | 86,468 |
| 0.735 | 9 | 0.106/0.113 | 9540/10,170 | 6.60 | 24,163 | 110,949 |
| 0.735 | 11 | 0.090/0.091 | 9900/10,010 | 1.11 | 28,244 | 134,197 |
| 0.735 | 13 | 0.067/0.070 | 8710/9100 | 4.47 | 32,713 | 158,558 |
| 0.845 | 7 | 0.167/0.161 | 11,690/11,270 | 3.59 | 19,658 | 86,468 |
| 0.845 | 9 | 0.133/0.127 | 11,970/11,430 | 4.51 | 24,163 | 110,949 |
| 0.845 | 11 | 0.103/0.099 | 11,330/10,890 | 3.88 | 28,244 | 134,197 |
| 0.845 | 13 | 0.083/0.082 | 10,790/10,660 | 1.20 | 32,713 | 158,558 |
| 0.970 | 7 | 0.235/0.201 | 16,450/14,070 | 14.46 | 19,658 | 86,468 |
| 0.970 | 9 | 0.163/0.154 | 14,670/13,860 | 5.52 | 24,163 | 110,949 |
| 0.970 | 11 | 0.129/0.115 | 14,190/12,650 | 10.85 | 28,244 | 134,197 |
| 0.970 | 13 | 0.105/0.095 | 13,650/12,350 | 9.52 | 32,713 | 158,558 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Michalolias, N.; Keramaris, E.; Kasiteropoulou, D.; Liakopoulos, A.; Pechlivanidis, G. Experiments and Numerical Analysis of Flow in an Open Channel with Gravel Bed. Proceedings 2018, 2, 581. https://doi.org/10.3390/proceedings2110581
Michalolias N, Keramaris E, Kasiteropoulou D, Liakopoulos A, Pechlivanidis G. Experiments and Numerical Analysis of Flow in an Open Channel with Gravel Bed. Proceedings. 2018; 2(11):581. https://doi.org/10.3390/proceedings2110581
Chicago/Turabian StyleMichalolias, Nikolaos, Evangelos Keramaris, Dorothea Kasiteropoulou, Antonios Liakopoulos, and George Pechlivanidis. 2018. "Experiments and Numerical Analysis of Flow in an Open Channel with Gravel Bed" Proceedings 2, no. 11: 581. https://doi.org/10.3390/proceedings2110581
APA StyleMichalolias, N., Keramaris, E., Kasiteropoulou, D., Liakopoulos, A., & Pechlivanidis, G. (2018). Experiments and Numerical Analysis of Flow in an Open Channel with Gravel Bed. Proceedings, 2(11), 581. https://doi.org/10.3390/proceedings2110581




