Interpolated Coefficient Mixed Finite Elements for Semilinear Time Fractional Diffusion Equations
Abstract
1. Introduction
2. A Fully Discrete ICPMFE Approximation
2.1. The Temporal Discretization
2.2. The Spatial Discretization
3. Stability Analysis
4. Convergence Analysis
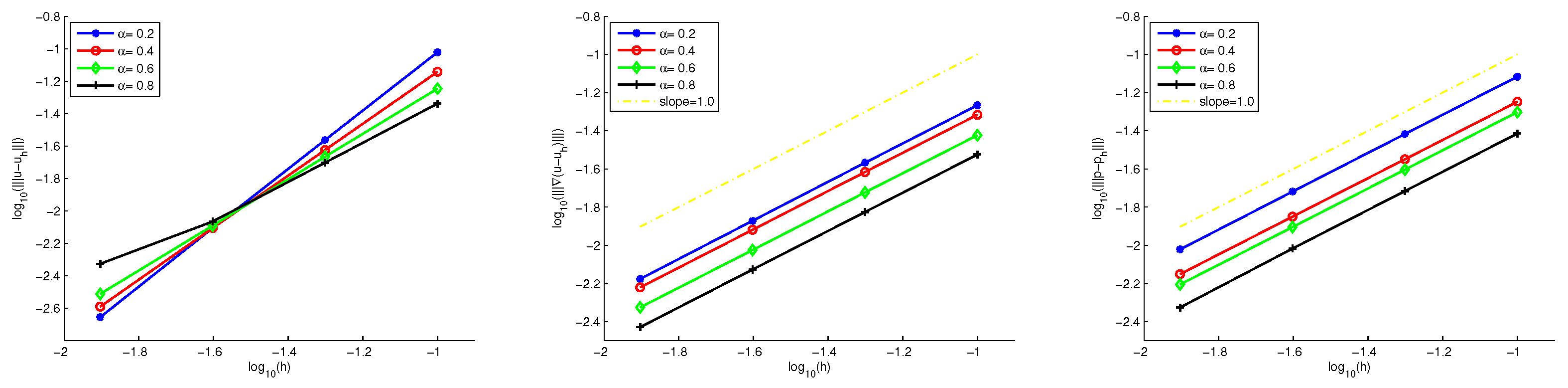
5. Numerical Experiments
| Algorithm 1 ICPMFE |
Step 1. Initialize ; Step 2. Compute discrete nonlinear system with respect to and ,
Step 3. Set and guess the initial , solve (50) by Newton iteration to obtain and ; Step 4. Calculate the iteration error: ; Step 5. If or , stop; else set go to Step 3; Step 6. If , stop; else set go to Step 2. |
6. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| FPDEs | Time fractional partial differential equations |
| FEM | Finite element method |
| MFEM | Mixed finite element method |
| IC | Interpolated coefficient |
| LBB | Ladyženskaja–Babuška–Brezzi |
| ICPMFE | Interpolated coefficient mixed finite element |
| STFRDEs | Semilinear time fractional reaction–diffusion equations |
References
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Li, C.; Chen, A. Numerical methods for fractional partial differential equations. Int. J. Comput. Math. 2018, 95, 1048–1099. [Google Scholar] [CrossRef]
- Li, C.; Zeng, F. Numerical Methods for Fractional Calculas; Chapman and Hall/CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Liu, F.; Zhuang, P.; Liu, Q. Numerical Methods for Fractional Partial Differential Equations and Their Applications; Science Press: Beijing, China, 2015. [Google Scholar]
- Li, X.; Xu, C. A space-time spectral method for the time fractional diffusion equation. SIAM J. Numer. Anal. 2009, 47, 2108–2131. [Google Scholar] [CrossRef]
- Lin, Y.; Xu, C. Finite difference/spectral approximation for the time-fractional diffusion equation. J. Comput. Phys. 2007, 225, 1533–1552. [Google Scholar] [CrossRef]
- Stynes, M.; O’Riordan, E.; Gracia, J. Error analysis of a finite difference method on graded meshes for a time-fractional diffusion equation. SIAM J. Numer. Anal. 2017, 55, 1057–1079. [Google Scholar] [CrossRef]
- Jin, B.; Lazarov, R.; Pasciak, J.; Zhou, Z. Error analysis of semidiscrete finite element methods for inhomogeneous time-fractional diffusion. IMA J. Numer. Anal. 2015, 35, 561–582. [Google Scholar] [CrossRef]
- Nochetto, R.; Otárola, E.; Salgado, A. A PDE approach to space-time fractional parabolic problems. SIAM J. Numer. Anal. 2016, 54, 848–873. [Google Scholar] [CrossRef]
- Feng, R.; Liu, Y.; Hou, Y.; Li, H.; Fang, Z. Mixed element algorithm based on a second-order time approximation scheme for a two-dimensional nonlinear time fractional coupled sub-diffusion model. Eng. Comput. 2022, 38, 51–68. [Google Scholar] [CrossRef]
- Shi, Z.; Zhao, Y.; Liu, F.; Tang, Y.; Wang, F.; Shi, Y. High accuracy analysis of an H1-Galerkin mixed finite element method for two-dimensional time fractional diffusion equations. Comput. Math. Appl. 2017, 74, 1903–1914. [Google Scholar] [CrossRef]
- Li, B.; Luo, H.; Xie, X. A space-time finite element method for fractional wave problems. Numer. Algor. 2020, 85, 1095–1121. [Google Scholar] [CrossRef]
- Li, B.; Wang, T.; Xie, X. Numerical analysis of two Galerkin discretizations with graded temporal grids for fractional evolution equations. J. Sci. Comput. 2020, 85, 59. [Google Scholar] [CrossRef]
- Liu, F.; Zhuang, P.; Turner, I.; Burrage, K.; Anh, V. A new fractional finite volume method for solving the fractional diffusion equation. Appl. Math. Model. 2014, 38, 3871–3878. [Google Scholar] [CrossRef]
- Li, M.; Zhao, J.; Huang, C.; Chen, S. Nonconforming virtual element method for the time fractional reaction-subdiffusion equation with non-smooth data. J. Sci. Comput. 2019, 81, 1823–1859. [Google Scholar] [CrossRef]
- Gu, X.; Sun, H.; Zhang, Y.; Zhao, Y. Fast implicit difference schemes for time-space fractional diffusion equations with the integral fractional Laplacian. Math. Methods Appl. Sci. 2021, 44, 441–463. [Google Scholar] [CrossRef]
- Jiang, S.; Zhang, J.; Zhang, Q.; Zhang, Z. Fast evaluation of the Caputo fractional derivative and its applications to fractional diffusion equations. Commun. Comput. Phys. 2017, 21, 650–678. [Google Scholar] [CrossRef]
- Shen, J.; Sun, Z.; Du, R. Fast finite difference schemes for time-fractional diffusion equations with a weak singularity at initial time. East Asian J. Appl. Math. 2018, 8, 834–858. [Google Scholar] [CrossRef]
- Alikhanov, A.; Huang, C. A high-order L2 type difference scheme for the time-fractional diffusion equation. Appl. Meth. Comput. 2021, 411, 126545. [Google Scholar] [CrossRef]
- Ren, J.; Liao, H.; Zhang, J.; Zhang, Z. Sharp H1-norm error estimates of two time-stepping schemes for reaction-subdiffusion problems. J. Comput. Appl. Math. 2021, 389, 113352. [Google Scholar] [CrossRef]
- Li, D.; Wu, C.; Zhang, Z. Linearized Galerkin fems for nonlinear time fractional parabolic problems with non-smooth solutions in time direction. J. Sci. Comput. 2019, 80, 403–419. [Google Scholar] [CrossRef]
- Zhao, Y.; Gu, X.; Ostermann, A. A preconditioning technique for an all-at-once system from Volterra subdiffusion equations with graded time steps. J. Sci. Comput. 2021, 88, 11. [Google Scholar] [CrossRef]
- Afolabi, Y.; Biala, T.; Iyiola, O.; Khaliq, A.; Wade, B. A second-order Crank-Nicolson-type scheme for nonlinear space-time reaction-diffusion equations on time-graded meshes. Fractal Fract. 2023, 7, 40. [Google Scholar] [CrossRef]
- Qin, H.; Chen, X.; Zhou, B. A family of transformed difference schemes for nonlinear time-fractional equations. Fractal Fract. 2023, 7, 96. [Google Scholar] [CrossRef]
- Xu, J. Two-Grid discretization techniques for linear and nonlinear PDEs. SIAM J. Numer. Anal. 1996, 33, 1759–1777. [Google Scholar] [CrossRef]
- Zlámal, M. Finite element methods for nonlinear parabolic equations. RAIRO Model. Anal. Numer. 1997, 11, 93–107. [Google Scholar] [CrossRef]
- Larsson, S.; Thomée, V.; Zhang, N. Interpolation of coefficients and transformation of dependent variable in element methods for the nonlinear heat equation. Math. Meth. Appl. Sci. 1989, 11, 105–124. [Google Scholar] [CrossRef]
- Xie, Z.; Chen, C. The interpolated coefficient FEM and its application in computing the multiple solutions of semilinear elliptic problems. Int. J. Numer. Anal. Model. 2005, 2, 97–106. [Google Scholar]
- Pani, A. An H1-Galerkin mixed finite element methods for parabolic partial differential equations. SIAM J. Numer. Anal. 1998, 35, 712–727. [Google Scholar] [CrossRef]
- Yang, D. A splitting positive definite mixed element method for miscible displacement of compressible flow in porous media. Numer. Methods Partial Differ. Eq. 2001, 17, 229–249. [Google Scholar] [CrossRef]
- Chen, S.; Chen, H. New mixed element schemes for a second-order elliptic problem. Math. Numer. Sin. 2010, 32, 213–218. (In Chinese) [Google Scholar]
- Hou, T.; Jiang, W.; Yang, Y.; Leng, H. Two-grid − P1 mixed finite element methods combined with Crank-Nicolson scheme for a class of nonlinear parabolic equations. Appl. Numer. Math. 2019, 137, 136–150. [Google Scholar] [CrossRef]
- Li, Q.; Chen, Y.; Huang, Y.; Wang, Y. Two-grid methods for semilinear time fractional reaction diffusion equations by expanded mixed finite element method. Appl. Numer. Math. 2020, 157, 38–54. [Google Scholar] [CrossRef]
- Henry, B.; Wearne, S. Fractional reaction-diffusion. Physica A 2000, 276, 448–455. [Google Scholar] [CrossRef]
- Angstmann, C.; Henry, B. Time fractional Fisher-KPP and Fitzhugh-Nagumo equations. Entropy 2020, 22, 1035. [Google Scholar] [CrossRef]
- Brezzi, F.; Fortin, M. Mixed and Hybrid Finite Element Methods; Springer: Berlin, Germany, 1999. [Google Scholar]
- Lord, G.; Powell, C.; Shardlow, T. An Introduction to Computational Stochastic PDEs; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Huang, C.; Stynes, M. Optimal spatial H1-norm analysis of a finite element method for a time-fractional diffusion equation. J. Comput. Appl. Math. 2020, 367, 112435. [Google Scholar] [CrossRef]
- Huang, C.; Stynes, M. Superconvergence of a finite element method for the multi-term time-fractional diffusion problem. J. Sci. Comput. 2020, 82, 10. [Google Scholar] [CrossRef]
- Ciarlet, P. The Finite Element Method for Elliptic Problems; North-Holland: Amsterdam, The Netherlands, 1978.
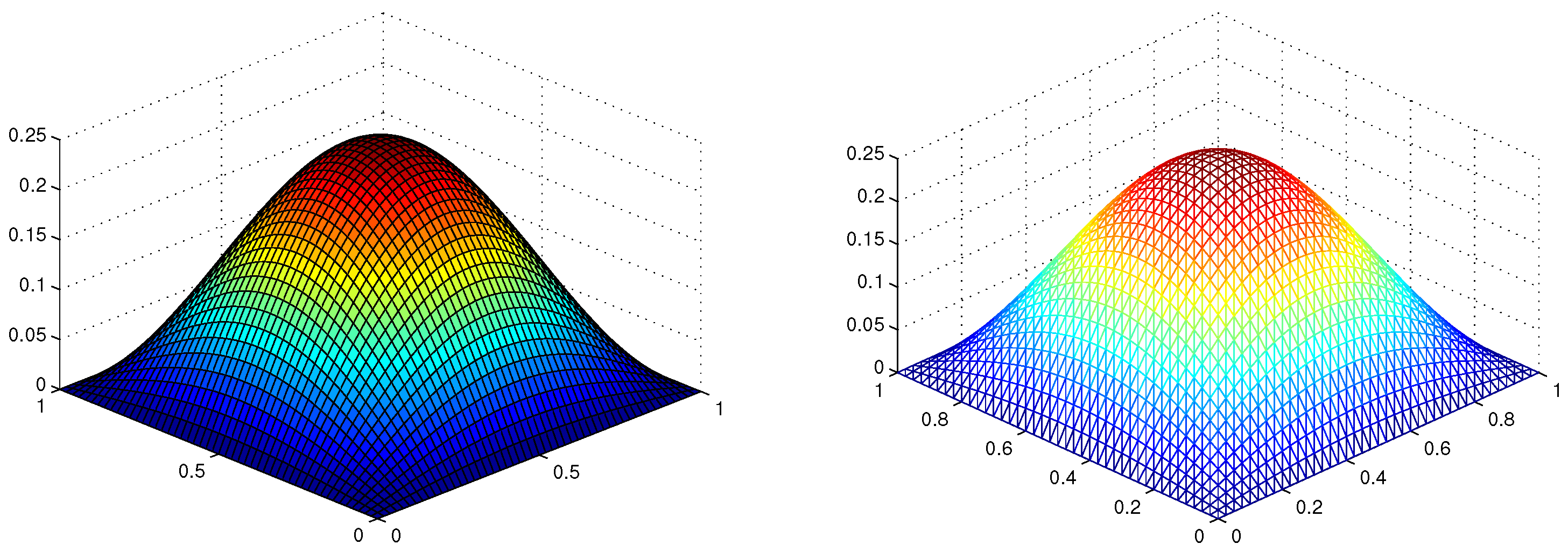

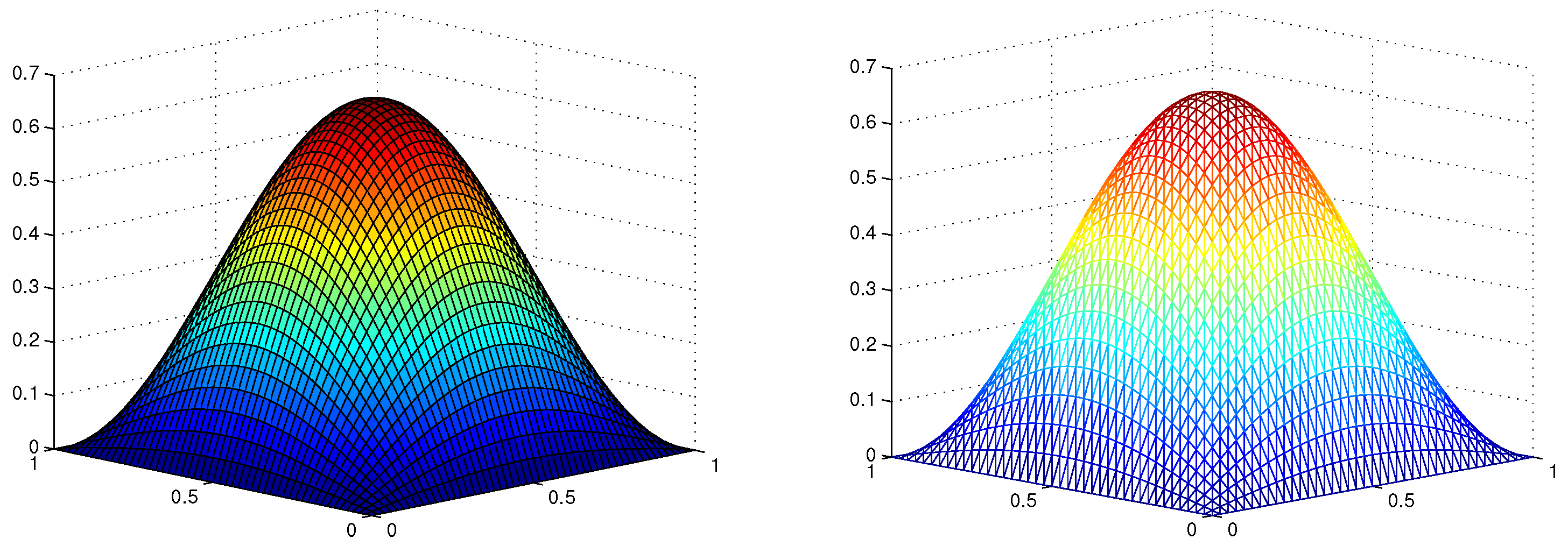
| N | 10 | 20 | 40 | 80 | |
|---|---|---|---|---|---|
| 0.2 | |||||
| 0.4 | |||||
| 0.6 | |||||
| 0.8 | |||||
| N | 10 | 20 | 40 | 80 | |
|---|---|---|---|---|---|
| 0.2 | |||||
| 0.4 | |||||
| 0.6 | |||||
| 0.8 | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Tang, Y. Interpolated Coefficient Mixed Finite Elements for Semilinear Time Fractional Diffusion Equations. Fractal Fract. 2023, 7, 482. https://doi.org/10.3390/fractalfract7060482
Li X, Tang Y. Interpolated Coefficient Mixed Finite Elements for Semilinear Time Fractional Diffusion Equations. Fractal and Fractional. 2023; 7(6):482. https://doi.org/10.3390/fractalfract7060482
Chicago/Turabian StyleLi, Xiaowu, and Yuelong Tang. 2023. "Interpolated Coefficient Mixed Finite Elements for Semilinear Time Fractional Diffusion Equations" Fractal and Fractional 7, no. 6: 482. https://doi.org/10.3390/fractalfract7060482
APA StyleLi, X., & Tang, Y. (2023). Interpolated Coefficient Mixed Finite Elements for Semilinear Time Fractional Diffusion Equations. Fractal and Fractional, 7(6), 482. https://doi.org/10.3390/fractalfract7060482






