Bifurcation of Exact Solutions for the Space-Fractional Stochastic Modified Benjamin–Bona–Mahony Equation
Abstract
1. Introduction
2. Mathematical Analysis
Phase Portrait Description
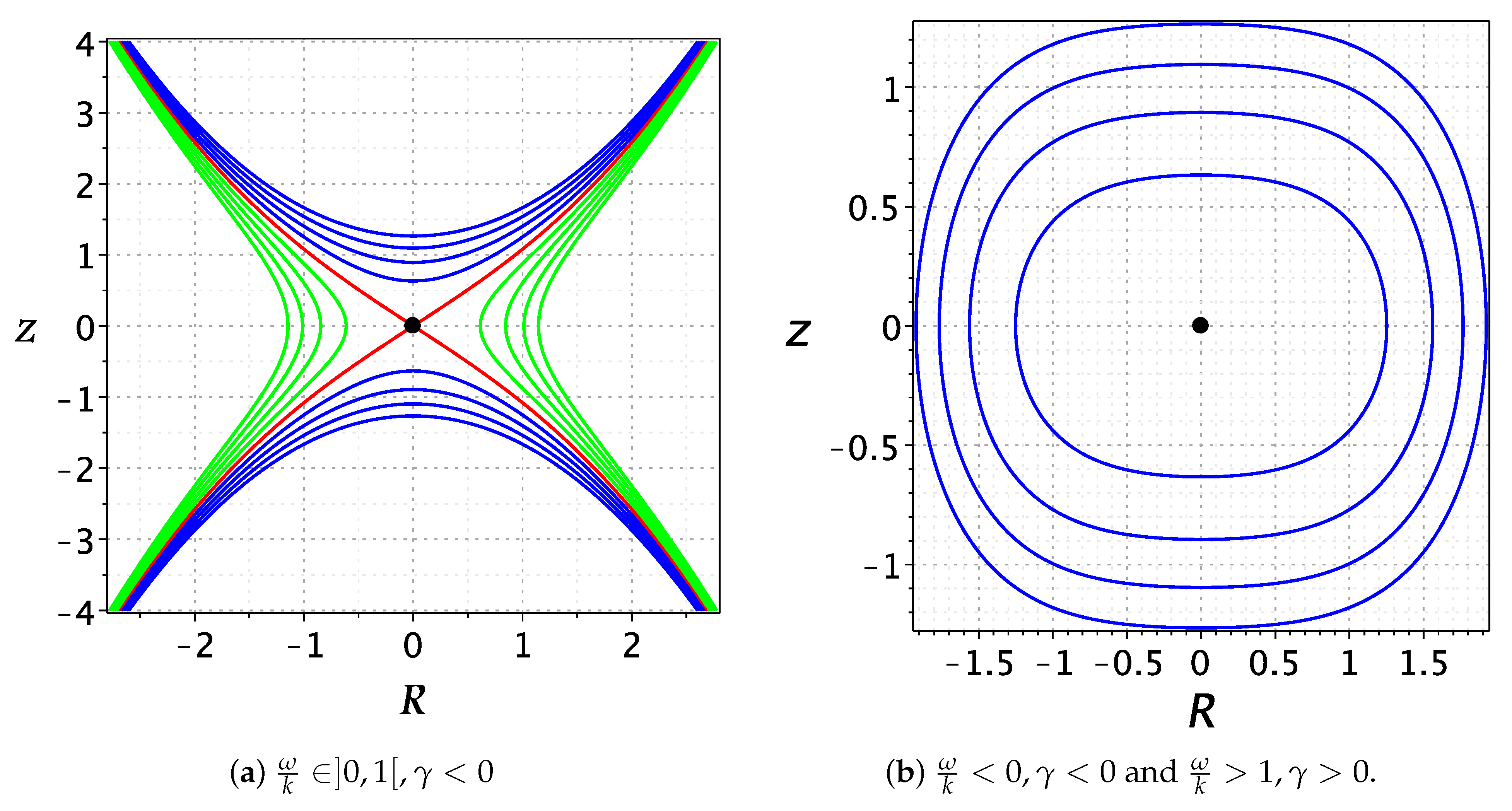
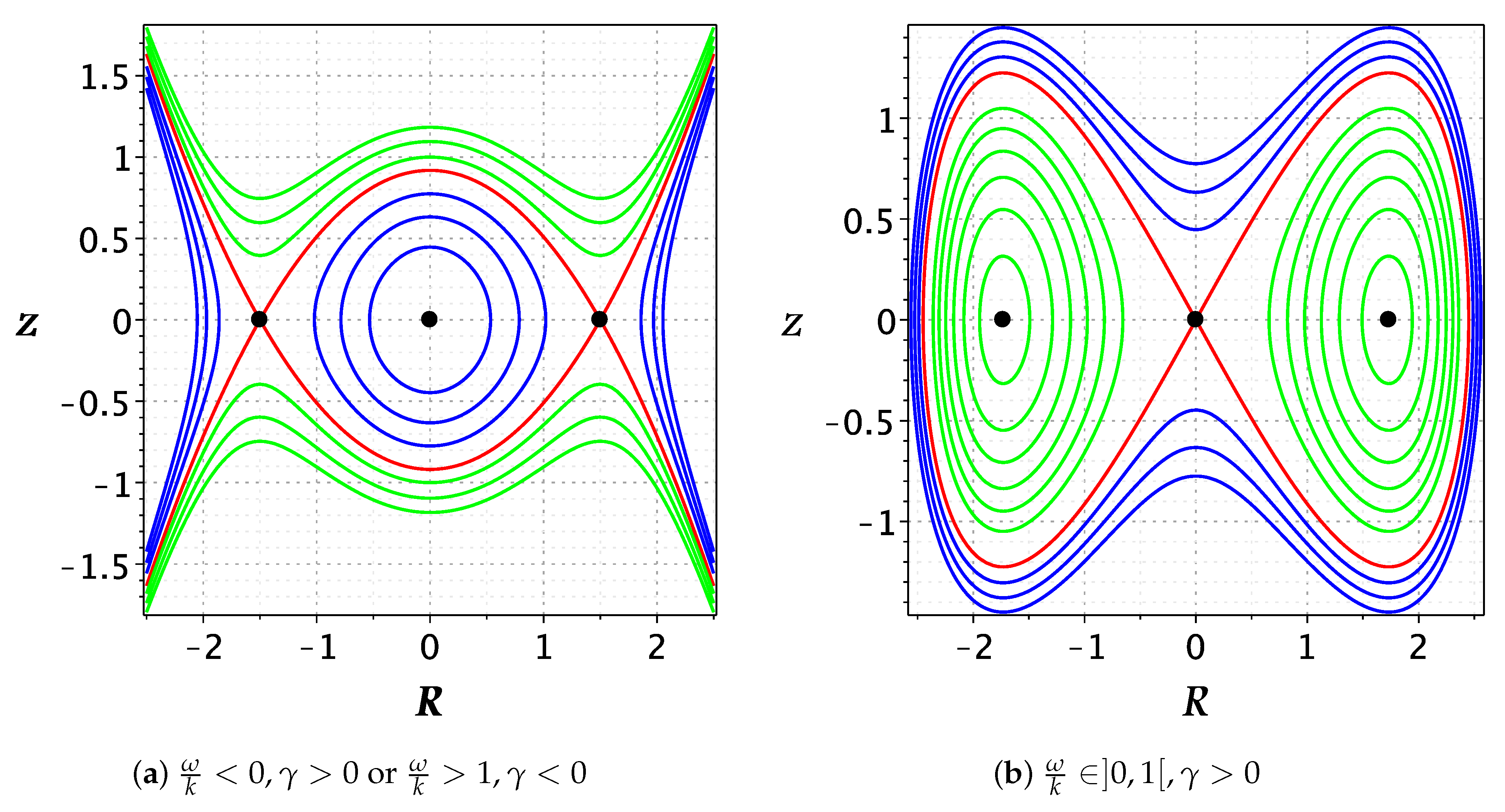
- System (8) has three equilibrium points O and when . For or , the equilibrium point O is the center, while are the saddle points. The phase plane, which is illustrated by Figure 2a, for this case, consists of three different types of orbits based on the values of E. The family of is unbounded orbits in green. The red orbit is a heteroclinic orbit or sometimes is named a connecting orbit due to it connecting the two saddle points . The family of orbits, , in blue, is composed of three separated subfamilies of orbits. Two of them are unbounded and arise outside the heteroclinic orbit, while the inside one is periodic around the center point O. In the case in which , the equilibrium point O is saddle, while the others are centers. All the orbits are bounded for all possible values of the parameter E. The family is a super periodic family of orbit. When , there are two ovals in the homoclinic orbit connecting the saddle point O with itself. For , there are two families of periodic orbits in green. Each of them appears in the left and right oval of the homoclinic orbit.
- In Figure 1b, if , the family of periodic orbits degenerates into the equilibrium point O.
- In Figure 2a, if , the periodic family of orbits in blue degenerates to the equilibrium point, and the two unbounded orbits in blue turn into the extension parts of the unbounded orbits in red. If , the periodic orbits in blue degenerate to the heteroclinic orbits in red, and the unbounded family of orbits in green is converted to the heteroclinic orbit in red as .
- In Figure 2b, if , the super periodic orbits in blue are converted to the homoclinic orbit in red, and the two periodic families in green are transformed into the homoclinic orbit as . Furthermore, the two periodic families of orbits in green will be shrunk into the two equilibrium points as .
3. Construction of the Solutions
Degeneracy of the Solutions
4. Physical Interpretation
- (a)
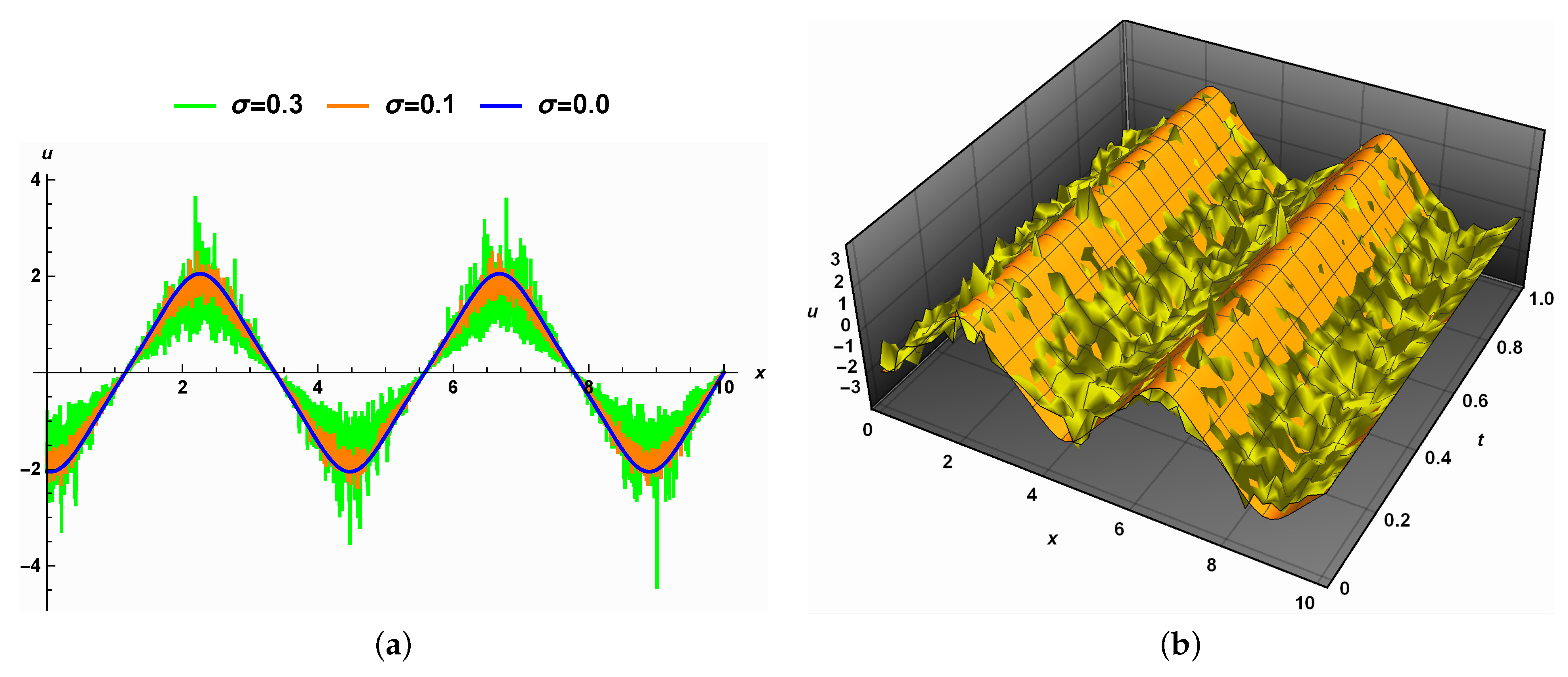
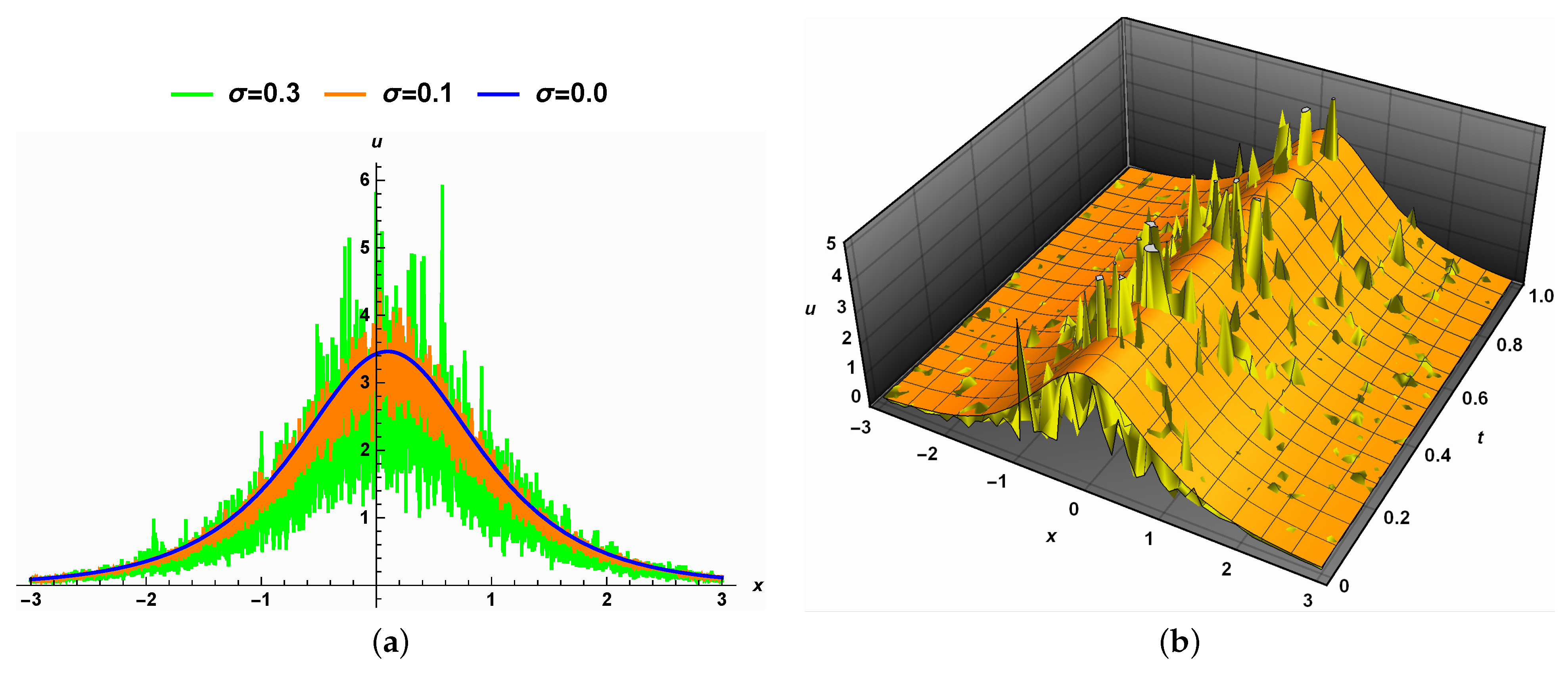
- Influence of the noise:Figure 3a illustrates how the noise influences the solution (19) at different values of . The representation of solution (19) illustrates how the noise impacts the blue curve, which represents the deterministic case. Figure 3b illustrates how the deterministic solution differs from the fractional stochastic solution.
- (b)
- Influence of the fractional order :Figure 4a describes the 2D representation of the solution (19) for different values of the fractional order in the absence of noise, i.e., . The amplitude of the solution is approximately fixed, while the width of the solution grows as the fractional order derivative increases. Figure 4b outlines the representation of the solution in both cases in which the order of the derivatives is fractional (yellow) and integer (orange).
- (c)
- Combined effects:
- (a)
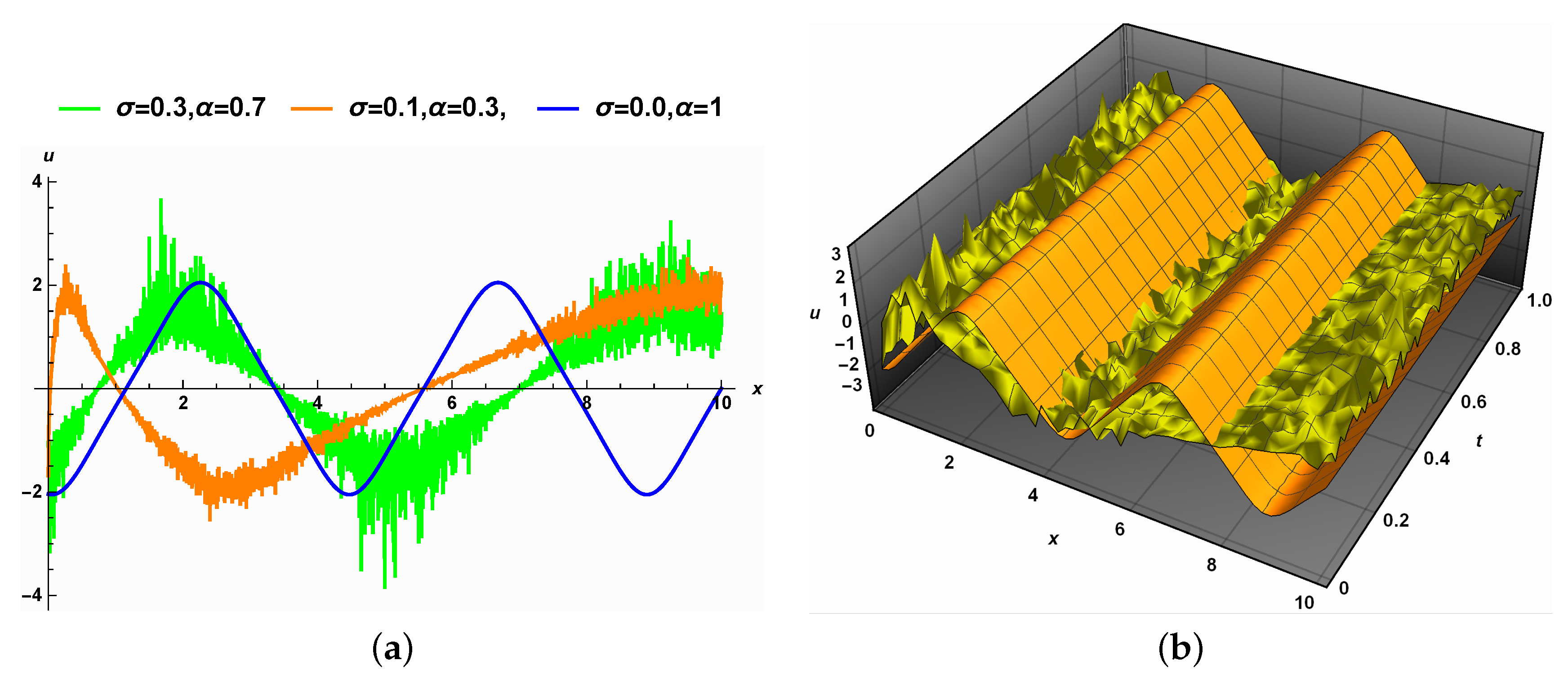
- Influence of the noise:Figure 6a displays the representation of the solution (27). The solution is perturbed around the solution in the deterministic case with , and this causes some small changes in the width of the solution and large changes in the amplitude, as the strength of the noise grows. Furthermore, the 3D representation in Figure 6b outlines the same conclusion.
- (b)
- Influence of the fractional order:Figure 7a clarifies the 2D representation of the solution (27) for different values of the fractional order in the deterministic case, i.e., in the absence of the noise. As the fractional order increases, the width of the solution increases, while the amplitude remains relatively unchanged. Figure 7b illustrates the 3D representation of the solution (27) when without the presence of noise, i.e., .
- (c)
- Effect of the combination of the noise and the fractional order:Figure 8a illustrates the representation of the solution (27) for distinct values of and in addition to the classical case that is represented in blue. The existence of both combines the two previous effects. The amplitude and the width of the solution increase as and grow. Figure 8b outlines the representation of the solution (27), and it gives the same conclusion.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Conformable Derivatives
- ,
- , ,
- ;
- ;
- .
- Let h and g be two functions, such that is differentiable map, and g is defined in the range of g; then, .
Appendix B. Wiener Process
- ,
- is a continuous function for ,
- For , are independent,
- has a normal distribution with mean zero and variance .
References
- Peregrine, D.H. Long waves on a beach. J. Fluid Mech. 1967, 27, 815–827. [Google Scholar] [CrossRef]
- Korteweg, D.J.; De Vries, G. XLI. On the change of form of long waves advancing in a rectangular canal, and on a new type of long stationary waves. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1895, 39, 422–443. [Google Scholar] [CrossRef]
- Benjamin, T.B.; Bona, J.L.; Mahony, J.J. Model equations for long waves in nonlinear dispersive systems. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1972, 272, 47–78. [Google Scholar]
- Peregrine, D.H. Calculations of the development of an undular bore. J. Fluid Mech. 1966, 25, 321–330. [Google Scholar] [CrossRef]
- Easwaran, C.V.; Majumbar, S.R. The evolution of perturbations of the renormalized long wave equation. J. Math. Phys. 1988, 29, 390–392. [Google Scholar] [CrossRef]
- Biswas, A. 1-soliton solution of the B (m, n) equation with generalized evolution. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 3226–3229. [Google Scholar] [CrossRef]
- Camassa, R.; Holm, D.D. An integrable shallow water wave equation with peaked solitons. Phys. Rev. Lett. 1974, 19, 1095–1097. [Google Scholar]
- Biswas, A. 1-Soliton solution of Benjamin–Bona–Mahoney equation with dual-power law nonlinearity. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 2744–2746. [Google Scholar] [CrossRef]
- Musette, M.; Lambert, F.; Decuyper, J.C. Soliton and antisoliton resonant interactions. J. Phys. A Math. Gen. 1987, 20, 6223. [Google Scholar] [CrossRef]
- Adler, V.E.; Postnikov, V.V. On vector analogs of the modified Volterra lattice. J. Phys. A Math. Theor. 2008, 41, 455203. [Google Scholar] [CrossRef]
- Fan, E. Soliton solutions for a generalized Hirota–Satsuma coupled KdV equation and a coupled MKdV equation. Phys. Lett. A 2001, 282, 18–22. [Google Scholar] [CrossRef]
- Fan, E.; Hon, B.Y. Double periodic solutions with Jacobi elliptic functions for two generalized Hirota–Satsuma coupled KdV systems. Phys. Lett. A 2002, 292, 335–337. [Google Scholar] [CrossRef]
- Rogers, C.; Rogers, C.; Schief, W.K. Bäcklund and Darboux Transformations: Geometry and Modern Applications in Soliton Theory; Cambridge University Press: Cambridge, UK, 2002; Volume 30. [Google Scholar]
- Olver, P.J. Applications of Lie Groups to Differential Equations; Springer Science and Business Media: Berlin/Heidelberg, Germany, 1993; Volume 107. [Google Scholar]
- Bluman, G.; Anco, S. Symmetry and Integration Methods for Differential Equations; Springer Science and Business Media: Berlin/Heidelberg, Germany, 2008; Volume 154. [Google Scholar]
- Ibragimov, N.H. Transformation Groups Applied to Mathematical Physics; Springer Science and Business Media: Berlin/Heidelberg, Germany, 1984; Volume 3. [Google Scholar]
- Kumar, S.; Almusawa, H.; Dhiman, S.K.; Osman, M.S.; Kumar, A. A study of Bogoyavlenskii’s (2 + 1)-dimensional breaking soliton equation: Lie symmetry, dynamical behaviors and closed-form solutions. Results Phys. 2021, 29, 104793. [Google Scholar] [CrossRef]
- Hirota, R. The Direct Method in Soliton Theory (No. 155); Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Hu, X.B.; Li, C.X.; Nimmo, J.J.; Yu, G.F. An integrable symmetric (2 + 1)-dimensional Lotka–Volterra equation and a family of its solutions. J. Phys. A Math. Gen. 2004, 38, 195. [Google Scholar] [CrossRef]
- Elbrolosy, M.E.; Elmandouh, A.A. Dynamical behaviour of nondissipative double dispersive microstrain wave in the microstructured solids. Eur. Phys. J. Plus 2021, 136, 955. [Google Scholar] [CrossRef]
- Nuwairan, M.A.; Elmandouh, A.A. Qualitative analysis and wave propagation of the nonlinear model for low-pass electrical transmission lines. Phys. Scr. 2021, 96, 095214. [Google Scholar] [CrossRef]
- Elmandouh, A.A. Integrability, qualitative analysis and the dynamics of wave solutions for Biswas–Milovic equation. Eur. Phys. J. Plus 2021, 136, 638. [Google Scholar] [CrossRef]
- Elmandouh, A.A. Bifurcation and new traveling wave solutions for the 2D Ginzburg–Landau equation. Eur. Phys. J. Plus 2020, 135, 648. [Google Scholar] [CrossRef]
- Elmandouh, A.A.; Elbrolosy, M.E. New traveling wave solutions for Gilson–Pickering equation in plasma via bifurcation analysis and direct method. Math. Methods Appl. Sci. 2022. [Google Scholar] [CrossRef]
- Tamang, J.; Abdikian, A.; Saha, A. Phase plane analysis of small amplitude electron-acoustic supernonlinear and nonlinear waves in magnetized plasmas. Phys. Scr. 2020, 95, 105604. [Google Scholar] [CrossRef]
- Elbrolosy, M.E. Qualitative analysis and new soliton solutions for the coupled nonlinear Schrödinger type equations. Phys. Scr. 2021, 96, 125275. [Google Scholar] [CrossRef]
- Verma, A.; Jiwari, R.; Koksal, M.E. Analytic and numerical solutions of nonlinear diffusion equations via symmetry reductions. Adv. Differ. Equ. 2014, 2014, 229. [Google Scholar] [CrossRef]
- Arnold, L. Trends and open problems in the theory of random dynamical systems. In Probability towards 2000; Springer: New York, NY, USA, 1998; pp. 34–46. [Google Scholar]
- Weinan, E.; Li, X.; Vanden-Eijnden, E. Some recent progress in multiscale modeling. Multiscale Model. Simul. 2004, 39, 3–21. [Google Scholar]
- Mohammed, W.W.; Iqbal, N.; Botmart, T. Additive noise effects on the stabilization of fractional-space diffusion equation solutions. Mathematics 2022, 10, 130. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Alshammari, M.; Cesarano, C.; Albadrani, S.; El-Morshedy, M. Brownian Motion Effects on the Stabilization of Stochastic Solutions to Fractional Diffusion Equations with Polynomials. Mathematics 2022, 10, 1458. [Google Scholar] [CrossRef]
- Das, S. Functional Fractional Calculus; Springer: Berlin, Germany, 2011; Volume 1. [Google Scholar]
- Dong, J.; Xu, M. Space–time fractional Schrödinger equation with time-independent potentials. J. Math. Anal. Appl. 2008, 344, 1005–1017. [Google Scholar] [CrossRef]
- Bayın, S.Ş. On the consistency of the solutions of the space fractional Schrödinger equation. J. Math. Phys. 2012, 53, 042105. [Google Scholar] [CrossRef]
- Elbrolosy, M.E.; Elmandouh, A.A. Dynamical behaviour of conformable time-fractional coupled Konno-Oono equation in magnetic field. Math. Probl. Eng. 2022, 2022, 3157217. [Google Scholar] [CrossRef]
- Saut, J.C.; Tzvetkov, N. Global well-posedness for the KP-BBM equations. Appl. Math. Res. eXpress 2004, 2004, 1–16. [Google Scholar] [CrossRef]
- Varlamov, V.; Liu, Y. Cauchy problem for the Ostrovsky equation. Discret. Contin. Dyn. Syst.-A 2004, 10, 731. [Google Scholar] [CrossRef]
- Khan, K.; Akbar, M.A.; Islam, S.R. Exact solutions for (1 + 1)-dimensional nonlinear dispersive modified Benjamin-Bona-Mahony equation and coupled Klein-Gordon equations. SpringerPlus 2014, 3, 724. [Google Scholar] [CrossRef] [PubMed]
- Baskonus, H.M.; Bulut, H. Analytical studies on the (1 + 1)-dimensional nonlinear dispersive modified Benjamin–Bona–Mahony equation defined by seismic sea waves. Waves Random Complex Media 2015, 25, 576–586. [Google Scholar] [CrossRef]
- Batool, F.; Akram, G. Application of extended Fan sub-equation method to (1 + 1)-dimensional nonlinear dispersive modified Benjamin-Bona-Mahony equation with fractional evolution. Opt. Quantum Electron. 2017, 49, 375. [Google Scholar] [CrossRef]
- Guner, O. Soliton solution of the generalized modified BBM equation and the generalized Boussinesq equation. J. Ocean Eng. Sci. 2017, 2, 248–252. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Al-Joudi, S. Applications of an Extended G′/G-expansion Method to Find Exact Solutions of Nonlinear PDEs in Mathematical Physics. Math. Probl. Eng. 2010, 2010, 768573. [Google Scholar] [CrossRef]
- Manafianheris, J. Exact Solutions of the BBM and MBBM Equations by the Generalized G′/G expansion Method Equations. Int. J. Genet. Eng. 2012, 19, 1789–1796. [Google Scholar] [CrossRef]
- Islam, M.S.; Roshid, M.M.; Rahman, A.L.; Akbar, M.A. Solitary wave solutions in plasma physics and acoustic gravity waves of some nonlinear evolution equations through enhanced MSE method. J. Phys. Commun. 2019, 3, 125011. [Google Scholar] [CrossRef]
- Alharbi, A.; Abdelrahman, M.A.; Almatrafi, M.B. Analytical and numerical investigation for the DMBBM equation. Comput. Model. Eng. Sci. 2020, 122, 743–756. [Google Scholar] [CrossRef]
- Khater, M.M.; Salama, S.A. Semi–analytical and numerical simulations of the modified Benjamin–Bona–Mahony model. J. Ocean Eng. Sci. 2022, 7, 264–271. [Google Scholar] [CrossRef]
- Wang, K.J. Variational principle and diverse wave structures of the modified Benjamin-Bona-Mahony equation arising in the optical illusions field. Axioms 2022, 11, 445. [Google Scholar] [CrossRef]
- Shakeel, M.; El-Zahar, E.R.; Shah, N.A.; Chung, J.D. Generalized Exp-Function Method to Find Closed Form Solutions of Nonlinear Dispersive Modified Benjamin–Bona–Mahony Equation Defined by Seismic Sea Waves. Mathematics 2022, 10, 1026. [Google Scholar] [CrossRef]
- Alotaibi, T.; Althobaiti, A. Exact Solutions of the Nonlinear Modified Benjamin-Bona-Mahony Equation by an Analytical Method. Fractal Fract. 2022, 6, 399. [Google Scholar] [CrossRef]
- Tian, Y.; Cui, J.; Zhang, R. Exact traveling wave solutions of the strain wave and (1+ 1)-dimensional Benjamin–Bona–Mahony equations via the simplest equation method. Mod. Phys. Lett. B 2022, 36, 2250103. [Google Scholar] [CrossRef]
- Ali, A.; Seadawy, A.R. Dispersive soliton solutions for shallow water wave system and modified Benjamin-Bona-Mahony equations via applications of mathematical methods. J. Ocean Eng. Sci. 2021, 6, 85–98. [Google Scholar] [CrossRef]
- Al-Askar, F.M.; Mohammed, W.W.; Alshammari, M. Impact of brownian motion on the analytical solutions of the space-fractional stochastic approximate long water wave equation. Symmetry 2022, 14, 740. [Google Scholar] [CrossRef]
- Nemytskii, V.; Stepanov, V. Qualitative Theory of Differential Equations; Dover: New York, NY, USA, 1989. [Google Scholar]
- Khalil, R.; Al Horani, M.; Yousef, A.; Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65–70. [Google Scholar] [CrossRef]
- Platen, E.; Bruti-Liberati, N. Numerical Solution of Stochastic Differential Equations with Jumps in Finance; Springer Science, Business Media: Berlin/Heidelberg, Germany, 2010; Volume 64. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elmandouh, A.; Fadhal, E. Bifurcation of Exact Solutions for the Space-Fractional Stochastic Modified Benjamin–Bona–Mahony Equation. Fractal Fract. 2022, 6, 718. https://doi.org/10.3390/fractalfract6120718
Elmandouh A, Fadhal E. Bifurcation of Exact Solutions for the Space-Fractional Stochastic Modified Benjamin–Bona–Mahony Equation. Fractal and Fractional. 2022; 6(12):718. https://doi.org/10.3390/fractalfract6120718
Chicago/Turabian StyleElmandouh, Adel, and Emad Fadhal. 2022. "Bifurcation of Exact Solutions for the Space-Fractional Stochastic Modified Benjamin–Bona–Mahony Equation" Fractal and Fractional 6, no. 12: 718. https://doi.org/10.3390/fractalfract6120718
APA StyleElmandouh, A., & Fadhal, E. (2022). Bifurcation of Exact Solutions for the Space-Fractional Stochastic Modified Benjamin–Bona–Mahony Equation. Fractal and Fractional, 6(12), 718. https://doi.org/10.3390/fractalfract6120718





