A Tuning Method via Borges Derivative of a Neural Network-Based Discrete-Time Fractional-Order PID Controller with Hausdorff Difference and Hausdorff Sum
Abstract
1. Introduction
2. Problem Statement
3. Main Results
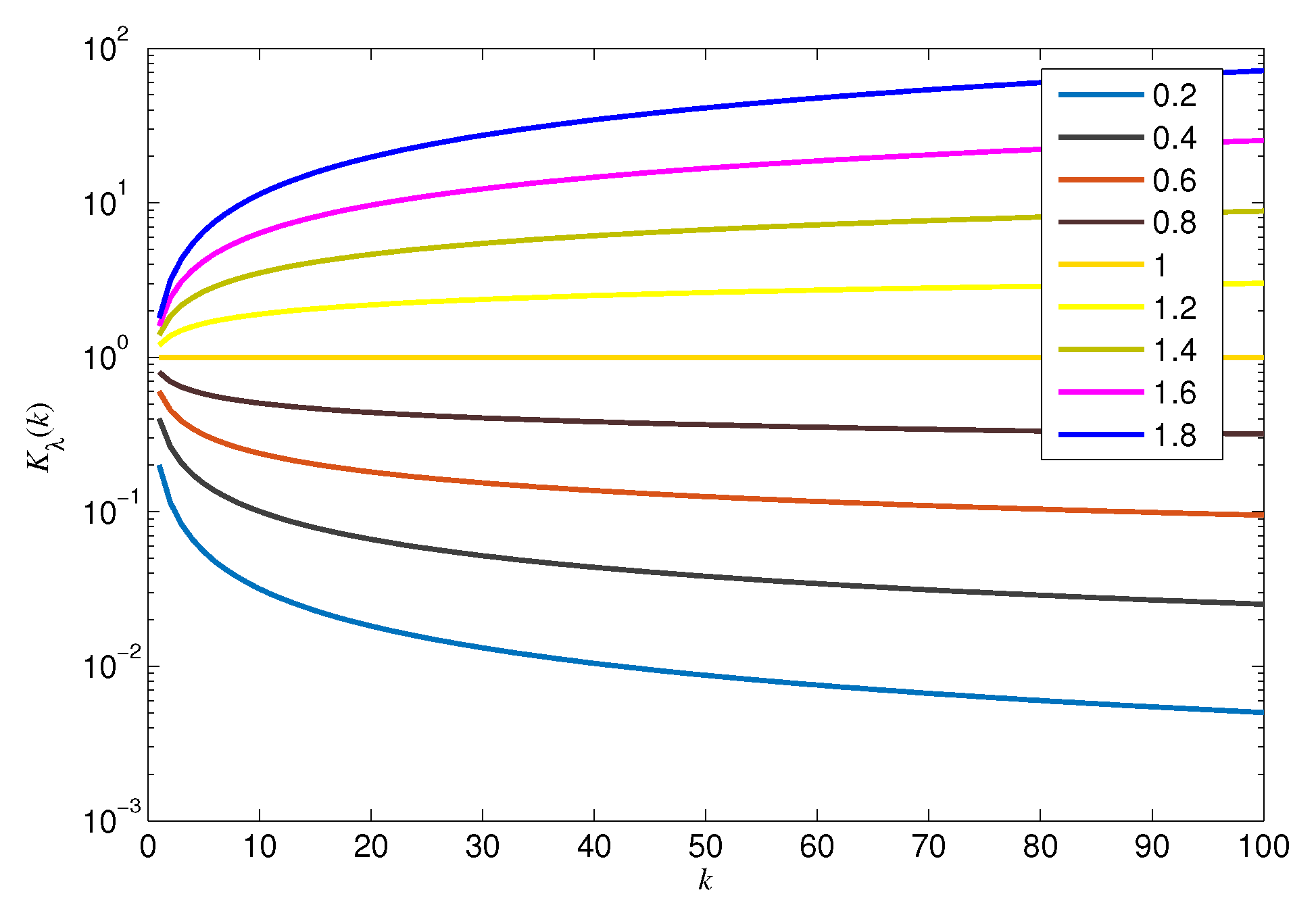
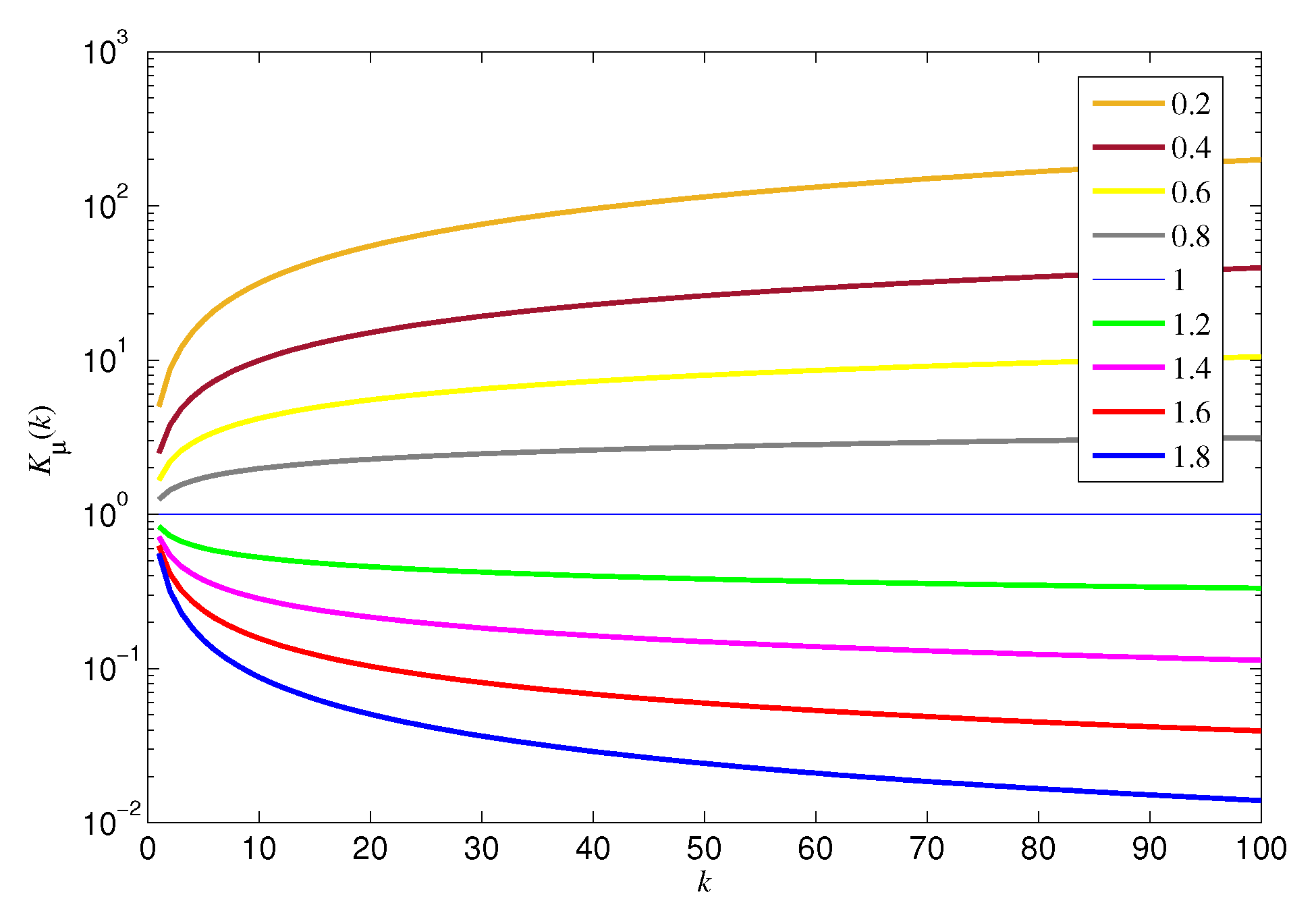
3.1. A Discrete-Time Fractional-Order PID Controller Based on the Hausdorff Difference and Hausdorff Sum
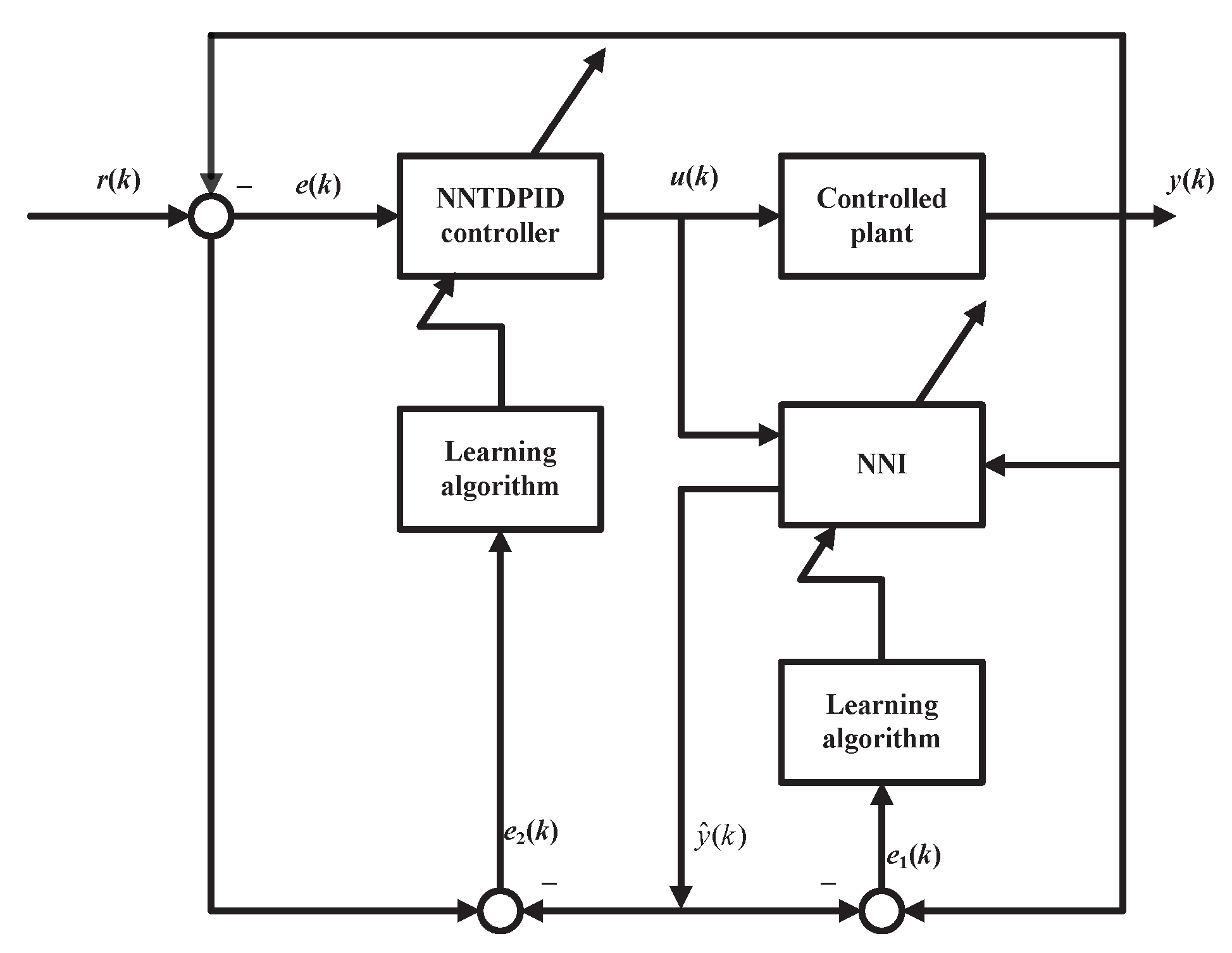
3.2. Tuning Method of Parameters and Orders of Discrete-Time Fractional-Order PID Controllers with Neural Networks
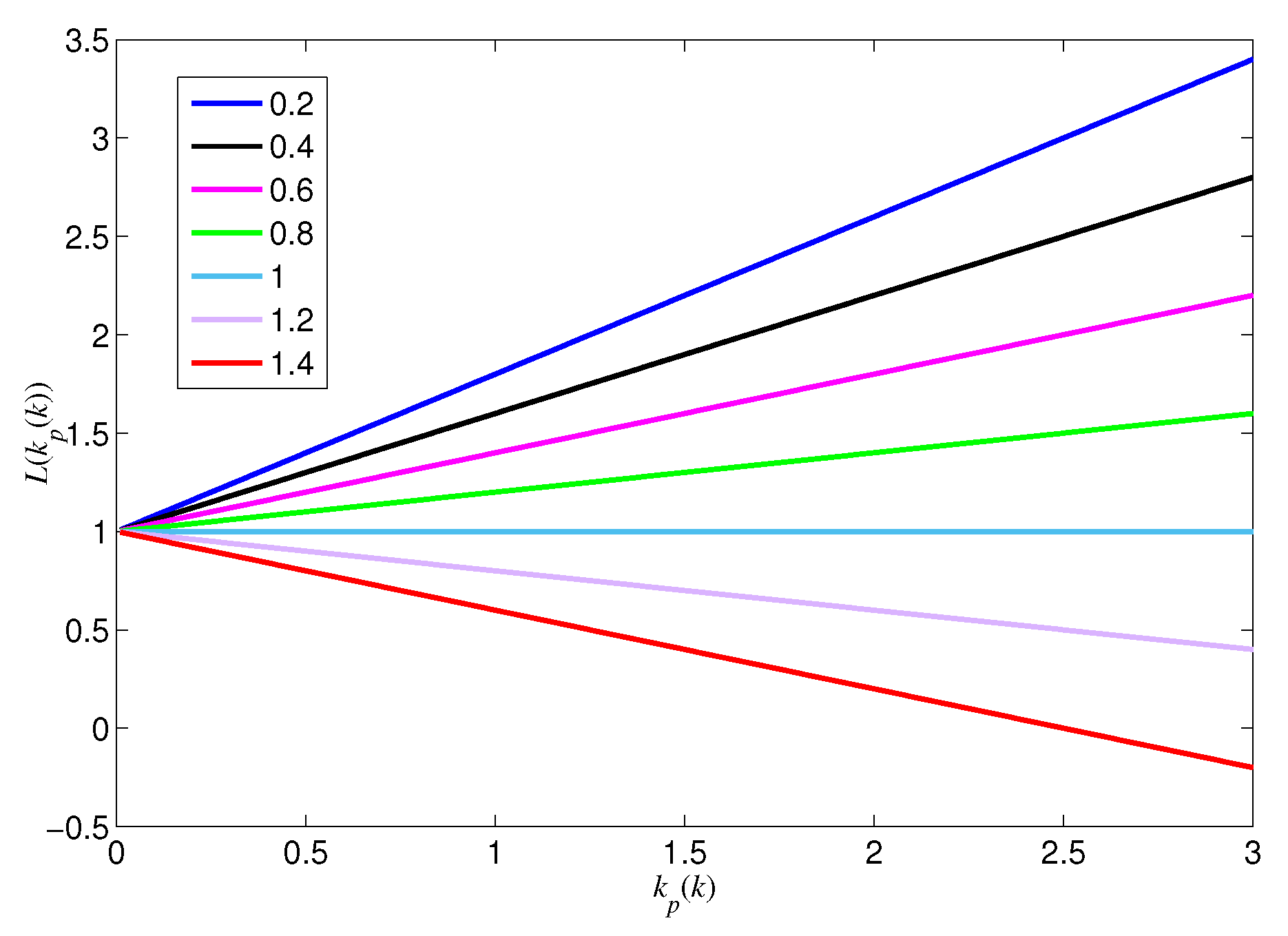
3.3. Tuning Method of Parameters and Orders Based on the Borges Derivative
4. Illustrative Examples
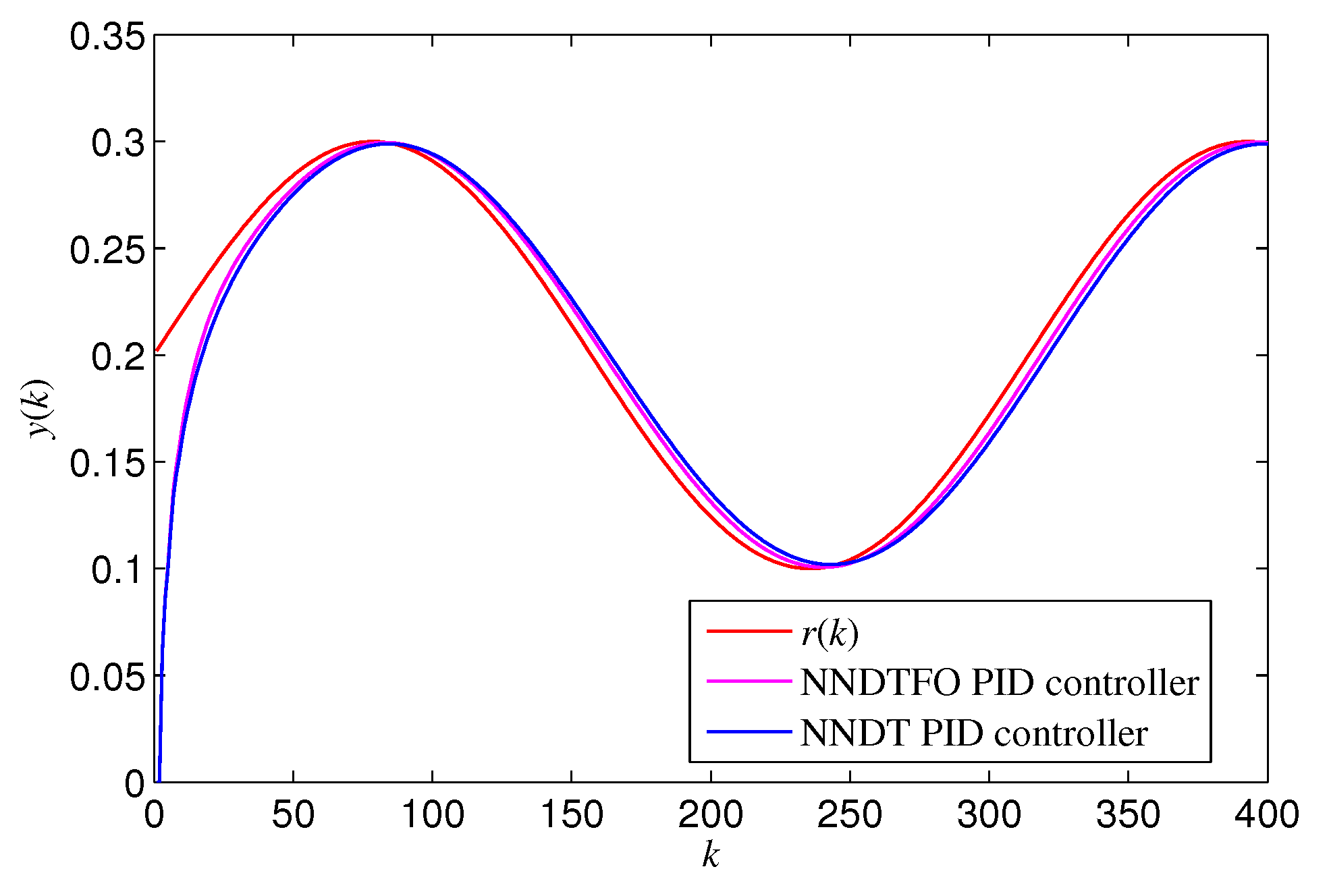
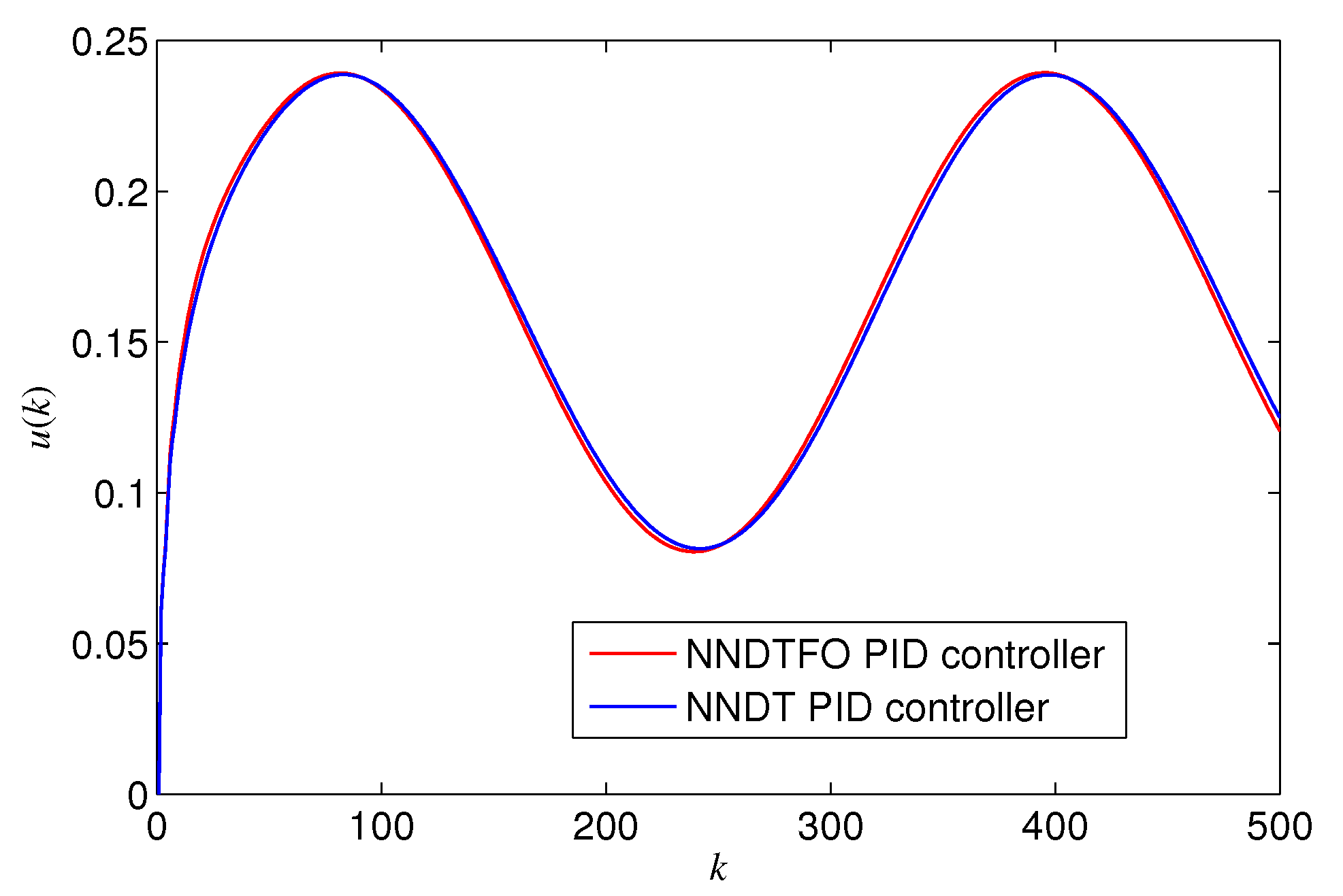
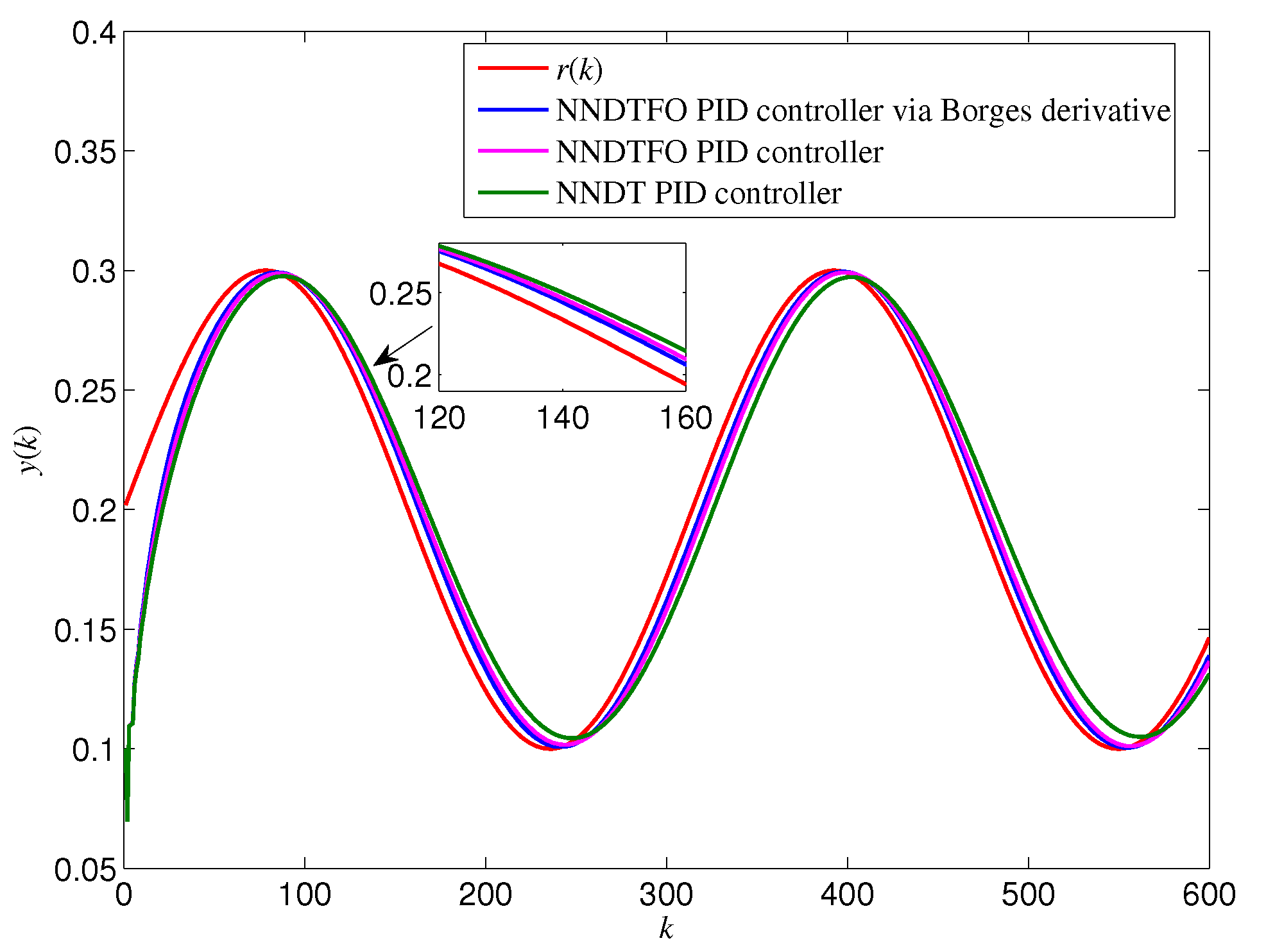
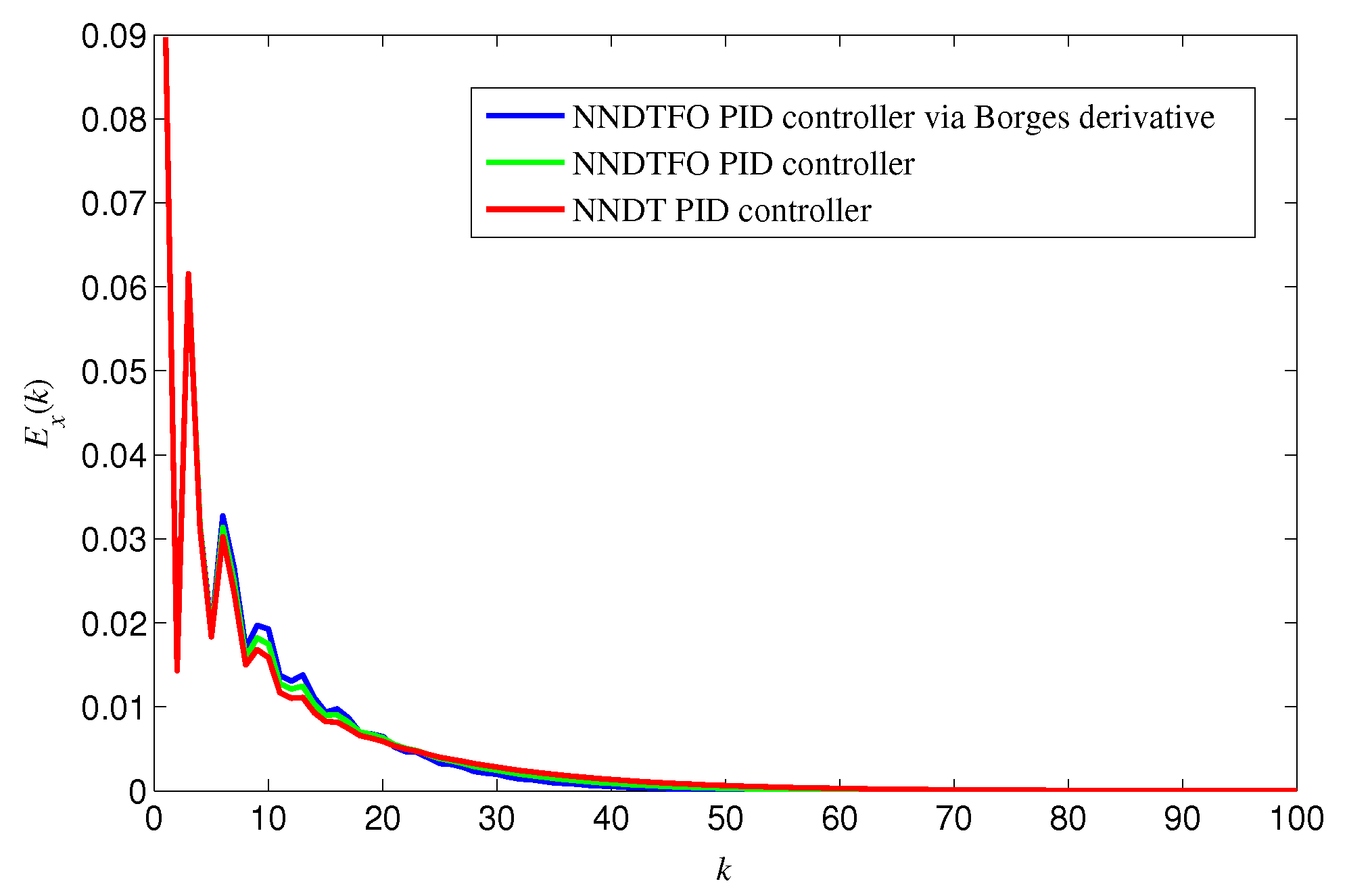
4.1. Example 1
4.2. Example 2
5. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shah, P.; Agashe, S. Review of fractional PID controller. Mechatronics 2016, 38, 29–41. [Google Scholar] [CrossRef]
- Tejado, T.; Vinagre, B.M.; Traver, J.E.; Prieto-Arranz, J.; Nuevo-Gallardo, C. Back to basics: Meaning of the parameters of fractional order PID controllers. Mathematics 2019, 7, 530. [Google Scholar] [CrossRef]
- Haroun, A.H.G.; Li, Y.Y. A novel optimized fractional-order hybrid fuzzy intelligent PID controller for interconnected realistic power systems. Trans. Inst. Meas. Control 2019, 41, 3065–3080. [Google Scholar] [CrossRef]
- Hamidian, H.; Beheshti, M.T.H. A robust fractional-order PID controller design based on active queue management for TCP network. Int. J. Syst. Sci. 2018, 49, 211–216. [Google Scholar] [CrossRef]
- Viola, J.; Angel, L.; Sebastian, J.M. Design and robust performance evaluation of a fractional order PID controller applied to a DC motor. IEEE-CAA J. Autom. Sin. 2017, 4, 304–314. [Google Scholar] [CrossRef]
- Yang, B.; Wang, J.B.; Wang, J.T.; Shu, H.C.; Li, D.Y.; Zeng, C.Y.; Chen, Y.J.; Zhang, X.S.; Yu, T. Robust fractional-order PID control of supercapacitor energy storage systems for distribution network applications: A perturbation compensation based approach. J. Clean. Prod. 2021, 279, 123362. [Google Scholar] [CrossRef]
- Kumar, J.; Kumar, V.; Rana, K.P.S. Fractional-order self-tuned fuzzy PID controller for three-link robotic manipulator system. Neural Comput. Appl. 2020, 32, 7235–7257. [Google Scholar] [CrossRef]
- Ray, P.K.; Paital, S.R.; Mohanty, A.; Foo, Y.S.E.; Krishnan, A.; Gooi, H.B.; Amaratunga, G.A.J. A hybrid firefly-swarm optimized fractional order interval type-2 fuzzy PID-pss for transient stability improvement. IEEE Trans. Ind. Appl. 2019, 55, 6486–6498. [Google Scholar] [CrossRef]
- Gao, P.; Zhang, G.M.; Ouyang, H.M.; Mei, L. An adaptive super twisting nonlinear fractional order PID sliding mode control of permanent magnet synchronous motor speed regulation system based on extended state observer. IEEE Access 2020, 8, 53498–53510. [Google Scholar] [CrossRef]
- Chevalier, A.; Francis, C.; Copot, C.; Ionescu, C.M.; Keyser, R.D. Fractional-order PID design: Toward stransition from state-of-art to state-of-use. ISA Trans. 2019, 84, 178–186. [Google Scholar] [CrossRef] [PubMed]
- Mandic, P.D.; Sekara, T.B.; Lazarevic, M.P.; Boskovic, M. Dominant pole placement with fractional order PID controllers: D-decomposition approach. ISA Trans. 2017, 67, 76–86. [Google Scholar] [CrossRef]
- Feliu-Batlle, V.; Castillo-Garcia, F.J. On the robust control of stable minimum phase plants with large uncertainty in a time constant. A fractional-order control approach. Automatica 2014, 50, 218–224. [Google Scholar] [CrossRef]
- Luo, Y.; Chen, Y.Q.; Wang, C.Y.; Pi, Y.G. Tuning fractional order proportional integral controllers for fractional order systems. J. Process Control 2010, 20, 823–831. [Google Scholar] [CrossRef]
- Machado, J.A.T. Optimal tuning of fractional controllers using genetic algorithms. Nonlinear Dyn. 2010, 62, 447–452. [Google Scholar]
- Hekimoglu, B. Optimal tuning of fractional order PID controller for DC motor speed control via chaotic atom search optimization algorithm. IEEE Access 2019, 7, 38100–38114. [Google Scholar] [CrossRef]
- Liu, L.; Shan, L.; Dai, Y.W.; Liu, C.L.; Qi, Z.D. Improved quantum bacterial foraging algorithm for tuning parameters of fractional-order PID controller. J. Syst. Eng. Electron. 2018, 29, 166–175. [Google Scholar] [CrossRef]
- Zhao, J.B.; Jing, W.C.; Wang, J.Z. An indirect optimization scheme for tuning a fractional order PI controller using extremum seeking. Mechatronics 2018, 56, 146–156. [Google Scholar] [CrossRef]
- Liu, X.Y. Optimization design on fractional order PID controller based on adaptive particle swarm optimization algorithm. Nonlinear Dyn. 2016, 84, 379–386. [Google Scholar] [CrossRef]
- Asgharnia, A.; Jamali, A.; Shahnazi, R.; Maheri, A. Load mitigation of a class of 5-MW wind turbine with RBF neural network based fractional-order PID controller. ISA Trans. 2020, 96, 272–286. [Google Scholar] [CrossRef]
- Chen, C.Y.; Zhu, S.; Wei, Y.C.; Yang, C.Y. Finite-time stability of delayed memristor-based fractional-order neural networks. IEEE Trans. Cybern. 2020, 50, 1607–1616. [Google Scholar] [CrossRef]
- Lv, Y.J.; Hu, C.; Yu, J.; Jiang, H.J.; Huang, T.W. Edge-based fractional-order adaptive strategies for synchronization of fractional-order coupled networks with reaction–diffusion terms. IEEE Trans. Cybern. 2020, 50, 1582–1594. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.H.; Zeng, Z.G. Multiple mittag-leffler stability of delayed fractional-order Cohen-Grossberg neural networks via mixed monotone operator pair. IEEE Trans. Cybern. 2020, 31995512. [Google Scholar] [CrossRef]
- Liu, P.; Zeng, Z.G.; Wang, J. Global synchronization of coupled fractional-order recurrent neural networks. IEEE Trans. Neural Netw. Learn. Syst. 2019, 30, 2358–2368. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.X.; Liu, X.G.; Tang, M.L.; Chen, L.F. Further results on stability and synchronization of fractional-order Hopfield neural networks. Neurocomputing 2019, 346, 12–19. [Google Scholar] [CrossRef]
- Krishna, B.T. Studies on fractional order differentiators and integrators: A survey. Signal Process. 2011, 91, 386–426. [Google Scholar] [CrossRef]
- El-Khazali, R. Discretization of fractional-order differentiators and integrators. IFAC Proc. Vol. 2014, 47, 2016–2021. [Google Scholar] [CrossRef]
- Sierociuk, D.; Dzielinski, A. Fractional Kalman filter algorithm for the states, parameters and order of fractional system estimation. Int. J. Appl. Math. Comput. Sci. 2006, 16, 129–140. [Google Scholar]
- Batir, N. On some properties of the Gamma function. Expo. Math. 2008, 26, 187–196. [Google Scholar] [CrossRef]
- Chen, W.; Liang, Y.J. New methodologies in fractional and fractal derivatives modeling. Chaos Solitons Fractals 2017, 102, 72–77. [Google Scholar] [CrossRef]
- Liang, Y.J.; Ye, A.Q.; Chen, W.; Gatto, R.G.; Colon-Perez, L.; Mareci, T.H.; Magin, R.L. A fractal derivative model for the characterization of anomalous diffusion in magnetic resonance imaging. Commun. Nonlinear Sci. Numer. Simul. 2016, 39, 529–537. [Google Scholar] [CrossRef]
- Cai, W.; Chen, W.; Xu, W.X. Characterizing the creep of viscoelastic materials by fractal derivative models. Int. J. Non-Linear Mech. 2016, 87, 58–63. [Google Scholar] [CrossRef]
- Atangana, A.; Khan, M.A. Validity of fractal derivative to capturing chaotic attractors. Chaos Solitons Fractals 2019, 126, 50–59. [Google Scholar] [CrossRef]
- Chen, W. Time-space fabric underlying anomalous diffusion. Chaos Solitons Fractals 2006, 28, 923–929. [Google Scholar] [CrossRef]
- Hu, D.L.; Chen, W.; Sun, H.G. Power-law stability of Hausdorff derivative nonlinear dynamical systems. Int. J. Syst. Sci. 2020, 51, 601–607. [Google Scholar] [CrossRef]
- Borges, E.P. A possible deformed algebra and calculus inspired in nonextensive thermostatistics. Phys. A Stat. Mech. Its Appl. 2004, 340, 95–101. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional-order systems and PIλDμ controllers. IEEE Trans. Autom. Control 1999, 44, 208–214. [Google Scholar] [CrossRef]




| E via NNDTFO PID Controller | E via NNDT PID Controller | |
|---|---|---|
| 0.01 | 0.0827 | 0.0895 |
| 0.02 | 0.0943 | 0.1118 |
| 0.03 | 0.1066 | 0.1395 |
| 0.04 | 0.1204 | 0.1763 |
| 0.05 | 0.1318 | 0.2044 |
| A | E via NNDTFO PID Controller | E via NNDT PID Controller |
|---|---|---|
| 0.10 | 0.0943 | 0.1118 |
| 0.15 | 0.1074 | 0.1434 |
| 0.20 | 0.1204 | 0.1832 |
| 0.25 | 0.1320 | 0.2295 |
| 0.30 | 0.1417 | 0.2810 |
| B | E via NNDTFO PID | E via NNDTFO PID | E via NNDT PID |
|---|---|---|---|
| Controller via Borges Derivative | Controller | Controller | |
| 0.002 | 0.0731 | 0.0894 | 0.1297 |
| 0.004 | 0.0737 | 0.0900 | 0.1303 |
| 0.006 | 0.0749 | 0.0911 | 0.1313 |
| 0.008 | 0.0765 | 0.0927 | 0.1328 |
| 0.010 | 0.0786 | 0.0947 | 0.1347 |
| E via NNDTFO PID | E via NNDTFO PID | E via NNDT PID | |
|---|---|---|---|
| Controller via Borges Derivative | Controller | Controller | |
| 0.025 | 0.0766 | 0.0812 | 0.0877 |
| 0.020 | 0.0432 | 0.0447 | 0.0468 |
| 0.015 | 0.0166 | 0.0169 | 0.0172 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, Z. A Tuning Method via Borges Derivative of a Neural Network-Based Discrete-Time Fractional-Order PID Controller with Hausdorff Difference and Hausdorff Sum. Fractal Fract. 2021, 5, 23. https://doi.org/10.3390/fractalfract5010023
Gao Z. A Tuning Method via Borges Derivative of a Neural Network-Based Discrete-Time Fractional-Order PID Controller with Hausdorff Difference and Hausdorff Sum. Fractal and Fractional. 2021; 5(1):23. https://doi.org/10.3390/fractalfract5010023
Chicago/Turabian StyleGao, Zhe. 2021. "A Tuning Method via Borges Derivative of a Neural Network-Based Discrete-Time Fractional-Order PID Controller with Hausdorff Difference and Hausdorff Sum" Fractal and Fractional 5, no. 1: 23. https://doi.org/10.3390/fractalfract5010023
APA StyleGao, Z. (2021). A Tuning Method via Borges Derivative of a Neural Network-Based Discrete-Time Fractional-Order PID Controller with Hausdorff Difference and Hausdorff Sum. Fractal and Fractional, 5(1), 23. https://doi.org/10.3390/fractalfract5010023





