Retrofittable Solutions Capability for Gas Turbine Compressors †
Abstract
:1. Introduction
2. Computational Framework
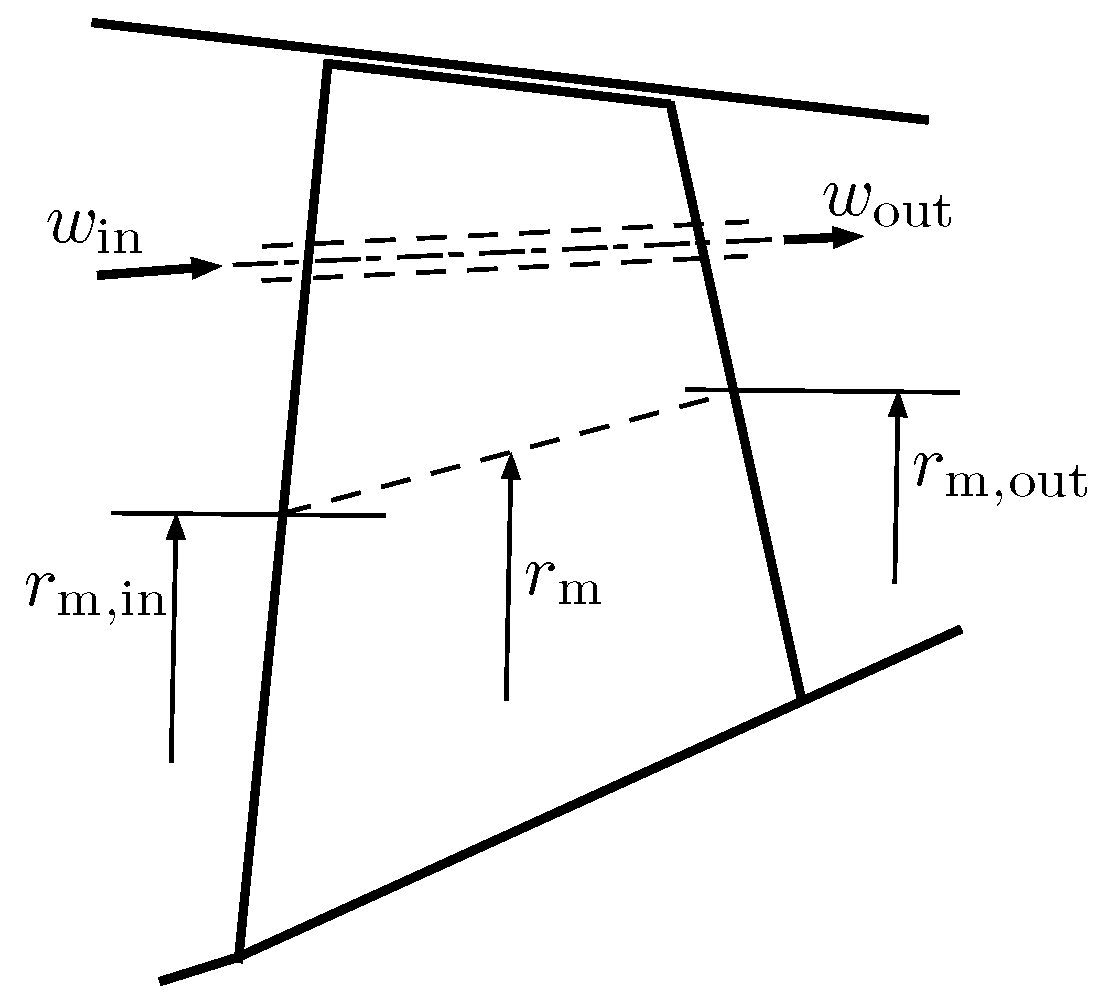
2.1. Domain Discretization
2.2. Boundary Conditions
2.3. Mass Extraction Treatment
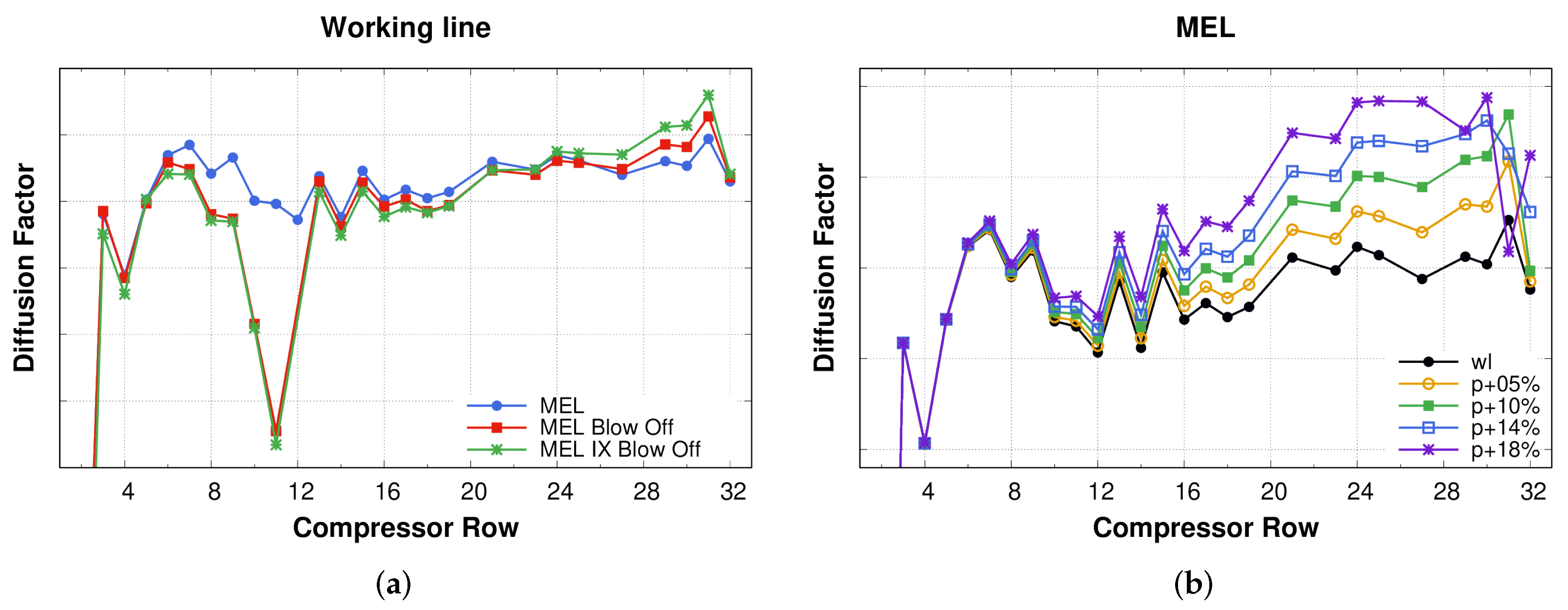
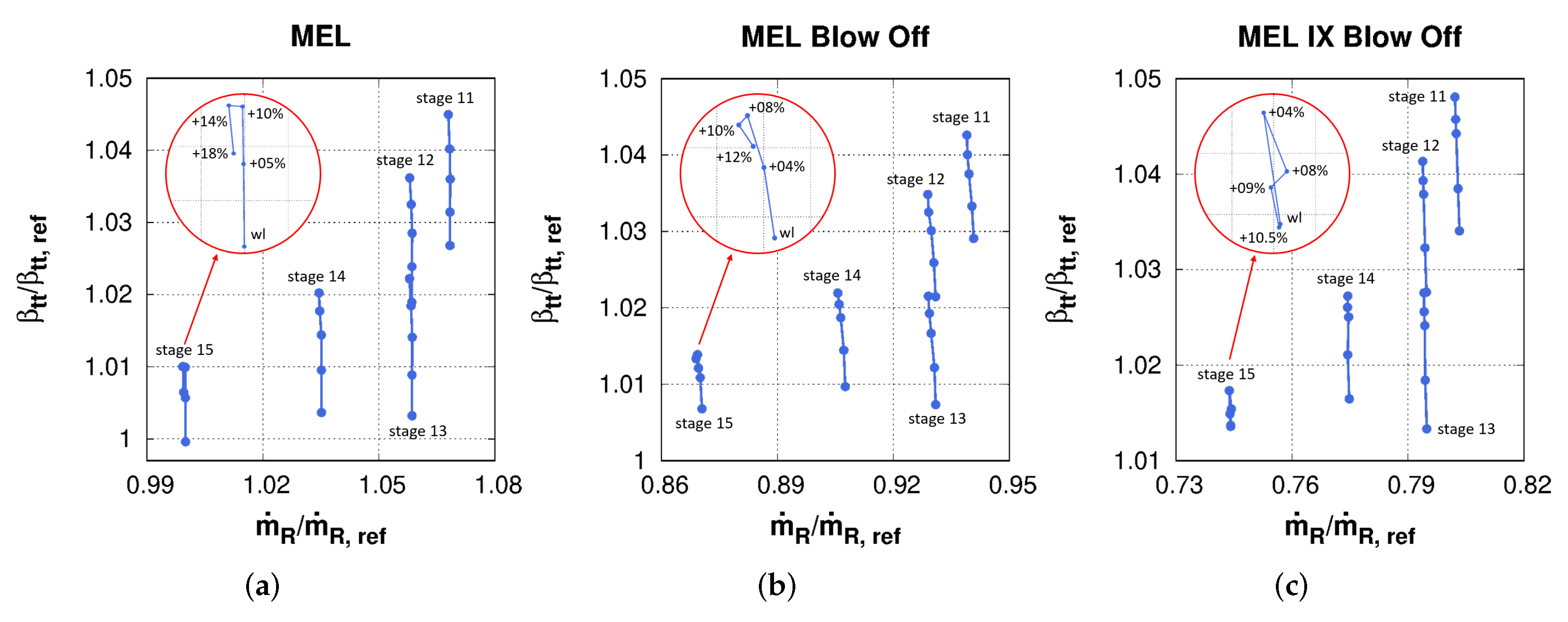
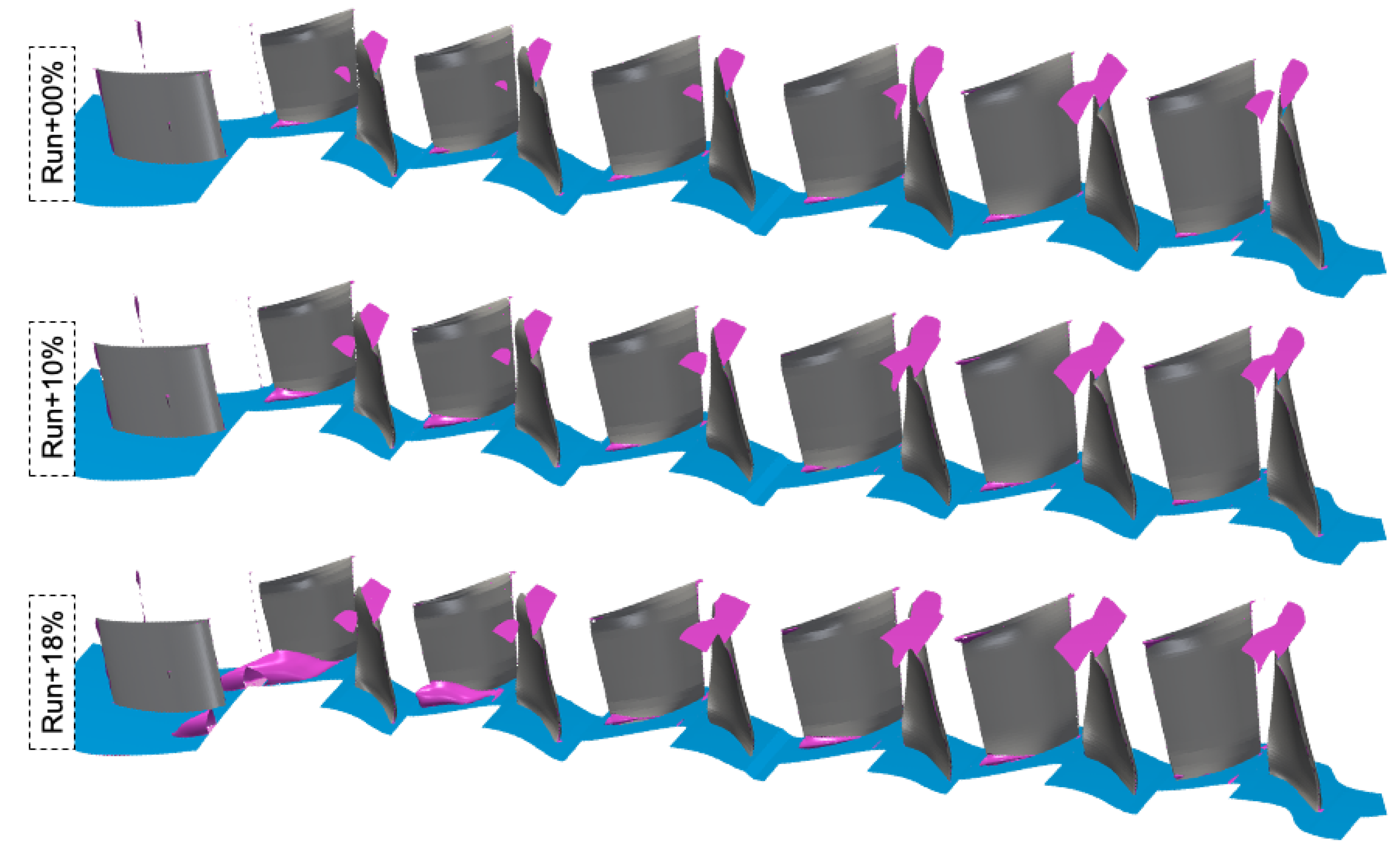
3. Results
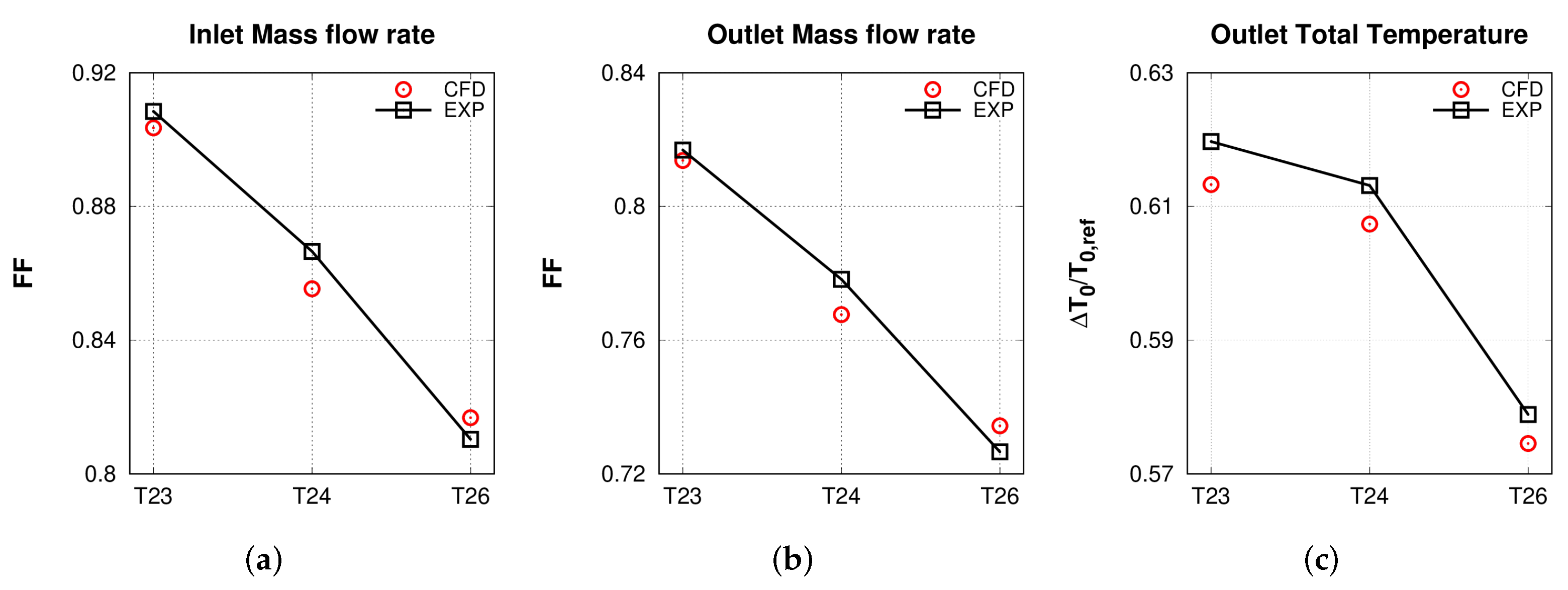
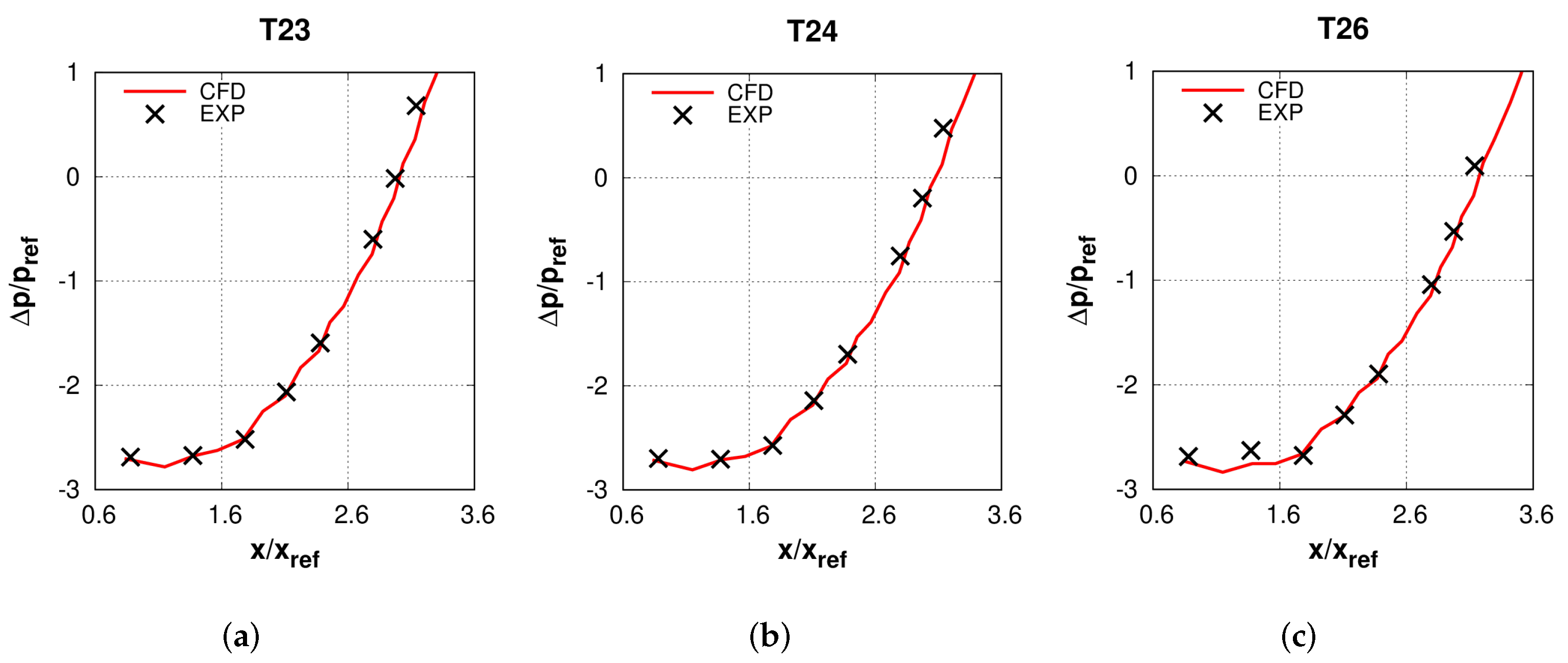
3.1. Comparison with Measurements
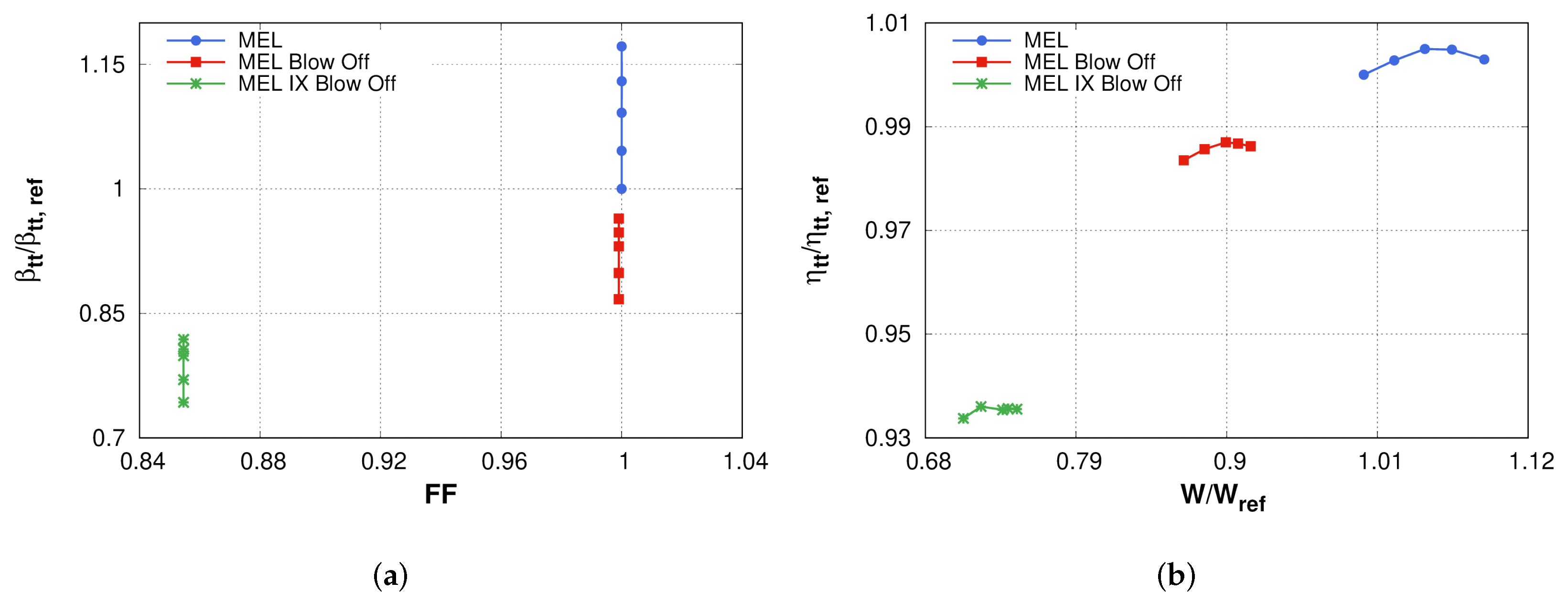
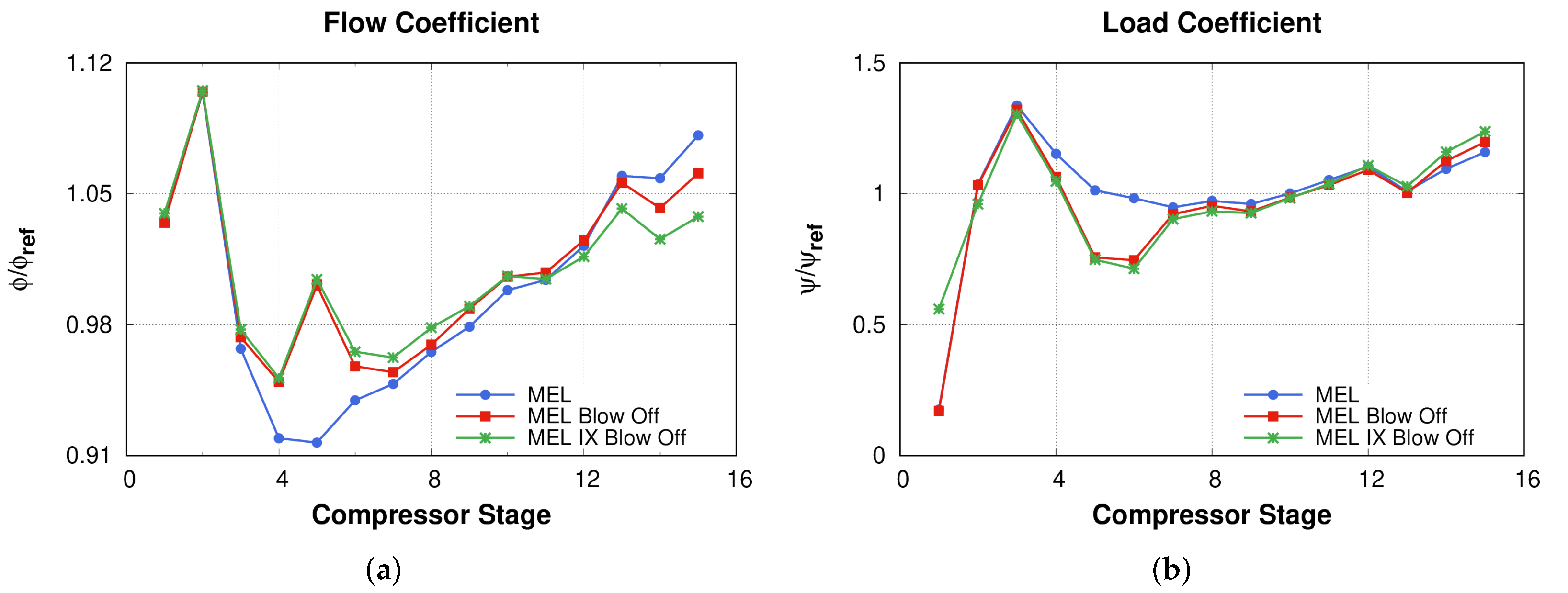
3.2. Minimum Environmental Load Reduction Strategies
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BO | Blow-off |
| IGV | Inlet guide vane |
| IX | IGV extra-closure |
| MEL | Minimum environmental load |
| OGV | Outlet guide vane |
| RES | Renewable energy systems |
| TET | Total exit temperature |
| Nomenclature | |
| Axial velocity by density ratio, | |
| c | Absolute velocity |
| C | Blade chord |
| Diffusion factor (Lieblein), | |
| Inlet flow function, | |
| h | Specific enthalpy |
| Mass flow rate | |
| N | Number of blades |
| p | Pressure |
| r | Radius |
| s | Blade row tangential spacing |
| u | Peripheral velocity, |
| T | Absolute temperature |
| x | Axial coordinate |
| w | Relative velocity |
| W | Compressor power input |
| Pressure ratio | |
| Isentropic efficiency | |
| Flow coefficient, | |
| Load coefficient, | |
| Blade row mean solidity, | |
| Subscripts | |
| 0 | Total quantity |
| in | Inlet |
| m | Mean |
| out | Outlet |
| ref | Reference |
| tt | Total-to-total |
| x | Axial |
| Density | |
| Tangential |
References
- Ruchti, C.; Olia, H.; Franitza, K.; Ehrsam, A.; Bauver, W. Combined Cycle Power Plants as Ideal Solution to Balance Grid Fluctuations—Fast Start-Up Capabilities. In Proceedings of the 43th Colloquium of Power Plant Technology., Dresden, Germany, 18–19 September 2011. [Google Scholar]
- Hailye/KQED, T. California ISO. Available online: https://www.kqed.org/science/610026/what-will-california-do-with-too-much-solar (accessed on 15 September 2021).
- Chiesa, P.; Lozza, G.; Mazzocchi, L. Using Hydrogen as Gas Turbine Fuel. J. Eng. Gas Turbines Power 2005, 127, 73–80. [Google Scholar] [CrossRef] [Green Version]
- Magni, F.; Grimm, F.; Sorato, S.; Micheli, M. GT13E2 Low Part Load Operation: Extended Flexibility Down to 30% Load. In Turbo Expo: Power for Land, Sea, and Air; Volume 3: Coal, Biomass and Alternative Fuels; Cycle Innovations; Electric Power; Industrial and Cogeneration; Organic Rankine Cycle Power Systems; ASME: New York, NY, USA, 2016. [Google Scholar] [CrossRef]
- Therkorn, D.; Gassner, M.; Lonneux, V.; Zhang, M.; Bernero, S. CCPP Operational Flexibility Extension Below 30% Load Using Reheat Burner Switch-Off Concept. In Turbo Expo: Power for Land, Sea, and Air; Volume 3: Coal, Biomass and Alternative Fuels; Cycle Innovations; Electric Power; Industrial and Cogeneration; ASME: New York, NY, USA, 2015. [Google Scholar] [CrossRef]
- Cioffi, M.; Piola, S.; Puppo, E.; Silingardi, A.; Bonzani, F. Minimum Environmental Load Reduction in Heavy Duty Gas Turbine by Bleeding Lines. In Turbo Expo: Power for Land, Sea, and Air; Volume 3A: Coal, Biomass and Alternative Fuels; Cycle Innovations; Electric Power; Industrial and Cogeneration; ASME: New York, NY, USA, 2014. [Google Scholar] [CrossRef]
- Cozzi, L.; Rubechini, F.; Marconcini, M.; Arnone, A.; Astrua, P.; Schneider, A.; Silingardi, A. Facing the Challenges in CFD Modelling of Multistage Axial Compressors. In Turbo Expo: Power for Land, Sea, and Air; Volume 2B: Turbomachinery; ASME: New York, NY, USA, 2017. [Google Scholar] [CrossRef]
- Cozzi, L.; Rubechini, F.; Giovannini, M.; Marconcini, M.; Arnone, A.; Schneider, A.; Astrua, P. Capturing Radial Mixing in Axial Compressors With Computational Fluid Dynamics. J. Turbomach. 2019, 141, 031012. [Google Scholar] [CrossRef]
- Diakunchak, I.S. Performance deterioration in industrial gas turbines. J. Eng. Gas Turbines Power 1992, 114, 161–168. [Google Scholar] [CrossRef]
- Ojo, C.O.; Schwille, C.; Nemet, A.; Zierer, T.; Nicklas, M. Optimization of Anti-Icing Limits for Alstom Gas Turbines Based on Theory of Ice Formation. In Turbo Expo: Power for Land, Sea, and Air; Volume 1: Aircraft Engine; Ceramics; Coal, Biomass and Alternative Fuels; Controls, Diagnostics and Instrumentation; Education; Electric Power; Awards and Honors; ASME: New York, NY, USA, 2009; pp. 871–879. [Google Scholar] [CrossRef]
- Mendoza, E.; Lin, T.; Jiang, X. Reduction of Gas Turbine Exhaust Temperature Limit due to HRSG Limitations by Change on Control Curve to Optimize Plant Operation and Performance. In Turbo Expo: Power for Land, Sea, and Air; Volume 3: Coal, Biomass and Alternative Fuels; Cycle Innovations; Electric Power; Industrial and Cogeneration; ASME: New York, NY, USA, 2015. [Google Scholar] [CrossRef]
- Arnone, A. Viscous Analysis of Three-Dimensional Rotor Flow Using a Multigrid Method. J. Turbomach. 1994, 116, 435–445. [Google Scholar] [CrossRef]
- Jameson, A.; Schmidt, W.; Turkel, E. Numerical Solutions of the Euler Equations by Finite Volume Methods Using Runge–Kutta Time–Stepping Schemes. In Proceedings of the 14th Fluid and Plasma Dynamics Conference, Palo Alto, CA, USA, 23–25 June 1981. AIAA Paper 81–1259. [Google Scholar]
- Wilcox, D.C. Multiscale Model for Turbulent Flows. AIAA J. 1988, 26, 1311–1320. [Google Scholar] [CrossRef]
- Burberi, C.; Michelassi, V.; Scotti del Greco, A.; Lorusso, S.; Tapinassi, L.; Marconcini, M.; Pacciani, R. Validation of steady and unsteady CFD strategies in the design of axial compressors for gas turbine engines. Aerosp. Sci. Technol. 2020, 107, 106307. [Google Scholar] [CrossRef]
- Mosele, S.G.; Garbarino, T.; Schneider, A.; Cozzi, L.; Arnone, A.; Goinis, G.; Hedkvist, S. Compressor Retrofittable Solutions in Heavy-Duty Gas Turbines for Minimum Environmental Load Reduction. E3S Web Conf. 2019, 13, 01012. [Google Scholar] [CrossRef] [Green Version]
- Giles, M.B. Non-Reflecting Boundary Conditions for the Euler Equations; Technical Report; CFDL Report 88-1; MIT Dept. of Aero. and Astro.: Boston, MA, USA, 1988. [Google Scholar]
- Giles, M.B. UNSFLO: A Numerical Method for Unsteady Inviscid Flow in Turbomachinery; Technical Report; GTL 195; MIT Dept. of Aero. and Astro.: Boston, MA, USA, 1988. [Google Scholar]
- Cioffi, M.; Puppo, E.; Silingardi, A. Fanno Design of Blow-Off Lines in Heavy Duty Gas Turbine. In Turbo Expo: Power for Land, Sea, and Air; Volume 4: Ceramics; Concentrating Solar Power Plants; Controls, Diagnostics and Instrumentation; Education; Electric Power; Fans and Blowers ASME: New York, NY, USA, 2013. [Google Scholar] [CrossRef]
- Rubechini, F.; Marconcini, M.; Arnone, A.; Cecchi, S.; Daccà, F. Some Aspects of CFD Modeling in the Analysis of a Low-Pressure Steam Turbine. In Turbo Expo: Power for Land, Sea, and Air; Paper No. GT2007-27235; ASME: New York, NY, USA, 2007. [Google Scholar]
- Vo, H.D.; Tan, C.S.; Greitzer, E.M. Criteria for Spike Initiated Rotating Stall. J. Turbomach. 2008, 130, 011023. [Google Scholar] [CrossRef]
- Lei, V.M.; Spakovszky, Z.S.; Greitzer, E.M. A Criterion for Axial Compressor Hub-Corner Stall. J. Turbomach. 2008, 130, 031006. [Google Scholar] [CrossRef] [Green Version]
- Schneider, A.; Silingardi, A.; Astrua, P.; Puppo, E.; Depalo, S. Rotor tip stall from a designer’s perspective. In Proceedings of the 12th European Turbomach Conference, Stockholm, Sweden, 3–7 April 2017. Paper ETC2017-343. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Ricci, M.; Mosele, S.G.; Benvenuto, M.; Astrua, P.; Pacciani, R.; Marconcini, M. Retrofittable Solutions Capability for Gas Turbine Compressors. Int. J. Turbomach. Propuls. Power 2022, 7, 3. https://doi.org/10.3390/ijtpp7010003
Ricci M, Mosele SG, Benvenuto M, Astrua P, Pacciani R, Marconcini M. Retrofittable Solutions Capability for Gas Turbine Compressors. International Journal of Turbomachinery, Propulsion and Power. 2022; 7(1):3. https://doi.org/10.3390/ijtpp7010003
Chicago/Turabian StyleRicci, Martina, Stefano Gino Mosele, Marcello Benvenuto, Pio Astrua, Roberto Pacciani, and Michele Marconcini. 2022. "Retrofittable Solutions Capability for Gas Turbine Compressors" International Journal of Turbomachinery, Propulsion and Power 7, no. 1: 3. https://doi.org/10.3390/ijtpp7010003
APA StyleRicci, M., Mosele, S. G., Benvenuto, M., Astrua, P., Pacciani, R., & Marconcini, M. (2022). Retrofittable Solutions Capability for Gas Turbine Compressors. International Journal of Turbomachinery, Propulsion and Power, 7(1), 3. https://doi.org/10.3390/ijtpp7010003







