A Cyclic Multi-Stage Implementation of the Full-Waveform Inversion for the Identification of Anomalies in Dams
Abstract
1. Introduction
- Materials and methods: the formulation/algorithm of the forward model and its boundary conditions, the inverse model, and the inversion process.
- Numerical simulation: the geometry of the dam, the acquisition geometry, the wave parameters, and the cyclic multi-stage implementation under different conditions.
- Results: FWI results for different acquisition geometries, cyclic multi-stage inversion, and for different levels of noise.
- Conclusion: a summary of the applicability of our approach to identifying damages in dams.
2. Materials and Methods
2.1. Forward Model
2.2. Inverse Analysis
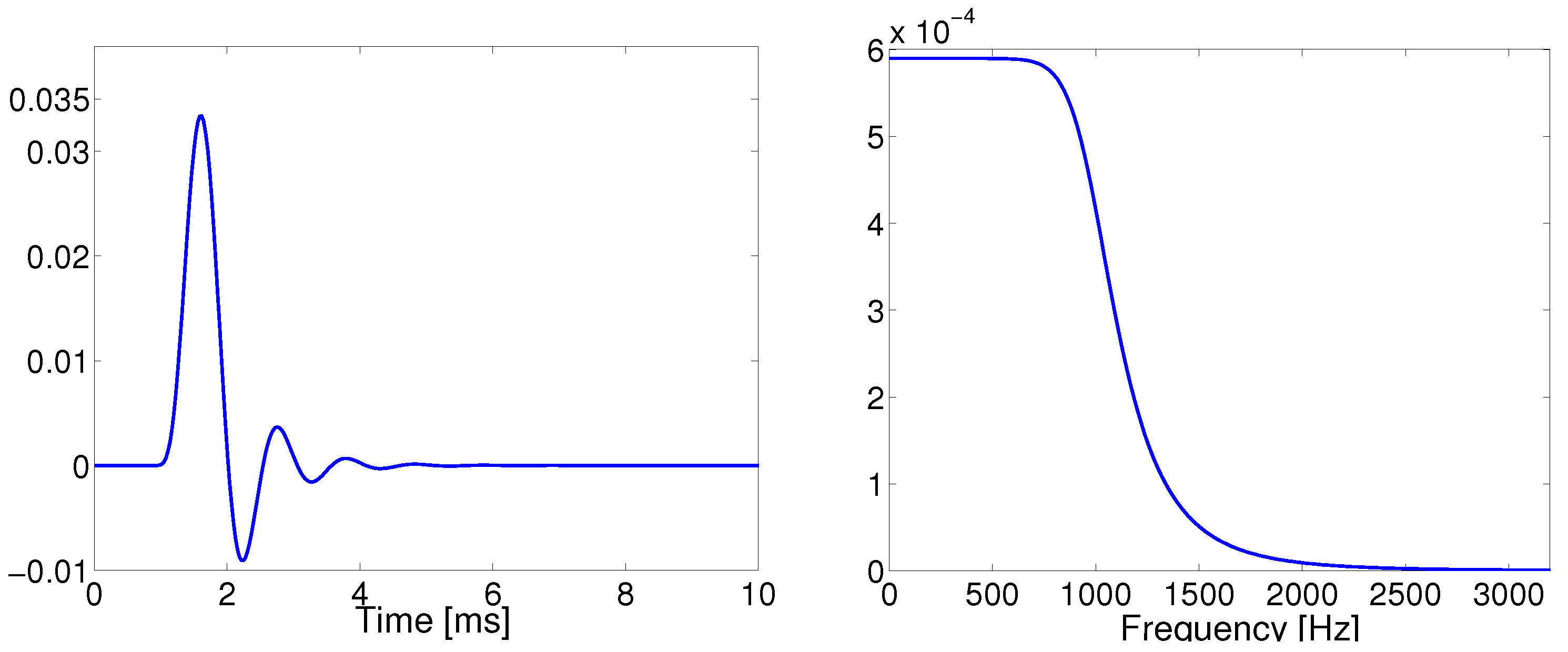
3. Numerical Simulation Example
4. Results
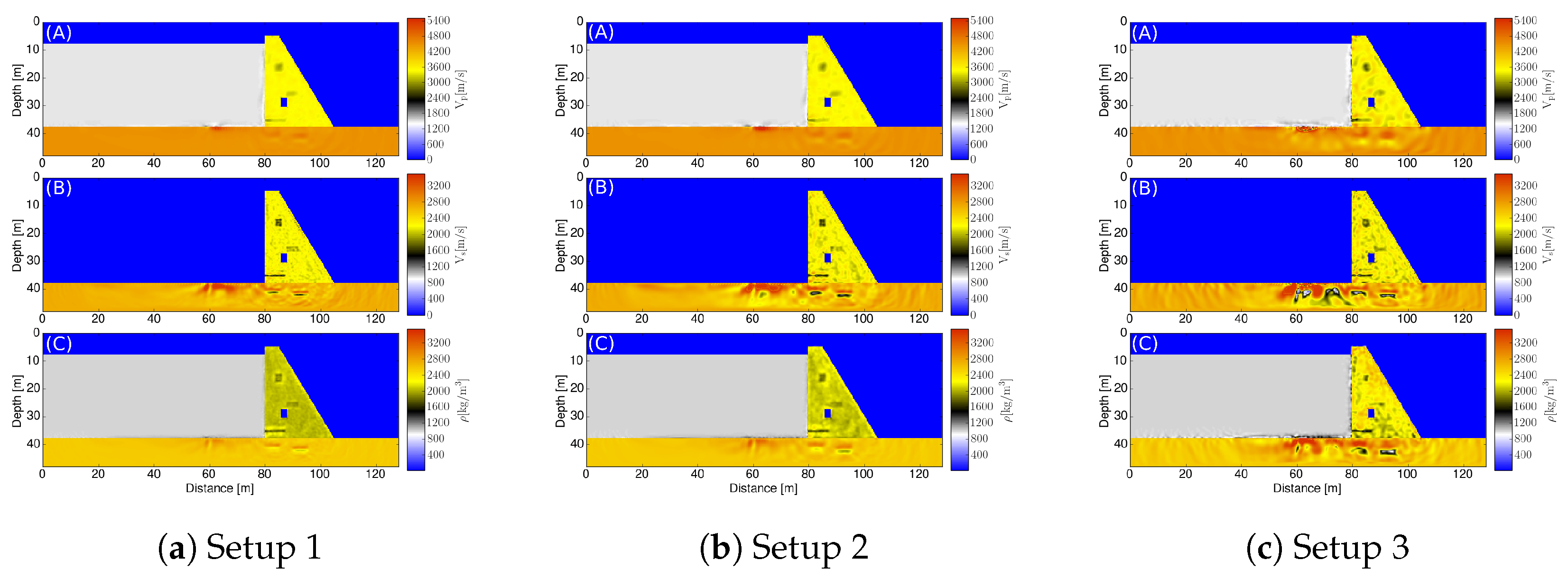
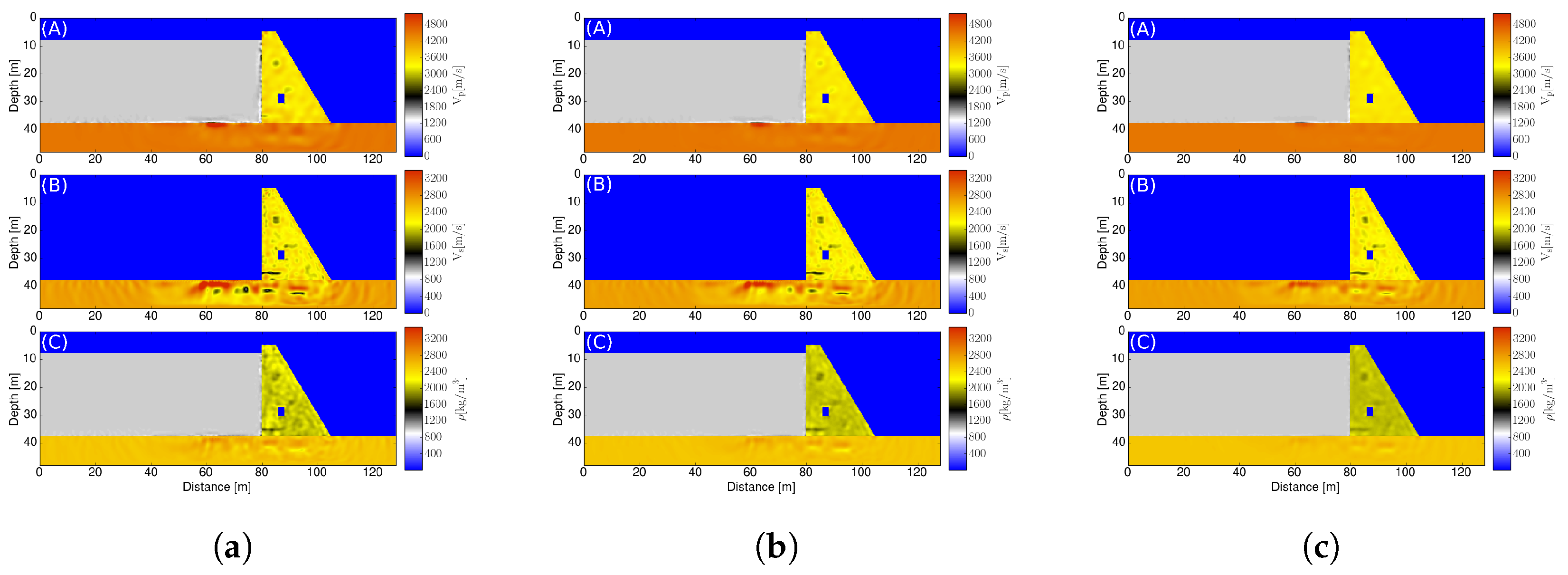
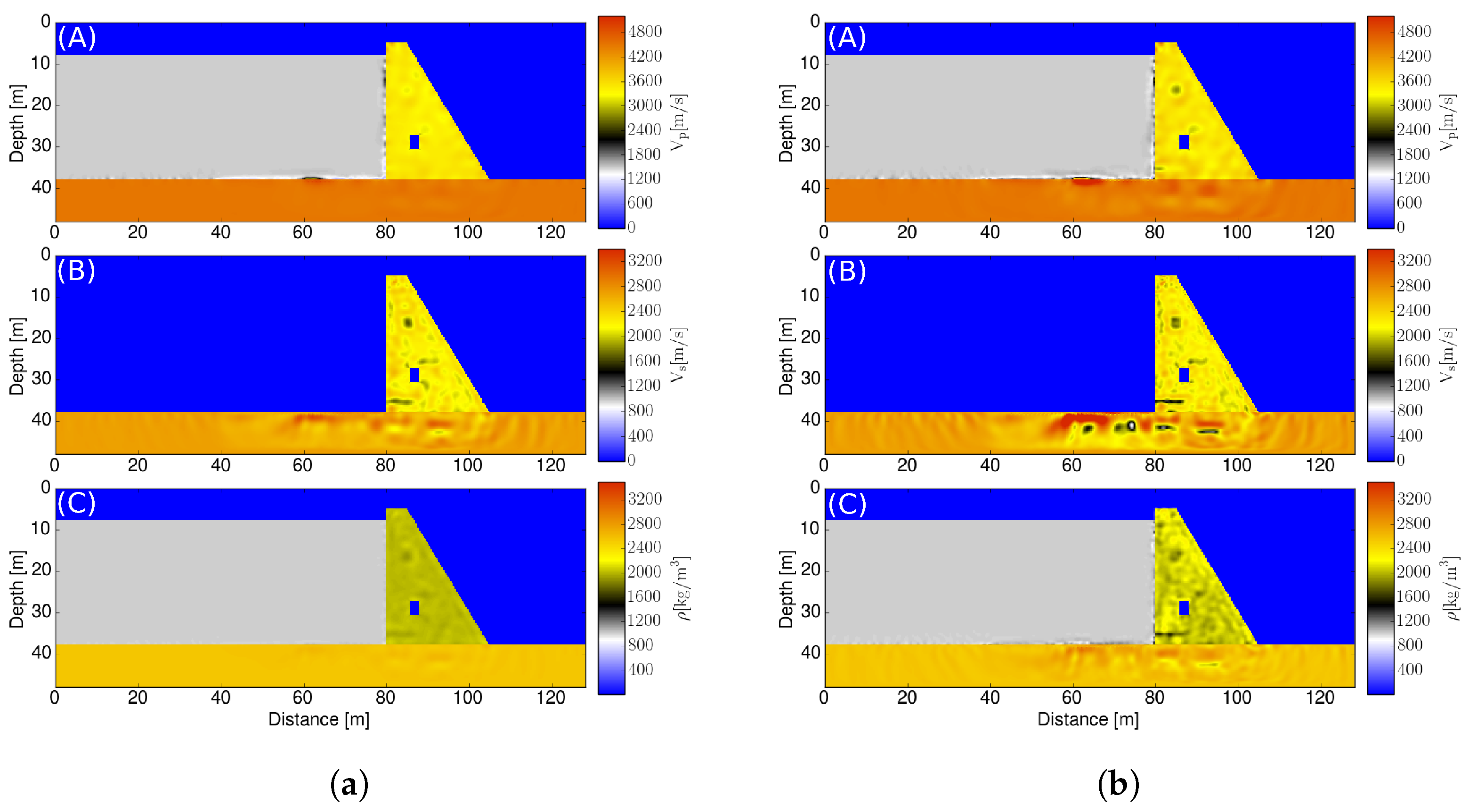
4.1. Optimal Acquisition Geometry Selection
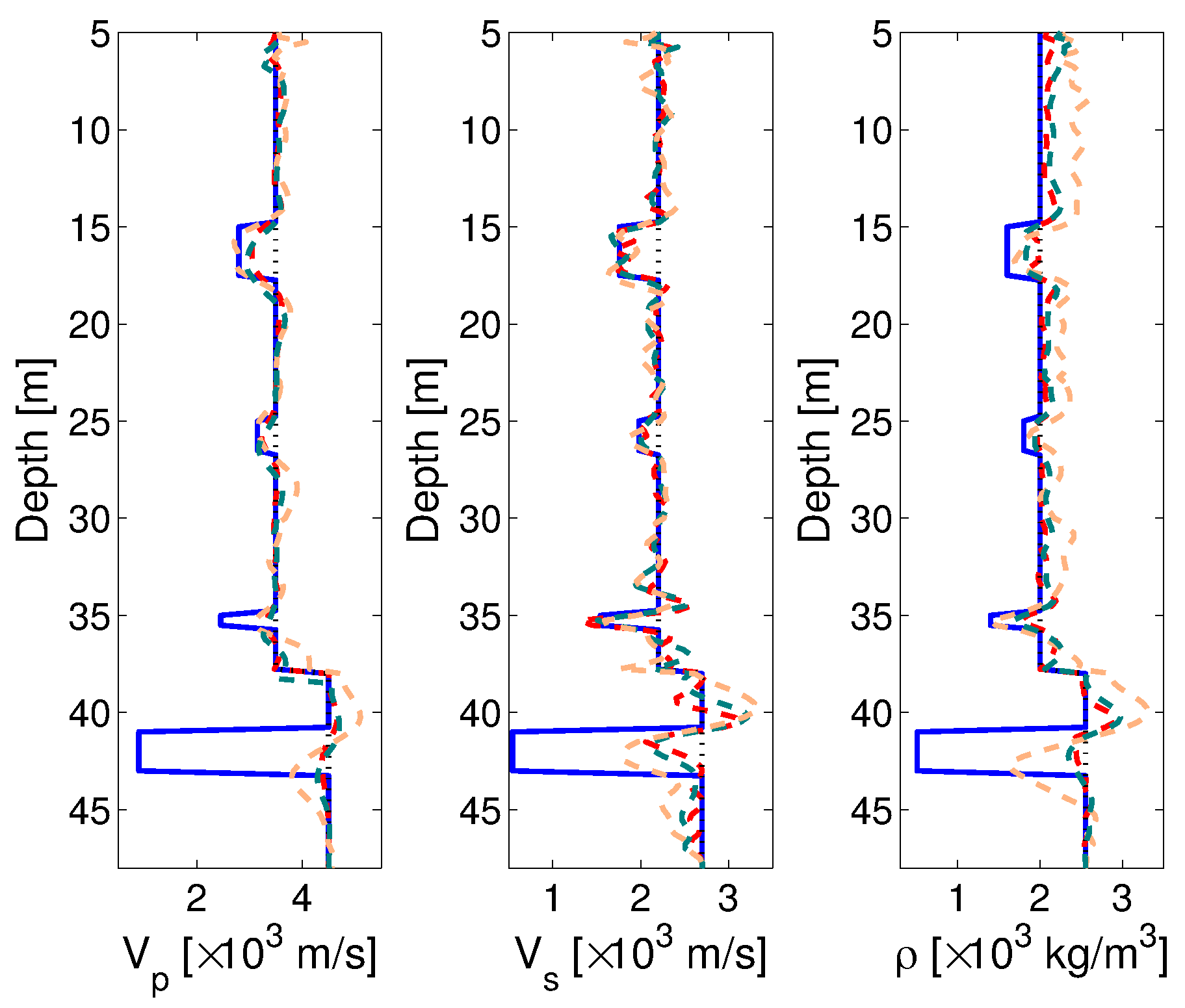
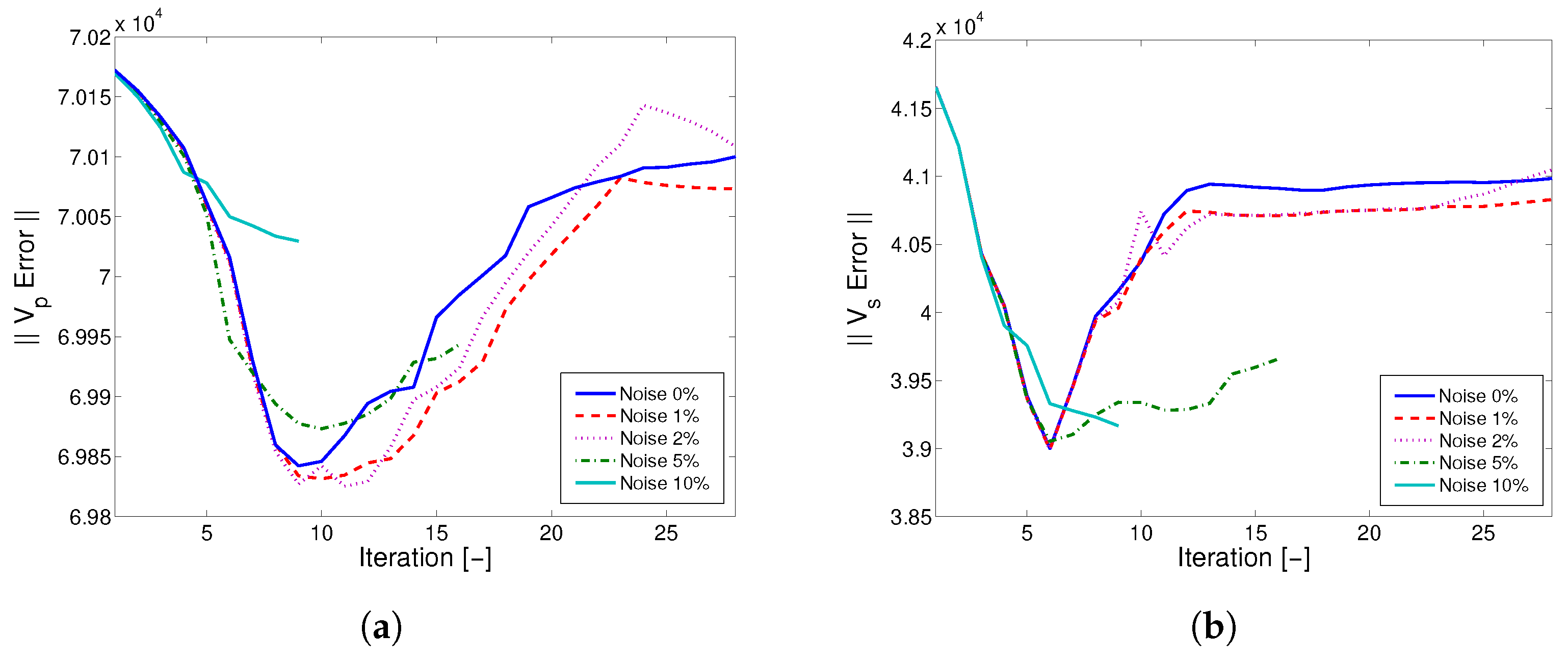
4.2. Influence of Noise (Disturbance in Obtained Data) on the Reconstruction Quality/Error
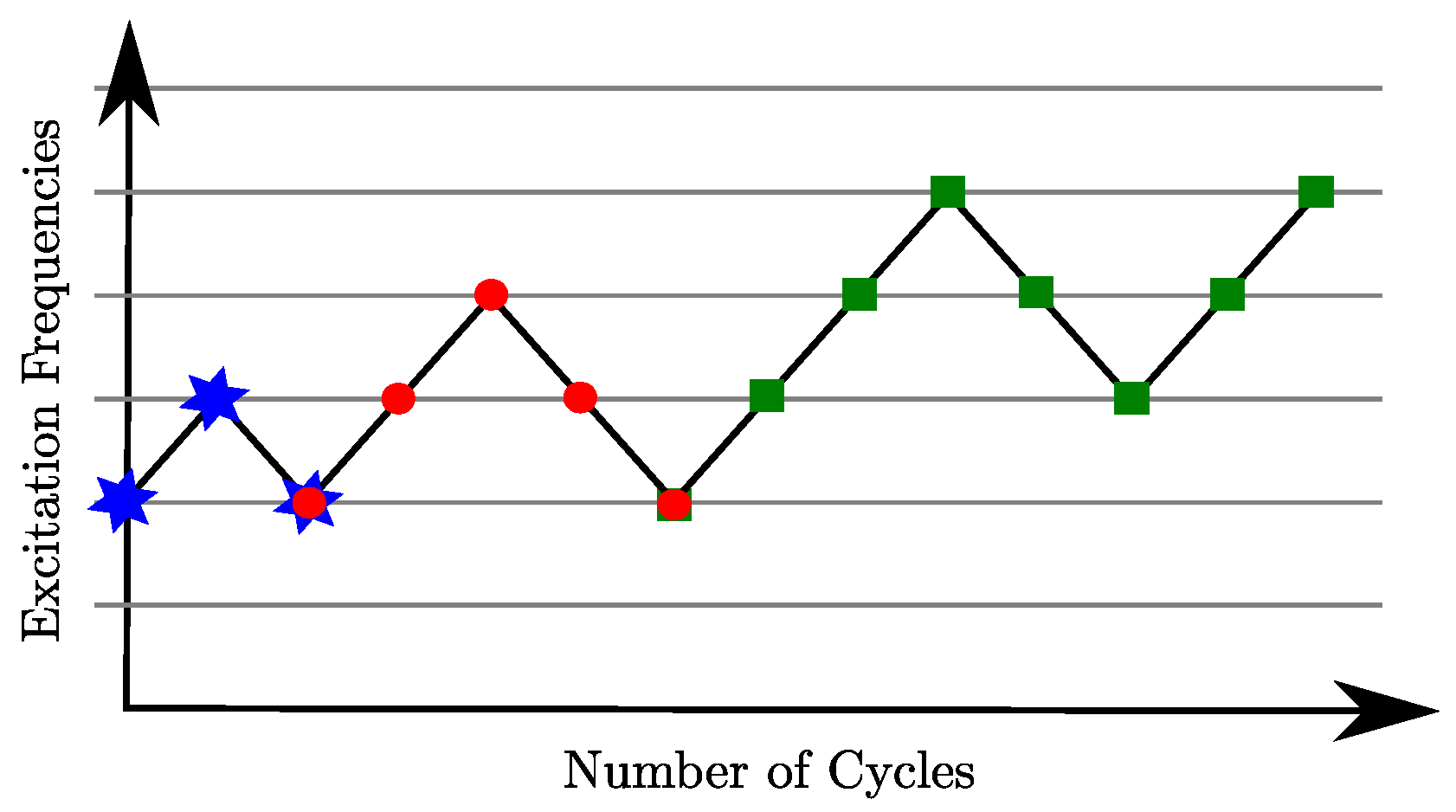
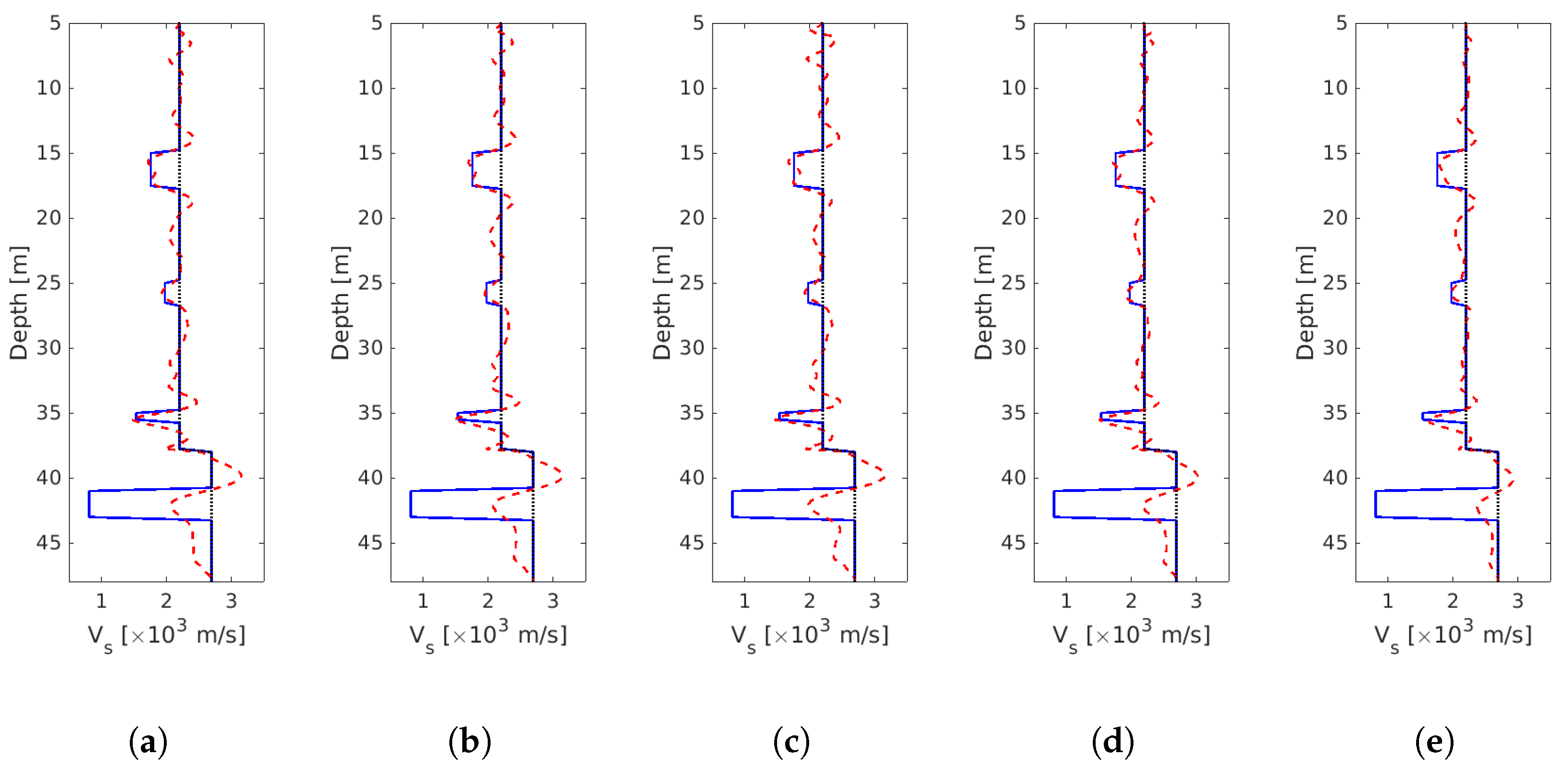
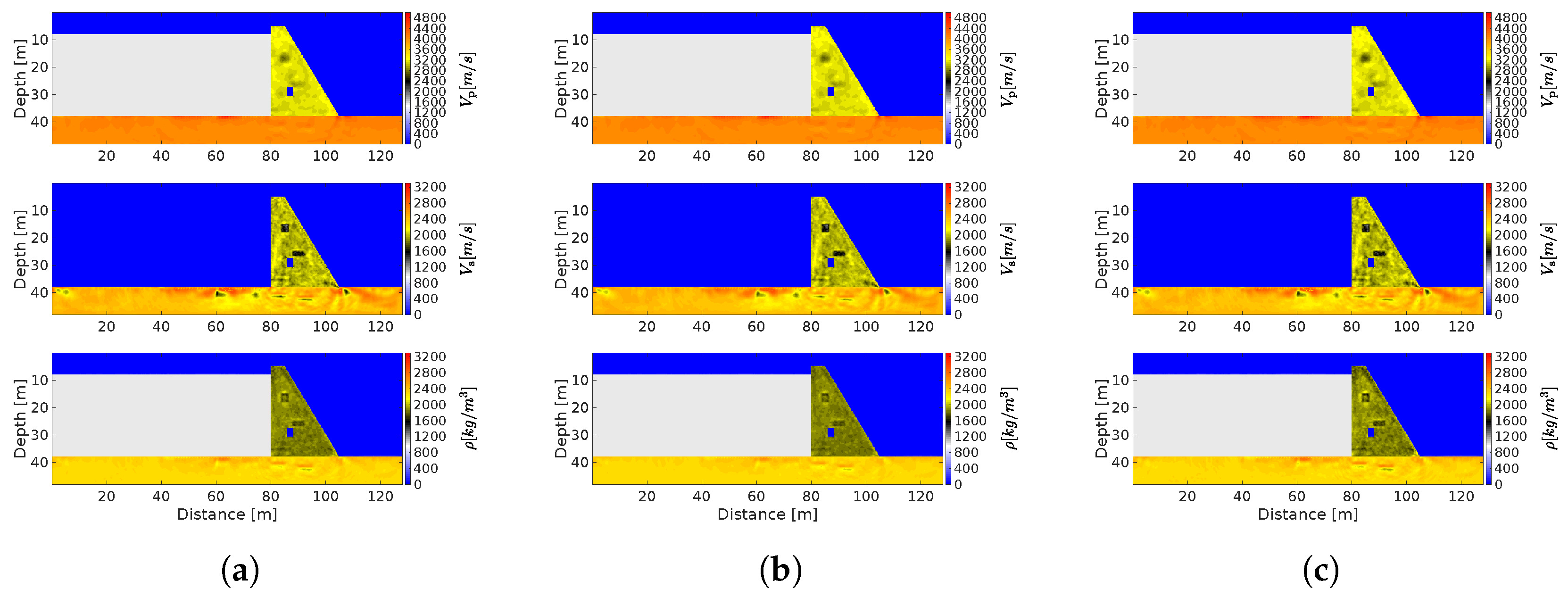
4.3. Cyclic Multi-Frequency Stage Inversion
4.3.1. Influence of Noise (Disturbance in Obtained Data) on the Reconstruction Quality for Cyclic Multi-Frequency Stage Inversion
4.3.2. Influence of Noise (Disturbance in Obtained Data) and Uncertainty in the Starting Model on the Reconstruction Quality for Cyclic-Multi-Frequency Stage Inversion
5. Conclusions
- The proposed FWI formulation is capable of effectively identifying and quantifying regions of weaknesses (i.e., heterogeneity) in both the dam structure and its foundation.
- The dam’s as-built material properties are used as a starting model for the inversion. This information is, in most cases, readily available, or can be easily estimated. If the material properties are completely unknown, it is recommended to underestimate and not to overestimate them.
- The efficiency of this method is influenced by the data acquisition geometry. Thus, we propose an acquisition setup which encloses regions in which critical damage is expected.
- The damaged regions are generally of lower velocities and smaller scale; thus, the model, with its shorter wavelength, resolves the anomalies better than the and models.
- A superimposition of the and models to identify damaged regions saturated with water increases the robustness of the method by leveraging the advantages of both models.
- The identified damages in the dam body had a better quality than the ones in the dam foundation for data corrupted with high noise levels. Thus, we propose, where possible, an acquisition setup which favors the recording of transmitted waves.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dokter, E.; Köhn, D.; Wilken, D.; Nil, D.; Rabbel, W. Full-waveform inversion of SH-and Love-wave data in near-surface prospecting. Geophys. Prospect. 2017, 65, 216–236. [Google Scholar] [CrossRef]
- Fichtner, A.; Kennett, B.L.; Igel, H.; Bunge, H.P. Full waveform tomography for radially anisotropic structure: New insights into present and past states of the Australasian upper mantle. Earth Planet. Sci. Lett. 2010, 290, 270–280. [Google Scholar] [CrossRef]
- Köhn, D.; De Nil, D.; al Hagrey, S.; Rabbel, W.; Khaledi, K.; König, D.; Schanz, T. Monitoring elastic parameter changes in the vicinity of salt caverns due to cyclic loading by seismic waveform inversion. In Proceedings of the Energy Geotechnics: Proceedings of the 1st International Conference on Energy Geotechnics, ICEGT 2016, Kiel, Germany, 29–31 August 2016; CRC Press: Boca Raton, FL, USA, 2016; p. 45. [Google Scholar]
- Nguyen-Tuan, L.; Lahmer, T.; Datcheva, M.; Stoimenova, E.; Schanz, T. A novel parameter identification approach for buffer elements involving complex coupled thermo-hydro-mechanical analyses. Comput. Geotech. 2016, 76, 23–32. [Google Scholar] [CrossRef]
- Operto, S.; Virieux, J.; Dessa, J.X.; Pascal, G. Crustal seismic imaging from multifold ocean bottom seismometer data by frequency domain full-waveform tomography: Application to the eastern Nankai trough. J. Geophys. Res. Solid Earth 2006, 111, B09306. [Google Scholar] [CrossRef]
- Operto, S.; Miniussi, A.; Brossier, R.; Combe, L.; Métivier, L.; Monteiller, V.; Ribodetti, A.; Virieux, J. Efficient 3-D frequency-domain mono-parameter full-waveform inversion of ocean-bottom cable data: Application to Valhall in the visco-acoustic vertical transverse isotropic approximation. Geophys. J. Int. 2015, 202, 1362–1391. [Google Scholar] [CrossRef]
- Peter, D.; Komatitsch, D.; Luo, Y.; Martin, R.; Le Goff, N.; Casarotti, E.; Le Loher, P.; Magnoni, F.; Liu, Q.; Blitz, C.; et al. Forward and adjoint simulations of seismic wave propagation on fully unstructured hexahedral meshes. Geophys. J. Int. 2011, 186, 721–739. [Google Scholar] [CrossRef]
- Plessix, R.E.; Perkins, C. Thematic Set: Full waveform inversion of a deep water ocean bottom seismometer dataset. First Break 2010, 28, 71–78. [Google Scholar] [CrossRef]
- Sirgue, L.; Barkved, O.; Dellinger, J.; Etgen, J.; Albertin, U.; Kommedal, J. Thematic set: Full waveform inversion: The next leap forward in imaging at Valhall. First Break 2010, 28, 65–70. [Google Scholar] [CrossRef]
- Tape, C.; Liu, Q.; Maggi, A.; Tromp, J. Seismic tomography of the southern California crust based on spectral-element and adjoint methods. Geophys. J. Int. 2010, 180, 433–462. [Google Scholar] [CrossRef]
- Tran, K.T.; Luke, B. Full waveform tomography to resolve desert alluvium. Soil Dyn. Earthq. Eng. 2017, 99, 1–8. [Google Scholar] [CrossRef]
- Tran, K.T.; McVay, M. Site characterization using Gauss–Newton inversion of 2-D full seismic waveform in the time domain. Soil Dyn. Earthq. Eng. 2012, 43, 16–24. [Google Scholar] [CrossRef]
- Vigh, D.; Jiao, K.; Watts, D.; Sun, D. Elastic full-waveform inversion application using multicomponent measurements of seismic data collection. Geophysics 2014, 79, R63–R77. [Google Scholar] [CrossRef]
- Zhu, H.; Bozdağ, E.; Peter, D.; Tromp, J. Structure of the European upper mantle revealed by adjoint tomography. Nat. Geosci. 2012, 5, 493–498. [Google Scholar] [CrossRef]
- Tang, W.H.; Yen, B.C. Dam safety inspection scheduling. J. Hydraul. Eng. 1991, 117, 214–229. [Google Scholar] [CrossRef]
- Alalade, M. An Enhanced Full Waveform Inversion Method for the Structural Analysis of Dams. Ph.D. Thesis, Bauhaus-Universität Weimar, Weimar, Germany, 2019. [Google Scholar] [CrossRef]
- Lee, W.; Hoopes, J.A. Prediction of cavitation damage for spillways. J. Hydraul. Eng. 1996, 122, 481–488. [Google Scholar] [CrossRef]
- Mata, J.; Leitão, N.S.; de Castro, A.T.; da Costa, J.S. Construction of decision rules for early detection of a developing concrete arch dam failure scenario. A discriminant approach. Comput. Struct. 2014, 142, 45–53. [Google Scholar] [CrossRef]
- Nanthakumar, S.; Lahmer, T.; Zhuang, X.; Zi, G.; Rabczuk, T. Detection of material interfaces using a regularized level set method in piezoelectric structures. Inverse Probl. Sci. Eng. 2016, 24, 153–176. [Google Scholar] [CrossRef]
- Xu, X.; Wu, J.; Shen, J.; He, Z. Case study: Application of GPR to detection of hidden dangers to underwater hydraulic structures. J. Hydraul. Eng. 2006, 132, 12–20. [Google Scholar] [CrossRef]
- Lahmer, T.; Könke, C.; Bettzieche, V. Optimal Monitoring of Gravity Dams by Multifield Considerations. 2011. Available online: http://www.talsperren.info/assets/applets/optimalmonitoring.pdf (accessed on 18 November 2022).
- Lahmer, T. Crack identification in hydro-mechanical systems with applications to gravity water dams. Inverse Probl. Sci. Eng. 2010, 18, 1083–1101. [Google Scholar] [CrossRef]
- Alalade, M.; Nguyen-Tuan, L.; Wuttke, F.; Lahmer. Inverse Analysis of Coupled Hydro-Mechanical Problem in Dynamically Excited Dams. In Proceedings of the 7th International Workshop on Reliable Engineering Computing: Computing with Polymorphic Uncertainty Data, Bochum, Germany, 15–17 June 2016; Freitag, S., Rafi, L., Muhanna, R.L.M., Eds.; 2016. Available online: https://www.researchgate.net/publication/308674834_Inverse_Analysis_of_Coupled_Hydro-Mechanical_Problem_in_Dynamically_Excited_Dams (accessed on 18 November 2022).
- Alalade, M.; Nguyen-Tuan, L.; Wuttke, F.; Lahmer, T. Damage identification in gravity dams using dynamic coupled hydro-mechanical XFEM. Int. J. Mech. Mater. Des. 2018, 14, 157–175. [Google Scholar] [CrossRef]
- Nguyen-Tuan, L.; Könke, C.; Lahmer, T. Damage identification using inverse analysis for 3D coupled thermo-hydro-mechanical problems. Comput. Struct. 2018, 196, 146–156. [Google Scholar] [CrossRef]
- Nguyen-Tuan, L.; Könke, C.; Bettzieche, V.; Lahmer, T. Damage identification using inverse analysis in coupled thermo-hydro-mechanical problems applied to masonry dams. Int. J. Numer. Anal. Methods Geomech. 2018, 42, 256–273. [Google Scholar] [CrossRef]
- Yao, F.H.; Guan, S.H.; Yang, H.; Chen, Y.; Qiu, H.F.; Ma, G.; Liu, Q.W. Long-term deformation analysis of Shuibuya concrete face rockfill dam based on response surface method and improved genetic algorithm. Water Sci. Eng. 2019, 12, 196–204. [Google Scholar] [CrossRef]
- Dou, S.Q.; Li, J.J.; Kang, F. Health diagnosis of concrete dams using hybrid FWA with RBF-based surrogate model. Water Sci. Eng. 2019, 12, 188–195. [Google Scholar] [CrossRef]
- Lailly, P.; Bednar, J. The seismic inverse problem as a sequence of before stack migrations. In Conference on Inverse Scattering: Theory and Application; SIAM: Philadelphia, PA, USA, 1983; pp. 206–220. [Google Scholar]
- Tarantola, A.; Santosa, F. The seismic reflection inverse problem. Inverse Probl. Acoust. Elastic Waves 1984, 14, 104–181. [Google Scholar]
- Métivier, L.; Brossier, R.; Mérigot, Q.; Oudet, E.; Virieux, J. Measuring the misfit between seismograms using an optimal transport distance: Application to full-waveform inversion. Geophys. J. Int. 2016, 205, 345–377. [Google Scholar] [CrossRef]
- Ntengue Chatue, P. Towards the Application of 2D Elastic Full-Waveform Inversion to Ultrasonic Data for Non-Destructive Testing. Master’s Thesis, Karlsruher Institut für Technologie (KIT), Karlsruhe, Germany, 2021. [Google Scholar] [CrossRef]
- Jazayeri, S.; Kruse, S.; Hasan, I.; Yazdani, N. Reinforced concrete mapping using full-waveform inversion of GPR data. Constr. Build. Mater. 2019, 229, 117102. [Google Scholar] [CrossRef]
- Krischer, L.; Strobach, E.; Boehm, C.; Afanasiev, M.; ANGST, U. Full-waveform inversion of ultrasonic echo signals to evaluate grouting quality of tendon ducts in post-tensioned concrete structures. In Proceedings of the NDT-CE 2022-The International Symposium on Nondestructive Testing in Civil Engineering, Zurich, Switzerland, 16–18 August 2022. [Google Scholar]
- Chen, J.; Wang, H.; Song, D.; Ge, X. A Frequency-Domain Full Waveform Inversion Method of Elastic Waves in Quantitative Defection Investigation; NISCAIR-CSIR: New Delhi, India, 2019. [Google Scholar]
- Alalade, M.; Kafle, B.; Wuttke, F.; Lahmer, T. Inverse analysis of cyclic constitutive models for unsaturated soil under consideration of oscillating functions. In Proceedings of the E3S Web of Conferences. EDP Sciences, Krakow, Poland, 17–19 May 2016; Volume 9, p. 08012. [Google Scholar]
- Aki, K.; Richards, P.G. Quantitative Seismology, Theory and Methods; WH Freeman & Co.: New York, NY, USA, 1980; Volume 1. [Google Scholar]
- Virieux, J. P-SV wave propagation in heterogeneous media: Velocity-stress finite-difference method. Geophysics 1986, 51, 889–901. [Google Scholar] [CrossRef]
- Levander, A.R. Fourth-order finite-difference P-SV seismograms. Geophysics 1988, 53, 1425–1436. [Google Scholar] [CrossRef]
- Courant, R.; Friedrichs, K.; Lewy, H. On the partial difference equations of mathematical physics. IBM J. 1967, 11, 215–234. [Google Scholar] [CrossRef]
- Zeng, C.; Xia, J.; Miller, R.D.; Tsoflias, G.P. An improved vacuum formulation for 2D finite-difference modeling of Rayleigh waves including surface topography and internal discontinuities. Geophysics 2012, 77, T1–T9. [Google Scholar] [CrossRef]
- Nocedal, J.; Wright, S. Numerical Optimization; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Shipp, R.M.; Singh, S.C. Two-dimensional full wavefield inversion of wide-aperture marine seismic streamer data. Geophys. J. Int. 2002, 151, 325–344. [Google Scholar] [CrossRef]
- Tarantola, A. Inverse Problem Theory and Methods for Model Parameter Estimation; SIAM: Philadelphia, PA, USA, 2005; Volume 89. [Google Scholar]
- Plessix, R.E. A review of the adjoint-state method for computing the gradient of a functional with geophysical applications. Geophys. J. Int. 2006, 167, 495–503. [Google Scholar] [CrossRef]
- Köhn, D. Time Domain 2D Elastic Full Waveform Tomography. Ph.D. Thesis, Christian-Albrechts-Universität zu Kiel, Kiel, Germany, 2011. Available online: http://nbn-resolving.de/urn:nbn:de:gbv:8-diss-67866 (accessed on 12 October 2022).
- Köhn, D.; De Nil, D.; Kurzmann, A.; Przebindowska, A.; Bohlen, T. On the influence of model parametrization in elastic full-waveform tomography. Geophys. J. Int. 2012, 191, 325–345. [Google Scholar] [CrossRef]
- Köhn, D.; De Nil, D.; Kurzmann, A.; Rabbel, W. Full Waveform Tomography-Part 1 Theory. 2015. Available online: https://www.yumpu.com/en/document/view/21547351/full-waveform-tomography-part-1-theory (accessed on 18 November 2022).
- Köhn, D.; Kurzmann, A.; De Nil, D.; Groos, L. DENISE—User Manual. 2014. Available online: https://danielkoehnsite.wordpress.com/software/ (accessed on 12 October 2022).
- Sirgue, L.; Pratt, R.G. Efficient waveform inversion and imaging: A strategy for selecting temporal frequencies. Geophysics 2004, 69, 231–248. [Google Scholar] [CrossRef]
- Métivier, L.; Brossier, R.; Virieux, J.; Operto, S. Full waveform inversion and the truncated Newton method. SIAM J. Sci. Comput. 2013, 35, B401–B437. [Google Scholar] [CrossRef]
- Pratt, R.G.; Shin, C.; Hick, G.J. Gauss–Newton and full Newton methods in frequency–space seismic waveform inversion. Geophys. J. Int. 1998, 133, 341–362. [Google Scholar] [CrossRef]

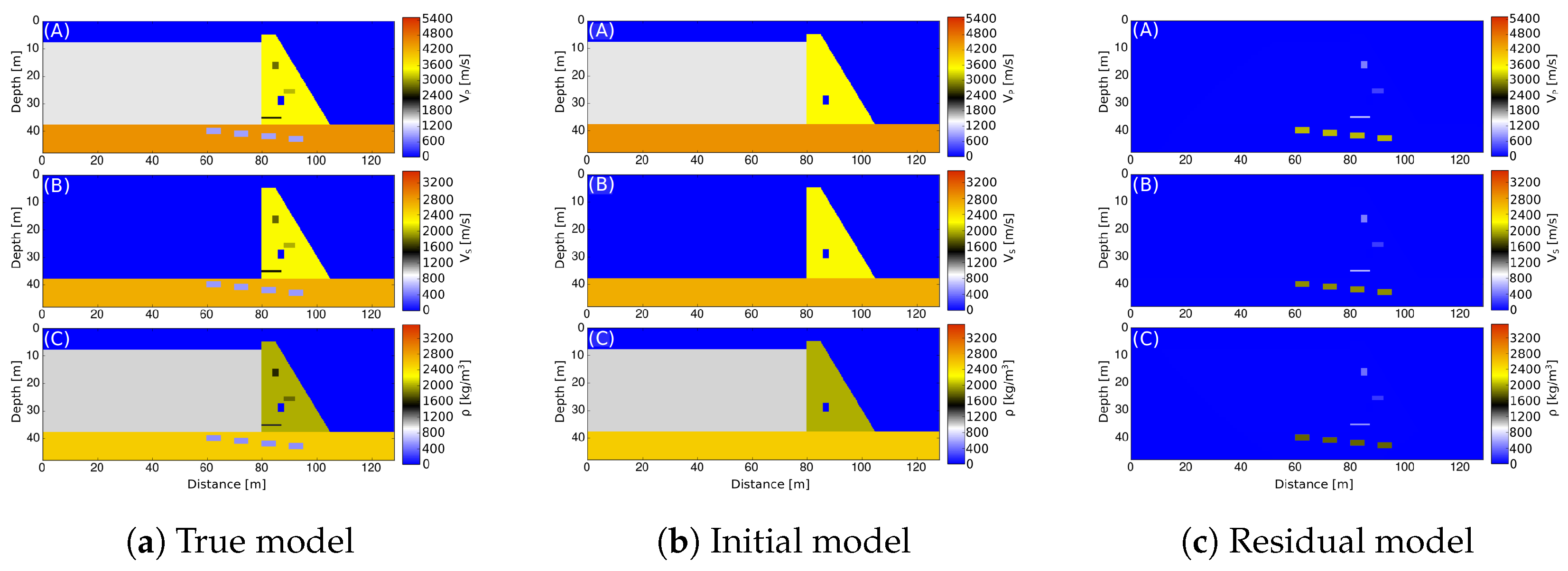







| Material | [m/s] | [m/s] | [kg/m] |
|---|---|---|---|
| Dam body | 3500 | 2200 | 2000 |
| Dam tunnel | 0 | 0 | 1.25 |
| Dam foundation | 4500 | 2700 | 2550 |
| Water | 1500 | 0 | 1000 |
| Air/Vacuum | 0 | 0 | 1.25 |
| Noise Level [%] | Computation Time [h] | Number of Iterations of | |||
|---|---|---|---|---|---|
| after 13 Iterations | Stage 1 | Stage 2 | Stage 3 | Stage 4 | |
| 0 | 0.68 | 14 | 6 | 6 | 6 |
| 1 | 0.85 | 7 | 15 | 6 | 5 |
| 2 | 0.83 | 7 | 15 | 6 | 6 |
| 5 | 0.75 | 5 | 7 | 5 | 5 |
| 10 | 0.78 | 4 | 3 | 3 | 3 |
| Cycle 1 | Cycle 2 | Cycle 3 | |||
|---|---|---|---|---|---|
| Frequency [Hz] | [s] | Frequency [Hz] | [s] | Frequency [Hz] | [s] |
| 0.4 | 0.03 | 0.4 | 0.06 | 0.6 | 0.1 |
| 0.6 | 0.03 | 0.6 | 0.06 | 0.8 | 0.1 |
| 0.4 | 0.03 | 0.8 | 0.06 | 1.0 | 0.1 |
| 0.6 | 0.06 | 0.8 | 0.1 | ||
| 0.4 | 0.06 | 0.6 | 0.1 | ||
| 0.8 | 0.1 | ||||
| 1.0 | 0.1 | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alalade, M.; Reichert, I.; Köhn, D.; Wuttke, F.; Lahmer, T. A Cyclic Multi-Stage Implementation of the Full-Waveform Inversion for the Identification of Anomalies in Dams. Infrastructures 2022, 7, 161. https://doi.org/10.3390/infrastructures7120161
Alalade M, Reichert I, Köhn D, Wuttke F, Lahmer T. A Cyclic Multi-Stage Implementation of the Full-Waveform Inversion for the Identification of Anomalies in Dams. Infrastructures. 2022; 7(12):161. https://doi.org/10.3390/infrastructures7120161
Chicago/Turabian StyleAlalade, Muyiwa, Ina Reichert, Daniel Köhn, Frank Wuttke, and Tom Lahmer. 2022. "A Cyclic Multi-Stage Implementation of the Full-Waveform Inversion for the Identification of Anomalies in Dams" Infrastructures 7, no. 12: 161. https://doi.org/10.3390/infrastructures7120161
APA StyleAlalade, M., Reichert, I., Köhn, D., Wuttke, F., & Lahmer, T. (2022). A Cyclic Multi-Stage Implementation of the Full-Waveform Inversion for the Identification of Anomalies in Dams. Infrastructures, 7(12), 161. https://doi.org/10.3390/infrastructures7120161










