Structural Stability of Cycle Paths—Introducing Cycle Path Deflection Bowl Parameters from FWD Measurements
Abstract
1. Introduction
2. Materials and Methods
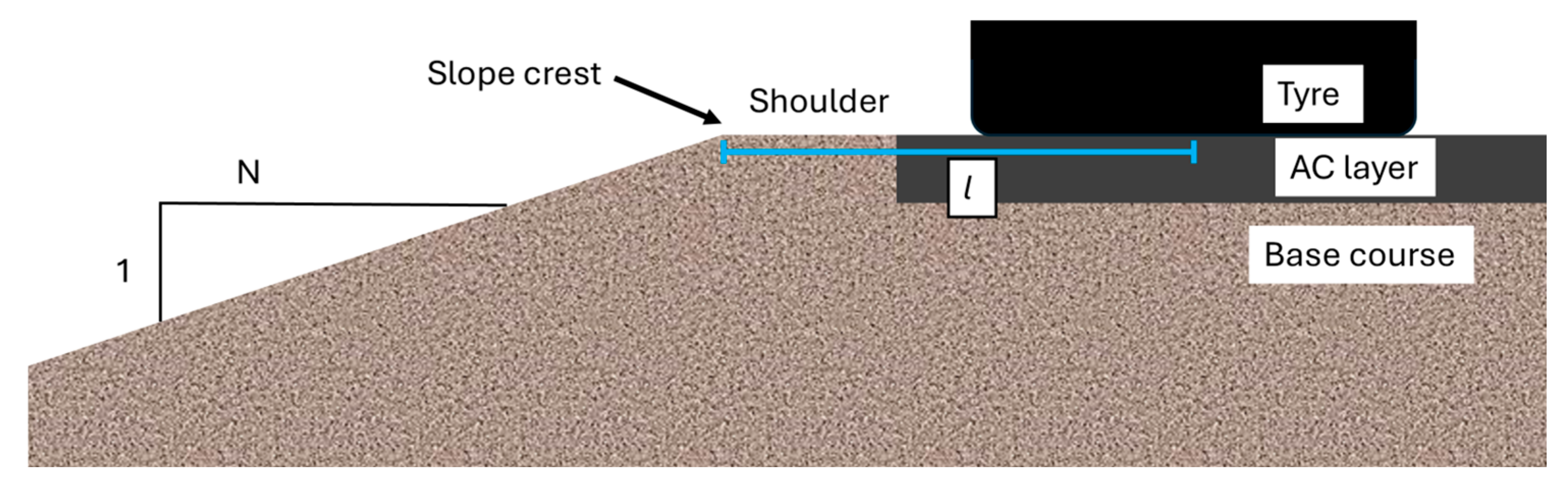
2.1. Selected Cycle Paths
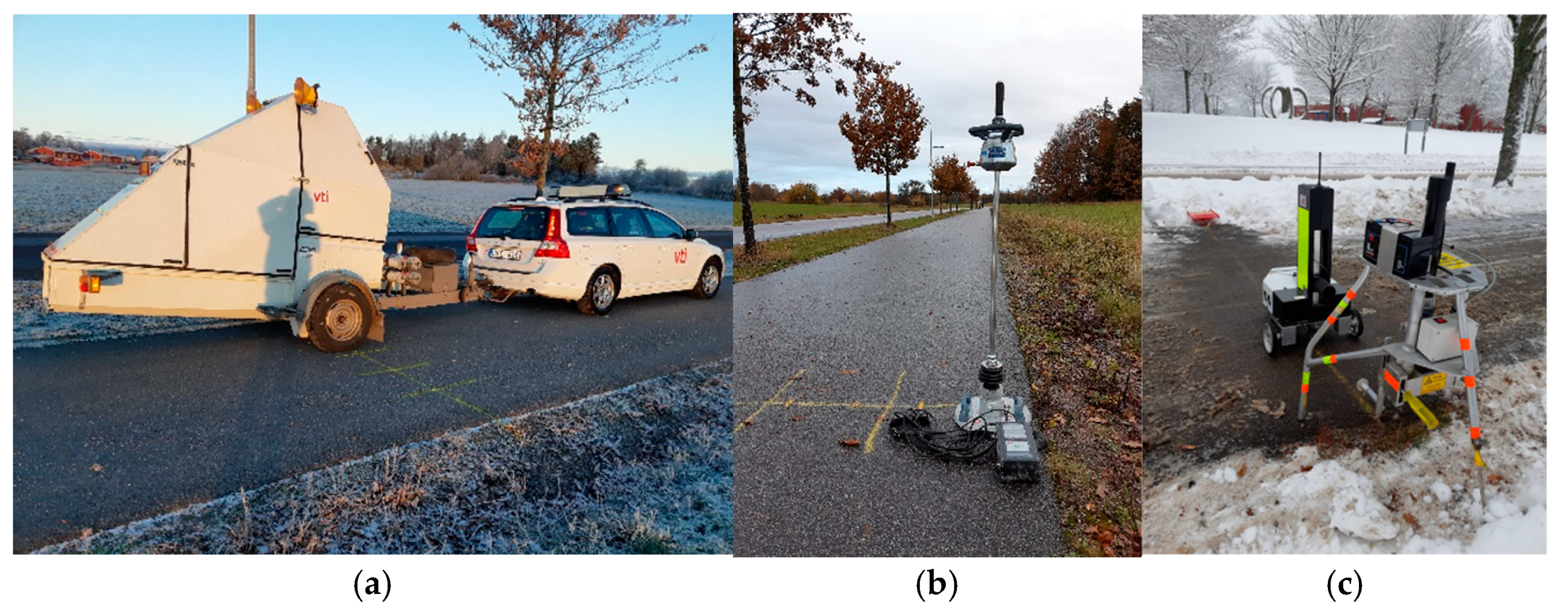
2.2. Equipment
2.3. Measurements and Measuring Procedure
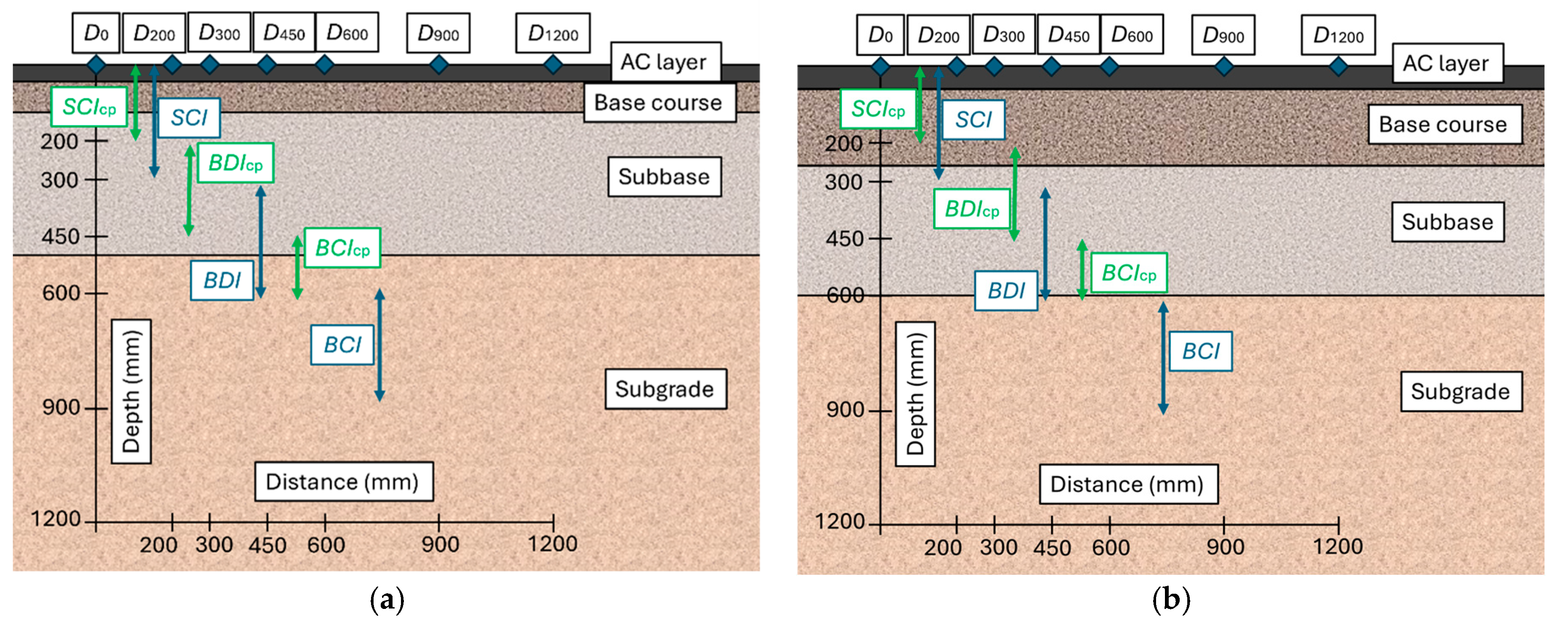
2.4. Data Analysis and Metrics
2.5. Climatic Conditions
3. Results
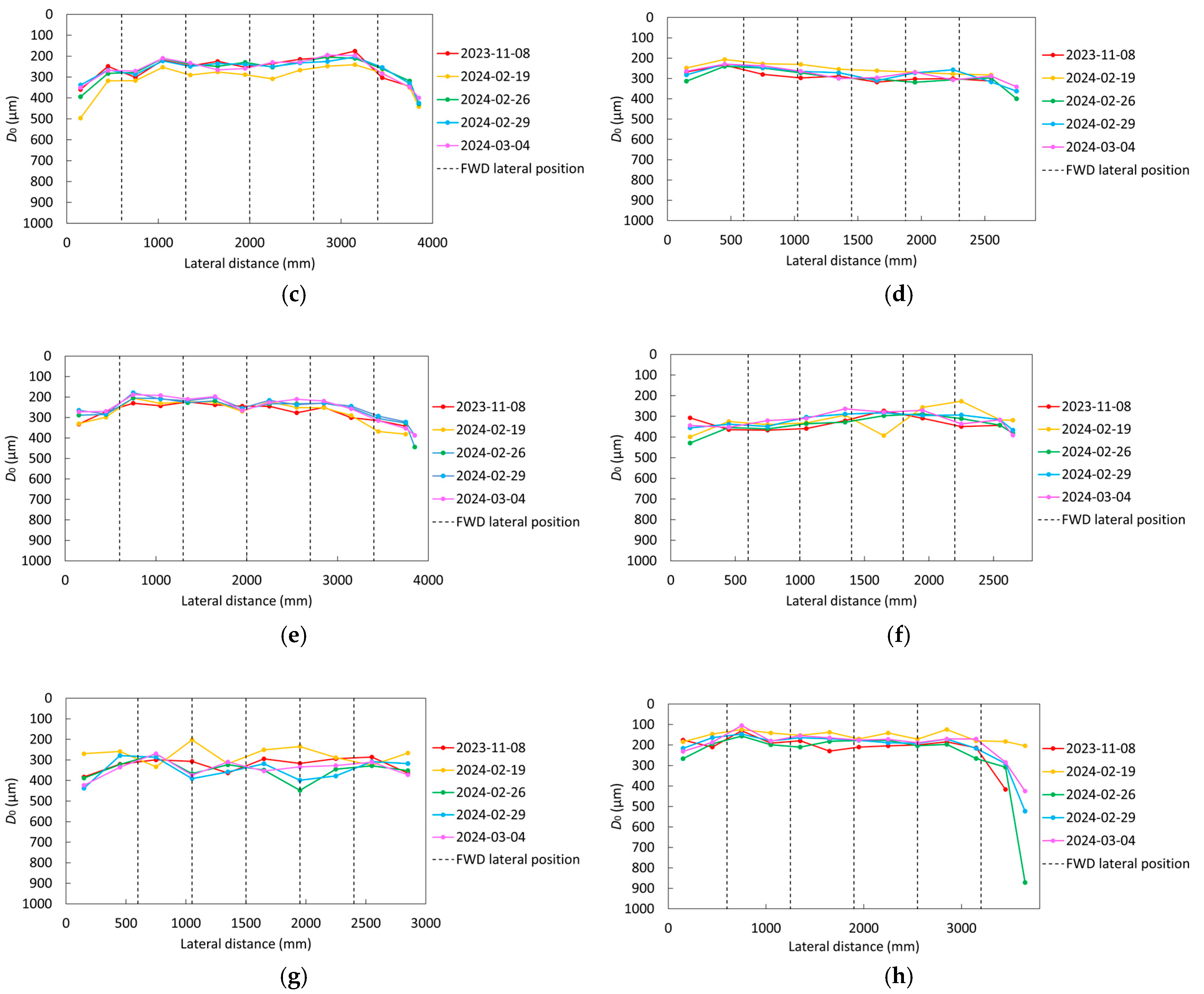
3.1. FWD Measurements
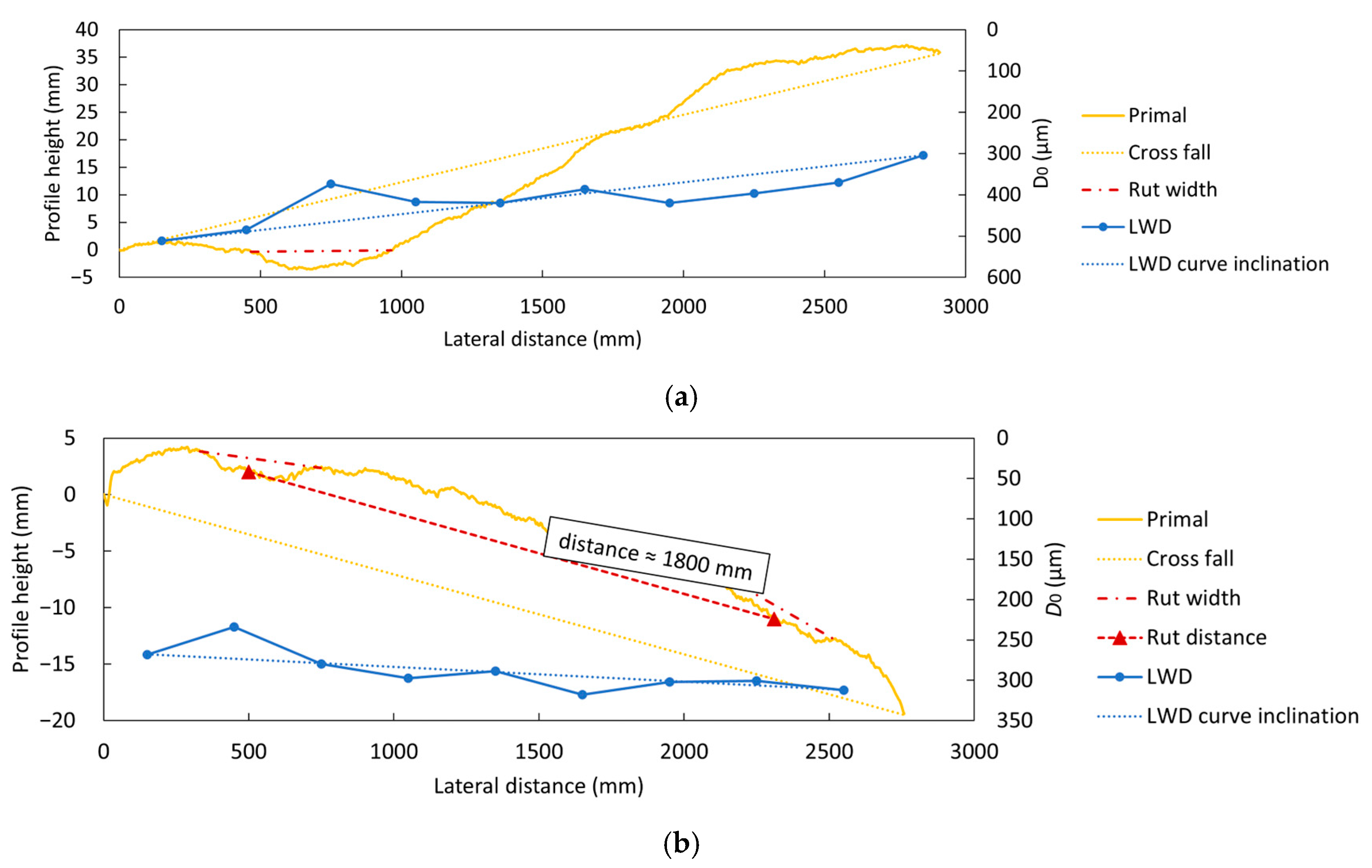
3.2. LWD Measurements
3.3. Transverse Profile Measurements
4. Discussion
4.1. Methods and Data
4.2. Results
5. Conclusions
- The traditional approach of load-bearing capacity evaluations with FWD measurements along the centre line is generally not a viable option for cycle paths.
- LWD measurements are easier to conduct than FWD measurements close to the cycle path edges and are thus a promising tool for the evaluation of load-bearing capacity close to the pavement edge on cycle paths. More studies are however needed to determine the accuracy and repeatability of the LWD measurements.
- Compaction from traffic loads provides better structural stability in the lateral positions between the centre line and the edges.
- Wider cycle paths (>3.8 m), designed with shoulders and sufficient drainage solutions, are important factors for increasing the structural stability of the cycle paths.
- The proposed Deflection Bowl Parameters SCIcp, BDIcp and BCIcp are useful tools to detect weaknesses in different structural parts of the investigated cycle paths.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AC | Asphalt concrete |
| BC | Base course |
| BCI | Base Curvature Index |
| BCIcp | Base Curvature Index cycle path |
| BDI | Base Damage Index |
| BDIcp | Base Damage index cycle path |
| BLI | Base Layer Index |
| CF | Curvature Function |
| D0 | Deflection at the centre of the FWD loading plate |
| DBP | Deflection Bowl Parameters |
| E | Elastic modulus |
| E0 | Surface module |
| Er | Average module |
| FWD | Falling weight deflectometer |
| GEOM | Geometric Factor |
| LLI | Lower Layer Index |
| LWD | Light falling weight deflectometer |
| MLI | Middle Layer Index |
| SCI | Surface Curvature Index |
| SCIcp | Surface Curvature Index cycle path |
| SG | Subgrade |
| SGU | The Geological Survey of Sweden |
| SMHI | Swedish Meteorological and Hydrological Institute |
| TSAP | Thin-surfaced asphalt pavement |
| UGL | Unbound granular layer |
| UGM | Unbound granular material |
References
- Larsson, M.; Niska, A.; Erlingsson, S. Degradation of Cycle Paths—A Survey in Swedish Municipalities. CivilEng 2022, 3, 184–210. [Google Scholar] [CrossRef]
- Ekdahl, P. Bära Eller Brista. Handbok I Tillståndsbedömning Av Belagda Gator Och Vägar; Swedish Association of Local Authorities and Regions: Stockholm, Sweden, 2019. (In Swedish) [Google Scholar]
- Nyberg, P.; Björnstig, U.; Bygren, L.-O. Road characteristics and bicycle accidents. Scand. J. Soc. Med. 1996, 24, 293–301. [Google Scholar] [CrossRef] [PubMed]
- Schepers, P.; Theuwissen, E.; Velasco, P.N.; Niaki, M.N.; van Boggelen, O.; Daamen, W.; Hagenzieker, M. The relationship between cycle track width and the lateral position of cyclists, and implications for the required cycle track width. J. Saf. Res. 2023, 87, 38–53. [Google Scholar] [CrossRef] [PubMed]
- Botma, H. Method to Determine Level of Service for Bicycle Paths and Pedestrian-Bicycle Paths. Transp. Res. Rec. 1995, 1502, 38–44. [Google Scholar]
- Wierbos, M.J.; Knoop, V.L.; Hänseler, F.S.; Hogendoorn, S.P. Capacity, Capacity Drop, and Relation of Capacity to the Path Width in Bicycle Traffic. Transp. Res. Rec. 2019, 2673, 693–702. [Google Scholar] [CrossRef]
- Thom, N. Principles of Pavement Engineering, 2nd ed.; Emerald Publishing Limited: London, UK, 2014. [Google Scholar]
- Charlier, R.; Hornych, P.; Srsen, M.; Hermansson, Å.; Bjarnason, G.; Erlingsson, S.; Pavsic, P. Water Influence on Bearing Capacity and Pavement Performance: Field Observations. In Water in Road Structures. Movement, Drainage and Effects, 1st ed.; Dawson, A., Ed.; Springer: Dordrecht, The Netherlands, 2009; Volume 5, pp. 175–192. [Google Scholar] [CrossRef]
- Aksnes, J. A Study of Load Responses Towards the Pavement Edge. Ph.D. Thesis, Norwegian University of Science and Technology, Trondheim, Norway, August 2002. [Google Scholar]
- Dablanc, L.; Beziat, A. Parking for Freight Vehicles in Dense Urban Centers—The Issue of Delivery Areas in Paris; MetroFreight Center of Excellence: Marne la Vallee, France, 2015. [Google Scholar]
- Korkiala-Tanttu, L. Calculation Method for Permanent Deformation of Unbound Pavement Materials. Ph.D. Thesis, Helsinki University of Technology, Helsinki, Finland, 30 January 2009. [Google Scholar]
- Bianchini, A. Thin asphalt pavement performance equation through direct computation of falling weight deflectometer-derived strains. Int. J. Pavement Eng. 2014, 15, 831–839. [Google Scholar] [CrossRef]
- Van Gurp, C. Use of Falling Weight Deflectometers in Pavement Evaluation. In Final Report of the Action; COST: Brussels, Belgium, 2005. [Google Scholar]
- Salour, F. Utvärdering Av Vägkonstruktioners Bärighet Med Fallviktsapparat; Swedish Road Administration: Borlänge, Sweden, 2020. (In Swedish) [Google Scholar]
- Martínez Arguelles, G.; Fuentes, L.G.; Torregroza Aldana, L.M. Review of the pavement management system of the Bogotá bike-path network. Rev. Ing. Construcción 2011, 26, 150–170. [Google Scholar] [CrossRef]
- Vavrova, M.; Chang, C.M. Incorporating Livability into Transportation Asset Management Practices through Bikeway Quality Networks. Transp. Res. Rec. 2019, 2673, 407–414. [Google Scholar] [CrossRef]
- Fladvad, M.; Karlsson, H. Dimensjonering Av Gang-Og Sykkelvegløsninger. Vurdering Av Krav I N200 Vegbygging; SINTEF: Trondheim, Norway, 2022. (In Norwegian) [Google Scholar]
- Ordaz, M.; Doyle, J.D.; Howard, I.L. Light Weight Deflectometer Evaluation of Low-Volume Road Structural Deterioration under Rapidly Increased Traffic Patterns. Transp. Res. Rec. 2024, 2678, 948–966. [Google Scholar] [CrossRef]
- Kestler, M.A.; Humphrey, D.N.; Steinert, B.C. Portable Falling-Weight Deflectometers for Tracking Seasonal Stiffness Variations in Asphalt-Surfaced Roads. In Proceedings of the Transportation Research Board 85th Annual Meeting, Washington, DC, USA, 22–26 January 2006. [Google Scholar]
- Horak, E.; Maina, J.; Guiamba, D.; Hartman, A. Correlation Study with the Light Weight Deflectometer in South Africa. In Proceedings of the 27th Southern African Transport Conference, Pretoria, South Africa, 7–11 July 2008. [Google Scholar]
- Sawangsuriya, A. Correlation between LWD and FWD deflections for asphalt pavement. In Proceedings of the 11th International Conference on the Bearing Capacity of Roads, Railways and Airfields, Trondheim, Norway, 28–30 June 2022. [Google Scholar]
- FEHRL. VTI Primal Longitudinal Profile Instrument. Available online: https://www.fehrl.org/facilities/detail/111 (accessed on 25 September 2024).
- TRV. Krav—VGU, Vägars Och Gators Utformning (Requirements—VGU, Design of Roads and Streets); Swedish Transport Administration: Borlänge, Sweden, 2022. (In Swedish) [Google Scholar]
- Karakas, A.S. Aging Effects on Mechanical Characteristics of Multi-Layer Asphalt Structure. In Modified Asphalt, 1st ed.; Rivera Armenta, J.L., Salazar-Cruz, B.A., Eds.; IntechOpen: London, UK, 2018. [Google Scholar]
- Blab, R.; Litzka, J. Measurements of the Lateral Distribution of Heavy Vehicles and Its Effect on the Design of Road Pavements. In Proceedings of the 4th International Symposium on Heavy Vehicle Weights and Dimensions, Ann Arbor, MI, USA, 25–29 June 1995. [Google Scholar]
- Arraigada, M.; Partl, M. Pavement Distress from Channelized and Lateral Wandering Loads Using Accelerated Pavement Tests. In Proceedings of the 9th International Conference on Maintenance and Rehabilitation of Pavements—Mairepav9, Dübendorf, Switzerland, 1–3 June 2020. [Google Scholar]
- Sinamnis, R.; Woods, L. Traffic channelisation and pavement deterioration: An investigation of the role of lateral wander on asphalt pavement rutting. Int. J. Pavement Eng. 2023, 24, 2118272. [Google Scholar] [CrossRef]
- Mallick, R.B.; El-Korchi, T. Pavement Engineering. Principles and Practice, 2nd ed.; CRC Press: Boca Ratón, FL, USA, 2013. [Google Scholar]
- Niska, A.; Blomqvist, G. Sweep-salting—A method for winter maintenance of bicycle paths. In Proceedings of the 15th International Winter Road Congress, Gdansk, Poland, 20–23 February 2018. [Google Scholar]
- Karlsson, H.; Rise, T. Effekt Av Salt Og Teleskader På Gang-Og Sykkelveger, En Litteraturstudie; Sintef: Trondheim, Norway, 2019. (In Norwegian) [Google Scholar]
- Howard, I.L. Preservation of Low-Volume Flexible Pavement Structural Capacity by Use of Seal Treatments. Transp. Res. Rec. 2011, 2204, 45–53. [Google Scholar] [CrossRef]
- Martin, T.; Choummanivong, L.; Toole, T. New pavement deterioration models for sealed low volume roads in Australia. In Proceedings of the 8th International Conference on Managing Pavement Assets, Santiago, Chile, 15–19 November 2019. [Google Scholar]
- TRV. Överbyggnad Väg, Dimensionering Och Utformning (Design of Road Super Structures); Swedish Transport Administration: Borlänge, Sweden, 2023. (In Swedish) [Google Scholar]
- Horak, E.; Emery, S. Falling Weight Deflectometer Bowl Parameters as Analysis Tool for Pavement Structural Evaluations. In Proceedings of the 22nd ARRB Conference: Research into Practice, Canberra, Australia, 29 October–2 November 2006. [Google Scholar]
- Cekerevac, C.; Baltzer, S.; Charlier, R.; Chazallon, C.; Erlingsson, S.; Gajewska, B.; Hornych, P.; Kraszewski, C.; Pavisc, P. Water Influence on Mechanical Behaviour of Pavements: Experimental Investigation. In Water in Road Structures. Movement, Drainage and Effects, 1st ed.; Dawson, A., Ed.; Springer: Dordrecht, The Netherlands, 2009; Volume 5, pp. 217–242. [Google Scholar] [CrossRef]
- Horak, E.; Maree, J.; van Wijk, A. Procedures for Using Impulse Deflectometer (IDM) Measurements in the Structural Evaluation of Pavements. In Annual Transportation Convention; South African Council for Scientific and Industrial Research, Division of Roads and Transport Technology: Pretoria, South Africa, 1989. [Google Scholar]
- Korkiala-Tanttu, L.; Laaksonen, R. Permanent Deformations of Unbound Materials of Road Pavement in Accelerated Pavement tests. In Proceedings of the 13th European Conference on Soil Mechanics and Geotechnical Engineering, Prague, Czech Republic, 25–28 August 2003. [Google Scholar]
- Maree, J.H.; Bellekens, R.J.L. The Effect of Asphalt Overlays on the Resilient Deflection Bowl Response of Typical Pavement Structures; Department of Transport, Chief Directorate National Roads: Pretoria, South Africa, 1991. [Google Scholar]
- Department of Transport and Main Roads. Pavement Rehabilitation Manual; The State of Queensland: Brisbane, Australia, 2020. [Google Scholar]
- Korkiala-Tanttu, L.; Dawson, A. Effects of side slope on low-volume road pavement performance: A full-scale assessment. Can. Geotech. J. 2008, 45, 853–866. [Google Scholar] [CrossRef]
- Doré, G.; Zubeck, H.K. Cold Regions Pavement Engineering, 1st ed.; ASCE: Reston, VA, USA, 2009. [Google Scholar]
- Leischner, S.; Wellner, F.; Canon Falla, G.; Oeser, M.; Wang, D. Design of Thin Surfaced Asphalt Pavements. In Proceedings of the 3rd International Conference on Transportation Geotechnics, Guimarães, Portugal, 4–7 September 2016. [Google Scholar]
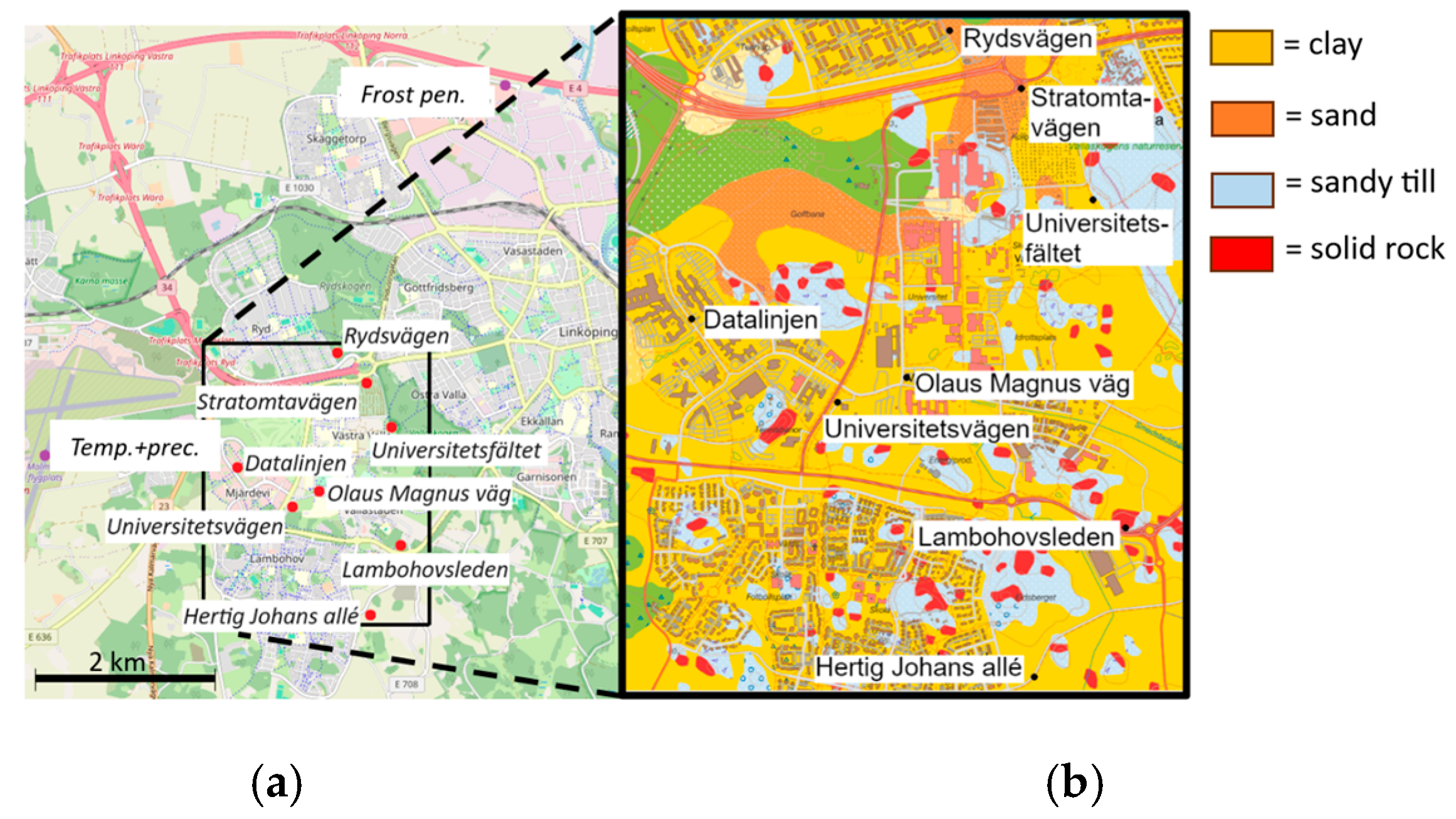



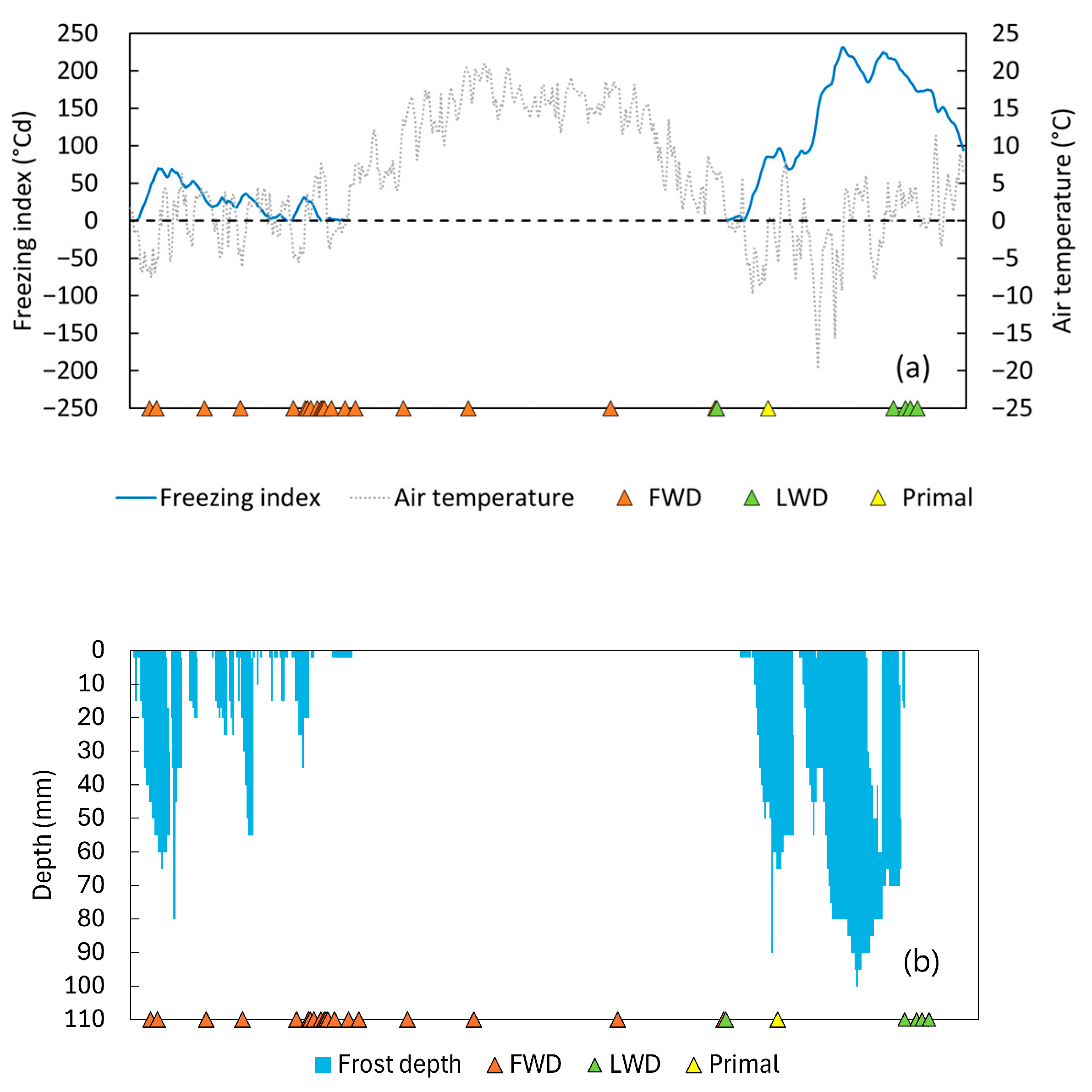

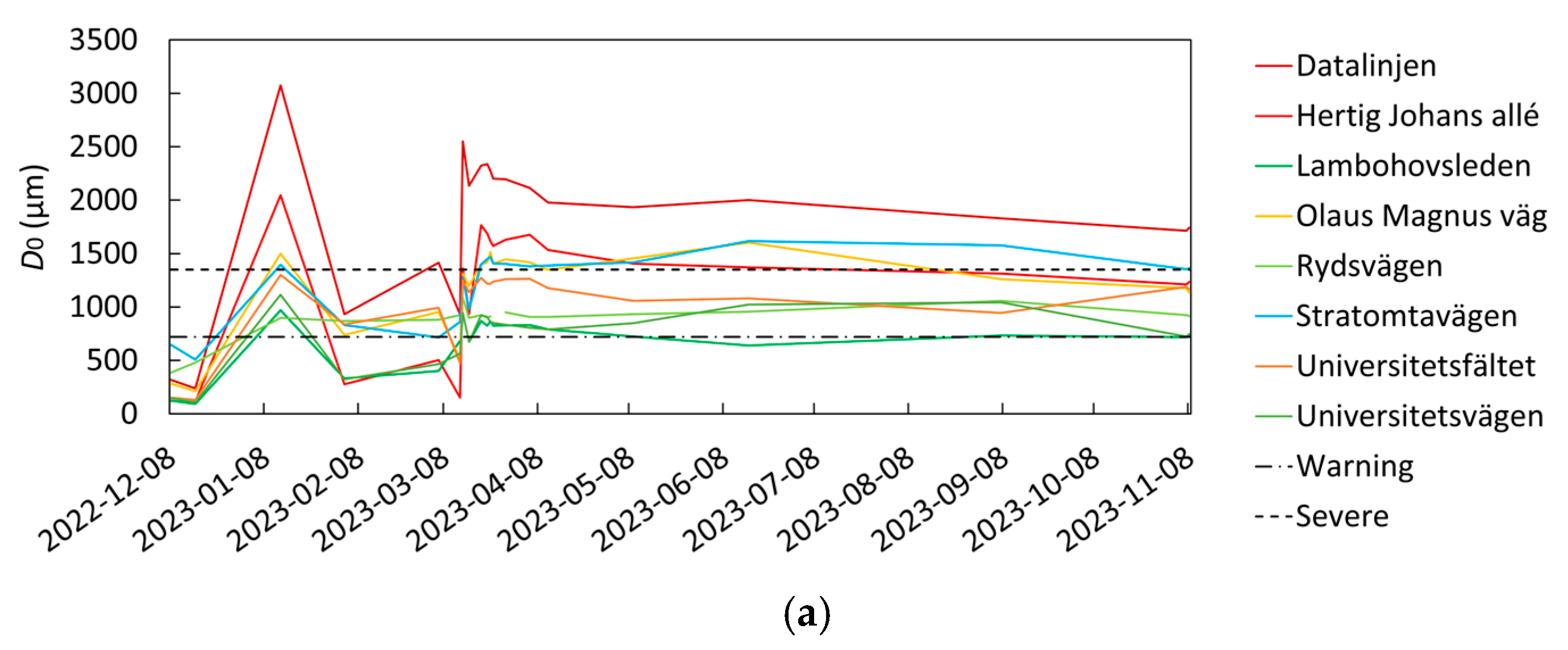
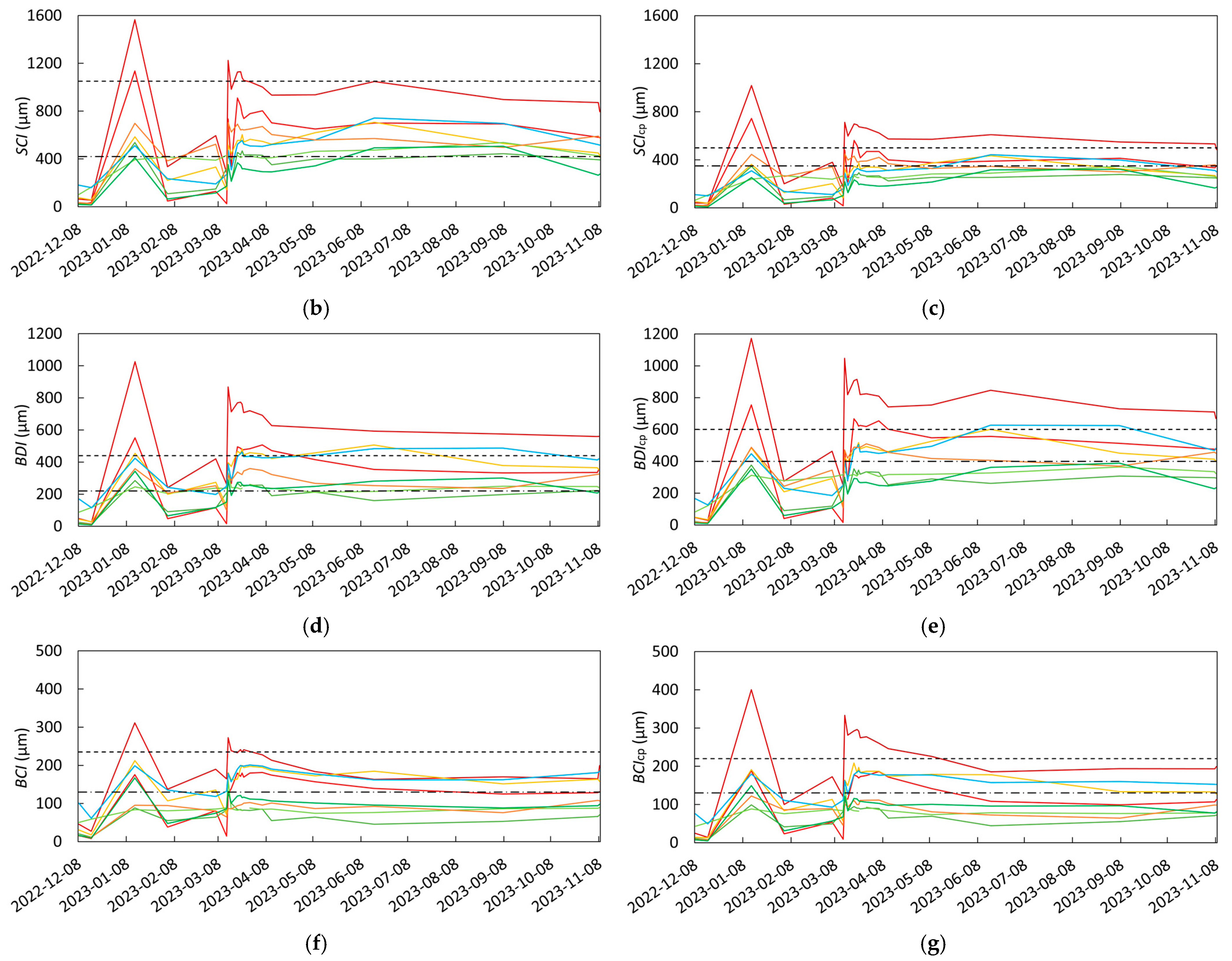


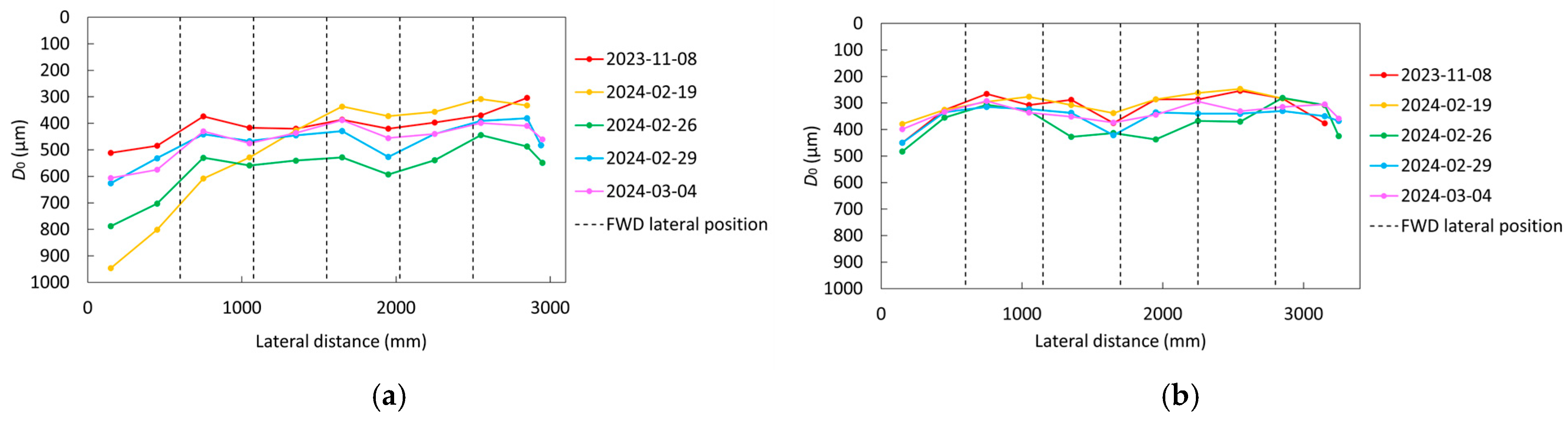

| Cycle Path | Datalinjen | Hertig Johans Allé | Lambohovsleden | Olaus Magnus Väg | Rydsvägen | Stratomtavägen | Universitetsfältet | Universitetsvägen |
|---|---|---|---|---|---|---|---|---|
| Age (years) | 27 | 9 | 8 | 28 | 3 | 28 | 24 | 21 |
| Width (m) | 3.1 | 3.4 | 4 | 2.9 | 4 | 2.8 | 3 | 3.8 |
| Wearing course (mm) | 30 | 40 | 40 | 30 | 45 | 20 | 30 | 35 |
| Bound base course (mm) | - | - | - | - | - | 50 | - | - |
| Base course (mm) | 120 | 120 | 80 | 120 | 80 | 130 | 120 | 120 |
| Subbase (mm) | 250 | 340 | 340 | 250 | 375 | 100 | 350 | 390 |
| Total thickness (mm) | 400 | 500 | 460 | 400 | 500 | 300 | 500 | 545 |
| Mix, wearing course 1 | 80 MAB 8 T | ABT11 | ABT11 160/220 | 80 MAB 12 T | ABT11 160/220 | 80 MAB 8 T | ABT11 180 | - |
| Mix, base course 2 | - | - | - | - | - | AG | - | - |
| Material, base course 3 | Natural gravel | Crushed rock | Crushed rock | Natural gravel | Crushed rock | Natural gravel | Crushed rock | Natural gravel |
| Material, subbase 4 | Natural gravel | Crushed rock | Crushed rock | Natural gravel | Crushed rock | Natural gravel | Crushed rock | Natural gravel |
| Soil type | Clay | Clay | Rock, sandy till | Clay | Fine sand | Fine sand | Clay | Clay |
| Permeability | Low | Low | Medium | Low | High | High | Low | Low |
| Drainage pipe Ø (mm) 5 | - | 110 | 2 × 110 | - | 110 | - | - | 110 |
| Cross fall (%) | 1.0 | 3.6 | 2.3 | 1.2 | 1.3 | 3.0 | 0.4 | 1.7 |
| Side slope inclination | - | 1:10 | 1:3 | - | 1:3 | 1:5 | 1:6 | 1:2 |
| Sweep-salted | Yes | No | Yes | No | Yes | Yes | No | Yes |
| Structural Condition Rating | Deflection Bowl Parameter Limit Values Horak and Emery, 2006 [34] | |||
|---|---|---|---|---|
| D0 (µm) | BLI (µm) | MLI (µm) | LLI (µm) | |
| Sound | <400 | <200 | <100 | <55 |
| Warning | 400–750 | 200–500 | 100–200 | 55–100 |
| Severe | >750 | >500 | >200 | >100 |
| Structural Condition Rating | Estimated Deflection Bowl Parameter Limit Values TRV, 2023 [33] | |||
| D0 (µm) | SCI (µm) | BDI (µm) | BCI (µm) | |
| Sound | <720 | <420 | <220 | <130 |
| Warning | 720–1350 | 420–1050 | 220–440 | 130–235 |
| Severe | >1350 | >1050 | >440 | >235 |
| Structural Condition Rating | Deflection Bowl Parameters (Based on FWD Data and Visual Inspection) | ||
|---|---|---|---|
| SCIcp (µm) | BDIcp (µm) | BCIcp (µm) | |
| Sound | <350 | <400 | <130 |
| Warning | 350–500 | 400–600 | 130–220 |
| Severe | >500 | >600 | >220 |
| Normal Conditions (7–11 °C) | ||||
|---|---|---|---|---|
| Cycle Path | Minimum Er (MPa) | Position with Minimum Er | Affected Structural Layer | Best Fit DBP |
| Datalinjen | 49 | D300 | SB | BDIcp |
| Hertig Johans allé | 71 | D300 | SB | BDIcp |
| Lambohovsleden | 138 | D300 | SB | BDIcp |
| Olaus Magnus väg | 62 | D600 | SG | BCIcp |
| Rydsvägen | 86 | D600 | SG | BCI |
| Stratomtavägen | 52 | D600 | SG | BCIcp |
| Universitetsfältet | 78 | D300 | SB | BDIcp |
| Universitetsvägen | 86 | D600 | SG | BCIcp |
| Spring Thaw Conditions | ||||
| Cycle Path | Minimum Er (MPa) | Position with Minimum Er | Affected Structural Layer | Best Fit DBP |
| Datalinjen | 31 | D300 | SB | BDIcp |
| Hertig Johans allé | 51 | D300 | SB | BDIcp |
| Lambohovsleden | 101 | D300 | SB | BDIcp |
| Olaus Magnus väg | 48 | D450 | SG | BCIcp |
| Rydsvägen | 64 | D450 | SB | BCIcp |
| Stratomtavägen | 49 | D450 | SG | BCIcp |
| Universitetsfältet | 77 | D300 | SB | BDIcp |
| Universitetsvägen | 61 | D450 | SB | BCIcp |
| Hot Pavement Temperature (>25°C) | ||||
| Cycle Path | Minimum Er (MPa) | Position with Minimum Er | Affected Structural Layer | Best Fit DBP |
| Datalinjen | 49 | D300 | SB | BDIcp |
| Hertig Johans allé | 69 | D300 | SB | BDIcp |
| Lambohovsleden | 154 | D200 | SB | SCI |
| Olaus Magnus väg | 52 | D300 | SB | BDIcp |
| Rydsvägen | 75 | D900 | SG | BCI |
| Stratomtavägen | 53 | D300 | SG | BDIcp |
| Universitetsfältet | 87 | D600 | SG | BCI |
| Universitetsvägen | 86 | D300 | SB | BDIcp |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Larsson, M.; Niska, A.; Erlingsson, S. Structural Stability of Cycle Paths—Introducing Cycle Path Deflection Bowl Parameters from FWD Measurements. Infrastructures 2025, 10, 7. https://doi.org/10.3390/infrastructures10010007
Larsson M, Niska A, Erlingsson S. Structural Stability of Cycle Paths—Introducing Cycle Path Deflection Bowl Parameters from FWD Measurements. Infrastructures. 2025; 10(1):7. https://doi.org/10.3390/infrastructures10010007
Chicago/Turabian StyleLarsson, Martin, Anna Niska, and Sigurdur Erlingsson. 2025. "Structural Stability of Cycle Paths—Introducing Cycle Path Deflection Bowl Parameters from FWD Measurements" Infrastructures 10, no. 1: 7. https://doi.org/10.3390/infrastructures10010007
APA StyleLarsson, M., Niska, A., & Erlingsson, S. (2025). Structural Stability of Cycle Paths—Introducing Cycle Path Deflection Bowl Parameters from FWD Measurements. Infrastructures, 10(1), 7. https://doi.org/10.3390/infrastructures10010007






