A Structural and Thermal Comparative Review of 3D-Printed Wall Shapes
Abstract
1. Introduction
2. Literature Review
2.1. Background of the 3DCP Industry
2.2. State of 3DCP Technology
2.3. Knowledge Gap in the 3DCP Industry
3. Study Setup in SolidWorks
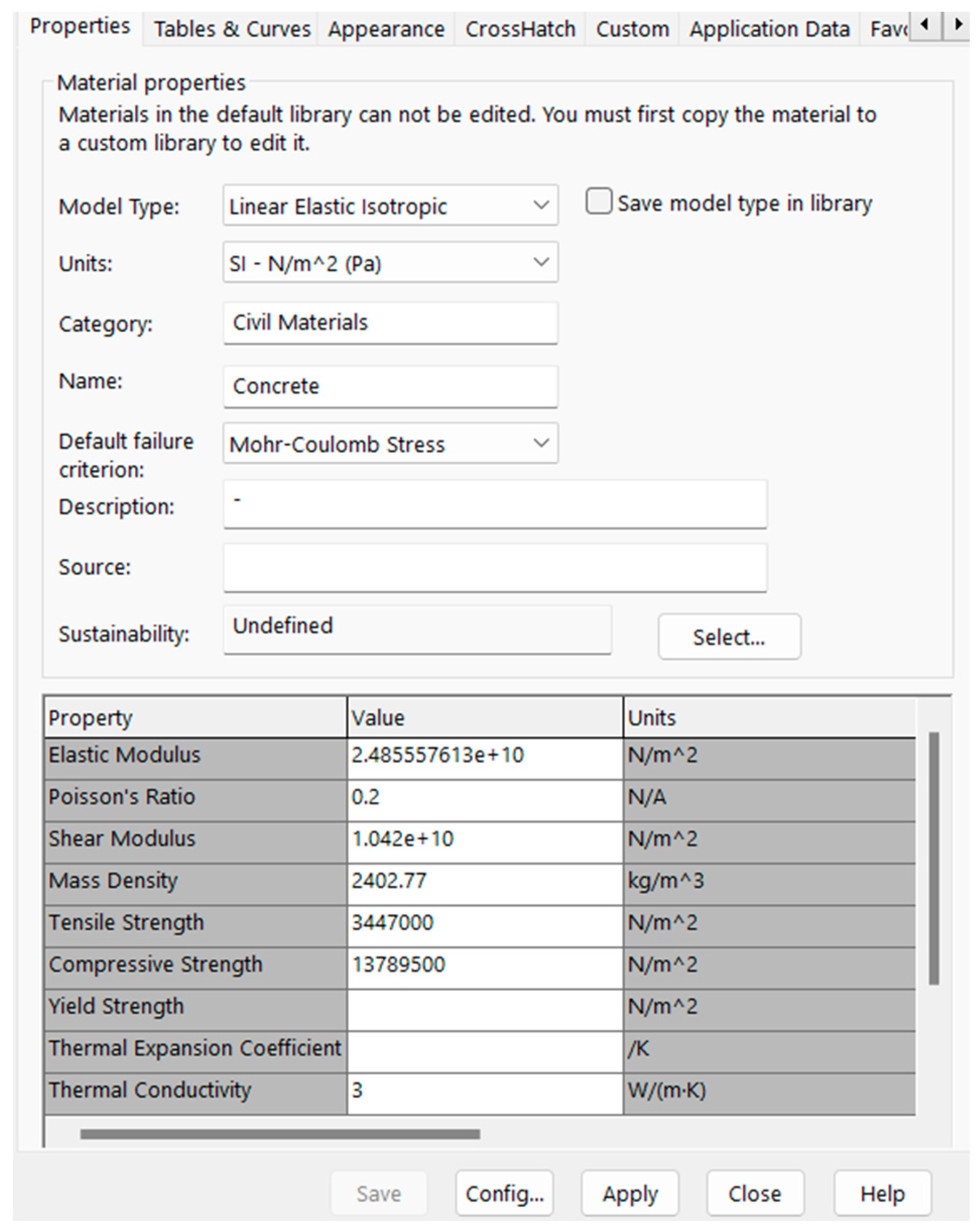
3.1. Structural Study Setup in SolidWorks
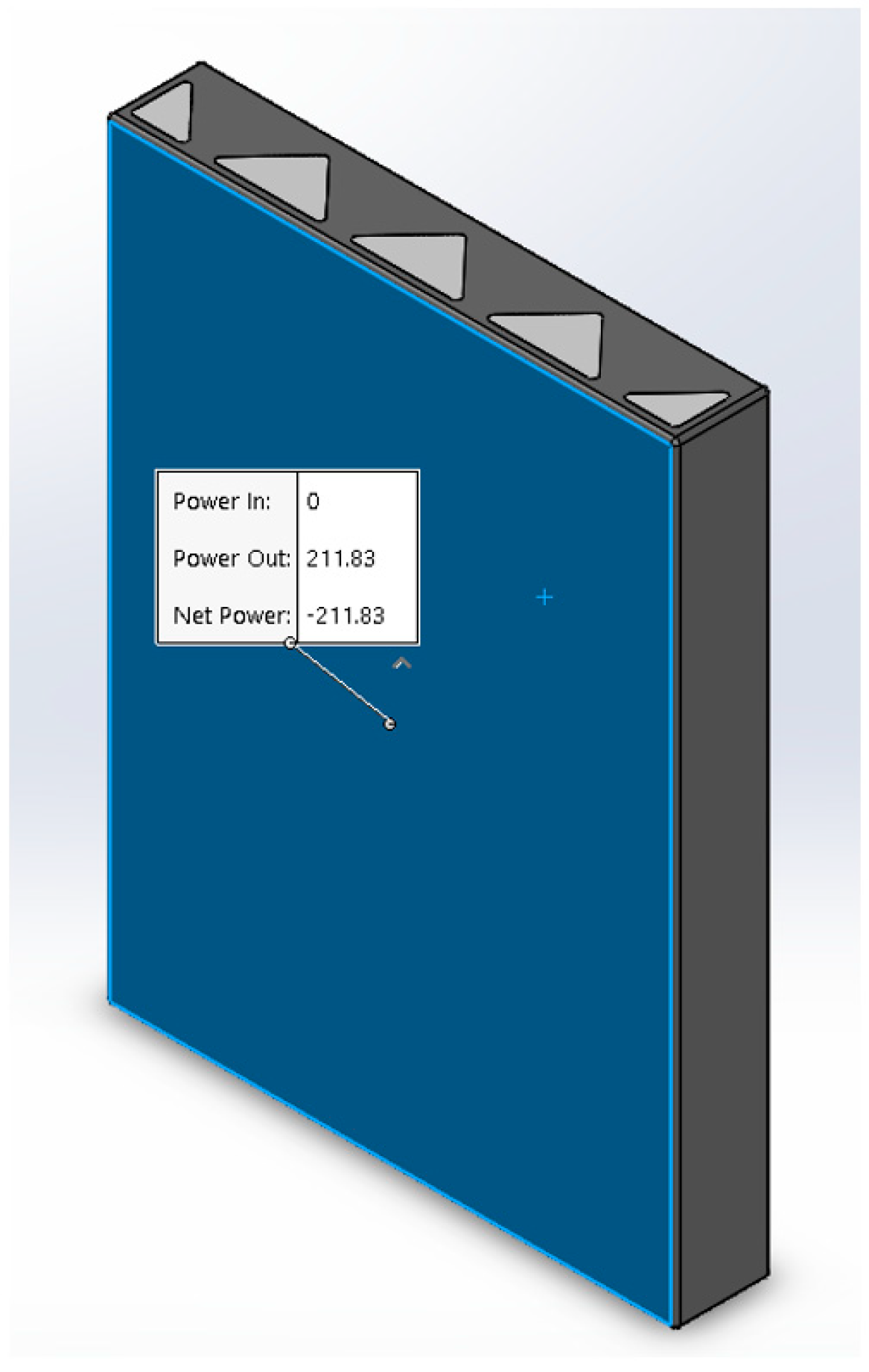
3.2. Thermal Study Setup in SolidWorks
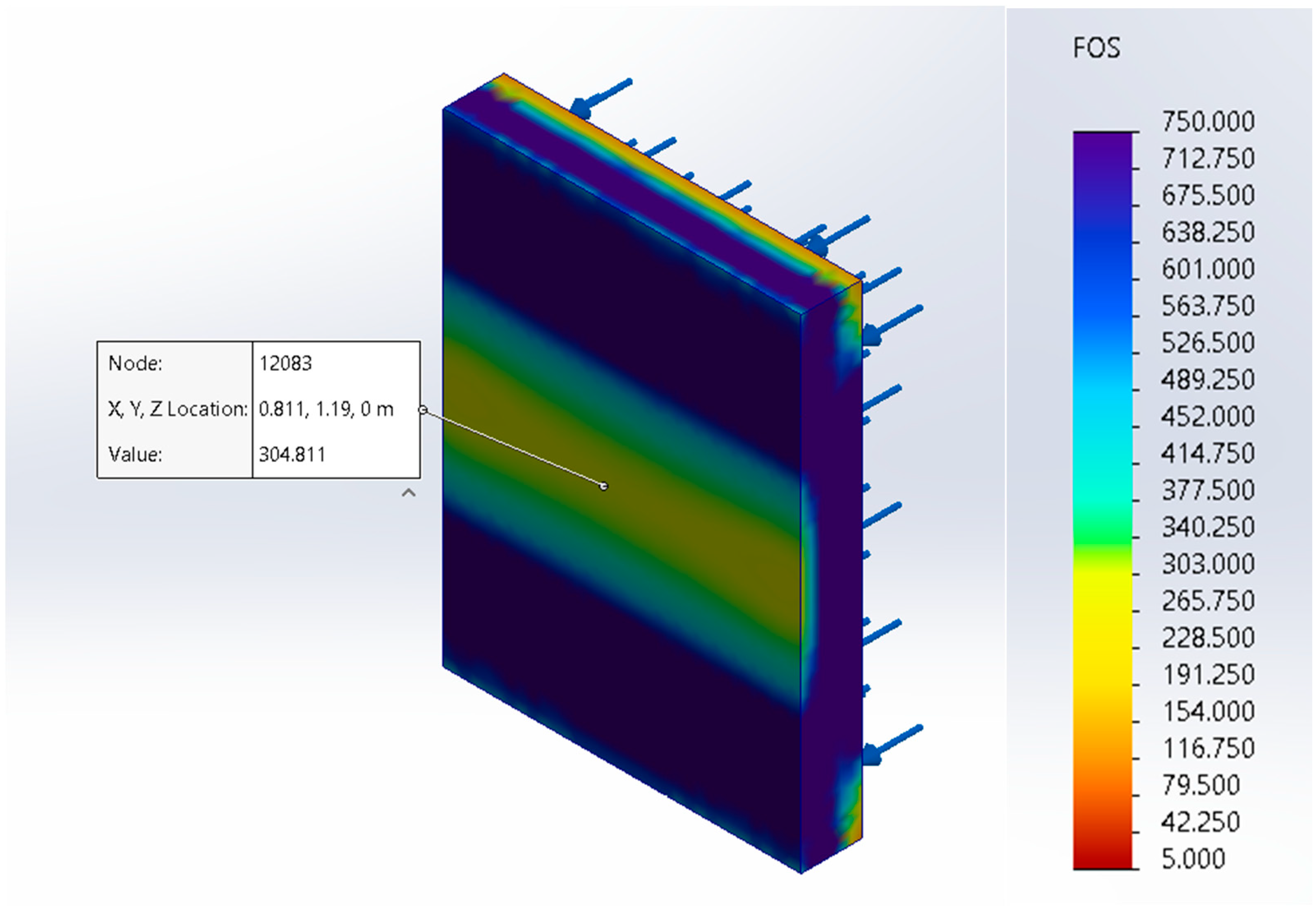
4. Solid Concrete Wall Structural Study
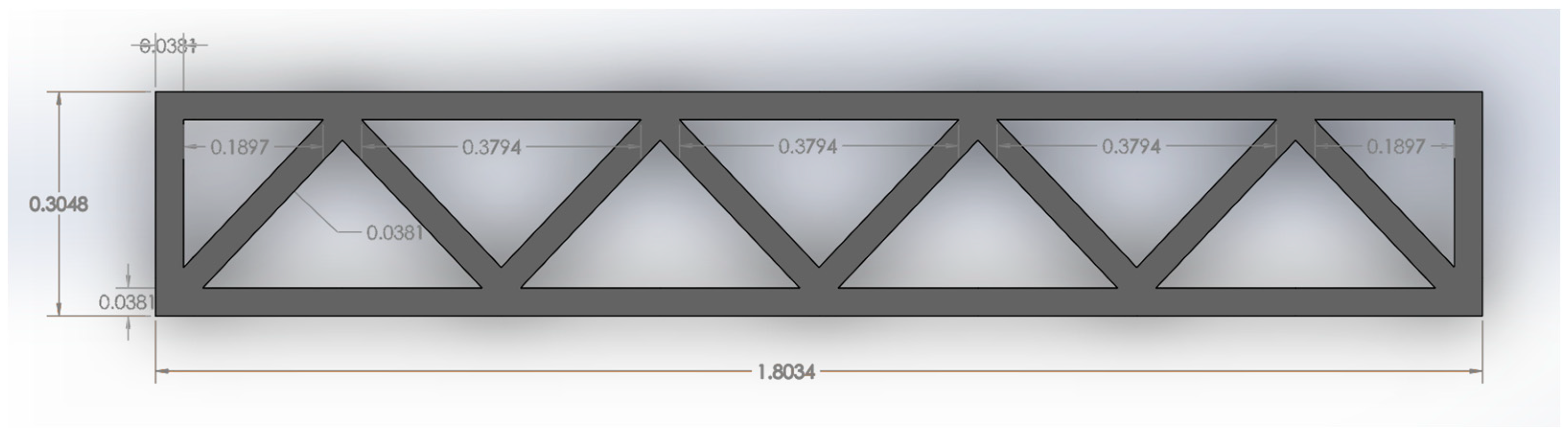
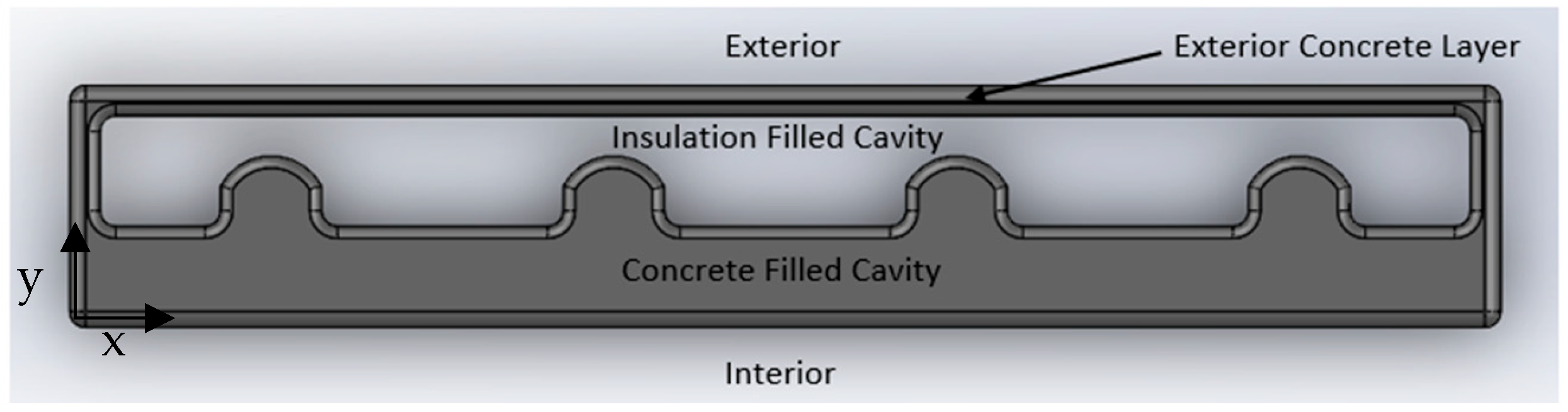
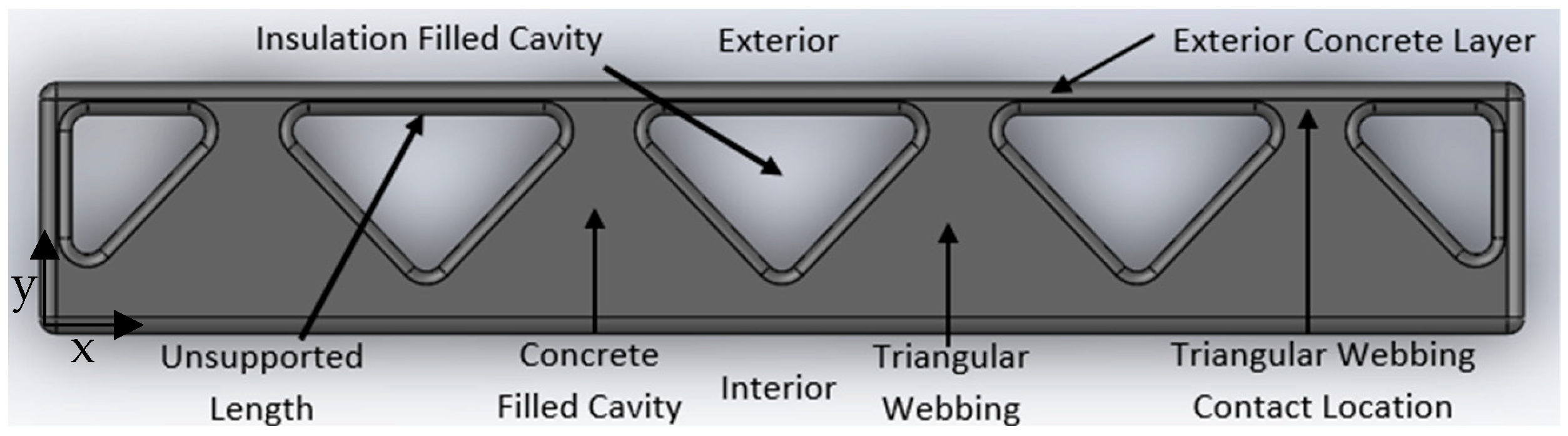
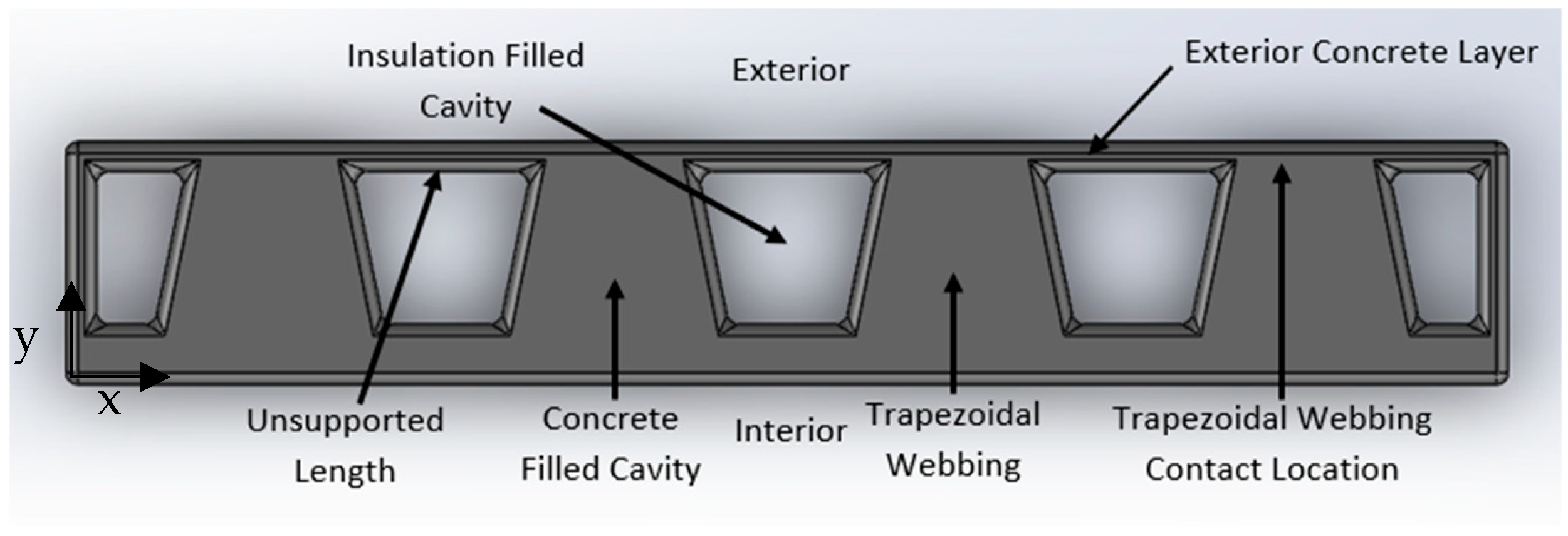
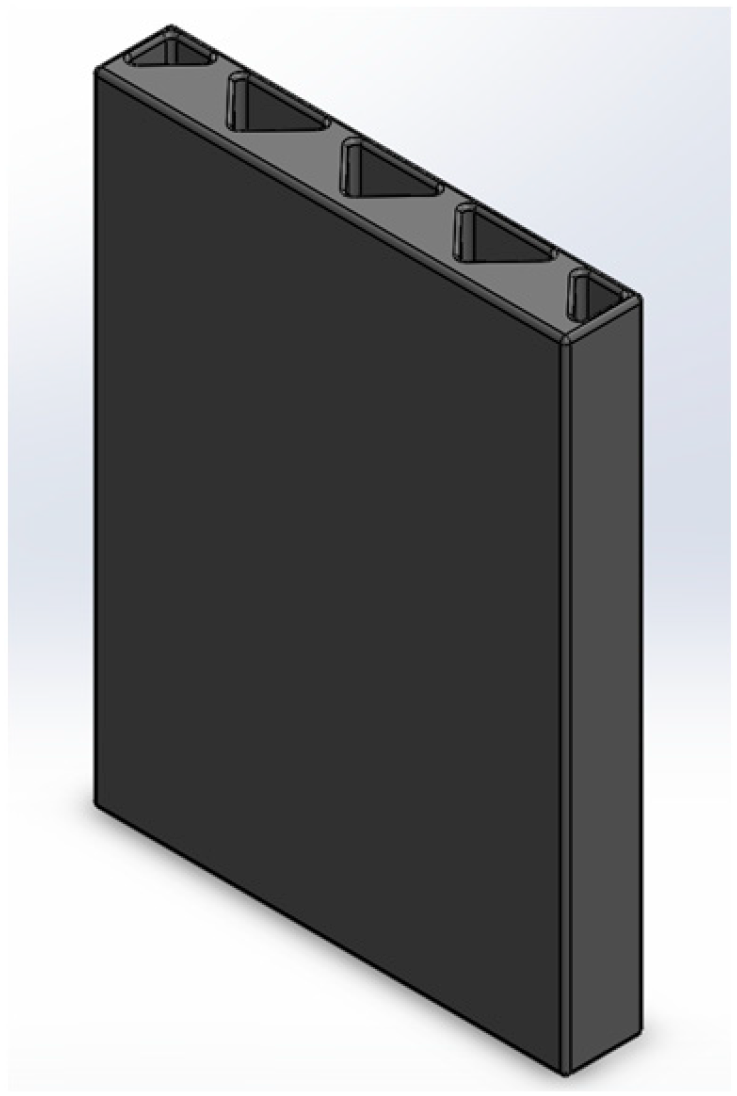
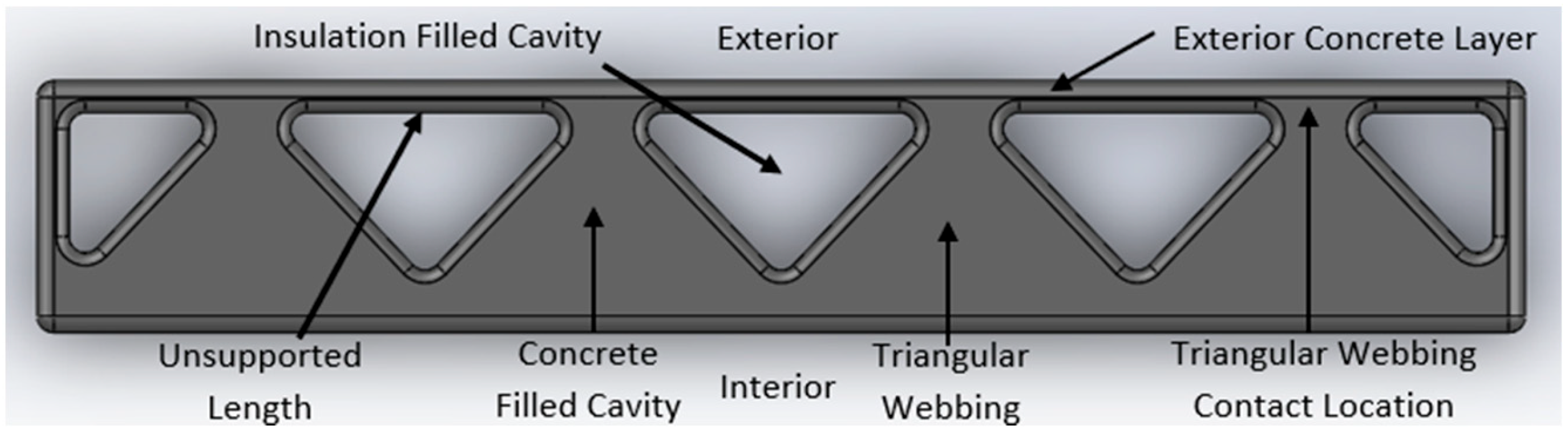
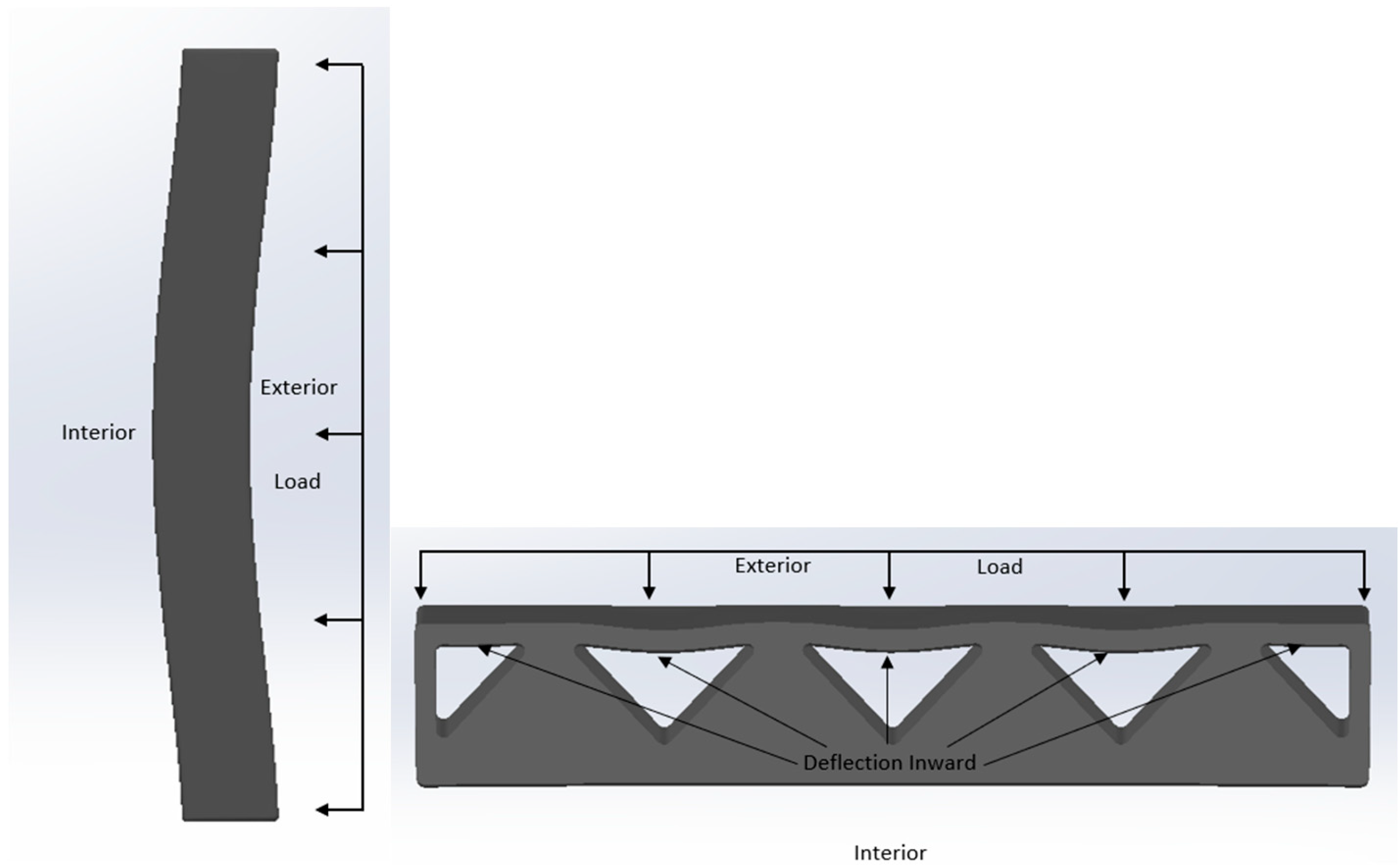
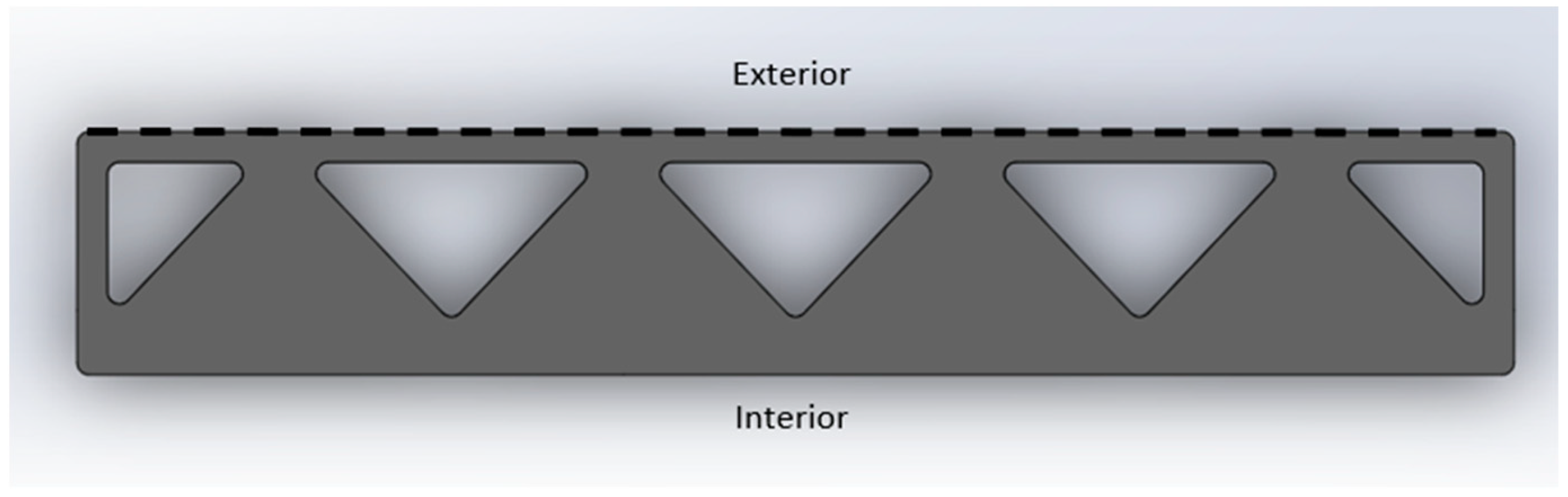
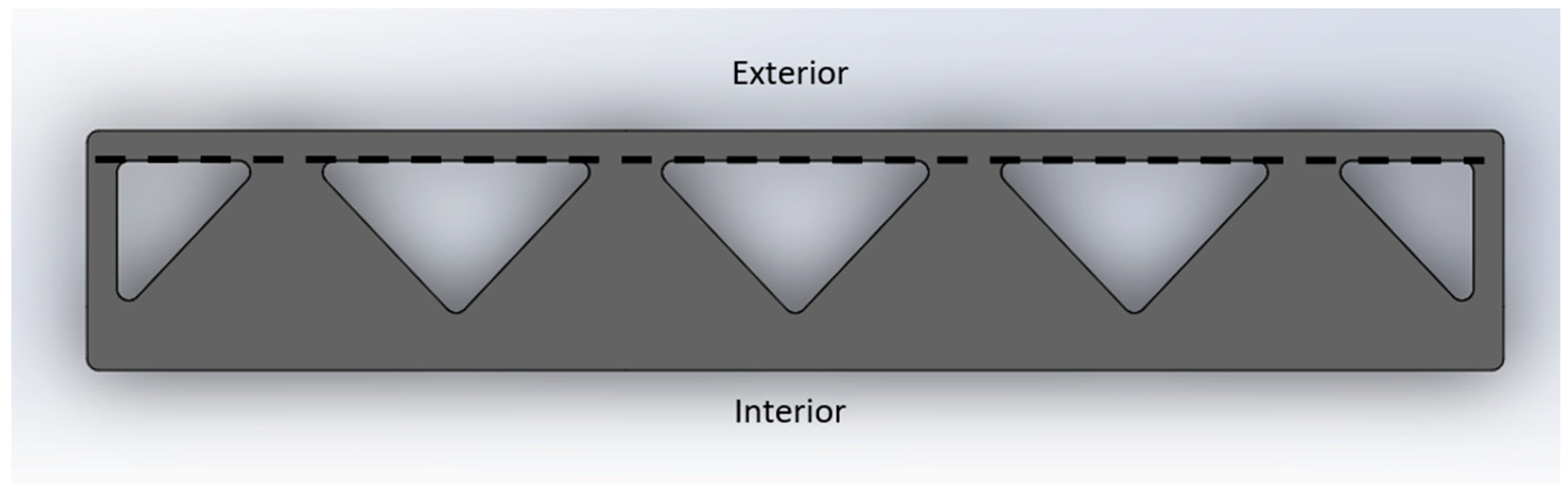
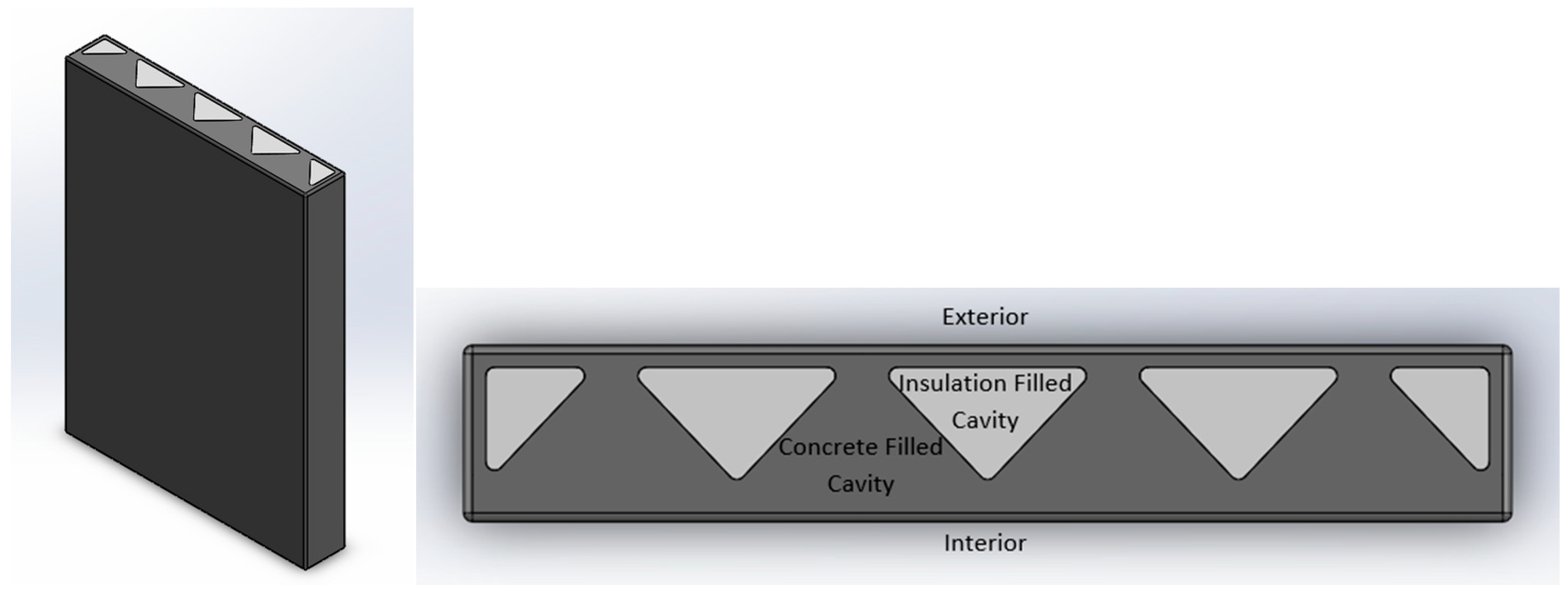
5. Zigzag Cavity Wall
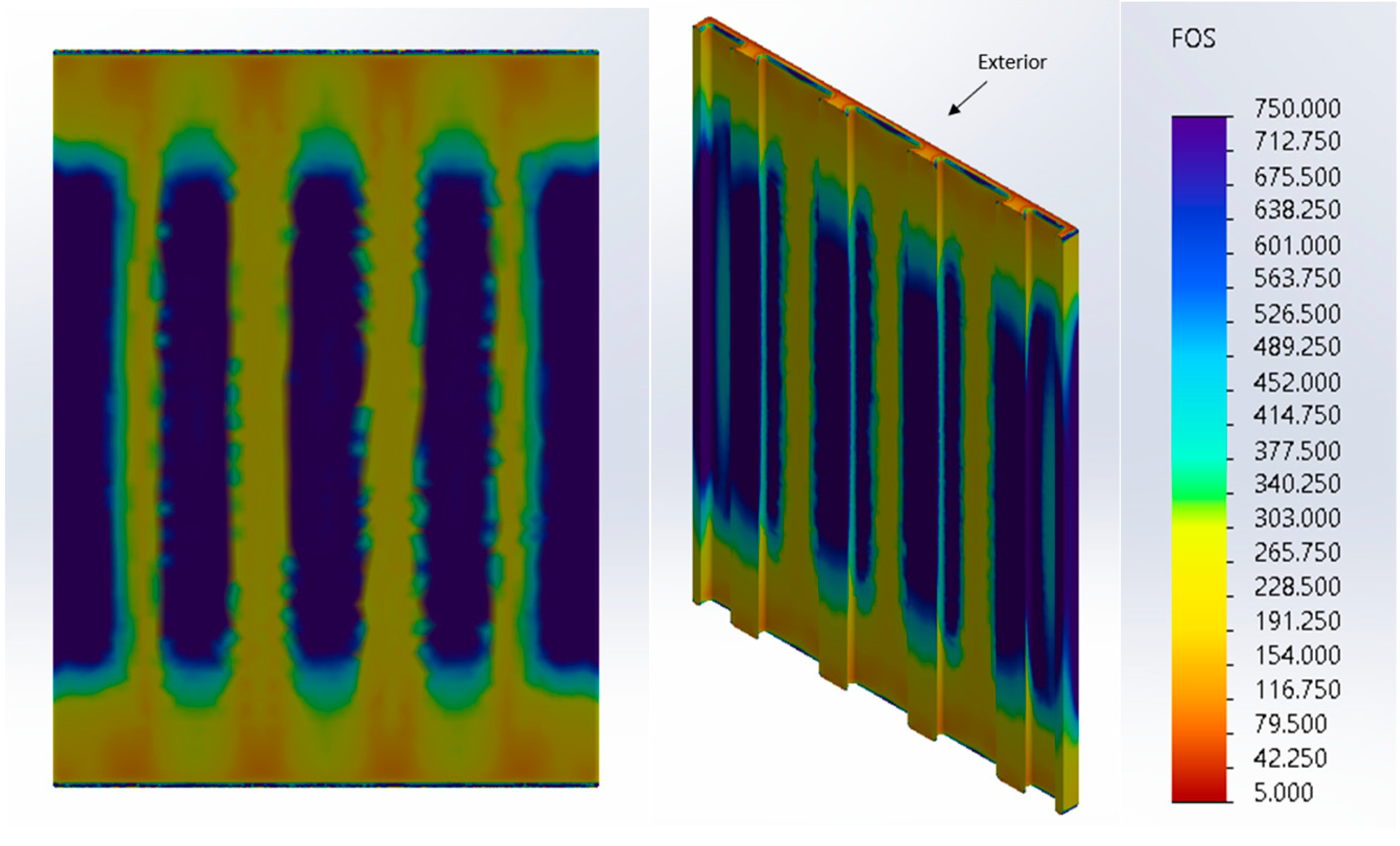
5.1. Zigzag Cavity Wall Structural Study
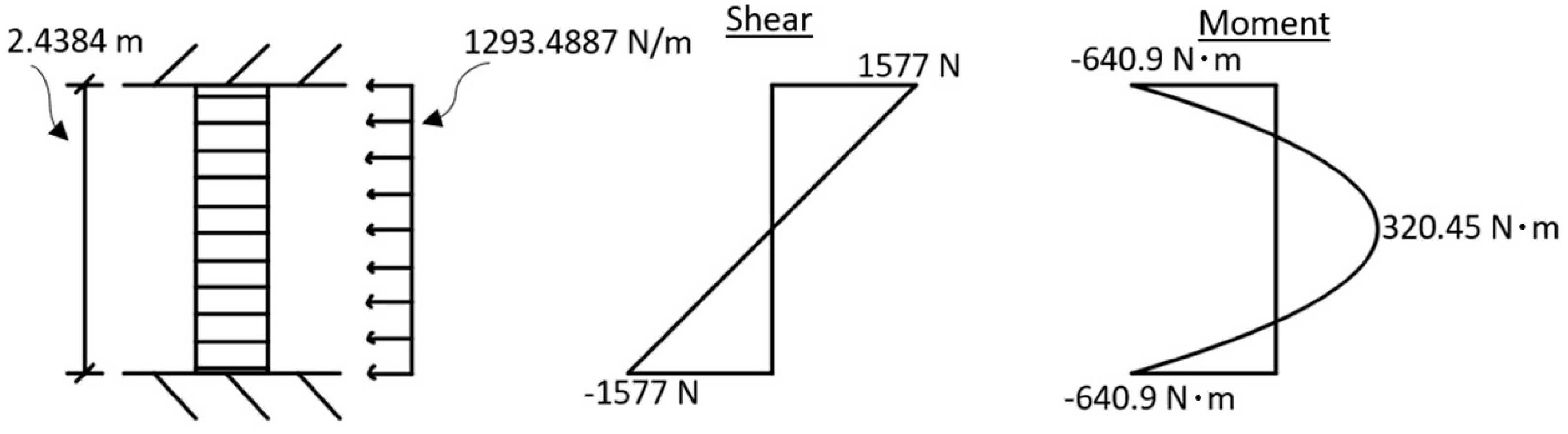
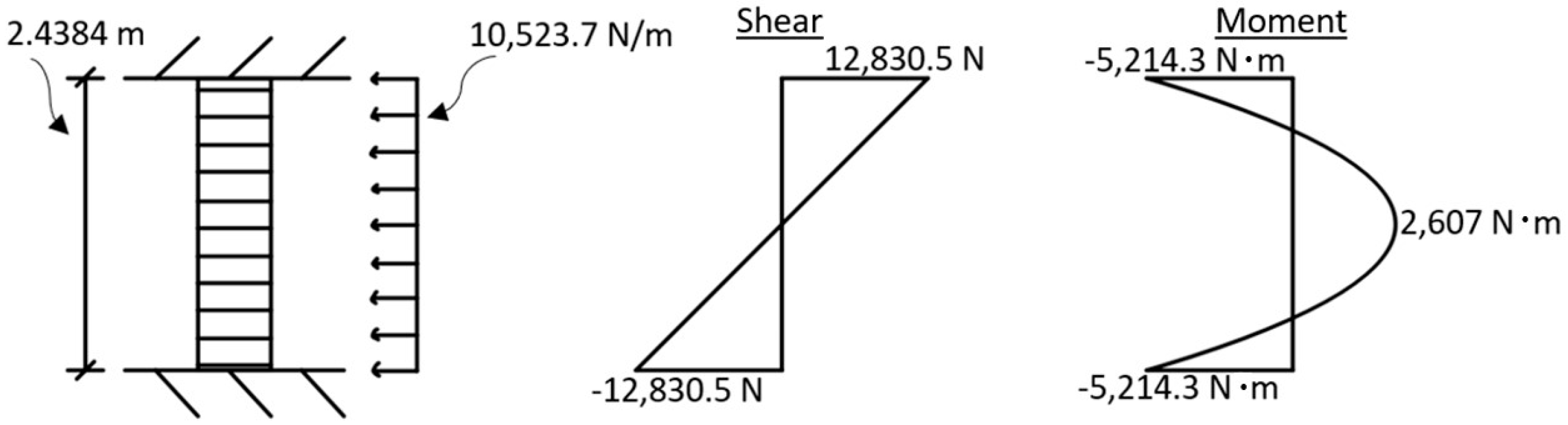
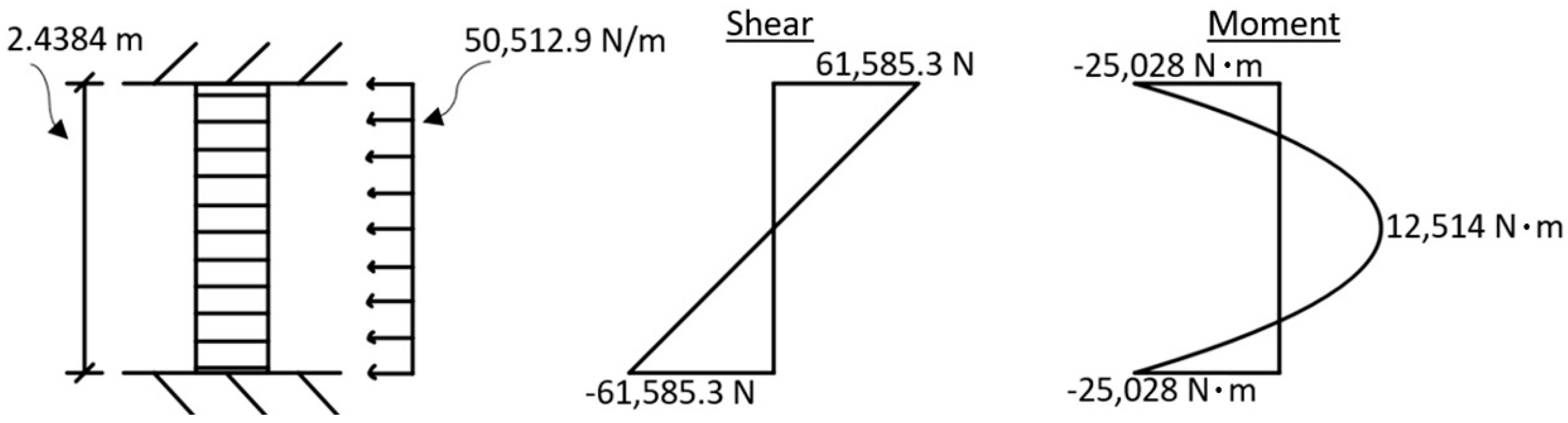
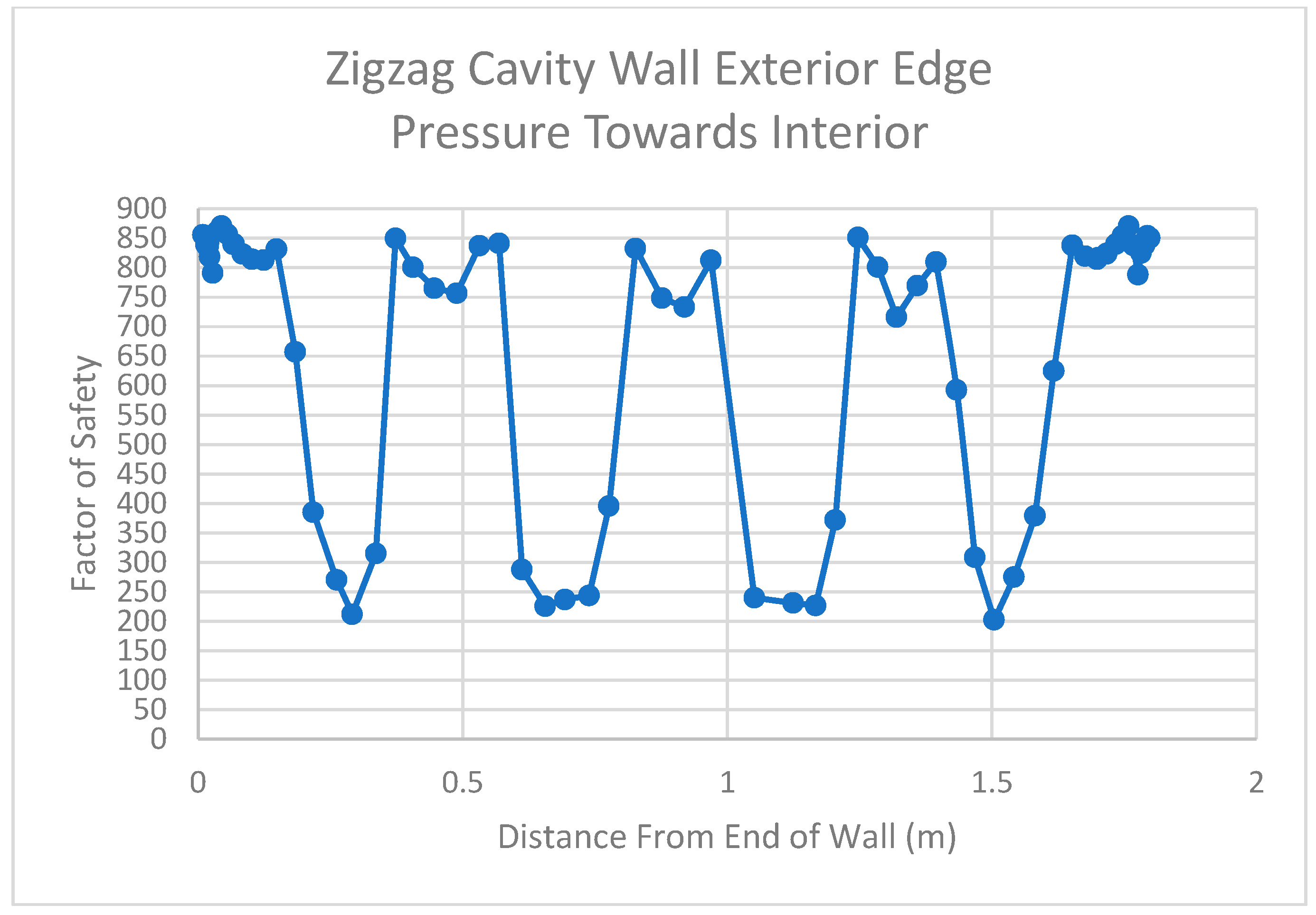
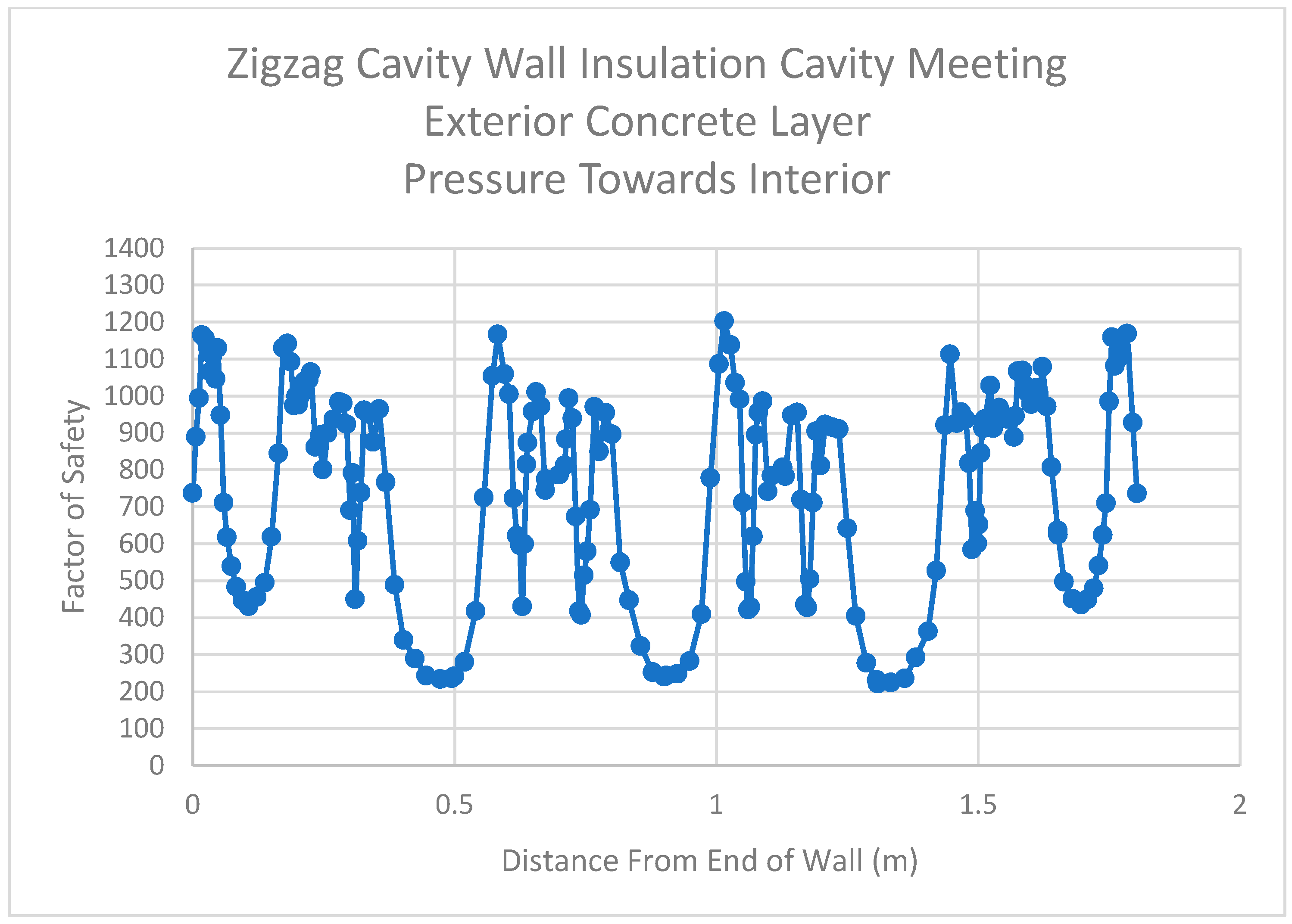
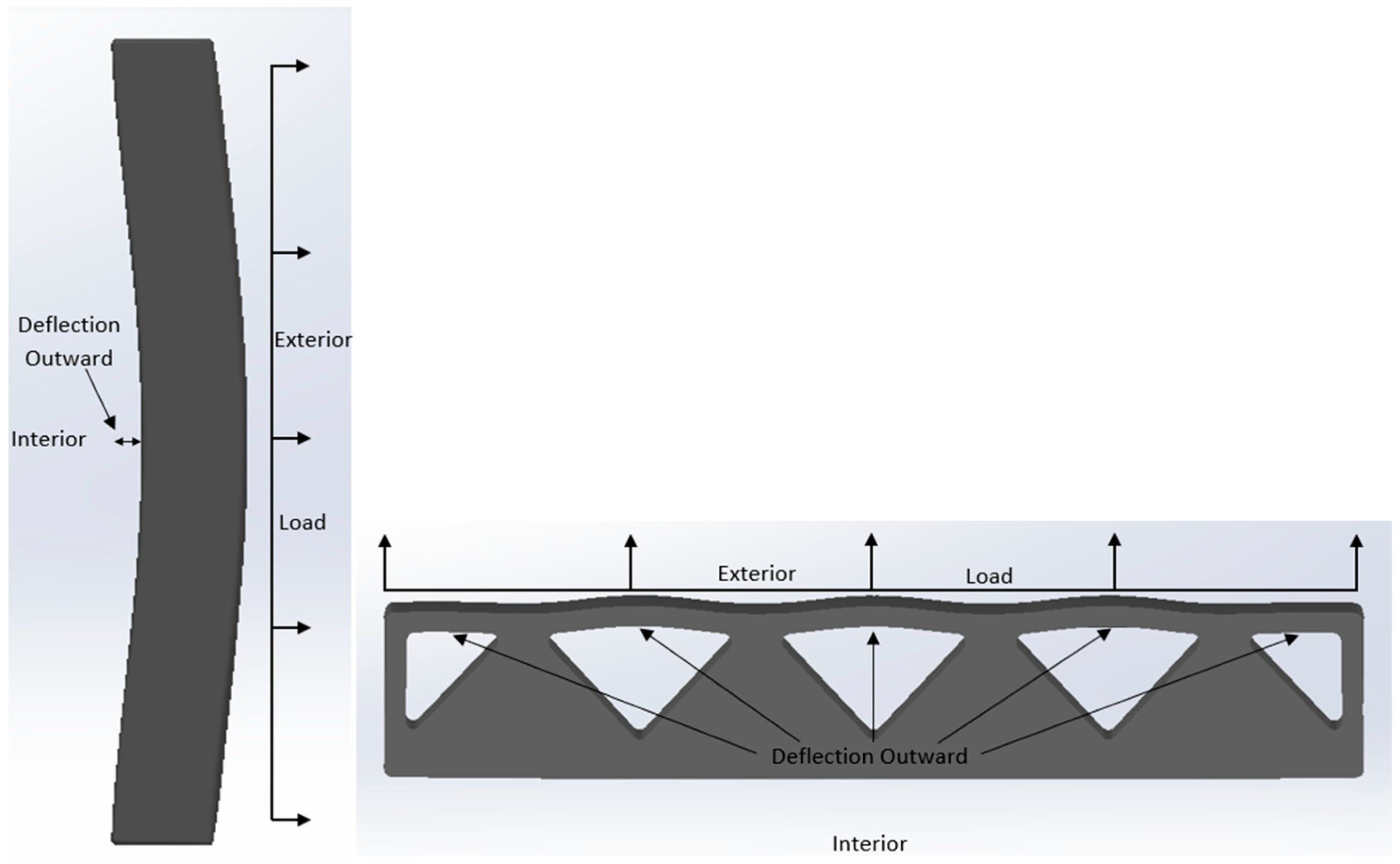
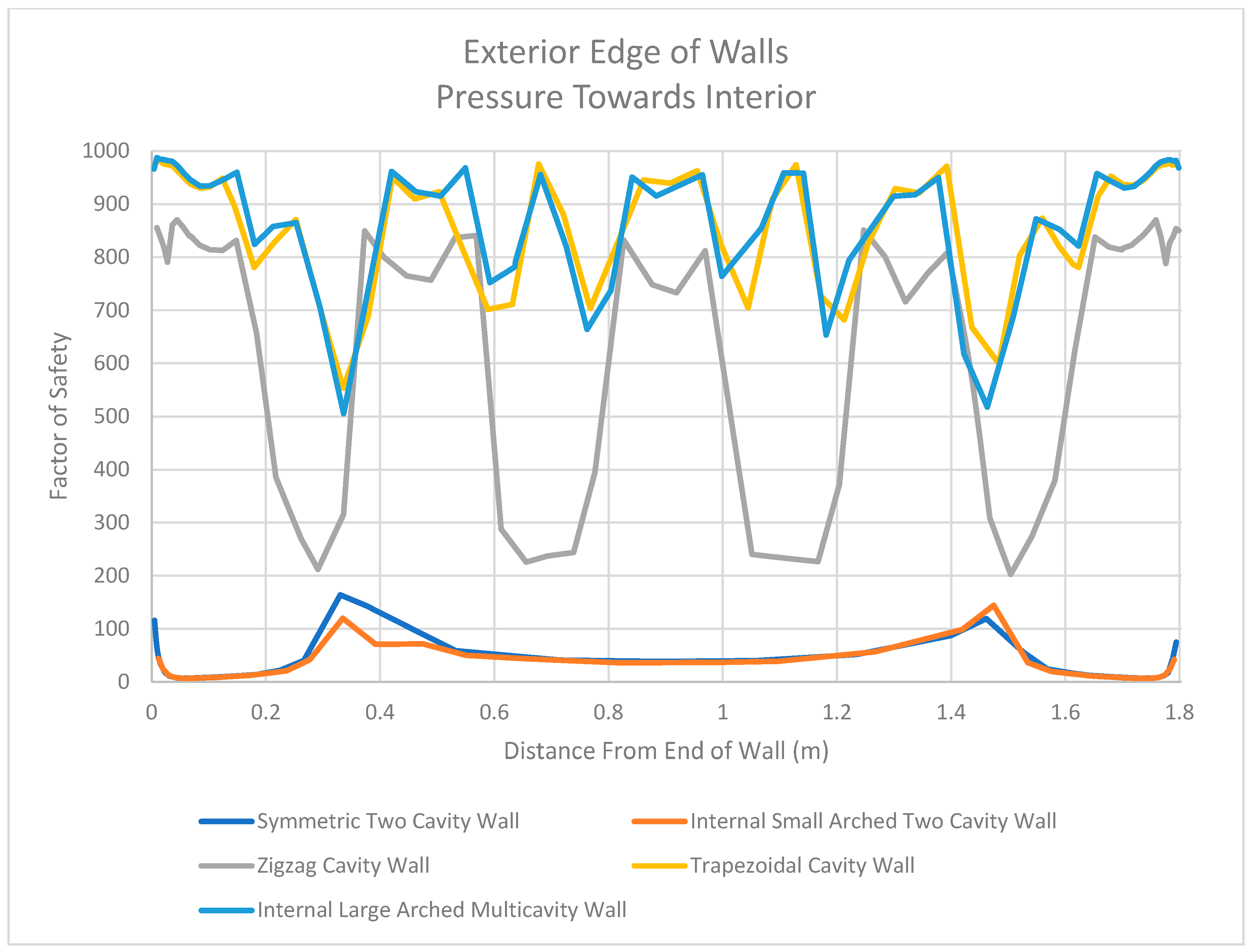
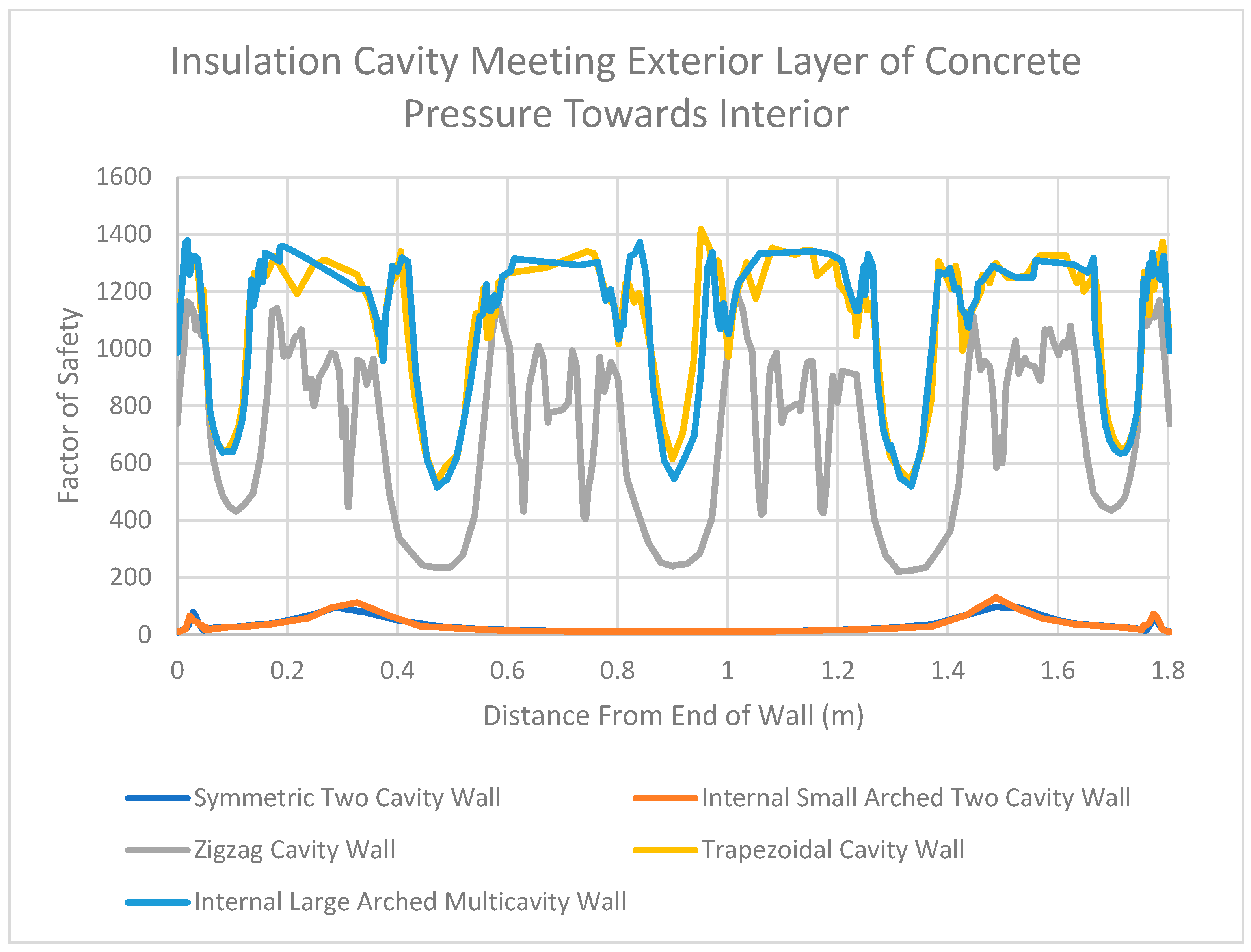
5.1.1. Pressure Applied on the Exterior Face towards the Interior
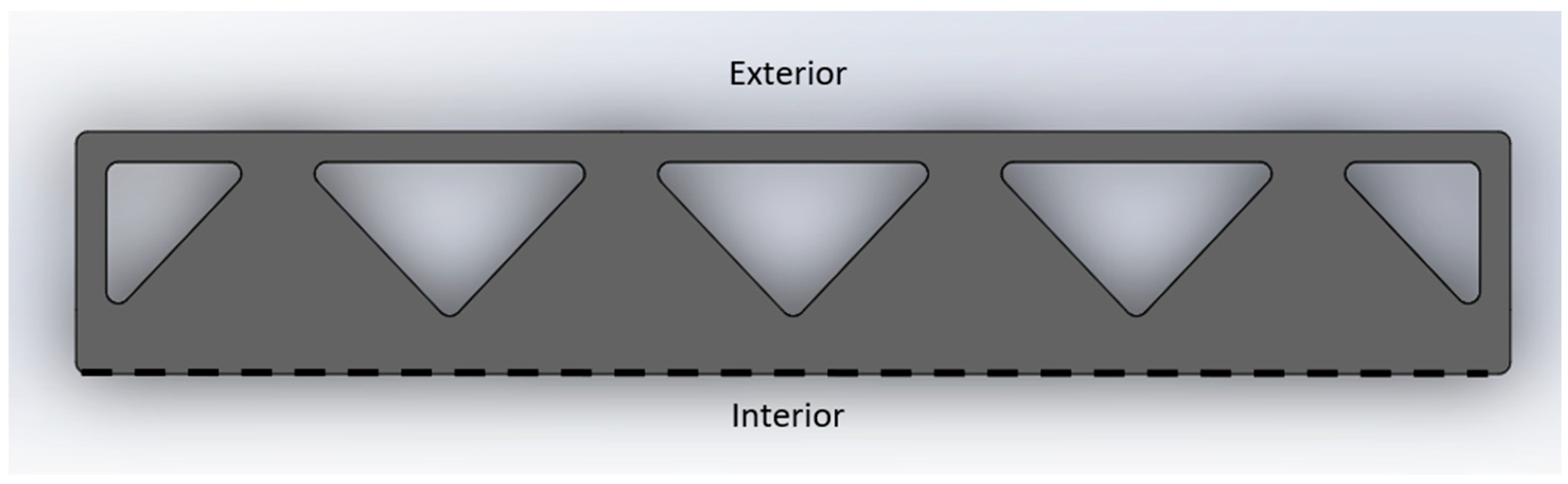
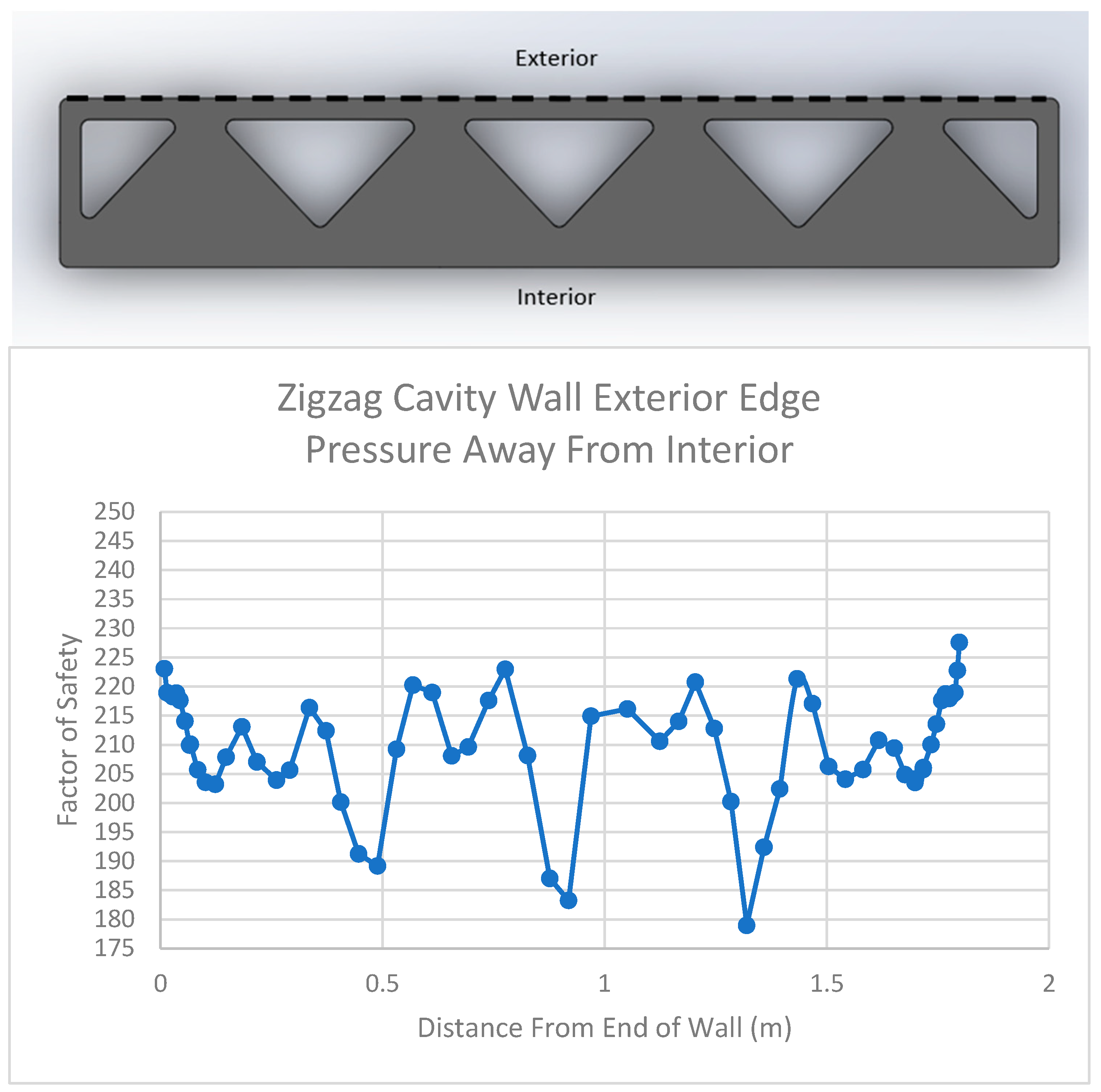
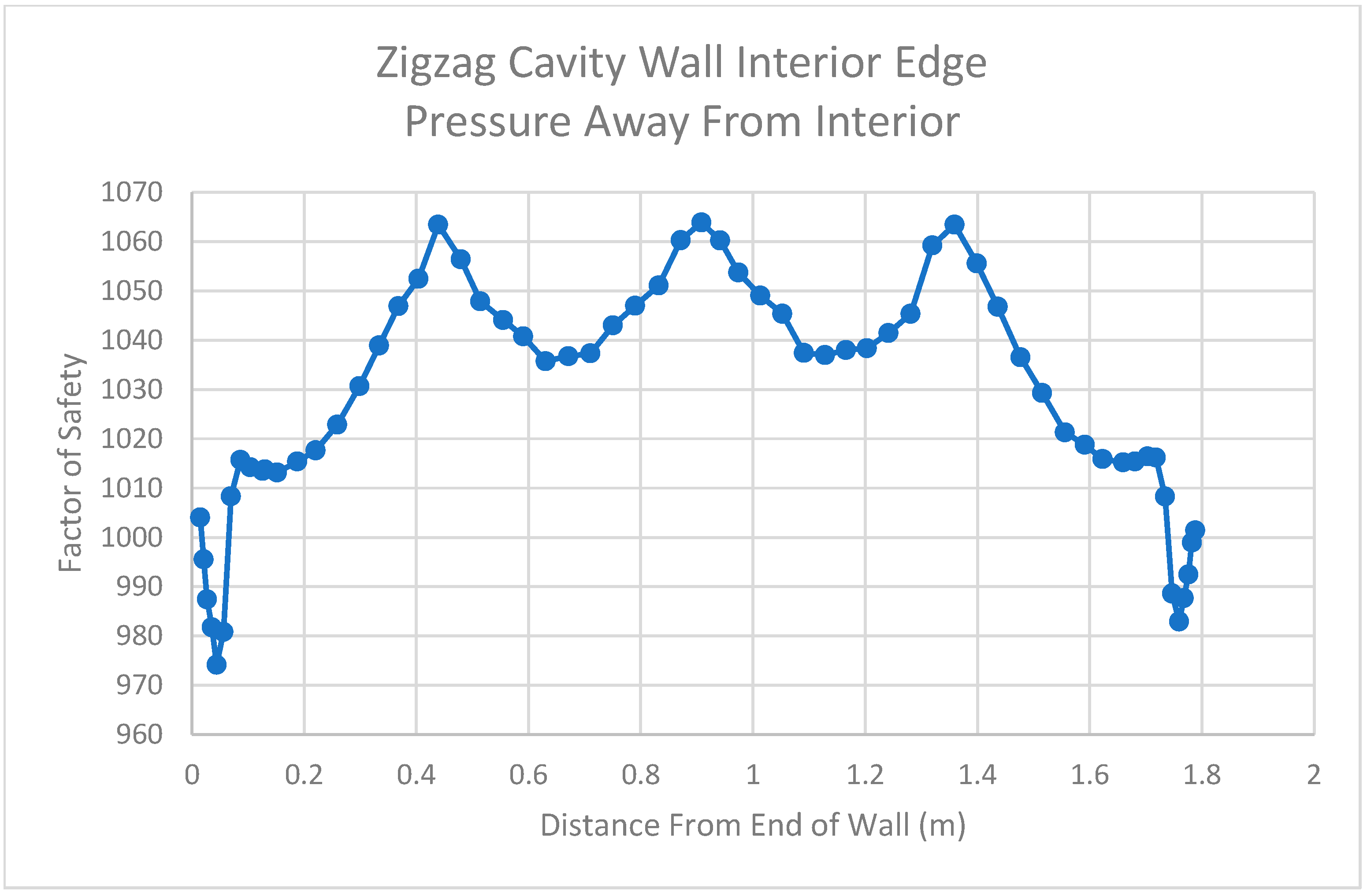
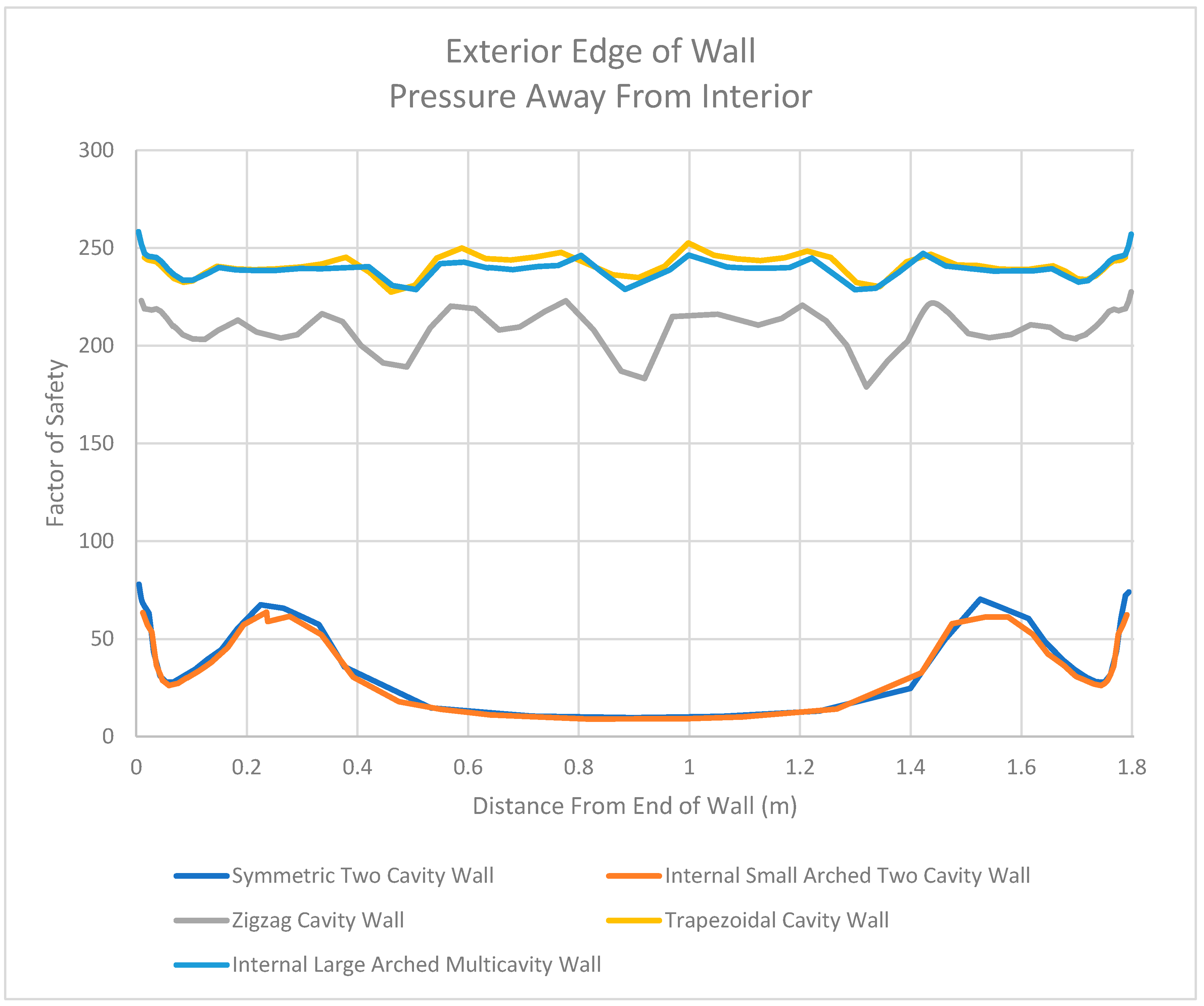
5.1.2. Pressure Applied on the Exterior Face away from the Interior
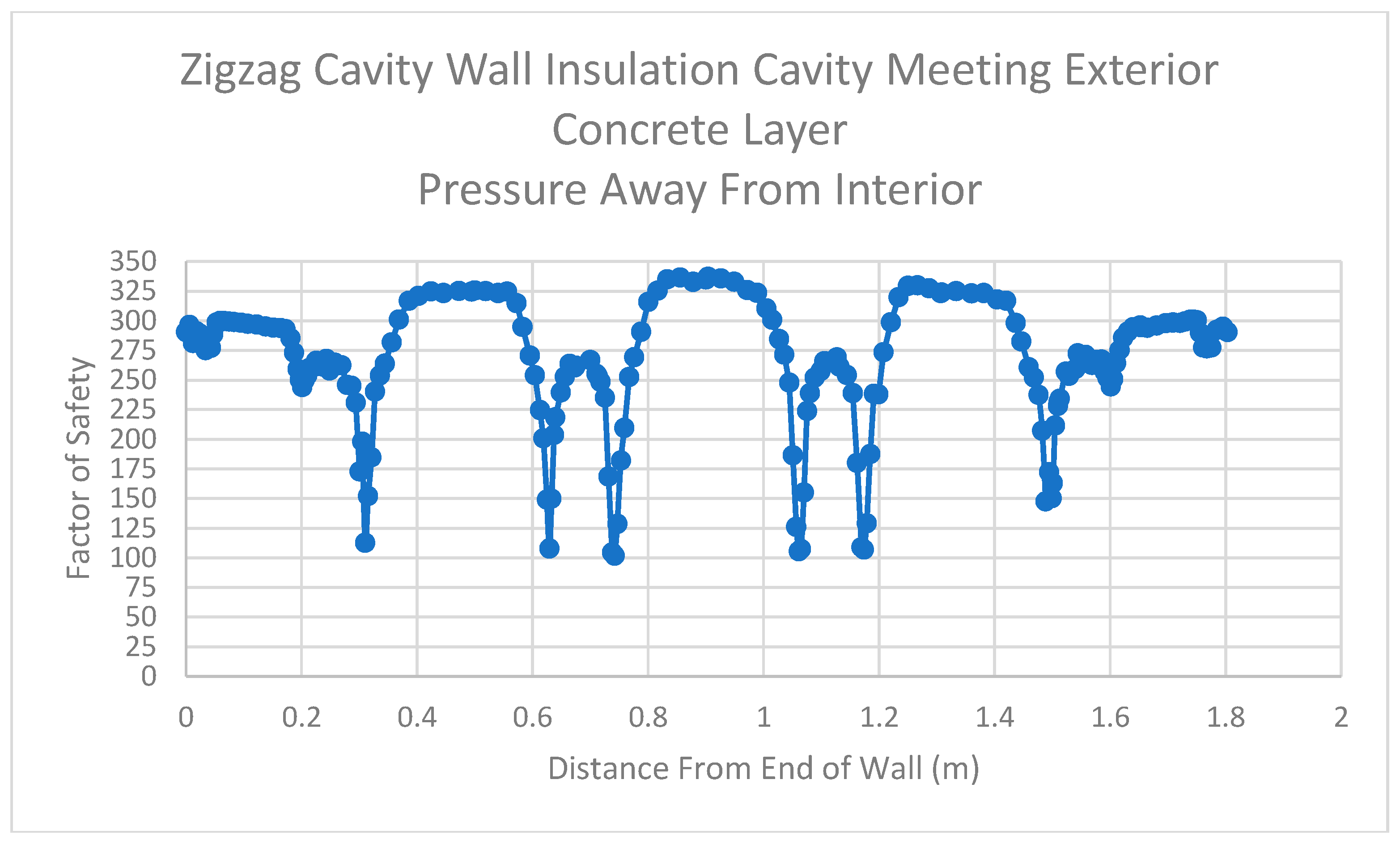
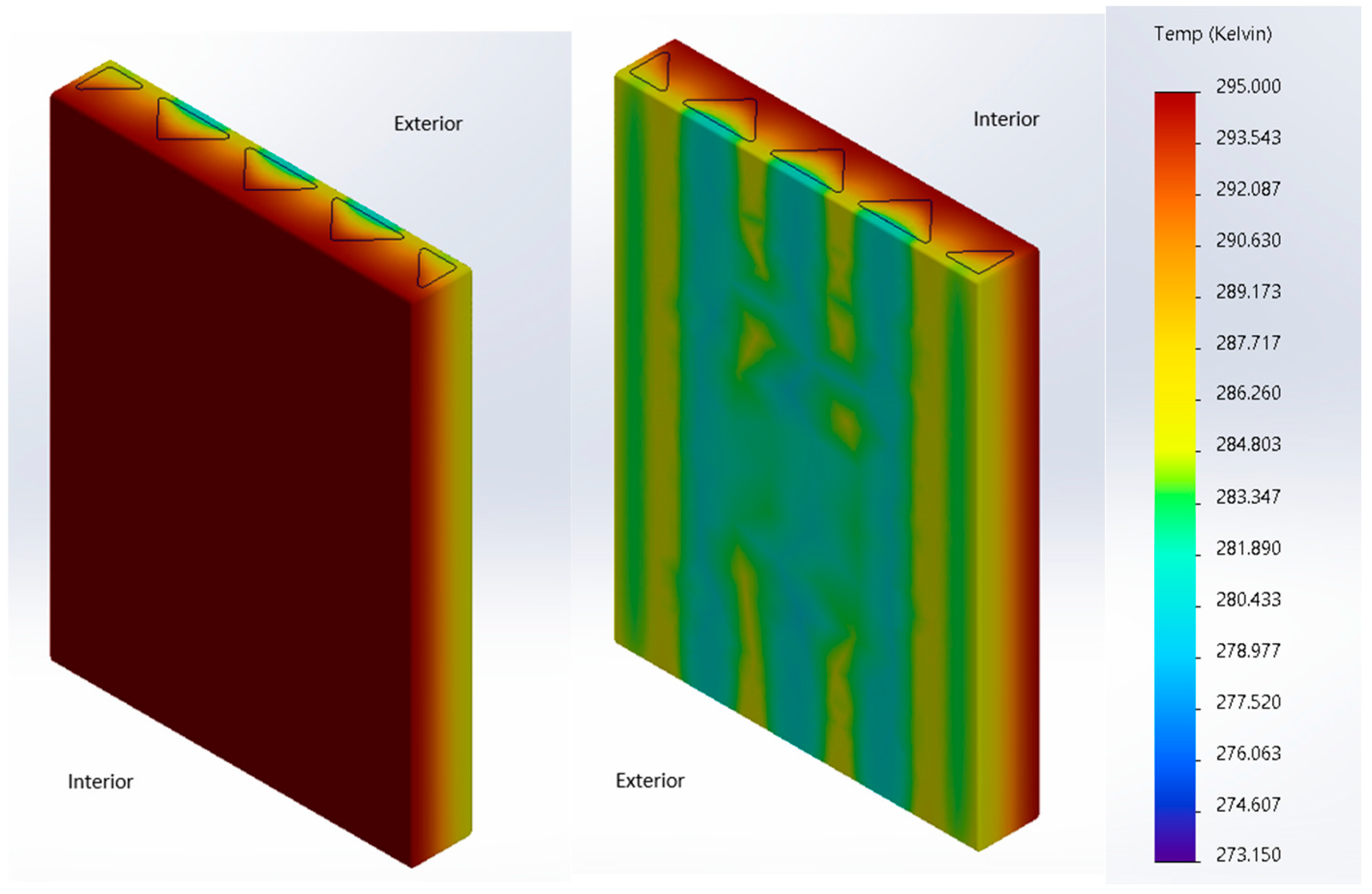
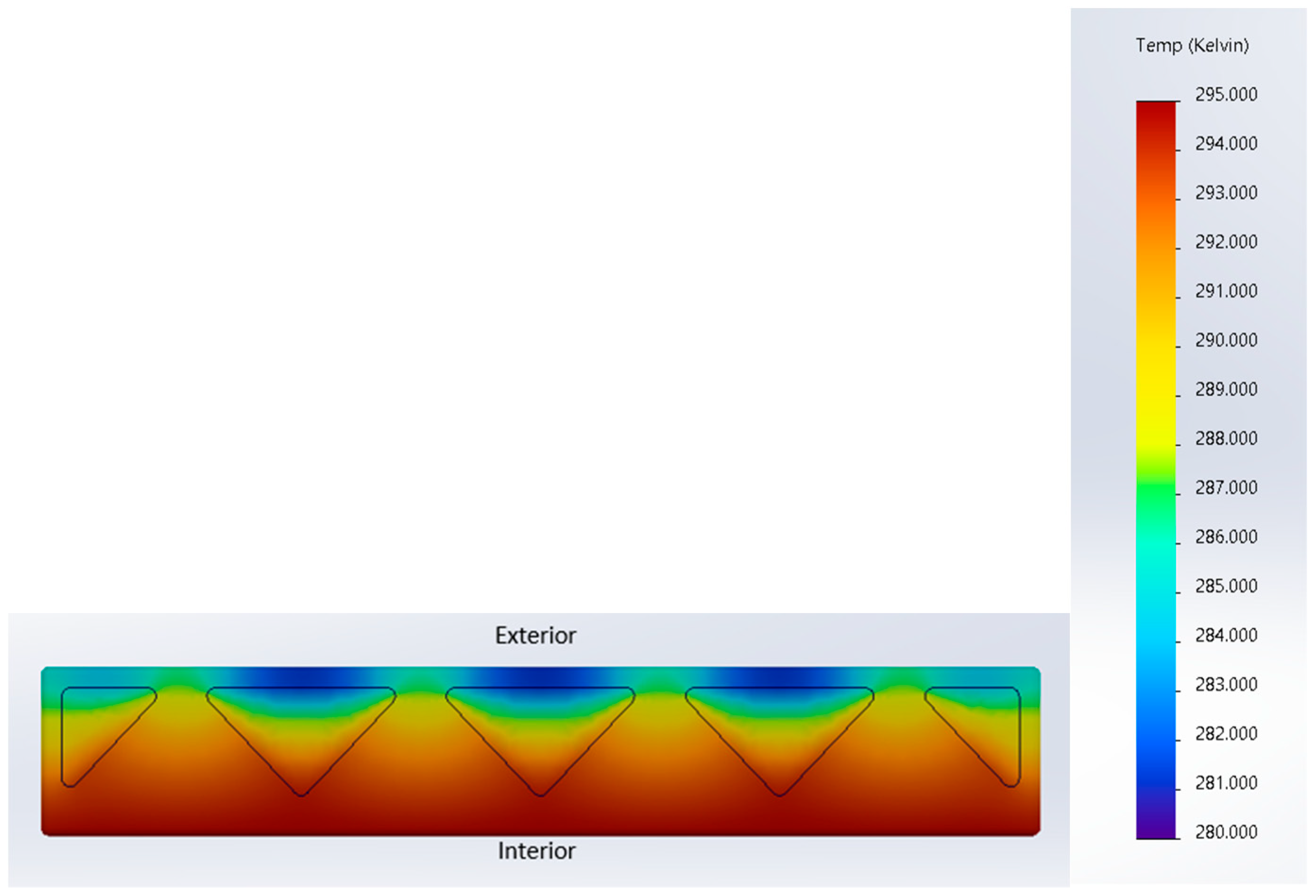
5.2. Zigzag Cavity Wall Thermal Study
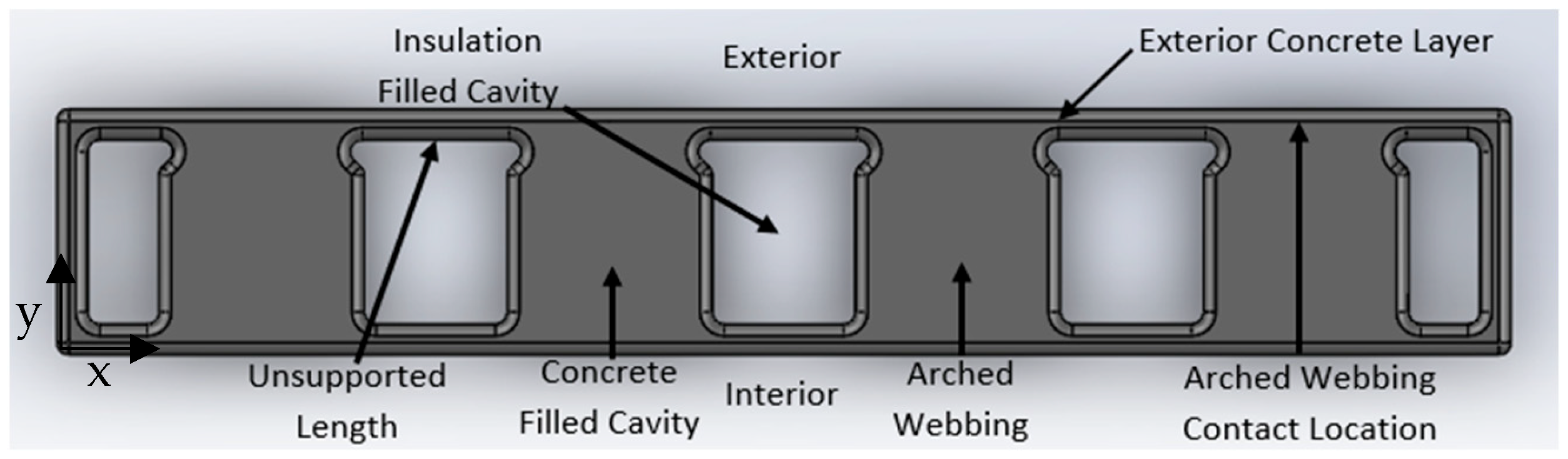
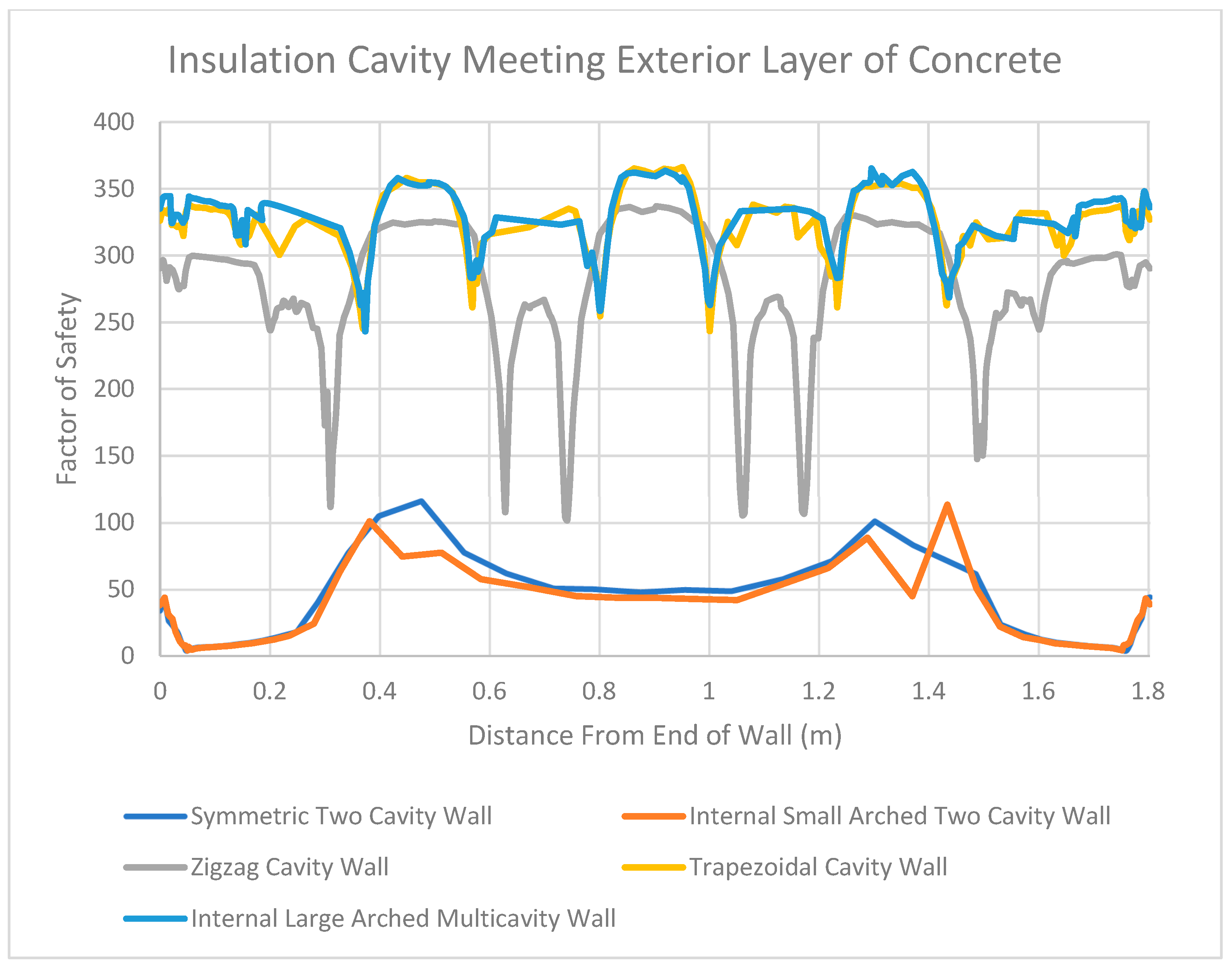
6. Relative Stiffness Determination for Different Wall Shapes
7. Discussion of Results
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Elfatah, A.S.A. 3D Printing in Architecture, Engineering and Construction. Eng. Res. J. 2019, 162, 119–137. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, J.; Dong, S.; Yu, X.; Han, B. A review of the current progress and application of 3D printed concrete. Compos. Part A Appl. Sci. Manuf. 2019, 125, 105533. [Google Scholar] [CrossRef]
- García-Alvarado, R.; Moroni-Orellana, G.; Banda-Pérez, P. Architectural evaluation of 3D-printed buildings. Buildings 2021, 11, 254. [Google Scholar] [CrossRef]
- Bos, F.; Wolfs, R.; Ahmed, Z.; Salet, T. Additive manufacturing of concrete in construction: Potentials and challenges of 3D concrete printing. Virtual Phys. Prototyp. 2016, 11, 209–225. [Google Scholar] [CrossRef]
- Verian, K.P.; Carli, M.D.; Bright, R.P.; Maandi, E. Research Development in 3DCP: Cured-on-Demand with Adhesion Enhancement Delivery System. ResearchGate, Laticrete International. 2018. Available online: https://www.researchgate.net/profile/Kho-Verian/publication/328757411_Research_Development_in_3DCP_Cured-on-Demand_with_Adhesion_Enhancement_Delivery_System/links/5be23cb292851c6b27ac6370/Research-Development-in-3DCP-Cured-on-Demand-with-Adhesion-Enhancement-Delivery-System.pdf (accessed on 2 January 2023).
- Buswell, R.A.; Leal de Silva, W.R.; Jones, S.Z.; Dirrenberger, J. 3D printing using concrete extrusion: A roadmap for Research. Cem. Concr. Res. 2018, 112, 37–49. [Google Scholar] [CrossRef]
- Dey, D.; Panda, B. An experimental study of thermal performance of 3D printed concrete slabs. Mater. Lett. 2023, 330, 133273. [Google Scholar] [CrossRef]
- Pessoa, S.; Guimarães, A.S.; Lucas, S.S.; Simões, N. 3D printing in the construction industry—A systematic review of the thermal performance in buildings. Renew. Sustain. Energy Rev. 2021, 141, 110794. [Google Scholar] [CrossRef]
- Wu, H.; Hu, R.; Yang, D.; Ma, Z. Micro-macro characterizations of mortar containing construction waste fines as replacement of cement and sand: A comparative study. Constr. Build. Mater. 2023, 383, 131328. [Google Scholar] [CrossRef]
- SolidWorks. Solidworks Simulation. 2022. Available online: https://www.solidworks.com/product/solidworks-simulation (accessed on 28 December 2022).
- Frei, W. Should I Fillet the Geometry in My Electromagnetic Heating Analysis? COMSOL. 2017. Available online: https://www.comsol.com/blogs/should-i-fillet-the-geometry-in-my-electromagnetic-heating-analysis/#:~:text=When%20building%20finite%20element%20models,a%20fillet%20avoids%20this%20singularity (accessed on 28 December 2022).
- Bi, Z. Finite Element Analysis Applications; Academic Press: Cambridge, MA, USA, 2019. [Google Scholar]
- SolidWorks. Stress Hot Spot Property Manager. Stress Hot Spot PropertyManager—2021—SOLIDWORKS Help, SolidWorks. 2021. Available online: https://help.solidworks.com/2021/english/SolidWorks/cworks/hidd_stress_hot_spot.htm (accessed on 28 December 2022).
- The Engineering Toolbox. Concrete Properties. Engineering ToolBox. 2008. Available online: https://www.engineeringtoolbox.com/concrete-properties-d_1223.html (accessed on 28 December 2022).
- ICON. Vulcan. ICON. 2022. Available online: https://www.iconbuild.com/vulcan (accessed on 30 March 2022).
- ACI 318-19; Building Code Requirements for Structural Concrete. American Concrete Institute: Farmington Hills, MI, USA, 2019.
- Talebi, H.R.; Kayan, B.A.; Asadi, I.; Hassan, Z. Investigation of Thermal Properties of Normal Weight Concrete for Different Strength Classes. J. Environ. Treat. Tech. 2020, 8, 908–914. [Google Scholar]
- SolidWorks. Mohr-Coulomb Stress Criterion. Mohr-Coulomb Stress Criterion—2018—SOLIDWORKS Help, SolidWorks. 2018. Available online: https://help.solidworks.com/2018/english/solidworks/cworks/r_mohr-coulomb_stress_criterion.htm (accessed on 28 December 2022).
- Elkady, A. #21 Abaqus Tutorial: Defining Concrete Damage Plasticity Model + Failure and Element Deletion. YouTube, YouTube. 2021. Available online: https://www.youtube.com/watch?v=wy84XGamn3g (accessed on 4 January 2023).
- The Constructor. Stress-Strain Curve for Concrete. The Constructor. 2018. Available online: https://theconstructor.org/concrete/stress-strain-curve-concrete/6159/ (accessed on 18 January 2023).
- ASCE/SEI 7-16; Minimum Design Loads and Associated Criteria for Buildings and Other Structures. American Society of Civil Engineers: Reston, VA, USA, 2017.
- SQ4D. 3D Printed Houses by SQ4D. SQ4D. 2022. Available online: https://www.sq4d.com/ (accessed on 12 April 2022).
- Petrock, S. Learn Solidworks Simulation in Less than 30 Min. Here’s All You Need to Know to Get Started. YouTube, YouTube. 2021. Available online: https://www.youtube.com/watch?v=Gthsy6OOjo0 (accessed on 28 December 2022).
- SolidWorks. Principal Stresses Definition. Principal Stresses Definition—2017—SOLIDWORKS Help. 2017. Available online: https://help.solidworks.com/2017/english/SolidWorks/cworks/c_principal_stresses_definition.htm?verRedirect=1 (accessed on 18 January 2023).
- Risinger, M. Is This the Future of Construction? 3D Concrete Printed Homes. YouTube. 2021. Available online: https://www.youtube.com/watch?v=_MsOXrprYXs (accessed on 30 March 2022).
- Gross, J. The Most Innovative 3D Printed House in the World [Mense-Korte]. YouTube. 2021. Available online: https://www.youtube.com/watch?v=qWBA-6NgIJg (accessed on 30 March 2022).
- Gross, J. Secrets of 3D Printed Concrete Exposed|Bold Black Buffalo 3D Shares All. YouTube. 2021. Available online: https://www.youtube.com/watch?v=R7lAnIWy5lg (accessed on 30 March 2022).
- WinSun. 3D Printing Architecture Profile. Yingchuang Building Technique Shanghai Co., Ltd. (Winsun). Available online: http://www.winsun3d.com/En/Product/pro_inner/id/1 (accessed on 21 September 2022).
- Apis Cor. 3D Houses. Apis Cor. 2022. Available online: https://www.apis-cor.com/showroom (accessed on 30 March 2022).
- CyBe. R & DRone Laboratory. CyBe. 2022. Available online: https://cybe.eu/cases/rdrone-lab/ (accessed on 30 March 2022).
- BING. Thermal Insulation Materials Made of Rigid Polyurethane Foam. Federation of European Rigid Polyurethane Foam Associations. 2006. Available online: https://highperformanceinsulation.eu/wp-content/uploads/2016/08/Thermal_insulation_materials_made_of_rigid_polyurethane_foam.pdf (accessed on 28 December 2022).
- SolidWorks. Types of Contact. Types of Contact—2020—SOLIDWORKS Help, SolidWorks. 2020. Available online: https://help.solidworks.com/2020/english/SolidWorks/cworks/ID_HELP_CONTACT_PAIR.htm?id=c5b1c459da654e7b9be880b23724a100#Pg0 (accessed on 28 December 2022).
- Kosky, P.G.; Balmer, R.; Keat, W.; Wise, G. Exploring Engineering: An Introduction to Engineering and Design; Academic Press: Waltham, MA, USA, 2013. [Google Scholar]
- SolidWorks. Heat Power/Energy Property Manager. Heat Power/Energy PropertyManager—2019—SOLIDWORKS Help, SolidWorks. 2019. Available online: https://help.solidworks.com/2019/english/SolidWorks/cworks/IDH_HELP_HEAT_POWER.htm?verRedirect=1 (accessed on 28 December 2022).
- American Society of Civil Engineers. ASCE 7 Hazard Tool. ASCE 7 Hazard Tool. 2021. Available online: https://asce7hazardtool.online/ (accessed on 14 February 2023).











| Variable | Value | ASCE 7-16 Section |
|---|---|---|
| Kz | 0.85 | 26.10.1 |
| Kzt | 1 | 26.8.2 |
| Kd | 0.85 | 26.6 |
| Ke | 1 | 26.9 |
| V (mph) | 90 | |
| Exposure Category | C | 26.7.3 |
| Risk Category | II | 1.5–1 |
| qh (psf) | 14.98 | 26.10–1 |
| qh (Pa) | 717.25 | 26.10–1 |
| Coefficient | Value |
|---|---|
| SMS | 0.375 |
| SM1 | 0.089 |
| SDS | 0.25 |
| SD1 | 0.06 |
| TL (s) | 6 |
| SDC | B |
| Coefficient | Value |
|---|---|
| SMS | 1.8 |
| SM1 | 0.84 |
| SDS | 1.2 |
| SD1 | 0.56 |
| TL (s) | 12 |
| SDC | D |
| Wall Type | Printed Concrete (m2) | Poured Concrete (m2) | Filled Insulation (m2) |
|---|---|---|---|
| Symmetric Two-Cavity Wall | 0.22 | 0.16 | 0.16 |
| Internal Small-Arched Multicavity Wall | 0.24 | 0.10 | 0.20 |
| Zigzag Cavity Wall | 0.24 | 0.15 | 0.15 |
| Trapezoidal Cavity Wall | 0.27 | 0.14 | 0.14 |
| Internal Large-Arched Multicavity Wall | 0.25 | 0.13 | 0.17 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bello, N.D.; Memari, A.M. A Structural and Thermal Comparative Review of 3D-Printed Wall Shapes. Designs 2023, 7, 80. https://doi.org/10.3390/designs7030080
Bello ND, Memari AM. A Structural and Thermal Comparative Review of 3D-Printed Wall Shapes. Designs. 2023; 7(3):80. https://doi.org/10.3390/designs7030080
Chicago/Turabian StyleBello, Nicholas D., and Ali M. Memari. 2023. "A Structural and Thermal Comparative Review of 3D-Printed Wall Shapes" Designs 7, no. 3: 80. https://doi.org/10.3390/designs7030080
APA StyleBello, N. D., & Memari, A. M. (2023). A Structural and Thermal Comparative Review of 3D-Printed Wall Shapes. Designs, 7(3), 80. https://doi.org/10.3390/designs7030080