Bio-lubricant Properties Analysis of Drilling an Innovative Design of Bioactive Kinetic Screw into Bone
Abstract
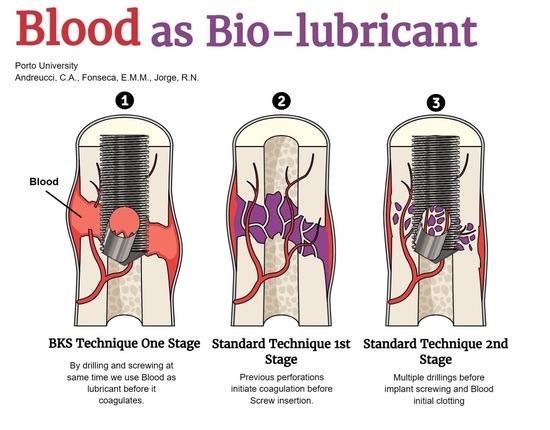
:1. Introduction
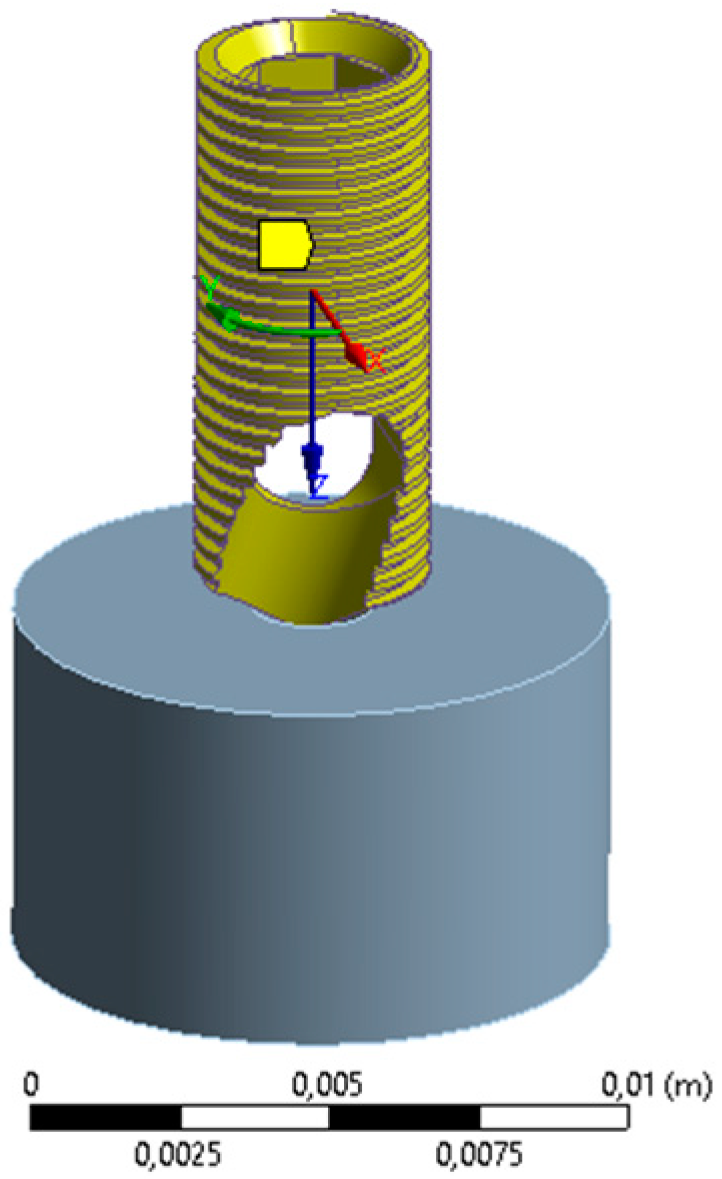
2. Materials and Methods
3. Results
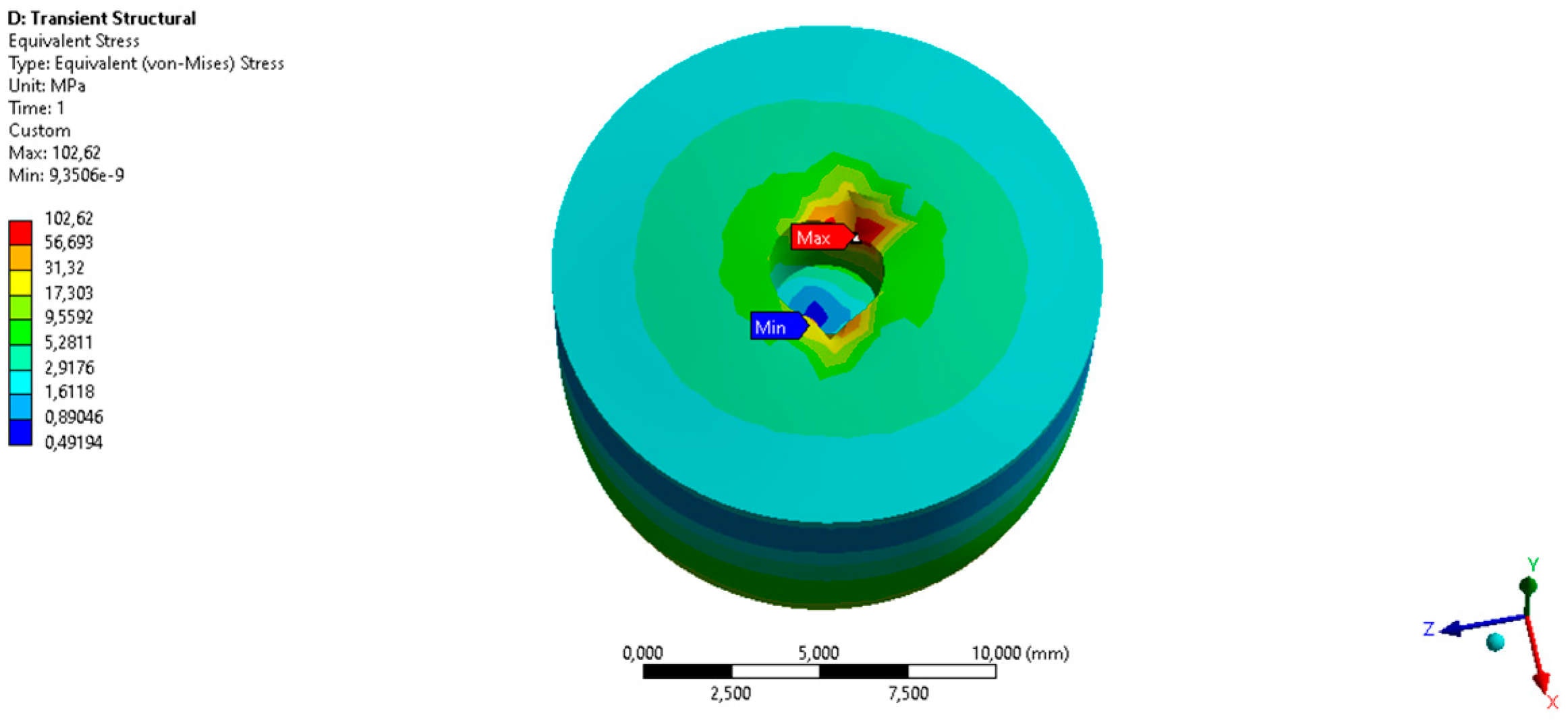
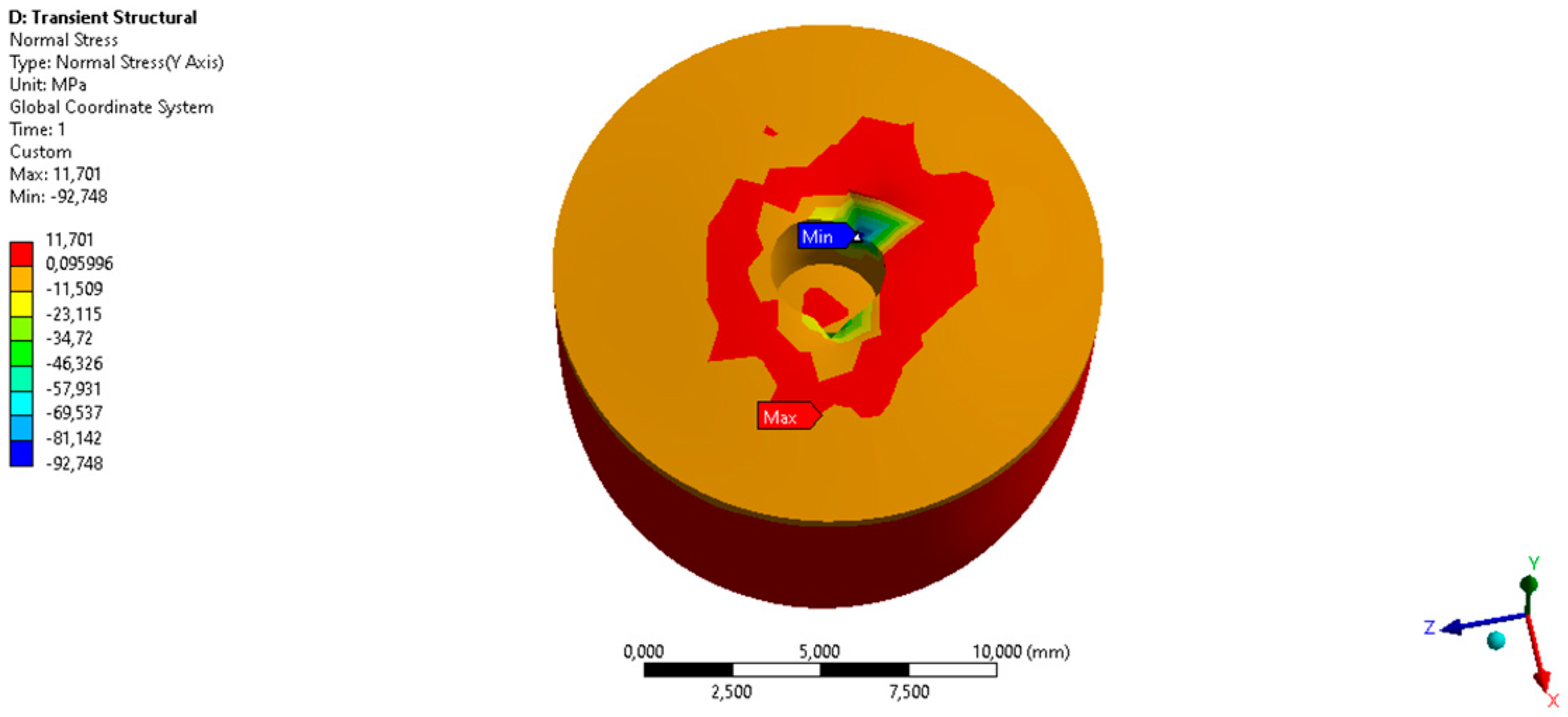
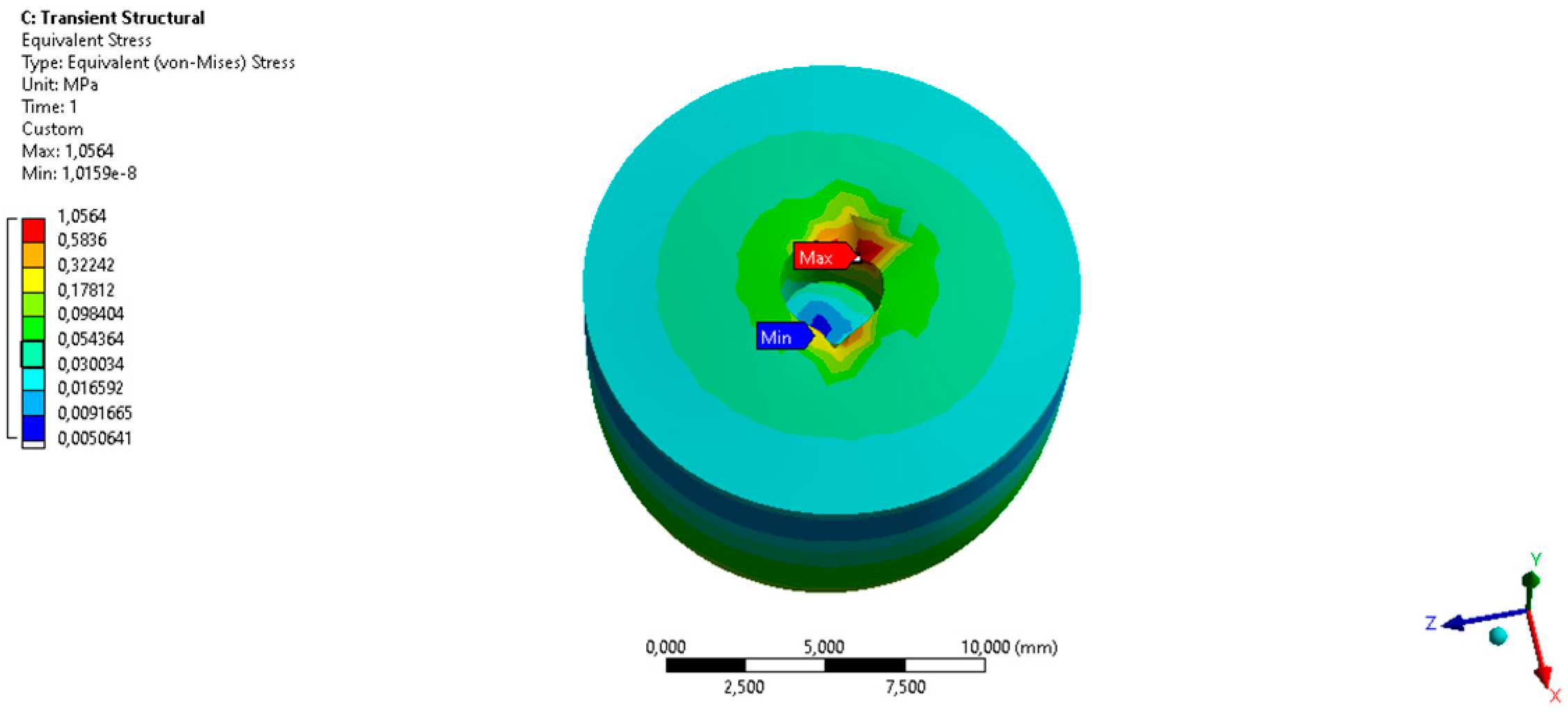
3.1. FEA Bone Density D1 (1.85 × 10−6 kg/mm3, 17,000 MPa)
3.2. FEA Bone Density D4 (0.45 × 10−6 kg/mm3, 175.12 MPa)
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Vahedi, A.; Bigdelou, P.; Farnoud, A.M. Quantitative analysis of red blood cell membrane phospholipids and modulation of cell-macrophage interactions using cyclodextrins. Sci. Rep. 2020, 10, 15111. [Google Scholar] [CrossRef] [PubMed]
- Minton, K. Red blood cells join the ranks as immune sentinels. Nat. Rev. Immunol. 2021, 21, 760–761. [Google Scholar] [CrossRef] [PubMed]
- Mathew, J.; Sankar, P.; Varacallo, M. Physiology, Blood Plasma. In StatPearls; StatPearls Publishing: Treasure Island, FL, USA, 2022. [Google Scholar]
- Generalov, V.M.; Safatov, A.S.; Kruchinina, M.V. Dielectric Properties of the Human Red Blood Cell. Meas. Tech. 2020, 63, 580–586. [Google Scholar] [CrossRef]
- Ahmed, M.H.; Ghatge, M.S.; Safo, M.K. Hemoglobin: Structure, Function and Allostery. Subcell. Biochem. 2020, 94, 345–382. [Google Scholar] [CrossRef]
- Tutwiler, V.; Singh, J.; Litvinov, R.I.; Bassani, J.L.; Purohit, P.K.; Weisel, J.W. Rupture of blood clots: Mechanics and pathophysiology. Sci. Adv. 2020, 6, eabc0496. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Sapkota, A.; Kikuchi, D.; Sakota, D.; Maruyama, O.; Takei, M. Red blood cells aggregability measurement of coagulating blood in extracorporeal circulation system with multiple-frequency electrical impedance spectroscopy. Biosens. Bioelectron. 2018, 112, 79–85. [Google Scholar] [CrossRef]
- Stauffer, E.; Loyrion, E.; Hancco, I. Blood viscosity and its determinants in the highest city in the world. J. Physiol. 2020, 598, 4121–4130. [Google Scholar] [CrossRef]
- Makowiecki, A.; Hadzik, J.; Błaszczyszyn, A.; Gedrange, T.; Dominiak, M. An evaluation of superhydrophilic surfaces of dental implants—a systematic review and meta-analysis. BMC Oral Health 2019, 19, 79. [Google Scholar] [CrossRef]
- Florencio-Silva, R.; Sasso, G.R.; Sasso-Cerri, E.; Simões, M.J.; Cerri, P.S. Biology of Bone Tissue: Structure, Function, and Factors That Influence Bone Cells. Biomed Res. Int. 2015, 2015, 421746. [Google Scholar] [CrossRef]
- Raj, A.; Rajak, D.K.; Gautam, S.; Guria, C.; Pathak, A.K. Shear Rate Estimation: A Detailed Review. In Proceedings of the Paper Presented at the Offshore Technology Conference, Houston, TX, USA, 2–5 May 2016. [Google Scholar] [CrossRef]
- Gu, C.; Meng, X.; Xie, Y.; Zhang, D. The influence of surface texturing on the transition of the lubrication regimes between a piston ring and a cylinder liner. Int. J. Eng. Res. 2017, 18, 785–796. [Google Scholar] [CrossRef]
- Veltkamp, B.; Velikov, K.P.; Venner, C.H.; Bonn, D. Lubricated Friction and the Hersey Number. Phys. Rev. Lett. 2021, 126, 044301. [Google Scholar] [CrossRef] [PubMed]
- Straumal, B.B.; Gornakova, A.S.; Kiselevskiy, M.V. Optimal surface roughness of Ti6Al4V alloy for the adhesion of cells with osteogenic potential. J. Mater. Res. 2022, 37, 2661–2674. [Google Scholar] [CrossRef]
- Kamynina, O.K.; Kravchuk, K.S.; Lazov, M.A. Effect of Surface Roughness on the Properties of Titanium Materials for Bone Implants. Russ. J. Inorg. Chem. 2021, 66, 1073–1078. [Google Scholar] [CrossRef]
- Boyan, B.D.; Lotz, E.M.; Schwartz, Z. Roughness and Hydrophilicity as Osteogenic Biomimetic Surface Properties. Tissue Eng. Part A 2017, 23, 1479–1489. [Google Scholar] [CrossRef] [PubMed]
- Wang, C.; Zhang, G.; Li, Z.; Zeng, X.; Xu, Y.; Zhao, S.; Hu, H.; Zhang, Y.; Ren, T. Tribological behavior of Ti-6Al-4V against cortical bone in different biolubricants. J. Mech. Behav. Biomed. Mater. 2019, 90, 460–471. [Google Scholar] [CrossRef]
- Lian, Z.; Guan, H.; Ivanovski, S.; Loo, Y.C.; Johnson, N.W.; Zhang, H. Effect of bone to implant contact percentage on bone remodeling surrounding a dental implant. Int. J. Oral Maxillofac. Surg. 2010, 39, 690–698. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.W.Y.; Bance, M.L. Physiology of Osseointegration. Otolaryngol. Clin. N. Am. 2019, 52, 231–242. [Google Scholar] [CrossRef]
- Rahmanpanah, H.; Mouloodi, S.; Burvill, C.; Gohari, S.; Davies, H.M.S. Prediction of load-displacement curve in a complex structure using artificial neural networks: A study on a long bone. Int. J. Eng. Sci. 2020, 154, 103319. [Google Scholar] [CrossRef]
- Maria, G.F.; Elza, M.F.; Renato, M.N. Three-dimensional dynamic finite element and experimental models for drilling processes. Proc. IMechE. Part L J. Mater. Des. Appl. 2015, 232, 35–43. [Google Scholar]
- Andreucci, C.A.; Fonseca, E.M.M.; Jorge, R.N. Increased Material Density within a New Biomechanism. Math. Comput. Appl. 2022, 27, 90. [Google Scholar] [CrossRef]
- Wang, S.-H.; Shen, Y.-W.; Fuh, L.-J.; Peng, S.-L.; Tsai, M.-T.; Huang, H.-L.; Hsu, J.-T. Relationship between Cortical Bone Thickness and Cancellous Bone Density at Dental Implant Sites in the Jawbone. Diagnostics 2020, 10, 710. [Google Scholar] [CrossRef] [PubMed]
- Schertzer, M.J.; Iglesias, P. Meta-Analysis Comparing Wettability Parameters and the Effect of Wettability on Friction Coefficient in Lubrication. Lubricants 2018, 6, 70. [Google Scholar] [CrossRef]
- Shacham, S.; Castel, D.; Gefen, A. Measurements of the static friction coefficient between bone and muscle tissues. J. Biomech. Eng. 2010, 132, 084502. [Google Scholar] [CrossRef] [PubMed]
- Dannaway, J.; Dabirrahmani, D. An investigation into the frictional properties between bone and various orthopedic implant surfaces—implant stability. J. Musculoskelet. 2015, 18, 1550015. [Google Scholar] [CrossRef]
- Haoyu, Z.; Zhang, X.; Jun, S.; Dagang, W. A study of misaligned compliant journal bearings lubricated by non-Newtonian fluid considering surface roughness. Trib. Int. 2023, 179, 108138. [Google Scholar] [CrossRef]
- Halldin, A.; Jinno, Y.; Galli, S.; Ander, M.; Jacobsson, M.; Jimbo, R. Implant stability and bone remodeling up to 84 days of implantation with an initial static strain. An in vivo and theoretical investigation. Clin. Oral Impl. Res. 2016, 27, 1310–1316. [Google Scholar] [CrossRef] [PubMed]
- Makary, C.; Rebaudi, A.; Mokbel, N.; Naaman, N. Peak Insertion Torque Correlated to Histologically and Clinically Evaluated Bone Density. Impl. Dent. 2011, 20, 182–191. [Google Scholar] [CrossRef] [PubMed]
- Andreucci, C.A.; Fonseca, E.M.M.; Natal, R.M.J. Structural analysis of the new Bioactive Kinetic Screw in titanium alloy vs. commercially pure titanium. J. Comp. Art. Int. Mec. Biomec. 2022, 2, 35–43. [Google Scholar] [CrossRef]
- Andreucci, C.A.; Alshaya, A.; Fonseca, E.M.M.; Jorge, R.N. Proposal for a New Bioactive Kinetic Screw in an Implant, Using a Numerical Model. Appl. Sci. 2022, 12, 779. [Google Scholar] [CrossRef]
- Andreucci, C.A.; Fonseca, E.M.M.; Jorge, R.N. 3D Printing as an Efficient Way to Prototype and Develop Dental Implants. BioMedInformatics 2022, 2, 44. [Google Scholar] [CrossRef]
- Trzepiecinski, T. A Study of the Coefficient of Friction in Steel Sheets Forming. Metals 2019, 9, 988. [Google Scholar] [CrossRef]
- Xing, G.; Manon, F.; Guillaume, H. Biomechanical behaviours of the bone–implant interface: A review. J. R. Soc. Interface 2019, 16, 20190259. [Google Scholar] [CrossRef]
- Milillo, L.; Cinone, F.; Lo Presti, F.; Lauritano, D.; Petruzzi, M. The Role of Blood Clot in Guided Bone Regeneration: Biological Considerations and Clinical Applications with Titanium Foil. Materials 2021, 14, 6642. [Google Scholar] [CrossRef] [PubMed]
- Tsiagadigui, J.G.; Ndiwe, B.; Yamben, M.N.; Fotio, N.; Belinga, F.E.; Njeugna, E. The effects of multiple drilling of a bone with the same drill bit: Thermal and force analysis. Heliyon 2022, 8, e08927. [Google Scholar] [CrossRef]
- Pallua, J.D.; Putzer, D.; Jäger, E.; Degenhart, G.; Arora, R.; Schmölz, W. Characterizing the Mechanical Behavior of Bone and Bone Surrogates in Compression Using pQCT. Materials 2022, 15, 5065. [Google Scholar] [CrossRef] [PubMed]
- Anesi, A.; Cavani, F. Editorial for the Special Issue on “Multidisciplinary Insights on Bone Healing”. Biology 2022, 11, 1776. [Google Scholar] [CrossRef]
- Anitua, E. Biological drilling: Implant site preparation in a conservative manner and obtaining autogenous bone grafts. Balk. J. Dent. Med. 2018, 22, 98–101. [Google Scholar] [CrossRef]
- Cirera, A.; Manzanares, M.C.; Sevilla, P.; Ortiz-Hernandez, M.; Galindo-Moreno, P.; Gil, J. Biofunctionalization with a TGFβ-1 Inhibitor Peptide in the Osseointegration of Synthetic Bone Grafts: An In Vivo Study in Beagle Dogs. Materials 2019, 12, 3168. [Google Scholar] [CrossRef] [Green Version]





| Ti6Al4V (Grade 5) | |
|---|---|
| Young’s Modulus, MPa | 2.0 × 105 |
| Poisson’s Ratio | 0.3 |
| Maximum Yield Stress, MPa | 1450 |
| Initial Yield Stress, MPa | 850 |
| Density, kg/mm3 | 4.51 × 10−6 |
| Coefficient of Thermal Expansion, 1/°C | 8.5 × 10−6 |
| Cortical Bone | |
|---|---|
| Poisson’s Ratio | 0.3 |
| Maximum Yield Stress, MPa | 125 |
| Initial Yield Stress, MPa | 10 |
| Coefficient of Thermal Expansion, 1/°C | 8.9 × 105 |
| Young’s Modulus, MPa (D1) | 17,000 |
| Young’s Modulus, MPa (D4) | 175.12 |
| Density, kg/mm3 (D1) | 1.85 × 10−6 |
| Density, kg/mm3 (D4) | 0.45 × 10−6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Andreucci, C.A.; Fonseca, E.M.M.; Jorge, R.N. Bio-lubricant Properties Analysis of Drilling an Innovative Design of Bioactive Kinetic Screw into Bone. Designs 2023, 7, 21. https://doi.org/10.3390/designs7010021
Andreucci CA, Fonseca EMM, Jorge RN. Bio-lubricant Properties Analysis of Drilling an Innovative Design of Bioactive Kinetic Screw into Bone. Designs. 2023; 7(1):21. https://doi.org/10.3390/designs7010021
Chicago/Turabian StyleAndreucci, Carlos Aurelio, Elza M. M. Fonseca, and Renato N. Jorge. 2023. "Bio-lubricant Properties Analysis of Drilling an Innovative Design of Bioactive Kinetic Screw into Bone" Designs 7, no. 1: 21. https://doi.org/10.3390/designs7010021
APA StyleAndreucci, C. A., Fonseca, E. M. M., & Jorge, R. N. (2023). Bio-lubricant Properties Analysis of Drilling an Innovative Design of Bioactive Kinetic Screw into Bone. Designs, 7(1), 21. https://doi.org/10.3390/designs7010021