Determination of a High-Power THz Detector for EA-FEL Radiation Using Optical Sampling of GaAs and ZnTe Crystals
Abstract
1. Introduction
2. Materials and Methods
Electrostatic Accelerator Free-Electron Lasers
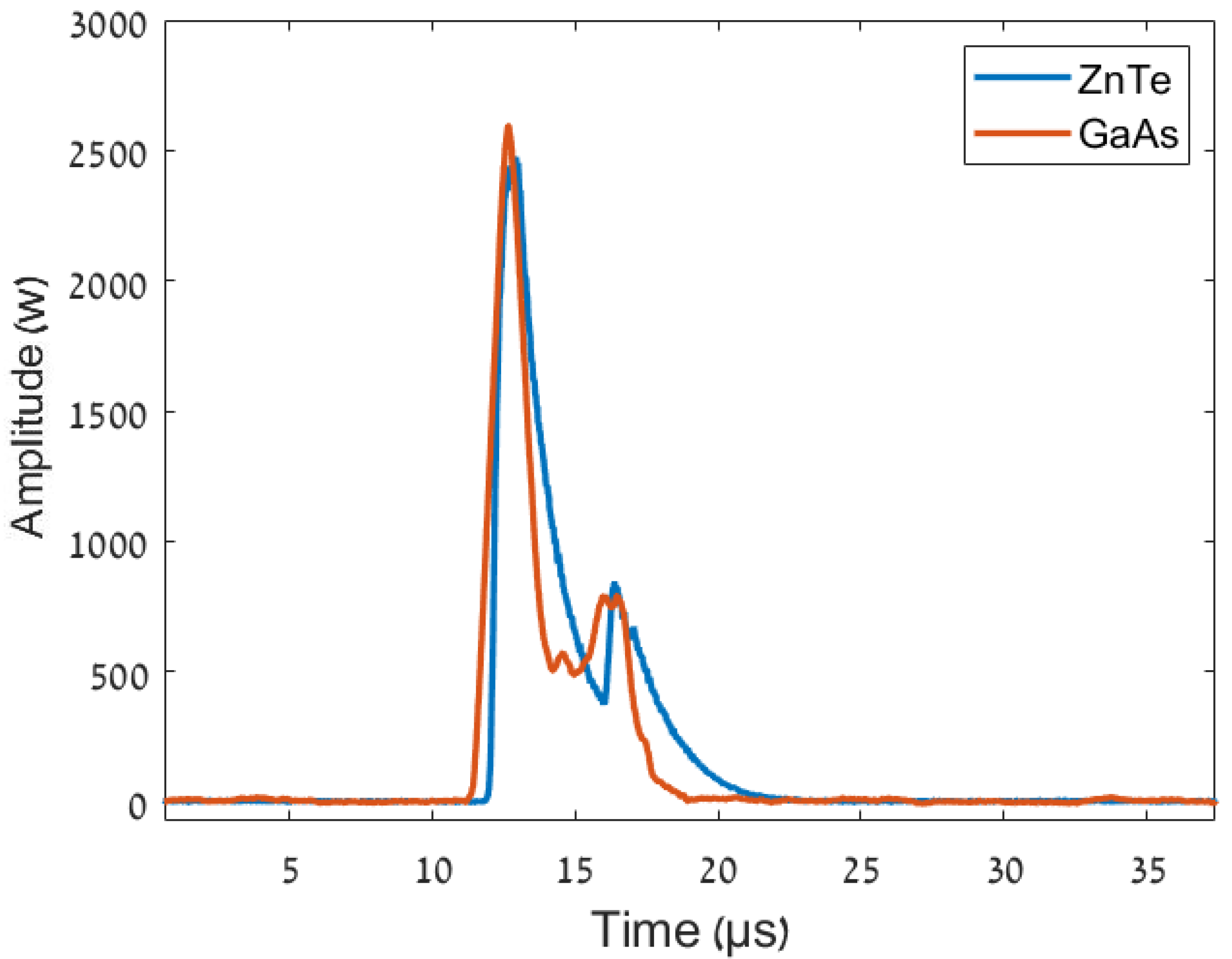
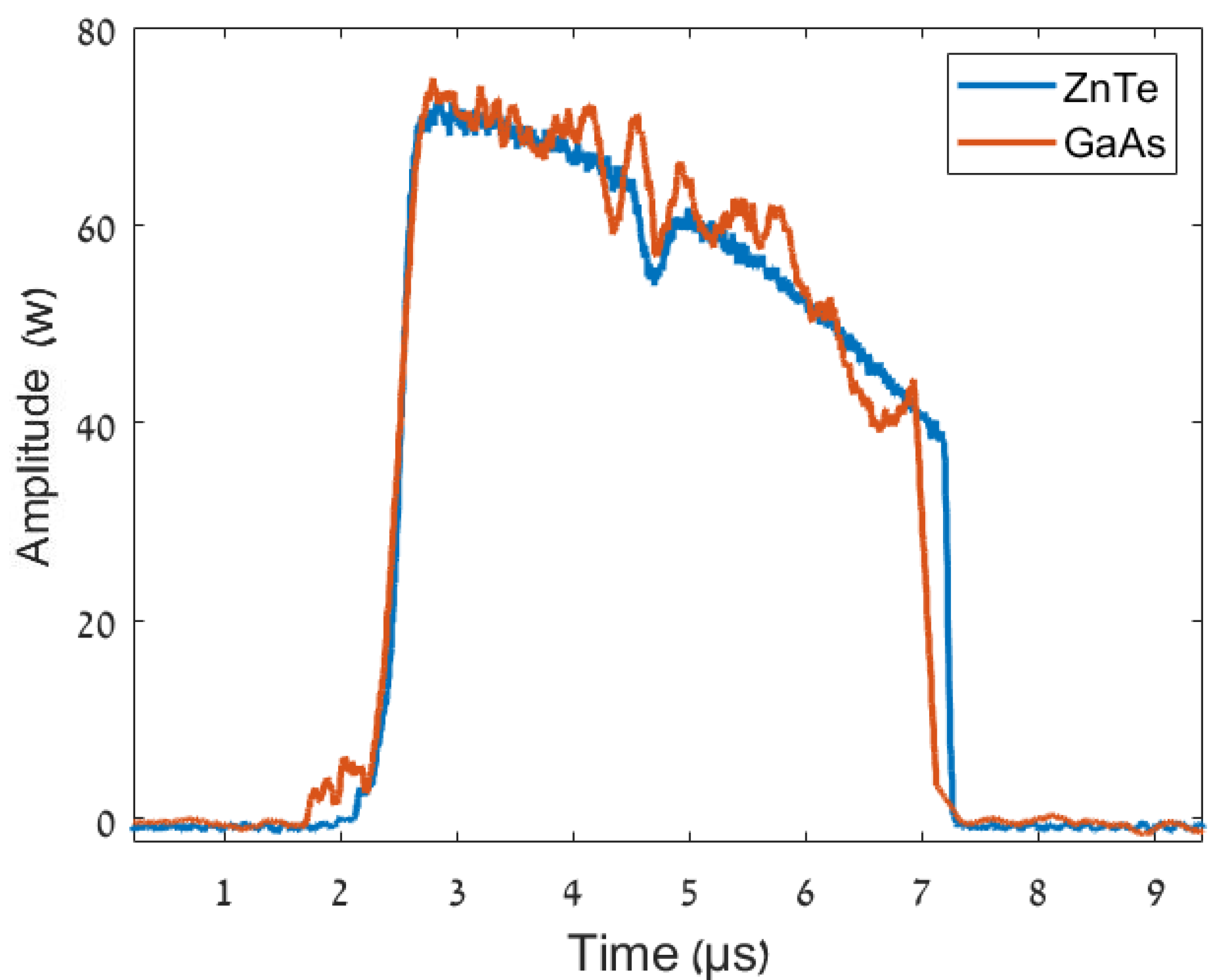
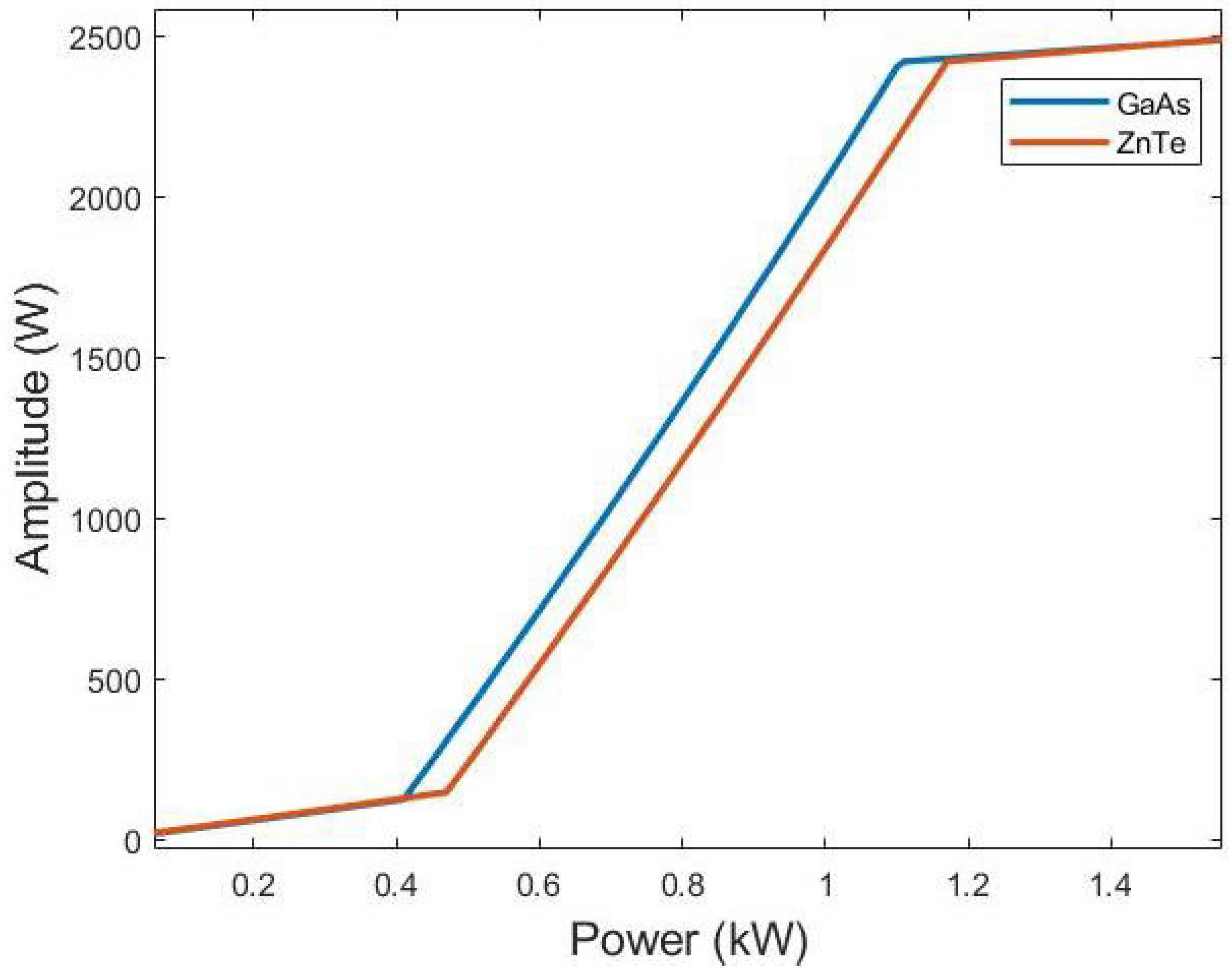
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ashish, Y.; Deepak, D. Terahertz technology and its applications. Drug Invent. Today 2013, 5, 157–163. [Google Scholar]
- Hafez1, H.; Chai, X.; Ibrahim, A. Intense terahertz radiation and their applications. J. Opt. 2016, 18, 093004. [Google Scholar] [CrossRef]
- Gabriel, M. Millimeter-Wave and Terahertz Integrated Circuit Antennas. Proc. IEEE 1992, 80, 1748–1770. [Google Scholar]
- Haj Yahya, A.; Avi, K. Comparison between up-conversion detection in glow-discharge detectors and the Schottky diode for MMW/THz high-power single pulse. Appl. Sci. 2021, 11, 4172. [Google Scholar] [CrossRef]
- Rogalski, A.; Sizov, F. Terahertz detectors and focal plane arrays. Opt. Electron. Rev. 2011, 19, 346–404. [Google Scholar] [CrossRef]
- Hou, L.; Hi, W. Fast THz continuous-wave detector based on weakly ionized plasma. IEEE Electron. Device Lett. 2012, 52, 1583–1585. [Google Scholar] [CrossRef]
- Jiang, Z.; Zhang, X.-C. Terahertz imaging via electro-optic effect. IEEE Trans. Microw. Theory Tech. 1999, 47, 2644–2650. [Google Scholar] [CrossRef]
- Pradarutti, B.; Matthäus, G. Highly efficient terahertz electro-optic sampling by material optimization at 1060 nm. Opt. Commun. 2008, 281, 5031–5035. [Google Scholar] [CrossRef]
- Wu, Q.; Zhanga, X. Free-space electro-optic sampling of terahertz beams. Appl. Phys. Lett. 1995, 67, 3523–3525. [Google Scholar] [CrossRef]
- Zhao, G.; Schouten, R.N. Design and performance of a THz emission and detection setup based on a semi-insulating GaAs emitter. Rev. Sci. Instrum. 2002, 73, 1715–1719. [Google Scholar] [CrossRef]
- Dexheimer, S.L. Principles and Applications. In Terahertz Spectroscopy, 1st ed.; CRC Press Taylor and Francis Group: Boca Raton, FL, USA, 2008; p. 360. [Google Scholar]
- Sabine, K.; Shawn, S. Electro-optic sampling of near-infrared waveforms. Nat. Photonics 2016, 10, 159–163. [Google Scholar]
- Tomasino, A.; Parisi, A. Wideband THz Time Domain Spectroscopy based on Optical Rectification and Electro-Optic Sampling. Sci. Rep. 2013, 3, 3116. [Google Scholar] [CrossRef] [PubMed]
- Bang, W.; Lei, C. Comparison of the detection performance of three nonlinear crystals for the electro-optic sampling of a FEL-THz source. In Proceedings of the 5th International Particle Accelerator Conference (IPAC 2014), Dresden, Germany, 15–20 June 2014; Volume 17, pp. 2891–2893. [Google Scholar]
- Xie, X.; Xu, J.; Zhang, X.-C. Terahertz wave generation and detection from a CdTe crystal characterized by different excitation wavelengths. Opt. Lett. 2006, 31, 978–980. [Google Scholar] [CrossRef] [PubMed]
- Kodo, K.; Manabu, S. Unidirectional radiation of widely tunable THz wave using a prism coupler under noncollinear phase matching condition. Appl. Phys. Lett. 1997, 71, 753–755. [Google Scholar]
- Ajay, N.; Aniruddha, S.W. A wideband coherent terahertz spectroscopy system using optical rectification and electro-optic sampling. Appl. Phys. Lett. 1996, 69, 2321–2323. [Google Scholar]
- Masaya, N.; Koichiro, T. Generation and detection of terahertz radiation by electro-optical process in GaAs using 1.56 mm fiber laser pulses. Appl. Phys. Lett. 2004, 85, 3974–3976. [Google Scholar]
- Marks, H.; Gover, A.; Lurie, Y. Enhancement Of Radiative Energy Extraction In An FEL Oscillartor by Post-Saturation Beam Energy Ramping. In Proceedings of the 38th Interntional Free Elctron Laser Conference, Santa Fe, NM, USA, 20–25 August 2017; pp. 244–246. [Google Scholar]
- Haj Yahya, A.; Avi, K. Improvement of the electro-optical process in GaAs for terahertz single pulse detection by using a fiber-coupling system. Appl. Sci. 2021, 11, 6859. [Google Scholar] [CrossRef]
- Marks, H.S.; Gover, A. Radiation Power Out-Coupling Optimization of a Free Electron Laser Oscillator. IEEE Trans. Microw. Theory Tech. 2016, 64, 1006–1014. [Google Scholar] [CrossRef]
- Liu, S.-G.; Liu, H.-X. Electrostatic free-electron laser. Phys. Rev. A 1987, 36, 3837. [Google Scholar] [CrossRef]
- Marks, M.; Borodin, D. Power and spectral evolution of a Free Electron Laser oscillator with electron beam energy ramping. Nuclear Inst. Methods Phys. Res. A 2021, 1004, 165376. [Google Scholar] [CrossRef]
- Marks, M.; Gover, A. Talbot Effect mm-Wave Resonator for an Electrostatic Accelerator Free Electron Laser. IEEE Trans. Microw. Theory Tech. 2018, 66, 3–10. [Google Scholar] [CrossRef]
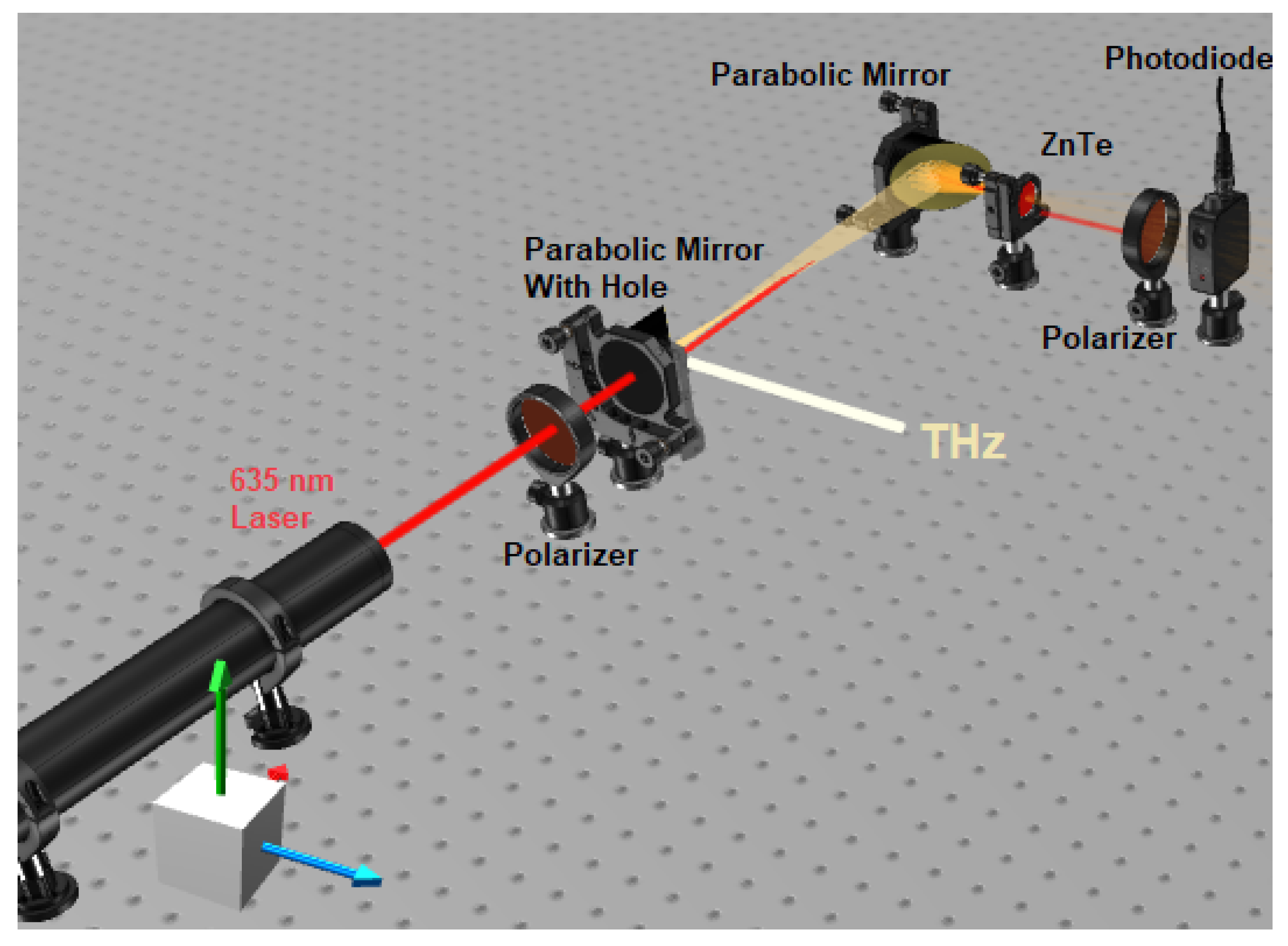
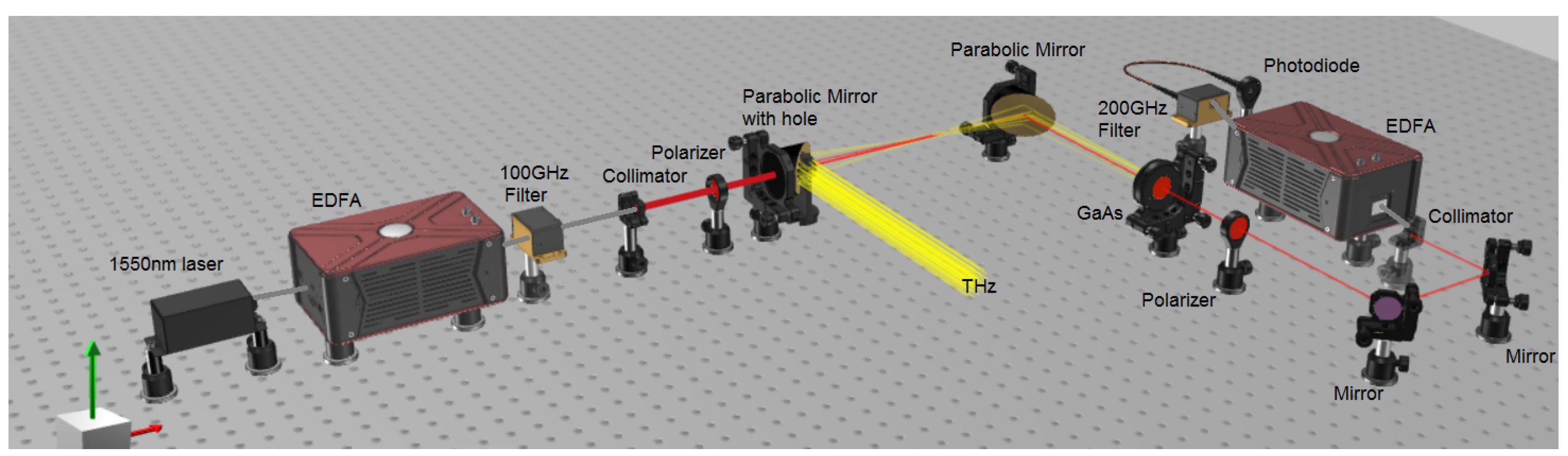
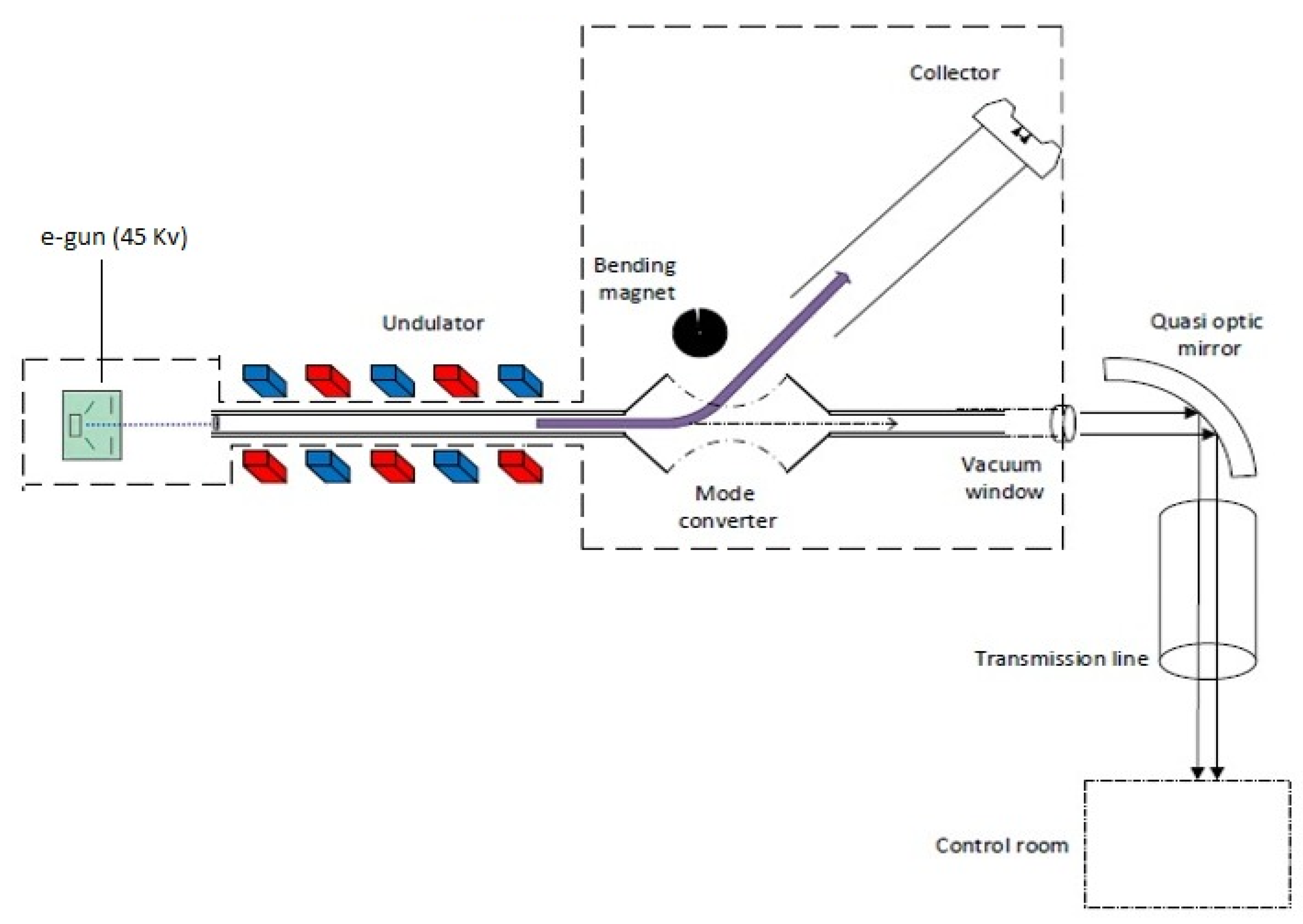
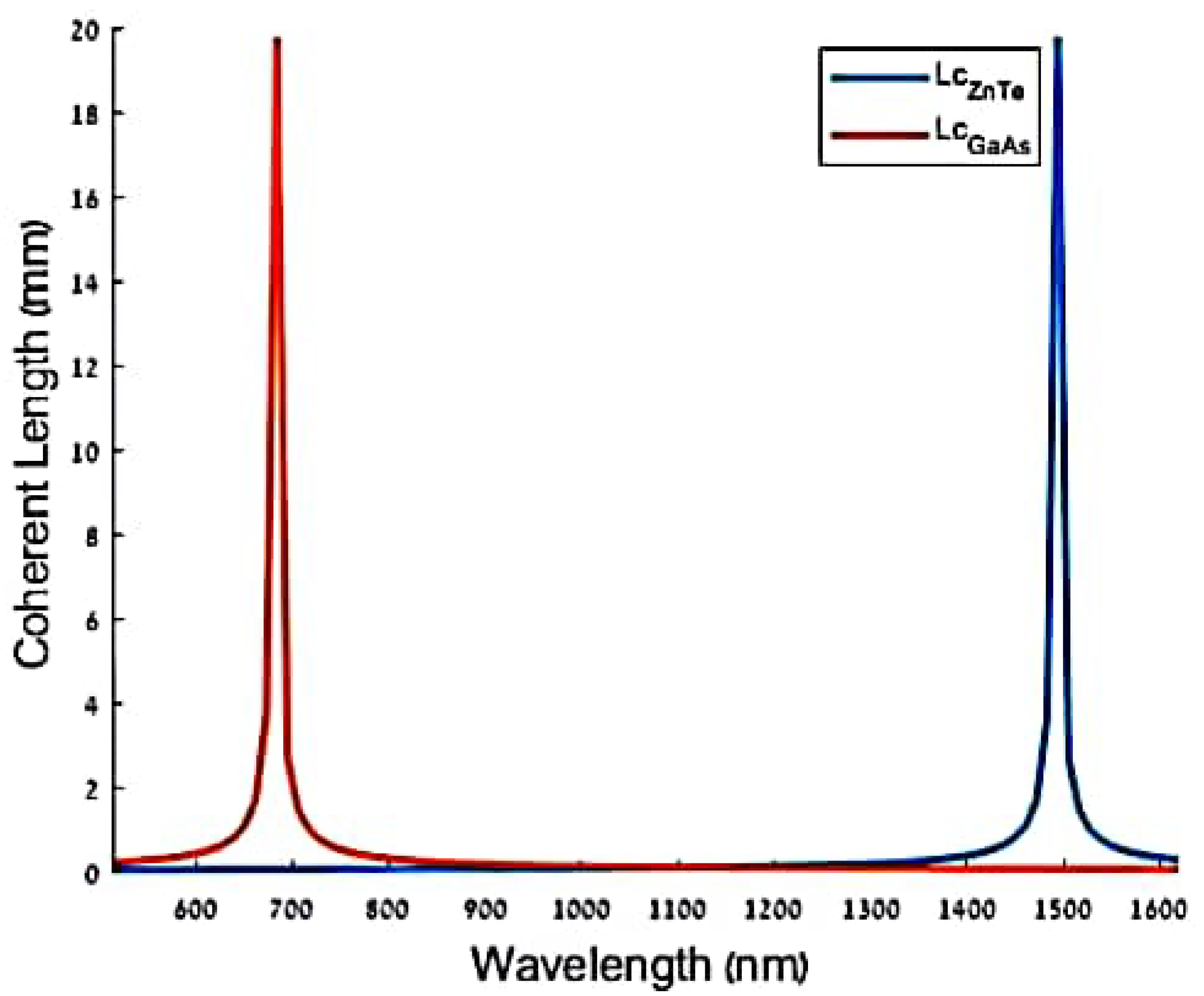
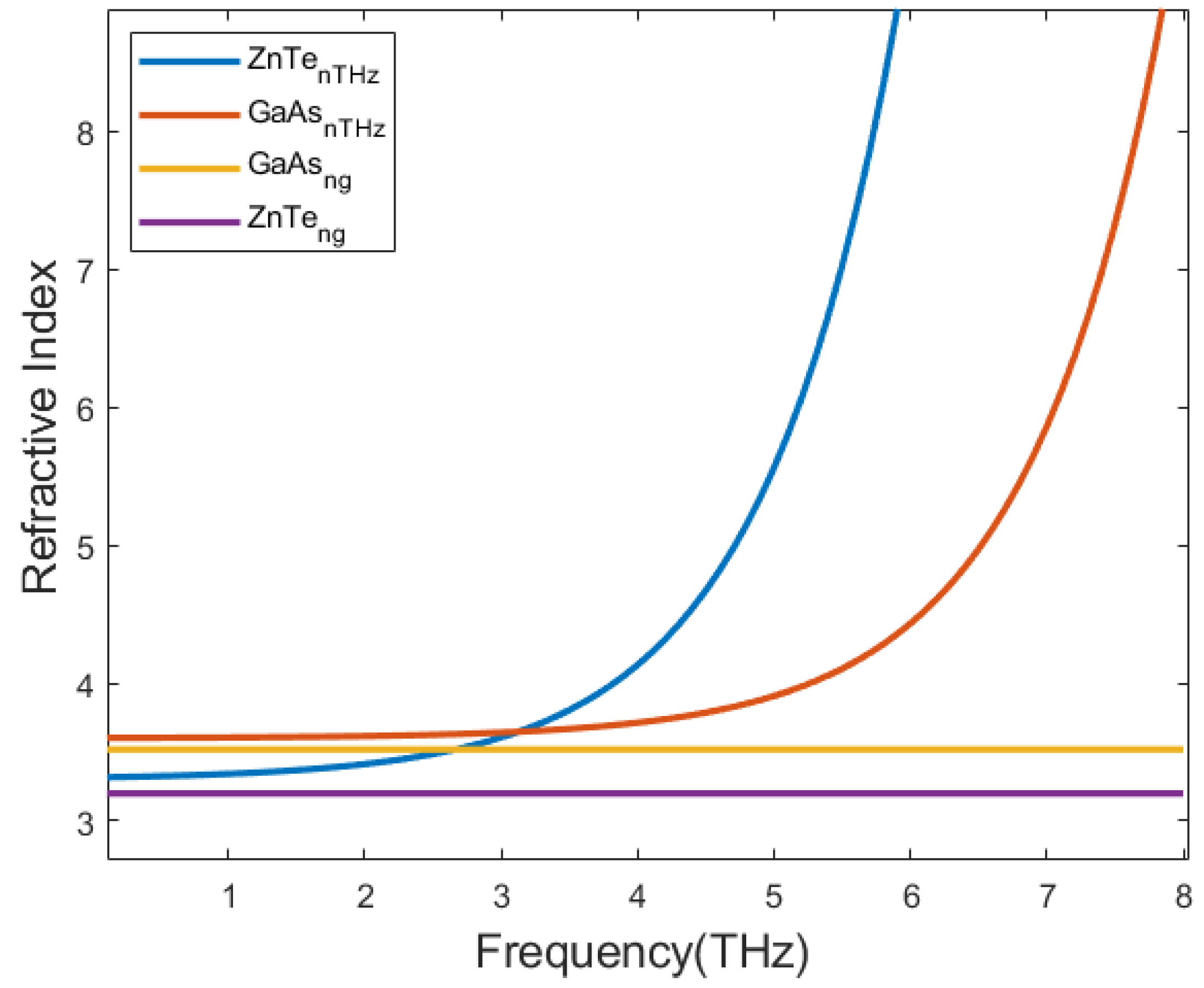
| Parameter 1 | ZnTe | GaAs | GaP |
|---|---|---|---|
| A | 4.27 | 8.95 | 2.680 |
| B | 3.01 | 2.054 | 6.40 |
| D | 0.142 | 0.39 | 0.090327 |
| Parameter | Value |
|---|---|
| Radiation Frequency | 95–110 GHz |
| Beam Energy | 1.35–1.45 MeV |
| Beam Current | 0.7–3 A |
| Free Spectral Range | 100 MHz |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haj Yahya, A.; Gerasimov, M.; Ciplis, J.; Balal, N.; Friedman, A. Determination of a High-Power THz Detector for EA-FEL Radiation Using Optical Sampling of GaAs and ZnTe Crystals. Designs 2022, 6, 109. https://doi.org/10.3390/designs6060109
Haj Yahya A, Gerasimov M, Ciplis J, Balal N, Friedman A. Determination of a High-Power THz Detector for EA-FEL Radiation Using Optical Sampling of GaAs and ZnTe Crystals. Designs. 2022; 6(6):109. https://doi.org/10.3390/designs6060109
Chicago/Turabian StyleHaj Yahya, Adnan, Michael Gerasimov, Johnathan Ciplis, Nezah Balal, and Aharon Friedman. 2022. "Determination of a High-Power THz Detector for EA-FEL Radiation Using Optical Sampling of GaAs and ZnTe Crystals" Designs 6, no. 6: 109. https://doi.org/10.3390/designs6060109
APA StyleHaj Yahya, A., Gerasimov, M., Ciplis, J., Balal, N., & Friedman, A. (2022). Determination of a High-Power THz Detector for EA-FEL Radiation Using Optical Sampling of GaAs and ZnTe Crystals. Designs, 6(6), 109. https://doi.org/10.3390/designs6060109








