Design and Analysis of Porous Functionally Graded Femoral Prostheses with Improved Stress Shielding
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Design Methodology of the Femur Bone
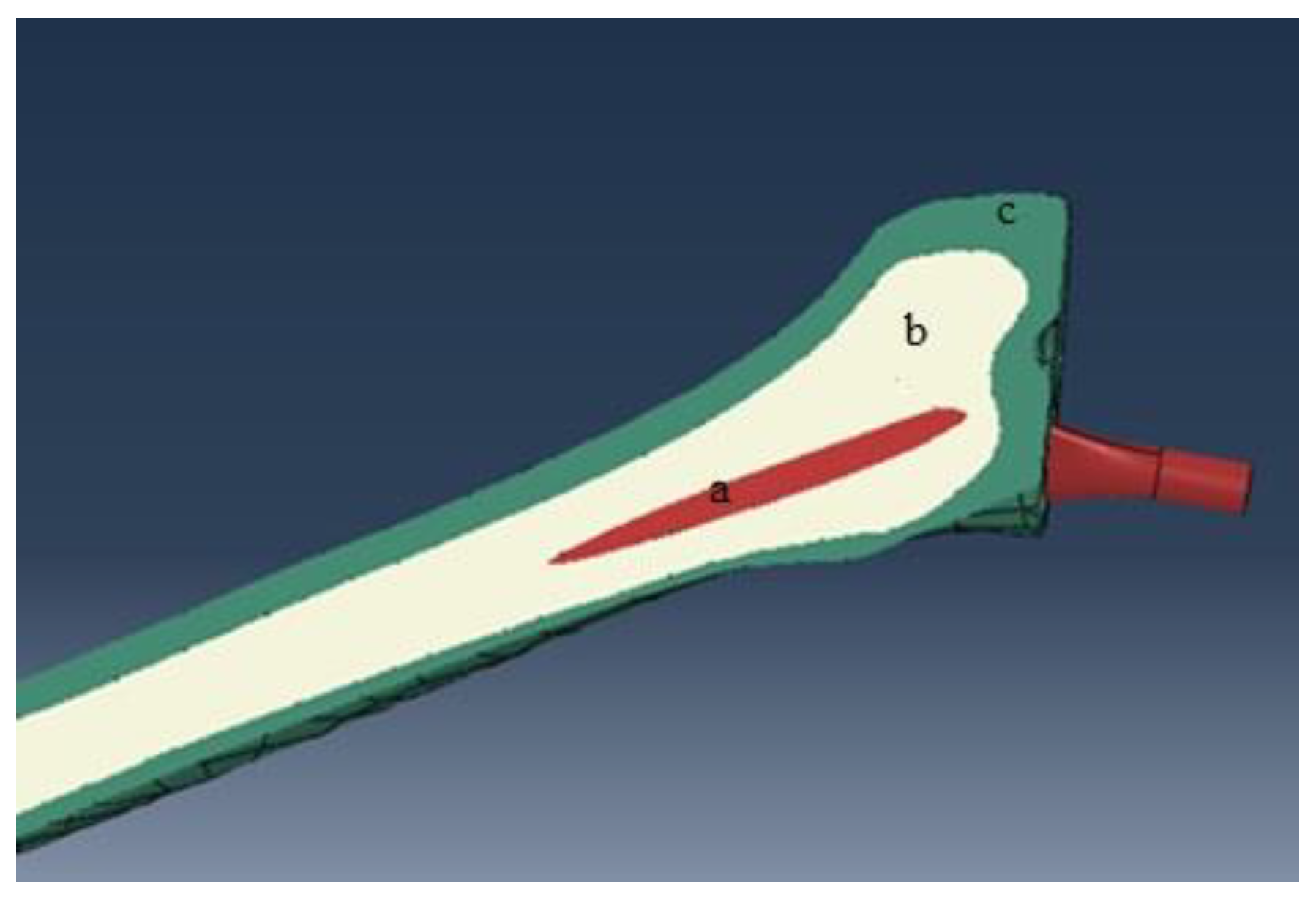
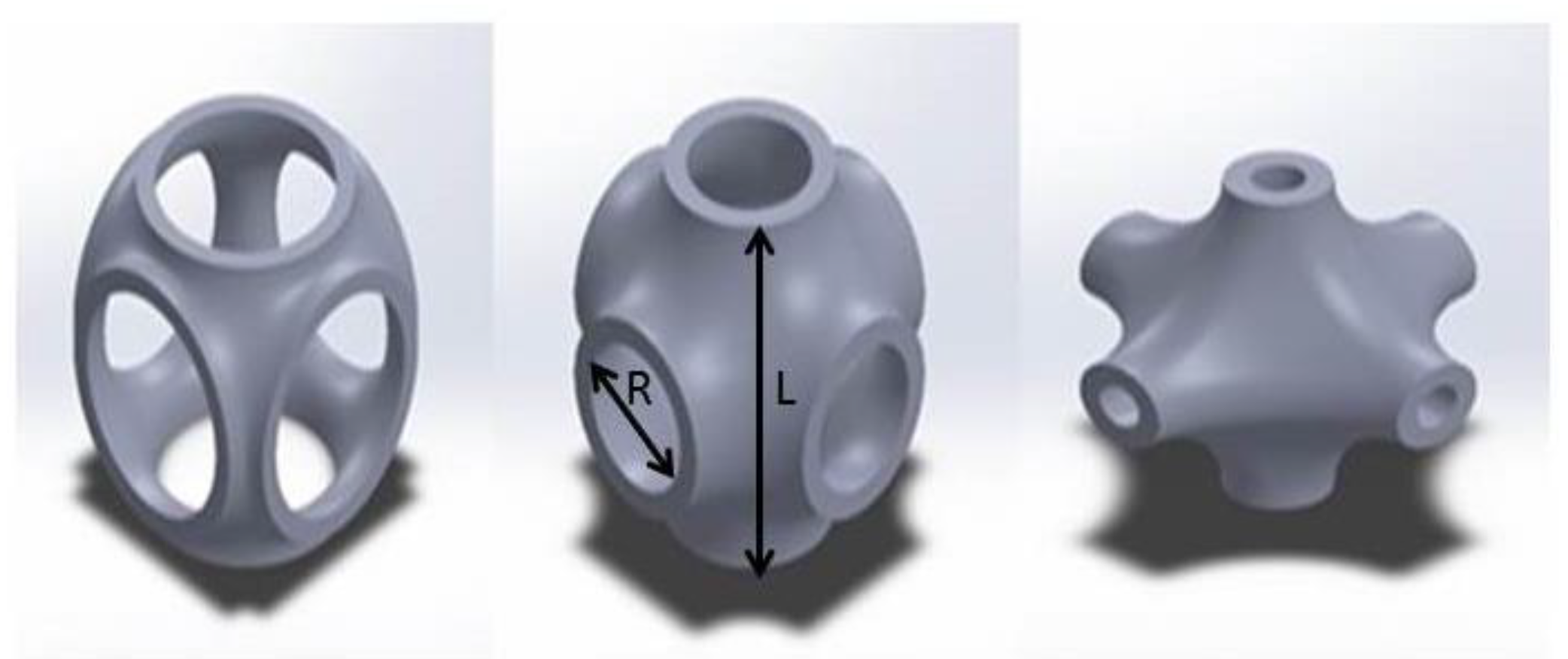
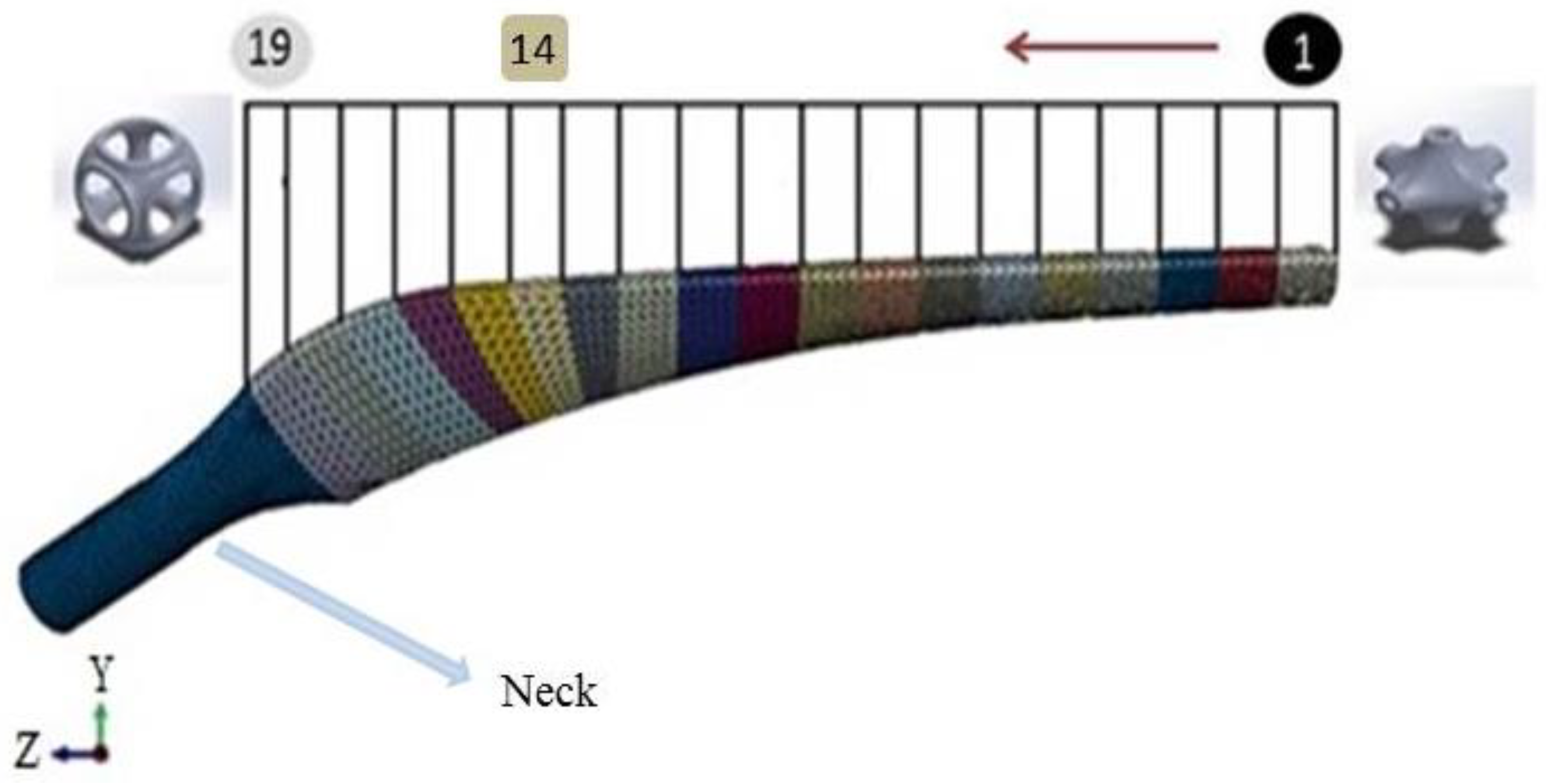
2.3. Designing of a Unit Cell and Implant
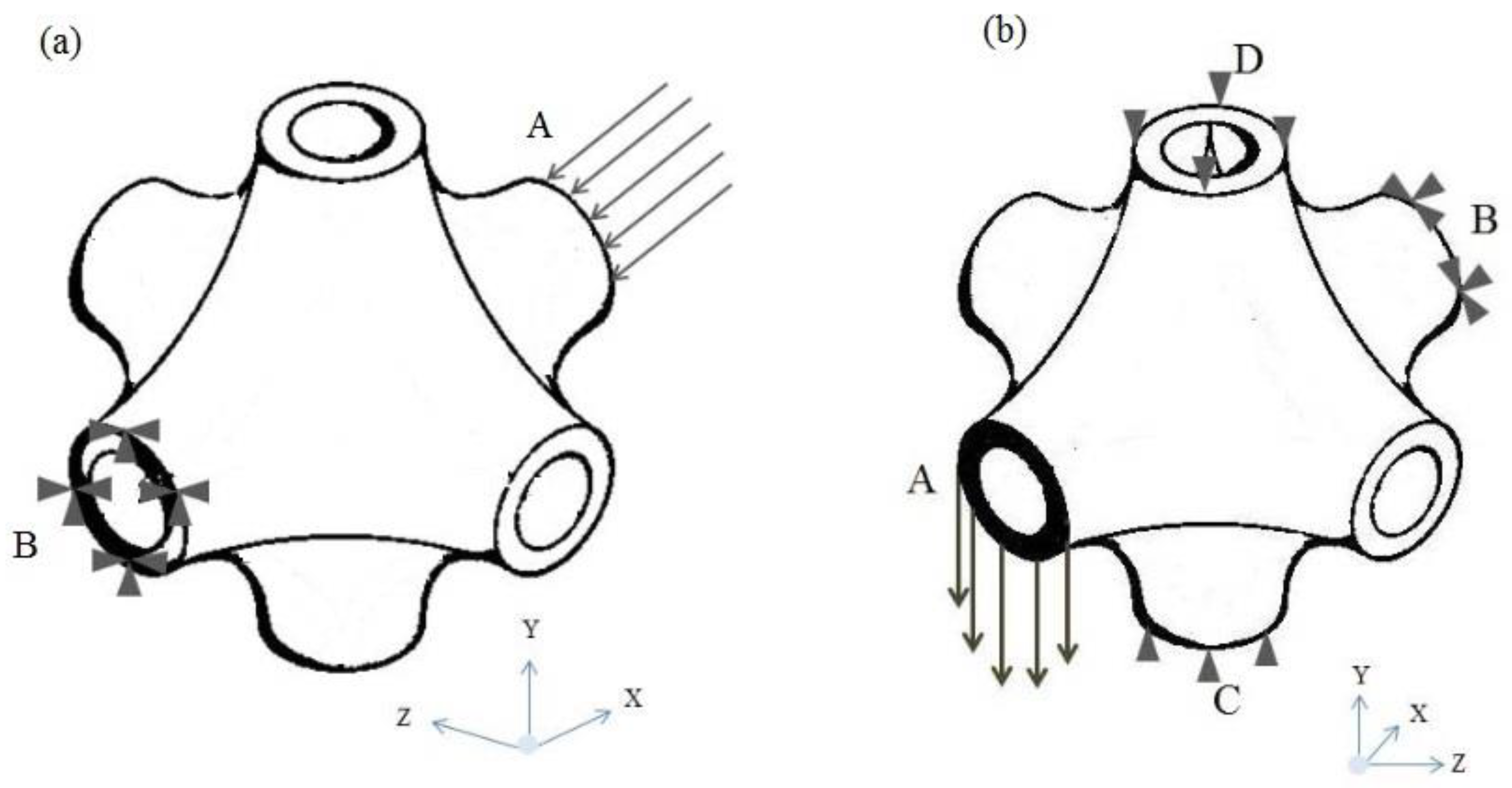
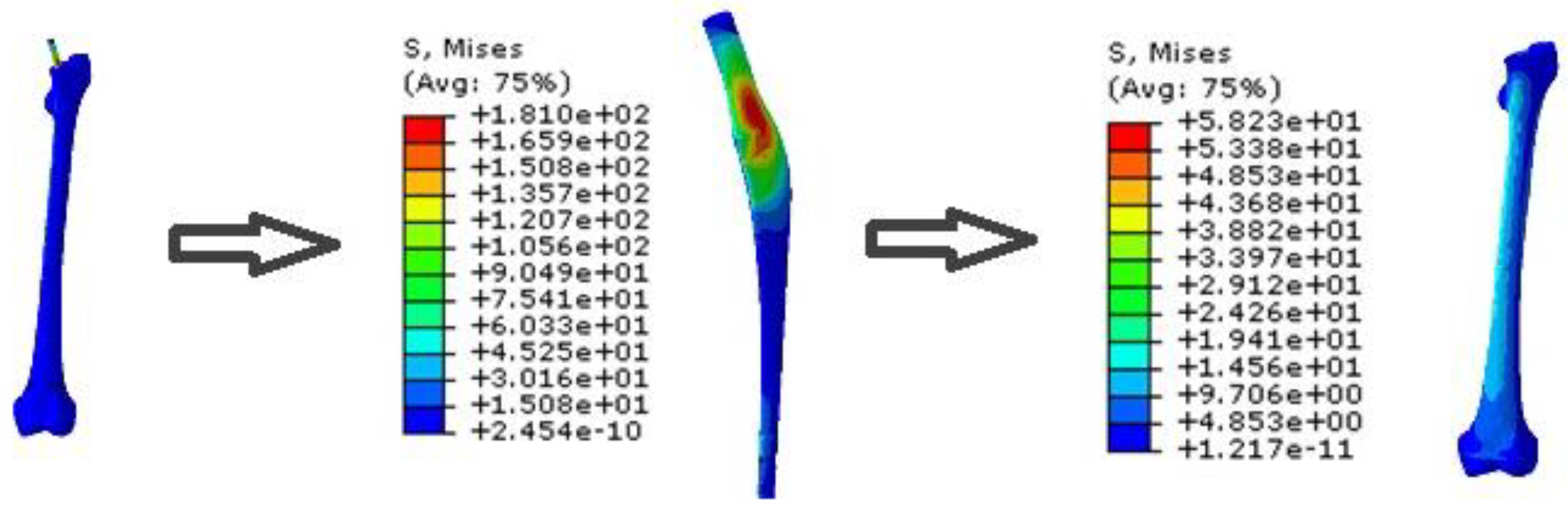
2.4. Finite Element Model and Boundary Conditions
3. Results and Discussion
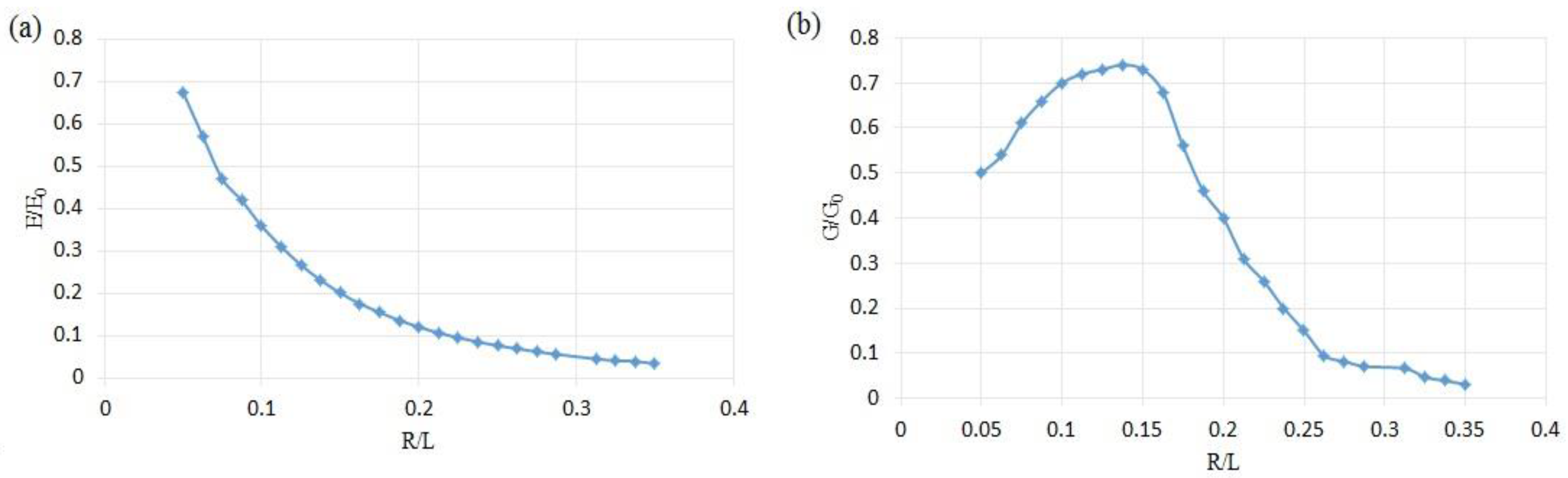
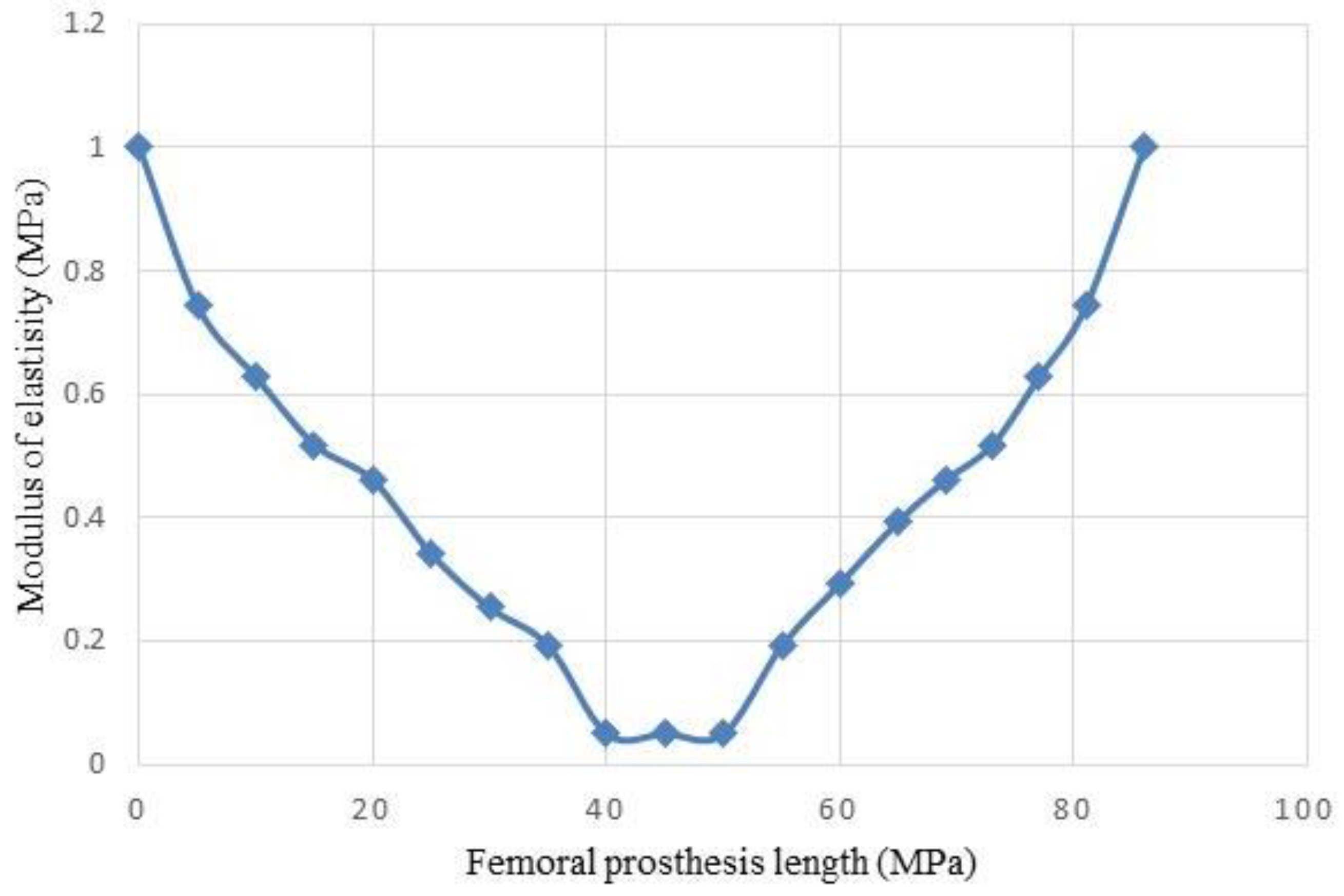
3.1. Mechanical Properties of the SP Unit Cells
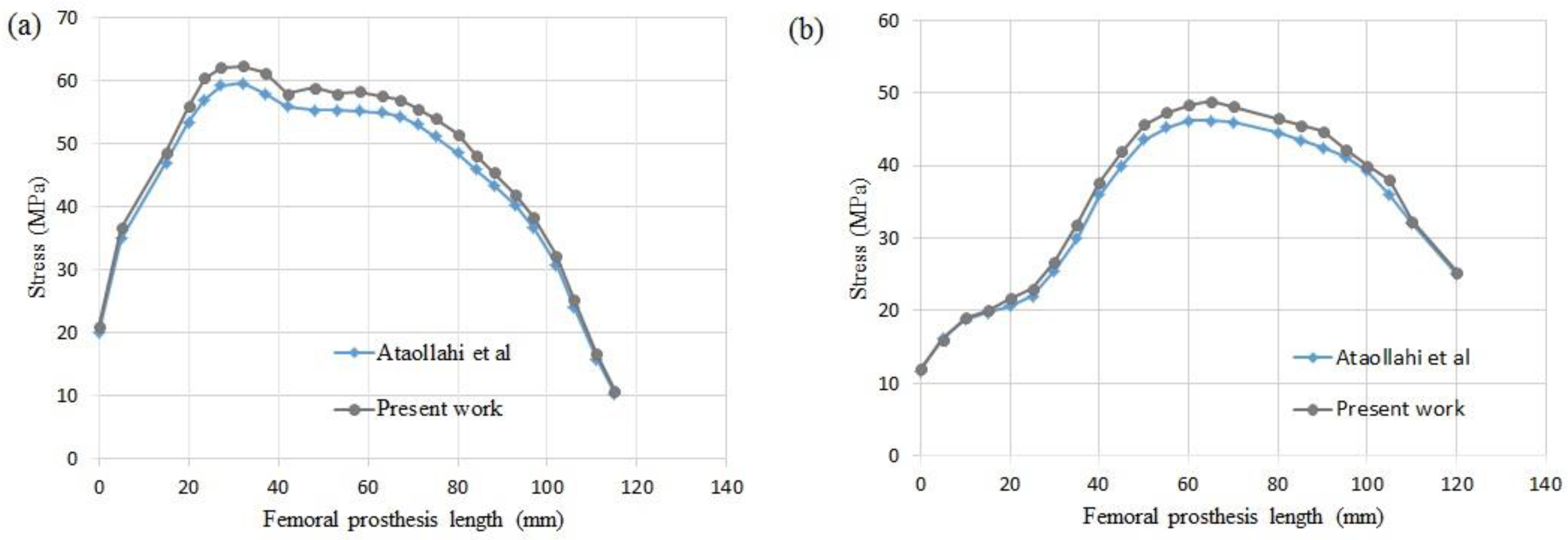
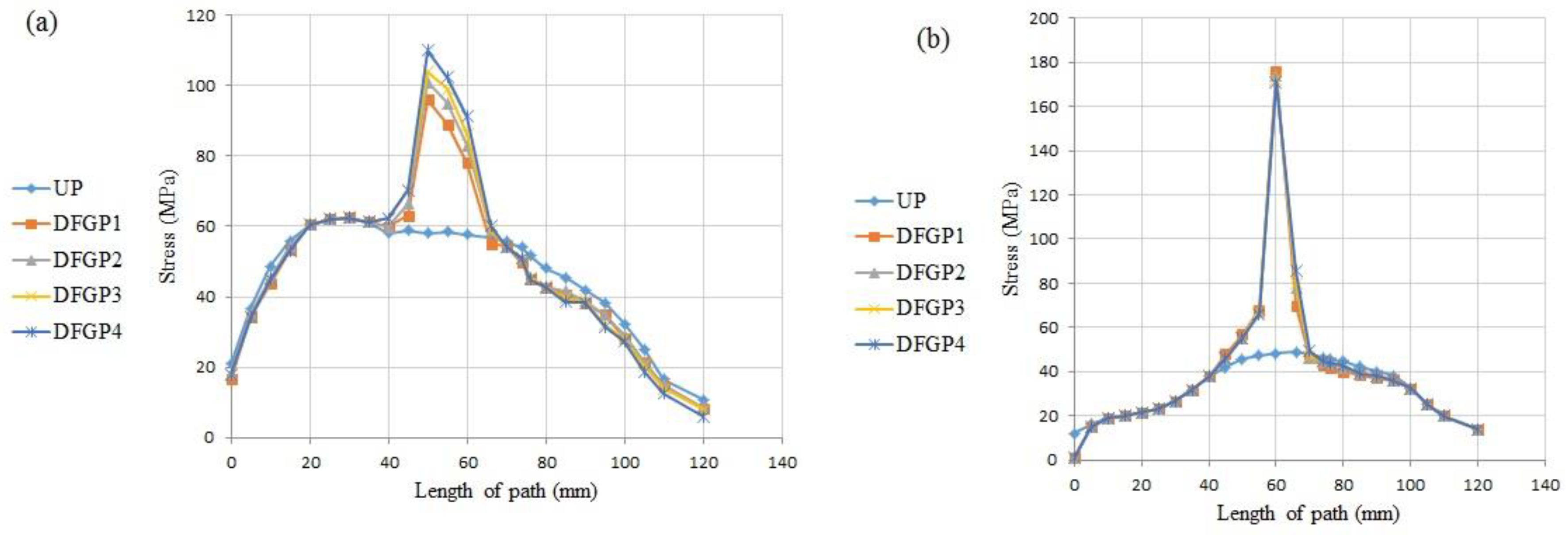
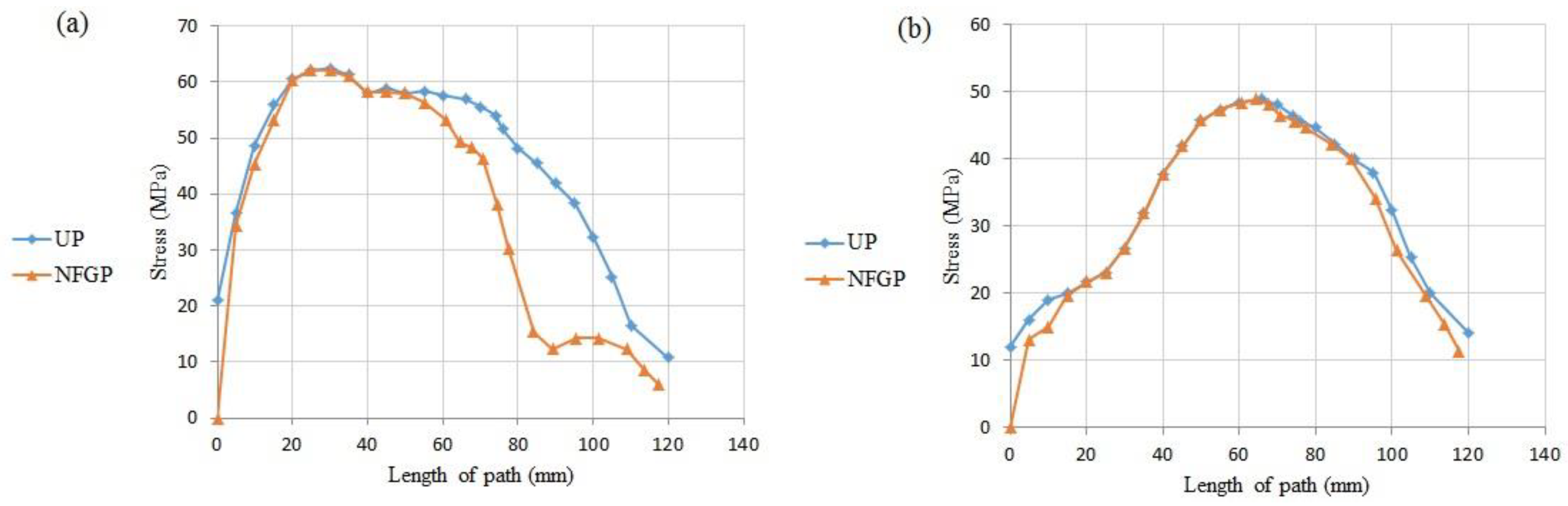
3.2. Investigation of the Bone and Prosthesis with Different Topologies
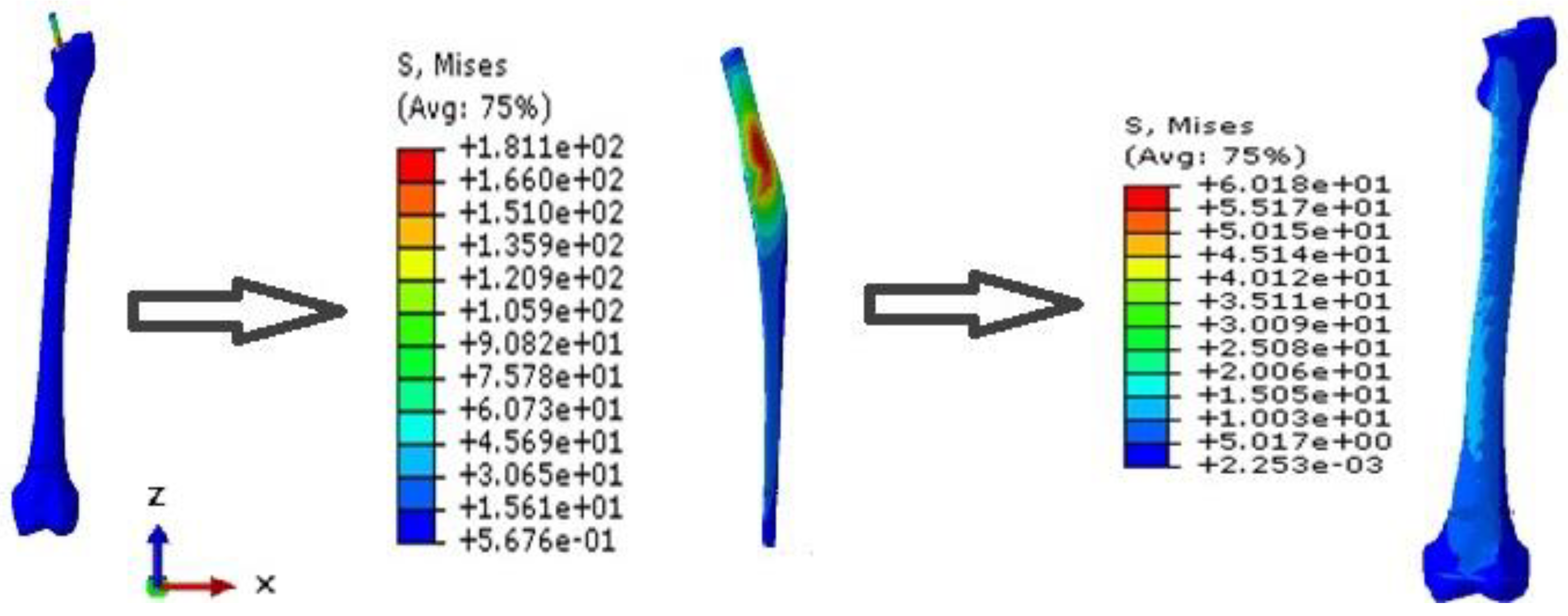
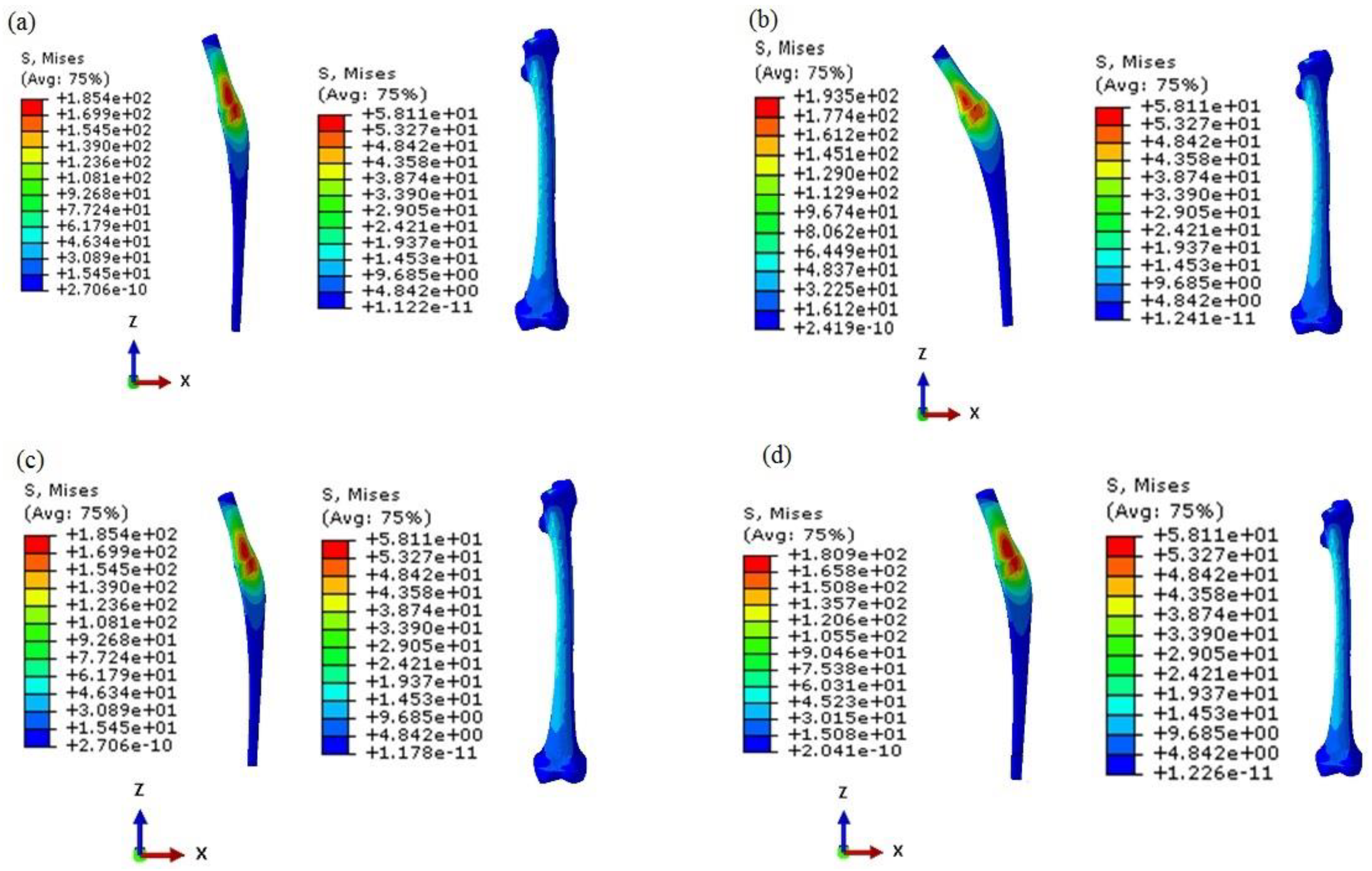
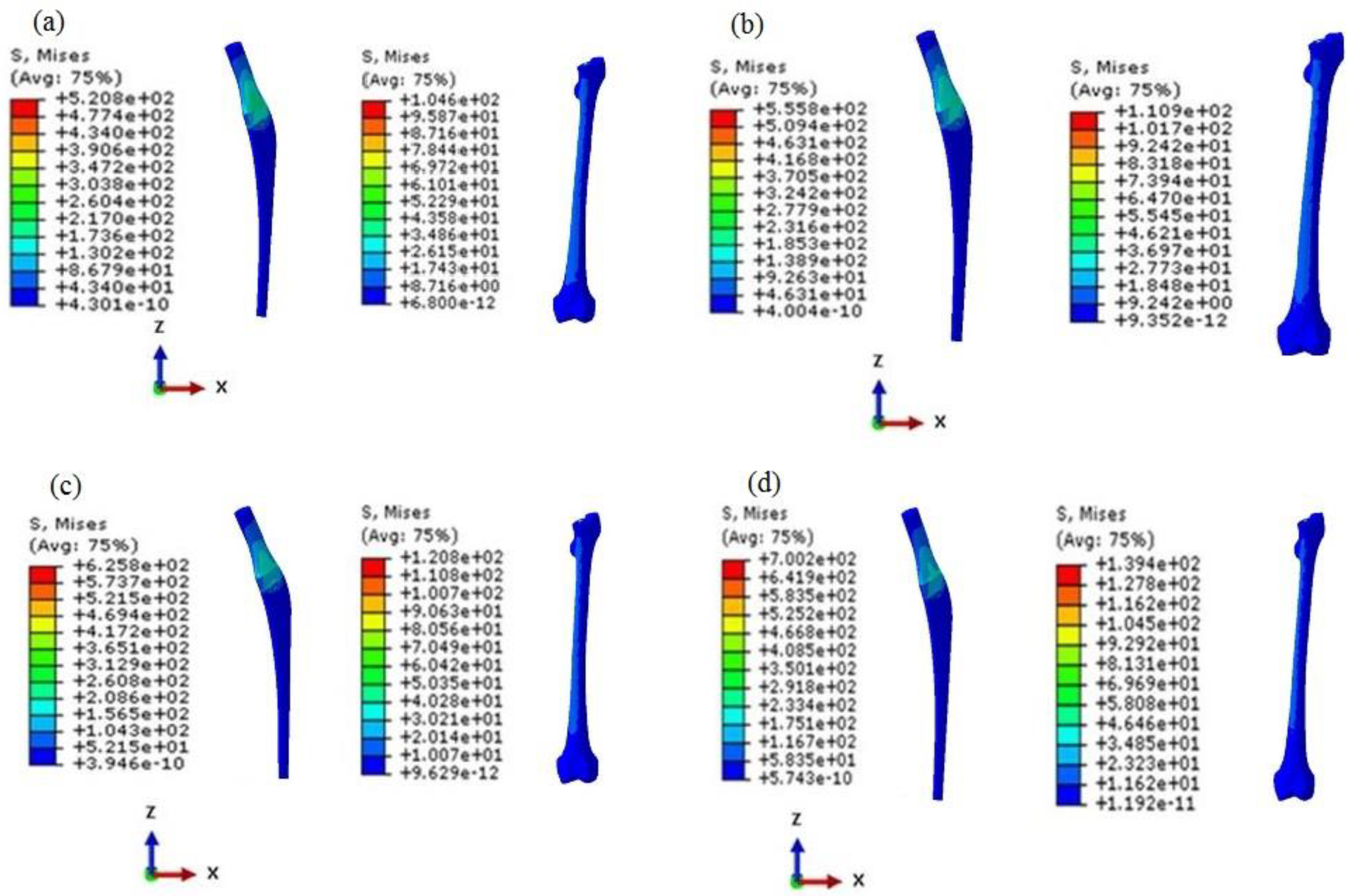
3.2.1. Uniform Prosthesis
3.2.2. Increasing FG Prosthesis
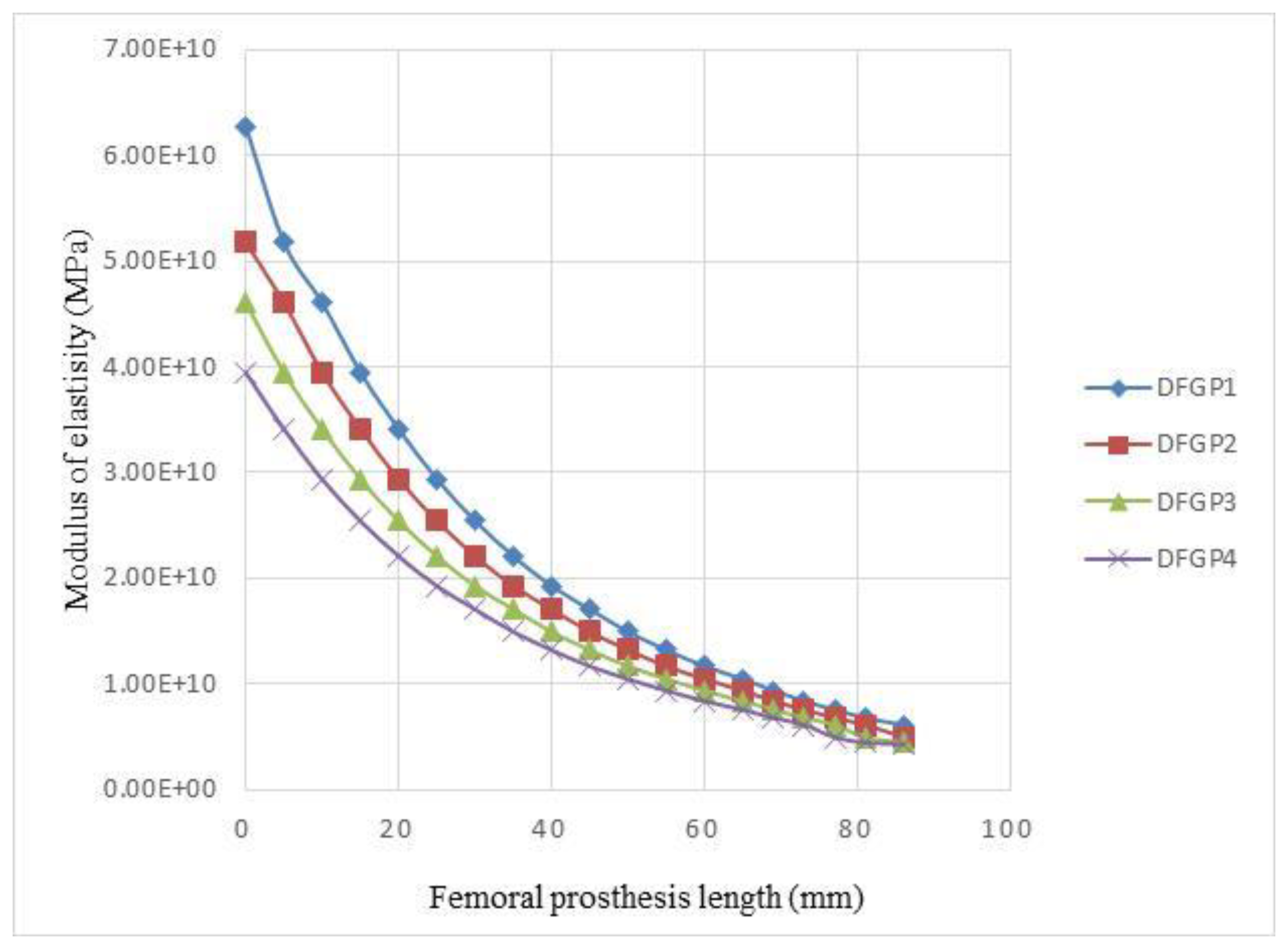
3.2.3. Decreasing FG Prosthesis
3.2.4. Neutral FG Prosthesis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Tarlochan, F.; Mehboob, H.; Mehboob, A.; Chang, S.-H. Influence of functionally graded pores on bone ingrowth in cementless hip prosthesis: A finite element study using mechano-regulatory algorithm. Biomech. Model. Mechanobiol. 2017, 17, 701–716. [Google Scholar] [CrossRef] [PubMed]
- Alkhatib, S.E.; Tarlochan, F.; Mehboob, H.; Singh, R.; Kadirgama, K.; Harun, W.S.B.W. Finite element study of functionally graded porous femoral stems incorporating body-centered cubic structure. Artif. Organs 2019, 43, E152–E164. [Google Scholar] [CrossRef] [PubMed]
- Şensoy, A.T.; Çolak, M.; Kaymaz, I.; Findik, F. Optimal material selection for total hip implant: A finite element case study. Arab. J. Sci. Eng. 2019, 44, 10293–10301. [Google Scholar] [CrossRef]
- Hung, J.-P.; Bai, Y.-W.; Hung, C.-Q.; Lee, T.-E. Biomechanical performance of the cemented hip stem with different surface finish. Appl. Sci. 2019, 9, 4082. [Google Scholar] [CrossRef]
- Sola, A.; Bellucci, D.; Cannillo, V. Functionally graded materials for orthopedic applications–an update on design and manufacturing. Biotechnol. Adv. 2016, 34, 504–531. [Google Scholar] [CrossRef] [PubMed]
- Arabnejad, S.; Johnston, B.; Tanzer, M.; Pasini, D. Fully porous 3D printed titanium femoral stem to reduce stress-shielding following total hip arthroplasty. J. Orthop. Res. 2017, 35, 1774–1783. [Google Scholar] [CrossRef]
- Castro, A.; Completo, A.; Simões, J.; Flores, P. Modelling and simulation of alternative designs for the femur–implant interface of Journey patellofemoral prosthesis. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2019, 233, 1619–1628. [Google Scholar] [CrossRef]
- Cheruvu, B.; Venkatarayappa, I.; Goswami, T. Stress shielding in cemented hip implants assessed from computed tomography. Biomed. J. Sci. Tech. Res. 2019, 18, 13637–13641. [Google Scholar]
- Bougherara, H.; Bureau, M.; Campbell, M.; Vadean, A.; Yahia, L.H. Design of a biomimetic polymer-composite hip prosthesis. J. Biomed. Mater. Res. Part A 2007, 82, 27–40. [Google Scholar] [CrossRef]
- Rezaei, F.; Hassani, K.; Solhjoei, N.; Karimi, A. Carbon/PEEK composite materials as an alternative for stainless steel/titanium hip prosthesis: A finite element study. Australas. Phys. Eng. Sci. Med. 2015, 38, 569–580. [Google Scholar] [CrossRef]
- Naidubabu, Y.; Mohana Rao, G.; Rajasekhar, K.; Ratna Sunil, B. Design and simulation of polymethyl methacrylate-titanium composite bone fixing plates using finite element analysis: Optimizing the composition to minimize the stress shielding effect. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2017, 231, 4402–4412. [Google Scholar] [CrossRef]
- Enab, T. Behavior of FGM-coated, HA-coated and uncoated femoral prostheses with different geometrical configurations. Int. J. Mech. Mechatron. Eng. 2016, 16, 198949870. [Google Scholar]
- Oshkour, A.; Osman, N.A.; Bayat, M.; Afshar, R.; Berto, F. Three-dimensional finite element analyses of functionally graded femoral prostheses with different geometrical configurations. Mater. Des. 2014, 56, 998–1008. [Google Scholar] [CrossRef]
- Shirzad, M.; Fathi, A.; Rabiee, S.M.; Ghaffari, S.; Zabihi-Neishabouri, E. Three-dimensional printing of truss-like structure for use in scaffold: Experimental, numerical, and analytical analyses. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2020. [Google Scholar] [CrossRef]
- Pompe, W.; Worch, H.; Epple, M.; Friess, W.; Gelinsky, M.; Greil, P.; Hempel, U.; Scharnweber, D.; Schulte, K. Functionally graded materials for biomedical applications. Mater. Sci. Eng. A 2003, 362, 40–60. [Google Scholar] [CrossRef]
- Hambli, R.; Hattab, N. Application of neural network and finite element method for multiscale prediction of bone fatigue crack growth in cancellous bone. In Multiscale Computer Modeling in Biomechanics and Biomedical Engineering; Springer: Berlin/Heidelberg, Germany, 2013; pp. 3–30. [Google Scholar]
- Mehrali, M.; Shirazi, F.S.; Mehrali, M.; Metselaar, H.S.C.; Kadri, N.A.B.; Osman, N.A.A. Dental implants from functionally graded materials. J. Biomed. Mater. Res. Part A 2013, 101, 3046–3057. [Google Scholar] [CrossRef] [PubMed]
- Mahmoudi, M.; Saidi, A.R.; Hashemipour, M.A.; Amini, P. The use of functionally graded dental crowns to improve biocompatibility: A finite element analysis. Comput. Methods Biomech. Biomed. Eng. 2018, 21, 161–168. [Google Scholar] [CrossRef]
- Khoo, W.; Chung, S.M.; Lim, S.C.; Low, C.Y.; Shapiro, J.M.; Koh, C.T. Fracture behavior of multilayer fibrous scaffolds featuring microstructural gradients. Mater. Des. 2019, 184, 108184. [Google Scholar] [CrossRef]
- Loh, G.H.; Pei, E.; Harrison, D.; Monzon, M.D. An overview of functionally graded additive manufacturing. Addit. Manuf. 2018, 23, 34–44. [Google Scholar] [CrossRef]
- Yang, Q.; Cao, H.; Tang, Y.; Yang, B. Out-of-plane bending of functionally graded thin plates with a circular hole. Appl. Sci. 2020, 10, 2231. [Google Scholar] [CrossRef]
- Zhang, X.-Y.; Yan, X.-C.; Fang, G.; Liu, M. Biomechanical influence of structural variation strategies on functionally graded scaffolds constructed with triply periodic minimal surface. Addit. Manuf. 2020, 32, 101015. [Google Scholar] [CrossRef]
- Zhao, M.; Liu, F.; Fu, G.; Zhang, D.Z.; Zhang, T.; Zhou, H. Improved mechanical properties and energy absorption of BCC lattice structures with triply periodic minimal surfaces fabricated by SLM. Materials 2018, 11, 2411. [Google Scholar] [CrossRef] [PubMed]
- Rahmani, R.; Antonov, M.; Kollo, L.; Holovenko, Y.; Prashanth, K.G. Mechanical behavior of ti6Al4V scaffolds filled with caSiO3 for implant applications. Appl. Sci. 2019, 9, 3844. [Google Scholar] [CrossRef]
- Kluess, D.; Soodmand, E.; Lorenz, A.; Pahr, D.; Schwarze, M.; Cichon, R.; Varady, P.A.; Herrmann, S.; Buchmeier, B.; Schröder, C.; et al. A round-robin finite element analysis of human femur mechanics between seven participating laboratories with experimental validation. Comput. Methods Biomech. Biomed. Eng. 2019, 22, 1020–1031. [Google Scholar] [CrossRef]
- Moita, J.S.; Correia, V.F.; Soares, C.M.M.; Herskovits, J. Higher-order finite element models for the static linear and nonlinear behaviour of functionally graded material plate-shell structures. Compos. Struct. 2019, 212, 465–475. [Google Scholar] [CrossRef]
- Colic, K.; Sedmak, A.; Grbovic, A.; Tatic, U.; Sedmak, S.; Djordjevic, B. Finite element modeling of hip implant static loading. Procedia Eng. 2016, 149, 257–262. [Google Scholar] [CrossRef]
- Pandithevan, P.; Prasannavenkadesan, V. Investigation on the influence of hip joint loading in peak stress during various activities. In Proceedings of the 2nd International Conference on Computer Vision & Image Processing, Jaipur, India, 27–29 September 2018; pp. 249–254. [Google Scholar]
- Andreaus, U.A.; Colloca, M.; Toscano, A. Mechanical behaviour of a prosthesized human femur: A comparative analysis between walking and stair climbing by using the finite element method. Biophys. Bioeng. Lett. 2008, 1, 2774. [Google Scholar]
- Reggiani, B.; Cristofolini, L.; Varini, E.; Viceconti, M. Predicting the subject-specific primary stability of cementless implants during pre-operative planning: Preliminary validation of subject-specific finite-element models. J. Biomech. 2007, 40, 2552–2558. [Google Scholar] [CrossRef]
- Ataollahi Oshkour, A.; Talebi, H.; Shirazi, S.; Farid, S.; Bayat, M.; Yau, Y.H.; Tarlochan, F.; Osman, A.; Azuan, N. Comparison of various functionally graded femoral prostheses by finite element analysis. Sci. World J. 2014, 807621. [Google Scholar] [CrossRef]
- Ridzwan, M.I.Z.; Shuib, S.; Hassan, A.Y.; Shokri, A.A.; Ibrahim, M.M. Problem of stress shielding and improvement to the hip implant designs: A review. J. Med. Sci. 2007, 7, 460–467. [Google Scholar]
- Zhu, S.; Wang, X.; Qin, F.; Inoue, A. A new Ti-based bulk glassy alloy with potential for biomedical application. Mater. Sci. Eng. A 2007, 459, 233–237. [Google Scholar] [CrossRef]
- Kapfer, S.C.; Hyde, S.T.; Mecke, K.; Arns, C.H.; Schröder-Turk, G.E. Minimal surface scaffold designs for tissue engineering. Biomaterials 2011, 32, 6875–6882. [Google Scholar] [CrossRef] [PubMed]
- Zhu, L.-Y.; Cheng, M.; Sun, W.-C.; Yang, J.-Q.; Li, L.; Jiang, Q. Influence of deformed primitive architecture on mechanical behavior of artificial porous meniscus. Mater. Des. 2020, 186, 108303. [Google Scholar] [CrossRef]
- Heller, M.O.; Bergmann, G.; Kassi, J.-P.; Claes, L.; Haas, N.P.; Duda, G.N. Determination of muscle loading at the hip joint for use in pre-clinical testing. J. Biomech. 2005, 38, 1155–1163. [Google Scholar] [CrossRef] [PubMed]
- Asadi-Eydivand, M.; Solati-Hashjin, M.; Fathi, A.; Padashi, M.; Osman, N.A.A. Optimal design of a 3D-printed scaffold using intelligent evolutionary algorithms. Appl. Soft Comput. 2016, 39, 36–47. [Google Scholar] [CrossRef]
- Torres-Sanchez, C.; McLaughlin, J.; Fotticchia, A. Porosity and pore size effect on the properties of sintered Ti35Nb4Sn alloy scaffolds and their suitability for tissue engineering applications. J. Alloys Compd. 2018, 731, 189–199. [Google Scholar] [CrossRef]
- Almeida, H.A.; Bártolo, P.J. Design of tissue engineering scaffolds based on hyperbolic surfaces: Structural numerical evaluation. Med. Eng. Phys. 2014, 36, 1033–1040. [Google Scholar] [CrossRef]

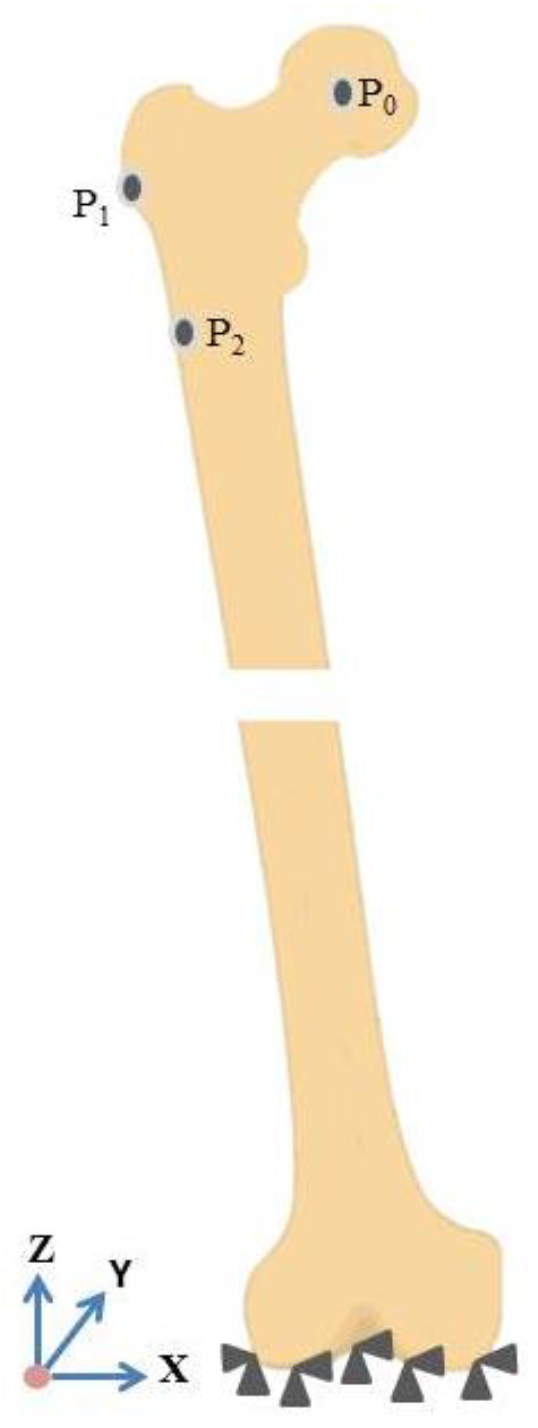




| Material | Plane | Modulus of Elasticity (GPa) | Modulus of Rigidity (GPa) | Poisson’s Ratio |
|---|---|---|---|---|
| xx | 11.5 | 3.6 | 0.51 | |
| Cortical bone | yy | 11.5 | 3.3 | 0.31 |
| zz | 17 | 3.3 | 0.31 | |
| Cancellous bone | - | 2.13 | - | 0.3 |
| Unit Cell Number | R/L | Porosity (%) | Surface Area of the Reaction Force (mm2) | Stress (MPa) | Unit Cell Number | R/L | Porosity (%) | Surface Area of the Reaction Force (mm2) | Stress (MPa) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.05 | 91.8 | 2.36 | 1350.5 | 13 | 0.1998 | 88.392 | 7.06 | 2401.6 |
| 2 | 0.0625 | 91.39 | 2.75 | 1143.9 | 14 | 0.2123 | 88.332 | 7.45 | 2134.4 |
| 3 | 0.0748 | 90.95 | 3.14 | 942 | 15 | 0.2248 | 88.306 | 7.85 | 1901.9 |
| 4 | 0.0873 | 90.535 | 3.53 | 837.79 | 16 | 0.2373 | 88.317 | 8.24 | 170.21 |
| 5 | 0.0998 | 90.151 | 3.92 | 719.6 | 17 | 0.2498 | 88.363 | 8.63 | 152.71 |
| 6 | 0.1123 | 89.803 | 4.31 | 620.3 | 18 | 0.2623 | 88.444 | 9.03 | 137.08 |
| 7 | 0.1248 | 89.492 | 4.71 | 534.16 | 19 | 0.2748 | 88.559 | 9.42 | 123.34 |
| 8 | 0.1373 | 89.217 | 5.1 | 463.15 | 20 | 0.2873 | 88.71 | 9.81 | 111.04 |
| 9 | 0.1498 | 88.977 | 5.49 | 401.54 | 21 | 0.3123 | 89.131 | 10.6 | 89.89 |
| 10 | 0.1623 | 88.775 | 5.89 | 350.98 | 22 | 0.3248 | 89.677 | 10.99 | 80.89 |
| 11 | 0.1748 | 88.612 | 6.2 | 311.16 | 23 | 0.3373 | 89.9 | 11.38 | 78.12 |
| 12 | 0.1873 | 88.482 | 6.67 | 2712.7 | 24 | 0.3498 | 90.04 | 11.77 | 65.47 |
| Topology | IFGP | DFGP | NFGP | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Range of R/L | IFGP1 | IFGP2 | IFGP3 | IFGP4 | DFGP1 | DFGP2 | DFGP3 | DFGP4 | NFGP |
| Upper | 0.2748 | 0.2873 | 0.3123 | 0.3248 | 0.2748 | 0.2873 | 0.3123 | 0.3248 | 0.2748 |
| Lower | 0.05 | 0.0625 | 0.0748 | 0.0873 | 0.05 | 0.0625 | 0.0748 | 0.0873 | 0.05 |
| Force (N) | Direction | Acts at Point | ||
|---|---|---|---|---|
| x | y | z | ||
| Hip contact | −378 | −229.6 | −1604.4 | P0 |
| Abductor | 406 | 30.1 | 605.5 | P1 |
| Tensor fascia lata, proximal part | 50.4 | 81.2 | 92.4 | P1 |
| Tensor fascia lata, distal part | −3.5 | −4.9 | −133 | P1 |
| Vastus lateralis | −6.3 | 129.5 | −650.3 | P2 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jafari Chashmi, M.; Fathi, A.; Shirzad, M.; Jafari-Talookolaei, R.-A.; Bodaghi, M.; Rabiee, S.M. Design and Analysis of Porous Functionally Graded Femoral Prostheses with Improved Stress Shielding. Designs 2020, 4, 12. https://doi.org/10.3390/designs4020012
Jafari Chashmi M, Fathi A, Shirzad M, Jafari-Talookolaei R-A, Bodaghi M, Rabiee SM. Design and Analysis of Porous Functionally Graded Femoral Prostheses with Improved Stress Shielding. Designs. 2020; 4(2):12. https://doi.org/10.3390/designs4020012
Chicago/Turabian StyleJafari Chashmi, Morassa, Alireza Fathi, Masoud Shirzad, Ramazan-Ali Jafari-Talookolaei, Mahdi Bodaghi, and Sayed Mahmood Rabiee. 2020. "Design and Analysis of Porous Functionally Graded Femoral Prostheses with Improved Stress Shielding" Designs 4, no. 2: 12. https://doi.org/10.3390/designs4020012
APA StyleJafari Chashmi, M., Fathi, A., Shirzad, M., Jafari-Talookolaei, R.-A., Bodaghi, M., & Rabiee, S. M. (2020). Design and Analysis of Porous Functionally Graded Femoral Prostheses with Improved Stress Shielding. Designs, 4(2), 12. https://doi.org/10.3390/designs4020012






