Lower Extremity Stretch-Shortening Cycle Performance in the Vertical and Horizontal Direction as Key Determinants of Success in Collegiate Male Taekwondo
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Approach to the Problem
2.2. Participants
2.3. Procedures
2.3.1. Countermovement Jump
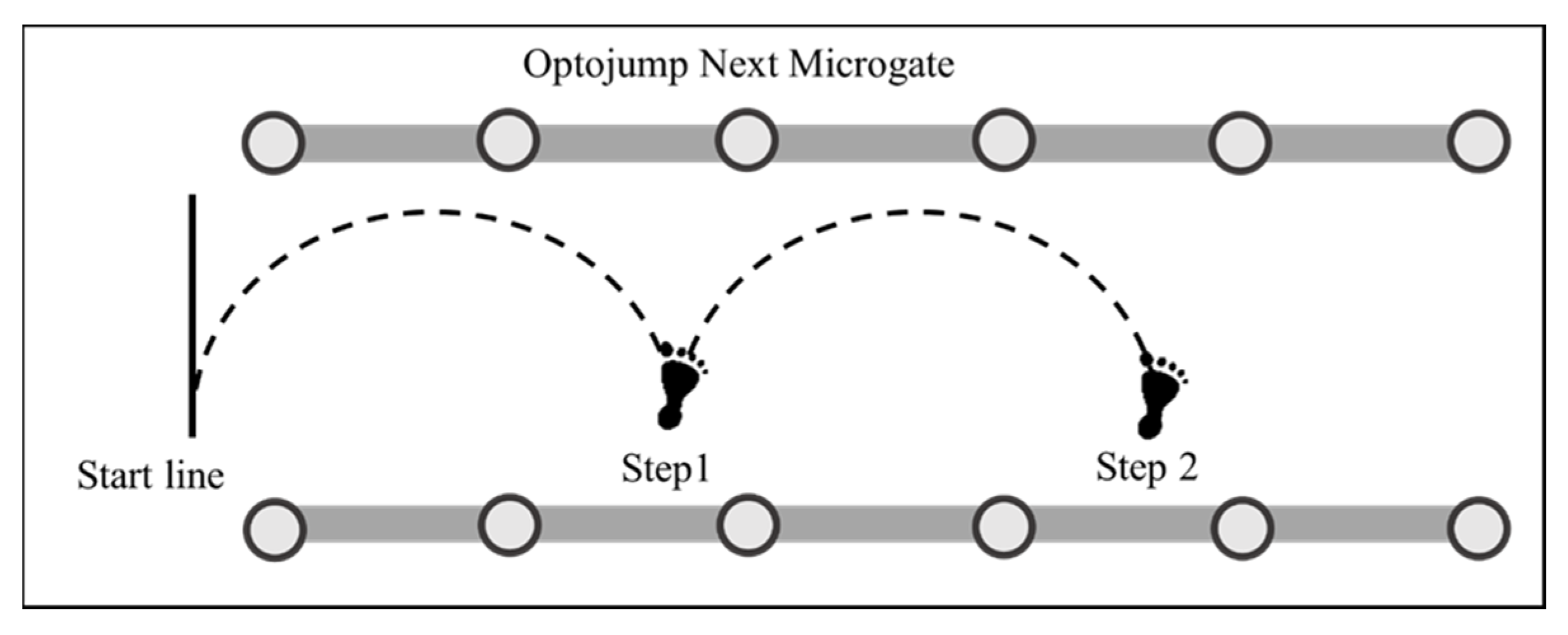
2.3.2. Single-Leg Lateral Hop
2.4. Statistical Analyses
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kazemi, M.; Waalen, J.; Morgan, C.; White, A.R. A profile of Olympic taekwondo competitors. J. Sports Sci. Med. 2006, 5, 114. [Google Scholar] [PubMed]
- Marković, G.; Mišigoj-Duraković, M.; Trninić, S. Fitness profile of elite Croatian female taekwondo athletes. Coll. Antropol. 2005, 29, 93–99. [Google Scholar] [PubMed]
- Turner, A. Routledge Handbook of Strength and Conditioning: Sport-Specific Programming for High Performance; Routledge: New York, NY, USA, 2018. [Google Scholar]
- Ball, N.; Nolan, E.; Wheeler, K. Anthropometrical, Physiological, and Tracked Power Profiles of Elite Taekwondo Athletes 9 Weeks before the Olympic Competition Phase. J. Strength Cond. Res. 2011, 25, 2752–2763. [Google Scholar] [CrossRef]
- Bridge, C.A.; Silva Santos, J.F.; Chaabene, H.; Pieter, W.; Franchini, E. Physical and Physiological Profiles of Taekwondo Athletes. Sports Med. 2014, 44, 713–733. [Google Scholar] [CrossRef]
- Casolino, E.; Cortis, C.; Lupo, C.; Chiodo, S.; Minganti, C.; Capranica, L. Physiological versus Psychological Evaluation in Taekwondo Elite Athletes. Int. J. Sports Physiol. Perform. 2012, 7, 322–331. [Google Scholar] [CrossRef][Green Version]
- Chiodo, S.; Tessitore, A.; Lupo, C.; Ammendolia, A.; Cortis, C.; Capranica, L. Effects of Official Youth Taekwondo Competitions on Jump and Strength Performance. Eur. J. Sport Sci. 2012, 12, 113–120. [Google Scholar] [CrossRef]
- Suzana, M.A.; Pieter, W.N. Motor ability profile of junior and senior taekwondo club athletes. Braz. J. Biomot. 2009, 3, 325–331. [Google Scholar]
- Noorul, H.; Pieter, W.; Erie, Z. Physical fitness of recreational adolescent taekwondo athletes. Braz. J. Biomot. 2008, 2, 230–240. [Google Scholar]
- Toskovic, N.; Blessing, D.; Williford, H. Physiologic Profile of Recreational Male and Female Novice and Experienced Tae Kwon Do Practitioners. J. Sports Med. Phys. Fit. 2004, 44, 164. [Google Scholar]
- Buckthorpe, M.; Morris, J.; Folland, J.P. Validity of Vertical Jump Measurement Devices. J. Sports Sci. 2012, 30, 63–69. [Google Scholar] [CrossRef]
- Cormie, P.; McBride, J.M.; McCaulley, G.O. Power-Time, Force-Time, and Velocity-Time Curve Analysis of the Countermovement Jump: Impact of Training. J. Strength Cond. Res. 2009, 23, 177–186. [Google Scholar] [CrossRef] [PubMed]
- McMahon, J.J.; Jones, P.A.; Suchomel, T.J.; Lake, J.; Comfort, P. Influence of the Reactive Strength Index Modified on Force–and Power–Time Curves. Int. J. Sports Physiol. Perform. 2018, 13, 220–227. [Google Scholar] [CrossRef] [PubMed]
- McMahon, J.J.; Murphy, S.; Rej, S.J.; Comfort, P. Countermovement-Jump-Phase Characteristics of Senior and Academy Rugby League Players. Int. J. Sports Physiol. Perform. 2017, 12, 803–811. [Google Scholar] [CrossRef] [PubMed]
- McMahon, J.J.; Rej, S.J.; Comfort, P. Sex Differences in Countermovement Jump Phase Characteristics. Sports 2017, 5, 8. [Google Scholar] [CrossRef]
- James, L.P.; Connick, M.; Haff, G.G.; Kelly, V.G.; Beckman, E.M. The Countermovement Jump Mechanics of Mixed Martial Arts Competitors. J. Strength Cond. Res. 2020, 34, 982–987. [Google Scholar] [CrossRef]
- Barker, L.A.; Harry, J.R.; Mercer, J.A. Relationships between Countermovement Jump Ground Reaction Forces and Jump Height, Reactive Strength Index, and Jump Time. J. Strength Cond. Res. 2018, 32, 248–254. [Google Scholar] [CrossRef]
- Coh, M.; Mackala, K. Differences between the Elite and Subelite Sprinters in Kinematic and Dynamic Determinations of Countermovement Jump and Drop Jump. J. Strength Cond. Res. 2013, 27, 3021–3027. [Google Scholar] [CrossRef]
- Chaabene, H.; Negra, Y.; Capranica, L.; Bouguezzi, R.; Hachana, Y.; Rouahi, M.A.; Mkaouer, B. Validity and Reliability of a New Test of Planned Agility in Elite Taekwondo Athletes. J. Strength Cond. Res. 2018, 32, 2542–2547. [Google Scholar] [CrossRef]
- Wojciechowska-Maszkowska, B.; Borysiuk, Z.; Wąsik, J.; Janisiów, P.; Nawarecki, D. Effects of Anaerobic Fatigue on Postural Control in Taekwondo Practitioners. J. Combat Sports Martial Arts 2012, 3, 103–107. [Google Scholar] [CrossRef]
- Birchmeier, T.; Lisee, C.; Geers, B.; Kuenze, C. Reactive Strength Index and Knee Extension Strength Characteristics Are Predictive of Single-Leg Hop Performance after Anterior Cruciate Ligament Reconstruction. J. Strength Cond. Res. 2019, 33, 1201–1207. [Google Scholar] [CrossRef]
- Hamilton, R.T.; Shultz, S.J.; Schmitz, R.J.; Perrin, D.H. Triple-Hop Distance as a Valid Predictor of Lower Limb Strength and Power. J. Athl. Train 2008, 43, 144–151. [Google Scholar] [CrossRef] [PubMed]
- Lin, W.-C.; Chu, M.-Y.; Chiang, C.-Y. The Relationship between Vertical Jump and Lateral Jump Performance in Division One Male Collegiate Taekwondo Athletes. In Proceedings of the 15th Physical Education and Sport Academic Organizations Annual Joint Assembly and Symposium, Taoyuan, Taiwan, 5–6 December 2020. [Google Scholar]
- Monteleone, B.J.; Ronsky, J.L.; Meeuwisse, W.H.; Zernicke, R.F. Lateral Hop Movement Assesses Ankle Dynamics and Muscle Activity. J. Appl. Biomech. 2012, 28, 215–221. [Google Scholar] [CrossRef] [PubMed]
- Owen, N.J.; Watkins, J.; Kilduff, L.P.; Bevan, H.R.; Bennett, M.A. Development of a Criterion Method to Determine Peak Mechanical Power Output in a Countermovement Jump. J. Strength Cond. Res. 2014, 28, 1552–1558. [Google Scholar] [CrossRef] [PubMed]
- Xu, J.; Turner, A.; Comfort, P.; Harry, J.R.; McMahon, J.J.; Chavda, S.; Bishop, C. A Systematic Review of the Different Calculation Methods for Measuring Jump Height During the Countermovement and Drop Jump Tests. Sports Med. 2023, 53, 1055–1072. [Google Scholar] [CrossRef]
- Lee, D.W.; Yang, S.J.; Cho, S.I.; Lee, J.H.; Kim, J.G. Single-Leg Vertical Jump Test as a Functional Test after Anterior Cruciate Ligament Reconstruction. Knee 2018, 25, 1016–1026. [Google Scholar] [CrossRef]
- Fleiss, J.L. Design and Analysis of Clinical Experiments; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Cormack, S.J.; Newton, R.U.; McGuigan, M.R.; Doyle, T.L. Reliability of Measures Obtained during Single and Repeated Countermovement Jumps. Int. J. Sports Physiol. Perform. 2008, 3, 131–144. [Google Scholar] [CrossRef]
- Hopkins, W.; Marshall, S.; Batterham, A.; Hanin, J. Progressive Statistics for Studies in Sports Medicine and Exercise Science. Med. Sci. Sports Exerc. 2009, 41, 3. [Google Scholar] [CrossRef]
- Bobbert, M.F.; Gerritsen, K.G.; Litjens, M.C.; Soest, A.J. Why Is Countermovement Jump Height Greater than Squat Jump Height? Med. Sci. Sports Exerc. 1996, 28, 1402–1412. [Google Scholar] [CrossRef]
- Kirby, T.J.; McBride, J.M.; Haines, T.L.; Dayne, A.M. Relative net vertical impulse determines jumping performance. J. Appl. Biomech. 2011, 27, 207–214. [Google Scholar] [CrossRef]
- Moreira, P.V.S.; Goethel, M.F.; Gonçalves, M. Neuromuscular performance of Bandal Chagui: Comparison of subelite and elite taekwondo athletes. Electromyogr. Kinesiol. 2016, 30, 55–65. [Google Scholar] [CrossRef]
- Santos, V.G.; Franchini, E.; Lima-Silva, A.E. Relationship between Attack and Skipping in Taekwondo Contests. J. Strength Cond. Res. 2011, 25, 1743–1751. [Google Scholar] [CrossRef] [PubMed]
- Gustavsson, A.; Neeter, C.; Thomeé, P.; Silbernagel, K.G.; Augustsson, J.; Thomeé, R.; Karlsson, J. A Test Battery for Evaluating Hop Performance in Patients with an ACL Injury and Patients Who Have Undergone ACL Reconstruction. Knee Surg. Sports Traumatol. Arthrosc. 2006, 14, 778–788. [Google Scholar] [CrossRef] [PubMed]
- Maulder, P.; Cronin, J. Horizontal and Vertical Jump Assessment: Reliability, Symmetry, Discriminative and Predictive Ability. Phys. Ther. Sport 2005, 6, 74–82. [Google Scholar] [CrossRef]


| CMJ Variable | Abbreviation | Description |
|---|---|---|
| Jump height (cm) | JH | Maximum JH (calculated using the velocity at take-off method) |
| Time to take-off (s) | TTT | Time spent from the onset threshold to take-off |
| Reactive strength index modified | RSImod | Jump height divided by TTT |
| Eccentric phase variables | ||
| Peak eccentric force (N·kg−1) | EccPF | Maximum force value attained during the eccentric phase |
| Force at 0 velocity (N·kg−1) | F@0V | Force exerted at the end of the countermovement (i.e., velocity is at zero) |
| Peak eccentric power (W·kg−1) | EccPP | Maximum power value attained during the eccentric phase |
| Peak eccentric velocity (m·s−1) | EccPV | Minimum velocity attained during the eccentric phase |
| Eccentric displacement (cm) | EccDis | Lowest point of the countermovement |
| Concentric phase variables | ||
| Peak concentric force (N·kg−1) | ConPF | Maximum force value attained during the concentric phase |
| Peak concentric power (W·kg−1) | ConPP | Maximum power value attained during the concentric phase |
| Peak concentric velocity (m·s−1) | ConPV | Maximum velocity attained during the concentric phase |
| MG (n = 8) | NMG (n = 9) | p | d | Magnitude Descriptor | ICC (95% CI) | % CV | |
|---|---|---|---|---|---|---|---|
| CMJ Variables | Mean ± SD | Mean ± SD | |||||
| JH (cm) | 44.8 ± 5.18 | 38.3 ± 3.95 | 0.01 | 1.40 | Large | 0.978 (0.942–0.992) | 0.57 |
| TTT (s) | 0.84 ± 0.47 | 0.84 ± 0.12 | 0.77 | 0.02 | Trivial | 0.921 (0.795–0.971) | 2.28 |
| RSImod | 0.54 ± 0.06 | 0.47 ± 0.07 | 0.06 | 1.03 | Moderate | 0.951 (0.869–0.982) | 2.8 |
| Eccentric phase variables | |||||||
| EccPF (N·kg−1) | 26.12 ± 2.65 | 23.07 ± 1.74 | 0.04 | 1.36 | Large | 0.928 (0.814–0.973) | 2.14 |
| F@0V (N·kg−1) | 26.05 ± 2.57 | 23.69 ± 1.72 | 0.04 | 1.08 | Moderate | 0.929 (0.815–0.974) | 2.04 |
| EccPP (W·kg−1) | −22.76 ± 5.51 | −19.15 ± 5.14 | 0.18 | −0.68 | Trivial | 0.933 (0.826–0.975) | 5.57 |
| EccPV (m·s−1) | −1.54 ± 0.22 | −1.37 ± 0.25 | 0.14 | 0.72 | Moderate | 0.938 (0.840–0.977) | 3.35 |
| EccDis (cm) | −41.57 ± 6.35 | −35.07 ± 5.99 | 0.05 | 1.05 | Moderate | 0.945 (0.842–0.981) | 3.26 |
| Concentric phase variables | |||||||
| ConPF (N·kg−1) | 26.21 ± 2.54 | 24.27 ± 1.62 | 0.09 | 0.91 | Moderate | 0.935 (0.829–0.976) | 1.81 |
| ConPP (W·kg−1) | 59.02 ± 6.07 | 54.73 ± 4.18 | 0.11 | 0.82 | Moderate | 0.970 (0.920–0.989) | 1.23 |
| ConPV (m·s−1) | 3.05 ± 0.17 | 2.85 ± 0.13 | 0.01 | 1.32 | Large | 0.982 (0.952–0.993) | 0.65 |
| MG (n = 8) | NMG (n = 9) | p | d | Magnitude Descriptor | |
|---|---|---|---|---|---|
| SLLH Variables | Mean ± SD | Mean ± SD | |||
| Step 1 (cm) | 169.63 ± 19.86 | 159.11 ± 13.00 | 0.21 | 0.61 | Moderate |
| Step 2 (cm) | 246.38 ± 31.36 | 235.67 ± 20.64 | 0.41 | 0.40 | Small |
| TotalD (cm) | 416.00 ± 49.17 | 394.78 ± 30.91 | 0.30 | 0.52 | Small |
| GCT (s) | 0.27 ± 0.02 | 0.29 ± 0.04 | 0.14 | 0.63 | Moderate |
| LRSI | 908.97 ± 116.20 | 811.36 ± 114.43 | 0.10 | 0.84 | Moderate |
| Variable | CMJ JH | CMJ RSImod | CMJ EccDis | CMJ TTT | CMJ EccPF | CMJ ConPF | CMJ F@0V | CMJ EccPP | CMJ ConPP | CMJ EccPV | CMJ ConPV |
|---|---|---|---|---|---|---|---|---|---|---|---|
| SLLH Step 1 | 0.565 | 0.495 | −0.268 | −0.044 | 0.155 | 0.203 | 0.150 | 0.022 | 0.497 | −0.080 | 0.544 |
| SLLH Step 2 | 0.305 | 0.370 | −0.066 | −0.197 | 0.253 | 0.268 | 0.257 | −0.016 | 0.186 | −0.020 | 0.267 |
| SLLH TotalD | 0.430 | 0.442 | −0.153 | −0.144 | 0.226 | 0.255 | 0.227 | −0.001 | 0.326 | −0.046 | 0.397 |
| SLLH GCT | −0.065 | −0.211 | 0.009 | 0.255 | −0.541 | −0.532 | −0.555 | 0.234 | −0.195 | 0.158 | −0.098 |
| SLLH FT | 0.060 | 0.079 | −0.117 | −0.073 | 0.134 | 0.038 | 0.136 | −0.169 | −0.044 | −0.154 | 0.032 |
| SLLH LRSI | 0.278 | 0.449 | −0.039 | −0.357 | 0.562 | 0.593 | 0.577 | −0.142 | 0.298 | −0.101 | 0.272 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chiang, C.-Y.; Chiang, Y.-C.; Lin, H.-Y.; Tseng, H.-C.; Chu, M.-Y.; Chang, J.-S. Lower Extremity Stretch-Shortening Cycle Performance in the Vertical and Horizontal Direction as Key Determinants of Success in Collegiate Male Taekwondo. J. Funct. Morphol. Kinesiol. 2025, 10, 15. https://doi.org/10.3390/jfmk10010015
Chiang C-Y, Chiang Y-C, Lin H-Y, Tseng H-C, Chu M-Y, Chang J-S. Lower Extremity Stretch-Shortening Cycle Performance in the Vertical and Horizontal Direction as Key Determinants of Success in Collegiate Male Taekwondo. Journal of Functional Morphology and Kinesiology. 2025; 10(1):15. https://doi.org/10.3390/jfmk10010015
Chicago/Turabian StyleChiang, Chieh-Ying, Yi-Chien Chiang, Hsuan-Yu Lin, Hao-Che Tseng, Mu-Yen Chu, and Jung-San Chang. 2025. "Lower Extremity Stretch-Shortening Cycle Performance in the Vertical and Horizontal Direction as Key Determinants of Success in Collegiate Male Taekwondo" Journal of Functional Morphology and Kinesiology 10, no. 1: 15. https://doi.org/10.3390/jfmk10010015
APA StyleChiang, C.-Y., Chiang, Y.-C., Lin, H.-Y., Tseng, H.-C., Chu, M.-Y., & Chang, J.-S. (2025). Lower Extremity Stretch-Shortening Cycle Performance in the Vertical and Horizontal Direction as Key Determinants of Success in Collegiate Male Taekwondo. Journal of Functional Morphology and Kinesiology, 10(1), 15. https://doi.org/10.3390/jfmk10010015






