Life Cycle Cost Analysis of Three Types of Power Lines in 10 kV Distribution Network

Abstract

There are three types of power lines in the 10 kV distribution network in China, i.e., copper power cables, overhead power conductors and aluminum alloy power cables. It is necessary to give a comprehensive evaluation to choose the type of power line in some delicate practical engineering. This paper presents a life cycle cost (LCC)-based analysis method for the three types of power lines. An LCC model of the power line in the 10 kV distribution network is established, which considers four parts: investment cost, operation and maintenance cost, failure cost and discard cost. A detailed calculation model for the four parts is presented, and to calculate the failure cost, the Monte Carlo algorithm is employed to simulate the values of expected energy not supplied (EENS). Two practical 10 kV power line projects in Fujian Province in China were analyzed based on the proposed LLC model and corresponding developed software, which has helped the power company select the appropriate power line successfully.

1. Introduction

As we all known, the distribution network is one of the main sections of the power system and takes the heavy responsibilities of social and economic development. Additionally, the 10 kV power lines play significant roles in the distribution network in China. Currently, three kinds of distribution lines are used widely, including copper cable, overhead conductor and aluminum alloy cable. In China, traditional distribution power lines are almost all copper cable and overhead conductor. In recent years, the application of aluminum alloy cables is becoming more extensive in the distribution network. The quantity of copper resources has been found in China to be about 89.72 million tons since 1949. However, the quantity of aluminum resources has been found to be much more than copper, which is about 3.87 billion tons. This indicates that China is lacking copper resources, but rich in aluminum resources, which highlights the advantages of using aluminum alloy in electric cables. As a result, it is necessary to study which kind of cable is the most economical in different practical situations.

There are many methods to modeling the economy of practical engineering projects, representatively including the net present value method, the uniform annual value method, the payback period method and the life cycle cost (LCC) method. The net present value method is a kind of simple method used to evaluate the investment project. This method uses the net present benefit and net present investment cost to figure out the net present value, then according to the net present value to evaluate the project [1,2]. The uniform annual value method is to convert the whole cash flow or net present value to the annual average net value according to the investment necessary remuneration rate. It usually only contains the investment cost and the discard cost [3]. The payback period method is a static method that is used to calculate the time to recover the total investment cost. It should be under the normal operating conditions and take the amortization of intangible assets into consideration. The payback period is measured by the rate of recovering the initial investment [4,5]. However, these methods do not take the whole service life of a project into consideration, so their analyses are not comprehensive. In some countries, such as America, a typical method was employed to measure the economic difference among different plans, called the life cycle cost analysis (LCCA) [6,7,8]. LCCA is an evaluation method of the project cost, which includes the investment cost of the project, also the operation and maintenance cost, failure cost and all the other costs until the end of the engineering project. The method evaluates the economic advantages and disadvantages of an engineering project by comparing the whole cost of different plans during the whole life of it. Until now, some results have been achieved in many engineering fields, which can provide experiences and references to other applications. In [9], LCCA is used to calculate the greenhouse gas emissions of the small autonomous hybrid power systems (SAHPS), which contributes to a better solution of the optimum economic and environmental performance of SAHPS. With respect to power distribution planning, LCCA can be used to establish the multi-objective function to find the optimal location and capacity of future substations, considering economy, reliability and safety [10].

The LCCA is a relatively comprehensive method in the economic evaluation of a project. However, few quantitative research works were done for the LCC of the 10 kV distribution lines, especially aluminum alloy cable, which has not been widely used. As a result, this paper aims to concentrate on the LCC of the 10 kV distribution lines and to compare the three types of power lines in two practical projects by quantitative analysis. During the calculation of the failure cost, the existing LCCA method is based on the historical data of a past similar project. However, few historical data of aluminum alloy cable can be found or be used. Therefore, to calculate the failure cost, it is necessary to propose another method that takes the high randomness of the failure rate into consideration. In this paper, a risk assessment model is proposed to evaluate the failure cost in the LCC, and the Monte Carlo algorithm is employed to simulate the values of expected energy not supplied (EENS). The main contributions of this paper are as follows.

• A LCC model of the 10 kV distribution power lines is proposed, containing investment cost, operation and maintenance cost, failure cost and discard cost.
• A risk assessment model is proposed by using the Monte Carlo algorithm to evaluate the failure cost.
• Quantitative analysis and the comparison of the LCC of the 10 kV distribution lines are presented.

The rest of the paper is organized as follows. In Section 2, we divide the whole life of the power cable into four parts, including investment, operation and maintenance, failure and discard. By analyzing each part, the whole LCC model is established. On this basis, in Section 3, an LCC evaluation software is developed to help calculate the LCC in projects. After that, two practical cases in Fujian province in China are analyzed, using the model and software that we have proposed. At last, conclusions are given in Section 4.

2. The Life Cycle Cost (LCC) Model

The LCC model of a power line is a model that analyzes the whole costs of a power line engineering project, including design and construction, operation and maintenance, failure and discard. It takes the safety and reliability of the line as the premise, the cost of the whole life cycle to be least as the target, evaluating the different power lines to find out the optimal power line plan in a project. In order to improve the applicability of the model, the model of all kinds of power distribution lines is considered [11]. Referring to a practical engineering project, the basic LCC framework is determined, which is shown in Figure 1.

According to Figure 1, we have:

$$LCC=C_i+C_o+C_f+C_d$$

(1)

where $C_i$ is the investment cost, $C_o$ is the operation and maintenance cost, $C_f$ is the failure cost and $C_d$ is the discard cost. It should be noted that the above four components are already discounted into present values. Specific modeling and analysis will be presented in the following parts.

2.1. Investment Cost

The investment cost of a power line is a one-time cost that is paid during the design, installation and commissioning, before the project is officially put into operation. It is symbolized as $C_i$. It mainly includes the purchasing cost of fixed assets and the design and installation cost. To express the whole investment cost, we have:

$$\begin{array}{ccc}\hfill C_i& =& C_{fi}+C_{ci}+C_{oi}\hfill \end{array}$$

(2)

$$\begin{array}{ccc}\hfill C_{fi}& =& (C_{mi}+C_{ai}+C_{li})I_1\hfill \end{array}$$

(3)

where $C_{fi}$ is the purchasing cost of fixed assets, including the purchasing cost of the cable $C_{mi}$ and the purchasing cost of accessories $C_{ai}$, and if it is an overhead conductor, it should also include the land acquisition cost $C_{li}$. For fixed assets, because of its time value, which means it has a different value at different time points, it is necessary to consider the discount coefficient $I_1$ according to the service life [12]. However, we discount the cost to the present, so $I_1=1$. $C_{ci}$ is the cost for the design and installation of the line, including the line measure cost, special tools and the initial cost of spare parts, machinery and transportation cost, labor and service charges, installation and commissioning cost, etc. $C_{oi}$ is other costs, including the cost of possible forest cutting and foundation construction, etc.

2.2. Operation and Maintenance Cost

After the construction of the power line is completed, it will be put into operation. At this stage, some costs will be produced, that is the operation and maintenance costs. We use $C_o$ to represent the operation and maintenance cost, which includes the total cost in the operation period of a power line. We have:

$$\begin{array}{ccc}\hfill C_o& =& (C_{eo}+C_{lo}+C_{co}+C_{oo})I_2\hfill \end{array}$$

(4)

$$\begin{array}{ccc}\hfill I_2& =& \frac{{(1+i)}^n-1}{i{(1+i)}^n}\hfill \end{array}$$

(5)

where $C_{eo}$ is the energy consumption cost, which means the energy loss of a power line converted to money; $C_{io}$ is the inspection and maintenance cost, including inspection machine cost, tool cost and labor cost; $C_{co}$ is the recondition cost, including recondition machine cost, material cost, service cost and labor cost; other possible costs are included in $C_{oo}$; $I_2$ is also a discount coefficient, in which i is the annual discount rate and n is the service life.

2.2.1. Energy Consumption Cost

After the power line is put into operation, the main operation cost is the energy consumption cost, which is related to the physical characteristics and operation state of the line. According to [13,14], for overhead conductors, we can formulate the energy consumption cost as follows:

$$C_{eo}=3I_{\max }^{2}RLTrP\frac{{(1+i_p)}^{n_p}-1}{i_p}\times {10}^{-6}$$

(6)

where $I_{\max }$ is the maximum load current; R is the AC resistance; L is the length of the power line; T is the operating hour of one year; r is the annual average load rate; P is the cost price of electricity per kilowatt; $i_p$ is the annual increasing rate of the electricity price; $n_p$ is calculation years.

For cables, beside the energy consumption in cable cores, which is calculated before, the energy consumption in the dielectric medium should be calculated, as well. We have:

$$\begin{array}{ccc}\hfill C_{eo}^{″}& =& C_{eo}+C_{eo}^{\prime }\hfill \end{array}$$

(7)

$$\begin{array}{ccc}\hfill C_{eo}^{\prime }& =& 2\pi f{U}^{2}C\tan \delta TLP\frac{{(1+i_p)}^{n_p}-1}{i_p}\times {10}^{-6}\hfill \end{array}$$

(8)

where $C_{eo}^{\prime }$ is the energy consumption in the dielectric medium; f is the frequency of the electric system; U is the operation line voltage; C is the working capacitance of cable per phase; tan $\delta$ is the value of the dielectric loss tangent.

Therefore, Equation (7) is the total energy consumption cost for cables.

2.2.2. Maintenance Cost

In order to ensure the safety and reliability of the power line, power companies need to have regular inspection and maintenance of the power line. The cost consumed can be formulated as follows:

$$C_{lo}=(p_1{t}_1+p_2{t}_2)Ln$$

(9)

where $p_1$ is the inspection cost each time, including the environmental inspection cost, professional inspection cost, ground temperature detection cost and induction current testing cost; $p_2$ is the regular maintenance cost; L is the length of the line; n is the service life; $t_1$ is the inspection cycle; $t_2$ is the maintenance cycle.

2.2.3. Recondition Cost

When the power line is running for a period of time, it is necessary to recondition it to eliminate the hidden trouble and ensure the stability of the line. The cost consumed can be formulated as follows:

$$C_{co}=\frac{pLn}{t}$$

(10)

where p is the inspection cost each time; t is the inspection cycle; L is the length of the line; n is the service life.

2.3. Failure Cost

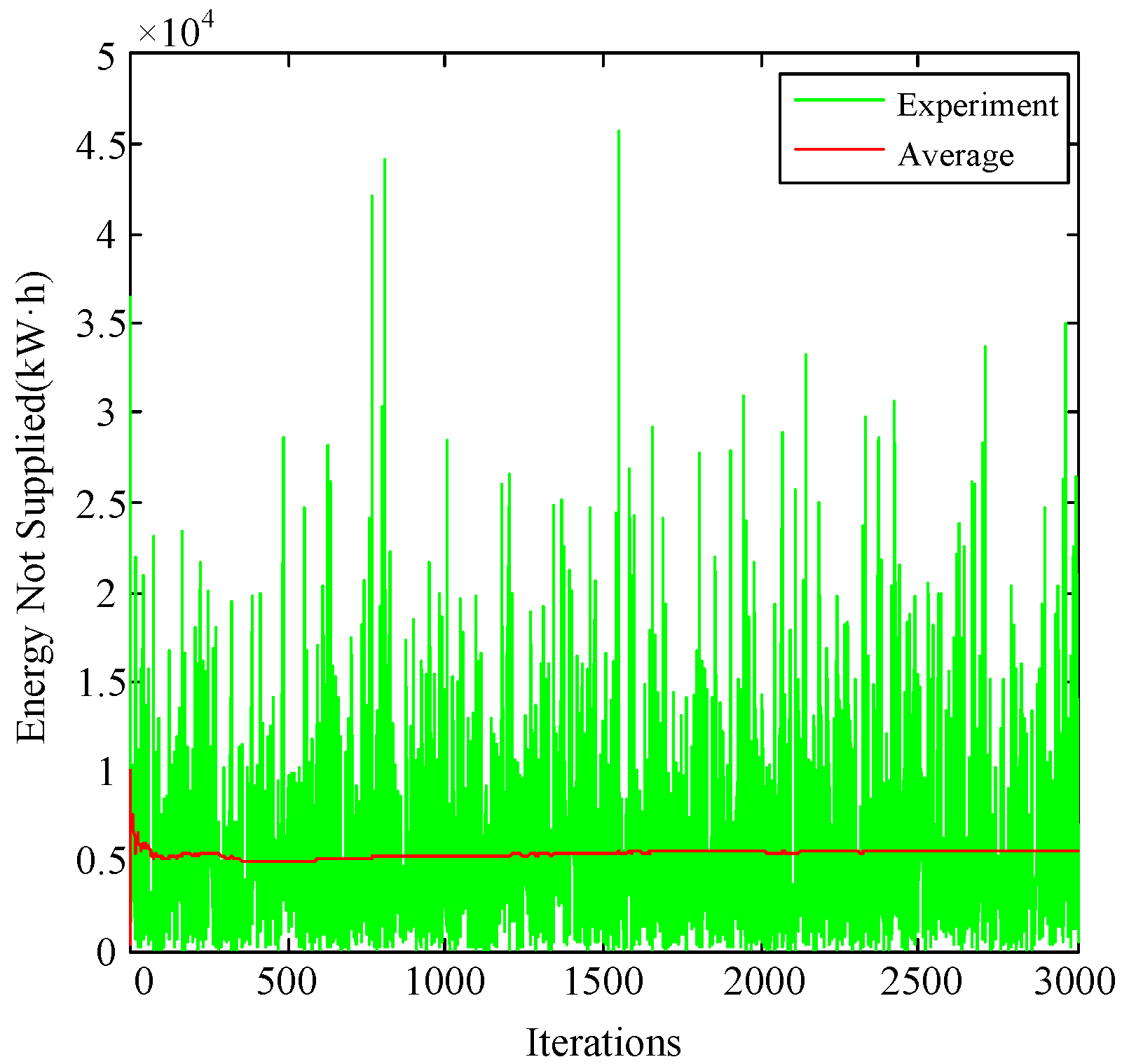
2.3.1. Risk Assessment Model

The failure cost of the power line is influenced by multiple factors, which are defined as economic losses caused by power outages. Power companies need to schedule maintenance when power lines have faults. Maintenance cost is related to fault times, which can be calculated as:

$$C_{cmf}={\lambda }_N{C}_{mf}$$

(11)

where $C_{cmf}$ is the maintenance cost; ${\lambda }_N$ is fault time; $C_{mf}$ is the average maintenance cost of each fault for the cable, which includes labor cost, equipment cost and material cost.

Apart from maintenance, the line fault will also break the power supply, which may cause loss to power companies. The loss cost can be separated into two parts: direct failure cost and indirect failure cost. Direct failure cost can be considered from the perspective of interruption cost [15,16]. In this paper, the expected energy not supplied is used to characterize the magnitude of the direct failure cost, which can be described as:

$$C_{df}=pEENS$$

(12)

where $C_{df}$ is the direct failure cost; p is the purchase and marketing price differentials of electricity, which generally is 0.3; $EENS$ is the value of the expected energy not supplied, which can be calculated as:

$$EENS={\lambda }_{N}LT$$

(13)

where L is load to the cable supply; T is the time for fault maintenance. Load can be obtained by predicting, although there often is a certain deviation. The actual load can be calculated as follows:

$$L=L_E\left(1-L_d\right)$$

(14)

where $L_E$ is the predictive value of the load; $L_d$ is the deviation rate of predictive value $L_E$, which obeys the law of a normal distribution [17]. The corresponding probability density function is defined as:

$$f\left(L_d\right)=\frac{1}{\sqrt{2\pi }\sigma }\exp \left(-\frac{{\left(L_d-E_d\right)}^2}{2{\sigma }^2}\right)$$

(15)

where $E_d$ is the mean value of the load forecasting deviation rate; ${\sigma }^2$ is the variance of the load forecasting deviation rate. The maintenance process of the fault is influenced by many factors, such as location, weather condition, maintenance level, and so on. Fault maintenance time is a random variable, which obeys an exponential distribution [18]. The corresponding probability density function is defined as:

$$f\left(T\right)=\mu e^{-\mu T}$$

(16)

where μ is the distributed parameter, the reciprocal if which is the mean value of the fault maintenance time $T_E$, namely $T_E=\frac{1}{\mu }$.

The indirect failure cost includes the cost of compensation, the impact on social production and the credibility of power companies. Because some adverse effects cannot be measured by money, it is difficult to estimate the indirect losses. The direct failure cost and a reasonable coefficient a can be used to indicate the value of indirect failure cost, namely,

$$C_{if}=a{C}_{df}$$

(17)

where $C_{if}$ is the indirect failure cost. For different kinds of users, the social impact caused by supply interruption is different. Thus, the corresponding coefficient a is different for users. The Delphi method can be used to obtain the value of coefficient a.

In summary, the failure cost includes the maintenance cost caused by the fault and the direct failure cost and the indirect failure cost caused by supply interruption, which can be described as:

$$\begin{array}{ccc}{C}_f^{\prime }& =& C_{cmf}+C_{df}+C_{if}\hfill \\ & =& C_{cmf}+\left(1+a\right)pEENS\hfill \\ & =& {\lambda }_N\left[C_{mf}+\left(1+a\right)pLT\right]\hfill \end{array}$$

(18)

where $C_f^{\prime }$ denotes the failure cost.

Equipment maintenance risk, lack of power supply risk, reputation risk, social impact risk and compensation risk are fully considered when calculating the failure cost. Thus, the failure cost can not only measure the loss caused by supply interruption and influence the reliability of the system, but also measure the adverse impact of the social production and reputation of the electric power company.

2.3.2. Risk Assessment Method

Model of the Fault Rate

From Equation (18), we can see that the value of the failure cost has a close relationship with the fault times. Failure of the cable is the result of the common interaction of itself and the external factors. The main factors of the cable itself include material defects, improper installation process, and so on. Meanwhile, the main external factors include external damage, insulation aging, chemical corrosion, insulation moisture and other factors. These factors constitute the source of the risk of cable failure. The running state of the cable within the whole life cycle can also be expressed by the failure rate. The relationship between fault times and the failure rate can be described as follows [19]:

$$\lambda =\frac{100{\lambda }_N}{T_{e}l}$$

(19)

where λ is the failure rate, $T_e$ is the exposure time corresponding to the failure times and l is the length of the cable. For the convenience of comparison between different cable failure costs and the use of the fault cost model, fault times ${\lambda }_N$ in Equation (18) are replaced by the failure rate λ.

Therefore, the failure cost per 100 km of cable per year $C_f$ can be calculated as:

$$C_f=\lambda \left[C_{mf}+\left(1+a\right)pLT\right]$$

(20)

As shown in Figure 2, the failure rate in the whole life cycle of the cable is the curve with time, which is called the bathtub curve. In the first stage, quality problems caused by manufacturing will be exposed, which result in a high failure rate; in the third stage of the life cycle, aging of the cable causes the failure rate to increase gradually. However, the duration of the first stage is very short. Meantime, the cable usually will be replaced before reaching the third stage, and the time of the third stage is short. In this paper, we assume that the failure rate does not change with time during the entire life cycle when calculating the failure cost. Namely, the failure rate of each year is the same and can be obtained from history data statistics.

Failure Simulation Method

The Monte Carlo method is a stochastic simulation method based on probability and statistical theory [20] , which is used to simulate the random process of cable failure when calculating the failure cost. The specific steps are:

• Firstly, the normal distribution probability model of the deviation rate of the predictive load and the exponential distribution of the probability distribution of the fault maintenance time are established;
• Then, sampling the values of predictive load and fault maintenance time;
• Finally, calculating the indexes by the statistical method.

For the failure cost model above, the Monte Carlo method can be applied to get the value of the expected energy-not-supplied $E_{\mathrm{ENS}}$, which is related to the two random variables, predictive load and fault maintenance time. For each value of load L, it has corresponding probability $P_1\left(L\right)$. Similarly, for each fault maintenance time T, it has corresponding probability $P_2\left(T\right)$. Let $E_S$ denote the result of an experiment, namely,

$$E_S\left(L,T\right)=\lambda LT$$

(21)

Load L and failure maintenance time T are random variables. Thus, the result of an experiment also is a random variable, the expected value of which can be calculated as:

$$E\left(E_S\left(L,T\right)\right)=\sum E_S\left(L,T\right)P_1\left(L\right)P_2\left(T\right)$$

(22)

where $E\left(E_S\left(L,T\right)\right)$ denotes the expected value of $E_S\left(L,T\right)$, which can be estimated as follows:

$$\widehat{E}\left(E_S\left(L,T\right)\right)=\frac{1}{N}\sum _{i=1}^N{E}_{Si}\left(L_i,T_i\right)$$

(23)

where $\widehat{E}\left(E_S\left(L,T\right)\right)$ is the estimate of the expected value of $E_S\left(L,T\right)$; N is the sampling times; $L_i$ is the value of the load for the i-th sample; $T_i$ is the value of the load for the i-th sample; $E_{Si}\left(L_i,T_i\right)$ is the result of the i-th sample. From Equation (23), one can see that $\widehat{E}\left(E_S\left(L,T\right)\right)$ is not the truth-value of $E\left(E_S\left(L,T\right)\right)$. Due to the fact that $E_S\left(L,T\right)$ is a random variable, expected value $\widehat{E}\left(E_S\left(L,T\right)\right)$ is a random variable, the error of which is determined by its variance.

$$V\left(\widehat{E}\left(E_S\left(L,T\right)\right)\right)=\frac{V\left(E_S\left(L,T\right)\right)}{N}$$

(24)

where $V\left(\widehat{E}\left(E_S\left(L,T\right)\right)\right)$ is the variance of the estimate of $E\left(E_S\left(L,T\right)\right)$; $V\left(E_S\left(L,T\right)\right)$ is the variance of $E_S\left(L,T\right)$, which can be estimated as follows:

$$\begin{array}{c}\widehat{V}\left(E_S\left(L,T\right)\right)=\frac{1}{N}{\displaystyle \sum _{i=1}^N}{\left[E_{Si}\left(L_i,T_i\right)-\widehat{E}\left(E_S\left(L,T\right)\right)\right]}^2\hfill \end{array}$$

(25)

Equation (24) indicates that the estimation error is proportional to the variance $V\left(E_S\left(L,T\right)\right)$ and is inversely proportional to the sampling times N. The convergence criterion of the Monte Carlo method is based on the error of $\widehat{E}\left(E_S\left(L,T\right)\right)$, which is the estimated value of $E\left(E_S\left(L,T\right)\right)$. The criterion is usually expressed as follows:

$$U=\frac{\sqrt{V\left(\widehat{E}\left(E_S\left(L,T\right)\right)\right)}}{\widehat{E}\left(E_S\left(L,T\right)\right)}$$

(26)

where U is the variance coefficient. Then, we can get:

$$U=\frac{\sqrt{\frac{V\left(E_S\left(L,T\right)\right)}{N}}}{\widehat{E}\left(E_S\left(L,T\right)\right)}$$

(27)

Therefore,

$$N=\frac{V\left(E_S\left(L,T\right)\right)}{{\left(U\widehat{E}\left(E_S\left(L,T\right)\right)\right)}^2}$$

(28)

From Equation (28), one can see that the amount of the calculation of the Monte Carlo method has little influence on the size or complexity of the system.

2.3.3. Line Fault Simulation

Based on the failure cost risk assessment model and the probability distribution model of load and failure maintenance time above, MATLAB R2013a (MathWorks, Natick, MA, USA) is used to realize the random simulation process of the line fault. The risks of copper cable and aluminum alloy cable are simulated in the numerical simulations. Assuming that two types of cable are under the same condition of operation, the system parameters are as follows: the load power of the cable supply $L=300$ kW; the expectation of the 4load forecasting deviation rate is 1.06%; the variance of the load forecasting deviation rate is 0.87%; the coefficient between the indirect failure cost and the direct failure cost $a=20$; the average maintenance cost of the fault for cable $C_{mf}=0.2$ million RMB per 100 km. The convergence criterion of the Monte Carlo simulation is the variance coefficient of the expected energy not supplied less than 0.01. The failure rate and the mean failure maintenance time of aluminum alloy cable and copper cable are obtained from State Grid Company. The failure rate of the aluminum alloy cable is 2.2-times per year per 100 km, while the failure rate of copper cable is two-times per year per 100 km. The mean failure maintenance time of aluminum alloy cable is $T_E=8$ h per time, while the mean failure maintenance time of copper cable is $T_E=8.8$ h per time. Simulation results are shown in Figure 3 and Figure 4. We can see that the simulation of the energy-not-supplied for both cables can be convergent after 3000-times of sampling. The expected energy-not-supplied of the aluminum alloy cable is $EENSc=5253.59$ kW·h, while it is $EENSc=4847.78$ kW·h for copper cable.

2.4. Discard Cost

Discard cost $C_d$ refers to the cost that is used to clean up and destroy the engineering project after its service life has ended. Part of the equipment has residual value, which can be sold to get some economic benefits. The discard cost of the power line includes the economic loss of early retirement and removal disposal cost, and it should have the residual cost subtracted. We have:

$$\begin{array}{ccc}\hfill C_d& =& (C_{rd}+C_{dd}-C_{sd})I_3\hfill \end{array}$$

(29)

$$\begin{array}{ccc}\hfill I_3& =& \frac{1}{{(1+i)}^n}\hfill \end{array}$$

(30)

where $C_{rd}$ is the economic loss of early retirement; $C_{dd}$ is the removal disposal cost; $C_{sd}$ is the residual cost; $I_3$ is the discount rate of the last year.

For cables, residual cost can be divided into:

$$C_{dd}=C_{ld}+C_{md}+C_{td}+C_{gd}$$

(31)

where $C_{ld}$ is the labor cost for removing the line; $C_{md}$ is the machine cost for removing the line; $C_{td}$ is the transportation cost; $C_{gd}$ is the land usage cost, which is converted by the land volume occupied by the insulation and sheath materials.

The residual cost $C_{sd}$ can be formulated as:

$$C_{sd}=M_{c}L{P}_c+\sum M_{i}L{P}_i$$

(32)

where $M_c$ is the metal weight of the line per kilometer; L is the length of the line; $P_c$ is the unit price of the discarded metal of the line; $M_i$ is the metal weight of the accessories per kilometer; $P_i$ is the unit price of the discarded metal of the accessories.

For overhead conductors, the discard cost does not include the land usage cost because of no insulation and sheath materials. However, $C_{fd}$, which is the cost of removing the pole tower and leveling land, should be included. Therefore, we have:

$$C_d^{\prime }=C_{rd}+C_{ld}+C_{md}+C_{td}+C_{ed}+C_{fd}-C_{sd}$$

(33)

2.5. Discussion of Three Types of Cable

Until now, the model of each period of LCC has been established. Combining the four parts together, Equation (1) can be obtained as the LCC model of the power lines. For different types of power lines, the main methods of calculating LCC cost are similar. However, there are some detailed differences. For instance, the accessories of aluminum alloy cable and overhead conductor are different, resulting in their different investment cost; the cables have energy consumption in the insulating medium when calculating operation and maintenance cost, but the overhead conductor does not; the overhead conductor does not have land usage cost, but has leveling land cost compared to cables. Therefore, one should take notice of these differences.

3. Software Development and Case Analysis

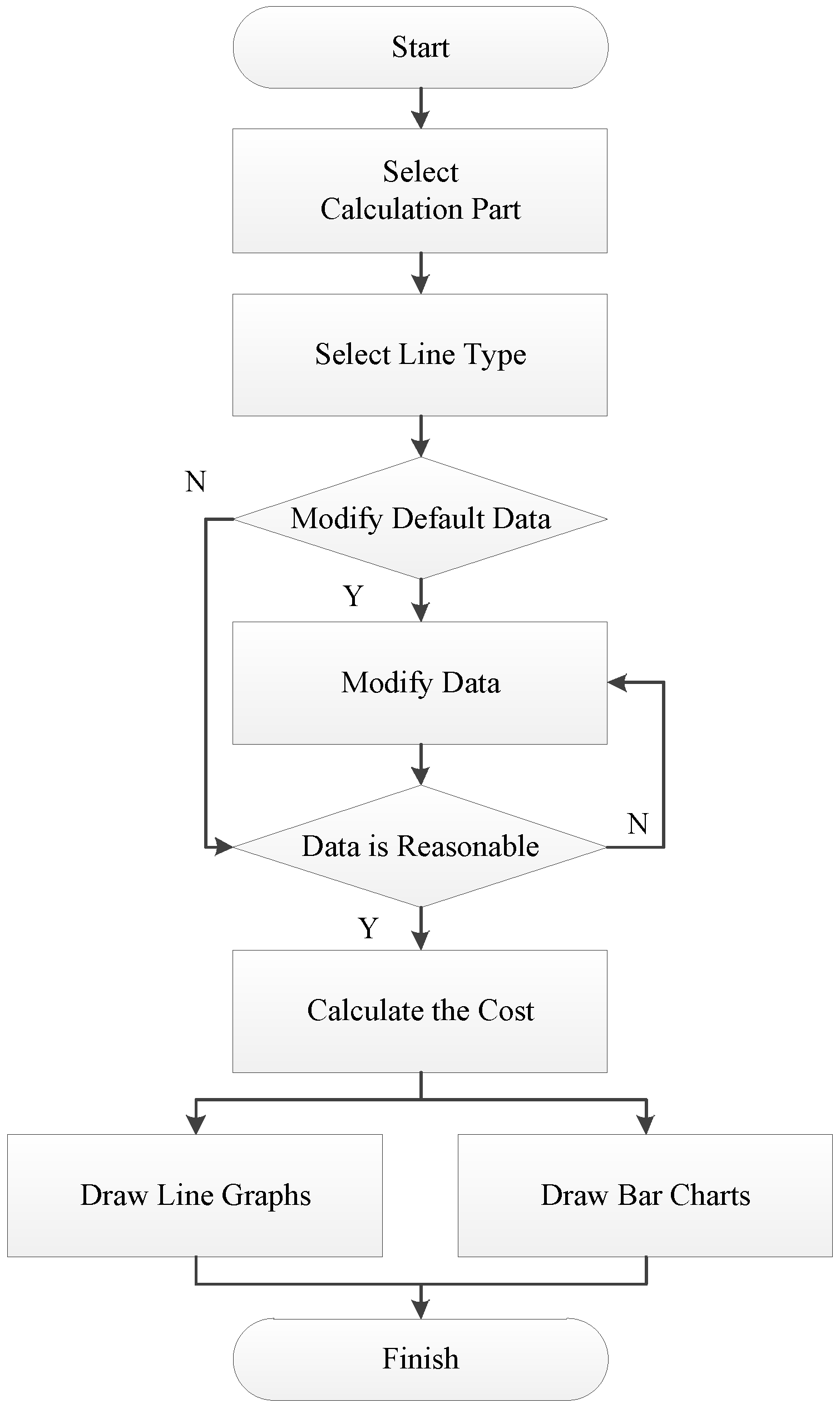
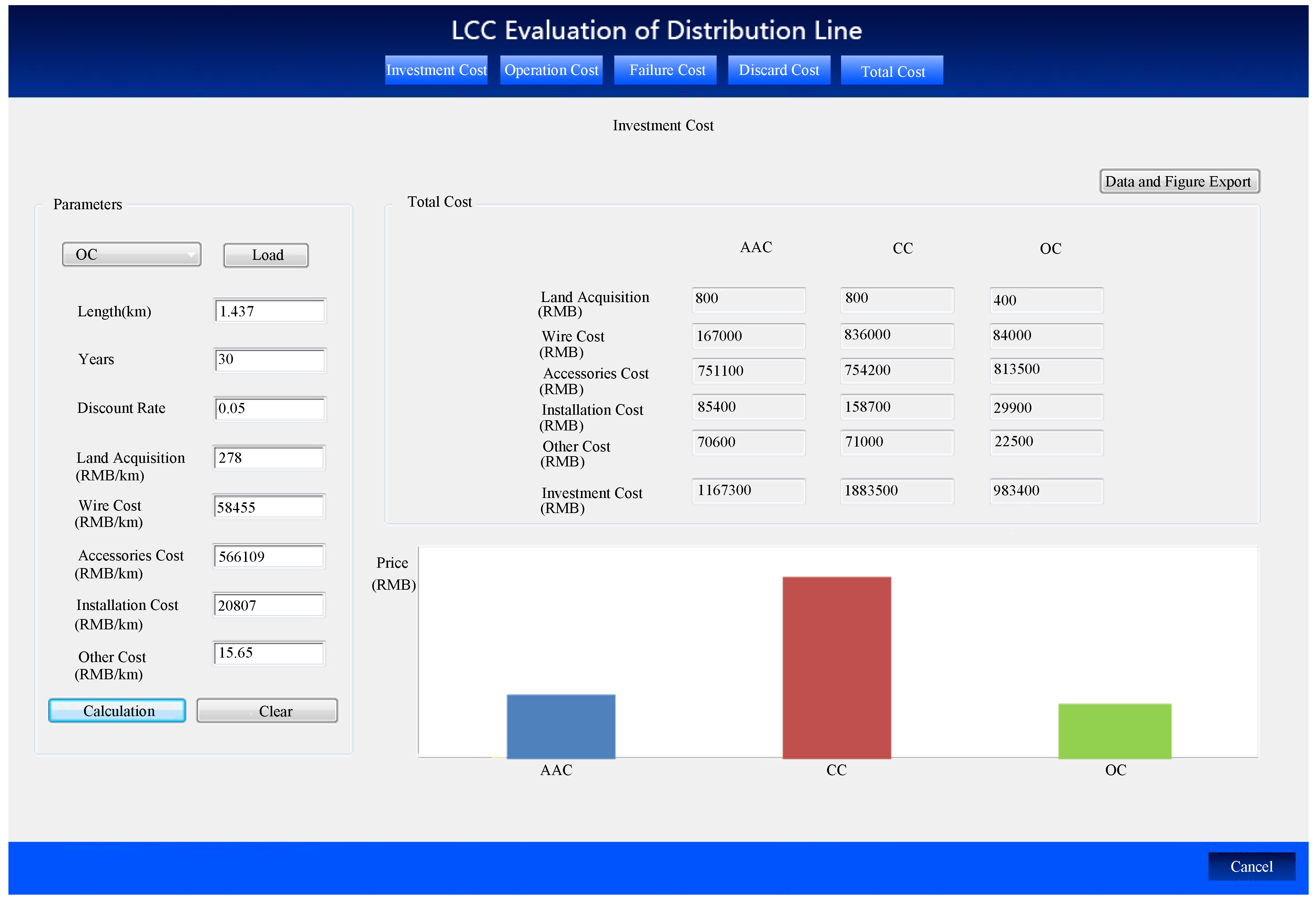
3.1. Software Development

To improve the convenience of the LCC model for power lines in the distribution network and make it easy to use, an LCC evaluation software based on the model we have built is developed. The software is designed with object-oriented programming ideas. C++ is used as the development language, and Microsoft Visual Studio 2010 (Microsoft, Redmond, WA, USA) is used as the integrated development tool. The flow chart of the software is shown in Figure 5, and parts of the interface are shown in Figure 6.

The software calculates the LCC of a power line according to the data provided by the power company and the parameters of the line and uses the line graphs and the bar charts to visually display the results of each part, which can make the user clear of the ratio of each part at a glance and can provide a better reference for the LCC control or the cable selection based on LCC. The whole software is divided into five parts, and they are the investment cost part, the operation and maintenance cost part, the failure cost part, the discard cost part and the total cost part. Each part uses a separate interface. The first four computing interfaces have their own data input frame, the result display frame and the drawing module. The last total cost interface is used to compare each part of the cost, using bar charts and line graphs to clearly show the proportion and the trend of each part of the cost. When the software initializes, each data input frame has a default value. If users want to change the data, they can input new data to the data input frame manually. The input parameters will be imported into the background program and handled. Then, the software will update the calculated results and draw the line graphs and bar charts in real time. The software has a high running efficiency and is easy to operate; therefore, it greatly improves the convenience of the application of the LCC model in practical power line projects.

3.2. Case 1

According to the LCC model that has been built, we choose two practical engineering projects in Fujian province of China for case analysis, using the LCC calculation software. The first case is a 10-kV power line in a southern county of Fujian province, and aluminum alloy cable, copper cable and overhead conductor are used, respectively. Taking the practical situation into consideration, the model of aluminum alloy cable is YJLHV22-3 × 300; the model of the copper cable is YJV22-3 × 240; and the model of the overhead conductor is LGJ-240. The purchasing parameters, physical parameters, operation and maintenance parameters, failure parameters and discard parameters are respectively shown in

Table 1. Purchasing parameters in Case 1. AAC, aluminum alloy cable; CC, copper cable; OC, overhead conductor.

Parameter	AAC	CC	OC
Purchasing Cost of Wire (RMB)	167,000	836,000	84,000
Purchasing Cost of Accessories (RMB)	751,100	754,200	813,500
Land Acquisition Cost (RMB)	800	800	400
Design and Installation Cost (RMB)	85,400	158,700	29,900
Other Cost (RMB)	70,600	71,000	22,500

,

Table 2. Physical parameters in Case 1.

Parameter	AAC	CC	OC
Wire Length (m)	1437	1437	1437
Maximum Load Current (A)	430	430	430
Line Voltage (kV)	10	10	10
AC Resistance (Ω)	0.131	0.0972	0.132
Operating Hour of One Year (h)	8760	8760	8760
Annual Average Load Rate (%)	45.66	45.66	45.66
Frequency (Hz)	50	50	50
Phase Working Capacity ($\mathsf{\mu }$F)	0.341	0.330	0
Dielectric Loss Tangent	0.008	0.008	0

,

Table 3. Operation and maintenance parameters in Case 1.

Parameter	AAC	CC	OC
Electricity Purchasing Price (RMB)	0.4	0.4	0.4
Electricity Sale Price (RMB)	0.5	0.5	0.5
Price Annual Increasing Rate (%)	2.0	2.0	2.0
Annual Discount Rate (%)	5	5	5
Inspection Cost (RMB)	125,300	91,100	5600
Inspection Cycle (times per year)	1	1	1
Maintenance Cost (RMB)	6300	4353	5853
Maintenance Cycle (times per year)	12	12	12
Recondition Cost (RMB)	8350	6530	8780
Recondition Cycle (times per year)	12	12	12

,

Table 4. Failure parameters in Case 1.

Parameter	AAC	CC	OC
Load Power (kW·h)	4300	4300	4300
Expectation of Load Forecasting Deviation Rate	1.06	1.06	1.06
Variance of Load Forecasting Deviation Rate	0.66	0.87	0.95
Failure Rate (times/year·100 km)	2.2	2	2.1
Average Failure Maintenance Time (h)	8	8.8	6
Coefficient between Indirect Failure Cost and Direct Failure Cost	20	20	20
Average Repair Cost (RMB/km)	3740	3566	2114

and

Table 5. Discard parameters in Case 1.

Parameter	AAC	CC	OC
Removal Disposal Cost (RMB)	10,345	13,753	3129
Subsequent Treatment Cost (RMB)	2047.7	2047.7	8372
Metal Weight of Wire (t/km)	2.44	6.41	1.108
Metal Weight of Accessories (t/km)	0.3	0.3	1.0
Recycling Price of Aluminum Alloy (RMB/t)	12,110	–	–
Recycling Price of Copper (RMB/t)	–	30,800	–
Recycling Price of Steel (RMB/t)	–	–	5500

. For convenience, the aluminum alloy cable is abbreviated as ACC, the copper cable as CC and the overhead conductor as OC.

We calculate the LCC of this power line in a design life of 30 years. The results are drawn in Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11.

3.2.1. Case 1 Result Analysis

From Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11, the detailed analyses are as follows.

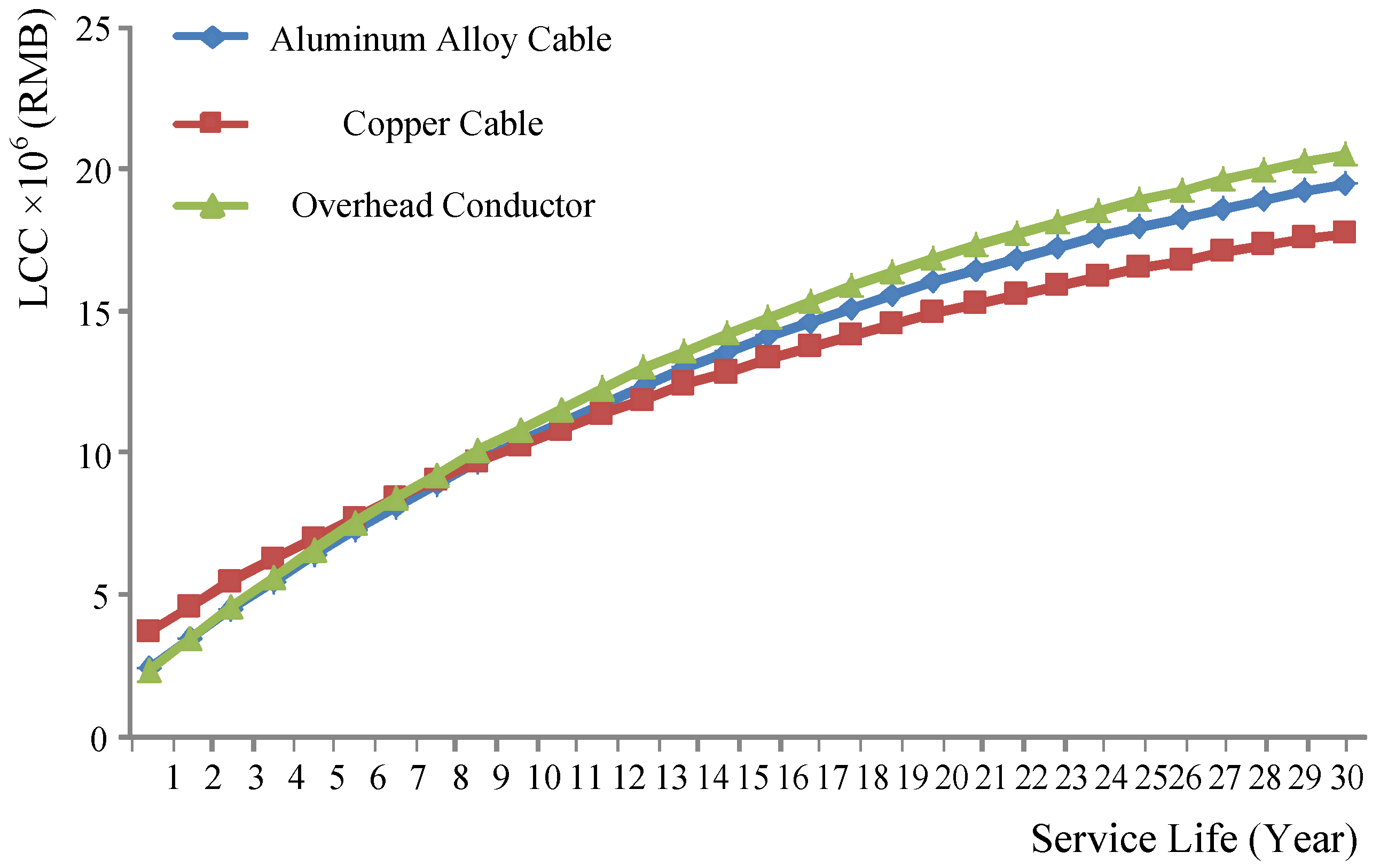
Each part of LCC

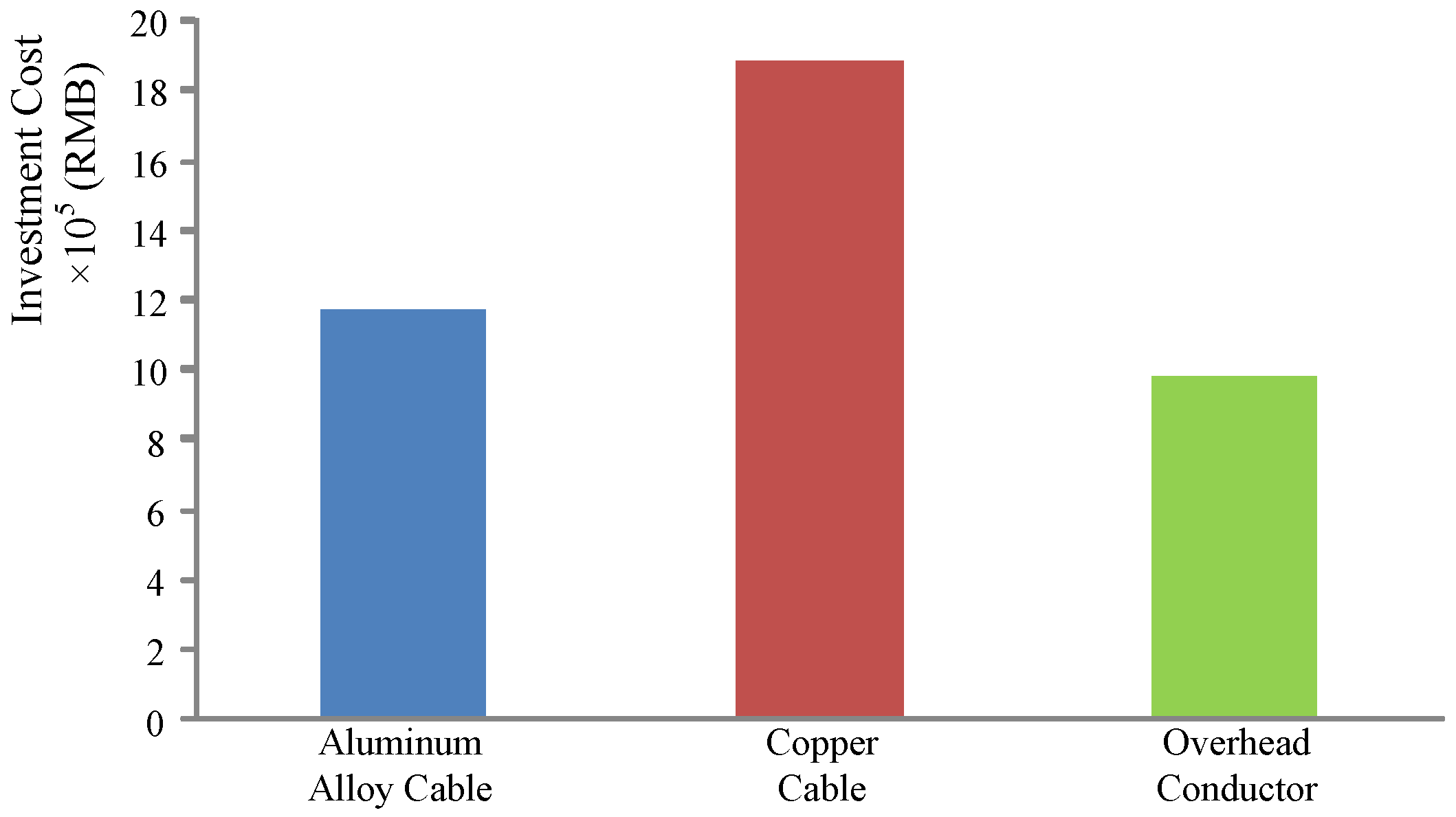
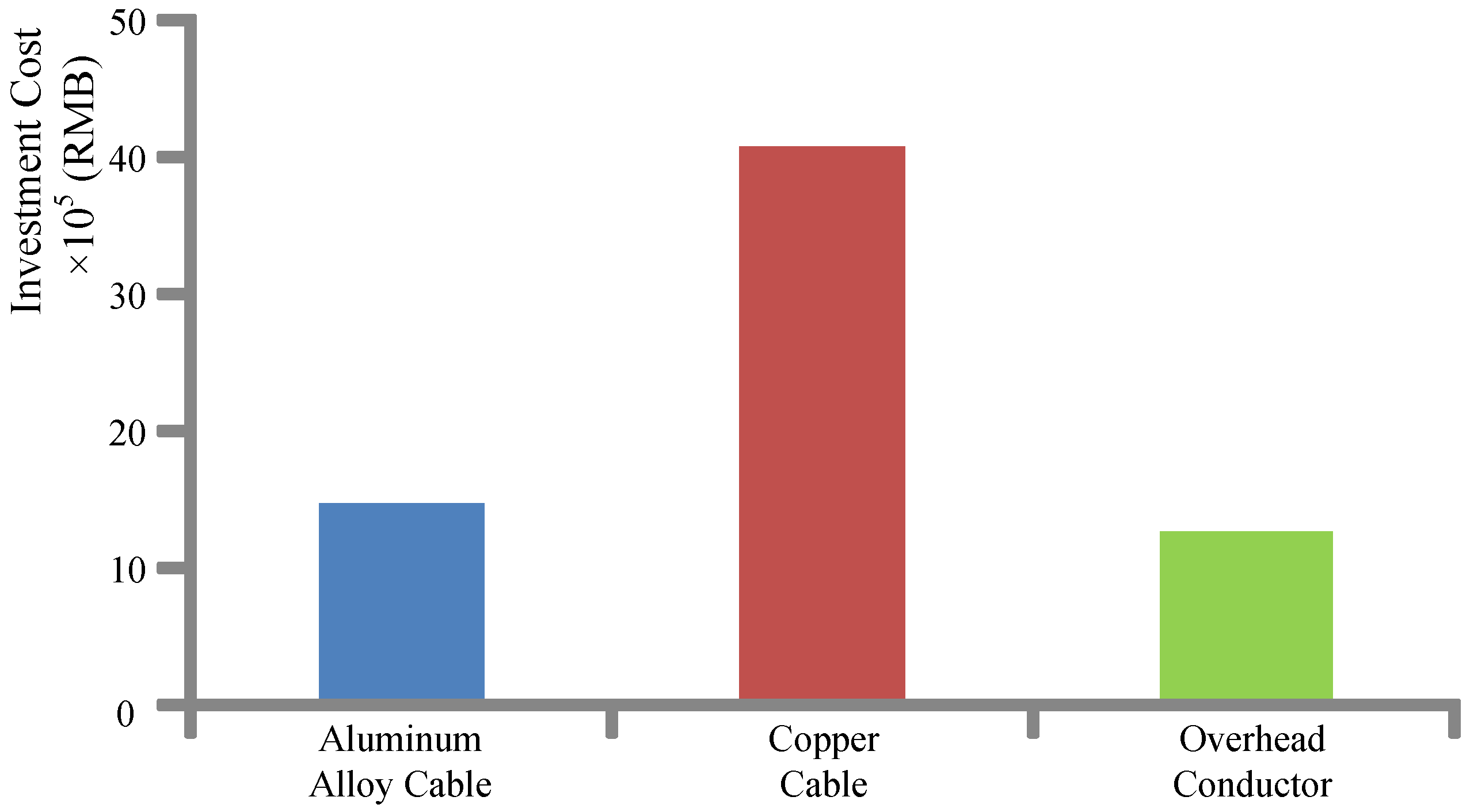
• Investment cost:
  From Figure 8, it is clear that the overhead conductor is the most economical line, while the copper cable requires the highest investment cost.
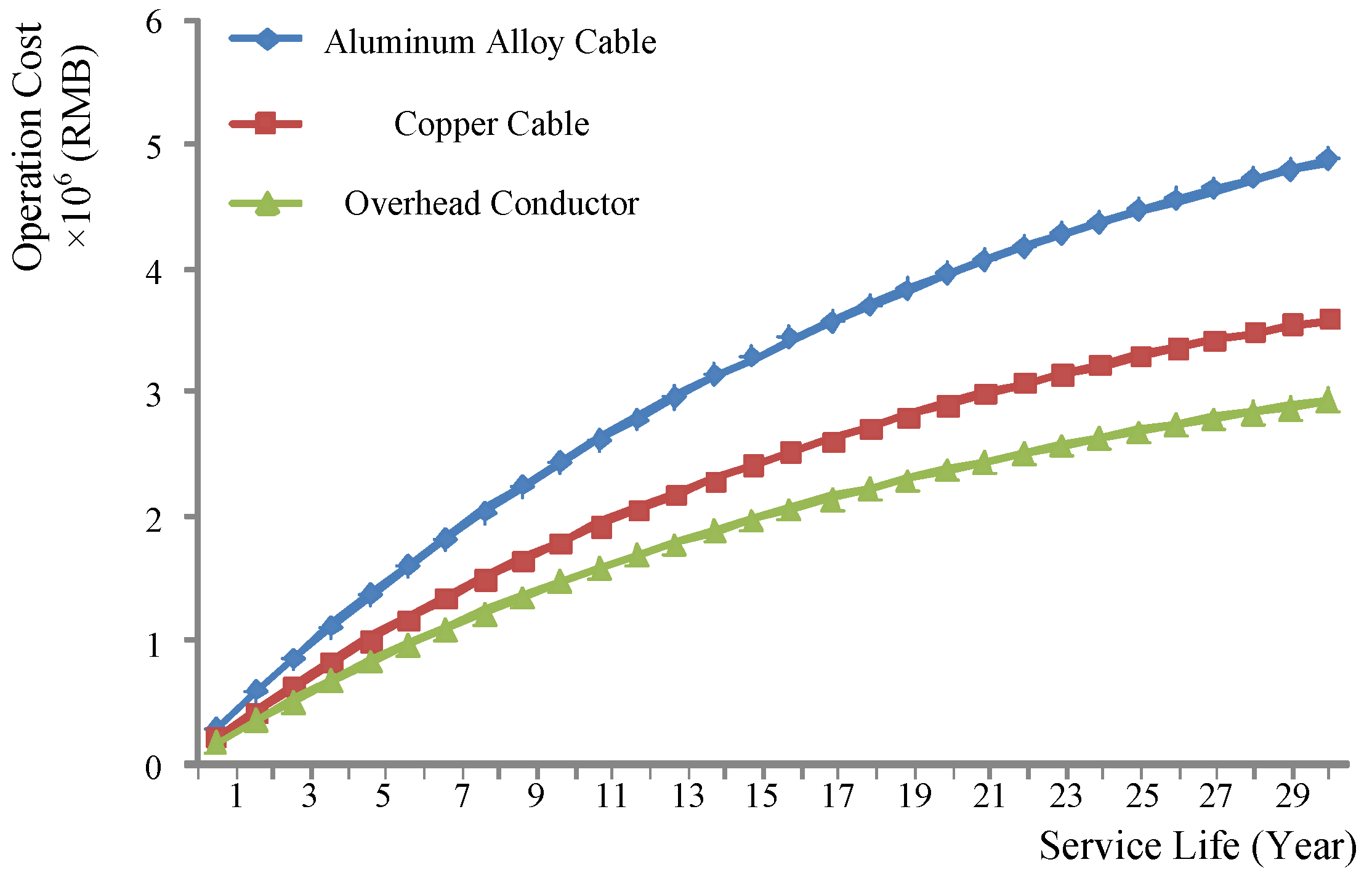
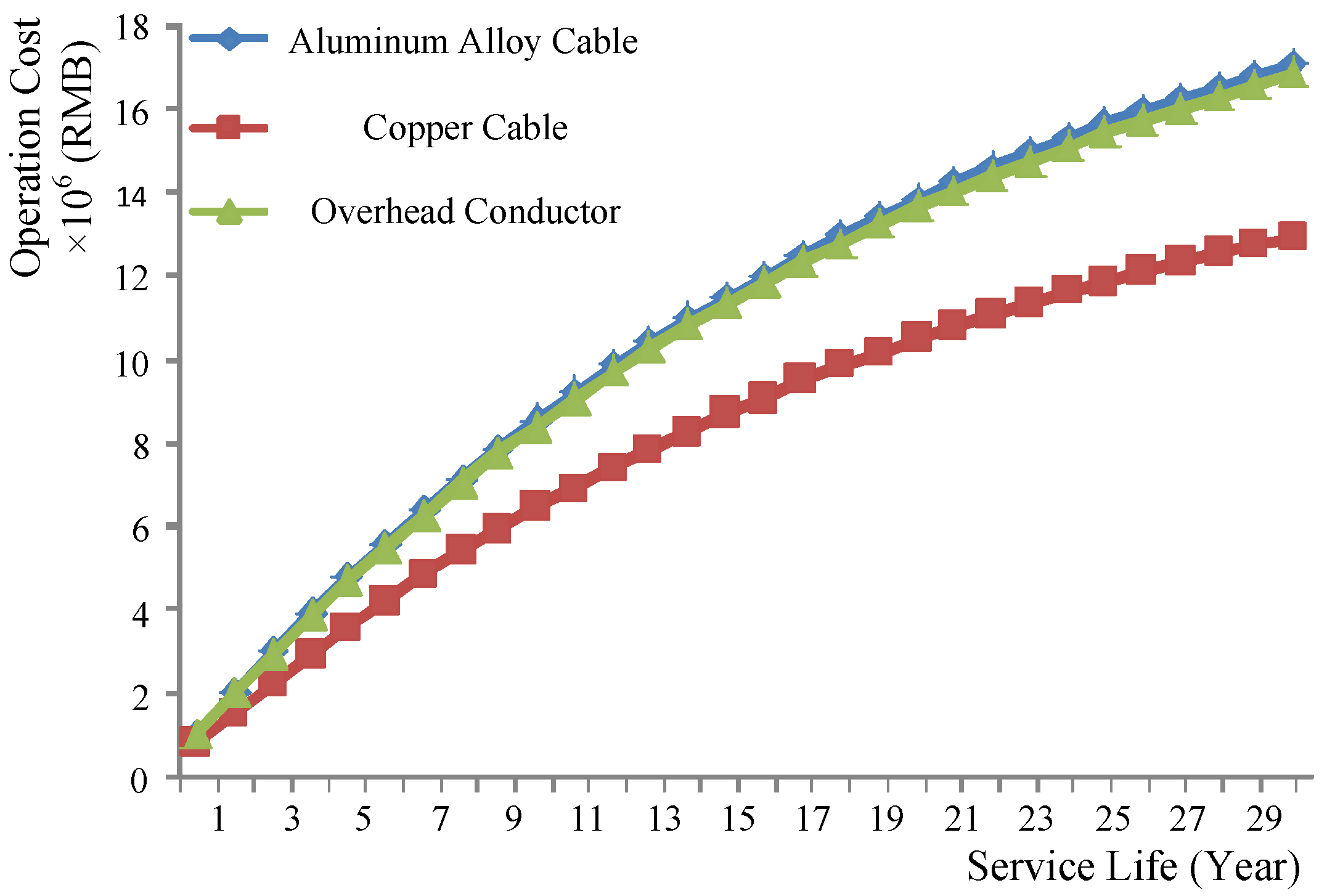
• Operation and maintenance cost:
  Figure 9 indicates that the overhead conductor is still the most economical line, but the aluminum alloy cable becomes the most expensive line in this period.
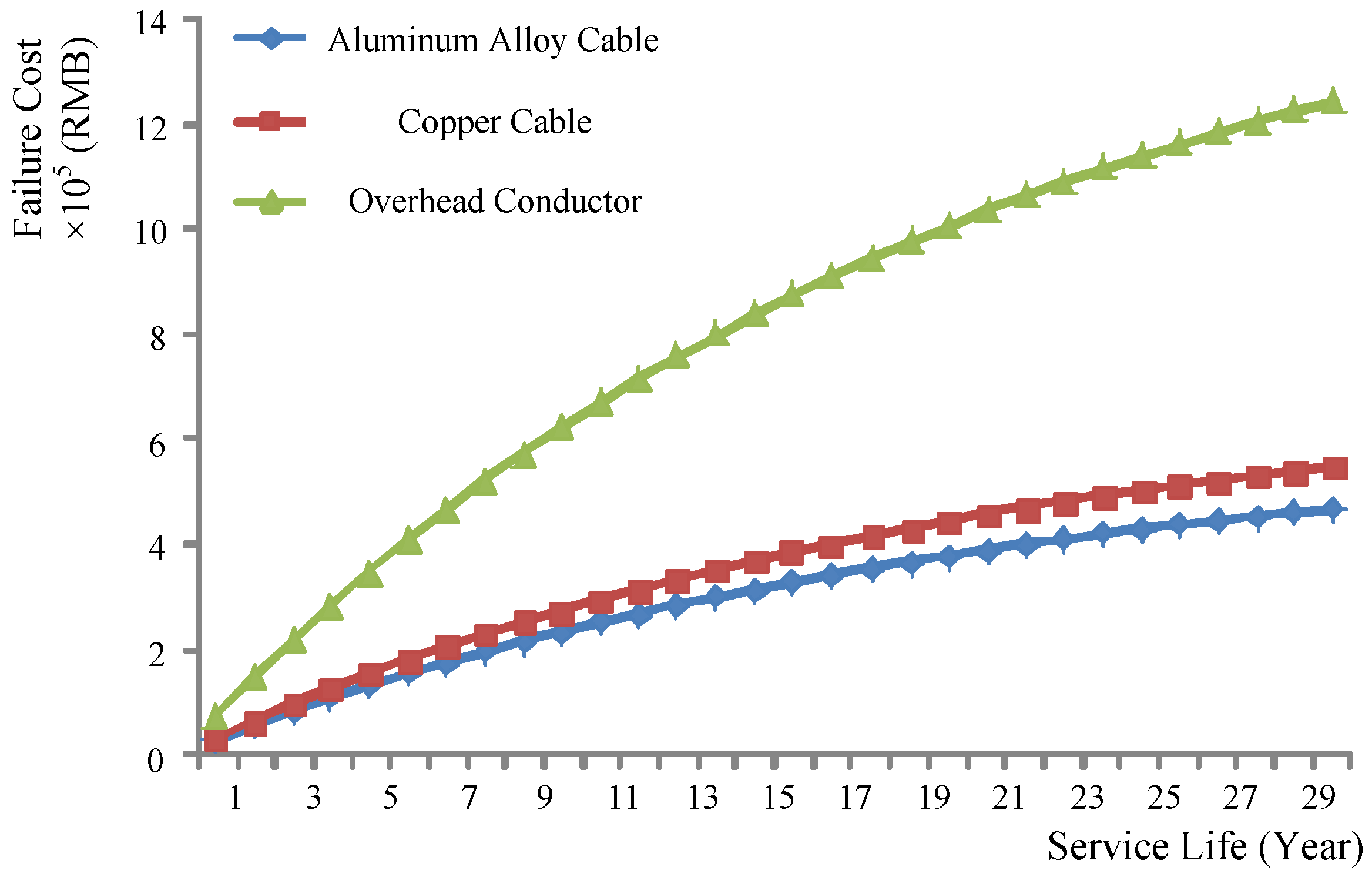
• Failure cost:
  From Figure 10, it can be seen that the aluminum alloy cable has the least failure cost, while the overhead conductor has the most.
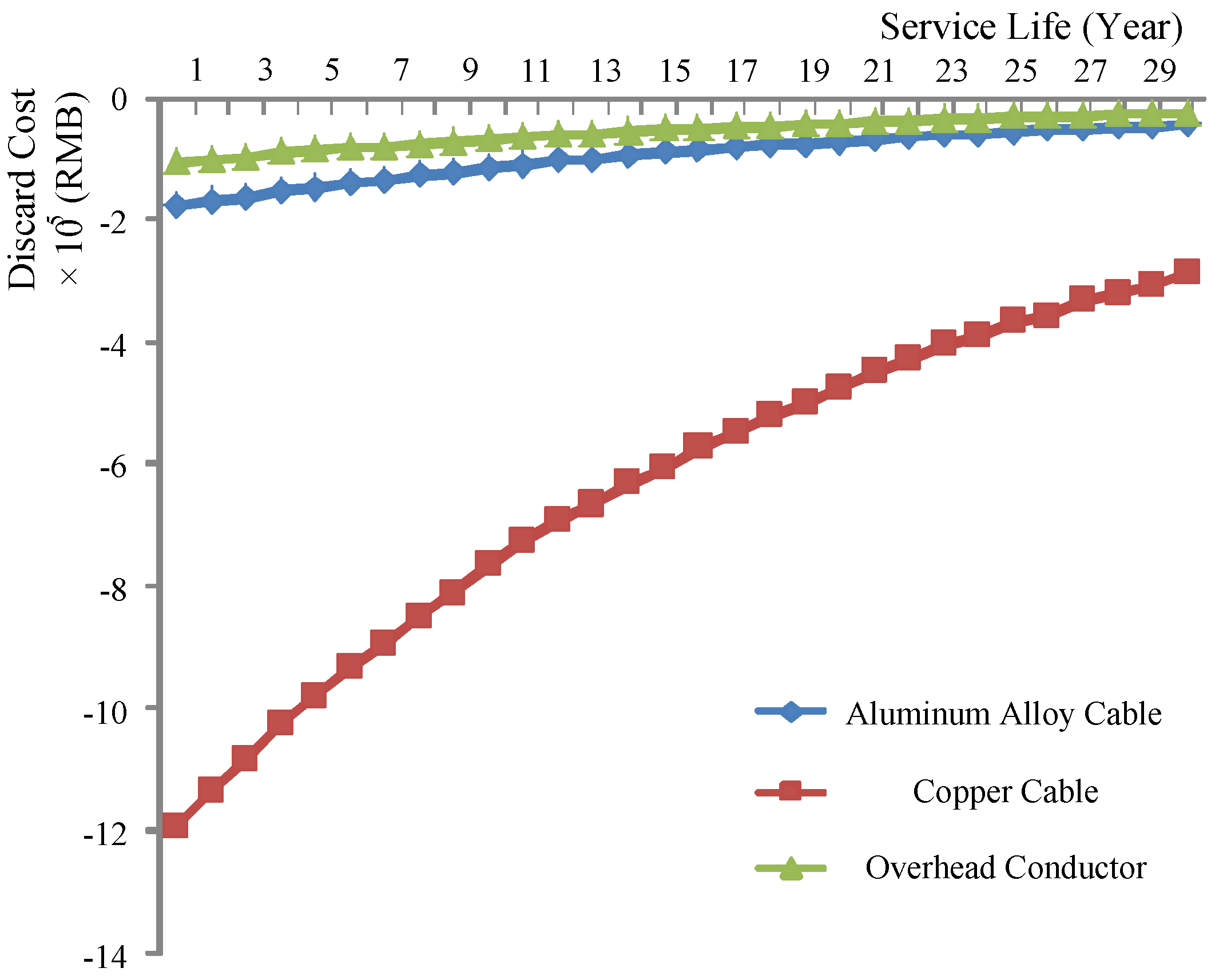
• Discard cost:
  Figure 11 illustrates that the copper cable has the least discard cost while the overhead conductor has the top cost. It is necessary to point out that the discard cost is negative because the residual cost of power lines is actually a kind of income. By recycling and selling the discard power line materials, the income can be used to offset the cost in other parts. As a result, the lower the discard cost is, the more it does to reduce the total LCC.

Influence degree of each part to LCC

In Case 1, the load rate is low; therefore, the operation and maintenance cost does not have the biggest influence on the LCC. Figure 8, Figure 9, Figure 10 and Figure 11 indicate that the investment cost takes the most part of the LCC while the failure cost and the discard cost have a minor influence on the LCC.

LCC balance point

From Figure 7, it can be seen that the LCC line graph of aluminum alloy cable has an intersection with the LCC line graph of the copper cable in the ninth year. This indicates that when the service life is less than nine years, the aluminum alloy cable has an economic advantage. However, due to short length and low load rate of the line, the advantage is not obvious. When the service life is longer than nine years, the copper cable is more economical. Although the investment cost of copper cable is higher, the energy loss is smaller, which leads to an advantage in long-term operation.

It is clear that the overhead conductor has an obvious economic advantage throughout the whole service life compared to other lines, due to two characteristics of the overhead conductor: the low investment cost and the low maintenance cost.

Therefore, when designing short-length and low load rate power lines, represented by Case 1, the overhead conductor is the most economical.

3.3. Case 2

The second line is a dual-circuit 10-kV power line, which is in a northern county of Fujian province. The model of aluminum alloy cable is YJLHV22-3 × 400; the model of the copper cable is YJV22-3 × 300; and the model of the overhead conductor is LGJ-240. The calculation parameters are shown in

Table 6. Purchasing parameters in Case 2.

Parameter	AAC	CC	OC
Purchasing Cost of Wire (RMB)	817,000	3,249,000	288,000
Purchasing Cost of Accessories (RMB)	119,800	112,100	77,200
Land Acquisition Cost (RMB)	800	800	400
Design and Installation Cost (RMB)	467,500	597,000	865,500
Other Cost (RMB)	100,600	107,500	33,600

,

Table 7. Physical parameters in Case 2.

Parameter	AAC	CC	OC
Wire Length (m)	4908	4908	4908
Maximum Load Current (A)	495	495	495
Line Voltage (kV)	10	10	10
AC Resistance (Ω)	0.103	0.0788	0.132
Operating Hour of One Year (h)	8760	8760	8760
Annual Average Load Rate (%)	62.79	62.79	62.79
Frequency (Hz)	50	50	50
Phase Working Capacity ($\mathsf{\mu }$F)	0.382	0.370	0
Dielectric Loss Tangent	0.008	0.008	0

,

Table 8. Operation and maintenance parameters in Case 2.

Parameter	AAC	CC	OC
Electricity Purchasing Price (RMB)	0.2	0.2	0.2
Electricity Sale Price (RMB)	0.5	0.5	0.5
Price Annual Increasing Rate (%)	2.0	2.0	2.0
Annual Discount Rate (%)	5	5	5
Inspection Cost (RMB)	250,600	182,200	18,800
Inspection Cycle (times per year)	1	1	1
Maintenance Cost (RMB)	4410	3208	1984
Maintenance Cycle (times per year)	12	12	12
Recondition Cost (RMB)	6614	4812	2976
Recondition Cycle (times per year)	12	12	12

,

Table 9. Failure parameters in Case 2.

Parameter	AAC	CC	OC
Load Power (kW·h)	4950	4950	4950
Expectation of Load Forecasting Deviation Rate	1.06	1.06	1.06
Variance of Load Forecasting Deviation Rate	0.66	0.87	0.95
Failure Rate (times/year·100 km)	2.2	2	2.1
Average Failure Maintenance Time (h)	8	8.8	6
Coefficient between Indirect Failure Cost and Direct Failure Cost	20	20	20
Average Repair Cost (RMB/km)	6588	10,980	2745

and

Table 10. Discard parameters in Case 2.

Parameter	AAC	CC	OC
Removal Disposal Cost (RMB)	17,666	23,486	5344
Subsequent Treatment Cost (RMB)	3496.9	3496.9	14,297
Metal Weight of Wire (t/km)	3.252	8.01	2.655
Metal Weight of Accessories (t/km)	0.3	0.3	1.0
Recycling Price of Aluminum Alloy (RMB/t)	12,110	–	–
Recycling Price of Copper (RMB/t)	–	30,800	–
Recycling Price of Steel (RMB/t)	–	–	5500

.

The LCC of this power line is figured out in a lifespan of 30 years. The line graphs are shown in Figure 12, Figure 13, Figure 14, Figure 15 and Figure 16.

3.3.1. Case 2 Result Analysis

From Figure 12, Figure 13, Figure 14, Figure 15 and Figure 16, the detailed analyses are as follows.

Each part of LCC

• Investment cost:
  Figure 13 shows that the investment cost of the overhead conductor is the least, while the investment cost of copper cable is the highest.
• Operation and maintenance cost:
  Figure 14 indicates that the operation and maintenance cost of copper cable is the least. However, the aluminum alloy cable and overhead conductor are similar.
• Failure cost:
  From Figure 15, it can be seen that the failure cost of aluminum alloy cable is less than copper, cable and the failure cost of copper cable is less than the overhead conductor.
• Discard cost:
  Figure 16 illustrates that the discard cost of copper cable is the least, while the discard cost of the overhead conductor is the most.

Influence degree of each part to LCC

In Case 2, due to the high load rate, the operation and maintenance cost becomes the most important influence factor of the LCC. Figure 13, Figure 14, Figure 15 and Figure 16 show that the investment cost takes the second place, and the failure cost and the discard cost still have a minor influence on the LCC.

LCC balance point

From Figure 12, it can be seen that the LCC balance point of the three lines appears at the 7th–8th year. In other words, when the service life is less than seven years, the copper cable is the most expensive, resulting from its high investment cost. Besides, the LCC of the aluminum alloy cable and the overhead conductor are very similar before the eighth year. As a result, choosing which type of line depends on the appearance requirements.

When the service life is longer than the eighth year, the copper cable gradually forms an economic advantage. For this high load rate and dual-circuit line, although the investment cost of the aluminum alloy cable is only 38% of the copper cable, the operation energy loss of the copper cable is much less than the other two types of lines. Therefore, from a long-term perspective, the copper cable is a better choice.

4. Conclusions

In this paper, the LCC of the 10 kV power line is studied. The power lines’ LCC model is established by analyzing each period of its life cycle, which include the investment period, operation and maintenance period, failure period and discard period. Besides, the economic differences among the aluminum alloy cable, copper cable and overhead conductor are compared through two practical engineering projects in Fujian province in China by an LCC evaluation software we developed. In these two cases, the LCC of a distribution power line is closely related to its working condition. The analysis results can be summarized as follows.

• Considering line length and capacity:
  When the line length is relatively short and its capacity is relatively small, the investment cost has the biggest influence on the LCC. Therefore, a low investment cost line is the better choice. However, when the line length is relatively long and its capacity is relatively large, the operation and maintenance cost takes the first place in the LCC, which leads to the low energy loss line becoming a better choice.
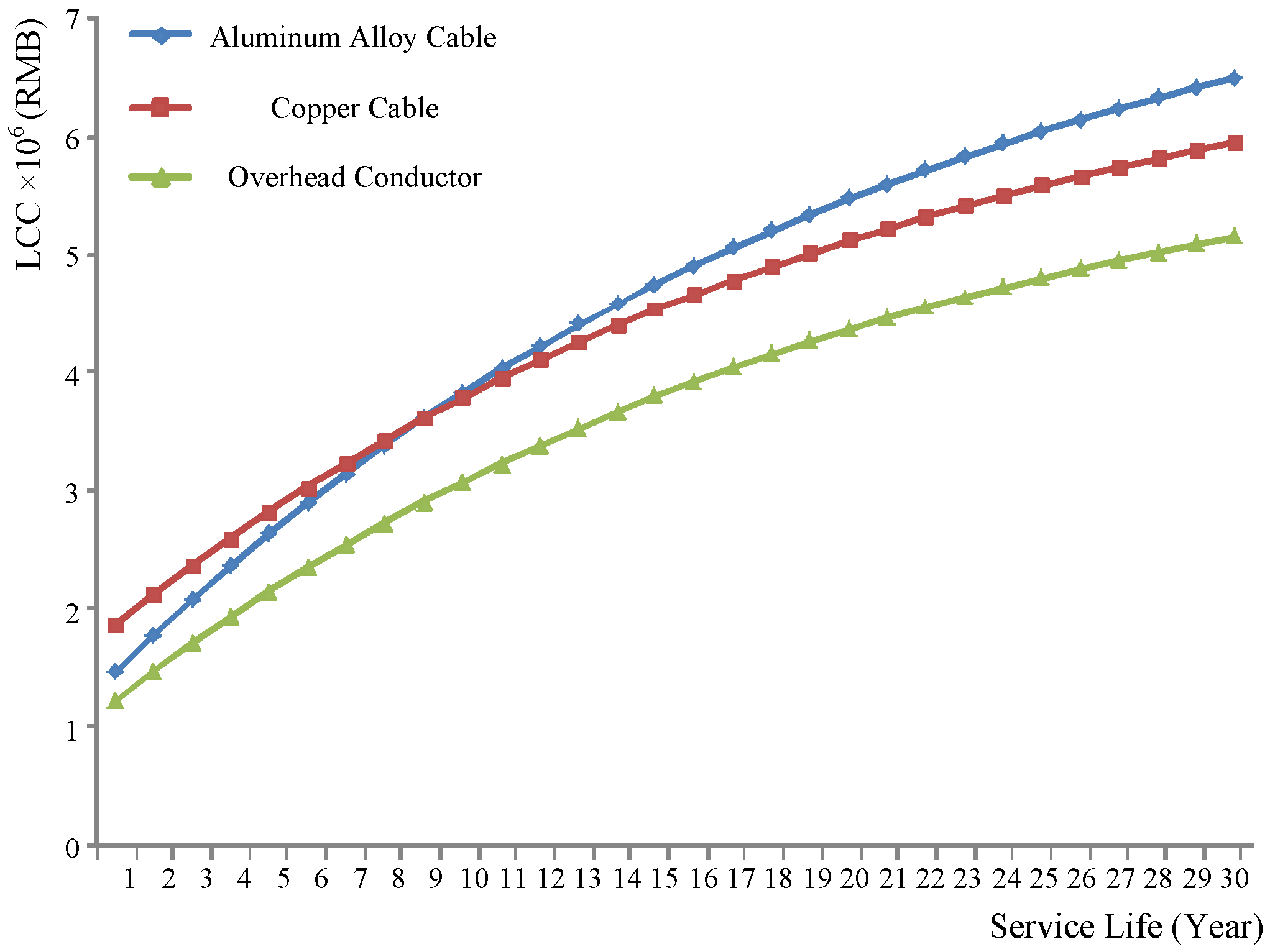
• Considering service life:
  When the service life is less than 10 years, the aluminum alloy cable or the overhead conductor is better than the copper cable. However, when the service life is more than 10 years, the copper cable is more economical.

This paper provides a kind of LCC model used in engineering applications for cable selection and can be a reference for further research on the LCC of the 10-kV power line.