Ultrafast Pump–Probe Spectroscopy in Organic Dirac Electron Candidate α-(BETS)2I3
Abstract
:1. Introduction
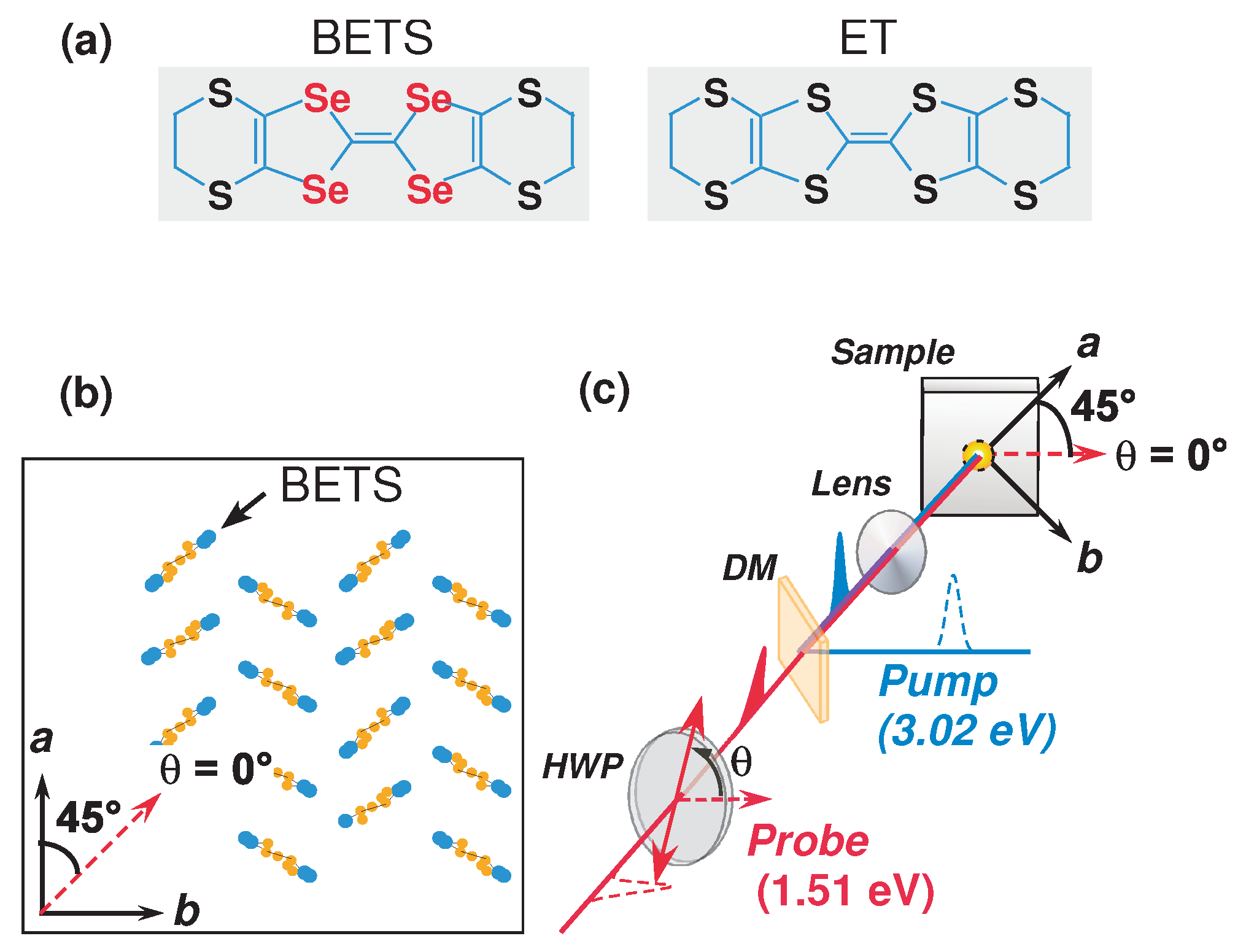
2. Materials and Methods
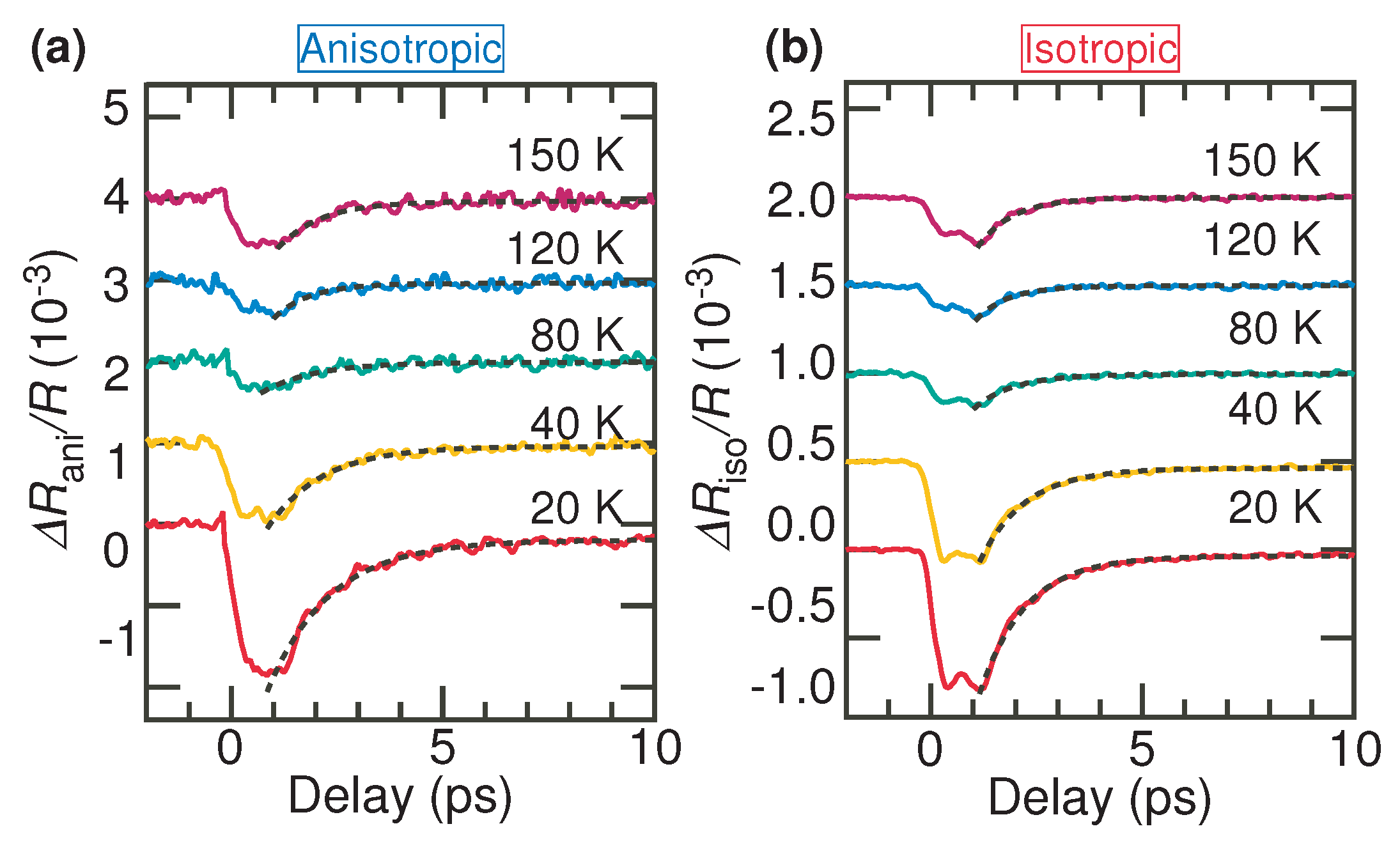
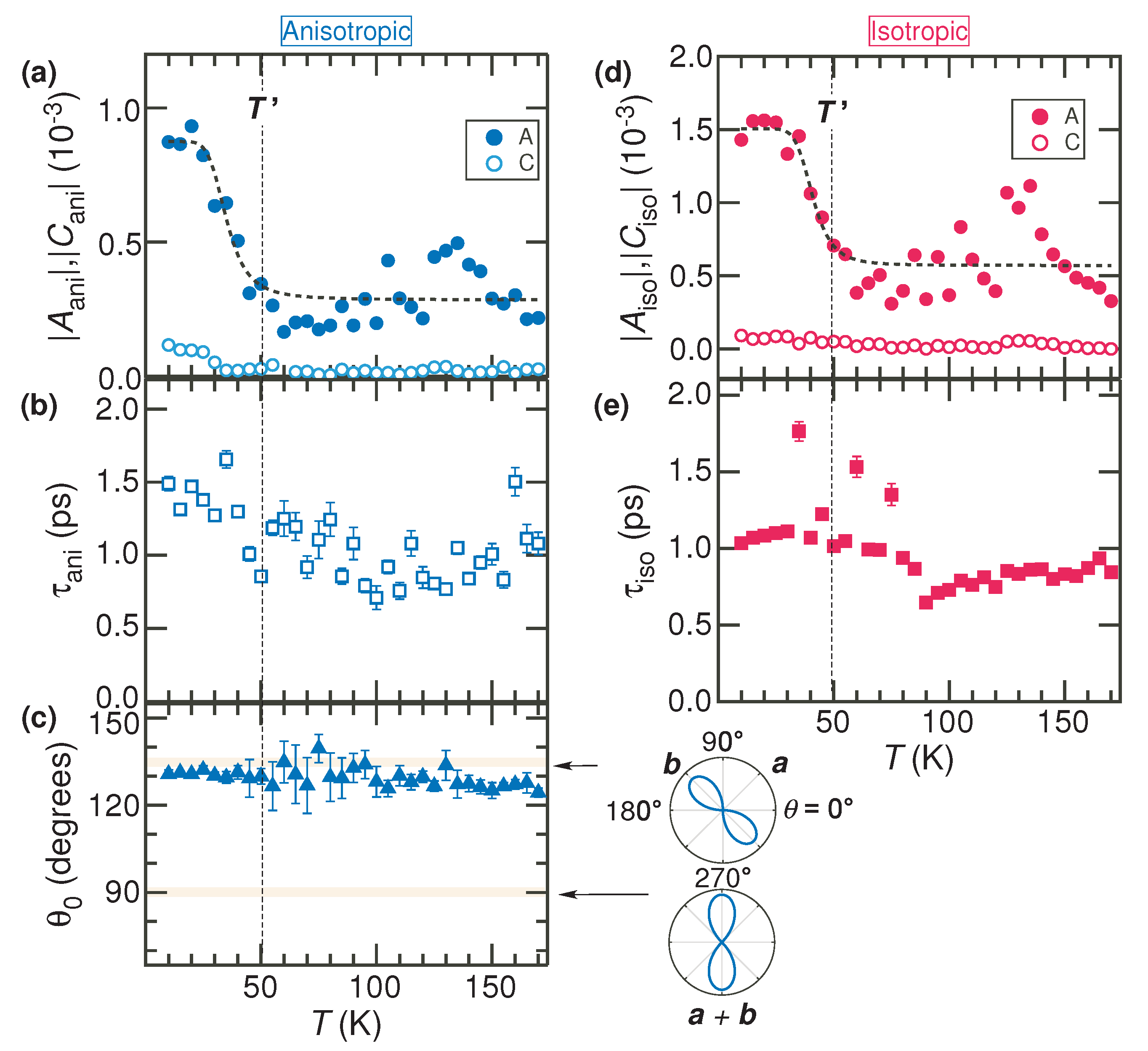
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ardavan, A.; Brown, S.; Kagoshima, S.; Kanoda, K.; Kuroki, K.; Mori, H.; Ogata, M.; Uji, S.; Wosnitza, J. Recent Topics of Organic Superconductors. J. Phys. Soc. Jpn. 2012, 81, 011004. [Google Scholar] [CrossRef]
- Naito, T. Modern History of Organic Conductors: An Overview. Crystals 2021, 11, 838. [Google Scholar] [CrossRef]
- Kato, R.; Kobayashi, H.; Kobayashi, A. Synthesis and properties of bis(ethylenedithio)tetraselenafulvalene (BEDT-TSeF) compounds. Synth. Met. 1991, 42, 2093–2096. [Google Scholar] [CrossRef]
- Kondo, R.; Kagoshima, S.; Tajima, N.; Kato, R. Crystal and Electronic Structures of the Quasi-Two-Dimensional Organic Conductor α-(BEDT-TTF)2I3 and Its Selenium Analogue α-(BEDT-TSeF)2I3 under Hydrostatic Pressure at Room Temperature. J. Phys. Soc. Jpn. 2009, 78, 114714. [Google Scholar] [CrossRef]
- Morinari, T.; Suzumura, Y. On the Possible Zero-Gap State in Organic Conductor α-(BEDT-TSF)2I3 under Pressure. J. Phys. Soc. Jpn. 2014, 83, 094701. [Google Scholar] [CrossRef]
- Tsumuraya, T.; Suzumura, Y. First-principles study of the effective Hamiltonian for Dirac fermions with spin-orbit coupling in two-dimensional molecular conductor α-(BETS)2I3. Eur. Phys. J. B 2021, 94, 17. [Google Scholar] [CrossRef]
- Kitou, S.; Tsumuraya, T.; Sawahata, H.; Ishii, F.; Hiraki, K.i.; Nakamura, T.; Katayama, N.; Sawa, H. Ambient-pressure Dirac electron system in the quasi-two-dimensional molecular conductor α-(BETS)2I3. Phys. Rev. B 2021, 103, 035135. [Google Scholar] [CrossRef]
- Oka, R.; Ohara, K.; Konishi, K.; Yamane, I.; Shimada, T.; Naito, T. Band Structure Evolution during Reversible Interconversion between Dirac and Standard Fermions in Organic Charge-Transfer Salts. Magnetochemistry 2023, 9, 153. [Google Scholar] [CrossRef]
- Shon, N.H.; Ando, T. Quantum Transport in Two-Dimensional Graphite System. J. Phys. Soc. Jpn. 1998, 67, 2421–2429. [Google Scholar] [CrossRef]
- Tajima, N.; Sugawara, S.; Tamura, M.; Nishio, Y.; Kajita, K. Electronic Phases in an Organic Conductor α-(BEDT-TTF)2I3: Ultra Narrow Gap Semiconductor, Superconductor, Metal, and Charge-Ordered Insulator. J. Phys. Soc. Jpn. 2006, 75, 051010. [Google Scholar] [CrossRef]
- Inokuchi, M.; Tajima, H.; Kobayashi, A.; Ohta, T.; Kuroda, H.; Kato, R.; Naito, T.; Kobayashi, H. Electrical and Optical Properties of α-(BETS)2I3 and α-(BEDT-STF)2I3. Bull. Chem. Soc. Jpn. 1995, 68, 547–553. [Google Scholar] [CrossRef]
- Ohki, D.; Yoshimi, K.; Kobayashi, A. Interaction-induced quantum spin Hall insulator in the organic Dirac electron system α-(BEDT-TSeF)2I3. Phys. Rev. B 2022, 105, 205123. [Google Scholar] [CrossRef]
- Ohki, D.; Yoshimi, K.; Kobayashi, A.; Misawa, T. Gap opening mechanism for correlated Dirac electrons in organic compounds α-(BEDT-TTF)2I3 and α-(BEDT-TSeF)2I3. Phys. Rev. B 2023, 107, L041108. [Google Scholar] [CrossRef]
- Hiraki, K.i.; Harada, S.; Arai, K.; Takano, Y.; Takahashi, T.; Tajima, N.; Kato, R.; Naito, T. Local Spin Susceptibility of α-D2I3 (D = bis(ethylendithio)tetraselenafulvalene (BETS) and bis(ethylendithio)dithiadiselenafulvalene (BEDT-STF)) Studied by 77Se NMR. J. Phys. Soc. Jpn. 2011, 80, 014715. [Google Scholar] [CrossRef]
- Kodama, K.; Nakamura, T.; Takahashi, T.; Ojima, E.; Kobayashi, H. Metal-insulator transition in α-(BEDT-TSeF)2I3 and α-(BEDT-TTF)2I3. Synth. Met. 1999, 103, 1963–1964. [Google Scholar] [CrossRef]
- Kawasugi, Y.; Masuda, H.; Uebe, M.; Yamamoto, H.M.; Kato, R.; Nishio, Y.; Tajima, N. Pressure-induced phase switching of Shubnikov–de Haas oscillations in the molecular Dirac fermion system α-(BETS)2I3. Phys. Rev. B 2021, 103, 205140. [Google Scholar] [CrossRef]
- Fujiyama, S.; Maebashi, H.; Tajima, N.; Tsumuraya, T.; Cui, H.B.; Ogata, M.; Kato, R. Large Diamagnetism and Electromagnetic Duality in Two-Dimensional Dirac Electron System. Phys. Rev. Lett. 2022, 128, 027201. [Google Scholar] [CrossRef]
- Konoike, T.; Terashima, T.; Uji, S.; Hattori, Y.; Kato, R. Magnetic Order in Organic Dirac Electron System α-(BETS)2I3. J. Phys. Soc. Jpn. 2022, 91, 043703. [Google Scholar] [CrossRef]
- Nomoto, T.; Imajo, S.; Akutsu, H.; Nakazawa, Y.; Kohama, Y. Correlation-driven organic 3D topological insulator with relativistic fermions. Nat. Commun. 2023, 14, 2130. [Google Scholar] [CrossRef]
- Tsuchiya, S.; Nakagawa, K.; Taniguchi, H.; Toda, Y. Polarization-resolved Ultrafast Spectroscopy in an Organic Mott Insulator κ-(BEDT-TTF)2Cu[N(CN)2]Cl. J. Phys. Soc. Jpn. 2019, 88, 074706. [Google Scholar] [CrossRef]
- Nakagawa, K.; Tsuchiya, S.; Taniguchi, H.; Toda, Y. Probing uniform and nonuniform charge ordering with polarized femtosecond optical pulses in geometrically frustrated θ-(BEDT-TTF)2MZn(SCN)4 (M= Rb, Cs). Phys. Rev. Res. 2023, 5, 013024. [Google Scholar] [CrossRef]
- Tsuchiya, S.; Kanai, N.; Oka, R.; Naito, T.; Toda, Y. Effects of Molecular Substitution in Organic Conductors α-(ET)2I3 and α-(STF)2I3 Studied by Polarized Femtosecond Spectroscopy. J. Phys. Soc. Jpn. 2023, 92, 094703. [Google Scholar] [CrossRef]
- Allen, P.B. Theory of thermal relaxation of electrons in metals. Phys. Rev. Lett. 1987, 59, 1460–1463. [Google Scholar] [CrossRef] [PubMed]
- Rothwarf, A.; Taylor, B.N. Measurement of Recombination Lifetimes in Superconductors. Phys. Rev. Lett. 1967, 19, 27–30. [Google Scholar] [CrossRef]
- Kabanov, V.V.; Demsar, J.; Podobnik, B.; Mihailovic, D. Quasiparticle relaxation dynamics in superconductors with different gap structures: Theory and experiments on YBa2Cu3O7-ffi. Phys. Rev. B 1999, 59, 1497–1506. [Google Scholar] [CrossRef]
- Bender, K.; Hennig, I.; Schweitzer, D.; Dietz, K.; Endres, H.; Keller, H.J. Synthesis, Structure and Physical Properties of a Two-Dimensional Organic Metal, Di[bis(ethylenedithiolo)tetrathiofulvalene] triiodide, (BEDT-TTF)+2I3. Mol. Cryst. Liq. Cryst. 1984, 108, 359–371. [Google Scholar] [CrossRef]
- Takano, Y.; Hiraki, K.; Yamamoto, H.; Nakamura, T.; Takahashi, T. Charge disproportionation in the organic conductor, α-(BEDT-TTF)2I3. J. Phys. Chem. Solids 2001, 62, 393–395. [Google Scholar] [CrossRef]
- Moroto, S.; Hiraki, K.-I.; Takano, Y.; Kubo, Y.; Takahashi, T.; Yamamoto, H.M.; Nakamura, T. Charge disproportionation in the metallic state of α-(BEDT-TTF)2I3. J. Phys. IV Fr. 2004, 114, 399. [Google Scholar] [CrossRef]
- Takahashi, T.; Nogami, Y.; Yakushi, K. Charge Ordering in Organic Conductors. J. Phys. Soc. Jpn. 2006, 75, 051008. [Google Scholar] [CrossRef]
- Yamamoto, K.; Iwai, S.; Boyko, S.; Kashiwazaki, A.; Hiramatsu, F.; Okabe, C.; Nishi, N.; Yakushi, K. Strong Optical Nonlinearity and its Ultrafast Response Associated with Electron Ferroelectricity in an Organic Conductor. J. Phys. Soc. Jpn. 2008, 77, 074709. [Google Scholar] [CrossRef]
- Dvorsek, D.; Kabanov, V.V.; Demsar, J.; Kazakov, S.M.; Karpinski, J.; Mihailovic, D. Femtosecond quasiparticle relaxation dynamics and probe polarization anisotropy in YSrxBa2−xCu4O8 (x = 0, 0.4). Phys. Rev. B 2002, 66, 020510. [Google Scholar] [CrossRef]
- Toda, Y.; Kawanokami, F.; Kurosawa, T.; Oda, M.; Madan, I.; Mertelj, T.; Kabanov, V.V.; Mihailovic, D. Rotational symmetry breaking in Bi2Sr2CaCu2O8+δ probed by polarized femtosecond spectroscopy. Phys. Rev. B 2014, 90, 094513. [Google Scholar] [CrossRef]
- Tsuchiya, S.; Sugawara, Y.; Tanda, S.; Toda, Y. Symmetry-dependent carrier relaxation dynamics and charge–density–wave transition in DyTe3 probed by polarized femtosecond spectroscopy. J. Opt. 2015, 17, 085501. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tsuchiya, S.; Katsumi, M.; Oka, R.; Naito, T.; Toda, Y. Ultrafast Pump–Probe Spectroscopy in Organic Dirac Electron Candidate α-(BETS)2I3. Condens. Matter 2023, 8, 88. https://doi.org/10.3390/condmat8040088
Tsuchiya S, Katsumi M, Oka R, Naito T, Toda Y. Ultrafast Pump–Probe Spectroscopy in Organic Dirac Electron Candidate α-(BETS)2I3. Condensed Matter. 2023; 8(4):88. https://doi.org/10.3390/condmat8040088
Chicago/Turabian StyleTsuchiya, Satoshi, Masato Katsumi, Ryuhei Oka, Toshio Naito, and Yasunori Toda. 2023. "Ultrafast Pump–Probe Spectroscopy in Organic Dirac Electron Candidate α-(BETS)2I3" Condensed Matter 8, no. 4: 88. https://doi.org/10.3390/condmat8040088
APA StyleTsuchiya, S., Katsumi, M., Oka, R., Naito, T., & Toda, Y. (2023). Ultrafast Pump–Probe Spectroscopy in Organic Dirac Electron Candidate α-(BETS)2I3. Condensed Matter, 8(4), 88. https://doi.org/10.3390/condmat8040088







