The Effect of Acyl Chain Position on the 2D Monolayer Formation of Monoacyl-sn-Glycerol at the Air/Water Interface: Quantum Chemical Modeling
Abstract
1. Introduction
2. Calculation Methodology
3. Results and Discussion
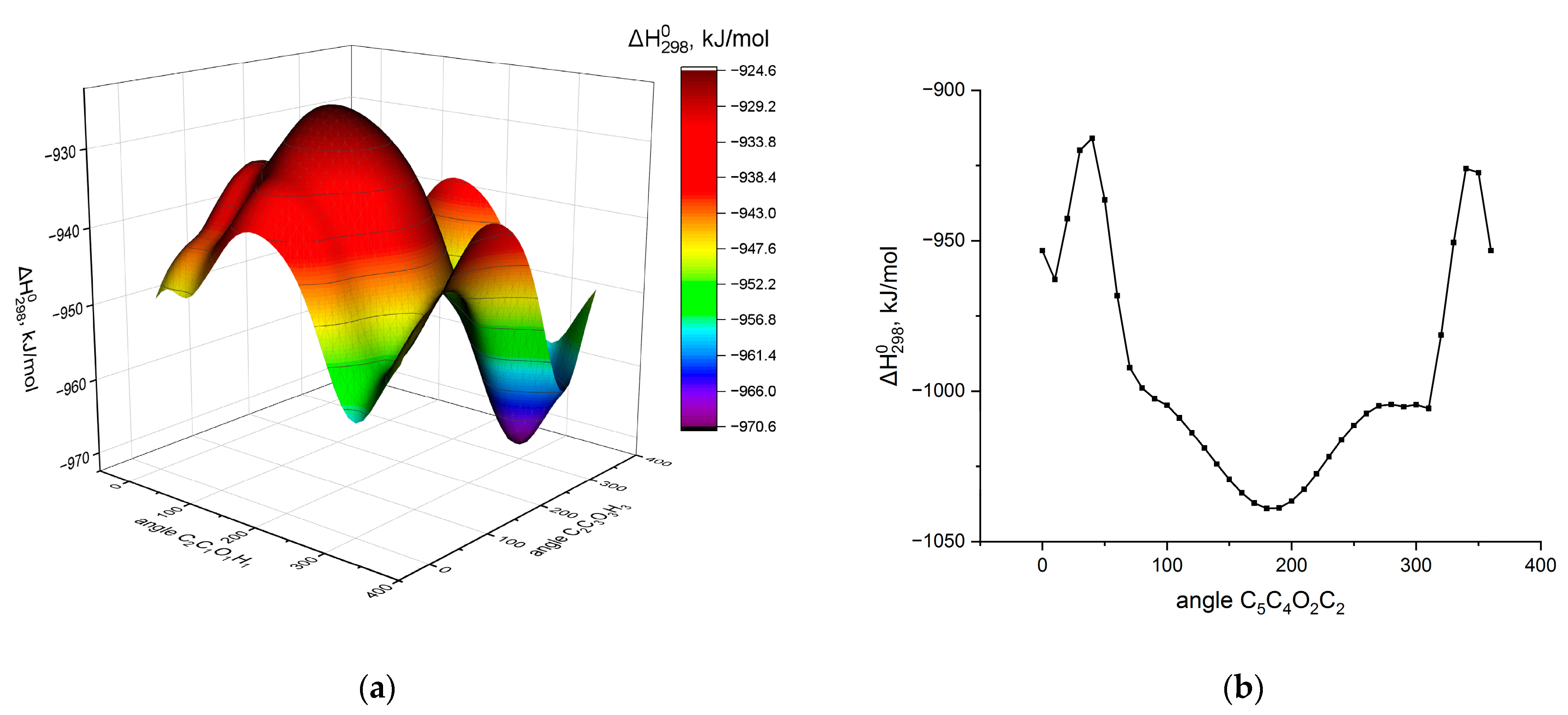
3.1. Conformational Analysis of Monomers
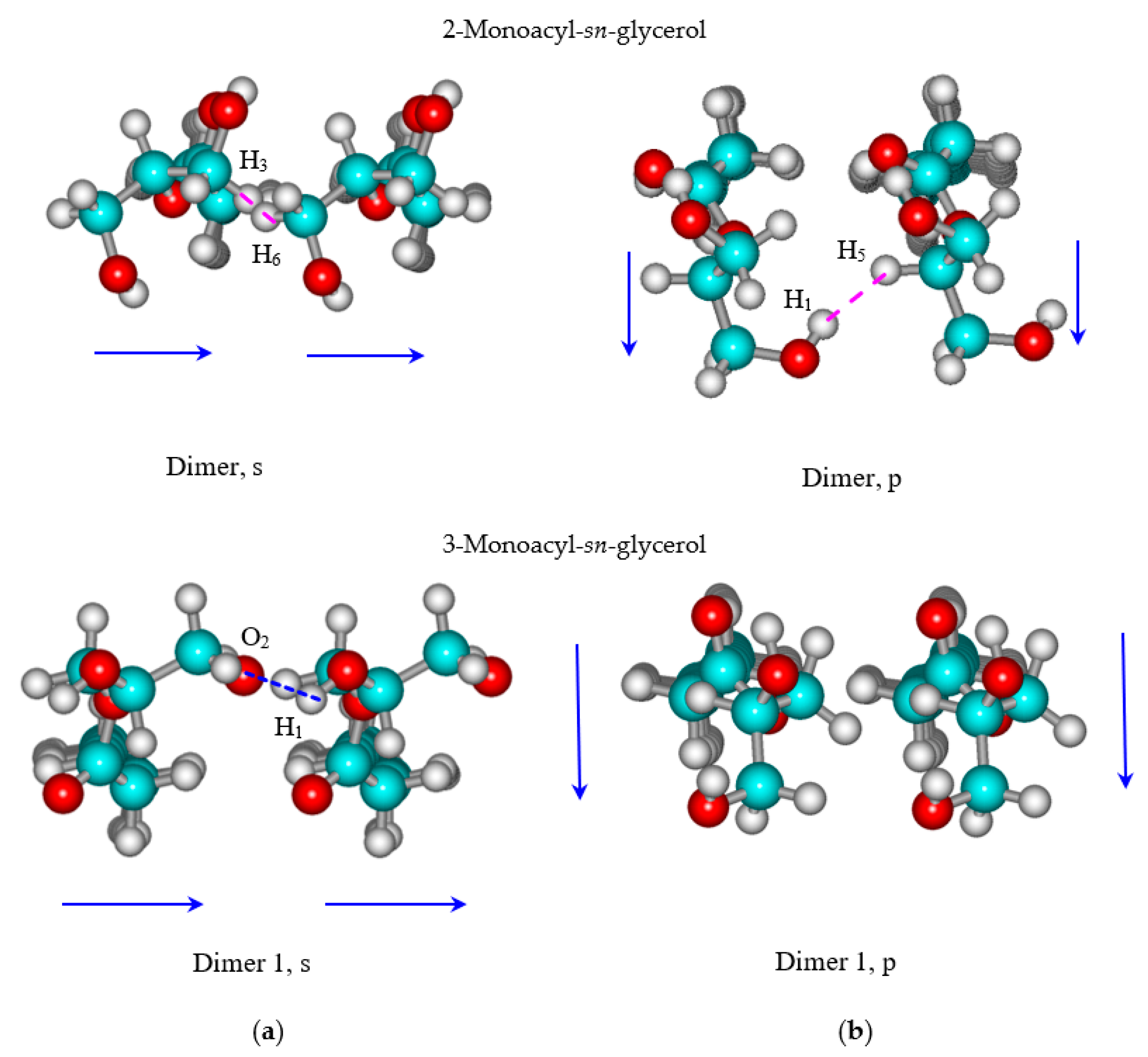
3.2. Dimers, Tetramers, and Other Small Clusters
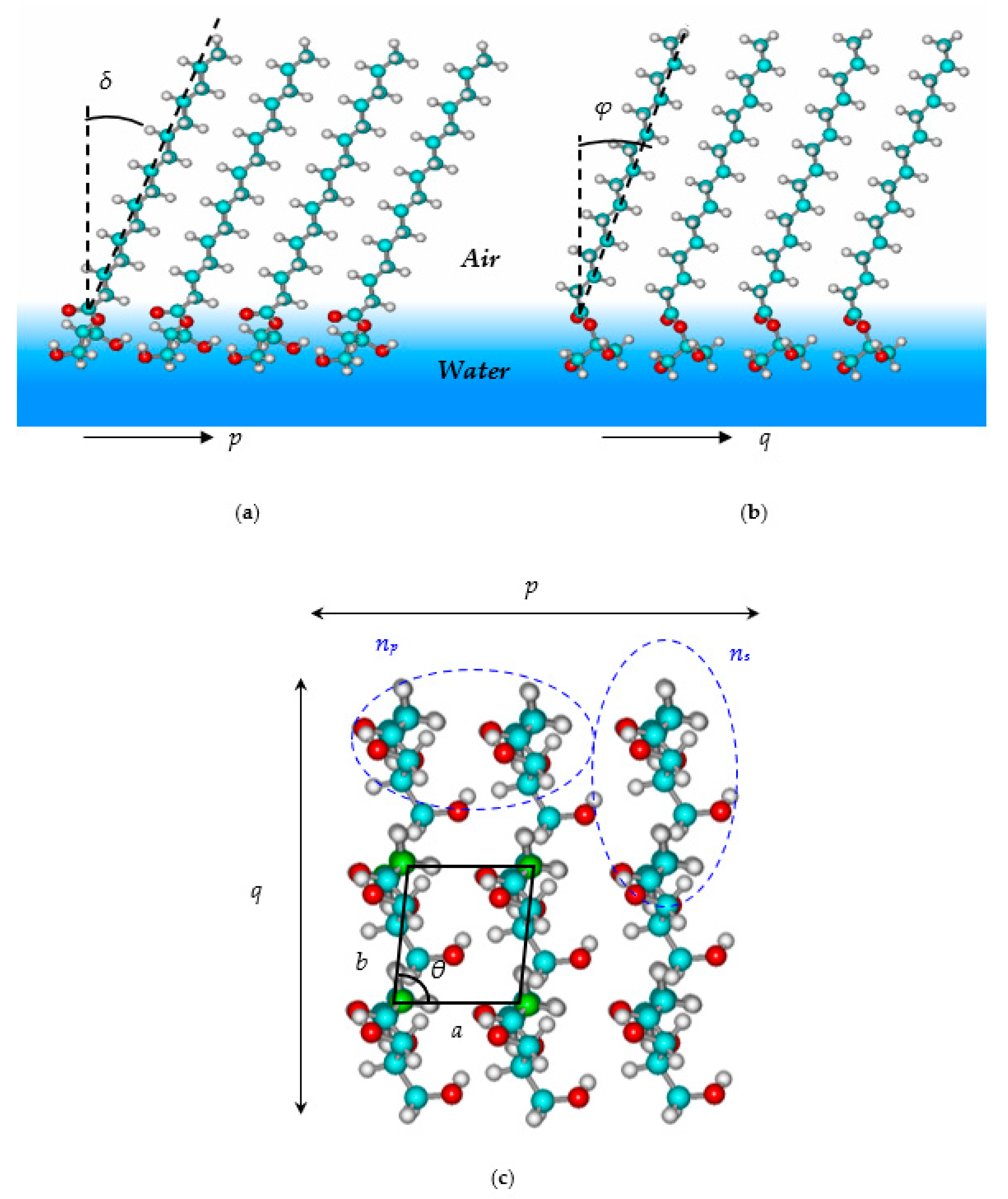
3.3. Large Clusters and 2D Films
4. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Conflicts of Interest
References
- Rarokar, N.R.; Menghani, S.; Kerzare, D.; Khedekar, B. Progress in synthesis of monoglycerides for use in food and pharmaceuticals. J. Exp. Food Chem. 2017, 3, 1000128. [Google Scholar] [CrossRef]
- Miao, S.; Lin, D. Monoglycerides: Categories, structures, properties, preparations, and applications in the food industry. In Encyclopedia of Food Chemistry; Melton, L., Shahidi, F., Varelis, P., Eds.; Academic Press: Cambridge, MA, USA, 2019; pp. 155–163. [Google Scholar] [CrossRef]
- Walz, M.-M.; Werner, J.; Ekholm, V.; Prisle, N.L.; Ohrwalld, G.; Bjoneholm, G.O. Alcohols at the aqueous surface: Chain length and isomer effects. Phys. Chem. Chem. Phys. 2016, 18, 6648–6656. [Google Scholar] [CrossRef] [PubMed]
- Vollhardt, D.; Siegel, S.; Cadenhead, D.A. Characteristic features of hydroxystearic acid monolayers at the air/water interface. J. Phys. Chem. B 2004, 108, 17448–17456. [Google Scholar] [CrossRef]
- Vollhardt, D.; Fainerman, V.B. Effect of the hydroxyl group position on the phase behavior of hydroxyoctadecanoic acid monolayers at the air/water interface. J. Phys. Chem. B 2004, 108, 297–302. [Google Scholar] [CrossRef]
- Wiedemann, G.; Brezesinski, G.; Vollhardt, D.; Möhwald, H. Disorder in langmuir monolayers. 1. disordered packing of alkyl chains. Langmuir 1998, 14, 6485–6492. [Google Scholar] [CrossRef]
- Kellner, B.M.; Cadenhead, D.A. Monolayer studies of hydroxyhexadecanoic acids. J. Colloid Interface Sci. 1978, 63, 452–460. [Google Scholar] [CrossRef]
- Huda, M.S.; Fujio, K.; Uzu, Y. Phase behavior of bipolar fatty acid monolayers. Bull. Chem. Soc. Jpn. 1996, 69, 3387–3394. [Google Scholar] [CrossRef]
- Neumann, V.; Gericke, A.; Huhnerfuss, H. Comparison of enantiomeric and racemic monolayers of 2-hydroxyhexadecanoic acid by external infrared reflection-absorption spectroscopy. Langmuir 1995, 11, 2206–2212. [Google Scholar] [CrossRef]
- Vollhardt, D.; Brezesinski, G.; Rudert, R.; Gonzalez, A. Effect of the alkanoyl group position at the glycerol backbone on the monolayer characteristics demonstrated by 2-monopalmitoyl-rac-glycerol. Langmuir 2017, 33, 12559–12568. [Google Scholar] [CrossRef]
- Vollhardt, D.; Emrich, G.; Siegel, S.; Rudert, R. phase transition in monolayers of straight chain and 2-methyl branched alcohols at the air-water interface. Langmuir 2002, 18, 6571–6577. [Google Scholar] [CrossRef]
- Sun, T.; Wyslouzil, B.E. Freezing of dilute aqueous-alcohol nanodroplets: The effect of molecular structure. J. Phys. Chem. B 2021, 125, 12329–12343. [Google Scholar] [CrossRef]
- Phan, C.M. Dynamic adsorption of octanols at air/water interface. Can. J. Chem. Eng. 2010, 88, 688–692. [Google Scholar] [CrossRef]
- Patino, J.M.R.; Sánchez, C.C.; Niño, M.R.R. Morphological and structural characteristics of monoglyceride monolayers at the air−water interface observed by brewster angle microscopy. Langmuir 1999, 15, 2484–2492. [Google Scholar] [CrossRef]
- Patino, J.M.R.; Sanchez, C.C.; Niño, M.R.R.; Cejudo, M.; Fernández, M.C. Structural dilatational characteristics relationships of monoglyceride monolayers at the air-water. Langmuir 2001, 17, 4003–4013. [Google Scholar] [CrossRef]
- Patino, J.M.R.; Sanchez, C.C.; Nino, M.R.R. Structural-dilatational characteristics relationships of monoglyceride monolayers at the air-water interface. J. Agric. Food Chem. 1999, 47, 4998–5008. [Google Scholar] [CrossRef]
- Vollhardt, D.; Brezesinski, G. Effect of chirality on monoacylglycerol ester monolayer characteristics: 3-monopalmitoyl-sn-glycerol. Colloids Surf. A Physicochem. Eng. Asp. 2017, 521, 281–293. [Google Scholar] [CrossRef]
- Vollhardt, D.; Brezesinski, G. Monolayer characteristics of 1-monostearoyl-rac-glycerol at the air-water interface. J. Phys. Chem. C 2015, 119, 9934–9946. [Google Scholar] [CrossRef]
- Pantoja-Romero, W.S.; Estrado-Lopez, E.D.; Picciani, H.S.; Olivera, O.N., Jr.; Lachter, E.R.; Pimentel, A.S. Efficient molecular packing of glycerol monostearate in Langmuir monolayers at the air-water interface. Colloids Surf. A Physicochem. Eng. Asp. 2016, 508, 85–92. [Google Scholar] [CrossRef]
- Kartashynska, E.S.; Vollhardt, D. 2D-Monolayer formation of 3-monoacyl-sn-glycerols at the air/water interface. A comprehensive quantum chemical approach. Colloids Surf. A Physicochem. Eng. Asp. 2023, 667, 131400. [Google Scholar] [CrossRef]
- Surjan, R.; Csaszar, P.; Poirier, R.A. Perturbation theoretical vs supermolecule calculations on intermolecular interactions. Acta Phys. Hung. 1990, 67, 387–400. [Google Scholar] [CrossRef]
- Michalkova, A.; Gorb, L.; Leszczynski, J. A quest for efficient methods of disintegration of organophosphorus compounds: Modeling adsorption and decomposition processes. In Molecular Materials with Specific Interactions—Modelling and Design; Sokalski, W.A., Ed.; Springer: Dordrecht, The Netherlands, 2007; pp. 565–592. [Google Scholar] [CrossRef]
- Hobza, P. Accurate ab initio calculations on large van der Waals clusters. Annu. Rep. Prog. Chem. Sect. C Phys. Chem. 1997, 93, 257–287. [Google Scholar] [CrossRef]
- Bijina, V.; Suresh, C.H. Molecular electrostatic potential reorganization theory to describe positive cooperativity in noncovalent trimer complexes. J. Phys. Chem. A 2020, 124, 7080–7087. [Google Scholar] [CrossRef] [PubMed]
- Grimme, S. Accurate description of van der Waals complexes by density functional theory including empirical corrections. J. Comput. Chem. 2004, 25, 1463–1473. [Google Scholar] [CrossRef] [PubMed]
- Vysotsky, Y.B.; Bryantsev, V.S.; Fainerman, V.B.; Vollhardt, D. Quantum chemical analysis of thermodynamics of the 2d cluster formation of odd n-alcohols at the air/water interface. J. Phys. Chem. B 2002, 106, 11285–11294. [Google Scholar] [CrossRef]
- Vysotsky, Y.B.; Belyaeva, E.A.; Kartashynska, E.S.; Fainerman, V.B.; Smirnova, N.A. Quantum chemical approach in the description of the amphiphile clusterization at the air/liquid and liquid/liquid interfaces with phase nature accounting. I. Aliphatic normal alcohols at the air/water interface. J. Phys. Chem. B 2015, 119, 3281–3296. [Google Scholar] [CrossRef]
- Thoma, M.; Schwendler, M.; Baltes, H.; Helm, C.A.; Pfohl, T.; Riegler, H.; Möhwald, H. Ellipsometry and X-ray reflectivity studies on monolayers of phosphatidylethanolamine and phosphatidylcholine in contact with n-dodecane, n-hexadecane, and bicyclohexyl. Langmuir 1996, 12, 1722–1728. [Google Scholar] [CrossRef]
- Knoble, C.M.; Desai, R.C. Phase transitions in monolayers. Annu. Rev. Phys. Chem. 1992, 43, 207–236. [Google Scholar] [CrossRef]
- Kurtz, R.E.; Toney, M.F.; Pople, J.A.; Lin, B.; Meron, M.; Majensky, J.; Lange, A.; Fuller, G.G. Langmuir monolayers of straight-chain and branched hexadecanol and eicosanol mixtures. Langmuir 2008, 24, 14005–14014. [Google Scholar] [CrossRef] [PubMed]
- Vollhardt, D.; Fainerman, V.B. Characterization of phase transition in adsorbed monolayers at the air/water interface. Adv. Coll. Interface Sci. 2010, 154, 1–19. [Google Scholar] [CrossRef]
- Weinbach, S.P.; Jacquemain, D.; Leveiller, F.; Kjaer, K.; Als-Nielsen, J.; Leiserowitz, L. Effect of cosolvent on the lateral order of spontaneously formed amphiphilic amide two-dimensional crystallites at the air-solution interface. J. Am. Chem. Soc. 1993, 115, 11110–11118. [Google Scholar] [CrossRef]
- Weissbuch, I.; Berfeld, M.; Bouwman, W.; Kjaer, K.; Als-Nielsen, J.; Lahav, M.; Leiserowitz, L. Separation of enantiomers and racemate formation in two-dimensions crystals at the water surface from racemic α-amino acid amphiphiles: Design and structure. J. Am. Chem. Soc. 1997, 119, 933–942. [Google Scholar] [CrossRef]
- Cholakova, D.; Denkov, N. Rotator phases in alkane systems: In bulk, surface layers and micro/nano-confinements. Adv. Colloid Interface Sci. 2019, 269, 7–42. [Google Scholar] [CrossRef] [PubMed]
- Stewart, J.J. MOPAC 2000.00 Manual; Fujitsu Limited: Tokyo, Japan, 1999. [Google Scholar]
- Csonka, G.I.; Angyan, J.C. The origin of the problems with the PM3 core repulsion function. J. Mol. Struct. 1997, 393, 31–38. [Google Scholar] [CrossRef]
- Metzger, T.G.; Ferguson, D.M.; Glauser, W.A. A computational analysis of interaction energies in methane and neopentane dimer system. J. Comput. Chem. 1997, 18, 70–79. [Google Scholar] [CrossRef]
- Vysotsky, Y.B.; Kartashynska, E.S.; Belyaeva, E.A.; Fainerman, V.B.; Vollhardt, D.; Miller, R. Computational quantum chemistry applied to monolayer formation at gas/liquid interfaces in Computational methods for complex liquid-fluid interfaces. In Progress in Colloid and Interface Science; Karbaschi, M., Miller, R., Rahni, M.T., Eds.; CRC: Boca Raton, FL, USA, 2016; pp. 199–249. [Google Scholar] [CrossRef]
- Dean, J. Lange’s Handbook of Chemistry; McGraw-Hill, Inc.: New York, NY, USA, 1999. [Google Scholar]
- Vysotsky, Y.B.; Fomina, E.S.; Belyaeva, E.A.; Aksenenko, E.V.; Vollhardt, D.; Miller, R. Quantum-chemical analysis of thermodynamics of two-dimensional cluster formation of α-amino acids at the air/water interface. J. Phys. Chem. B 2009, 113, 16557–16567. [Google Scholar] [CrossRef]
- Fomina, E.S.; Vysotsky, Y.B.; Vollhardt, D.; Fainerman, V.B.; Miller, R. Quantum chemical analysis of the thermodynamics of 2D cluster formation of 2-hydroxycarboxylic acids at the air/water interface. Soft Matter 2013, 9, 7601–7616. [Google Scholar] [CrossRef]
- Fomina, E.S.; Vysotsky, Y.B.; Belyaeva, E.A.; Vollhardt, D.; Fainerman, V.B.; Miller, R. On hexagonal orientation of fatty alcohols in monolayers at the air/water interface: Quantum-chemical approach. J. Phys. Chem. C 2014, 118, 4122–4130. [Google Scholar] [CrossRef]
- Vysotsky, Y.B.; Belyaeva, E.A.; Fainerman, V.B.; Aksenenko, E.V.; Vollhardt, D.; Miller, R. Quantum chemical analysis of the thermodynamics of 2-dimensional cluster formation of alkylamines at the air/water interface. J. Phys. Chem. C 2007, 11, 15342–15349. [Google Scholar] [CrossRef]
- Vysotsky, Y.B.; Kartashynska, E.S.; Belyaeva, E.A.; Vollhardt, D.; Fainerman, V.B.; Miller, R. Quantization of the molecular tilt angle of amphiphile monolayers at the air/water interface. J. Phys. Chem. C 2015, 119, 5523–5533. [Google Scholar] [CrossRef]
- Vysotsky, Y.B.; Fomina, E.S.; Belyaeva, E.A.; Fainerman, V.B.; Vollhardt, D.; Miller, R. Quantum chemical analysis of the thermodynamics of 2d cluster formation of aliphatic amides at the air/water interface. J. Phys. Chem. C 2012, 116, 26358–26376. [Google Scholar] [CrossRef]
- Vollhardt, D.; Brezesinski, G. Lattice structures and phase behavior of amphiphilic monoglycerol monolayers. Adv. Colloid Interface Sci. 2019, 273, 102030. [Google Scholar] [CrossRef] [PubMed]
- Vollhardt, D.; Dobner, B.; Brezesinski, G. Influence of linkage type (ether or ester) on the monolayer characteristics of single-chain glycerols at thr air-water interface. PCCP 2020, 22, 23207–23214. [Google Scholar] [CrossRef] [PubMed]
- Vysotsky, Y.B.; Fomina, E.S.; Belyaeva, E.A.; Vollhardt, D.; Fainerman, V.B.; Miller, R. Temperature effect on the monolayer formation of substituted alkanes at the air/water interface: A quantum chemical approach. J. Phys. Chem. B 2012, 116, 8996–9006. [Google Scholar] [CrossRef] [PubMed]


| Type of Infinite 2D Cluster | ||||||
|---|---|---|---|---|---|---|
| VΔH | UΔH | VΔS | UΔS | VΔG | UΔG | |
| 2-monoacyl-sn-glycerol | −10.58 | −10.41 | −279.53 | −19.92 | 72.72 | −4.47 |
| 3-monoacyl-sn-glycerol | −25.50 | −10.41 | −300.38 | −19.92 | 64.01 | −4.47 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kartashynska, E.S. The Effect of Acyl Chain Position on the 2D Monolayer Formation of Monoacyl-sn-Glycerol at the Air/Water Interface: Quantum Chemical Modeling. Condens. Matter 2023, 8, 58. https://doi.org/10.3390/condmat8030058
Kartashynska ES. The Effect of Acyl Chain Position on the 2D Monolayer Formation of Monoacyl-sn-Glycerol at the Air/Water Interface: Quantum Chemical Modeling. Condensed Matter. 2023; 8(3):58. https://doi.org/10.3390/condmat8030058
Chicago/Turabian StyleKartashynska, Elena S. 2023. "The Effect of Acyl Chain Position on the 2D Monolayer Formation of Monoacyl-sn-Glycerol at the Air/Water Interface: Quantum Chemical Modeling" Condensed Matter 8, no. 3: 58. https://doi.org/10.3390/condmat8030058
APA StyleKartashynska, E. S. (2023). The Effect of Acyl Chain Position on the 2D Monolayer Formation of Monoacyl-sn-Glycerol at the Air/Water Interface: Quantum Chemical Modeling. Condensed Matter, 8(3), 58. https://doi.org/10.3390/condmat8030058






