New Scaling Laws for Pinning Force Density in Superconductors
Abstract
1. Introduction
- The electric field, E, along the sample;
- The applied magnetic field, Bappl;
- The sample temperature, T;
- The transport current density, J.
2. Problem Description
- Low reduced applied magnetic field range, where can be approximated by the projection;
- High reduced applied magnetic field range, where can be approximated by the projection;
- Middle reduced applied magnetic field, where and branches are overlapped and an approximation can be achieved by constructing some new function, where both branches are presented with some weights.
3. Results
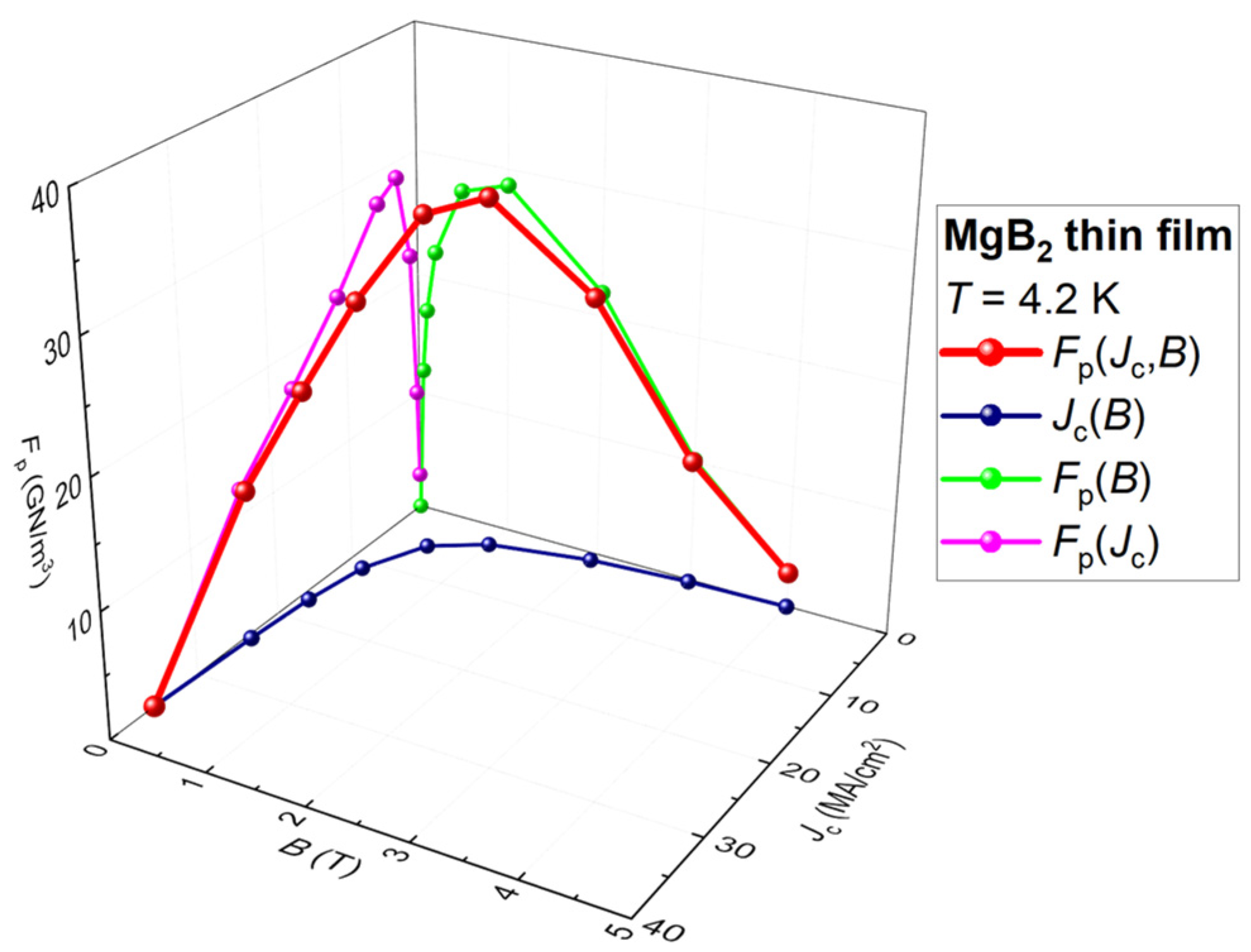
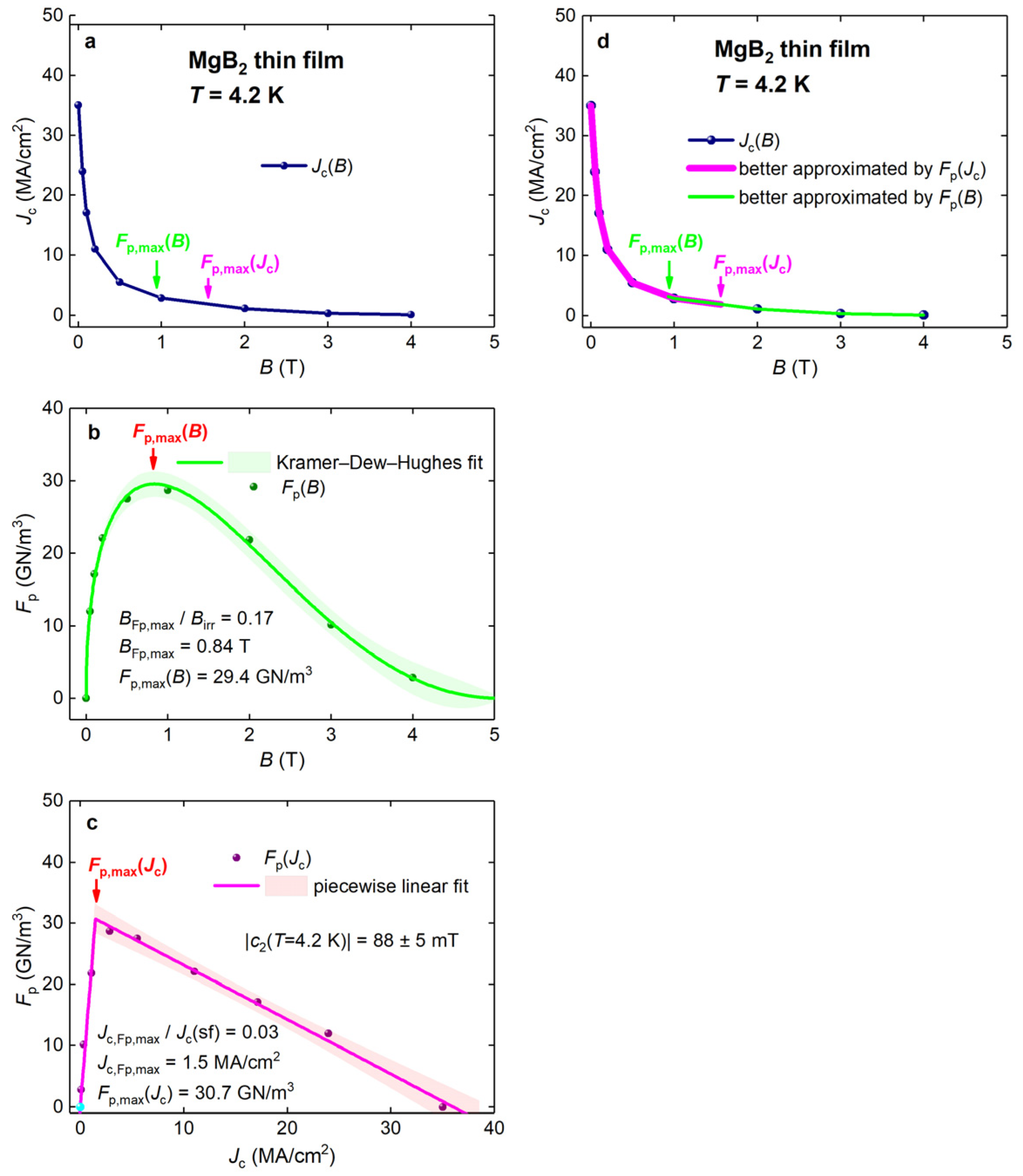
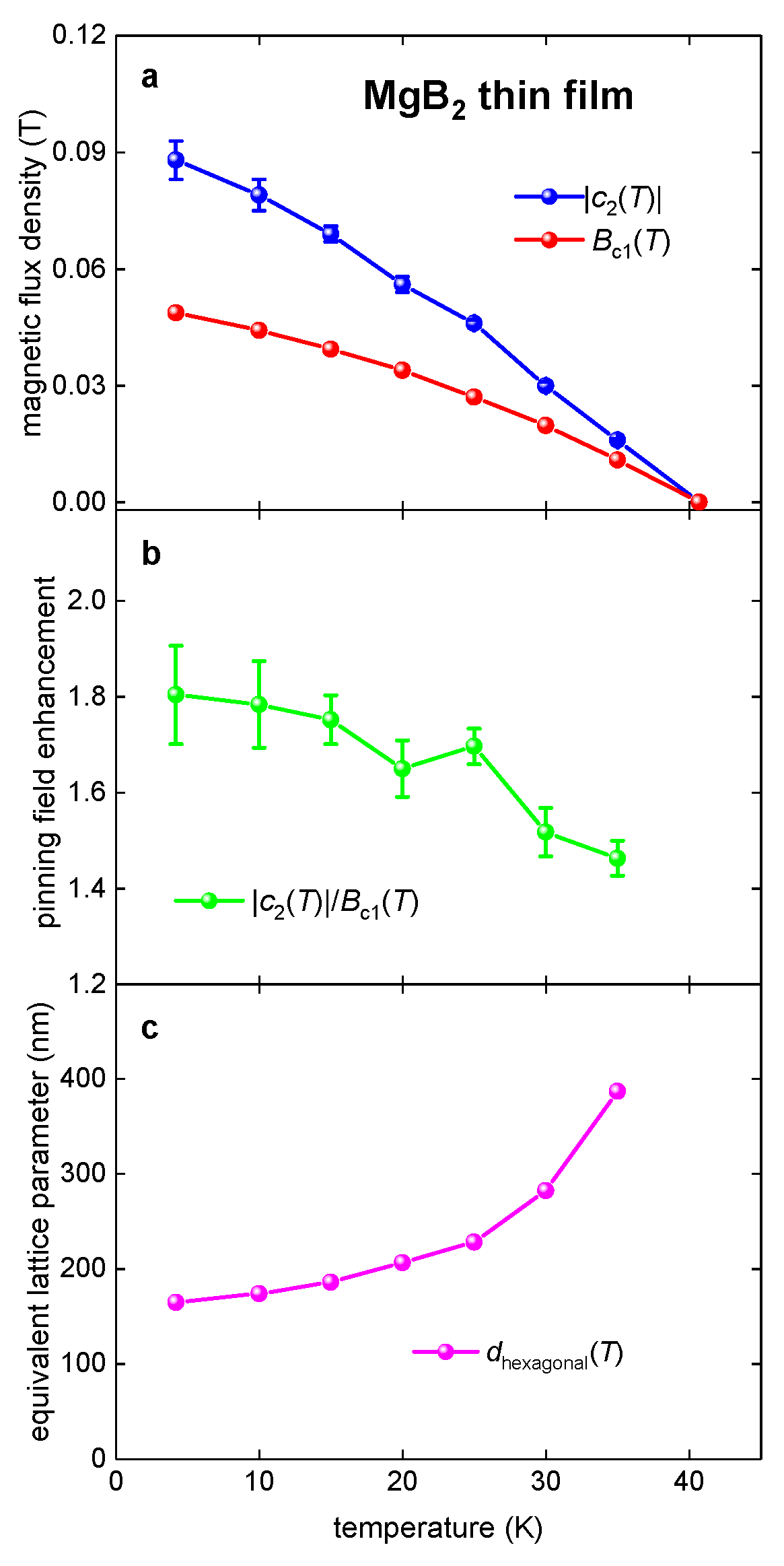
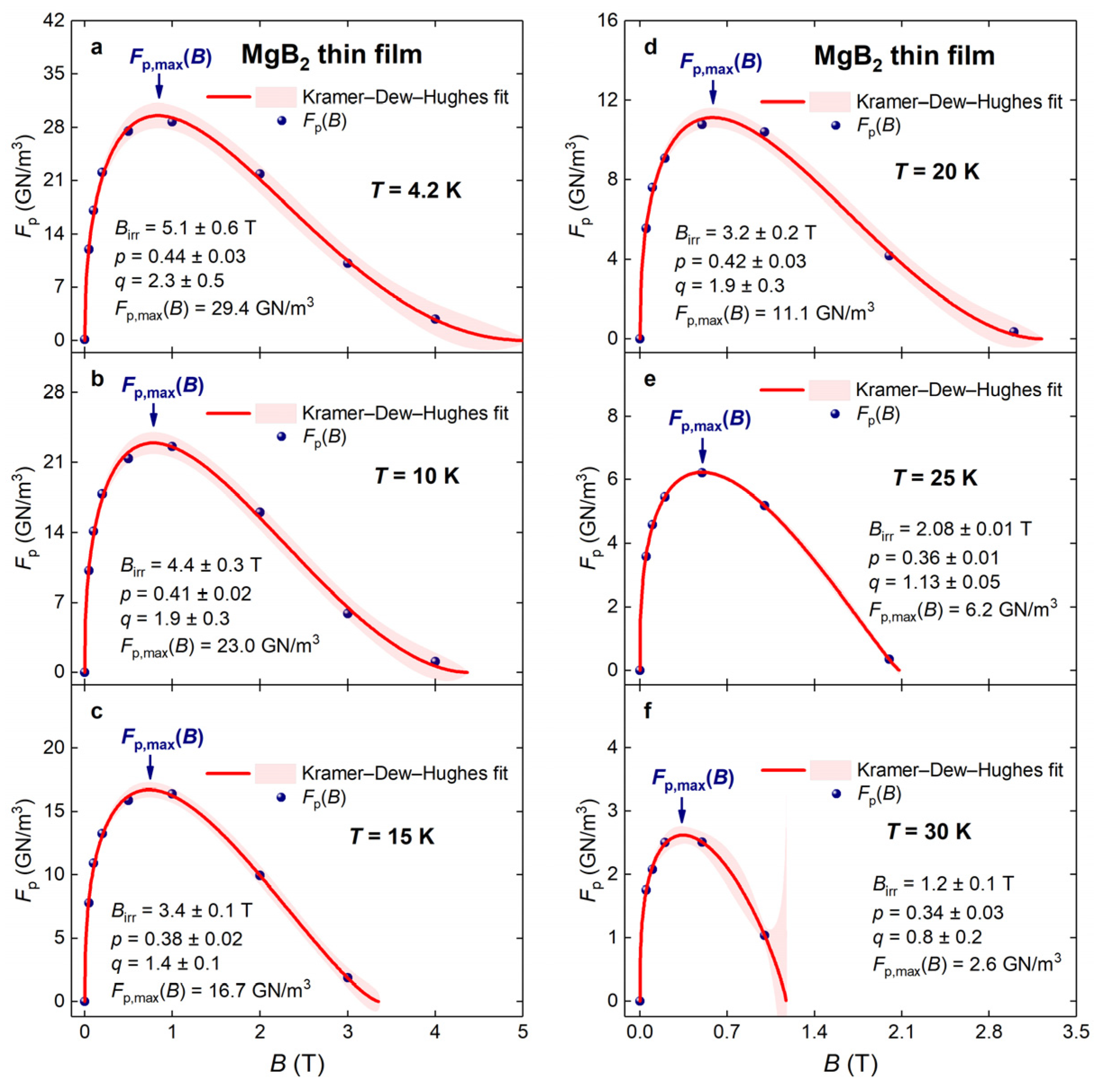
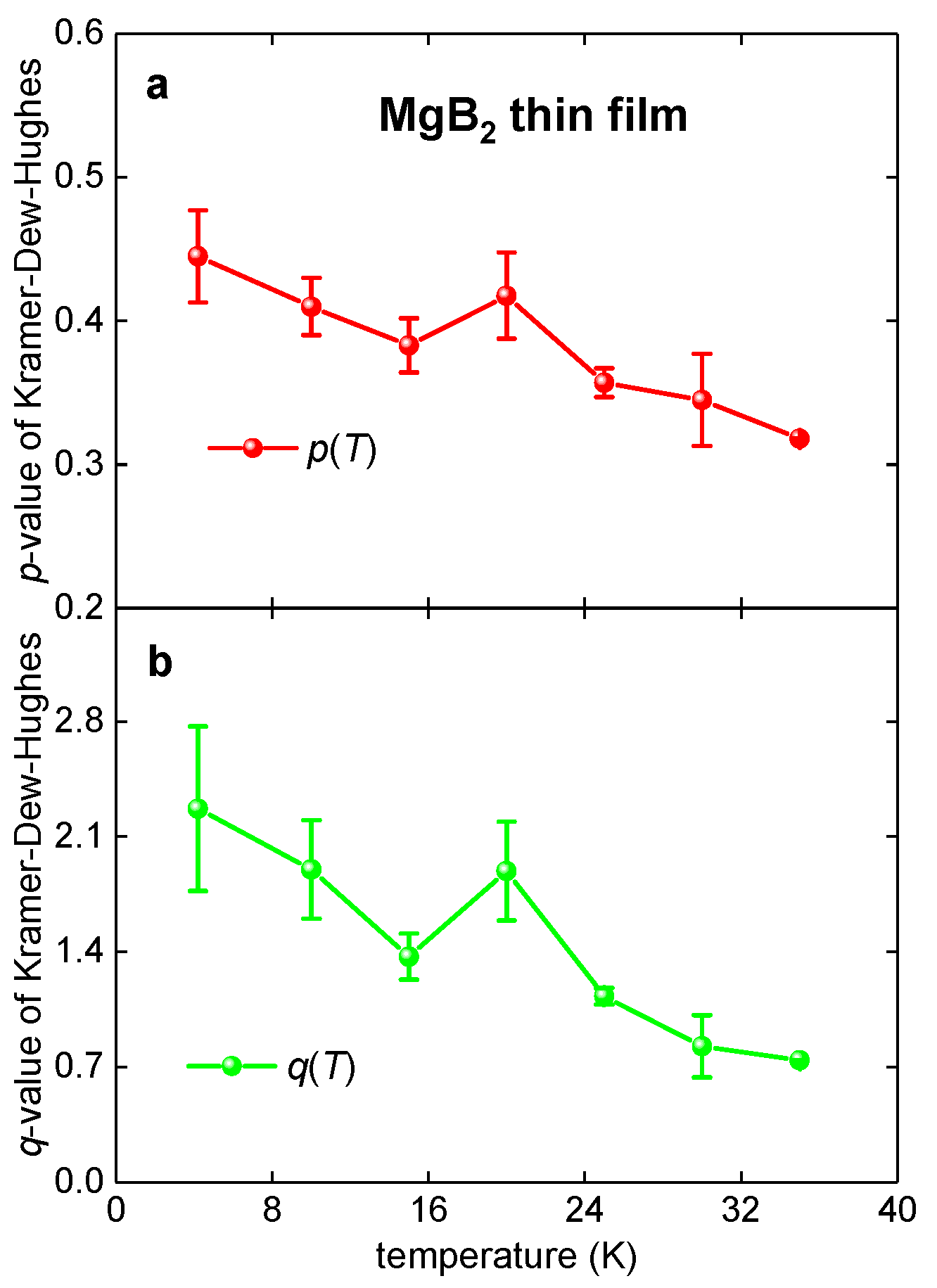
3.1. MgB2
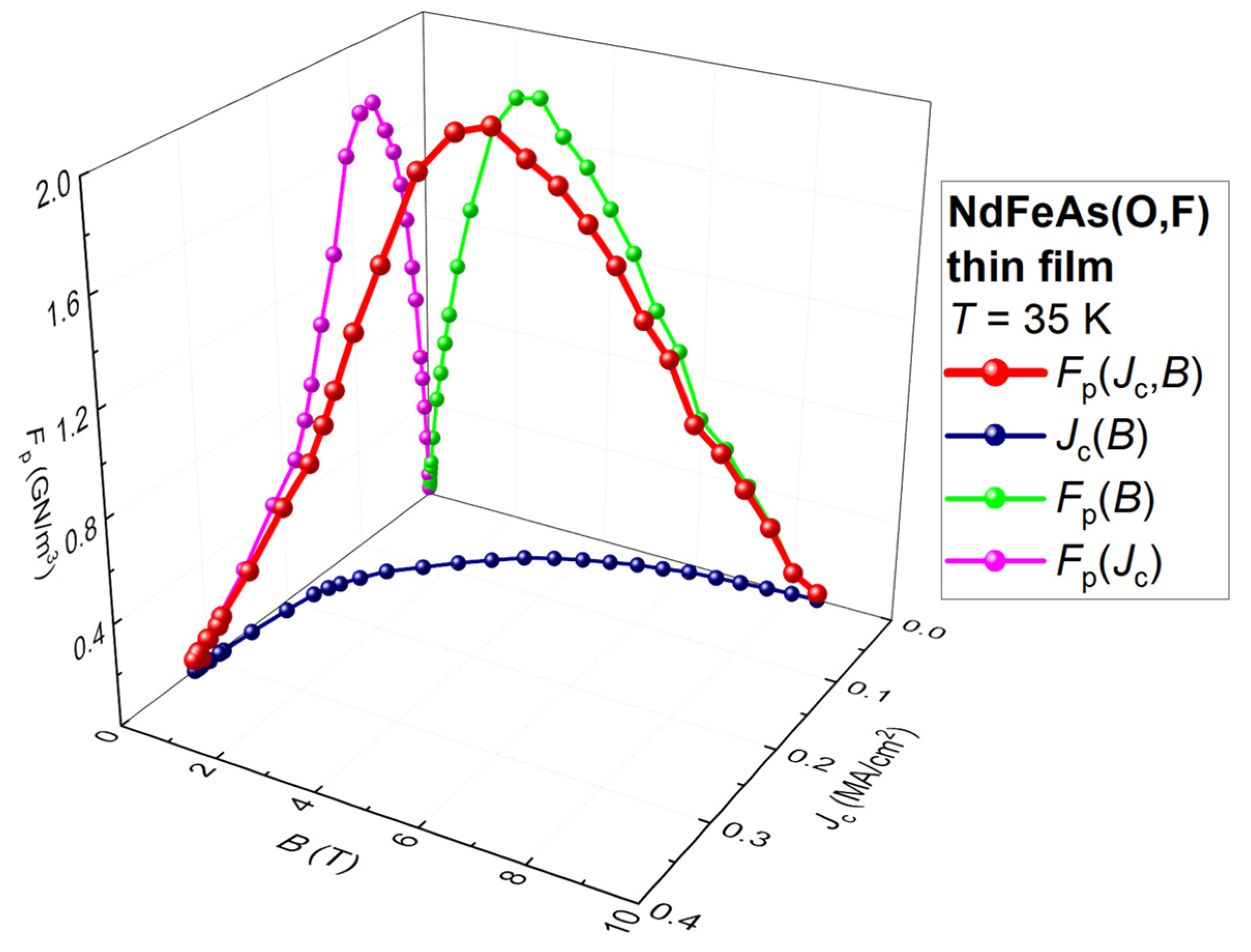
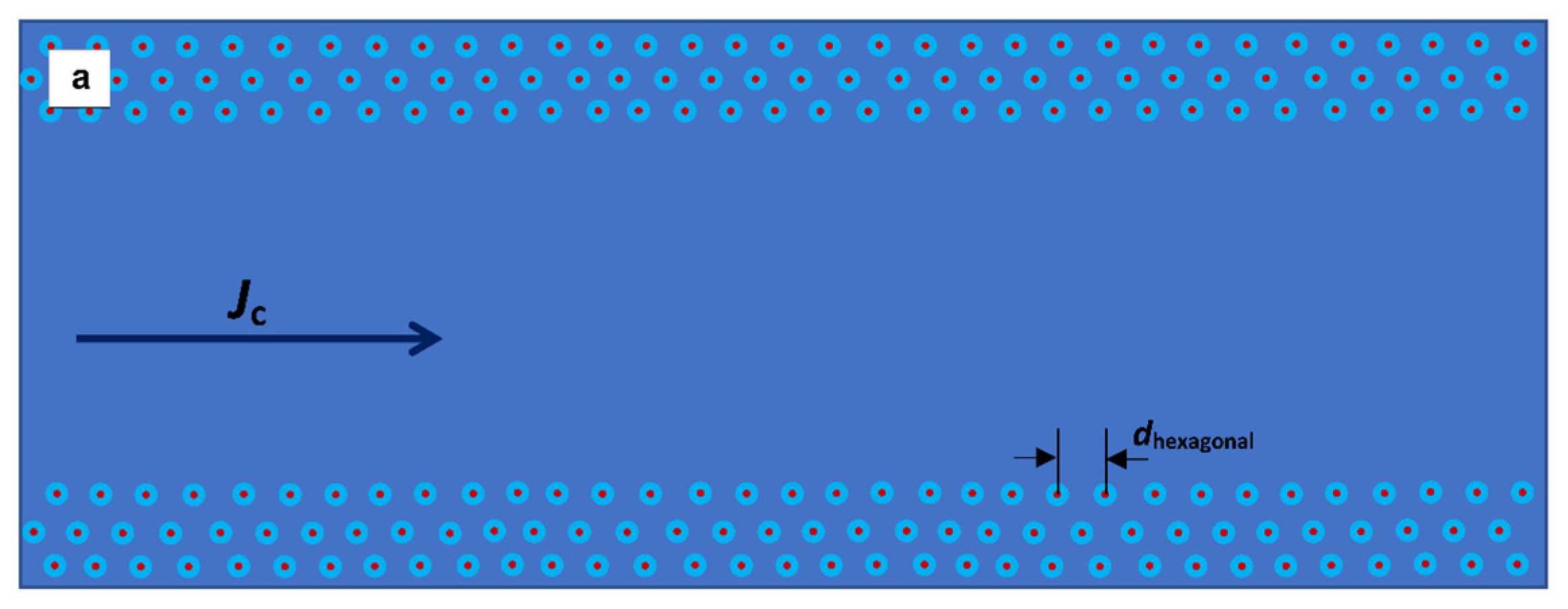
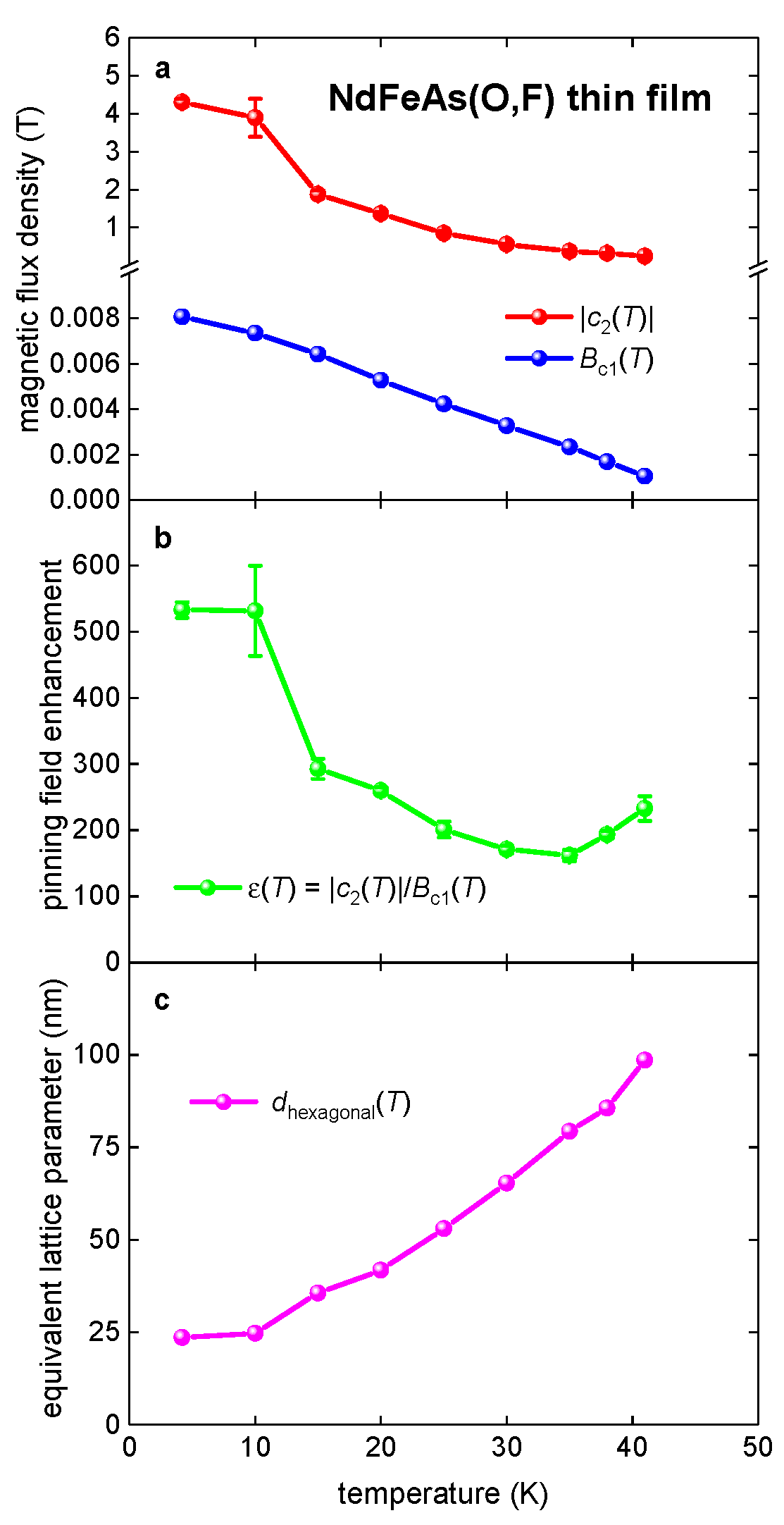
3.2. Pnictide Thin Films
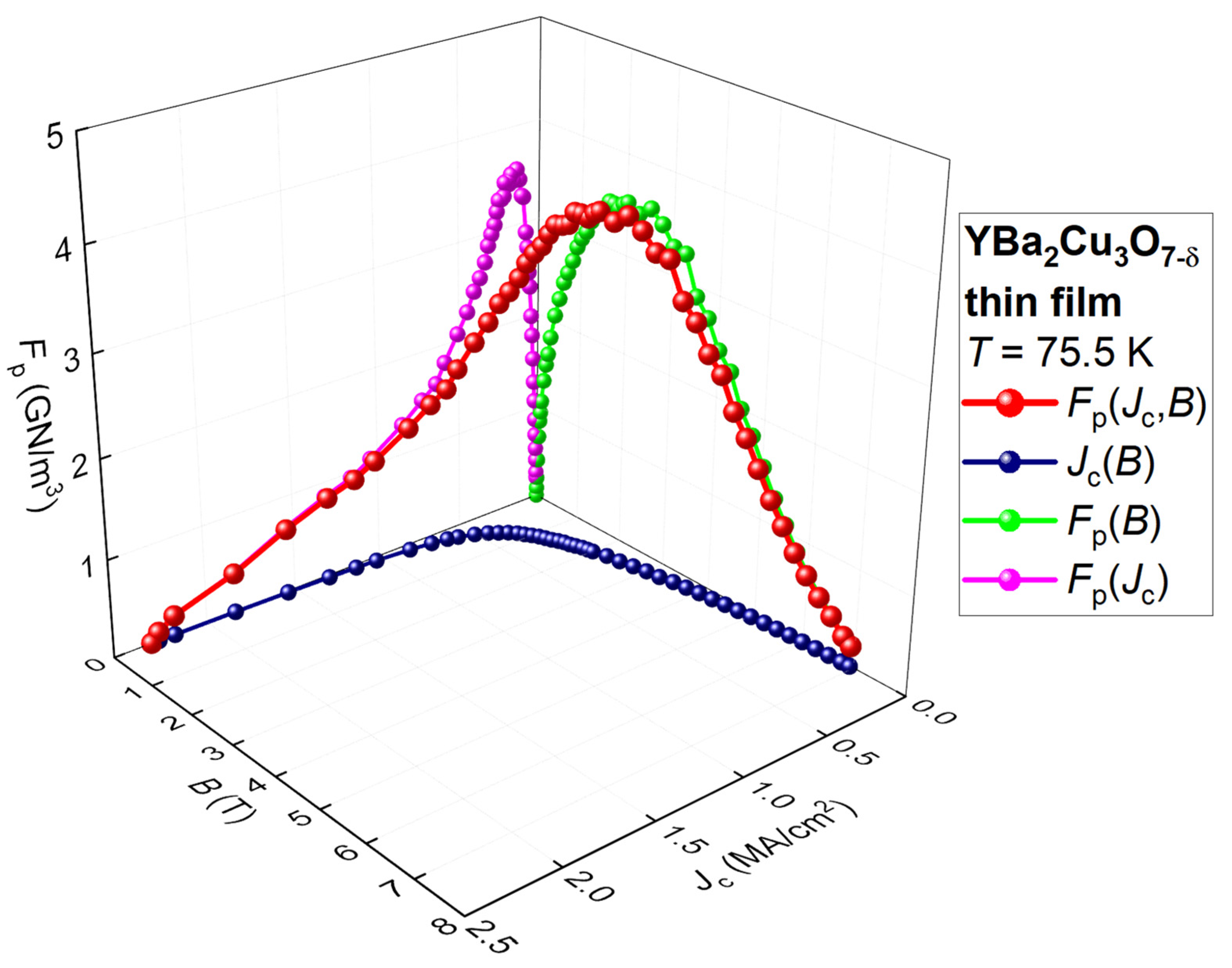
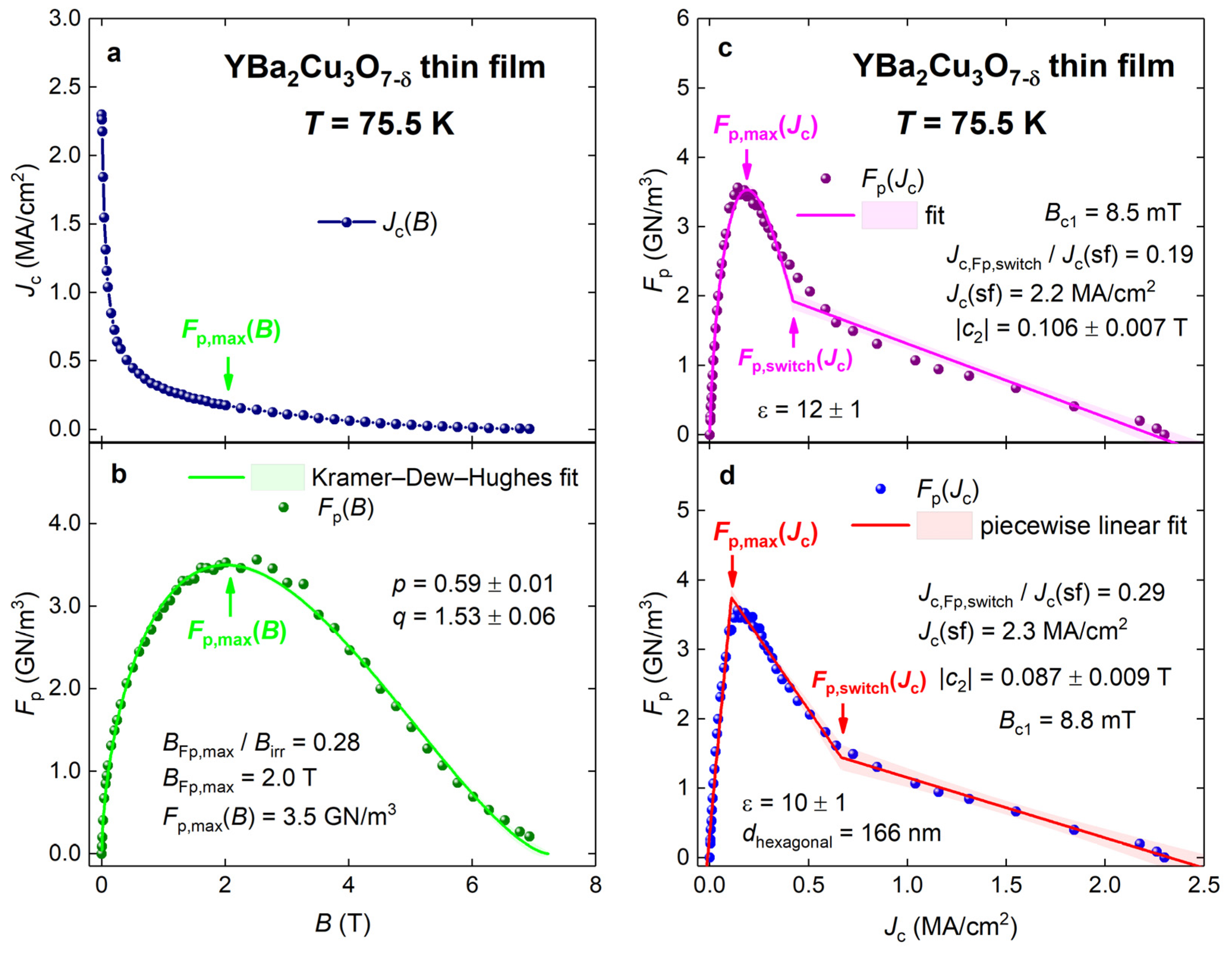
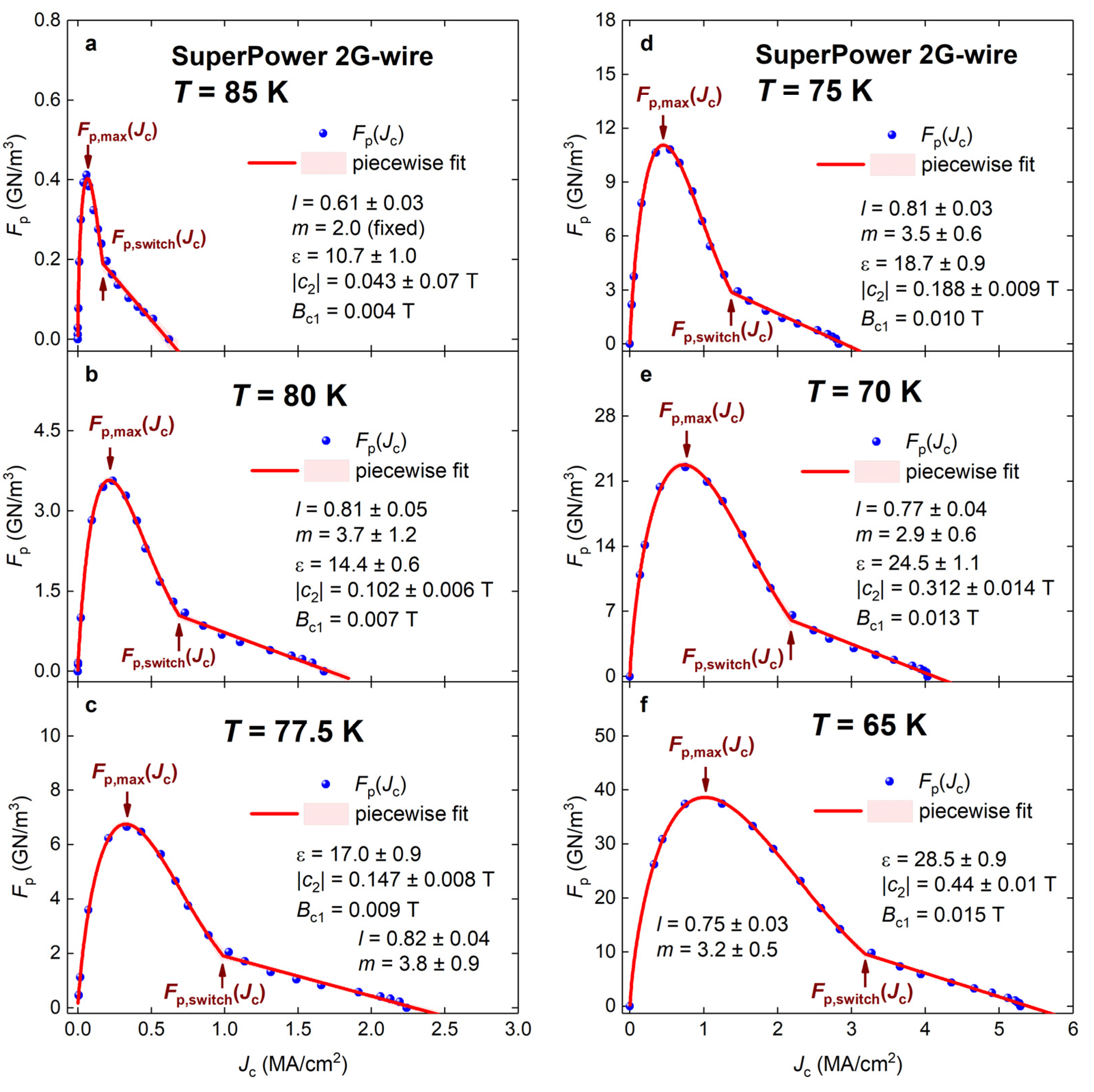
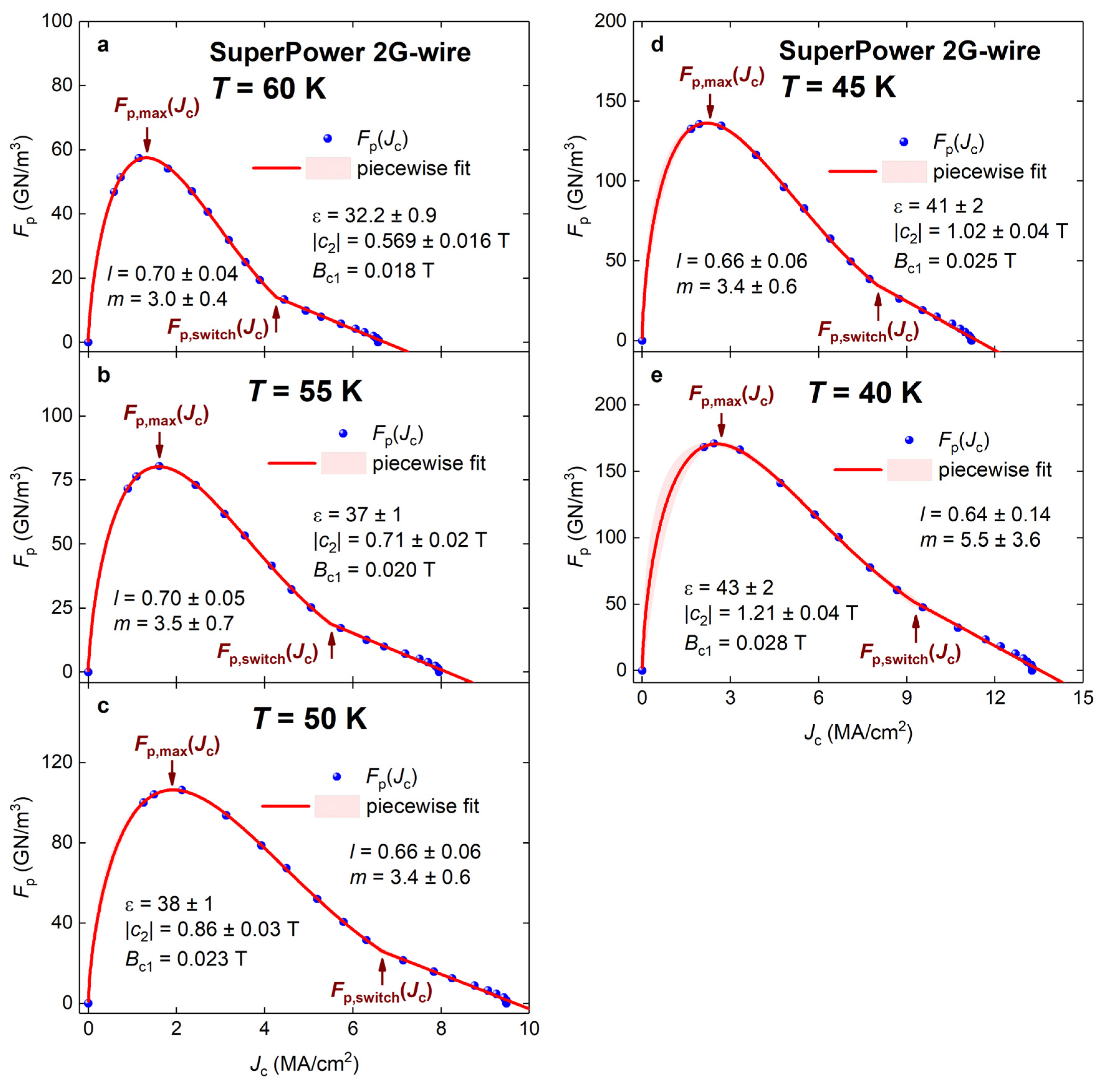
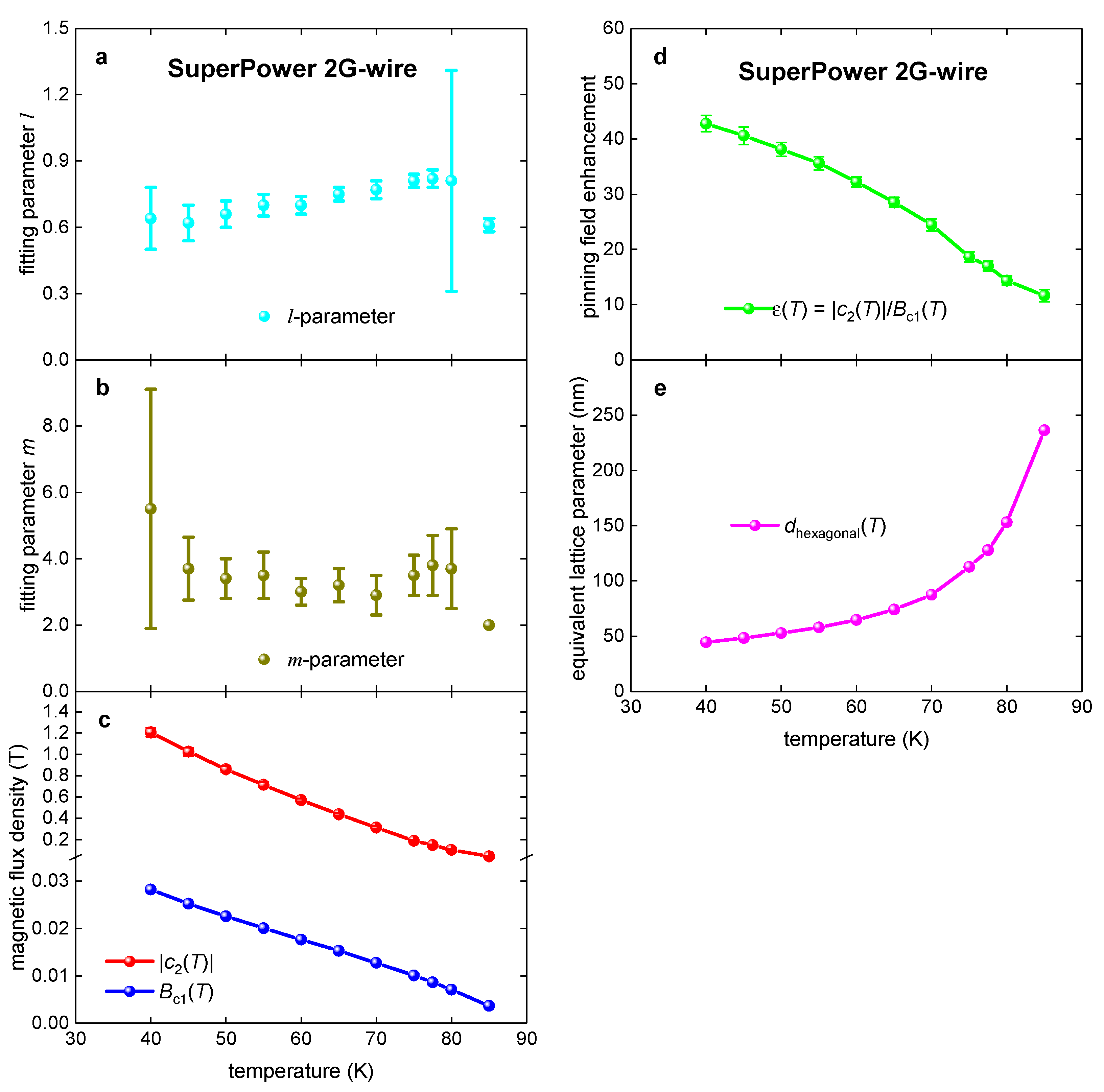
3.3. REBCO Thin Films
- Low reduced applied magnetic field range, where the approximation is the line (which corresponds to the schematic diagram shown in Figure 9a);
- High reduced applied magnetic field range, where the approximation is the curve;
- Middle reduced applied magnetic field, where the line and curve can be approximated by a median curve or line.
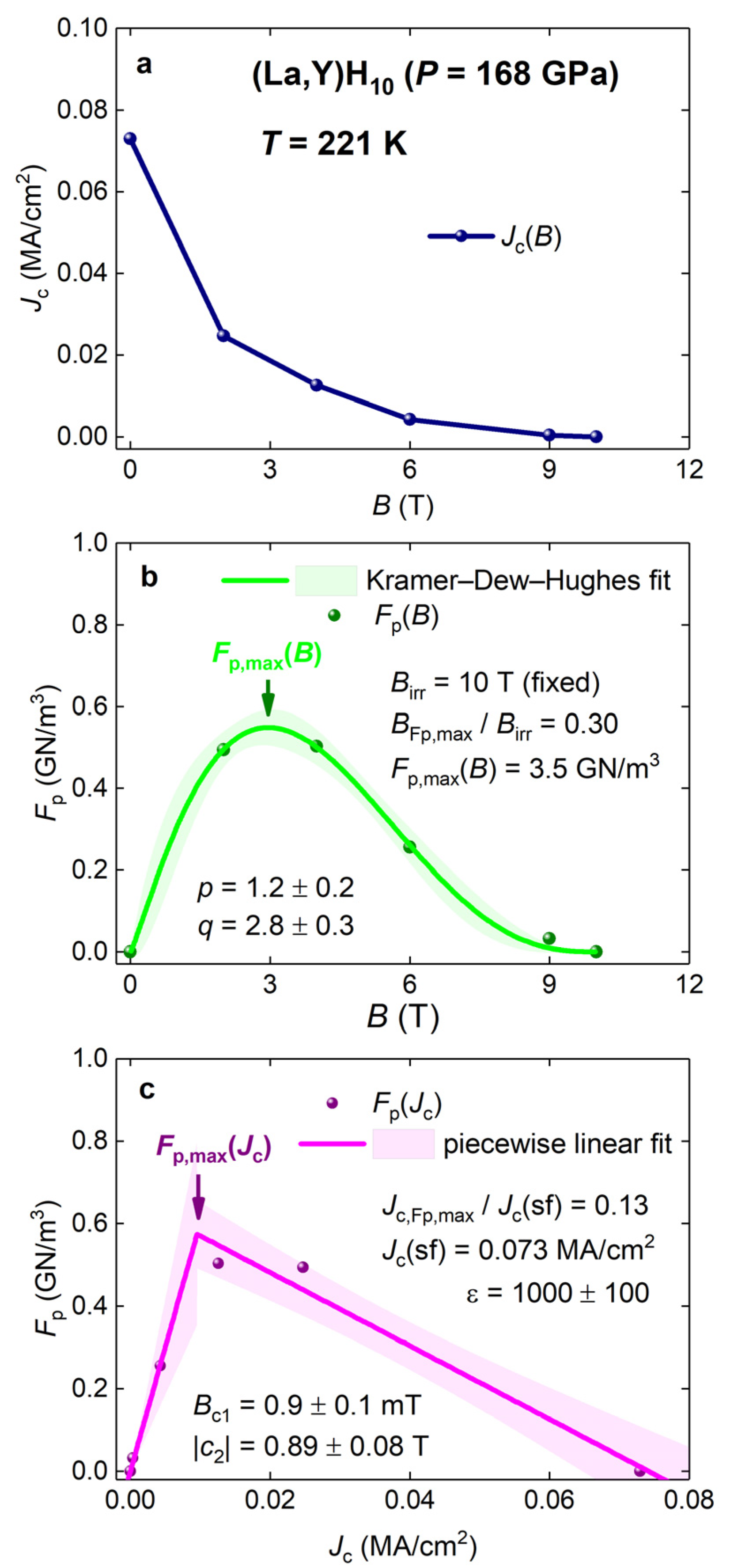
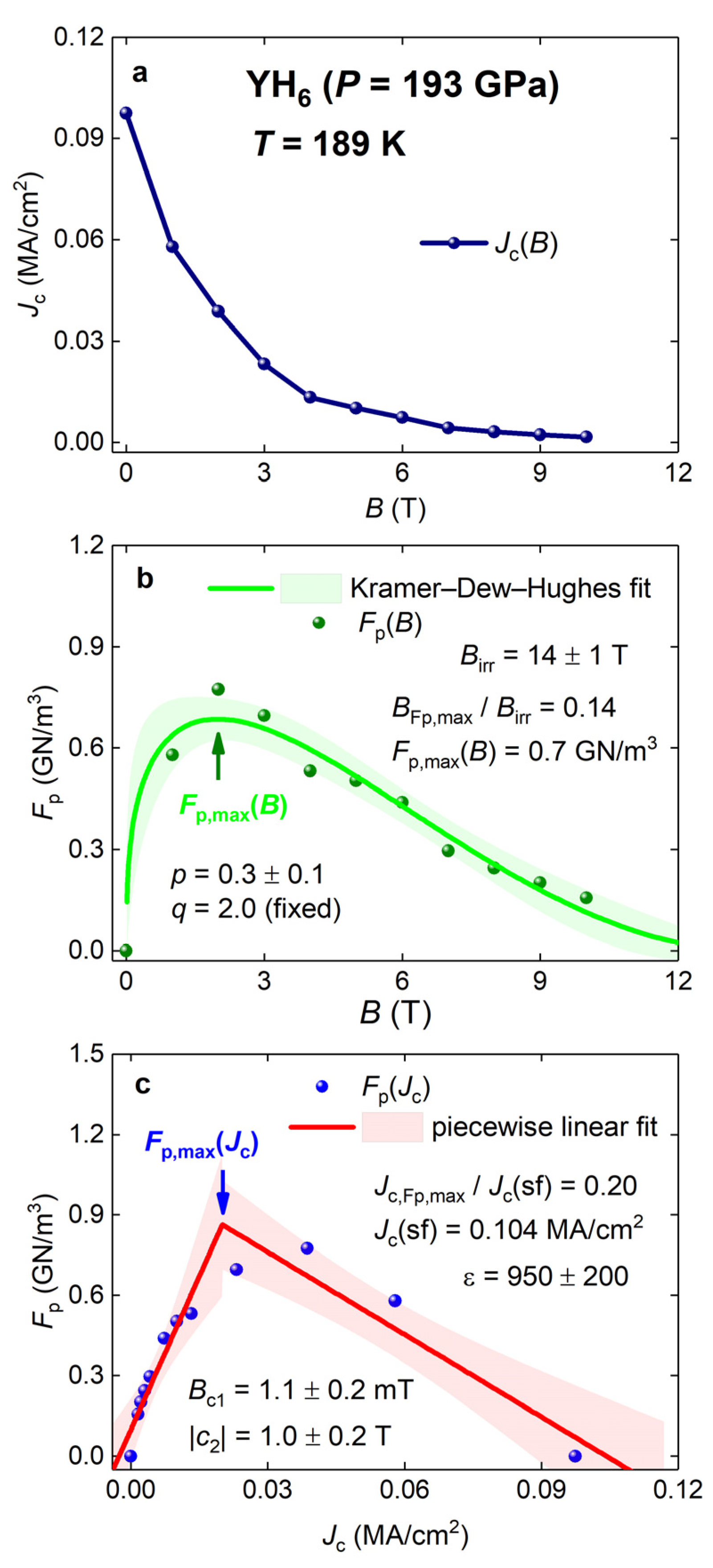
3.4. Near-Room-Temperature Superconductors (La,Y)H10 and YH6
4. Discussion
5. Conclusions
- MgB2 thin films;
- NdFeAs(O,F) thin film;
- REBCO 2G-wire;
- Near-room-temperature superconductors (La,Y)H10 and YH6 thick films.
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Anderson, P.W. Theory of flux creep in hard superconductors. Phys. Rev. Lett. 1962, 9, 309–311. [Google Scholar] [CrossRef]
- Anderson, P.W.; Kim, Y.B. Hard superconductivity: Theory of the motion of Abrikosov flux lines. Rev. Mod. Phys. 1964, 36, 39–43. [Google Scholar] [CrossRef]
- Fietz, W.A.; Webb, W.W. Hysteresis in superconducting alloys—Temperature and field dependence of dislocation pinning in niobium alloys. Phys. Rev. 1969, 178, 657–667. [Google Scholar] [CrossRef]
- Kramer, E.J. Scaling laws for flux pinning in hard superconductors. J. Appl. Phys. 1973, 44, 1360–1370. [Google Scholar] [CrossRef]
- Dew-Hughes, D. Flux pinning mechanisms in type II superconductors. Phil. Mag. 1974, 30, 293–305. [Google Scholar] [CrossRef]
- Jirsa, M.; Koblischka, M.R.; Higuchi, T.; Muralidhar, M.; Murakami, M. Comparison of different approaches to modelling the fishtail shape in RE-123 bulk superconductors. Physica C 2000, 338, 235–245. [Google Scholar] [CrossRef]
- Godeke, A.; ten Haken, B.; ten Kate, H.H.J.; Larbalestier, D.C. A general scaling relation for the critical current density in Nb3Sn. Supercond. Sci. Technol. 2006, 19, R100–R116. [Google Scholar] [CrossRef]
- Ekin, J.W. Unified scaling law for flux pinning in practical superconductors: I. Separability postulate, raw scaling data and parameterization at moderate strains. Supercond. Sci. Technol. 2010, 23, 083001. [Google Scholar] [CrossRef]
- Ekin, J.W.; Cheggour, N.; Goodrich, L.; Splett, J.; Bordini, B.; Richter, D. Unified Scaling Law for flux pinning in practical superconductors: II. Parameter testing, scaling constants, and the Extrapolative Scaling Expression. Supercond. Sci. Technol. 2016, 29, 123002. [Google Scholar] [CrossRef]
- Ekin, J.; Cheggour, N.; Goodrich, L.; Splett, J. Unified scaling law for flux pinning in practical superconductors: III. Minimum datasets, core parameters, and application of the Extrapolative Scaling Expression. Supercond. Sci. Technol. 2017, 30, 033005. [Google Scholar] [CrossRef]
- Tarantini, C.; Kametani, F.; Balachandran, S.; Heald, S.M.; Wheatley, L.; Grovenor, C.R.M.; Moody, M.P.; Su, Y.-F.; Lee, P.J.; Larbalestier, D.C. Origin of the enhanced Nb3Sn performance by combined Hf and Ta doping. Sci. Rep. 2021, 11, 17845. [Google Scholar] [CrossRef] [PubMed]
- Jirsa, M.; Pust, L. A comparative study of irreversible magnetisation and pinning force density in (RE)Ba2Cu3O7 and some other high-Tc compounds in view of a novel scaling scheme. Phys. C 1997, 291, 17–24. [Google Scholar] [CrossRef]
- Fietz, W.A.; Beasley, M.R.; Silcox, J.; Webb, W.W. Magnetization of superconducting Nb-25%Zr wire. Phys. Rev. 1964, 136, A335–A345. [Google Scholar] [CrossRef]
- Senatore, C.; Barth, C.; Bonura, M.; Kulich, M.; Mondonico., G. Field and temperature scaling of the critical current density in commercial REBCO coated conductors. Supercond. Sci. Technol. 2016, 29, 014002. [Google Scholar] [CrossRef]
- Francis, A.; Abraimov, D.; Viouchkov, Y.; Su, Y.; Kametani, F.; Larbalestier, D.C. Development of general expressions for the temperature and magnetic field dependence of the critical current density in coated conductors with variable properties. Supercond. Sci. Technol. 2020, 33, 044011. [Google Scholar] [CrossRef]
- Eisterer, M. Calculation of the volume pinning force in MgB2 superconductors. Phys. Rev. B 2008, 77, 144524. [Google Scholar] [CrossRef]
- Paturi, P.; Malmivirta, M.; Palonen, H.; Huhtinen, H. Dopant diameter dependence of Jc(B) in doped YBCO films. IEEE Trans. Appl. Supercond. 2016, 26, 8000705. [Google Scholar] [CrossRef]
- Paturi, P.; Irjala, M.; Abrahamsen, A.B.; Huhtinen, H. Defining Bc, B* and Bϕ for YBCO thin films. IEEE Trans. Appl. Supercond. 2009, 19, 3431–3434. [Google Scholar] [CrossRef]
- Iida, K.; Sato, H.; Tarantini, C.; Hänisch, J.; Jaroszynski, J.; Hiramatsu, H.; Holzapfel, B.; Hosono, H. High-field transport properties of a P-doped BaFe2As2 film on technical substrate. Sci. Rep. 2017, 7, 39951. [Google Scholar] [CrossRef]
- Koblischka, M.R.; Higuchi, T.; Yoo, S.I.; Murakami, M. Scaling of pinning forces in NdBa2Cu3O7-δ superconductors. J. Appl. Phys. 1999, 85, 3241–3246. [Google Scholar] [CrossRef]
- Branch, P.; Tsui, Y.; Osamura, K.; Hampshire, D.P. Weakly-emergent strain dependent properties of high field superconductors. Sci. Rep. 2019, 9, 13998. [Google Scholar] [CrossRef] [PubMed]
- Talantsev, E.F.; Strickland, N.M.; Wimbush, S.C.; Storey, J.G.; Tallon, J.L.; Long, N.J. Hole doping dependence of critical current density in YBa2Cu3O7-δ conductors. Appl. Phys. Lett. 2014, 104, 242601. [Google Scholar] [CrossRef]
- Zeng, X.H. Superconducting MgB2 thin films on silicon carbide substrates by hybrid physical–chemical vapor deposition. Appl. Phys. Lett. 2003, 82, 2097–2099. [Google Scholar] [CrossRef]
- Koblischka, M.R.; Wiederhold, A.; Koblischka-Veneva, A.; Chang, C. Pinning force scaling analysis of polycrystalline MgB2. J. Supercond. Nov. Magn. 2020, 33, 3333–3339. [Google Scholar] [CrossRef]
- Iida, K.; Hänisch, J.; Kondo, K.; Chen, M.; Hatano, T.; Wang, C.; Saito, H.; Hata, S.; Ikuta, H. High Jc and low anisotropy of hydrogen doped NdFeAsO superconducting thin. Sci. Rep. 2021, 11, 5636. [Google Scholar] [CrossRef]
- Arvapalli, S.S.; Miryala, M.; Sunsanee, P.; Jirsa, M.; Murakami, M. Superconducting properties of sintered bulk MgB2 prepared from hexane-mediated high-energy-ultra-sonicated boron. Mater. Sci. Eng. B 2021, 265, 115030. [Google Scholar] [CrossRef]
- Kim, Y.B.; Hempstead, C.F.; Strand, A.R. Critical persistent currents in hard superconductors. Phys. Rev. Lett. 1962, 9, 306–309. [Google Scholar] [CrossRef]
- Kim, Y.B.; Hempstead, C.F.; Strand, A.R. Magnetization and critical supercurrents. Phys. Rev. 1963, 129, 528–535. [Google Scholar] [CrossRef]
- Lyard, L.; Klein, T.; Marcus, J.; Brusetti, R.; Marcenat, C.; Konczykowski, M.; Mosser, V.; Kim, K.H.; Kang, B.W.; Lee, H.S.; et al. Geometrical barriers and lower critical field in MgB2 single crystals. Phys. Rev. B 2004, 70, 180504. [Google Scholar] [CrossRef]
- Niedermayer, C.; Bernhard, C.; Holden, T.; Kremer, R.K.; Ahn, K. Muon spin relaxation study of the magnetic penetration depth in MgB2. Phys. Rev. B 2002, 65, 094512. [Google Scholar] [CrossRef]
- Kogan, V.G.; Martin, C.; Prozorov, R. Superfluid density and specific heat within a self-consistent scheme for a two-band superconductor. Phys. Rev. B 2009, 80, 014507. [Google Scholar] [CrossRef]
- Kim, H.; Cho, K.; Tanatar, M.A.; Taufour, V.; Kim, S.K.; Bud’Ko, S.L.; Canfield, P.C.; Kogan, V.G.; Prozorov, R. Self-consistent two-gap description of MgB2 superconductor. Symmetry 2019, 11, 1012. [Google Scholar] [CrossRef]
- Talantsev, E.F. Critical de Broglie wavelength in superconductors. Mod. Phys. Lett. B 2018, 32, 1850114. [Google Scholar] [CrossRef]
- Talantsev, E.F.; Tallon, J.L. Universal self-field critical current for thin-film superconductors. Nat. Comms. 2015, 6, 7820. [Google Scholar] [CrossRef]
- Finnemore, D.K.; Ostenson, J.E.; Bud’ko, S.L.; Lapertot, G.; Canfield, P.C. Thermodynamic and transport properties of superconducting Mg10B2. Phys. Rev. Lett. 2001, 86, 2420–2422. [Google Scholar] [CrossRef]
- Civale, L.; Marwick, A.D.; Worthington, T.K.; Kirk, M.A.; Thompson, J.R.; Krusin-Elbaum, L.; Sun, Y.; Clem, J.R.; Holtzberg, F. Vortex confinement by columnar defects in YBa2Cu3O7 crystals: Enhanced pinning at high fields and temperatures . Phys. Rev. Lett. 1991, 67, 648–651. [Google Scholar] [CrossRef]
- Chen, Z.; Kametani, F.; Gurevich, A.; Larbalestier, D. Pinning, thermally activated depinning and their importance for tuning the nanoprecipitate size and density in high Jc YBa2Cu3O7-x films. Phys. C Supercond. 2009, 469, 2021–2028. [Google Scholar] [CrossRef]
- Strickland, N.M.; Wimbush, S.C.; Kennedy, J.V.; Ridgway, M.C.; Talantsev, E.F.; Long, N.J. Effective low-temperature flux pinning by Au ion irradiation in HTS coated conductors. IEEE Trans. Appl. Supercond. 2015, 25, 6939646. [Google Scholar] [CrossRef]
- Poole, P.P.; Farach, H.A.; Creswick, R.J.; Prozorov, R. Superconductivity; Academic Press: London, UK, 2007; Chapter 12. [Google Scholar]
- Zhang, C.; Wang, D.; Liu, Z.-H.; Zhang, Y.; Ma, P.; Feng, Q.-R.; Wang, Y.; Gan, Z.-Z. Fabrication of superconducting nanowires from ultrathin MgB2 films via focused ion beam milling. AIP Adv. 2015, 5, 027139. [Google Scholar] [CrossRef]
- Yang, C.; Ni, Z.M.; Guo, X.; Hu, H.; Wang, Y.; Zhang, Y.; Feng, Q.R.; Gan, Z.Z. Intrinsic flux pinning mechanisms in different thickness MgB2 films. AIP Adv. 2017, 7, 035117. [Google Scholar] [CrossRef]
- Koblischka, M.R.; Wiederhold, A.; Koblischka-Veneva, A.; Chang, C.; Berger, K.; Nouailhetas, Q.; Douine, B.; Murakami, M. On the origin of the sharp, low-field pinning force peaks in MgB2 superconductors. AIP Adv. 2020, 10, 015035. [Google Scholar] [CrossRef]
- Tarantini, C.; Iida, K.; Hänisch, J.; Kurth, F.; Jaroszynski, J.; Sumiya, N.; Chihara, M.; Hatano, T.; Ikuta, H.; Schmidt, S.; et al. Intrinsic and extrinsic pinning in NdFeAs(O,F): Vortex trapping and lock-in by the layered structure. Sci. Rep. 2016, 6, 36047. [Google Scholar] [CrossRef] [PubMed]
- Guo, Z.; Guo, Z.; Gao, H.; Kondo, K.; Hatano, T.; Iida, K.; Hänisch, J.; Ikuta, H.; Hata, S. Nanoscale texture and microstructure in a NdFeAs(O,F)/IBAD-MgO superconducting thin film with superior critical current properties. ACS Appl. Electron. Mater. 2021, 3, 3158–3166. [Google Scholar] [CrossRef]
- Larkin, A.I.; Ovchinnikov Yu, N. Pinning in type II superconductors. J. Low Temp. Phys. 1979, 34, 409–428. [Google Scholar] [CrossRef]
- Xue, Y.Y.; Huang, Z.J.; Hor, P.H.; Chu, C.W. Evidence for collective pinning in high-temperature superconductors. Phys. Rev. B 1991, 43, 13598–13601. [Google Scholar] [CrossRef]
- Brandt, E.H.; Indenbom, M. Type-II-superconductor strip with current in a perpendicular magnetic field. Phys. Rev. B 1993, 48, 12893. [Google Scholar] [CrossRef]
- Talantsev, E.F.; Iida, K.; Ohmura, T.; Matsumoto, T.; Crump, W.P.; Strickland, N.M.; Wimbush, S.C.; Ikuta, H. P-wave superconductivity in iron-based superconductors. Sci. Rep. 2019, 9, 14245. [Google Scholar] [CrossRef]
- MacMagnus-Driscoll, J.L.; Foltyn, S.R.; Jia, Q.; Wang, H.; Serquis, A.; Civale, L.; Maiorov, B.; Hawley, M.E.; Maley, M.P.; Peterson, D.E. Strongly enhanced current densities in superconducting coated conductors of YBa2Cu3O7–x + BaZrO3. Nat. Mater. 2004, 3, 439–443. [Google Scholar] [CrossRef]
- Haugan, T.; Barnes, P.N.; Wheeler, R.; Meisenkothen, F.; Sumption, M. Addition of nanoparticle dispersions to enhance flux pinning of the YBa2Cu3O7-x superconductor. Nature 2004, 430, 867–870. [Google Scholar] [CrossRef]
- Tsuchiya, K.; Wang, X.; Fujita, S.; Ichinose, A.; Yamada, K.; Terashima, A.; Kikuchi, A. Superconducting properties of commercial REBCO-coated conductors with artificial pinning centers. Supercond. Sci. Technol. 2021, 34, 105005. [Google Scholar] [CrossRef]
- Mitchell, N.; Zheng, J.; Vorpahl, C.; Corato, V.; Sanabria, C.; Segal, M.; Sorbom, B.N.; Slade, R.A.; Brittles, G.; Bateman, R.; et al. Superconductors for fusion: A roadmap. Supercond. Sci. Technol. 2021, 34, 103001. [Google Scholar] [CrossRef]
- Poole, P.P.; Zasadzinski, J.F.; Zasadzinski, R.K.; Allen, P.B. Characteristic parameters. In Handbook on Superconductivity, 1st ed.; Poole, P.P., Farach, H.A., Creswick, R.J., Eds.; Academic Press: London, UK, 2000. [Google Scholar]
- Xu, A.; Delgado, L.; Khatri, N.; Liu, Y.; Selvamanickam, V.; Abraimov, D.; Jaroszynski, J.; Kametani, F.; Larbalestier, D.C. Strongly enhanced vortex pinning from 4 to 77 K in magnetic fields up to 31 T in 15 mol.% Zr-added (Gd,Y)-Ba-Cu-O superconducting tapes. APL Mater. 2014, 2, 046111. [Google Scholar] [CrossRef]
- Yeshurun, Y.; Malozemoff, A.P.; Holtzberg, F.; Dinger, T.R. Magnetic relaxation and the lower critical fields in a Y-Ba-Cu-O crystal. Phys. Rev. B 1988, 38, 11828–11831. [Google Scholar] [CrossRef] [PubMed]
- Krusin-Elbaum, L.; Malozemoff, A.P.; Yeshurun, Y.; Cronemeyer, D.C.; Holtzberg, F. Temperature dependence of lower critical fields in Y-Ba-Cu-O crystals. Phys. Rev. B 1989, 39, 2936. [Google Scholar] [CrossRef] [PubMed]
- Wimbush, S.; Strickland, N. A public database of high-temperature superconductor critical current data. IEEE Trans. Appl. Supercond. 2017, 27, 16516413. [Google Scholar] [CrossRef]
- Drozdov, A.P.; Eremets, M.I.; Troyan, I.A.; Ksenofontov, V.; Shylin, S.I. Conventional superconductivity at 203 kelvin at high pressures in the sulfur hydride system. Nature 2015, 525, 73–76. [Google Scholar] [CrossRef]
- Boeri, L.; Hennig, R.; Hirschfeld, P.; Profeta, G.; Sanna, A.; Zurek, E.; E Pickett, W.; Amsler, M.; Dias, R.; I Eremets, M.; et al. The 2021 room-temperature superconductivity roadmap. J. Phys. Condens. Matter 2022, 34, 183002. [Google Scholar] [CrossRef]
- Semenok, D.V.; Troyan, I.A.; Ivanova, A.G.; Kvashnin, A.G.; Kruglov, I.A.; Hanfland, M.; Sadakov, A.V.; Sobolevskiy, O.A.; Pervakov, K.S.; Lyubutin, I.S.; et al. Superconductivity at 253 K in lanthanum–yttrium ternary hydrides. Mater. Today 2021, 48, 18–28. [Google Scholar] [CrossRef]
- Talantsev, E.F.; Crump, W.P.; Storey, J.G.; Tallon, J.L. London penetration depth and thermal fluctuations in the sulphur hydride 203 K superconductor. Ann. Der Phys. 2017, 529, 1600390. [Google Scholar] [CrossRef]
- Troyan, I.A.; Semenok, D.V.; Kvashnin, A.G.; Sadakov, A.V.; Sobolevskiy, O.A.; Pudalov, V.M.; Ivanova, A.G.; Prakapenka, V.B.; Greenberg, E.; Gavriliuk, A.G.; et al. Anomalous high-temperature superconductivity in YH6. Adv. Mater. 2021, 33, 2006832. [Google Scholar] [CrossRef]
- Kong, P.; Minkov, V.S.; Kuzovnikov, M.A.; Drozdov, A.P.; Besedin, S.P.; Mozaffari, S.; Balicas, L.; Balakirev, F.F.; Prakapenka, V.B.; Chariton, S.; et al. Superconductivity up to 243 K in the yttrium hydrogen system under high pressure. Nat. Commun. 2021, 12, 5075. [Google Scholar] [CrossRef] [PubMed]
- Silhanek, A.V.; Van Look, L.; Jonckheere, R.; Zhu, B.Y.; Raedts, S.; Moshchalkov, V.V. Enhanced vortex pinning by a composite antidot lattice in a superconducting Pb film. Phys. Rev. B 2005, 72, 014507. [Google Scholar] [CrossRef]
- Gutierrez, J.; Raes, B.; Van de Vondel, J.; Silhanek, A.V.; Kramer, R.B.G.; Ataklti, G.W.; Moshchalkov, V.V. First vortex entry into a perpendicularly magnetized superconducting thin film. Phys. Rev. B 2013, 88, 184504. [Google Scholar] [CrossRef]
- Adami, O.-A.; Jelić, Ž.L.; Xue, C.; Abdel-Hafiez, M.; Hackens, B.; Moshchalkov, V.V.; Milošević, M.V.; Van de Vondel, J.; Silhanek, A.V. Onset, evolution, and magnetic braking of vortex lattice instabilities in nanostructured superconducting films. Phys. Rev. B 2015, 92, 134506. [Google Scholar] [CrossRef]
- Backmeister, L.; Aichner, B.; Karrer, M.; Wurster, K.; Kleiner, R.; Goldobin, E.; Koelle, D.; Lang, W. Ordered Bose glass of vortices in superconducting YBa2Cu3O7thin films with a periodic pin lattice created by focused helium ion irradiation. Nanomaterials 2022, 12, 3491. [Google Scholar] [CrossRef] [PubMed]
- Hänisch, J.; Huang, Y.; Li, D.; Yuan, J.; Jin, K.; Dong, X.; Talantsev, E.; Holzapfel, B.; Zhao, Z. Anisotropy of flux pinning properties in superconducting (Li, Fe) OHFeSe thin films. Supercond. Sci. Technol. 2020, 33, 114009. [Google Scholar] [CrossRef]
- Li, D.; Shen, P.; Tian, J.; He, G.; Ni, S.; Wang, Z.; Xi, C.; Pi, L.; Zhang, H.; Yuan, J.; et al. A disorder-sensitive emergent vortex phase identified in high-Tc superconductor (Li,Fe)OHFeSe. Supercond. Sci. Technol. 2022, 35, 064007. [Google Scholar] [CrossRef]
- Talantsev, E.F.; Crump, W.P. Weak-links criterion for pnictide and cuprate superconductors. Supercond. Sci. Technol. 2018, 31, 124001. [Google Scholar] [CrossRef]
- Rupich, M.W. Second-generation (2G) coated high-temperature superconducting cables and wires for power grid applications. In Superconductors in the Power Grid: Materials and Applications; Woodhead Publishing: Sawston, UK, 2015; pp. 97–130. [Google Scholar]
- Talantsev, E.F.; Badcock, R.A.; Mataira, R.; Chong, C.V.; Bouloukakis, K.; Hamilton, K.; Long, N.J. Critical current retention of potted and unpotted REBCO Roebel cables under transverse pressure and thermal cycling. Supercond. Sci. Technol. 2017, 30, 045014. [Google Scholar] [CrossRef]
- van der Laan, D.C.; Kim, C.H.; Pamidi, S.V.; Weiss, J.D. A turnkey gaseous helium-cooled superconducting CORC® dc power cable with integrated current leads. Supercond. Sci. Technol. 2022, 35, 065002. [Google Scholar] [CrossRef]
- Noe, M.; Steurer, M. High-temperature superconductor fault current limiters: Concepts, applications, and development status. Supercond. Sci. Technol. 2007, 20, R15. [Google Scholar] [CrossRef]
- Yazdani-Asrami, M.; Staines, M.; Sidorov, G.; Eicher, A. Heat transfer and recovery performance enhancement of metal and superconducting tapes under high current pulses for improving fault current-limiting behavior of HTS transformers. Supercond. Sci. Technol. 2020, 33, 095014. [Google Scholar] [CrossRef]
- Yamamoto, M.; Yamaguchi, M.; Kaiho, K. Superconducting transformers. IEEE Trans. Power Deliv. 2000, 15, 599–603. [Google Scholar] [CrossRef]
- Morandi, A.; Trevisani, L.; Ribani, P.L.; Fabbri, M.; Martini, L.; Bocchi, M. Superconducting transformers: Key design aspects for power applications. J. Phys. Conf. Ser. 2007, 97, 012318. [Google Scholar] [CrossRef]
- Murphy, J.P.; Mullins, M.J.; Barnes, P.N.; Haugan, T.J.; Levin, G.A.; Majoros, M.; Sumption, M.D.; Collings, E.W.; Polak, M.; Mozola, P. Experiment setup for calorimetric measurements of losses in HTS coils due to AC current and external magnetic fields. IEEE Trans. Appl. Supercond. 2013, 23, 4701505. [Google Scholar] [CrossRef]
- Staines, M.; Glasson, N.; Pannu, M.; Thakur, K.P.; Badcock, R.; Allpress, N.; D’Souza, P.; Talantsev, E. The development of a Roebel cable based 1 MVA HTS transformer. Supercond. Sci. Technol. 2012, 25, 014002. [Google Scholar] [CrossRef]
- Harshman, R.; Fiory, A.T. High-Tc superconductivity in hydrogen clathrates mediated by Coulomb interactions between hydrogen and central-atom electrons. J. Supercond. Novel Magn. 2020, 33, 2945–2961. [Google Scholar] [CrossRef]
- Uemura, Y.J. Classifying superconductors in a plot of Tc versus Fermi temperature TF. Physica C 1991, 185–189, 733–734. [Google Scholar] [CrossRef]
- Talantsev, E.F.; Crump, W.P.; Tallon, J.L. Universal scaling of the self-field critical current in superconductors: From sub-nanometre to millimetre size. Sci. Rep. 2017, 7, 10010. [Google Scholar] [CrossRef]
- Homes, C.C.; Dordevic, S.V.; Strongin, M.; Bonn, D.A.; Liang, R.; Hardy, W.N.; Komiya, S.; Ando, Y.; Yu, G.; Kaneko, N.; et al. A universal scaling relation in high-temperature superconductors. Nature 2004, 430, 539–541. [Google Scholar] [CrossRef]
- Koblischka, R.; Koblischka-Veneva, A. Calculation of Tc of superconducting elements with the Roeser–Huber formalism. Metals 2022, 12, 337. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Talantsev, E.F. New Scaling Laws for Pinning Force Density in Superconductors. Condens. Matter 2022, 7, 74. https://doi.org/10.3390/condmat7040074
Talantsev EF. New Scaling Laws for Pinning Force Density in Superconductors. Condensed Matter. 2022; 7(4):74. https://doi.org/10.3390/condmat7040074
Chicago/Turabian StyleTalantsev, Evgueni F. 2022. "New Scaling Laws for Pinning Force Density in Superconductors" Condensed Matter 7, no. 4: 74. https://doi.org/10.3390/condmat7040074
APA StyleTalantsev, E. F. (2022). New Scaling Laws for Pinning Force Density in Superconductors. Condensed Matter, 7(4), 74. https://doi.org/10.3390/condmat7040074





