Spiral Thermal Waves Generated by Self-Propelled Camphor Boats
Abstract
1. Introduction
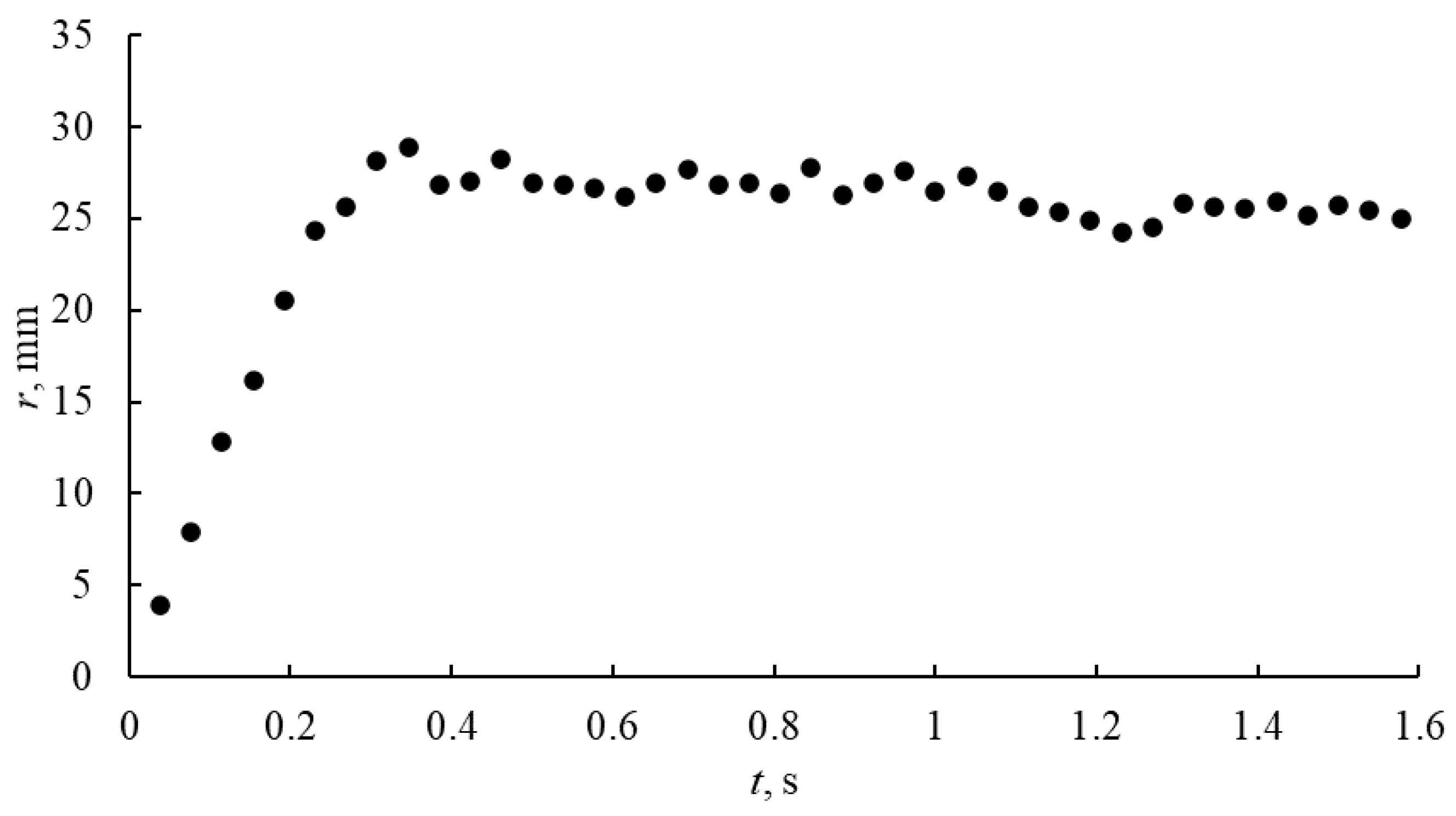
2. Results
3. Discussion
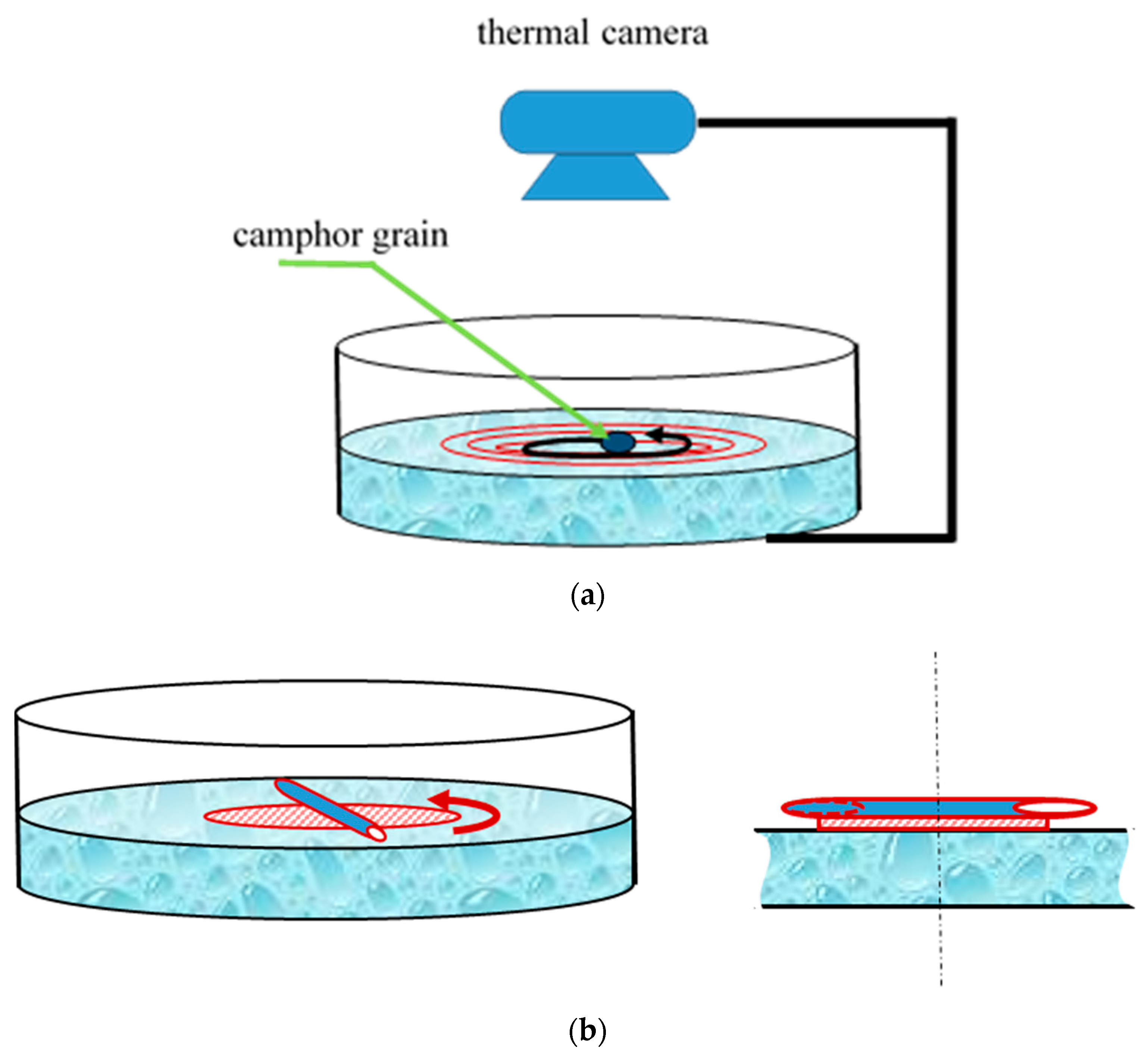
4. Materials and Methods
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| 2D | two-dimensional |
| ca | Circa (approximately) |
| Re | Reynolds number |
| Pr | Prandtl number |
References
- Lin, C.C.; Shu, F.H. On the Spiral Structure of Disc Galaxies, II. Outline of the Theory of Density Waves. Proc. Natl. Acad. Sci. USA 1966, 55, 229–234. [Google Scholar] [CrossRef]
- Li, L.; Liao, X.; Chan, K.H.; Zhang, K. On Nonlinear Multiarmed Spiral Waves in Slowly Rotating Fluid System. Phys. Fluids 2010, 22, 011701. [Google Scholar] [CrossRef]
- Barkley, D. Linear Stability Analysis of Rotating Spiral Waves in Excitable Media. Phys. Rev. Lett. 1992, 68, 2090–2093. [Google Scholar] [CrossRef]
- Net, M.; Mercader, I.; Knobloch, E. Binary Fluid Convection in a Rotating Cylinder. Phys. Fluids 1995, 7, 1553–1567. [Google Scholar] [CrossRef]
- Hassell, M.P.; Comins, H.N.; May, R.M. Spatial Structure and Chaos in Insect Population Dynamics. Nature 1991, 353, 255–258. [Google Scholar] [CrossRef]
- Keener, J.P.; Tyson, J.J. Spiral Waves in the Belousov-Zhabotinsky Reaction. Phys. D 1986, 21, 307–324. [Google Scholar] [CrossRef]
- Pfeiffer, P. Chemical Oscillations and Spiral Waves. In Spirals and Vortices. The Frontiers Collection; Tsuji, K., Müller, S., Eds.; Springer Nature: Cham, Switzerland, 2019. [Google Scholar]
- Adamatzky, A.; Fullarton, C.; Phillips, N.; Costello, B.D.L.; Draper, T.C. Thermal Switch of Oscillation Frequency in Belousov–Zhabotinsky Liquid Marbles. R. Soc. Open Sci. 2019, 6, 190078. [Google Scholar] [CrossRef]
- Adamatzky, A.; Holley, J.; Dittrich, P.; Gorecki, J.; Costello, B.D.L.; Zauner, K.-P.; Bull, L. On Architectures of Circuits Implemented in Simulated Belousov–Zhabotinsky Droplets. BioSystems 2012, 109, 72–77. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Li, S. Capillarity-Driven Migration of Small Objects: A Critical Review. Eur. Phys. J. E 2019, 42, 1–23. [Google Scholar] [CrossRef] [PubMed]
- Brosseau, Q.; Usabiaga, F.B.; Lushi, E.; Wu, Y.; Ristroph, L.; Zhang, J.; Ward, M.; Shelley, M.J. Relating Rheotaxis and Hydrodynamic Actuation Using Asymmetric Gold-Platinum Phoretic Rods. Phys. Rev. Lett. 2019, 123, 178004. [Google Scholar] [CrossRef]
- Pimienta, V.; Antoine, C. Self-Propulsion on Liquid Surfaces. Curr. Opin. Colloid Interface Sci. 2014, 19, 290–299. [Google Scholar] [CrossRef]
- Ryazantsev, Y.S.; Velarde, M.G.; Guzmán, E.; Rubio, R.G.; Ortega, F.; Montoya, J.-J. On the Autonomous Motion of Active Drops or Bubbles. J. Colloid Interface Sci. 2018, 527, 180–186. [Google Scholar] [CrossRef] [PubMed]
- Ooi, C.H.; Evans, G.M.; Gendelman, O.; Bormashenko, E.; Nguyen, A.V. A Floating Self-Propelling Liquid Marble Containing Aqueous Ethanol Solutions. RSC Adv. 2015, 5, 101006–101012. [Google Scholar] [CrossRef]
- Yuan, Z.; Hu, Z.; Chu, F.; Wu, X. Enhanced and Guided Self-Propelled Jumping on the Superhydrophobic Surfaces with Macrotexture. Appl. Phys. Lett. 2019, 115, 163701. [Google Scholar] [CrossRef]
- Frenkel, M.; Vilk, A.; Legchenkova, I.; Shoval, S.; Bormashenko, E. Mini-Generator of Electrical Power Exploiting the Marangoni Flow Inspired Self-Propulsion. ACS Omega 2019, 4, 15265–15268. [Google Scholar] [CrossRef]
- Liu, C.; Sun, J.; Zhuang, Y.; Wei, J.; Li, J.; Dong, L.; Yan, D.; Hu, A.; Zhou, X.; Wang, Z. Self-Propelled Droplet-Based Electricity Generation. Nanoscale 2018, 10, 23164–23169. [Google Scholar] [CrossRef]
- Zhang, L.; Yuan, Y.; Qiu, X.; Zhang, T.; Chen, Q.; Huang, X. Marangoni Effect-Driven Motion of Miniature Robots and Generation of Electricity on Water. Langmuir 2017, 33, 12609–12615. [Google Scholar] [CrossRef]
- Rayleigh, L. Measurements of the Amount of Oil Necessary in Order to Check the Motions of Camphor upon Water. Proc. R. Soc. Lond. 1890, 47, 364–367. [Google Scholar]
- Hayashima, Y.; Nagayama, M.; Nakata, S. A Camphor Grain Oscillates While Breaking Symmetry. J. Phys. Chem. B 2001, 105, 5353–5357. [Google Scholar] [CrossRef]
- Suematsu, N.J.; Nakata, S. Evolution of Self-Propelled Objects: From the Viewpoint of Nonlinear Science. Chem. Eur. J. 2018, 24, 6308–6324. [Google Scholar] [CrossRef]
- Nakata, S.; Kohira, M.I.; Hayashima, Y. Mode Selection of a Camphor Boat in a Dual-Circle Canal. Chem. Phys. Lett. 2000, 322, 419–423. [Google Scholar] [CrossRef]
- Koyano, Y.; Kitahata, H.; Nakata, S.; Gorecki, J. On a Simple Model That Explains Inversion of a Self-Propelled Rotor under Periodic Stop-And-Release-Operations. Chaos Interdiscip. J. Nonlinear Sci. 2020, 30, 023105. [Google Scholar] [CrossRef] [PubMed]
- Tadmor, R.; Baksi, A.; Gulec, S.; Jadhav, S.; N’Guessan, H.E.; Sen, K.; Somasi, V.; Tadmor, M.; Wasnik, P.; Yadav, S. Drops That Change Their Mind: Spontaneous Reversal from Spreading to Retraction. Langmuir 2019, 35, 15734–15738. [Google Scholar] [CrossRef] [PubMed]
- Bormashenko, E.; Bormashenko, Y.; Grynyov, R.; Aharoni, H.; Whyman, G.; Binks, B.P. Self-Propulsion of Liquid Marbles: Leidenfrost-Like Levitation Driven by Marangoni Flow. J. Phys. Chem. C 2015, 119, 9910–9915. [Google Scholar] [CrossRef]
- Venerus, D.C.; Simavilla, D.N. Tears of Wine: New Insights on an Old Phenomenon. Sci. Rep. 2015, 5, 16162. [Google Scholar] [CrossRef]
- Suematsu, N.J.; Sasaki, T.; Nakata, S.; Kitahata, H. Quantitative Estimation of the Parameters for Self-Motion Driven by Difference in Surface Tension. Langmuir 2014, 30, 8101–8108. [Google Scholar] [CrossRef]
- Haynes, W.M. CRC Handbook of Chemistry and Physics, 91st ed.; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]
- Winfree, A.T.; Delong, M.R. Spiral Waves of Chemical Activity. Science 1972, 175, 634–636. [Google Scholar] [CrossRef]
- Turing, A.M. The Chemical Basis of Morphogenesis. Philos. Trans. R. Soc. Lond. B 1952, 237, 37–72. [Google Scholar]
- Nakata, S.; Hiromatsu, S.-I. Intermittent Motion of a Camphor Float. Coll. Surf. A Physicochem. Eng. Asp. 2003, 224, 157–163. [Google Scholar] [CrossRef]
- Tadmor, R. Marangoni Flow Revisited. J. Coll. Interf. Sci. 2009, 332, 451–454. [Google Scholar] [CrossRef]
- Xie, H.; Ma, K.; Zhang, K.; Zhou, J.; Li, L.; Yang, W.; Gong, Y.; Cai, L.; Gong, K. Cell Cycle Arrest and Senescence in P53-Wild Type Renal Carcinoma by Enhancer RNA—P53-Bound Enhancer Regions 2(p53BER2) through p53-Dependent Pathway. J. Phys. Chem. B 2020, 124, 695–699. [Google Scholar]
- Chepelianskii, A.D.; Chevy, F.; Raphaël, E. Capillary-Gravity Waves Generated by a Slow Moving Object. Phys. Rev. Lett. 2008, 100, 074504. [Google Scholar] [CrossRef] [PubMed]
- Closa, F.; Chepelianskii, A.D.; Raphaël, E. Capillary-Gravity Waves Generated by a Sudden Object Motion. Phys. Fluids 2010, 22, 052107. [Google Scholar] [CrossRef]
- Burghelea, T.; Steinberg, V. Onset of Wave Drag Due to Generation of Capillary-Gravity Waves by a Moving Object as a Critical Phenomenon. Phys. Rev. Lett. 2001, 86, 2557–2560. [Google Scholar] [CrossRef] [PubMed]
- Burghelea, T.; Steinberg, V. Wave Drag Due to Generation of Capillary-Gravity Surface Waves. Phys. Rev. E 2002, 66, 051204. [Google Scholar] [CrossRef] [PubMed]
- Frenkel, M.; Whyman, G.; Shulzinger, E.; Starostin, A.; Bormashenko, E. Self-Propelling Rotator Driven by Soluto-Capillary Marangoni Flows. Appl. Phys. Lett. 2017, 110, 131604. [Google Scholar] [CrossRef]
- Li, S.; Liu, J.; Hou, J. Curvature-Driven Bubbles or Droplets on the Spiral Surface. Sci. Rep. 2016, 6, 37888. [Google Scholar] [CrossRef]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vilk, A.; Legchenkova, I.; Frenkel, M.; Bormashenko, E. Spiral Thermal Waves Generated by Self-Propelled Camphor Boats. Condens. Matter 2020, 5, 51. https://doi.org/10.3390/condmat5030051
Vilk A, Legchenkova I, Frenkel M, Bormashenko E. Spiral Thermal Waves Generated by Self-Propelled Camphor Boats. Condensed Matter. 2020; 5(3):51. https://doi.org/10.3390/condmat5030051
Chicago/Turabian StyleVilk, Alla, Irina Legchenkova, Mark Frenkel, and Edward Bormashenko. 2020. "Spiral Thermal Waves Generated by Self-Propelled Camphor Boats" Condensed Matter 5, no. 3: 51. https://doi.org/10.3390/condmat5030051
APA StyleVilk, A., Legchenkova, I., Frenkel, M., & Bormashenko, E. (2020). Spiral Thermal Waves Generated by Self-Propelled Camphor Boats. Condensed Matter, 5(3), 51. https://doi.org/10.3390/condmat5030051






