Effect of Inter-Well Interactions on Non-Linear Beam Splitters for Matter-Wave Interferometers
Abstract
1. Introduction
2. The Model
- (i)
- Rabi with . Here, interactions are negligible and the tunnelling term dominates. The energy spectrum is linear, such as in an harmonic oscillator with levels separated by ;
- (ii)
- Fock with . Here, interactions dominate and the spectrum has a quadratic form, such as in a pairwise quasi-degenerate states with opposite imbalance ();
- (iii)
- Josephson with . Here, both interactions and tunnelling play a relevant role. The spectrum starts with a linear behavior and then becomes quadratic with pairwise quasi-degenerate states.
- (a)
- For large and negative , the ground state is the NOON state [56].
- (b)
- For attractive energies such that , the energy spectrum starts with a quadratic behavior, with pairwise degenerate states and then it becomes linear with levels separated by .
- (c)
- For , the energy spectrum is quadratic with pairwise degenerate states for large negative values of the interaction energy.
- (i)
- Fock regime: ;
- (ii)
- Rabi regime: the ground state is still close to a coherent state and one has ;
- (iii)
- Josephson regime: for low-lying energy states one can see that .
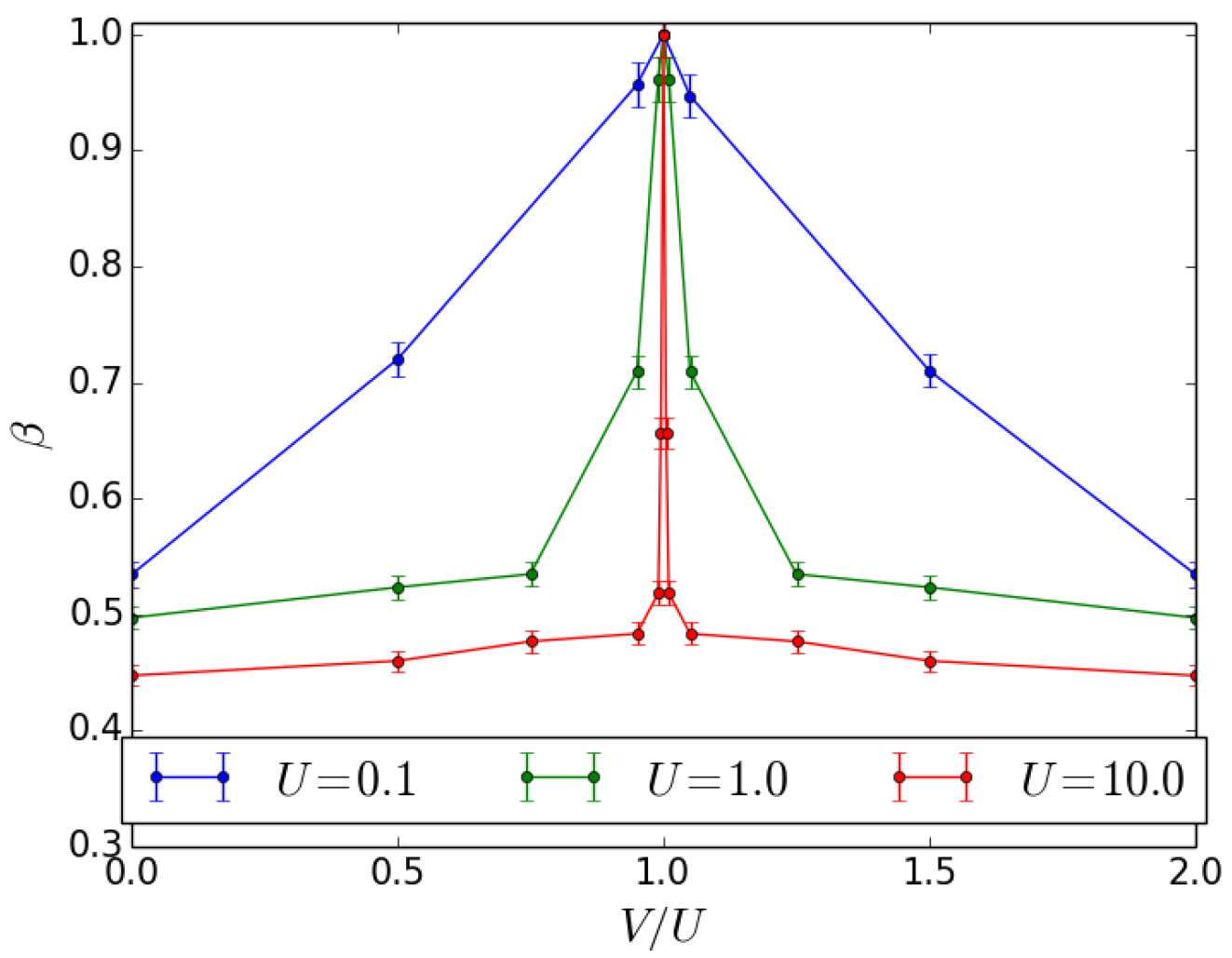
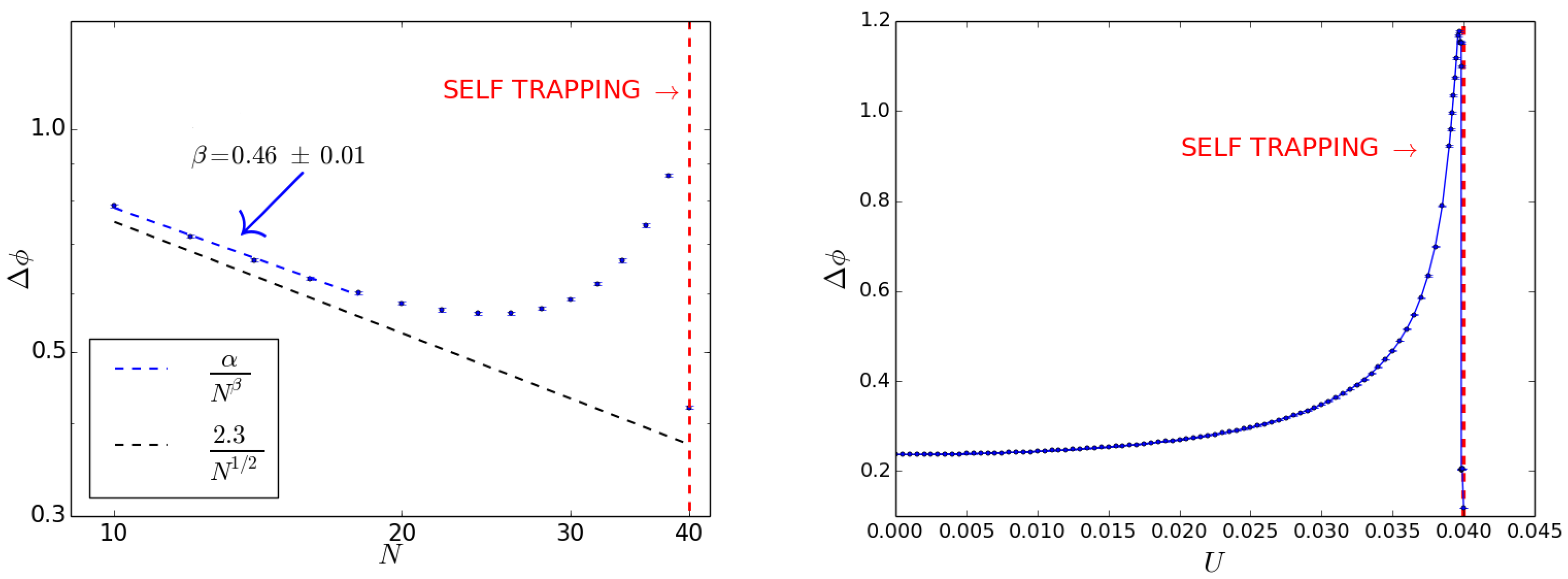
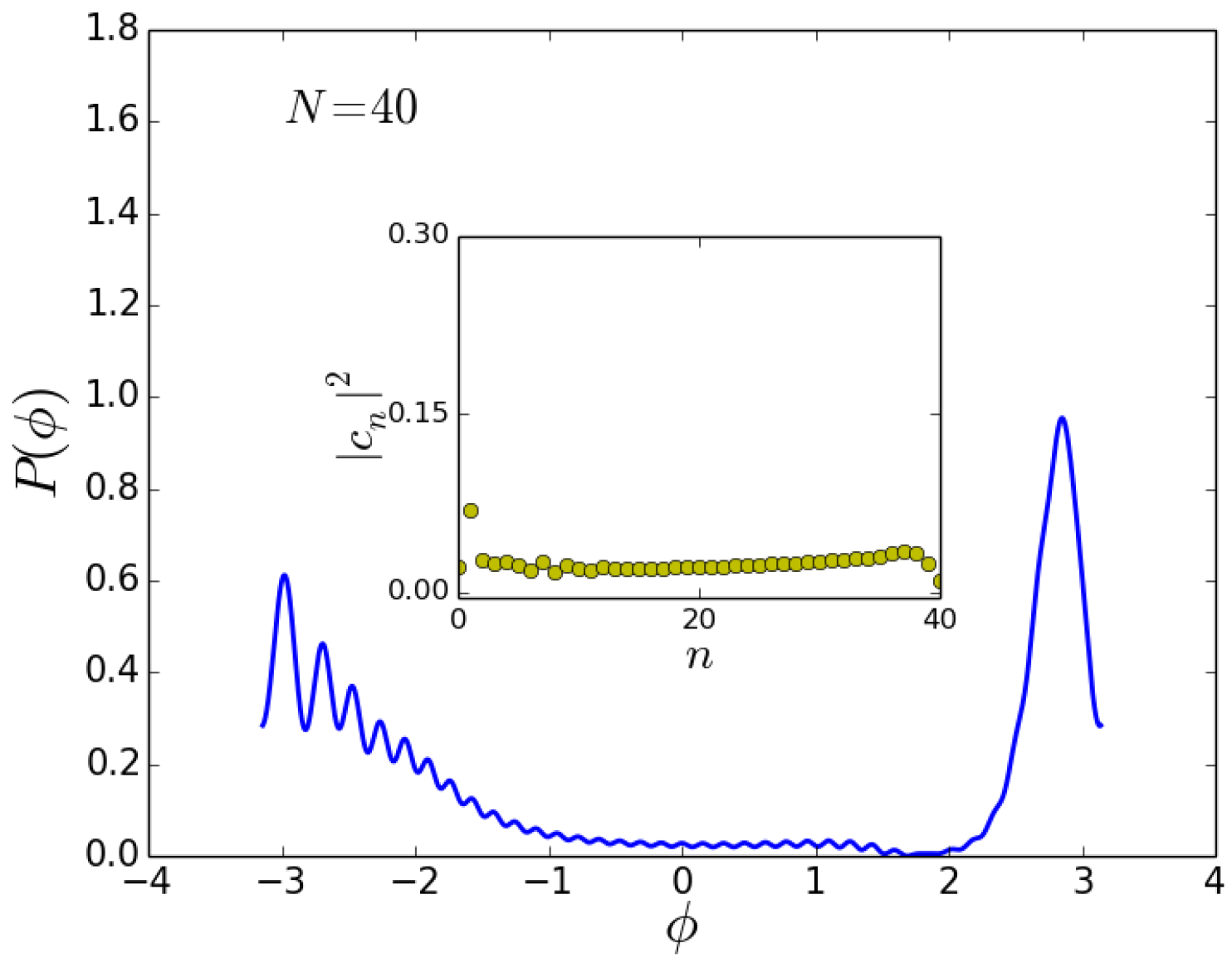
2.1. Self-Trapping Regime
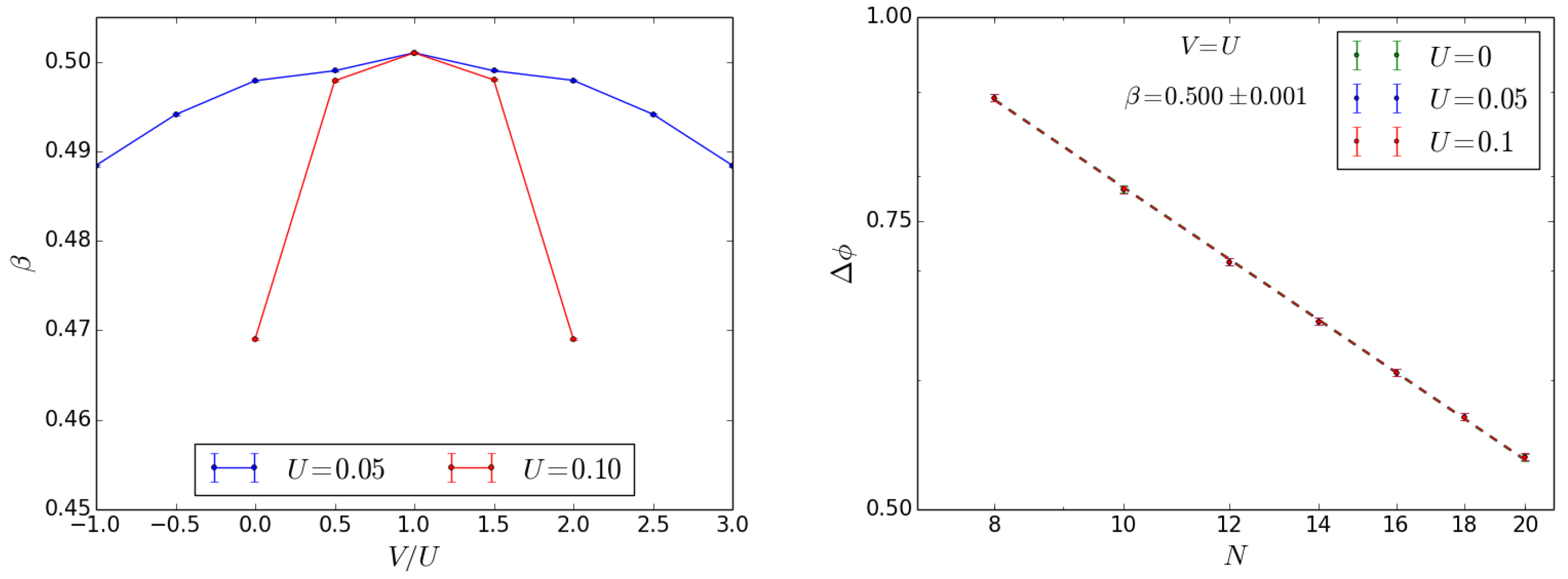
2.2. Squeezing and Sensitivity
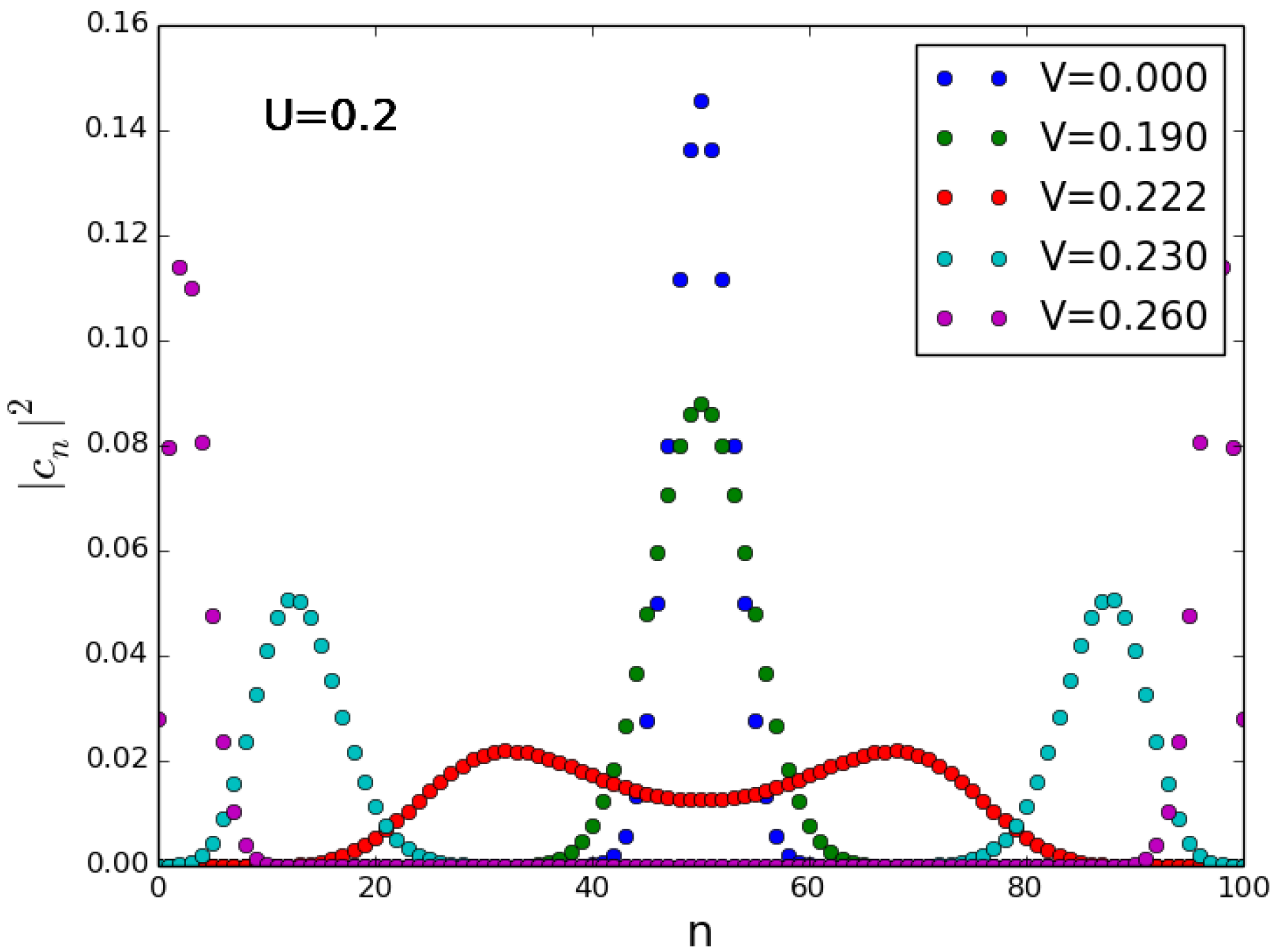
3. Inter-Well Interactions
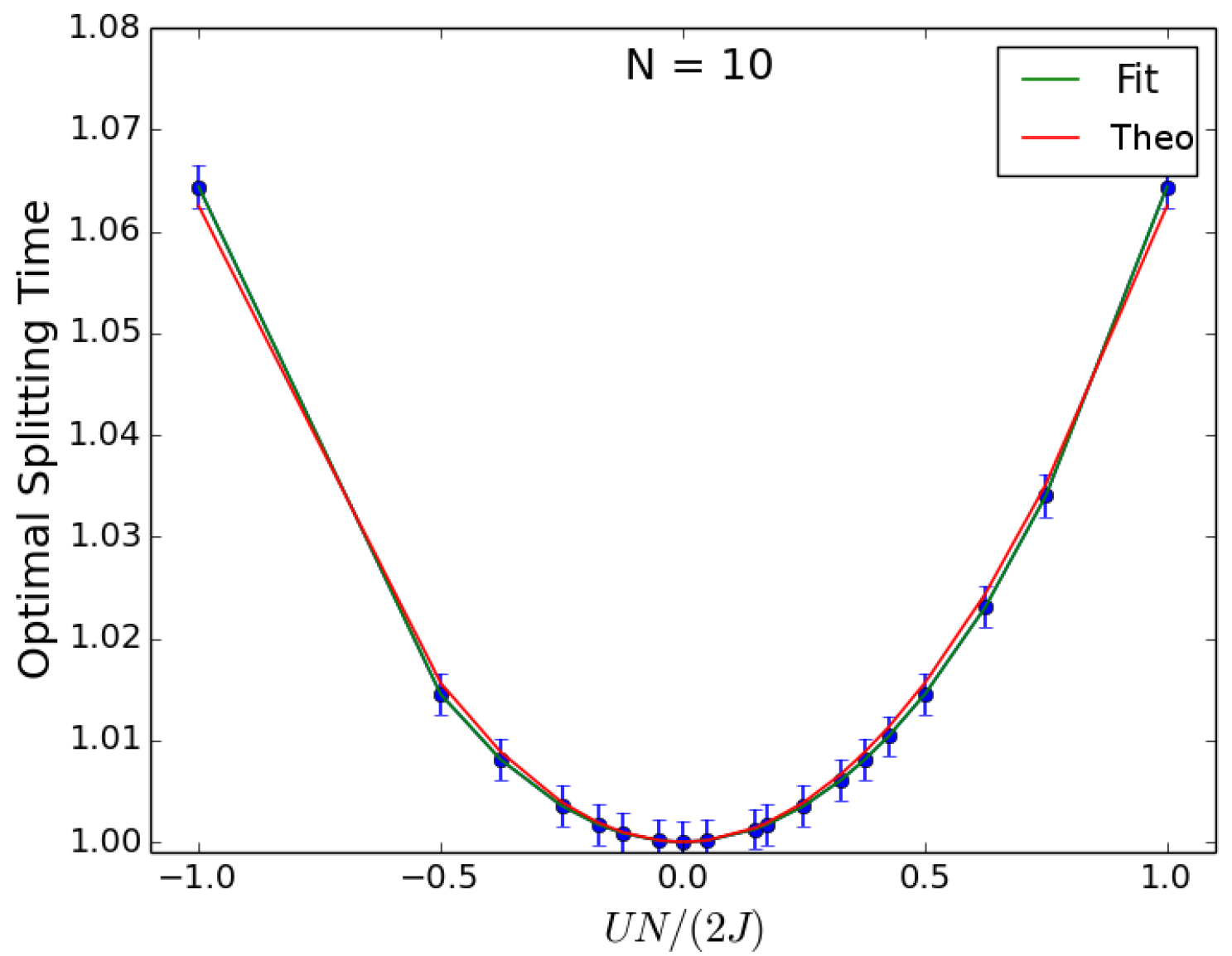
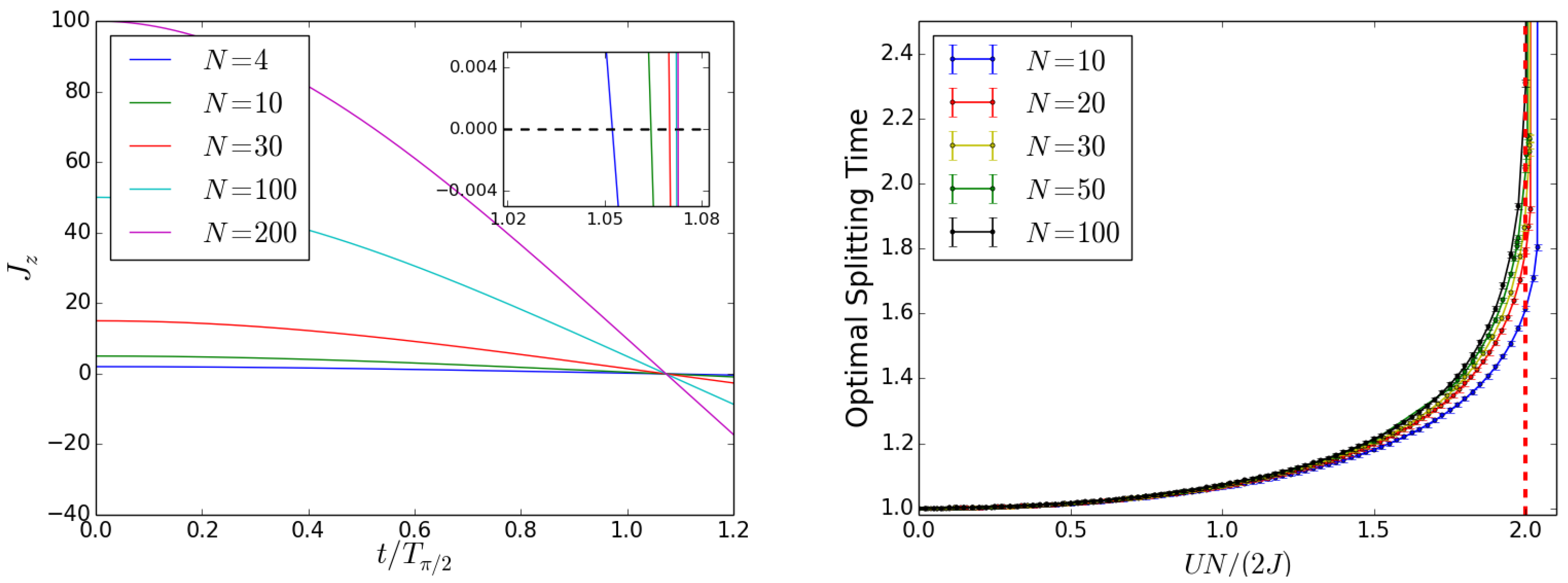
4. Beam Splitter
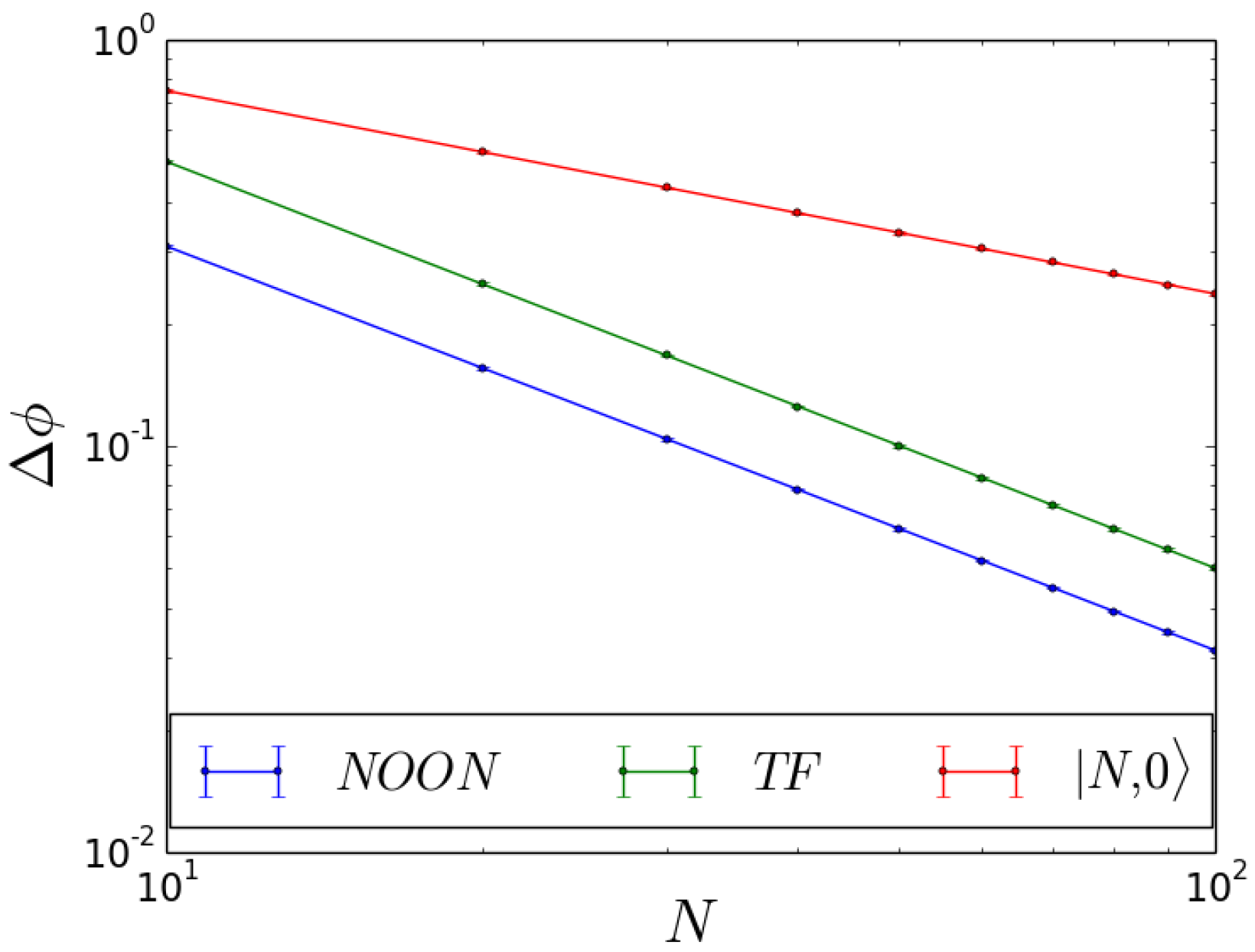
4.1. Initial State Twin Fock
4.2. Initial State
4.3. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Pezzé, L.; Smerzi, A.; Oberthaler, M.K.; Schmied, R.; Treutlein, P. Quantum metrology with nonclassical states of atomic ensembles. Rev. Mod. Phys. 2018, 90, 035005. [Google Scholar] [CrossRef]
- Pezzé, L.; Smerzi, A.; Berman, G.P.; Bishop, A.R.; Collins, L.A. Non-Linear Beam Splitter in Bose-Einstein Condensate Interferometers. Phys. Rev. A 2006, 74, 1–19. [Google Scholar] [CrossRef]
- Cronin, A.D.; Schmiedmayer, J.; Pritchard, D.E. Optics and interferometry with atoms and molecules. Rev. Mod. Phys. 2009, 81, 1051–1129. [Google Scholar] [CrossRef]
- Gross, C. Spin squeezing, entanglement and quantum metrology with Bose–Einstein condensates. J. Phys. B 2012, 45, 103001. [Google Scholar] [CrossRef]
- Bloch, I.; Dalibard, J.; Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 2008, 80, 885–964. [Google Scholar] [CrossRef]
- Gauguet, A.; Canuel, B.; Lévèque, T.; Chaibi, W.; Landragin, A. Characterization and limits of a cold-atom Sagnac interferometer. Phys. Rev. A 2009, 80, 063604. [Google Scholar] [CrossRef]
- Durr, S.; Nonn, T.; Rempe, G. Origin of quantum-mechanical complementarity probed by a “which-way” experiment in an atom interferometer. Nature 1998, 395, 33–37. [Google Scholar] [CrossRef]
- Rosi, G.; Sorrentino, F.; Cacciapuoti, L.; Prevedelli, M.; Tino, G.M. Precision measurement of the Newtonian gravitational constant using cold atoms. Nature 2014, 510, 518–521. [Google Scholar] [CrossRef]
- Smerzi, A.; Fantoni, S.; Giovanazzi, S.; Shenoy, S.R. Quantum Coherent Atomic Tunneling between Two Trapped Bose-Einstein Condensates. Phys. Rev. Lett. 1997, 79, 4950–4953. [Google Scholar] [CrossRef]
- Williams, J.; Walser, R.; Cooper, J.; Cornell, E.; Holland, M. Nonlinear Josephson-type oscillations of a driven, two-component Bose-Einstein condensate. Phys. Rev. A 1999, 59, R31–R34. [Google Scholar] [CrossRef]
- Cataliotti, F.S.; Burger, S.; Fort, C.; Maddaloni, P.; Minardi, F.; Trombettoni, A.; Smerzi, A.; Inguscio, M. Josephson Junction Arrays with Bose-Einstein Condensates. Science 2001, 293, 843–846. [Google Scholar] [CrossRef] [PubMed]
- Albiez, M.; Gati, R.; Fölling, J.; Hunsmann, S.; Cristiani, M.; Oberthaler, M.K. Direct Observation of Tunneling and Nonlinear Self-Trapping in a Single Bosonic Josephson Junction. Phys. Rev. Lett. 2005, 95, 010402. [Google Scholar] [CrossRef] [PubMed]
- Smerzi, A.; Trombettoni, A.; Lopez-Arias, T.; Fort, C.; Maddaloni, P.; Minardi, F.; Inguscio, M. Macroscopic oscillations between two weakly coupled Bose-Einstein condensates. Eur. Phys. J. B 2003, 31, 457–461. [Google Scholar] [CrossRef]
- Shin, Y.; Saba, M.; Pasquini, T.A.; Ketterle, W.; Pritchard, D.E.; Leanhardt, A.E. Atom Interferometry with Bose-Einstein Condensates in a Double-Well Potential. Phys. Rev. Lett. 2004, 92, 050405. [Google Scholar] [CrossRef] [PubMed]
- Schumm, T.; Hofferberth, S.; Andersson, L.M.; Wildermuth, S.; Groth, S.; Bar-Joseph, I.; Schmiedmayer, J.; Kruger, P. Matter-wave interferometry in a double well on an atom chip. Nat. Phys. 2005, 1, 57–62. [Google Scholar] [CrossRef]
- Anker, T.; Albiez, M.; Gati, R.; Hunsmann, S.; Eiermann, B.; Trombettoni, A.; Oberthaler, M.K. Nonlinear Self-Trapping of Matter Waves in Periodic Potentials. Phys. Rev. Lett. 2005, 94, 020403. [Google Scholar] [CrossRef]
- Levy, S.; Lahoud, E.; Shomroni, I.; Steinhauer, J. The a.c. and d.c. Josephson effects in a Bose-Einstein condensate. Nature 2007, 449, 579. [Google Scholar] [CrossRef]
- Hall, B.V.; Whitlock, S.; Anderson, R.; Hannaford, P.; Sidorov, A.I. Condensate Splitting in an Asymmetric Double Well for Atom Chip Based Sensors. Phys. Rev. Lett. 2007, 98, 030402. [Google Scholar] [CrossRef]
- Estève, J.; Gross, C.; Weller, A.; Giovanazzi, S.; Oberthaler, M.K. Squeezing and entanglement in a Bose–Einstein condensate. Nature 2008, 455, 1216. [Google Scholar] [CrossRef]
- Gross, C.; Zibold, T.; Nicklas, E.; Estève, J.; Oberthaler, M.K. Nonlinear atom interferometer surpasses classical precision limit. Nature 2010, 464, 1165–1169. [Google Scholar] [CrossRef]
- Riedel Max, F.; Böhi, P.; Li, Y.; Hänsch Theodor, W.; Sinatra, A.; Treutlein, P. Atom-chip-based generation of entanglement for quantum metrology. Nature 2010, 464, 1170–1173. [Google Scholar] [CrossRef] [PubMed]
- LeBlanc, L.J.; Bardon, A.B.; McKeever, J.; Extavour, M.H.T.; Jervis, D.; Thywissen, J.H.; Piazza, F.; Smerzi, A. Dynamics of a Tunable Superfluid Junction. Phys. Rev. Lett. 2011, 106, 025302. [Google Scholar] [CrossRef] [PubMed]
- Lücke, B.; Scherer, M.; Kruse, J.; Pezzé, L.; Deuretzbacher, F.; Hyllus, P.; Topic, O.; Peise, J.; Ertmer, W.; Arlt, J.; et al. Twin Matter Waves for Interferometry Beyond the Classical Limit. Science 2011, 334, 773–776. [Google Scholar] [CrossRef] [PubMed]
- Valtolina, G.; Burchianti, A.; Amico, A.; Neri, E.; Xhani, K.; Seman, J.A.; Trombettoni, A.; Smerzi, A.; Zaccanti, M.; Inguscio, M.; et al. Josephson effect in fermionic superfluids across the BEC-BCS crossover. Science 2015, 350, 1505–1508. [Google Scholar] [CrossRef]
- Trenkwalder, A.; Spagnolli, G.; Semeghini, G.; Coop, S.; Landini, M.; Castilho, P.; Pezzé, L.; Modugno, G.; Inguscio, M.; Smerzi, A.; et al. Quantum phase transitions with parity-symmetry breaking and hysteresis. Nat. Phys. 2016, 12, 826–829. [Google Scholar] [CrossRef]
- Spagnolli, G.; Semeghini, G.; Masi, L.; Ferioli, G.; Trenkwalder, A.; Coop, S.; Landini, M.; Pezzé, L.; Modugno, G.; Inguscio, M.; et al. Crossing Over from Attractive to Repulsive Interactions in a Tunneling Bosonic Josephson Junction. Phys. Rev. Lett. 2017, 118, 230403. [Google Scholar] [CrossRef] [PubMed]
- Burchianti, A.; Scazza, F.; Amico, A.; Valtolina, G.; Seman, J.A.; Fort, C.; Zaccanti, M.; Inguscio, M.; Roati, G. Connecting Dissipation and Phase Slips in a Josephson Junction between Fermionic Superfluids. Phys. Rev. Lett. 2018, 120, 025302. [Google Scholar] [CrossRef] [PubMed]
- Xhani, K.; Neri, E.; Galantucci, L.; Scazza, F.; Burchianti, A.; Lee, K.-L.; Barenghi, C.F.; Trombettoni, A.; Inguscio, M.; Zaccanti, M.; et al. Critical Transport and Vortex Dynamics in a Thin Atomic Josephson Junction. Phys. Rev. Lett. 2020, 124, 045301. [Google Scholar] [CrossRef] [PubMed]
- Pezzé, L.; Collins, L.A.; Smerzi, A.; Berman, G.P.; Bishop, A.R. Sub-shot-noise phase sensitivity with a Bose-Einstein condensate Mach-Zehnder interferometer. Phys. Rev. A 2005, 72, 043612. [Google Scholar] [CrossRef]
- Pezzé, L.; Smerzi, A. Phase sensitivity of a Mach-Zehnder interferometer. Phys. Rev. A 2006, 73, 011801. [Google Scholar] [CrossRef]
- Burkov, A.A.; Lukin, M.D.; Demler, E. Decoherence Dynamics in Low-Dimensional Cold Atom Interferometers. Phys. Rev. Lett. 2007, 98, 200404. [Google Scholar] [CrossRef]
- Pezzé, L.; Smerzi, A. Entanglement, Nonlinear Dynamics, and the Heisenberg Limit. Phys. Rev. Lett. 2009, 102, 100401. [Google Scholar] [CrossRef] [PubMed]
- Grond, J.; Hohenester, U.; Schmiedmayer, J.; Smerzi, A. Mach-Zehnder interferometry with interacting trapped Bose-Einstein condensates. Phys. Rev. A 2011, 84, 023619. [Google Scholar] [CrossRef]
- Pawlowski, K.; Fadel, M.; Treutlein, P.; Castin, Y.; Sinatra, A. Mesoscopic quantum superpositions in bimodal Bose-Einstein condensates: Decoherence and strategies to counteract it. Phys. Rev. A 2017, 95, 063609. [Google Scholar] [CrossRef]
- Wrubel, J.P.; Schwettmann, A.; Fahey, D.P.; Glassman, Z.; Pechkis, H.K.; Griffin, P.F.; Barnett, R.; Tiesinga, E.; Lett, P.D. Spinor Bose-Einstein-condensate phase-sensitive amplifier for SU(1,1) interferometry. Phys. Rev. A 2018, 98, 023620. [Google Scholar] [CrossRef]
- Zhang, Q.; Schwettmann, A. Quantum interferometry with microwave-dressed F = 1 spinor Bose-Einstein condensates: Role of initial states and long-time evolution. Phys. Rev. A 2019, 100, 063637. [Google Scholar] [CrossRef]
- Braun, D.; Adesso, G.; Benatti, F.; Floreanini, R.; Marzolino, U.; Mitchell, M.W.; Pirandola, S. Quantum-enhanced measurements without entanglement. Rev. Mod. Phys. 2018, 90, 035006. [Google Scholar] [CrossRef]
- Huang, Y.P.; Moore, M.G. Optimized Double-Well Quantum Interferometry with Gaussian Squeezed States. Phys. Rev. Lett. 2008, 100, 250406. [Google Scholar] [CrossRef]
- Grond, J.; von Winckel, G.; Schmiedmayer, J.; Hohenester, U. Optimal control of number squeezing in trapped Bose-Einstein condensates. Phys. Rev. A 2009, 80, 053625. [Google Scholar] [CrossRef]
- Jin, G.R.; An, Y.; Yan, T.; Lu, Z.S. Dynamical generation of phase-squeezed states in two-component Bose-Einstein condensates. Phys. Rev. A 2010, 82, 063622. [Google Scholar] [CrossRef]
- Mazzarella, G.; Malomed, B.; Salasnich, L.; Salerno, M.; Toigo, F. Rabi–Josephson oscillations and self-trapped dynamics in atomic junctions with two bosonic species. J. Phys. B 2011, 44, 035301. [Google Scholar] [CrossRef]
- Chwedeńczuk, J.; Hyllus, P.; Piazza, F.; Smerzi, A. Sub-shot-noise interferometry from measurements of the one-body density. New J. Phys. 2012, 14, 093001. [Google Scholar] [CrossRef]
- Mullin, W.J.; Laloë, F. Interference effects in potential wells. Phys. Rev. A 2015, 91, 053629. [Google Scholar] [CrossRef]
- Prakash, H.; Mishra, D.K. Quantum analysis of a beam splitter with second-order nonlinearity and generation of nonclassical light. J. Opt. Soc. Am. B 2016, 33, 1552–1557. [Google Scholar] [CrossRef]
- Berrada, T.; van Frank, S.; Bücker, R.; Schumm, T.; Schaff, J.-F.; Schmiedmayer, J.; Julía-Díaz, B.; Polls, A. Matter-wave recombiners for trapped Bose-Einstein condensates. Phys. Rev. A 2016, 93, 063620. [Google Scholar] [CrossRef]
- Vodola, D.; Lepori, L.; Ercolessi, E.; Gorshkov, A.V.; Pupillo, G. Kitaev Chains with Long-Range Pairing. Phys. Rev. Lett. 2014, 113, 156402. [Google Scholar] [CrossRef] [PubMed]
- Gong, Z.-X.; Maghrebi, M.F.; Hu, A.; Foss-Feig, M.; Richerme, P.; Monroe, C.; Gorshkov, A.V. Kaleidoscope of quantum phases in a long-range interacting spin-1 chain. Phys. Rev. B 2016, 93, 205115. [Google Scholar] [CrossRef]
- Lepori, L.; Vodola, D.; Pupillo, G.; Gori, G.; Trombettoni, A. Effective theory and breakdown of conformal symmetry in a long-range quantum chain. Ann. Phys. (N. Y.) 2016, 374, 35–66. [Google Scholar] [CrossRef]
- Defenu, N.; Trombettoni, A.; Ruffo, S. Criticality and phase diagram of quantum long-range O(N) models. Phys. Rev. B 2017, 96, 104432. [Google Scholar] [CrossRef]
- Blaß, B.; Rieger, H.; Roósz, G.; Iglói, F. Quantum Relaxation and Metastability of Lattice Bosons with Cavity-Induced Long-Range Interactions. Phys. Rev. Lett. 2018, 121, 095301. [Google Scholar] [CrossRef]
- Defenu, N.; Enss, T.; Kastner, M.; Morigi, G. Dynamical Critical Scaling of Long-Range Interacting Quantum Magnets. Phys. Rev. Lett. 2018, 121, 240403. [Google Scholar] [CrossRef] [PubMed]
- Lerose, A.; Žunkovič, B.; Silva, A.; Gambassi, A. Quasilocalized excitations induced by long-range interactions in translationally invariant quantum spin chains. Phys. Rev. B 2019, 99, 121112. [Google Scholar] [CrossRef]
- Lahaye, T.; Menotti, C.; Santos, L.; Lewenstein, M.; Pfau, T. The physics of dipolar bosonic quantum gases. Rep. Prog. Phys. 2009, 72, 126401. [Google Scholar] [CrossRef]
- Dalton, B.J.; Ghanbari, S. Two mode theory of Bose–Einstein condensates: Interferometry and the Josephson mode. J. Mod. Opt. 2012, 59, 287–353. [Google Scholar] [CrossRef]
- Leggett, A.J. Bose-Einstein condensation in the alkali gases: Some fundamental concepts. Rev. Mod. Phys. 2001, 73, 307–356. [Google Scholar] [CrossRef]
- Ho, T.-L.; Ciobanu, C.V. The Schrödinger Cat Family in Attractive Bose Gases. J. Low Temp. Phys. 2004, 135, 257–266. [Google Scholar] [CrossRef]
- Baroni, C. Effects of Interactions on the Performance of Ultracold Quantum Interferometers. Master’s Thesis, University of Pisa, Pisa, Italy, September 2017. [Google Scholar]
- Javanainen, J.; Ivanov, M.Y. Splitting a trap containing a Bose-Einstein condensate: Atom number fluctuation. Phys. Rev. A 1999, 60, 2351–2359. [Google Scholar] [CrossRef]
- Raghavan, S.; Smerzi, A.; Fantoni, S.; Shenoy, S.R. Coherent oscillations between two weakly coupled Bose-Einstein Condensates: Josephson effects, π-oscillations, and macroscopic quantum self trapping. Phys. Rev. A 1999, 59, 620. [Google Scholar] [CrossRef]
- Raghavan, S.; Smerzi, A.; Kenkre, V.M. Transitions in coherent oscillations between two trapped Bose-Einstein condensates. Phys. Rev. A 1999, 60, R1787–R1790. [Google Scholar] [CrossRef]
- Franzosi, R.; Penna, V.; Zecchina, R. Quantum dynamics of coupled bosonic wells within the Bose-Hubbard picture. Int. J. Mod. Phys. B 2000, 14, 943. [Google Scholar] [CrossRef]
- Smerzi, A.; Raghavan, S. Macroscopic quantum fluctuations in the Josephson dynamics of two weakly linked Bose-Einstein condensates. Phys. Rev. A 2000, 61, 063601. [Google Scholar] [CrossRef]
- Dell’Anna, L. Analytical approach to the two-site Bose-Hubbard model: From Fock states to Schrödinger cat states and entanglement entropy. Phys. Rev. A 2012, 85, 053608. [Google Scholar] [CrossRef]
- Wineland, D.J.; Bollinger, J.J.; Itano, W.M.; Heinzen, D.J. Squeezed atomic states and projection noise in spectroscopy. Phys. Rev. A 1994, 50, 67–88. [Google Scholar] [CrossRef] [PubMed]
- Leibfried, D.; Barrett, M.D.; Schaetz, T.; Britton, J.; Chiaverini, J.; Itano, W.M.; Jost, J.D.; Langer, C.; Wineland, D.J. Toward Heisenberg-Limited Spectroscopy with Multiparticle Entangled States. Science 2004, 304, 1476–1478. [Google Scholar] [CrossRef]
- Roos, C.F.; Chwalla, M.; Kim, K.; Riebe, M.; Blatt, R. ‘Designer atoms’ for quantum metrology. Nature 2006, 443, 316–319. [Google Scholar] [CrossRef] [PubMed]
- Gerry, C.C.; Mimih, J. The parity operator in quantum optical metrology. Contemp. Phys. 2010, 51, 497–511. [Google Scholar] [CrossRef]
- Mazzarella, G.; Dell’Anna, L. Two-mode dipolar bosonic junctions. Eur. Phys. J. Spec. Top. 2012, 217, 197. [Google Scholar] [CrossRef]
- Sackett, C.A.; Hulet, R.G. Dynamics of Bose-Einstein condensation in a gas with attractive interactions. J. Opt. B Quantum Semiclassical Opt. 2001, 3, R1. [Google Scholar] [CrossRef]




© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baroni, C.; Gori, G.; Chiofalo, M.L.; Trombettoni, A. Effect of Inter-Well Interactions on Non-Linear Beam Splitters for Matter-Wave Interferometers. Condens. Matter 2020, 5, 31. https://doi.org/10.3390/condmat5020031
Baroni C, Gori G, Chiofalo ML, Trombettoni A. Effect of Inter-Well Interactions on Non-Linear Beam Splitters for Matter-Wave Interferometers. Condensed Matter. 2020; 5(2):31. https://doi.org/10.3390/condmat5020031
Chicago/Turabian StyleBaroni, Cosetta, Giacomo Gori, Maria Luisa Chiofalo, and Andrea Trombettoni. 2020. "Effect of Inter-Well Interactions on Non-Linear Beam Splitters for Matter-Wave Interferometers" Condensed Matter 5, no. 2: 31. https://doi.org/10.3390/condmat5020031
APA StyleBaroni, C., Gori, G., Chiofalo, M. L., & Trombettoni, A. (2020). Effect of Inter-Well Interactions on Non-Linear Beam Splitters for Matter-Wave Interferometers. Condensed Matter, 5(2), 31. https://doi.org/10.3390/condmat5020031





