Quantum State of the Fermionic Carriers in a Transport Channel Connecting Particle Reservoirs
Abstract
:1. Introduction
2. The Model
2.1. The Particle Reservoir
2.2. The Transport Channel
2.3. The Coupling Hamiltonian
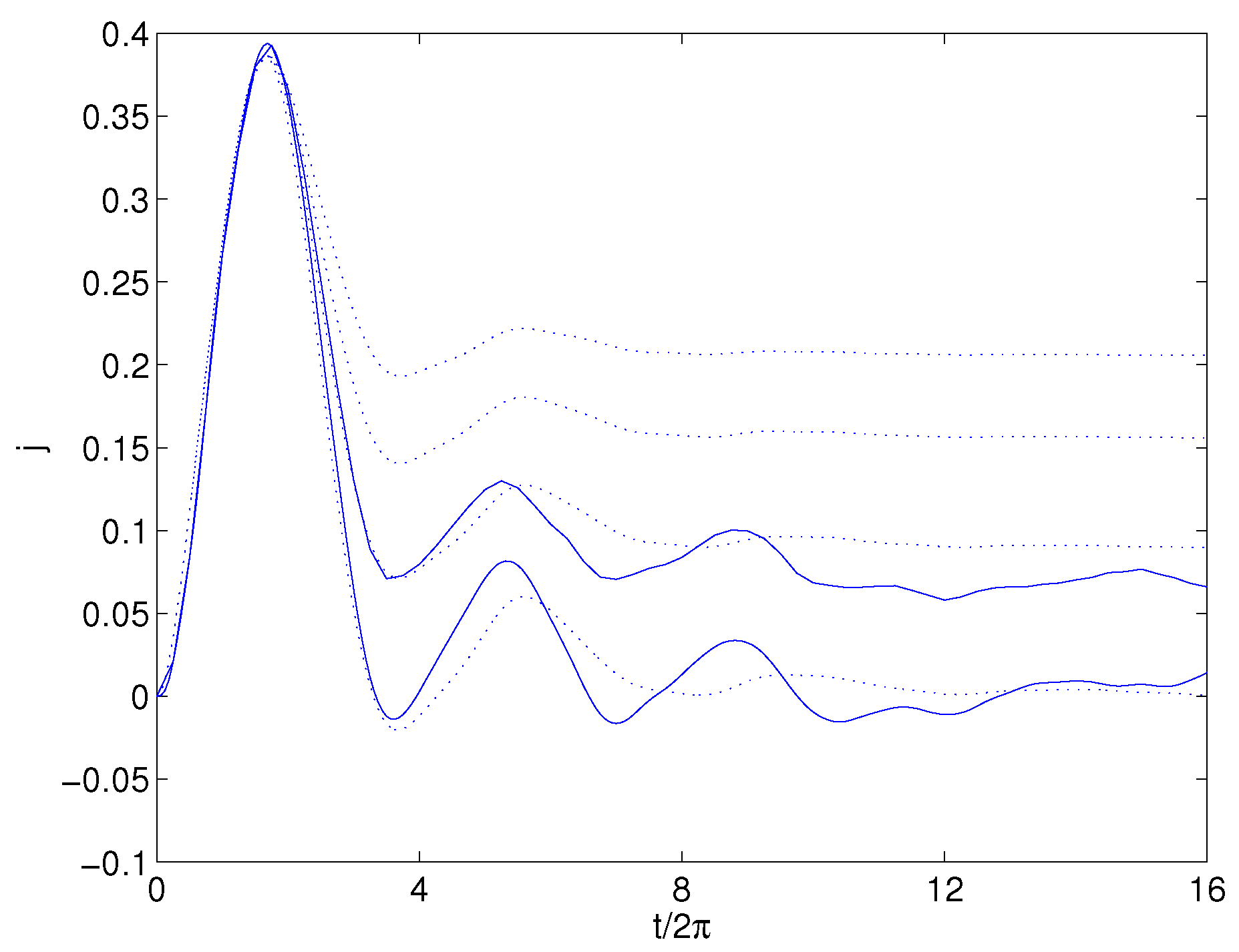
3. System Dynamics
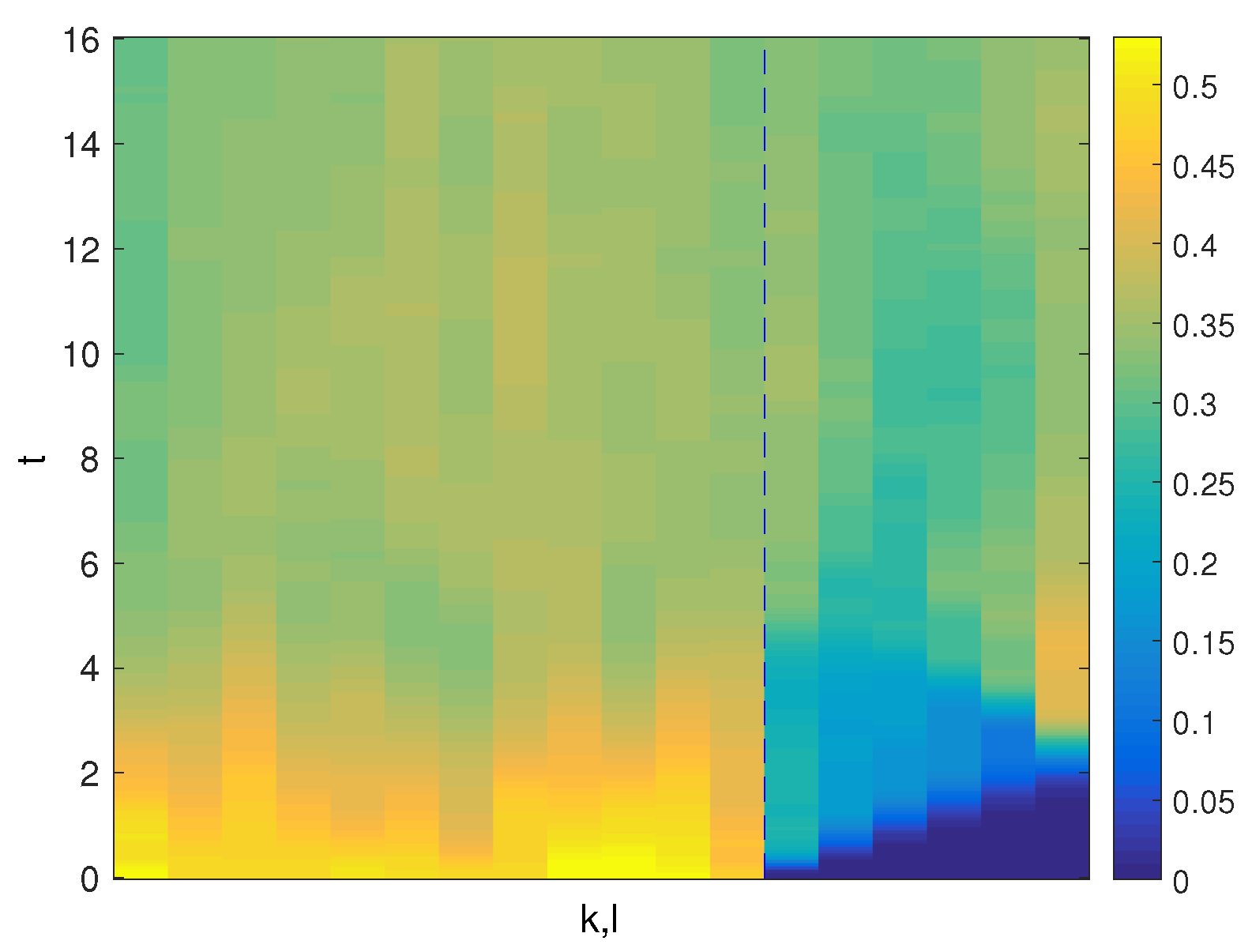
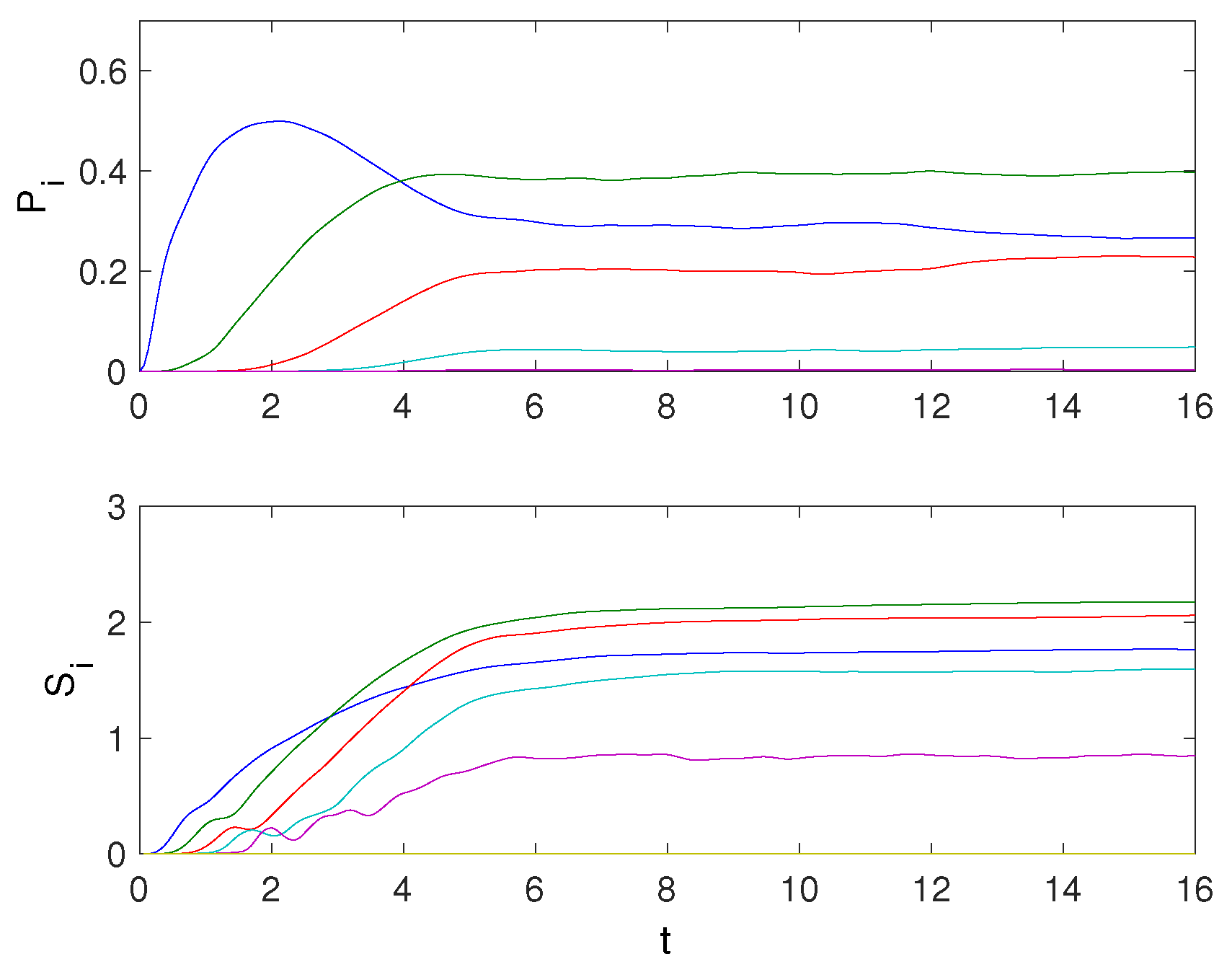
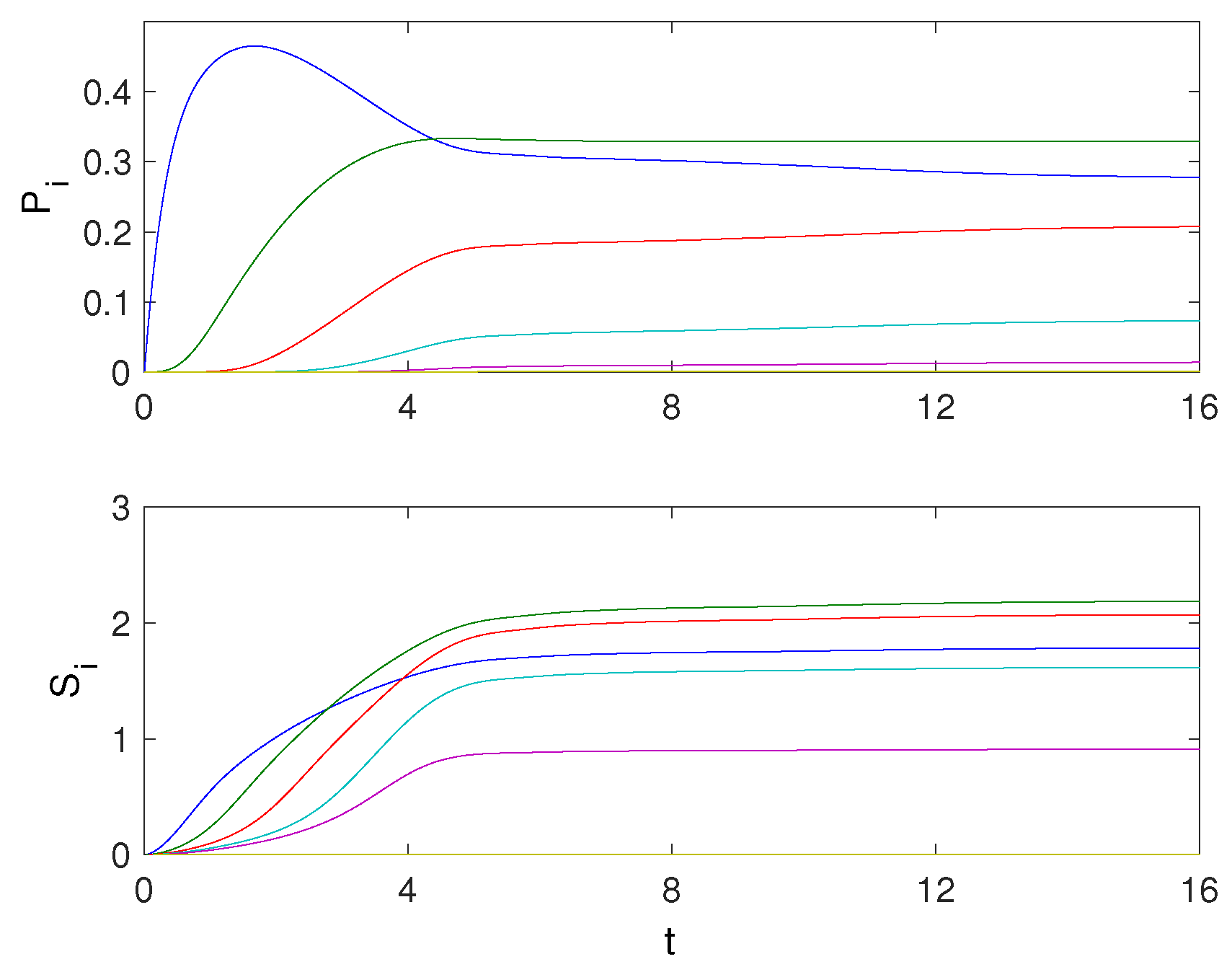
3.1. Population Dynamics
3.2. Decoherence Dynamics
4. Master Equation Approach
4.1. Populations and Decoherence Dynamics
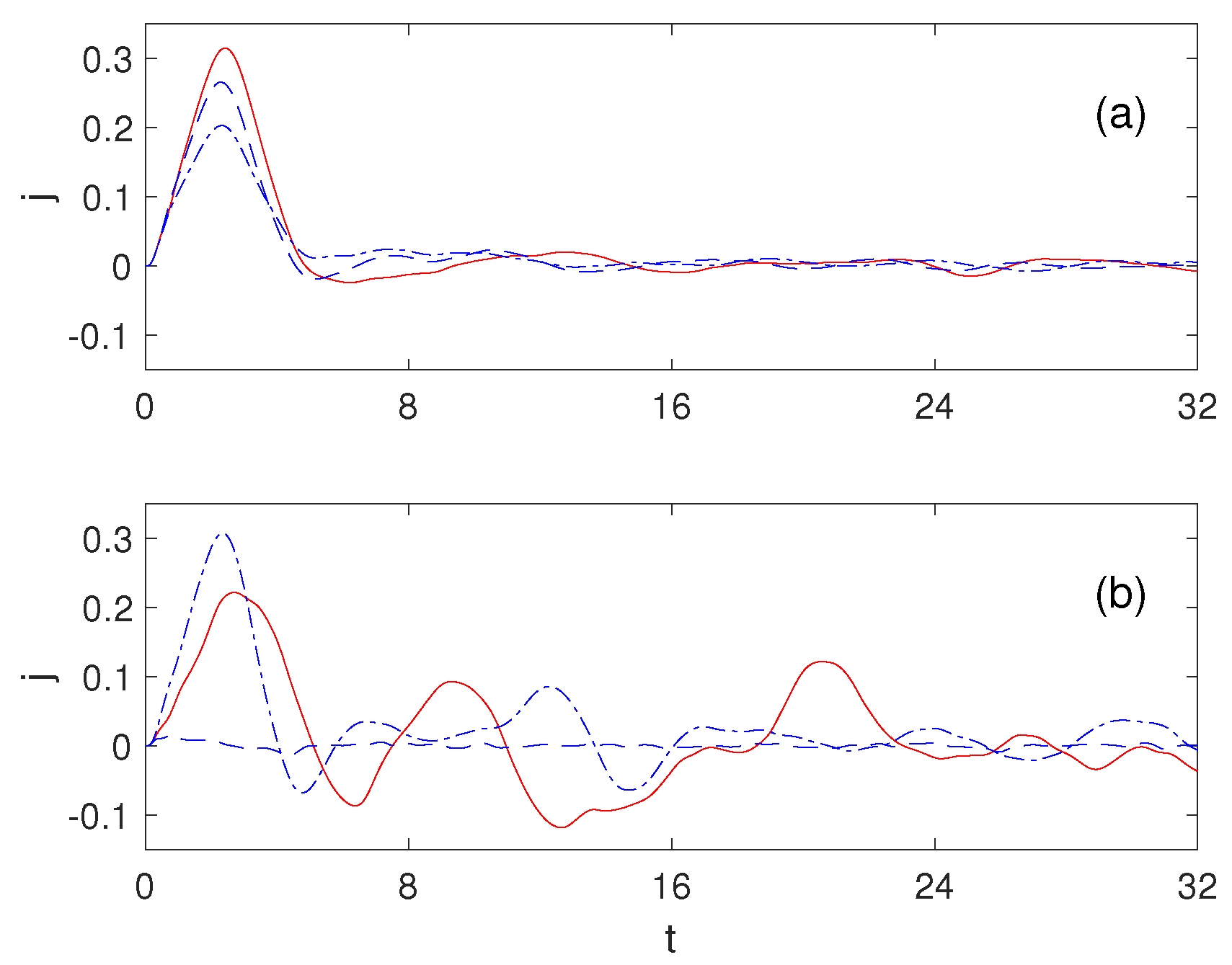
4.2. Stationary Current between Two Reservoirs
4.3. Quasi-Stationary Current
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Datta, S. Electronic Transport in Mesoscopic Systems; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Ferry, D.; Goodnick, S.M. Transport in Nanostructures; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Ihn, T. Semiconductor Nanostructures: Quantum States and Electronic Transport; Oxford University Press: Oxford, UK, 2010. [Google Scholar]
- Brantut, J.P.; Meineke, J.; Stadler, D.; Krinner, S.; Esslinger, T. Conduction of Ultracold Fermions Through a Mesoscopic Channel. Science 2012, 337, 1069–1071. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Husmann, D.; Uchino, S.; Krinner, S.; Lebrat, M.; Giamarchi, T.; Esslinger, T.; Brantut, J.P. Connecting strongly correlated superfluids by a quantum point contact. Science 2015, 350, 1498–1501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Krinner, S.; Stadler, D.; Husmann, D.; Brantut, J.P.; Esslinger, T. Observation of quantized conductance in neutral matter. Nature 2015, 517, 64–67. [Google Scholar] [CrossRef] [PubMed]
- Krinner, S.; Esslinger, T.; Brantut, J.P. Two-terminal transport measurements with cold atoms. J. Phys. Condens. Matter 2017, 29, 343003. [Google Scholar] [CrossRef]
- Bruderer, M.; Belzig, W. Mesoscopic transport of fermions through an engineered optical lattice connecting two reservoirs. Phys. Rev. A 2012, 85, 013623. [Google Scholar] [CrossRef]
- Gutman, D.B.; Gefen, Y.; Mirlin, A.D. Cold bosons in the Landauer setup. Phys. Rev. B 2012, 85, 125102. [Google Scholar] [CrossRef]
- Nietner, C.; Schaller, G.; Brandes, T. Transport with ultracold atoms at constant density. Phys. Rev. A 2014, 89, 013605. [Google Scholar] [CrossRef]
- Prosen, T. Exact Nonequilibrium Steady State of an Open Hubbard Chain. Phys. Rev. Lett. 2014, 112, 030603. [Google Scholar] [CrossRef]
- Simpson, D.; Gangardt, D.; Lerner, I.; Krüger, P. One-Dimensional Transport of Bosons between Weakly Linked Reservoirs. Phys. Rev. Lett. 2014, 112, 100601. [Google Scholar] [CrossRef] [Green Version]
- Chien, C.C.; Ventra, M.D.; Zwolak, M. Landauer, Kubo, and microcanonical approaches to quantum transport and noise: A comparison and implications for cold-atom dynamics. Phys. Rev. A 2014, 90, 023624. [Google Scholar] [CrossRef]
- Dujardin, J.; Argüelles, A.; Schlagheck, P. Elastic and inelastic transmission in guided atom lasers: A truncated Wigner approach. Phys. Rev. A 2015, 91, 033614. [Google Scholar] [CrossRef]
- Kordas, G.; Witthaut, D.; Wimberger, S. Non-equilibrium dynamics in dissipative Bose-Hubbard chains. Ann. Phys. 2015, 527, 619–628. [Google Scholar] [CrossRef] [Green Version]
- Olsen, M.K.; Bradley, A.S. Quantum ultracold atomtronics. Phys. Rev. A 2015, 91, 043635. [Google Scholar] [CrossRef] [Green Version]
- Caliga, S.C.; Straatsma, C.J.E.; Zozulya, A.A.; Anderson, D.Z. Principles of an atomtronic transistor. New J. Phys. 2016, 18, 015012. [Google Scholar] [CrossRef] [Green Version]
- Lai, C.Y.; Chien, C.C. Challenges and constraints of dynamically emerged source and sink in atomtronic circuits: From closed-system to open-system approaches. Sci. Rep. 2016, 6, 37256. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kolovsky, A.R.; Denis, Z.; Wimberger, S. Landauer-Büttiker equation for bosonic carriers. Phys. Rev. A 2018, 98, 043623. [Google Scholar] [CrossRef]
- Chakraborty, A.; Sensarma, R. Power-law tails and non-Markovian dynamics in open quantum systems: An exact solution from Keldysh field theory. Phys. Rev. B 2018, 97, 104306. [Google Scholar] [CrossRef] [Green Version]
- Mintchev, M.; Santoni, L.; Sorba, P. Microscopic Features of Bosonic Quantum Transport and Entropy Production. Ann. Phys. 2018, 530, 1800170. [Google Scholar] [CrossRef]
- Sekera, T.; Bruder, C.; Belzig, W. Thermoelectricity in a junction between interacting cold atomic Fermi gases. Phys. Rev. A 2016, 94, 033618. [Google Scholar] [CrossRef] [Green Version]
- Bohigas, O.; Flores, J. Spacing and individual eigenvalue distributions of two-body random Hamiltonians. Phys. Lett. B 1971, 35, 383–386. [Google Scholar] [CrossRef]
- French, J.B.; Wong, S.S.M. Some random-matrix level and spacing distributions for fixed-particle-rank interactions. Phys. Lett. B 1971, 35, 5–7. [Google Scholar] [CrossRef]
- Flambaum, V.V.; Izrailev, F.M. Distribution of occupation numbers in finite Fermi systems and role of interaction in chaos and thermalization. Phys. Rev. E 1997, 55, R13–R16. [Google Scholar] [CrossRef]
- Kolovsky, A.R.; Shepelyansky, D.L. Dynamical thermalization in isolated quantum dots and black holes. EPL (Europhys. Lett.) 2017, 117, 10003. [Google Scholar] [CrossRef] [Green Version]
- Kolovsky, A.R.; Shepelyansky, D.L. Evaporative cooling and self-thermalization in an open system of interacting fermions. arXiv 2019, arXiv:1902.06929. [Google Scholar]
- Stöckmann, H.J. Quantum Chaos: Introd; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Haake, F. Quantum Signatures of Chaos; Springer: Berlin, Germany, 2010. [Google Scholar]
- Åberg, S. Onset of chaos in rapidly rotating nuclei. Phys. Rev. Lett. 1990, 64, 3119–3122. [Google Scholar] [CrossRef]
- Breuer, H.P.; Petruccione, F. Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2002. [Google Scholar]
- Daley, A.J. Quantum trajectories and open many-body quantum systems. Adv. Phys. 2014, 63, 77–149. [Google Scholar] [CrossRef] [Green Version]
- Kolovsky, A.R. Number of degrees of freedom for a thermostat. Phys. Rev. E 1994, 50, 3569–3576. [Google Scholar] [CrossRef]
- Kolovsky, A.R. Microscopic models of source and sink for atomtronics. Phys. Rev. A 2017, 96, 011601. [Google Scholar] [CrossRef] [Green Version]
- Goetsch, P.; Graham, R. Decoherence by spontaneous emission in atomic-momentum transfer experiments. Phys. Rev. A 1996, 54, 5345–5348. [Google Scholar] [CrossRef] [Green Version]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kolovsky, A.R.; Maksimov, D.N. Quantum State of the Fermionic Carriers in a Transport Channel Connecting Particle Reservoirs. Condens. Matter 2019, 4, 85. https://doi.org/10.3390/condmat4040085
Kolovsky AR, Maksimov DN. Quantum State of the Fermionic Carriers in a Transport Channel Connecting Particle Reservoirs. Condensed Matter. 2019; 4(4):85. https://doi.org/10.3390/condmat4040085
Chicago/Turabian StyleKolovsky, Andrey R., and Dmitrii N. Maksimov. 2019. "Quantum State of the Fermionic Carriers in a Transport Channel Connecting Particle Reservoirs" Condensed Matter 4, no. 4: 85. https://doi.org/10.3390/condmat4040085
APA StyleKolovsky, A. R., & Maksimov, D. N. (2019). Quantum State of the Fermionic Carriers in a Transport Channel Connecting Particle Reservoirs. Condensed Matter, 4(4), 85. https://doi.org/10.3390/condmat4040085




