Ultrafast Transport Transient in n-Doped ZnS in Wurtzite and Zincblende Phases
Abstract
:1. Introduction
2. Transport Equations
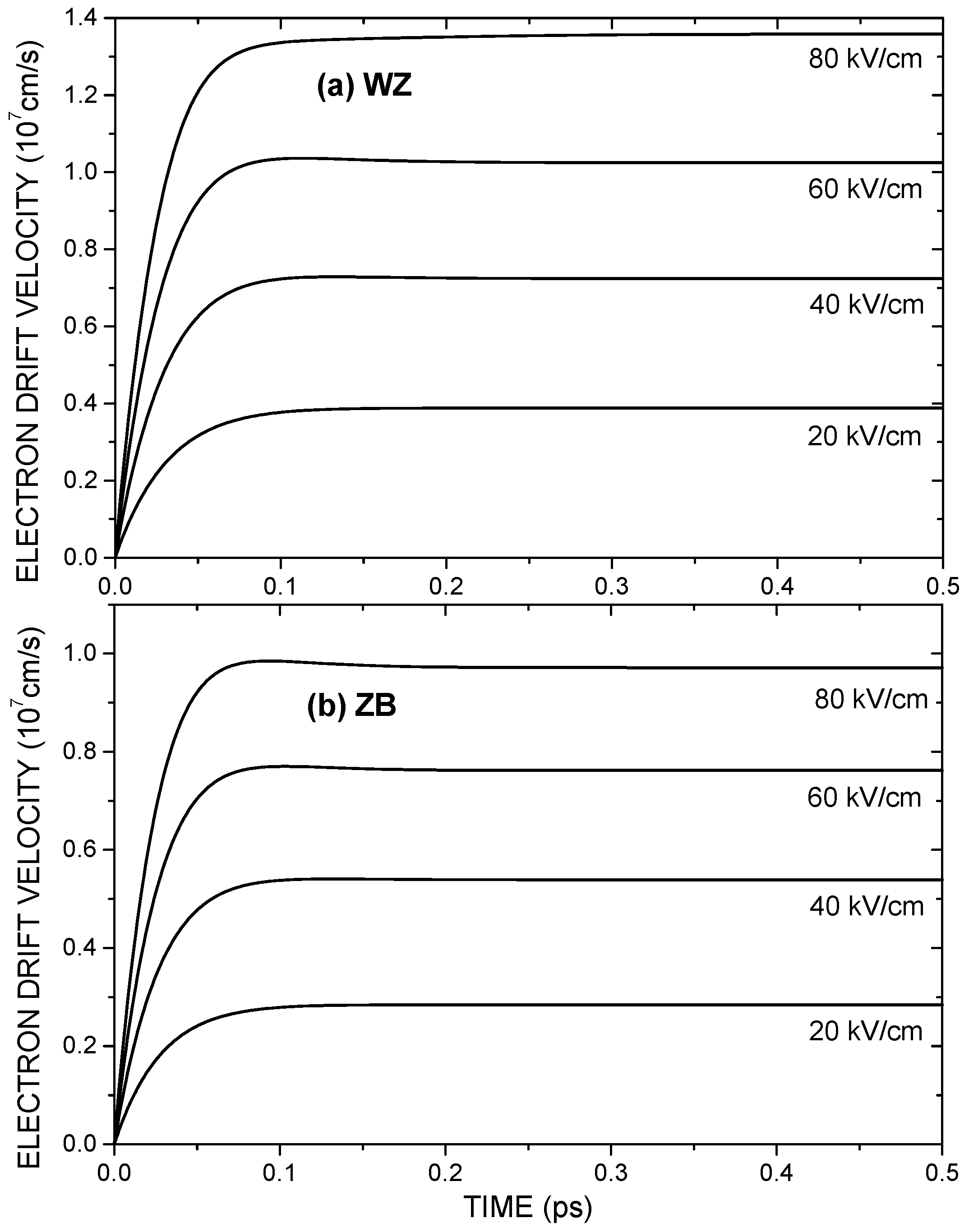
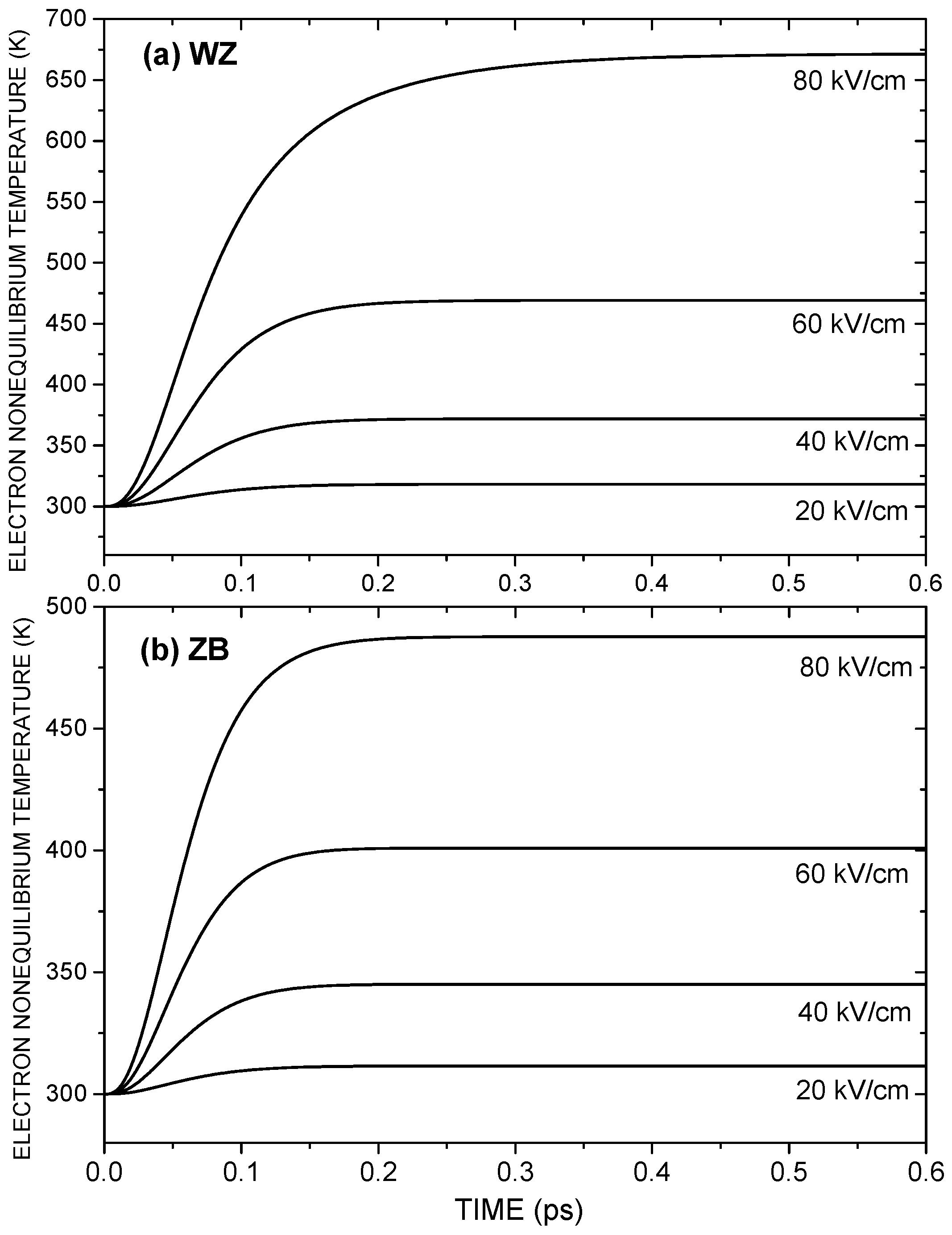
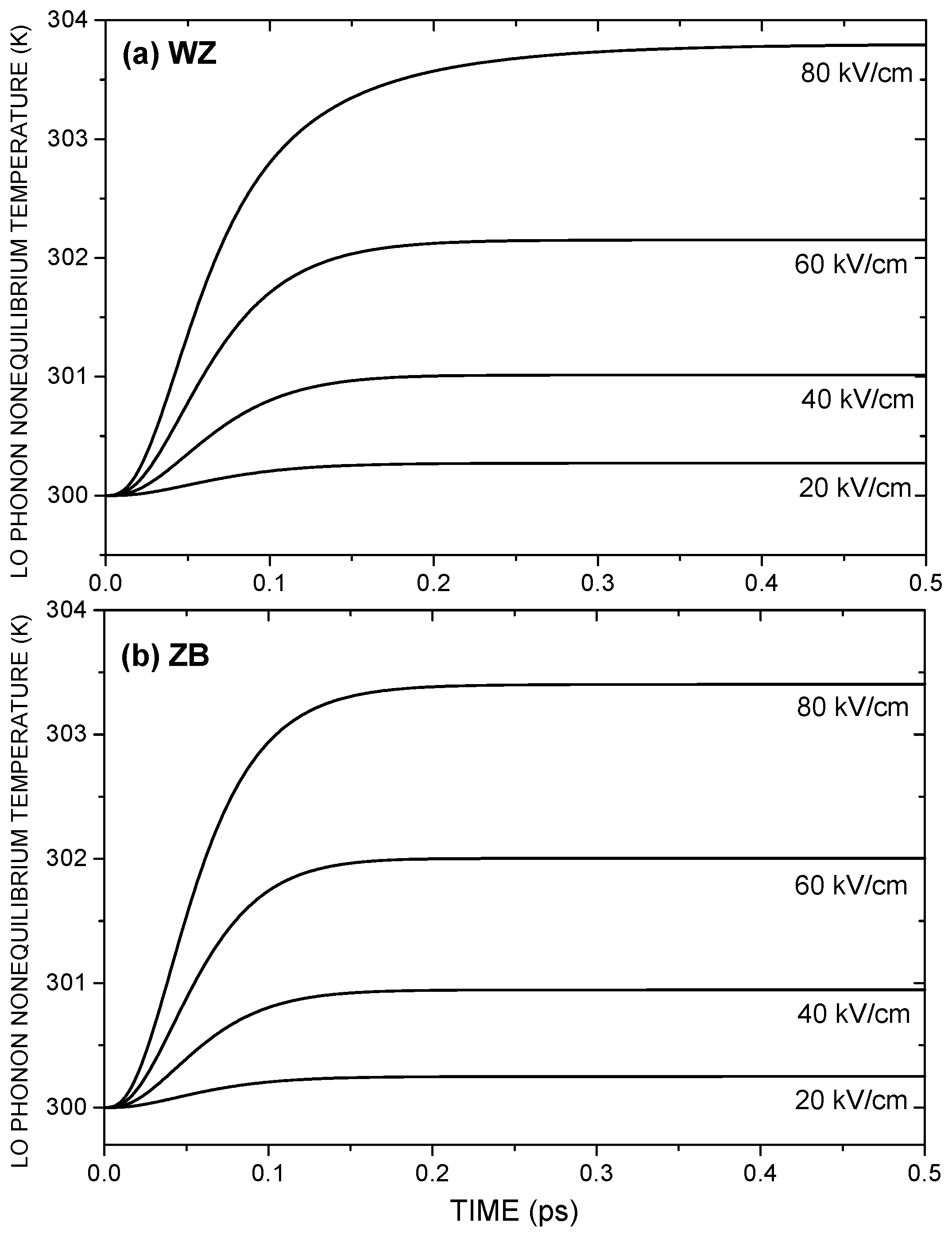
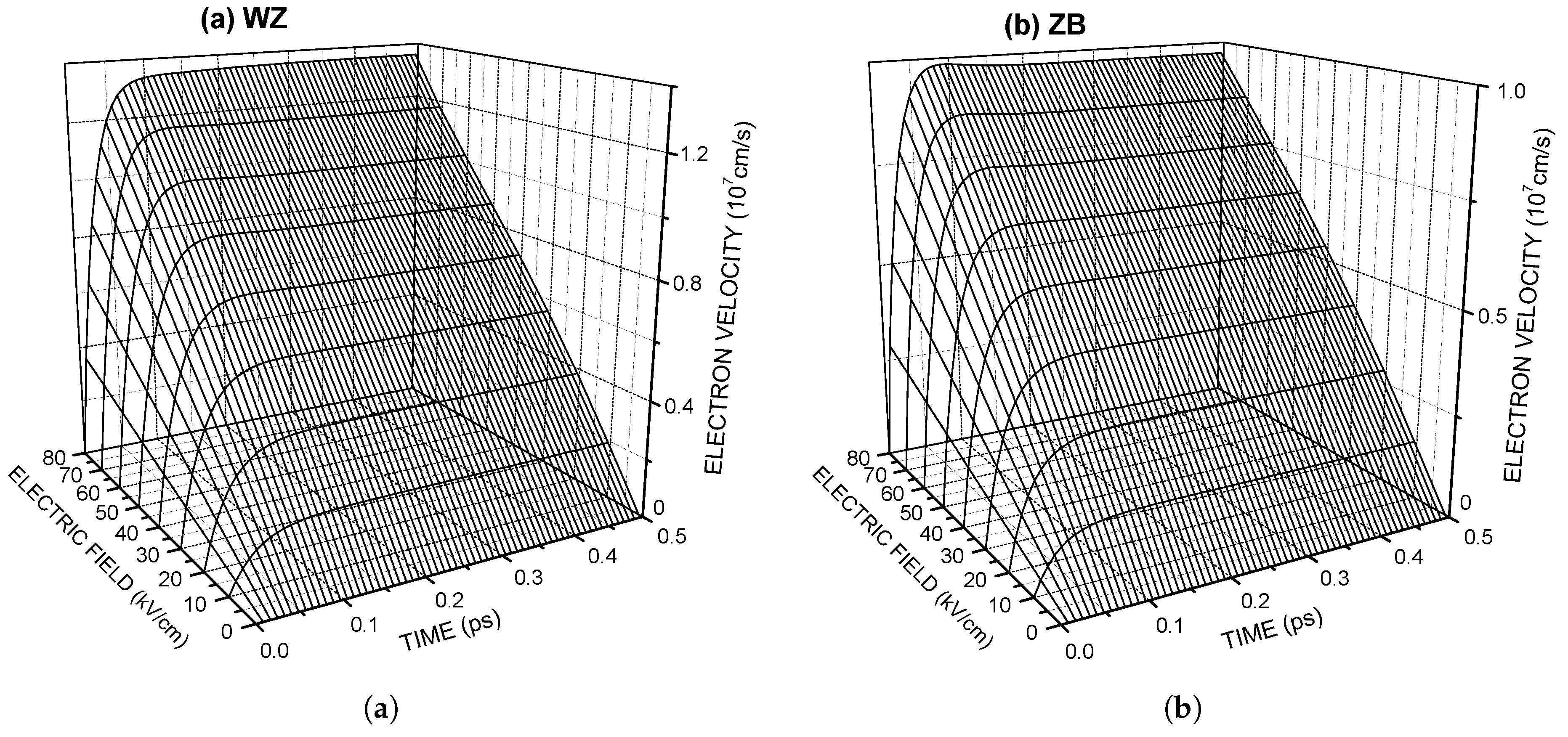
3. Results
4. Final Comments
Conflicts of Interest
References
- Nakamura, S.; Fasol, G. The Blue Laser Diode; Springer: Berlin, Germany, 1997. [Google Scholar]
- Mohammad, S.N.; Morkoç, H. Progress and prospects of group-III nitride semiconductors. Prog. Quantum Electron. 1996, 20, 361–525. [Google Scholar] [CrossRef]
- Akasaki, I.; Amano, H. Crystal growth and conductivity control of group III nitride semiconductors and their application to short wavelength light emitters. Jpn. J. Appl. Phys. 1997, 36, 5393–5408. [Google Scholar] [CrossRef]
- Takahashi, K.; Yoshikawa, A.; Sandhu, A. Wide Bandgap Semiconductors; Springer: Berlin, Germany, 2006. [Google Scholar]
- Piprek, J. Nitride Semiconductor Devices: Principles and Simulation; Wiley: New York, NY, USA, 2007. [Google Scholar]
- Morkoç, H. Handbook of Nitride Semiconductors and Devices; Wiley: New York, NY, USA, 2008. [Google Scholar]
- Morton, D.C.; Williams, F.E. A new thin-film electroluminescent material-ZnF2:Mn. Appl. Phys. Lett. 1979, 35, 671–672. [Google Scholar] [CrossRef]
- Okamoto, K.; Hamakawa, Y. Bright green electroluminescence in thin-film ZnS:TbF3. Appl. Phys. Lett. 1979, 35, 508–511. [Google Scholar] [CrossRef]
- Bryant, F.J.; Krier, A.; Zhong, G.Z. Blue electroluminescence in reverse-biased ZnS(Zn,Al) diodes. Solid State Electron. 1985, 28, 847–854. [Google Scholar] [CrossRef]
- Perry, T.S.; Wallich, P. Computer displays: New choices, new tradeoffs: Advances on several fronts let users and designers juggle lightness, brightness, and price as well as much-sought-after flatness. IEEE Spectr. 1985, 22, 52–53. [Google Scholar] [CrossRef]
- Ono, Y.A. Electroluminescent Displays; World Scientific: Singapore, 1995. [Google Scholar]
- Brennan, K. Theory of high-field electronic transport in bulk ZnS and ZnSe. J. Appl. Phys. 1988, 64, 4024–4030. [Google Scholar] [CrossRef]
- Dür, M.; Goodinick, S.M.; Pennathur, S.S.; Wager, J.F.; Reigrotzki, M.; Redmer, R. High-field transport and electroluminescence in ZnS phosphor layers. J. Appl. Phys. 1998, 83, 3176–3185. [Google Scholar] [CrossRef]
- Ruda, H.E.; Lai, B. Electron transport in ZnS. J. Appl. Phys. 1990, 68, 1714–1719. [Google Scholar] [CrossRef]
- Zubarev, D.N.; Morozov, V.; Röpke, G. Statistical Mechanics of Nonequilibrium Processes; Akademie: Berlin, Germany, 1996; Volumes 1 and 2. [Google Scholar]
- Luzzi, R.; Vasconcellos, A.R.; Ramos, J.G.P. Predictive Statistical Mechanics: A Nonequilibrium Ensemble Formalism; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2002. [Google Scholar]
- Luzzi, R.; Vasconcellos, A.R.; Ramos, J.G.P. Statistical Foundations of Irreversible Thermodynamics; Springer: Stuttgart, Germany, 2000. [Google Scholar]
- Rodrigues, C.G.; Freire, V.N.; Vasconcellos, A.R.; Luzzi, R. Electron mobility in nitride materials. Braz. J. Phys. 2002, 32, 439–441. [Google Scholar] [CrossRef]
- Rodrigues, C.G. Electron mobility in n-doped zinc sulphide. Microelectr. J. 2006, 37, 657–660. [Google Scholar] [CrossRef]
- Rodrigues, C.G.; Vasconcellos, A.R.; Luzzi, R. Non-linear electron mobility in n-doped III-nitrides. Braz. J. Phys. 2006, 36, 255–257. [Google Scholar] [CrossRef]
- Rodrigues, C.G.; Vasconcellos, A.R.; Luzzi, R. Optical properties of III-Nitrides under electric fields. Eur. Phys. J. B 2009, 72, 67–75. [Google Scholar] [CrossRef]
- Rodrigues, C.G.; Vasconcellos, A.R.; Luzzi, R. Evolution kinetics of nonequilibrium longitudinal-optical phonons generated by drifting electrons in III-nitrides: Longitudinal-optical-phonon resonance. J. Appl. Phys. 2010, 108, 033716. [Google Scholar] [CrossRef]
- Kuzemsky, A.L. Statistical Mechanics and the Physics of Many-Particle Model Systems; World Scientific: Singapore, 2017. [Google Scholar]
- Kuzemsky, A.L. Theory of transport processes and the method of the nonequilibrium statistical operator. Int. J. Mod. Phys. B 2007, 21, 2821–2949. [Google Scholar] [CrossRef]
- Kuzemsky, A.L. Electronic transport in metallic systems and generalized kinetic equations. Int. J. Mod. Phys. B 2011, 25, 3071–3183. [Google Scholar] [CrossRef]
- Harrison, W.A. Scattering of electrons by lattice vibrations in nonpolar crystals. Phys. Rev. 1956, 104, 1281–1290. [Google Scholar] [CrossRef]
- Rodrigues, C.G.; Vasconcellos, A.R.; Luzzi, R. A kinetic theory for nonlinear quantum transport. Transp. Theor. Stat. Phys. 2000, 29, 733–757. [Google Scholar] [CrossRef]
- Bhattacharyya, K.; Goodnick, S.M.; Wager, J.F. Monte Carlo simulation of electron transport in alternatingcurrent thinfilm electroluminescent devices. J. Appl. Phys. 1998, 73, 3390–3395. [Google Scholar] [CrossRef]
- Rodrigues, C.G.; Vasconcellos, A.R.; Luzzi, R.; Freire, V.N. Transient transport in III-nitrides: Interplay of momentum and energy relaxation times. J. Phys. Condens. Mat. 2007, 19, 346214. [Google Scholar] [CrossRef]

© 2017 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rodrigues, C.G. Ultrafast Transport Transient in n-Doped ZnS in Wurtzite and Zincblende Phases. Condens. Matter 2017, 2, 12. https://doi.org/10.3390/condmat2010012
Rodrigues CG. Ultrafast Transport Transient in n-Doped ZnS in Wurtzite and Zincblende Phases. Condensed Matter. 2017; 2(1):12. https://doi.org/10.3390/condmat2010012
Chicago/Turabian StyleRodrigues, Clóves G. 2017. "Ultrafast Transport Transient in n-Doped ZnS in Wurtzite and Zincblende Phases" Condensed Matter 2, no. 1: 12. https://doi.org/10.3390/condmat2010012
APA StyleRodrigues, C. G. (2017). Ultrafast Transport Transient in n-Doped ZnS in Wurtzite and Zincblende Phases. Condensed Matter, 2(1), 12. https://doi.org/10.3390/condmat2010012




