Using Footpad Sculpturing to Enhance the Maneuverability and Speed of a Robotic Marangoni Surfer
Abstract
:1. Introduction
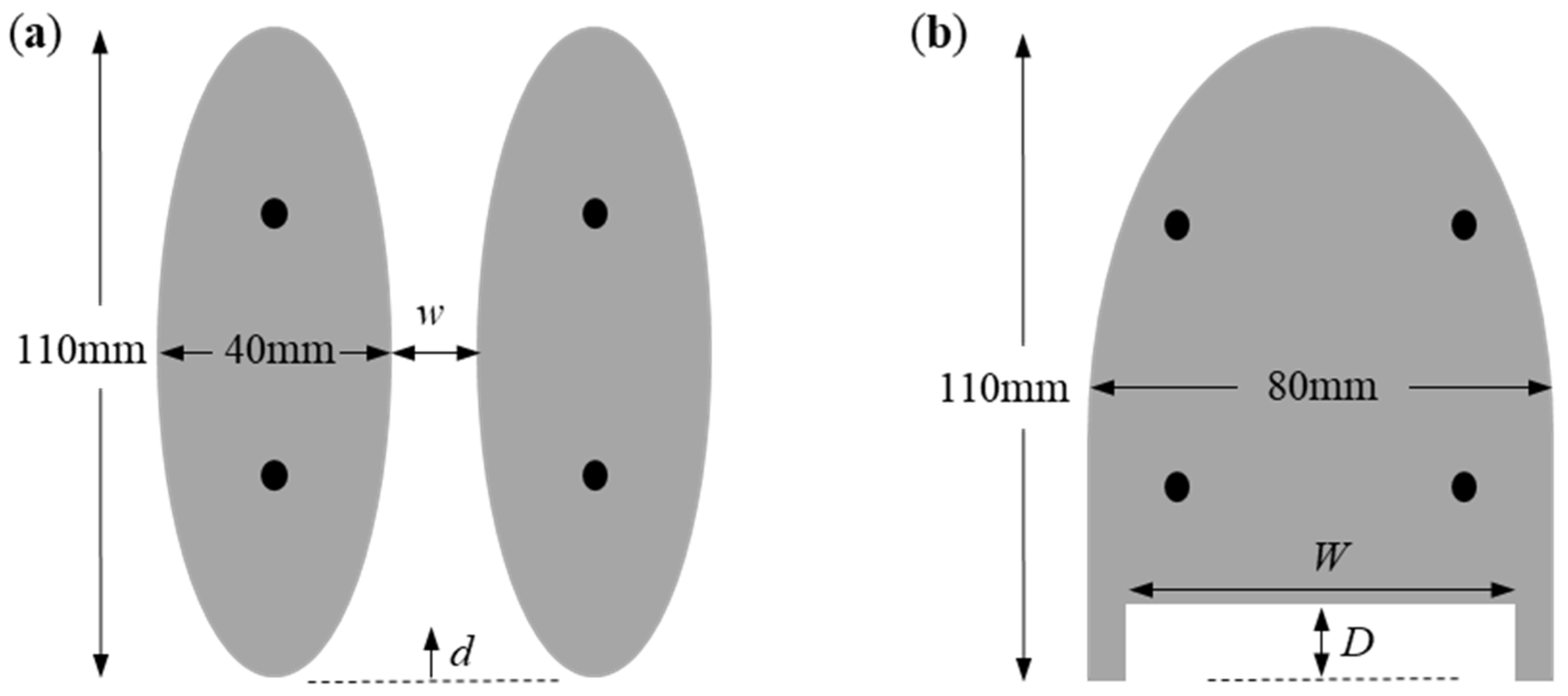
2. Robot Design
3. Experiments
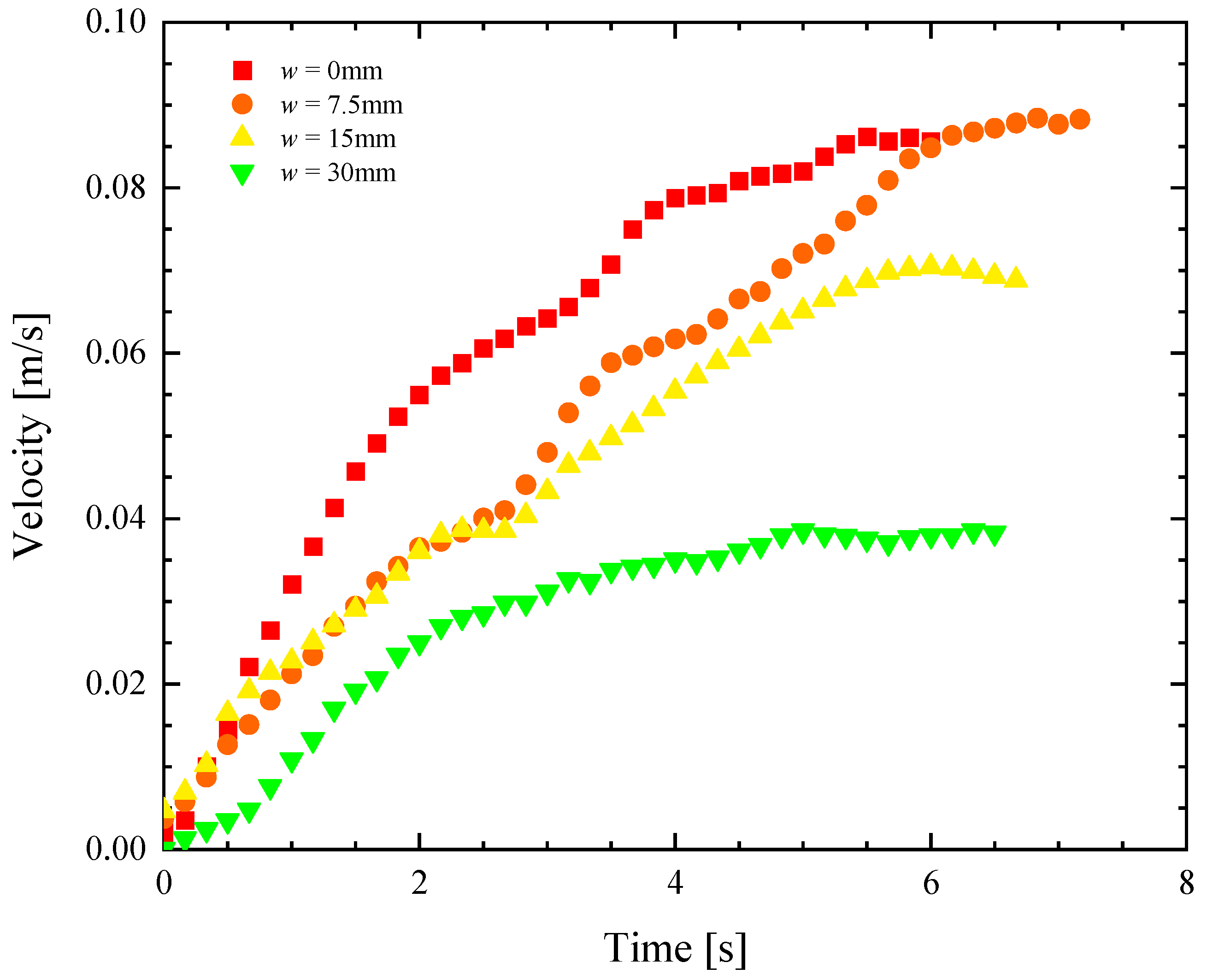
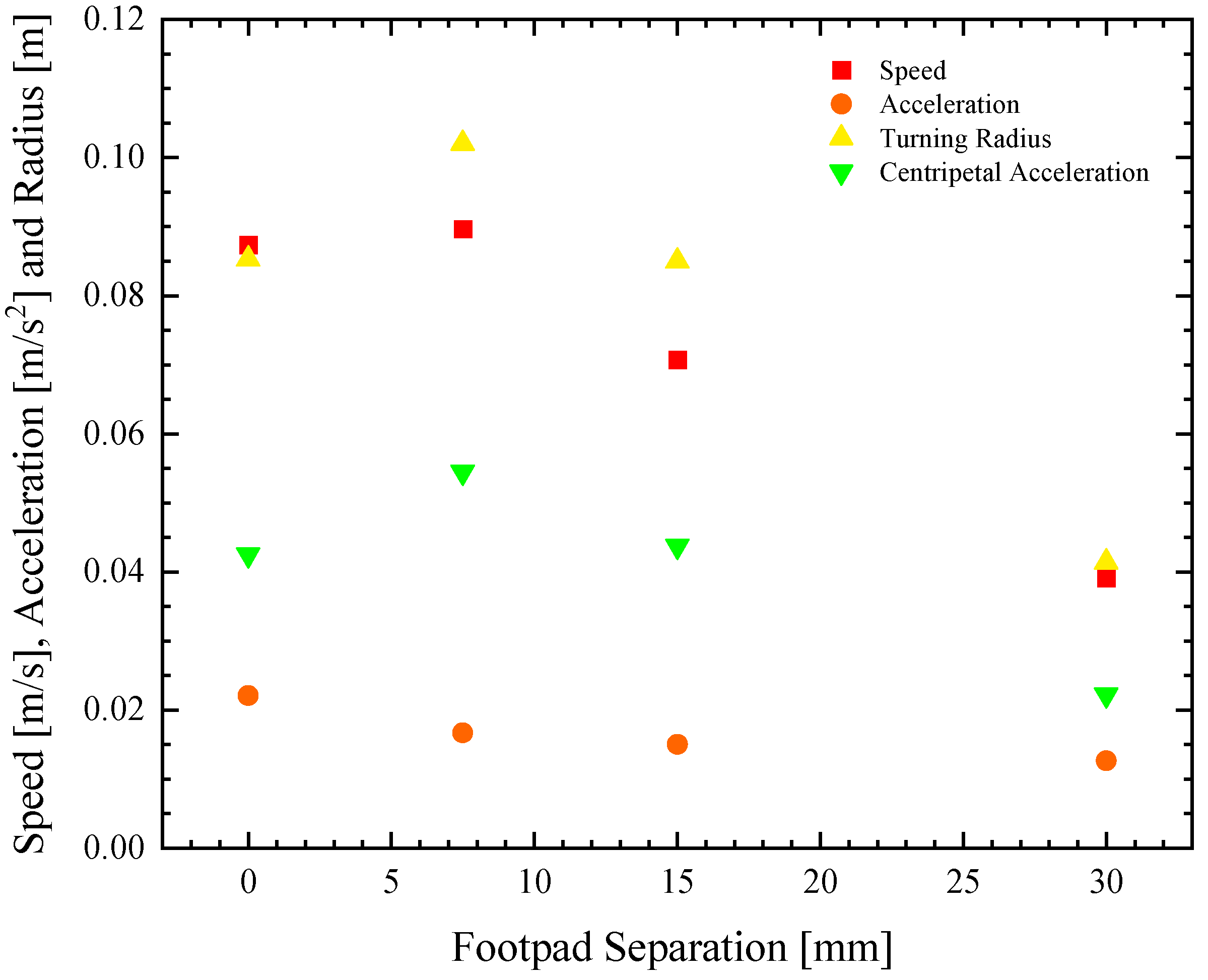
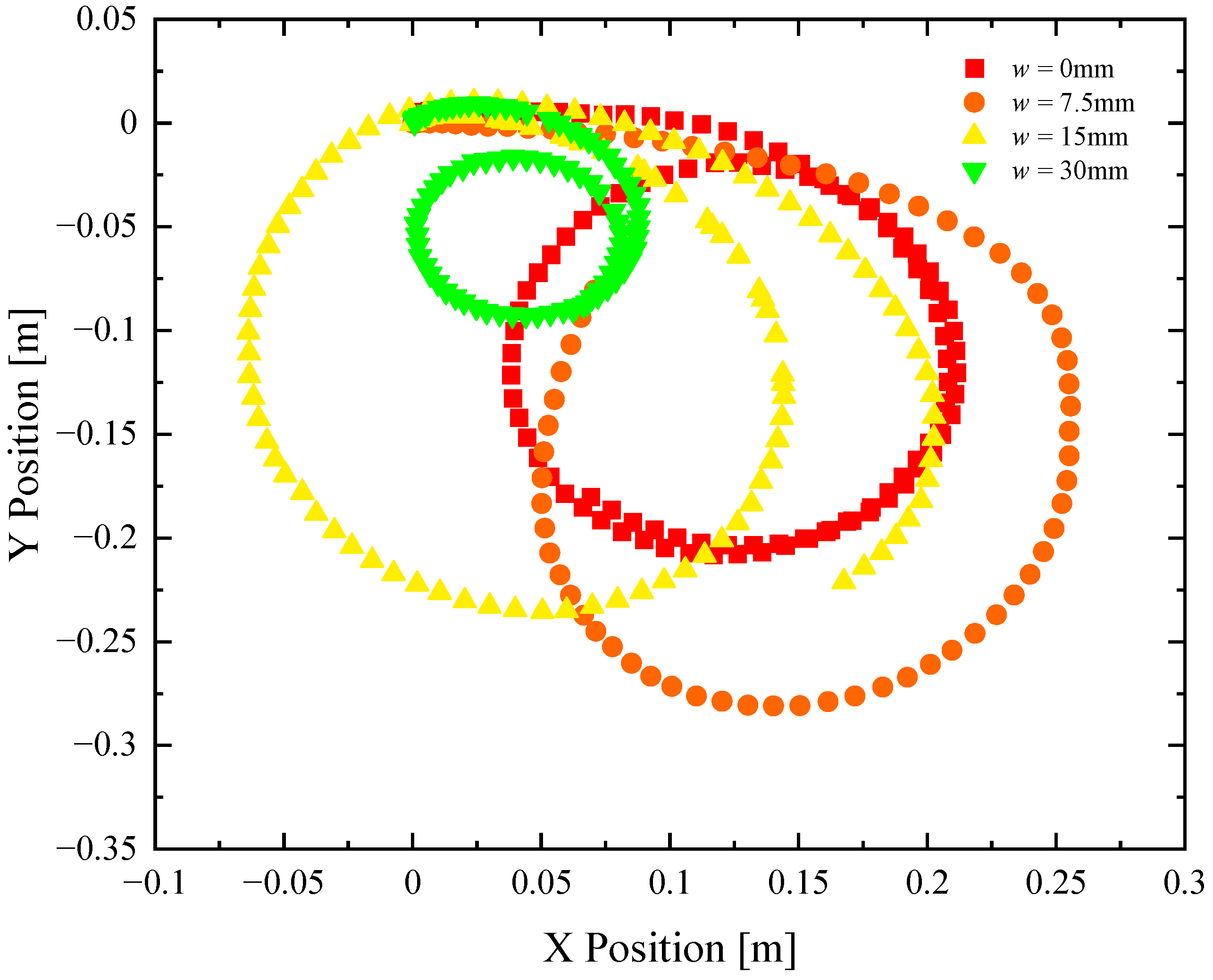
4. Results and Discussion
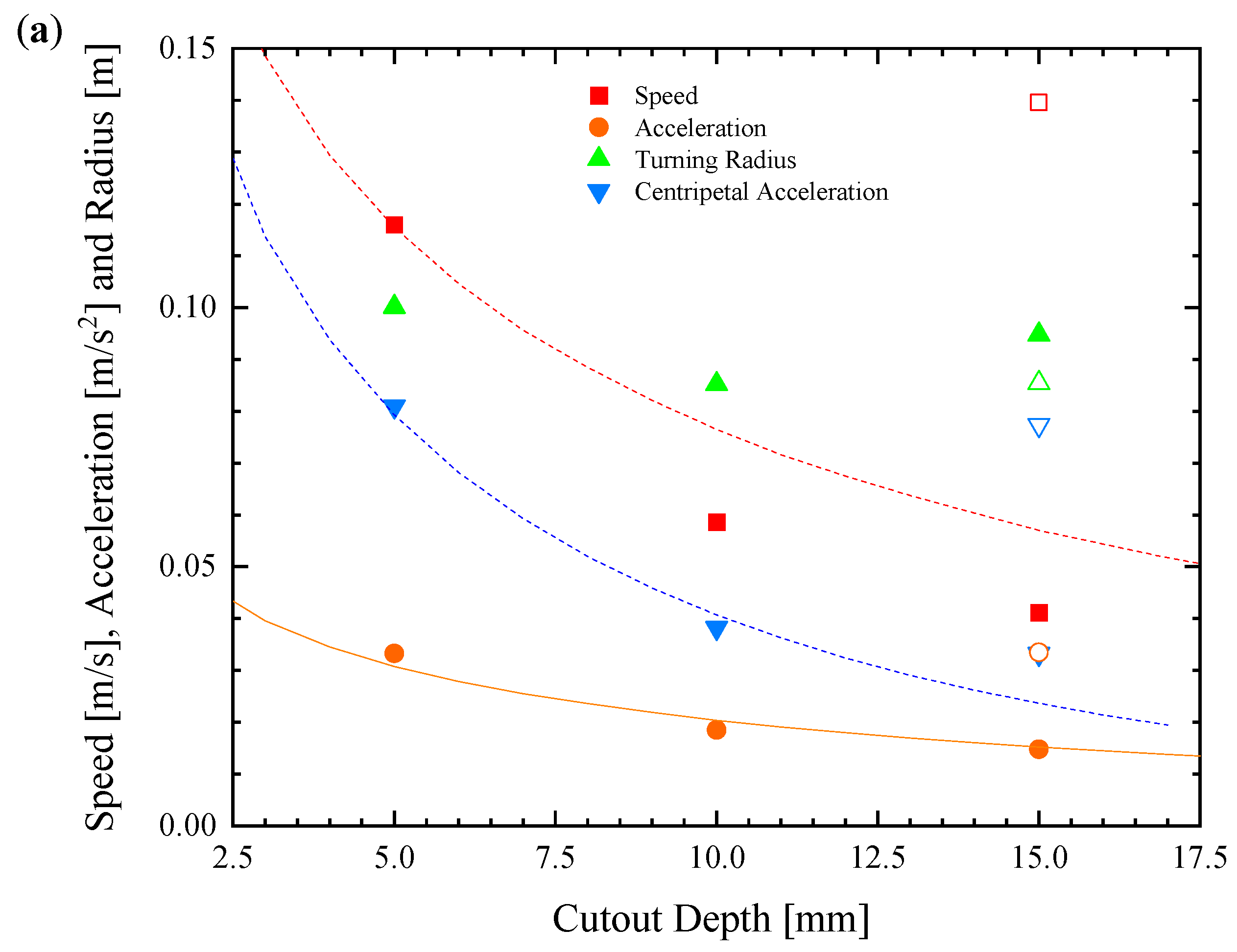
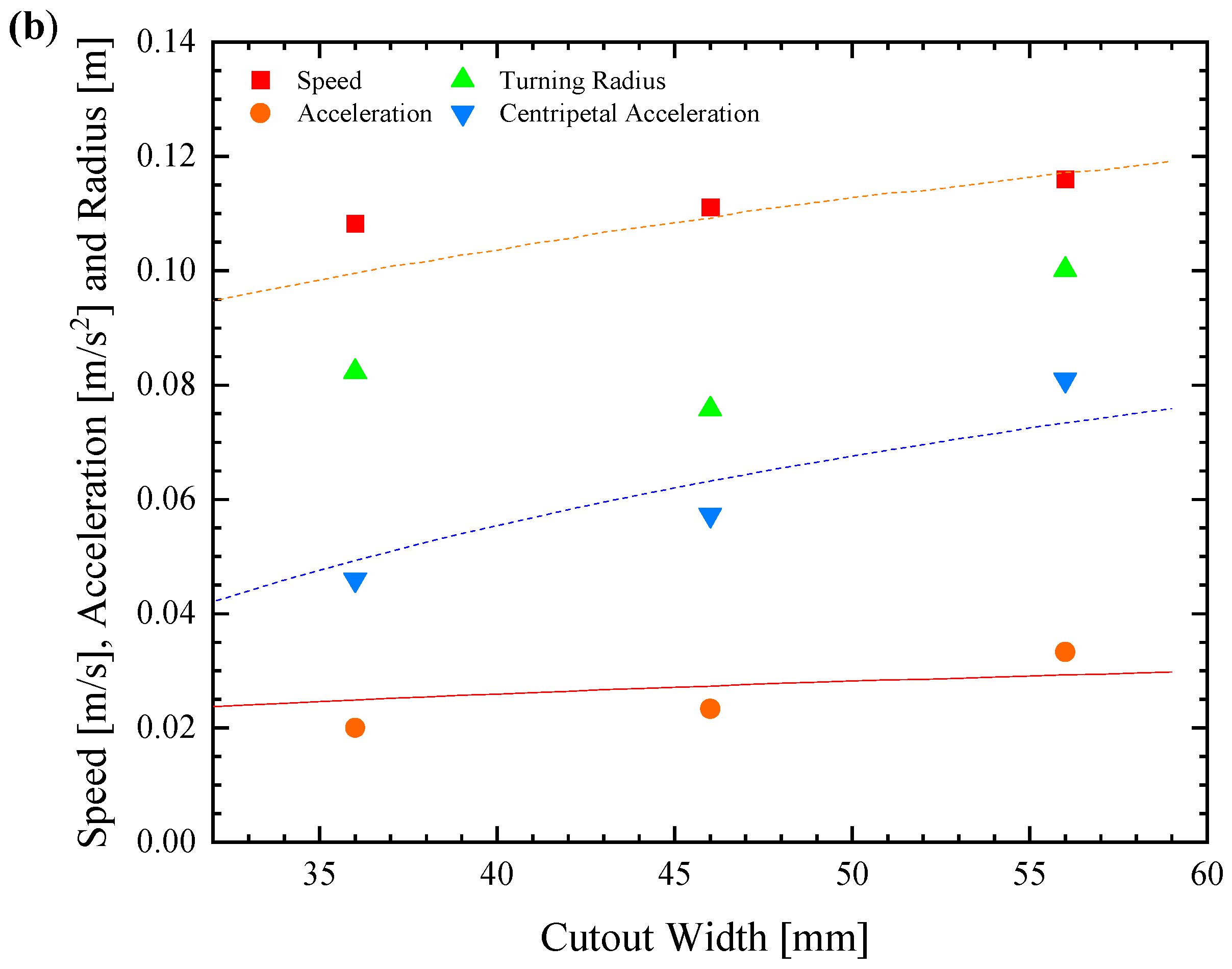
4.1. Two-Footpad Design
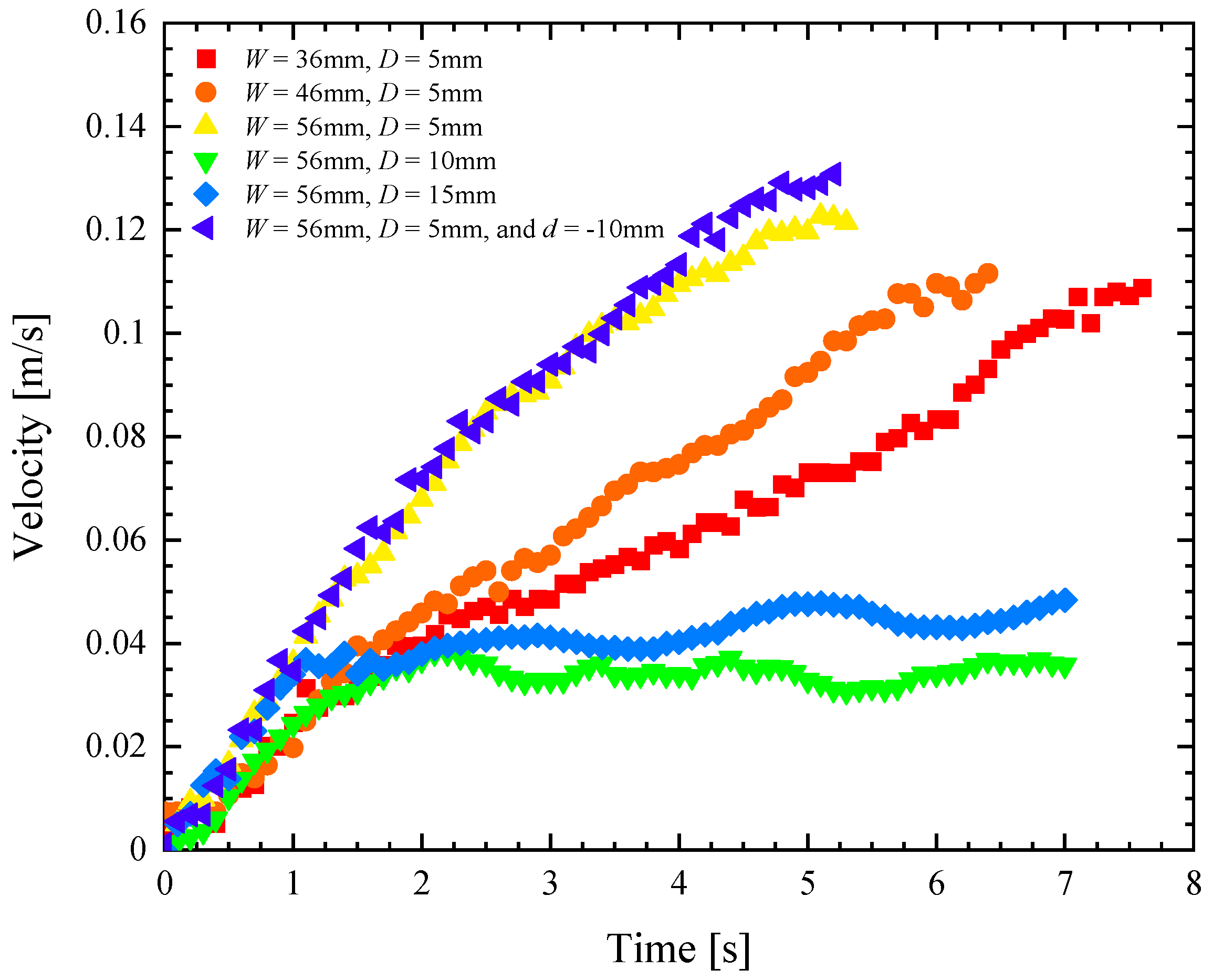
4.2. Single Footpad Design
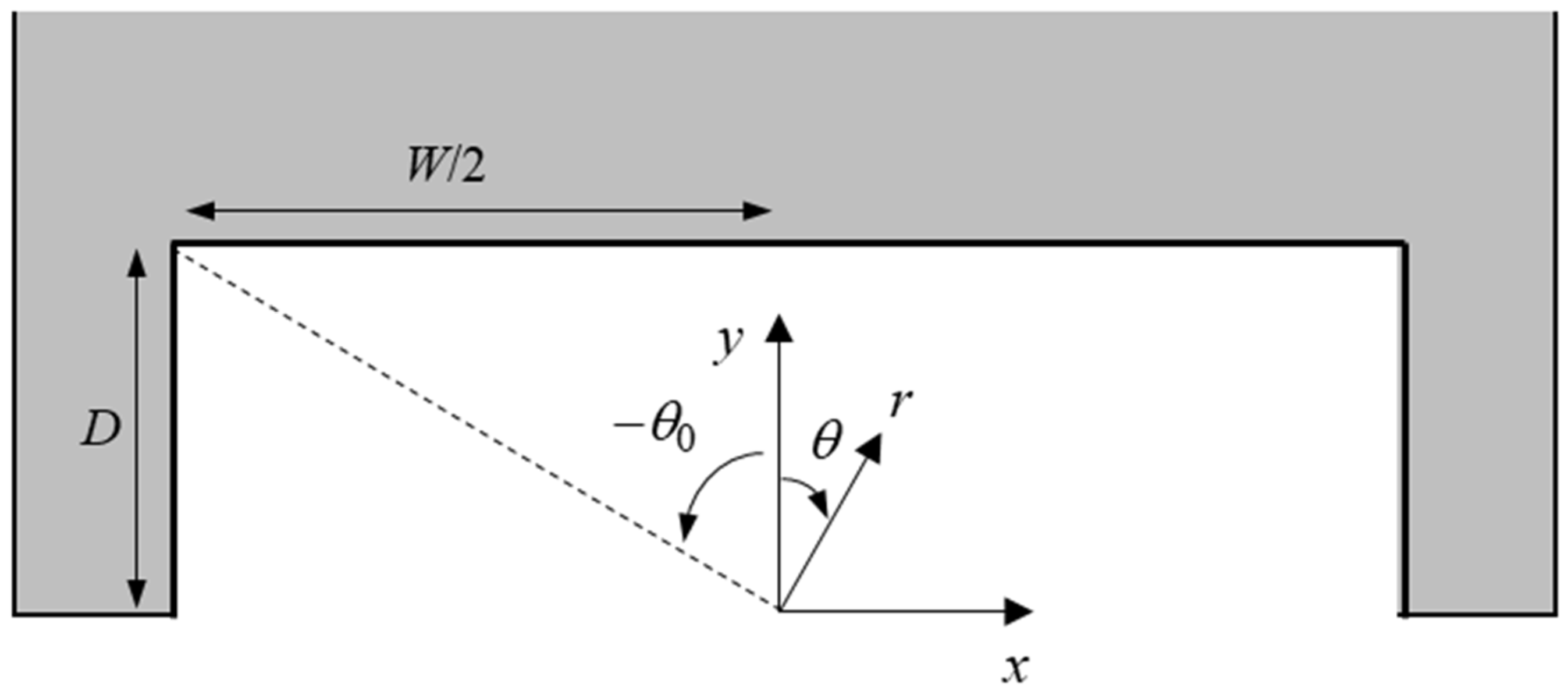
4.3. Theory
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Miyamoto, S. On a special mode of locomotion utilizing surface tension at the water-edge in some semiaquatic insects. Kontyu 1955, 23, 45–52. [Google Scholar]
- Nachtigall, W. Locomotion: Mechanics and hydrodynamics of swimming in aquatic insects. In The Physiology of Insecta; Academic Press: New York, NY, USA, 1974; pp. 381–432. [Google Scholar]
- Andersen, N.M. A comparative study of locomotion on the water surface in semiaquatic bugs (Insects, Hemiptera, Gerromorpha). Vidensk. Meddr. Dan. Naturhist. Foren. 1976, 139, 337–396. [Google Scholar]
- Suter, R.B.; Rosenberg, R.B.; Loeb, S.; Wildman, H.; Long, J. Locomotion on the water surface: Propulsive mechanisms of the fisher spider dolomedes triton. J. Exp. Biol. 1997, 200, 2523–2538. [Google Scholar] [CrossRef] [PubMed]
- Bowdan, E. Walking and rowing in the water strider, gerris remigis. J. Comp. Physiol. 1978, 123, 43–49. [Google Scholar] [CrossRef]
- Suter, R.B.; Wildman, H. Locomotion on the water surface: Hydrodynamic constraints on rowing velocity require a gait changer. J. Exp. Biol. 1999, 202, 2771–2785. [Google Scholar] [CrossRef]
- Betz, O. Performance and adaptive value of tarsal morphology in rove beetles of the genus Stenus (Coleoptera, Staphylinidae). J. Exp. Biol. 2002, 205, 1097–1113. [Google Scholar] [CrossRef]
- Stratton, G.E.; Suter, R.B.; Miller, P.R. Evolution of water surface locomotion by spiders: A comparative approach. Biol. J. Linn. Soc. 2004, 81, 63–78. [Google Scholar] [CrossRef]
- Bush, J.W.M.; Hu, D.L. Walking on Walking on Water: Biolocomotion at the Interface. Annu. Rev. Fluid Mech. 2006, 38, 339–369. [Google Scholar] [CrossRef]
- Hu, D.L.; Bush, J.W. The hydrodynamics of water-walking arthropods. J. Fluid Mech. 2010, 644, 5–33. [Google Scholar] [CrossRef]
- Lang, C.; Seifert, K.; Dettner, K. Skimming Skimming behaviour and spreading potential of Stenus species and Dianous coerulescens (coleoptera: Staphylinidae). Naturwissenschaften 2012, 99, 937–947. [Google Scholar] [CrossRef]
- Hu, D.L.; Chan, B.; Bush, J.W.M. The hydrodynamics of water strider locomotion. Nature 2003, 424, 663–666. [Google Scholar] [CrossRef] [PubMed]
- Kwak, B.; Bae, J. Locomotion of arthropods in aquatic environment and their applications in robotics. Bioinspir. Biomim. 2018, 13, 041002. [Google Scholar] [CrossRef] [PubMed]
- Bush, J.W.M.; Hu, D.L.; Prakash, M. The Integument of Water-Walking Arthropods: Form and Function. Adv. Insect Physiol. 2008, 34, 117–192. [Google Scholar]
- Rayleigh, L. Measurements of the amount of oil necessary in order to check the motions of camphor upon water. Proc. R. Soc. Lond. 1889, 47, 364–367. [Google Scholar]
- Masoud, H.; Stone, H.A. A reciprocal theorem for Marangoni propulsion. J. Fluid Mech. 2014, 741, R4. [Google Scholar] [CrossRef]
- Kang, S.J.; Sur, S.; Rothstein, J.P.; Masoud, H. Forward, reverse, and no motion of Marangoni surfers under confinement. Phys. Rev. Fluids 2020, 5, 084004. [Google Scholar] [CrossRef]
- Vandadi, V.; Kang, S.J.; Masoud, H. Reverse Marangoni surfing. J. Fluid Mech. 2017, 811, 612–621. [Google Scholar] [CrossRef]
- Sur, S.; Masoud, H.; Rothstein, J.P. Translational and Rotational Motion of Disk-Shaped Marangoni Surfers. Phys. Fluids 2019, 31, 102101. [Google Scholar] [CrossRef]
- Sur, S.; Uvanovic, N.; Masoud, H.; Rothstein, J.P. The effect of shape on the motion and stability of Marangoni surfers. J. Fluids Eng. 2021, 143, 011301. [Google Scholar] [CrossRef]
- Hu, D.L.; Prakash, M.; Chan, B.; Bush, J.W.M. Water-walking devices. In Animal Locomotion; Taylor, G.K., Triantafyllou, M.S., Tropea, C., Eds.; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Song, Y.S.; Sitti, M. Surface-tension-driven biologically inspired water strider robots: Theory and experiments. IEEE Trans. Robot. 2007, 23, 578–589. [Google Scholar] [CrossRef]
- Zhao, J.; Zhang, X.; Pan, Q. A water walking robot inspired by water strider. In Proceedings of the IEEE International Conference on Mechatronics and Automation, Chengdu, China, 5–8 August 2012; pp. 962–967. [Google Scholar]
- Ozcan, O.; Wang, H.; Taylor, J.D.; Sitti, M. STRIDE II: A water strider-inspired miniature robot with circular footpads. Int. J. Adv. Robot. Syst. 2014, 11, 85. [Google Scholar] [CrossRef]
- Sun, J.; Li, X.; Song, J.; Huang, L.; Liu, X.; Liu, J.; Zhang, Z.; Zhao, C. Water strider-inspired design of a water walking robot using superhydrophobic al surface. J. Dispers. Sci. Technol. 2018, 39, 1840–1847. [Google Scholar] [CrossRef]
- Burton, L.J.; Cheng, N.; Bush, J.W. The cocktail boat. Integr. Comp. Biol. 2014, 54, 969–973. [Google Scholar] [CrossRef]
- Musin, A.; Grynyov, R.; Frenkel, M.; Bormashenko, E. Self-propulsion of a metallic superoleophobic micro-boat. J. Colloid Interface Sci. 2016, 479, 182–188. [Google Scholar] [CrossRef] [PubMed]
- Akella, V.S.; Singh, D.K.; Mandre, S.; Bandi, M.M. Dynamics of a camphoric acid boat at the air–water interface. Phys. Lett. A 2018, 382, 1176–1180. [Google Scholar] [CrossRef]
- Zhang, L.; Yuan, Y.; Qiu, X.; Zhang, T.; Chen, Q.; Huang, X. Marangoni effect-driven motion of miniature robots and generation of electricity on water. Langmuir 2017, 33, 12609–12615. [Google Scholar] [CrossRef] [PubMed]
- Kwak, B.; Bae, J. Skimming and steering of a non-tethered miniature robot on the water surface using Marangoni propulsion. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; pp. 3217–3222. [Google Scholar]
- Kwak, B.; Choi, S.; Bae, J. Directional motion on water surface with keel extruded footpads propelled by Marangoni effect. IEEE Robot. Autom. Lett. 2020, 54, 6829–6836. [Google Scholar] [CrossRef]
- Kwak, B.; Lee, D.; Bae, J. Flexural joints for improved linear motion of a Marangoni propulsion robot: Design and experiment. In Proceedings of the 7th IEEE International Conference on Biomedical Robotics and Biomechatronics (Biorob), Enschede, The Netherlands, 26–29 August 2018; pp. 1321–1326. [Google Scholar]
- Renney, C.; Brewer, A.; Mooibroek, T.J. Easy demonstration of the Marangoni effect by prolonged and directional motion: “Soap boat 2.0”. J. Chem. Educ. 2013, 90, 1353–1357. [Google Scholar] [CrossRef]
- Basualdo, F.N.P.; Bolopion, A.; Gauthier, M.; Lambert, P. A microrobotic platform actuated by thermocapillary flows for manipulation at the air-water interface. Sci. Robot. 2021, 6, eabd3557. [Google Scholar] [CrossRef]
- Choi, Y.; Park, C.; Lee, A.C.; Bae, J.; Kim, H.; Choi, H.; Jeong, Y.; Choi, J.; Lee, H.; Kwon, S. Photopatterned microswimmers with programmable motion without external stimuli. Nat. Commun. 2021, 12, 4724. [Google Scholar] [CrossRef]
- Luo, C.; Li, H.; Qiao, L.; Liu, X. Development of surface tension-driven microboats and microflotillas. Microsyst. Technol. 2012, 18, 1525–1541. [Google Scholar] [CrossRef]
- Yoshida, K.; Onoe, H. Marangoni-Propulsion Micro-Robots Integrated with a Wireless Photonic Colloidal Crystal Hydrogel Sensor forExploring the Aquatic Environment. Adv. Intell. Syst. 2022, 4, 2100248. [Google Scholar] [CrossRef]
- Lin, C.-H.; Kinane, C.; Zhang, Z.; Pena-Francesch, A. Functionalchemicalmotorcoatingsfor modularpoweringof self-propelled particles. ACS Appl. Mater. Interfaces 2022, 14, 39332–39342. [Google Scholar] [CrossRef] [PubMed]
- Timm, M.L.; Jafari Kang, S.; Rothstein, J.P.; Masoud, H. A remotely controlled Marangoni surfer. Bioinspir. Biomim. 2021, 16, 066014. [Google Scholar] [CrossRef]
- Maggi, C.; Saglimbeni, F.; Dipalo, M.; De Angelis, F.; Di Leonardo, R. Micromotors with asymmetric shape that efficiently convert light into work by thermocapillary effects. Nat. Commun. 2015, 6, 7855. [Google Scholar] [CrossRef]
- Kwak, B.; Choi, S.; Maeng, J.; Bae, J. Marangoni effect inspired robotic self-propulsion over a water surface using a flow-imbibition-powered microfluidic pump. Sci. Rep. 2021, 11, 17469. [Google Scholar] [CrossRef]
- Gabrielli, M.G.; von Karman, T. What price speed? J. Am. Soc. Nav. Eng. 2009, 63, 188–200. [Google Scholar]
- White, F.M. Viscous Fluid Flow, 2nd ed.; McGraw-Hill: New York, NY, USA, 1991; pp. xxi, 614. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bechard, S.; Timm, M.L.; Masoud, H.; Rothstein, J.P. Using Footpad Sculpturing to Enhance the Maneuverability and Speed of a Robotic Marangoni Surfer. Biomimetics 2023, 8, 440. https://doi.org/10.3390/biomimetics8050440
Bechard S, Timm ML, Masoud H, Rothstein JP. Using Footpad Sculpturing to Enhance the Maneuverability and Speed of a Robotic Marangoni Surfer. Biomimetics. 2023; 8(5):440. https://doi.org/10.3390/biomimetics8050440
Chicago/Turabian StyleBechard, Samuel, Mitchel L. Timm, Hassan Masoud, and Jonathan P. Rothstein. 2023. "Using Footpad Sculpturing to Enhance the Maneuverability and Speed of a Robotic Marangoni Surfer" Biomimetics 8, no. 5: 440. https://doi.org/10.3390/biomimetics8050440
APA StyleBechard, S., Timm, M. L., Masoud, H., & Rothstein, J. P. (2023). Using Footpad Sculpturing to Enhance the Maneuverability and Speed of a Robotic Marangoni Surfer. Biomimetics, 8(5), 440. https://doi.org/10.3390/biomimetics8050440





