Controlling a One-Legged Robot to Clear Obstacles by Combining the SLIP Model with Air Trajectory Planning
Abstract
1. Introduction
2. One-Legged Robot
2.1. Structural Design
2.2. Kinematic Model
3. Foot Trajectory Planning Based on the Bézier Curve
4. Jumping Control Based on SLIP Model
4.1. Jumping Height Control
4.2. Horizontal Speed Control
4.3. Body Attitude Control
4.4. Gravity Compensation
4.5. Finite-State Machine
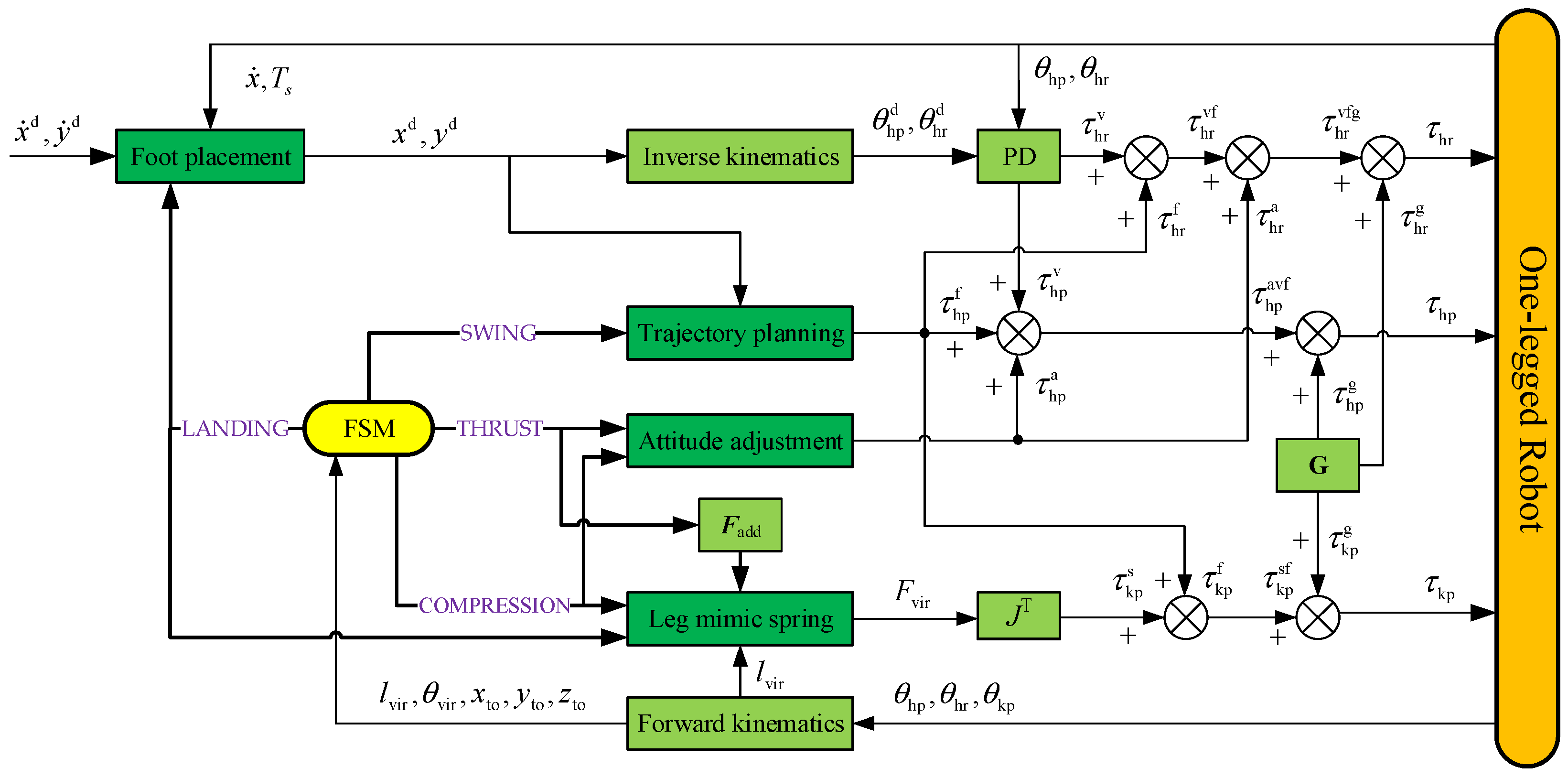
4.6. Controller of One-Legged Hopping Robot
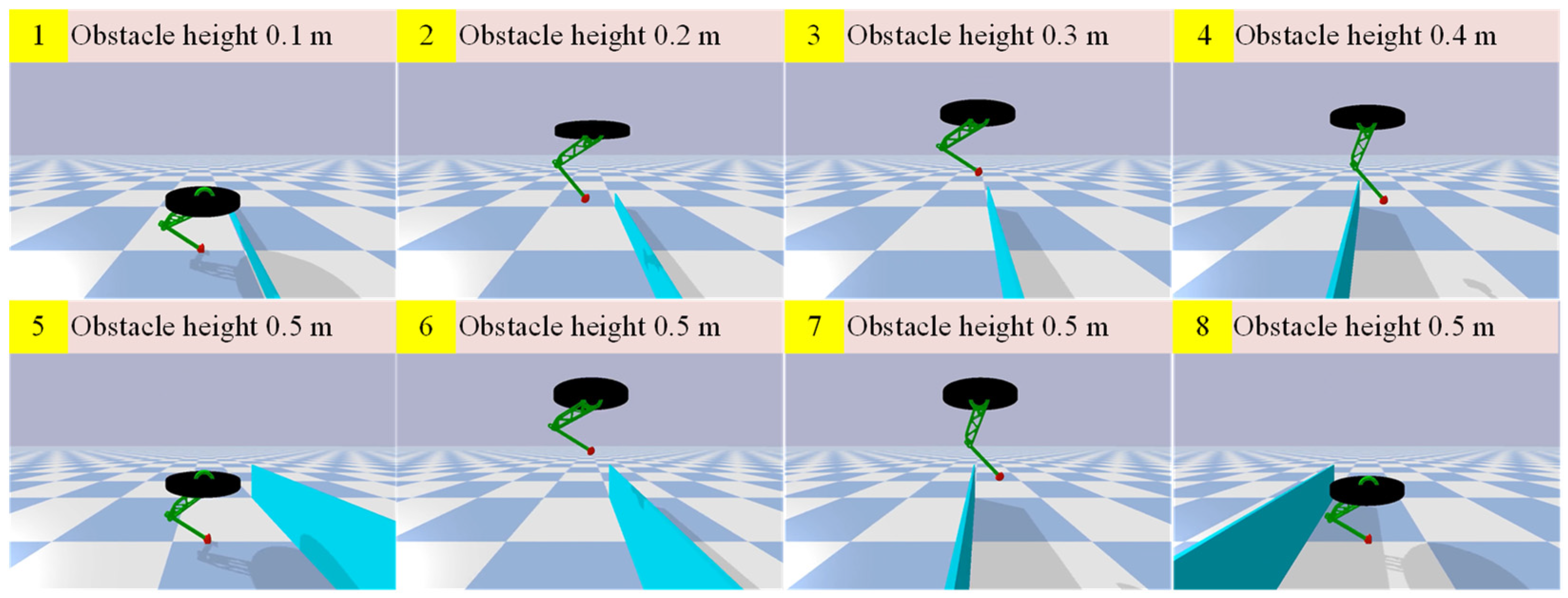
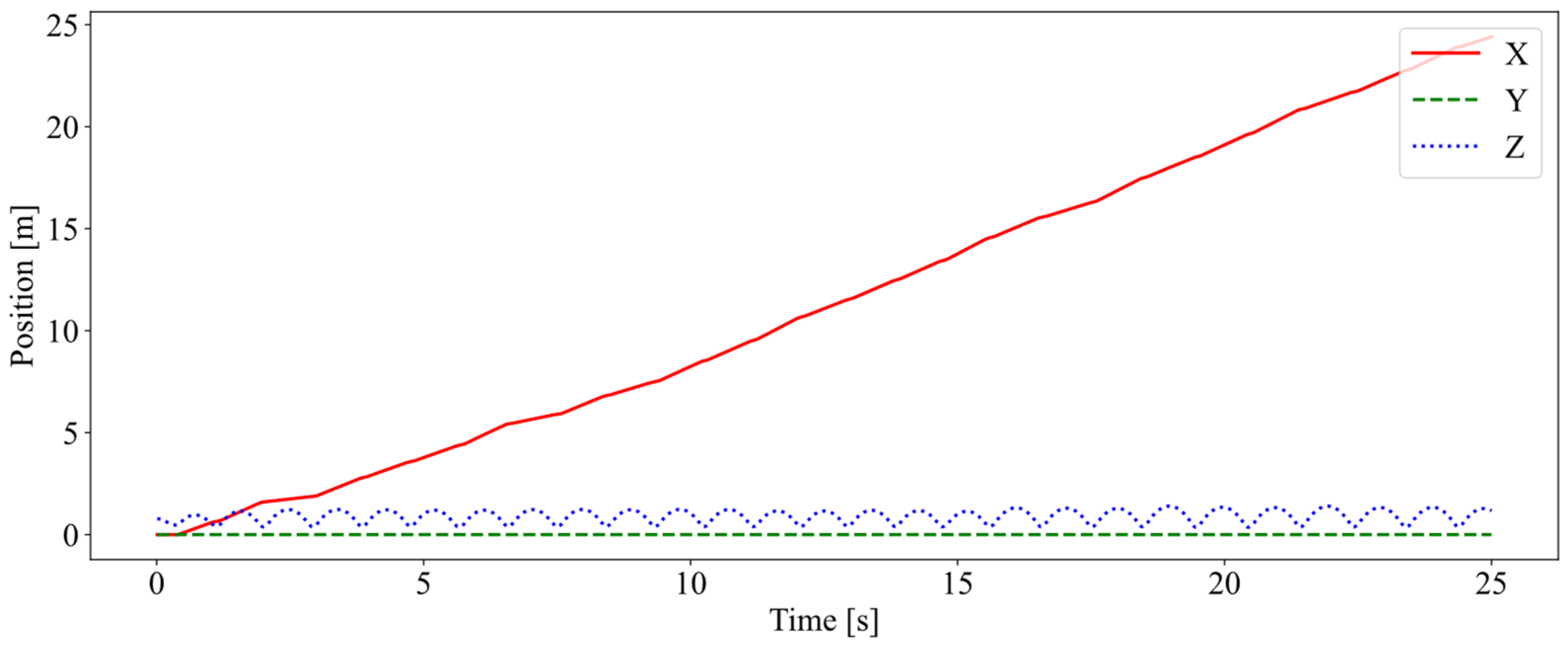
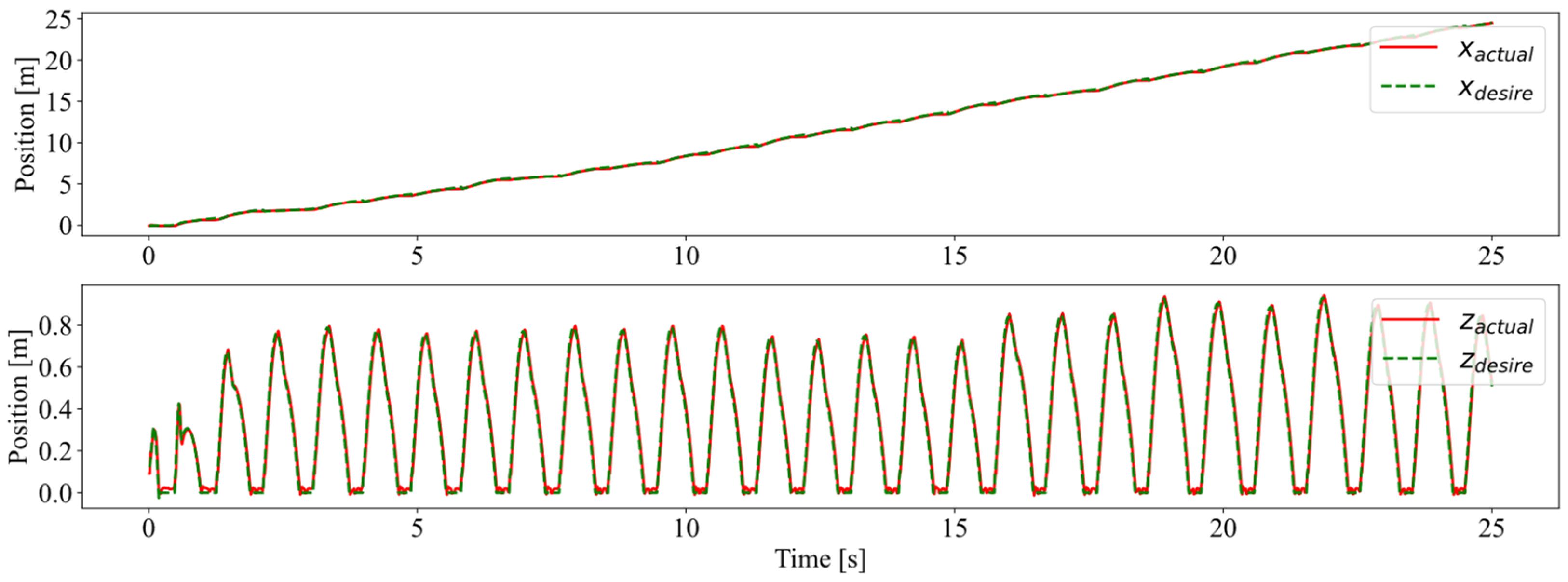
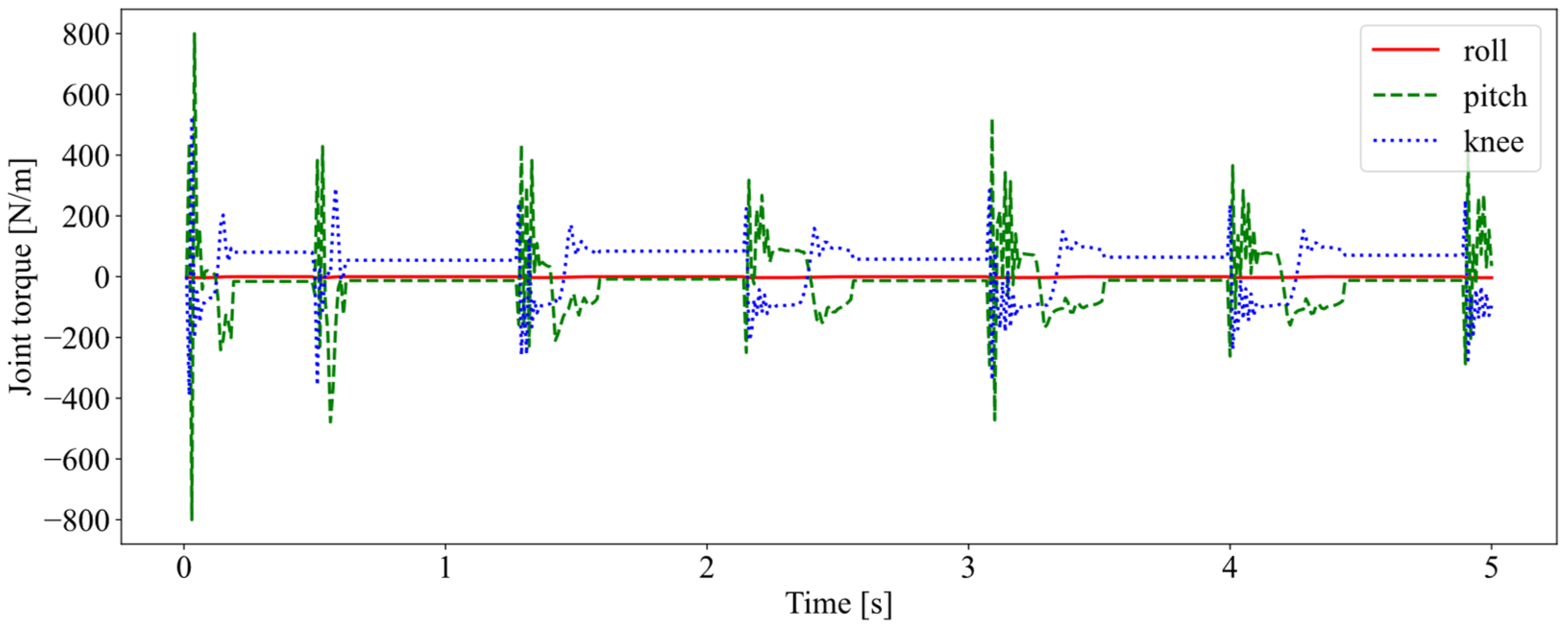
5. Jump Simulation Experiment
6. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Miki, T.; Lee, J.; Hwangbo, J.; Wellhausen, L.; Koltun, V.; Hutter, M. Learning robust perceptive locomotion for quadrupedal robots in the wild. Sci. Robot. 2022, 62, eabk2822. [Google Scholar] [CrossRef] [PubMed]
- Jeon, S.H.; Kim, S.; Kim, D. Online Optimal Landing Control of the MIT Mini Cheetah. In Proceedings of the 2022 IEEE International Conference on Robotics and Automation (ICRA), Philadelphia, PA, USA, 23–27 May 2022; pp. 178–184. [Google Scholar]
- Rathod, N.; Bratta, A.; Focchi, M.; Zanon, M.; Villarreal, O.; Semini, C.; Bemporad, A. Model Predictive Control with Environment Adaptation for Legged Locomotion. IEEE Access 2021, 9, 145710–145727. [Google Scholar] [CrossRef]
- Saputra, A.A.; Botzheim, J.; Ijspeert, A.J.; Kubota, N. Combining Reflexes and External Sensory Information in a Neuromusculoskeletal Model to Control a Quadruped Robot. IEEE Trans. Cybern. 2022, 52, 7981–7994. [Google Scholar] [CrossRef] [PubMed]
- ATLAS. Available online: https://www.bostondynamics.com/atlas (accessed on 22 November 2022).
- Luca, A.D.; Muratore, L.; Tsagarakis, N.G. A Hybrid Primitive-Based Navigation Planner for the Wheeled-Legged Robot CENTAURO. In Proceedings of the 2022 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Kyoto, Japan, 23–27 October 2022; pp. 1–8. [Google Scholar]
- Dao, J.; Green, K.; Duan, H.; Fern, A.; Hurst, J. Sim-to-Real Learning for Bipedal Locomotion Under Unsensed Dynamic Loads. In Proceedings of the 2022 IEEE International Conference on Robotics and Automation (ICRA), Philadelphia, PA, USA, 23–27 May 2022; pp. 10449–10455. [Google Scholar]
- Huang, Q.; Dong, C.; Yu, Z.; Chen, X.; Li, Q.; Chen, H.; Liu, H. Resistant Compliance Control for Biped Robot Inspired by Humanlike Behavior. IEEE ASME Trans. Mechatron. 2022, 27, 3463–3473. [Google Scholar] [CrossRef]
- Yang, C.; Yuan, K.; Zhu, Q.; Yu, W.; Li, Z. Multi-expert learning of adaptive legged locomotion. Sci. Robot. 2022, 5, eabb2174. [Google Scholar] [CrossRef]
- Chi, W.; Jiang, X.; Zheng, Y. A Linearization of Centroidal Dynamics for the Model-Predictive Control of Quadruped Robots. In Proceedings of the 2022 IEEE International Conference on Robotics and Automation (ICRA), Philadelphia, PA, USA, 23–27 May 2022; pp. 4656–4663. [Google Scholar]
- Raibert, M.H. Legged Robots That Balance; MIT Press: Cambridge, MA, USA, 1986. [Google Scholar]
- Weng, Y.; Chen, X.; Yu, Z.; Qi, H.; Ma, X.; Zhu, M.; Huang, Q. Leg Mechanism Design of a Jumping Robot with Variable Reduction Ratio Joint. In Proceedings of the 2022 International Conference on Intelligent Robotics and Applications, Harbin, China, 1–3 August 2022; pp. 250–261. [Google Scholar]
- Yim, J.K.; Fearing, R.S. Precision Jumping Limits from Flight-phase Control in Salto -1P. In Proceedings of the 2018 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Madrid, Spain, 1–5 October 2018; pp. 2229–2236. [Google Scholar]
- He, Z.; Meng, F.; Chen, X.; Yu, Z.; Fan, X.; Sato, R.; Huang, Q. Controllable height hopping of a parallel legged robot. Appl. Sci. 2021, 11, 1421. [Google Scholar] [CrossRef]
- Chen, Z.; Jin, B.; Zhu, S.; Pang, Y.; Chen, G. Dynamic Hopping Height Control of Single-Legged Hopping Robot. Wearable Sens. Robot. 2017, 399, 365–382. [Google Scholar]
- Kalouche, S. GOAT: A legged robot with 3D agility and virtual compliance. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; pp. 4110–4117. [Google Scholar]
- Ugurlu, B.; Kawamura, A. ZMP-based online jumping pattern generation for a one-legged robot. IEEE Trans. Ind. Electron. 2010, 57, 1701–1709. [Google Scholar] [CrossRef]
- Jiang, X.; Chen, X.; Yu, Z.; Zhang, W.; Meng, L.; Huang, Q. Motion planning for bipedal robot to perform jump maneuver. Appl. Sci. 2018, 8, 139. [Google Scholar] [CrossRef]
- Park, H.W.; Wensing, P.M.; Kim, S. Online planning for autonomous running jumps over obstacles in high-speed quadrupeds. In Proceedings of the 2015 Robotics: Science and Systems Conference (RSS), Rome, Italy, 13–17 July 2015; pp. 1–9. [Google Scholar]
- Nguyen, C.; Bao, L.; Nguyen, Q. Continuous Jumping for Legged Robots on Stepping Stones via Trajectory Optimization and Model Predictive Control. arXiv 2022, arXiv:2204.01147. [Google Scholar]
- Chignoli, M.; Kim, D.; Stanger-Jones, E.; Kim, S. The MIT humanoid robot: Design, motion planning, and control for acrobatic behaviors. In Proceedings of the 2020 IEEE-RAS 20th International Conference on Humanoid Robots (Humanoids), Munich, Germany, 19–21 July 2021; pp. 1–8. [Google Scholar]
- Chignoli, M.; Morozov, S.; Kim, S. Rapid and reliable quadruped motion planning with omnidirectional jumping. In Proceedings of the 2022 IEEE International Conference on Robotics and Automation (ICRA), Philadelphia, PA, USA, 23–27 May 2022; pp. 6621–6627. [Google Scholar]
- Chignoli, M. Trajectory Optimization for Dynamic Aerial Motions of Legged Robots. Master’s Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2021. [Google Scholar]
- Song, Z.; Yue, L.; Sun, G.; Ling, Y.; Wei, H.; Gui, L.; Liu, Y.H. An Optimal Motion Planning Framework for Quadruped Jumping. arXiv 2022, arXiv:2207.12002. [Google Scholar]
- Nguyen, C.; Nguyen, Q. Contact-timing and Trajectory Optimization for 3D Jumping on Quadruped Robots. arXiv 2022, arXiv:2110.06764. [Google Scholar]
- Nguyen, Q.; Powell, M.J.; Katz, B.; Carlo, J.D.; Kim, S. Optimized Jumping on the MIT Cheetah 3 Robot. In Proceedings of the 2019 IEEE International Conference on Robotics and Automation (ICRA), Montreal, QC, Canada, 20–24 May 2019; pp. 7448–7454. [Google Scholar]
- Bellegarda, G.; Nguyen, Q. Robust quadruped jumping via deep reinforcement learning. arXiv 2020, arXiv:2011.07089. [Google Scholar]
- Campanaro, L.; Gangapurwala, S.; Martini, D.D.; Merkt, W.; Havoutis, I. CPG-ACTOR: Reinforcement Learning for Central Pattern Generators. arXiv 2021, arXiv:2102.12891. [Google Scholar]
- Mo, X.; Ge, W.; Romano, D.; Donati, E.; Stefanini, C. Modelling jumping in locusta migratoria and the influence of substrate roughness. Entomol. Gen. 2019, 38, 317–332. [Google Scholar] [CrossRef]
- Fercher, C. The biomechanics of movement of horses engaged in jumping over different obstacles in competition and training. J. Equine Vet. Sci. 2017, 49, 69–80. [Google Scholar] [CrossRef]
- Alexander, R.M. Leg design and jumping technique for humans, other vertebrates and insects. Philos. Trans. R. Soc. London. Ser. B Biol. Sci. 1995, 347, 235–248. [Google Scholar]
- Gabriel, J.M. The effect of animal design on jumping performance. J. Zool. 1984, 204, 533–539. [Google Scholar] [CrossRef]
- Alexander, R.M. The mechanics of jumping by a dog (Canis familiaris). J. Zool. 1974, 173, 549–573. [Google Scholar] [CrossRef]
- Alexander, R.M.; Vernon, A. The mechanics of hopping by kangaroos (Macropodidae). J. Zool. 1975, 177, 265–303. [Google Scholar] [CrossRef]
- Burrows, M. Jumping performance of froghopper insects. J. Exp. Biol. 2006, 209, 4607–4621. [Google Scholar] [CrossRef] [PubMed]
- Mo, X.; Ge, W.; Miraglia, M.; Inglese, F.; Zhao, D.; Stefanini, C.; Romano, D. Jumping locomotion strategies: From animals to bioinspired robots. Appl. Sci. 2020, 10, 8607. [Google Scholar] [CrossRef]
- Pfau, T.; Rivaz, A.; Brighton, S.; Weller, R. Kinetics of jump landing in agility dogs. Vet. J. 2011, 190, 278–283. [Google Scholar] [CrossRef] [PubMed]
- Blickhan, R. The spring-mass model for running and hopping. J. Biomech. 1989, 22, 1217–1227. [Google Scholar] [CrossRef] [PubMed]
- Mo, X.; Romano, D.; Miraglia, M.; Ge, W.; Stefanini, C. Effect of substrates’ compliance on the jumping mechanism of locusta migratoria. Front. Bioeng. Biotechnol. 2020, 8, 661. [Google Scholar] [CrossRef]
- Farley, C.T.; Gonzalez, O. Leg stiffness and stride frequency in human running. J. Biomech. 1996, 29, 181–186. [Google Scholar] [CrossRef]
- Ferris, D.P.; Louie, M.; Farley, C.T. Running in the real world: Adjusting leg stiffness for different surfaces. Proc. R. Soc. Lond. 1998, 265, 989–994. [Google Scholar] [CrossRef]
- Kim, S.; Park, S. Leg stiffness increases with speed to modulate gait frequency and propulsion energy. J. Biomech. 2011, 44, 1253–1258. [Google Scholar] [CrossRef]
- Guo, W.; Cai, C.; Li, M.; Zha, F.; Wang, P.; Jiang, Z. Estimation of leg stiffness using an approximation to the planar spring–mass system in high-speed running. Int. J. Adv. Robot. Syst. 2020, 17, 1729881419890713. [Google Scholar] [CrossRef]
- Yin, P.; Wang, P.; Li, M.; Sun, L. A Novel Control Strategy for Quadruped Robot Walking Over Irregular Terrain. In Proceedings of the 2011 IEEE Conference on Robotics, Automation and Mechatronics (RAM), Qingdao, China, 17–19 September 2011; pp. 184–189. [Google Scholar]
- Kim, K.Y.; Park, J.H. Ellipse-based leg-trajectory generation for galloping quadruped robots. J. Mech. Sci. Technol. 2009, 22, 2099–2106. [Google Scholar] [CrossRef]
- Sakakibara, Y.; Kan, K.; Hosoda, Y.; Hattori, M.; Fujie, M. Foot Trajectory for a Quadruped Walking Machine. In Proceedings of the 1990 IEEE International Workshop on Intelligent Robots and Systems, Towards a New Frontier of Applications, Ibaraki, Japan, 3–6 July 1990; pp. 315–322. [Google Scholar]
- Rong, X.; Li, Y.; Ruan, J.; Li, B. Design and simulation for a hydraulic actuated quad-ruped robot. J. Mech. Sci. Technol. 2012, 26, 1171–1177. [Google Scholar] [CrossRef]
- Yuan, L.; Zhang, Z.; Ouyang, R. Trajectory Planning of Quadruped Robot Based on the Principle of Optimal Power. Int. J. Mech. Res. 2016, 5, 138–147. [Google Scholar] [CrossRef]
- Hyun, D.J.; Seok, S.; Lee, J.; Kim, S. High speed trot-running: Implementation of a hierarchical controller using proprioceptive impedance control on the MIT Cheetah. Int. J. Robot. Res. 2014, 33, 1417–1445. [Google Scholar] [CrossRef]
- Hudson, P.E.; Corr, S.A.; Wilson, A.M. High speed galloping in the cheetah (Acinonyx jubatus) and the racing greyhound (Canis familiaris): Spatio temporal and kinetic characteristics. J. Exp. Biol. 2012, 215, 2425–2434. [Google Scholar] [CrossRef]



| Parameter | Value | Definition |
|---|---|---|
| m1 | 6 kg | Femur link mass |
| m2 | 4 kg | Tibia link mass |
| l1 | 0.4 m | Femur link length |
| l2 | 0.35 m | Tibia link length |
| θ1 | / | Hip joint roll angle |
| θ2 | / | Hip joint pitch angle |
| θ3 | / | Knee joint pitch angle |
| lvir | / | Virtual leg length |
| θvir | / | Virtual leg pitch angle |
| θbr | / | Body roll angle |
| θbp | / | Body pitch angle |
| State | Trigger Event | Action |
|---|---|---|
| COMPRESSION | Foot touches ground |
|
| THRUST | Virtual leg extension |
|
| SWING | Foot not touching |
|
| LANDING | Leg stops swinging |
|
| Parameter | Symbol | Value |
|---|---|---|
| Virtual spring stiffness | 11,000 | |
| Virtual spring damping | 60 | |
| Original length of the spring | 0.675 | |
| Amplitude of added force | 2800 | |
| Speed increment coefficient | 0.01 | |
| Position gain of foot | 4000 | |
| Velocity gain of foot | 60 | |
| Proportional gain of attitude | 3000 | |
| Derivative gain of attitude | 80 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, S.; Zhang, X. Controlling a One-Legged Robot to Clear Obstacles by Combining the SLIP Model with Air Trajectory Planning. Biomimetics 2023, 8, 66. https://doi.org/10.3390/biomimetics8010066
Huang S, Zhang X. Controlling a One-Legged Robot to Clear Obstacles by Combining the SLIP Model with Air Trajectory Planning. Biomimetics. 2023; 8(1):66. https://doi.org/10.3390/biomimetics8010066
Chicago/Turabian StyleHuang, Senwei, and Xiuli Zhang. 2023. "Controlling a One-Legged Robot to Clear Obstacles by Combining the SLIP Model with Air Trajectory Planning" Biomimetics 8, no. 1: 66. https://doi.org/10.3390/biomimetics8010066
APA StyleHuang, S., & Zhang, X. (2023). Controlling a One-Legged Robot to Clear Obstacles by Combining the SLIP Model with Air Trajectory Planning. Biomimetics, 8(1), 66. https://doi.org/10.3390/biomimetics8010066






