Development of Structurally Graded Alumina–Polymer Composites as Potential Orthodontic Bracket Materials
Abstract
1. Introduction
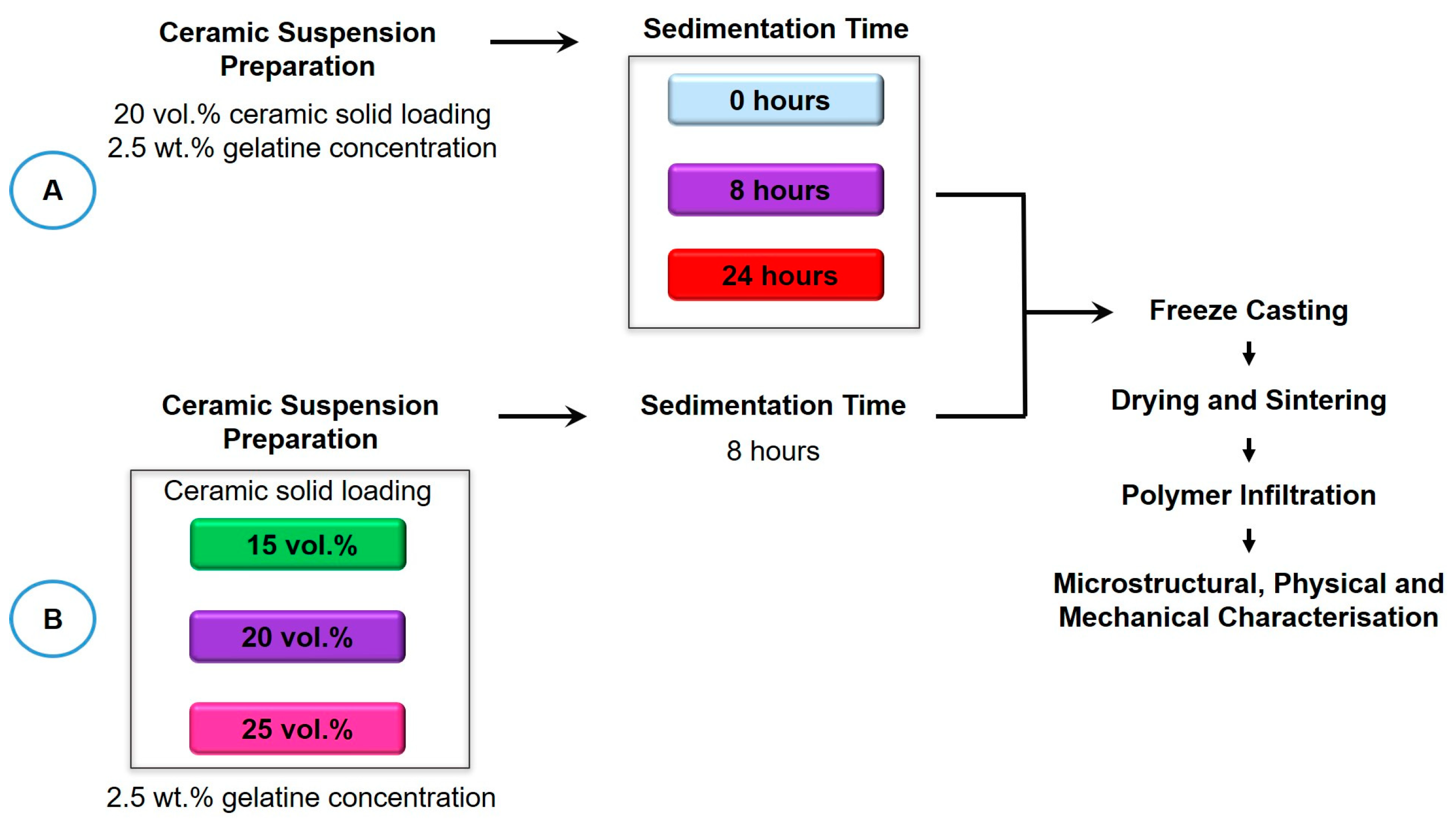
2. Materials and Methods
2.1. Preparation of Ceramic Slurries
2.2. Unidirectional Freeze Casting
2.3. Polymer Infiltration
2.4. Sample Preparation for Characterisation
2.5. Microstructural Characterisation
2.6. Vicker’s Hardness
2.7. Porosity and Ceramic Fraction Through Archimedes’ Principle
2.8. Compressive Strength and Modulus of Elasticity
2.9. Fracture Toughness
2.10. Statistical Analysis
3. Results
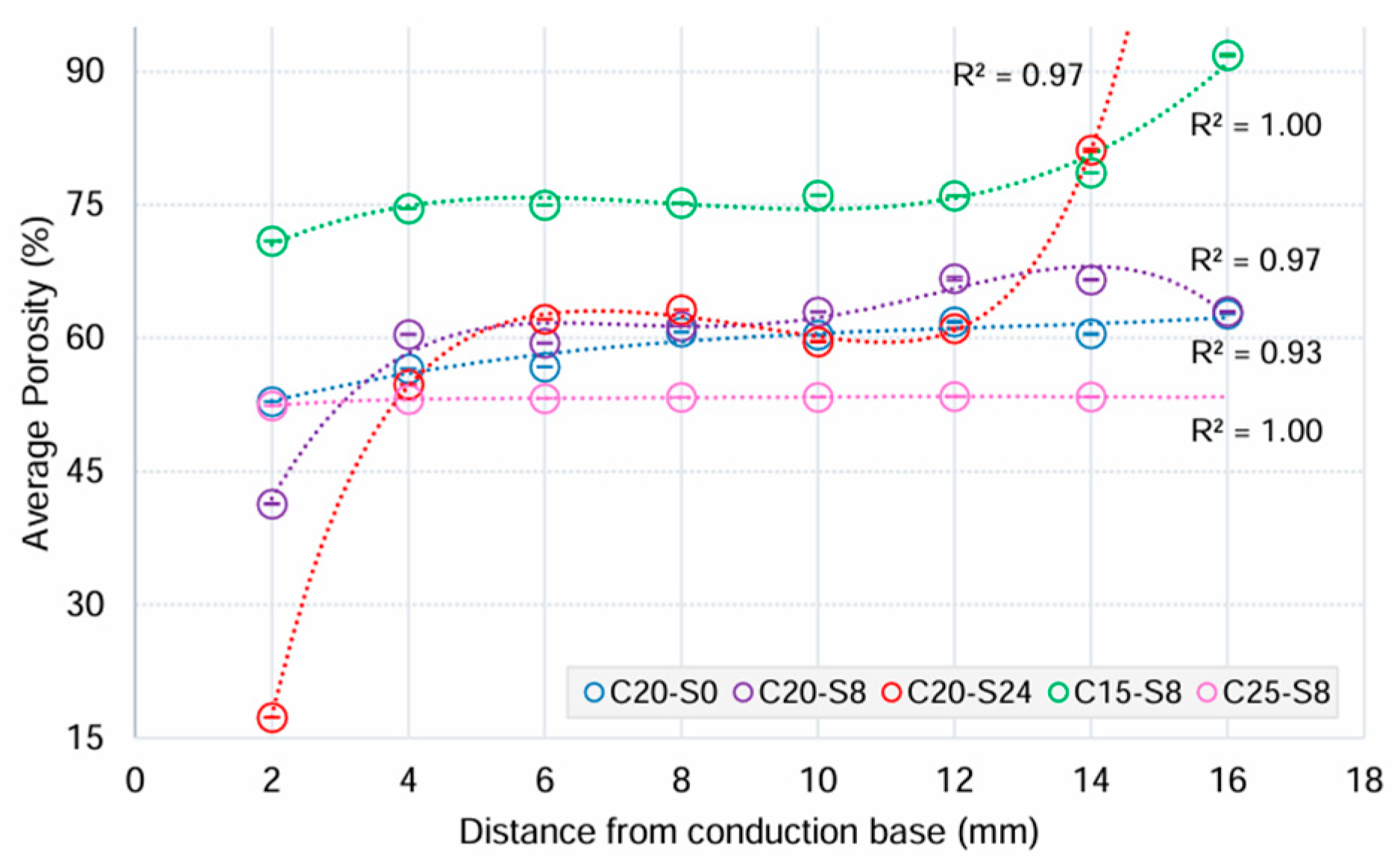
3.1. Ceramic Scaffold Microstructure
3.2. Hardness
3.3. Porosity and Ceramic Fraction Through Archimedes’ Principle
3.4. Compressive Strength
3.5. Modulus of Elasticity
3.6. Fracture Toughness
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Keim, R.G.; Gottlieb, E.L.; Vogels, D.S.; Vogels, P.B. 2017 JCO Orthodontic Practice Study. J. Clin. Orthod. 2017, 51, 639–656. [Google Scholar]
- Kim, Y. Study on the perception of orthodontic treatment according to age: A questionnaire survey. Korean J. Orthod. 2017, 47, 215. [Google Scholar] [CrossRef]
- BOS. The number of adults seeking orthodontic treatment in the UK continues to rise. Br. Dent. J. 2018, 224, 847. [Google Scholar]
- Tan, E.L.Y.; Song, Y.L.; Zhang, Z.; Yong, K.J.J.; Zhang, Y.; Yeo, X. Orthodontic treatment in National Dental Centre of Singapore: Trends toward higher proportion of adult patients. APOS Trends Orthod. 2019, 9, 89–93. [Google Scholar]
- Cedro, M.K.; Moles, D.R.; Hodges, S.J. Adult orthodontics—Who’s doing what? J. Orthodont. 2010, 37, 107–117. [Google Scholar] [CrossRef]
- Christensen, L.; Luther, F. Adults seeking orthodontic treatment: Expectations, periodontal and TMD issues. Br. Dent. J. 2015, 218, 111–117. [Google Scholar] [CrossRef]
- Thorstenson, G.; Kusy, R. Influence of stainless steel inserts on the resistance to sliding of esthetic brackets with second-order angulation in the dry and wet states. Angle Orthod. 2003, 73, 167–175. [Google Scholar] [CrossRef]
- Faltermeier, A.; Rosentritt, M.; Faltermeier, R.; Müßig, D. Influence of fibre and filler reinforcement of plastic brackets: An in vitro study. Eur. J. Orthod. 2007, 29, 304–309. [Google Scholar] [CrossRef]
- Matsui, S.; Umezaki, E.; Komazawa, D.; Otsuka, Y.; Suda, N. Evaluation of mechanical properties of esthetic brackets. J. Dent. Biomech. 2015, 6, 1758736015574401. [Google Scholar] [CrossRef]
- Aird, J.C.; Durning, P. Fracture of polycarbonate edgewise brackets: A clinical and SEM study. Br. J. Orthod. 1987, 14, 191–195. [Google Scholar] [CrossRef]
- Eliades, T. Orthodontic materials research and applications: Part 2. Current status and projected future developments in materials and biocompatibility. Am. J. Orthod. Dentofac. Orthop. 2007, 131, 253–262. [Google Scholar] [CrossRef]
- Ripley, K.T. An In Vitro Comparative Study of Shear and Tensile Bond Strengths for Stainless Steel and Ceramic Orthodontic Brackets. Ph.D. Dissertation, University of Iowa, Iowa, IA, USA, 1988. [Google Scholar]
- Viazis, A.D.; Cavanaugh, G.; Bevis, R.R. Bond strength of ceramic brackets under shear stress: An in vitro report. Am. J. Orthod. Dentofac. Orthop. 1990, 98, 214–221. [Google Scholar] [CrossRef]
- Kitahara-Céia, F.M.F.; Mucha, J.N.; dos Santos, P.A.M. Assessment of enamel damage after removal of ceramic brackets. Am. J. Orthod. Dentofac. Orthop. 2008, 134, 548–555. [Google Scholar] [CrossRef]
- Cochrane, N.J.; Lo, T.W.; Adams, G.G.; Schneider, P.M. Quantitative analysis of enamel on debonded orthodontic brackets. Am. J. Orthod. Dentofac. Orthop. 2017, 152, 312–319. [Google Scholar] [CrossRef]
- Göhring, T.N.; Gallo, L.; Lüthy, H. Effect of water storage, thermocycling, the incorporation and site of placement of glass-fibers on the flexural strength of veneering composite. Dent. Mater. 2005, 21, 761–772. [Google Scholar] [CrossRef]
- Kusy, R.P.; Whitley, J.Q. Degradation of plastic polyoxymethylene brackets and the subsequent release of toxic formaldehyde. Am. J. Orthod. Dentofac. Orthop. 2005, 127, 420–427. [Google Scholar] [CrossRef]
- Ali, O.; Makou, M.; Papadopoulos, T.; Eliades, G. Laboratory evaluation of modern plastic brackets. Eur. J. Orthod. 2012, 34, 595–602. [Google Scholar] [CrossRef]
- Zinelis, S.; Eliades, T.; Eliades, G.; Makou, M.; Silikas, N. Comparative assessment of the roughness, hardness, and wear resistance of aesthetic bracket materials. Dent. Mater. 2005, 21, 890–894. [Google Scholar] [CrossRef]
- Dobrin, R.J.; Kamel, I.L.; Musich, D.R. Load-deformation characteristics of polycarbonate orthodontic brackets. Am. J. Orthod. 1975, 67, 24–33. [Google Scholar] [CrossRef]
- Viazis, A.D.; DeLong, R.; Bevis, R.R.; Rudney, J.D.; Pintado, M.R. Enamel abrasion from ceramic orthodontic brackets under an artificial oral environment. Am. J. Orthod. Dentofac. Orthop. 1990, 98, 103–109. [Google Scholar] [CrossRef]
- Scott, G.E., Jr. Fracture Toughness and Surface Cracks—The Key to Understanding Ceramic Brackets. Angle Orthod. 1988, 58, 5–8. [Google Scholar] [CrossRef] [PubMed]
- Bishara, S.E.; Ortho, D.; Truelove, T.S. Comparisons of different debonding techniques for ceramic brackets: An in vitro study: Part I. Background and methods. Am. J. Orthod. Dentofac. Orthop. 1990, 98, 145–153. [Google Scholar] [CrossRef]
- Bordeaux, J.M.; Moore, R.N.; Bagby, M.D. Comparative evaluation of ceramic bracket base designs. Am. J. Orthod. Dentofac. Orthop. 1994, 105, 552–560. [Google Scholar] [CrossRef]
- Olsen, M.E.; Bishara, S.E.; Jakobsen, J.R. Evaluation of the shear bond strength of different ceramic bracket base designs. Angle Orthod. 1997, 67, 179–182. [Google Scholar] [CrossRef] [PubMed]
- Al-Jawoosh, S.; Ireland, A.; Su, B. Characterisation of mechanical and surface properties of novel biomimetic interpenetrating alumina-polycarbonate composite materials. Dent. Mater. 2020, 36, 1595–1607. [Google Scholar] [CrossRef]
- Al-Jawoosh, S. Fabrication and Characterisation of a Novel Biomimetic Interpenetrating Composite—A Potential Orthodontic Bracket Material. Ph.D. Thesis, University of Bristol, Bristol, UK, 2019. Available online: https://research-information.bris.ac.uk/ws/portalfiles/portal/193478337/Thesis.pdf (accessed on 19 March 2025).
- Preiss, A.; Su, B.; Collins, S.; Simpson, D. Tailored graded pore structure in zirconia toughened alumina ceramics using double-side cooling freeze casting. J. Eur. Ceram. Soc. 2012, 32, 1575–1583. [Google Scholar] [CrossRef]
- BSEN-843-4:2005; Advanced Technical Ceramics. Mechanical Properties of Monolithic Ceramics at Room Temperature. Vickers, Knoop and Rockwell Superficial Hardness. BSI: London, UK, 2017.
- ASTM-C373-16; Standard Test Methods for Determination of Water Absorption and Associated Properties by Vacuum Method for Pressed Ceramic Tiles and Glass Tiles and Boil Method for Extruded Ceramic Tiles and Non-Tile Fired Ceramic Whiteware Products. ASTM International: West Conshohocken, PA, USA, 2016.
- ASTM-E1820-18; Standard Test Method for Measurement of Fracture Toughness1. ASTM International: West Conshohocken, PA, USA, 2018.
- Fukasawa, T.; Deng, Z.Y.; Ando, M.; Ohji, T.; Goto, Y. Pore structure of porous ceramics synthesized from water-based slurry by freeze-dry process. J. Mater. Sci. 2001, 36, 2523–2527. [Google Scholar] [CrossRef]
- Sofie, S.W.; Dogan, F. 2001. Freeze casting of aqueous alumina slurries with glycerol. J. Am. Ceram. Soc. 2001, 84, 1459–1464. [Google Scholar] [CrossRef]
- Deville, S.; Meille, S.; Seuba, J. A meta-analysis of the mechanical properties of ice-templated ceramics and metals. Sci. Technol. Adv. Mater. 2015, 16, 043501. [Google Scholar] [CrossRef]
- Deville, S. Freeze-casting of porous ceramics: A review of current achievements and issues. Adv. Eng. Mater. 2008, 10, 155–169. [Google Scholar] [CrossRef]
- Nelson, I.; Naleway, S.E. Intrinsic and extrinsic control of freeze casting. J. Mater. Res. Technol. 2019, 8, 2372–2385. [Google Scholar] [CrossRef]
- Gaudillere, C.; Serra, J.M. Freeze-casting: Fabrication of highly porous and hierarchical ceramic supports for energy applications. Boletín Soc. Española Cerámica Vidr. 2016, 55, 45–54. [Google Scholar] [CrossRef]
- Ho, M.W.; Lam, C.K.; Lau, K.T.; Ng, D.H.; Hui, D. Mechanical properties of epoxy-based composites using nanoclays. Compos. Struct. 2006, 75, 15–421. [Google Scholar] [CrossRef]
- Deville, S.; Saiz, E.; Tomsia, A.P. Ice-templated porous alumina structures. Acta Mater. 2007, 55, 1965–1974. [Google Scholar] [CrossRef]
- Li, W.L.; Lu, K.; Walz, J.Y. Freeze casting of porous materials: Review of critical factors in microstructure evolution. Int. Mater. Rev. 2012, 57, 37–60. [Google Scholar] [CrossRef]
- Lasalle, A.; Guizard, C.; Maire, E.; Adrien, J.; Deville, S. Particle redistribution and structural defect development during ice templating. Acta Mater. 2012, 60, 4594–4603. [Google Scholar] [CrossRef]
- He, L.H.; Swain, M.V. Nanoindentation derived stress–strain properties of dental materials. Dent. Mater. 2007, 23, 814–821. [Google Scholar] [CrossRef]
- Min, J.; Arola, D.D.; Yu, D.; Yu, P.; Zhang, Q.; Yu, H.; Gao, S. Comparison of human enamel and polymer-infiltrated-ceramic-network material “ENAMIC” through micro-and nano-mechanical testing. Ceram. Int. 2016, 42, 10631–10637. [Google Scholar] [CrossRef]
- Guo, S.J.; Zhang, F.H.; Wang, B.T.; Zhang, C. Settlement prediction model of slurry suspension based on sedimentation rate attenuation. Water Sci. Eng. 2012, 5, 79–92. [Google Scholar] [CrossRef]
- Szczesio-Wlodarczyk, A.; Domarecka, M.; Kopacz, K.; Sokolowski, J.; Bociong, K. An evaluation of the properties of urethane dimethacrylate-based dental resins. Materials 2021, 14, 2727. [Google Scholar] [CrossRef]
- Algharaibeh, S.; Wan, H.; Al-Fodeh, R.; Ireland, A.J.; Zhang, D.; Su, B. Fabrication and mechanical properties of biomimetic nacre-like ceramic/polymer composites for chairside CAD/CAM dental restorations. Dent. Mater. 2022, 38, 121–132. [Google Scholar] [CrossRef] [PubMed]
- Al-Jawoosh, S.; Ireland, A.J.; Su, B. Fabrication and characterisation of a novel biomimetic anisotropic ceramic/polymer-infiltrated composite material. Dent. Mater. 2018, 34, 994–1002. [Google Scholar] [CrossRef] [PubMed]
- Meille, S.; Lombardi, M.; Chevalier, J.; Montanaro, L. Mechanical properties of porous ceramics in compression: On the transition between elastic, brittle, and cellular behavior. J. Eur. Ceram. Soc. 2012, 32, 3959–3967. [Google Scholar] [CrossRef]
- Wu, J.; Luo, B.; Liu, X.; Zhang, L. Control of the structure and mechanical property of porous WS2 scaffold during freeze casting. J. Porous Mater. 2018, 25, 37–43. [Google Scholar] [CrossRef]
- Chun, K.J.; Choi, H.H.; Lee, J.Y. Comparison of mechanical property and role between enamel and dentin in the human teeth. J. Dent. Biomech. 2014, 5, 1758736014520809. [Google Scholar] [CrossRef]
- Asmani, M.; Kermel, C.; Leriche, A.; Qurak, M. Influence of porosity on Young’s modulus and Poisson’s ratio in alumina ceramics. J. Eur. Ceram. Soc. 2001, 21, 1081–1086. [Google Scholar] [CrossRef]
- Coldea, A.; Swain, M.V.; Thiel, N. Mechanical properties of polymer-infiltrated-ceramic-network materials. Dent. Mater. 2013, 29, 419–426. [Google Scholar] [CrossRef]
- He, L.H.; Swain, M.V. Understanding the mechanical behaviour of human enamel from its structural and compositional characteristics. J. Mech. Behav. Biomed. Mater. 2008, 1, 18–29. [Google Scholar] [CrossRef]
- Kinney, J.H.; Balooch, M.; Marshall, S.J.; Marshall Jr, G.W.; Weihs, T.P. Hardness and Young’s modulus of human peritubular and intertubular dentine. Arch. Oral. Biol. 1996, 41, 9–13. [Google Scholar] [CrossRef]
- Xu, H.; Smith, D.; Jahanmir, S.; Romberg, E.; Kelly, J.; Thompson, V.; Rekow, E. Indentation damage and mechanical properties of human enamel and dentin. J. Dent. Res. 1998, 77, 472–480. [Google Scholar] [CrossRef]
- He, L.H.; Swain, M.V. A novel polymer infiltrated ceramic dental material. Dent. Mater. 2011, 27, 527–534. [Google Scholar] [CrossRef] [PubMed]
- Belli, R.; Wendler, M.; Zorzin, J.I.; Lohbauer, U. Practical and theoretical considerations on the fracture toughness testing of dental restorative materials. Dent. Mater. 2018, 34, 97–119. [Google Scholar] [CrossRef] [PubMed]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties, 2nd ed.; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Iwasa, M.; Brandt, R. Fracture Toughness of Single Crystal Alumina. In Structure and Properties of MgO and Al2O3; WD, K., Ed.; American Ceramic Society: Columbus, OH, USA, 1986. [Google Scholar]
- Anstis, G.; Chantikul, P.; Lawn, B.R.; Marshall, D. A critical evaluation of indentation techniques for measuring fracture toughness: I, direct crack measurements. J. Am. Ceram. Soc. 1981, 64, 533–538. [Google Scholar] [CrossRef]
- Gogotsi, G.A. Fracture toughness of ceramics and ceramic composites. Ceram. Int. 2003, 29, 777–784. [Google Scholar] [CrossRef]
- Guazzato, M.; Albakry, M.; Ringer, S.P.; Swain, M.V. Strength, fracture toughness and microstructure of a selection of all-ceramic materials. Part I. Pressable and alumina glass-infiltrated ceramics. Dent. Mater. 2004, 20, 441–448. [Google Scholar] [CrossRef]
- Park, S.; Quinn, J.B.; Romberg, E.; Arola, D. On the brittleness of enamel and selected dental materials. Dent. Mater. 2008, 24, 1477–1485. [Google Scholar] [CrossRef]



| C20-S8 | Ceramic-Rich | Ceramic–Polymer | Polymer-Rich | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Distance from Conduction Base (mm) | ||||||||||
| 5 | 10 | 15 | ||||||||
| Mean | SD | 95% CI | Mean | SD | 95% CI | Mean | SD | 95% CI | ||
| Porosity | (%) | 47.74 | 0.93 | 0.61 | 53.34 | 1.23 | 0.80 | 55.19 | 2.50 | 1.63 |
| Ceramic Volume Fraction | (%) | 52.26 | 0.93 | 0.61 | 46.66 | 1.23 | 0.80 | 44.81 | 2.50 | 1.63 |
| Compressive Strength | (MPa) | 120.92 | 16.64 | 13.31 | 91.20 | 18.23 | 14.59 | 60.25 | 9.16 | 6.79 |
| Modulus of Elasticity | (GPa) | 35.29 | 6.70 | 5.36 | 31.40 | 3.06 | 2.45 | 19.84 | 4.41 | 3.27 |
| Fracture Toughness—KIC | (MPa·m1/2) | 1.78 | 0.23 | 0.20 | 1.54 | 0.21 | 0.17 | 0.78 | 0.22 | 0.18 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wong, Y.M.; Ireland, A.J.; Su, B. Development of Structurally Graded Alumina–Polymer Composites as Potential Orthodontic Bracket Materials. Biomimetics 2025, 10, 227. https://doi.org/10.3390/biomimetics10040227
Wong YM, Ireland AJ, Su B. Development of Structurally Graded Alumina–Polymer Composites as Potential Orthodontic Bracket Materials. Biomimetics. 2025; 10(4):227. https://doi.org/10.3390/biomimetics10040227
Chicago/Turabian StyleWong, Yin Mun, Anthony J. Ireland, and Bo Su. 2025. "Development of Structurally Graded Alumina–Polymer Composites as Potential Orthodontic Bracket Materials" Biomimetics 10, no. 4: 227. https://doi.org/10.3390/biomimetics10040227
APA StyleWong, Y. M., Ireland, A. J., & Su, B. (2025). Development of Structurally Graded Alumina–Polymer Composites as Potential Orthodontic Bracket Materials. Biomimetics, 10(4), 227. https://doi.org/10.3390/biomimetics10040227







