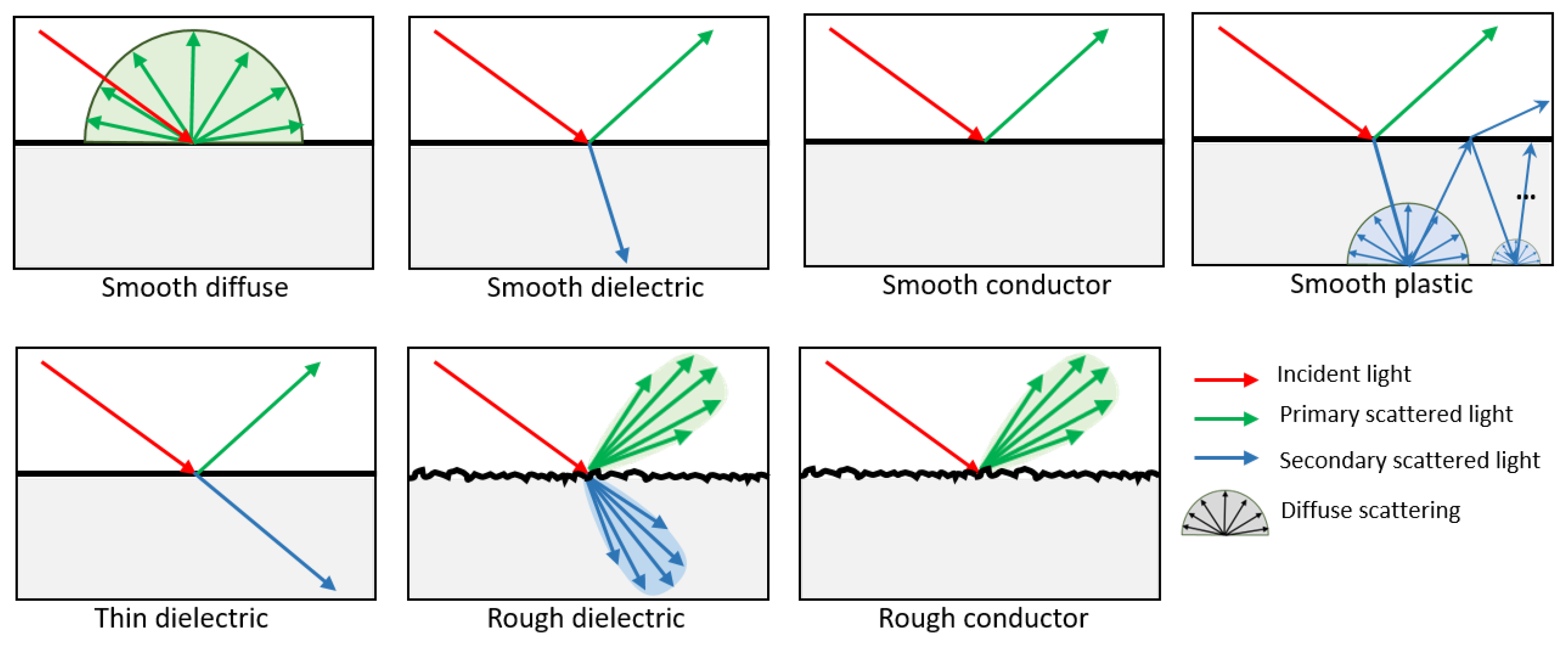
Figure 1.
Schematic overview of the most important surface scattering models in SWEET.
Figure 1.
Schematic overview of the most important surface scattering models in SWEET.
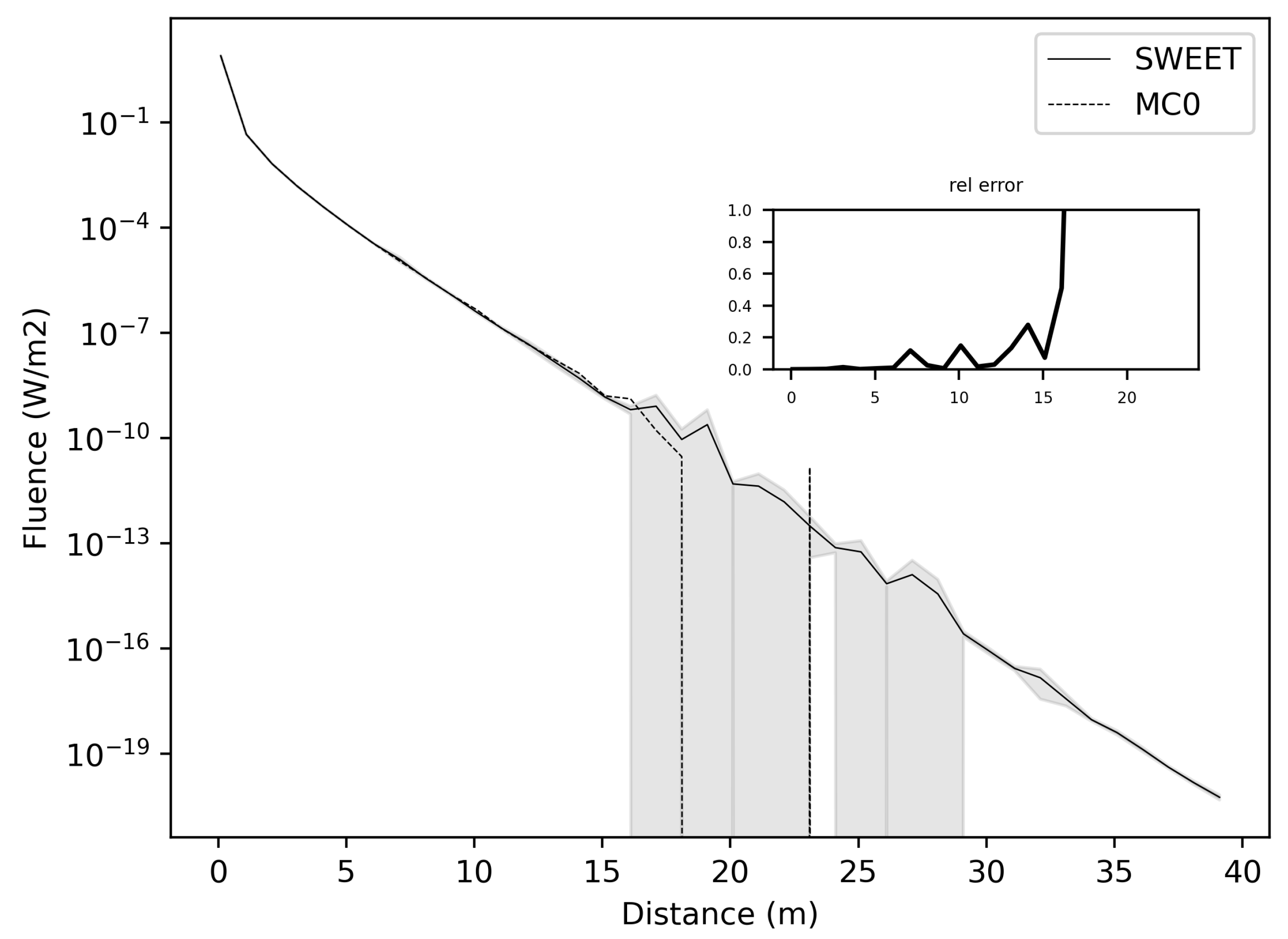
Figure 2.
Comparing SWEET to Steven’s Monte Carlo code for a simple situation on fluence evaluation, which is computed as the integral of the radiance over the solid angles of 3D space (L is the radiance at position r and in a direction ), for asymetry phase parameter: g = 0.0, optical parameters: = 0.5 m−1 and = 0.5 m−1, the 95% confidence intervals for SWEET are in grey.
Figure 2.
Comparing SWEET to Steven’s Monte Carlo code for a simple situation on fluence evaluation, which is computed as the integral of the radiance over the solid angles of 3D space (L is the radiance at position r and in a direction ), for asymetry phase parameter: g = 0.0, optical parameters: = 0.5 m−1 and = 0.5 m−1, the 95% confidence intervals for SWEET are in grey.
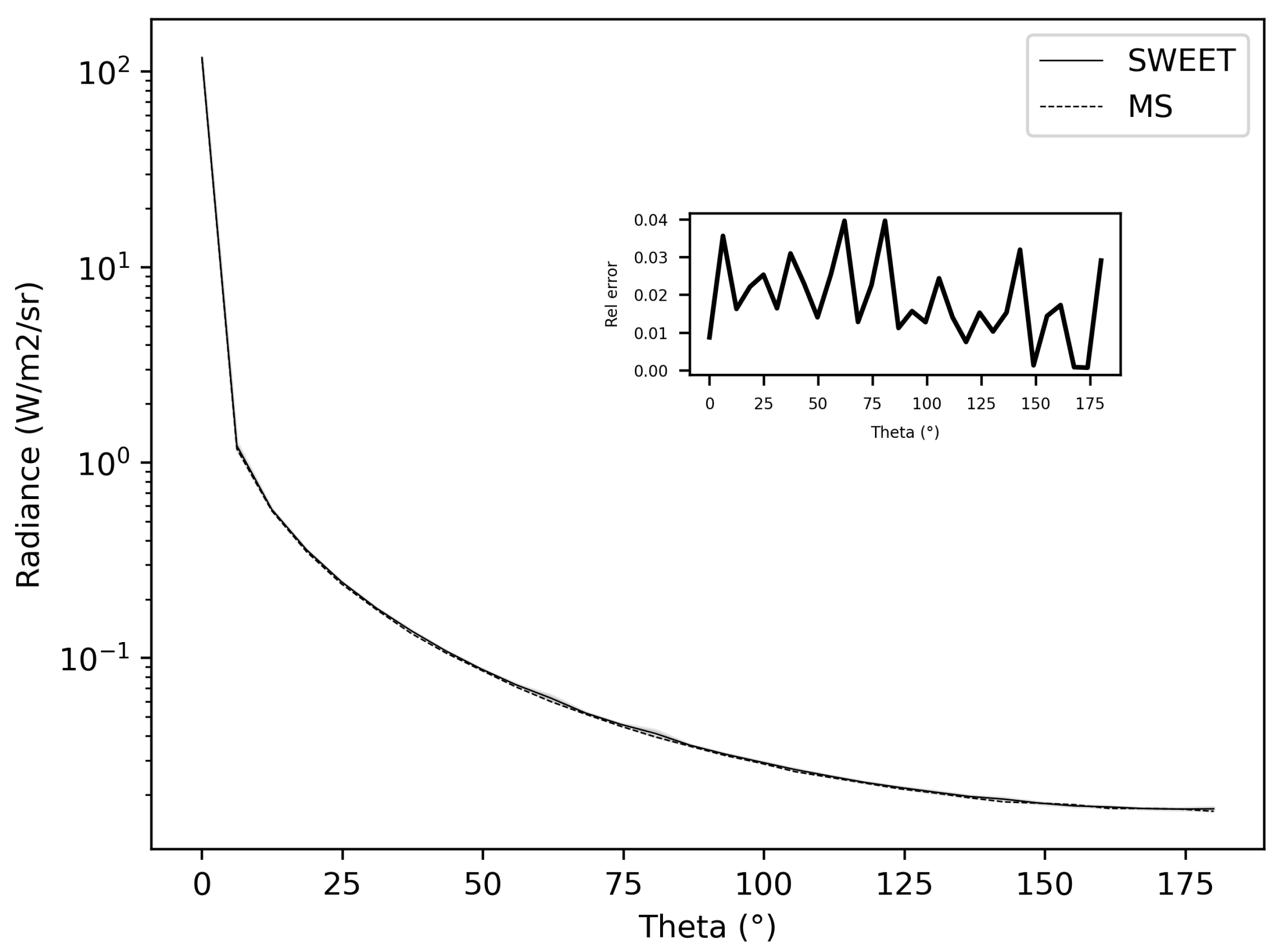
Figure 3.
Comparing SWEET to Mitsuba for a simple situation on radiance evaluation at a distance of 1 mm, for asymetry phase parameter: g = 0.5, optical parameters: = 0.9 m−1 and = 0.1 m−1.
Figure 3.
Comparing SWEET to Mitsuba for a simple situation on radiance evaluation at a distance of 1 mm, for asymetry phase parameter: g = 0.5, optical parameters: = 0.9 m−1 and = 0.1 m−1.
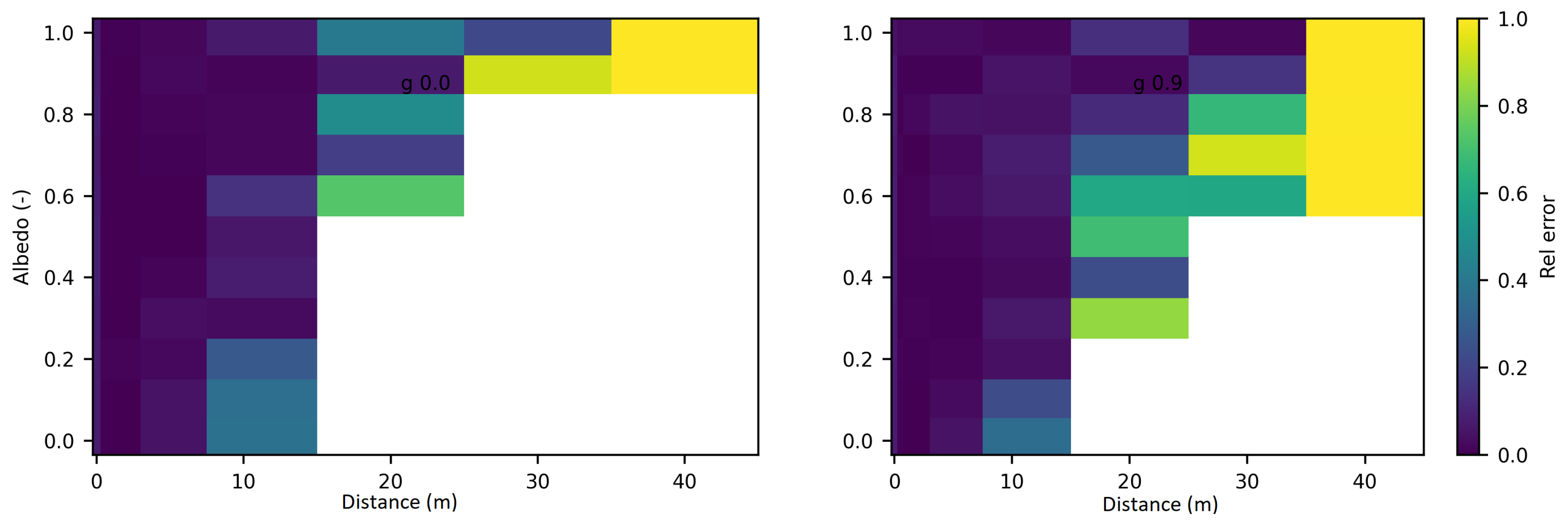
Figure 4.
Relative discrepancies for fluence between SWEET to Steven’s Monte Carlo code for a set of distances, optical coeficients ( & ) and anisotropy phase parameter (g). Albedo , where is taken equal to 1.0; g = 0 in the left and g = 0.9 in the right.
Figure 4.
Relative discrepancies for fluence between SWEET to Steven’s Monte Carlo code for a set of distances, optical coeficients ( & ) and anisotropy phase parameter (g). Albedo , where is taken equal to 1.0; g = 0 in the left and g = 0.9 in the right.
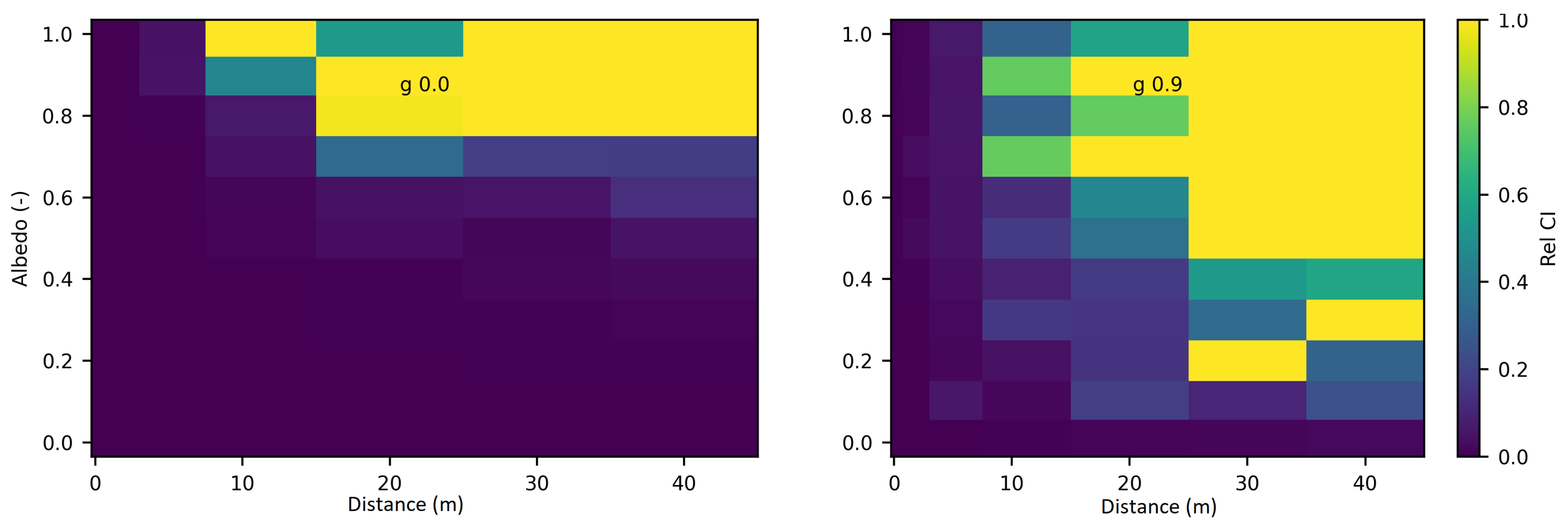
Figure 5.
Relative 95% confidence intervals of SWEET for fluence for a set of distances, optical coeficients ( & ) and anisotropy phase parameter (g). Albedo , g = 0 in the left and g = 0.9 in the right.
Figure 5.
Relative 95% confidence intervals of SWEET for fluence for a set of distances, optical coeficients ( & ) and anisotropy phase parameter (g). Albedo , g = 0 in the left and g = 0.9 in the right.
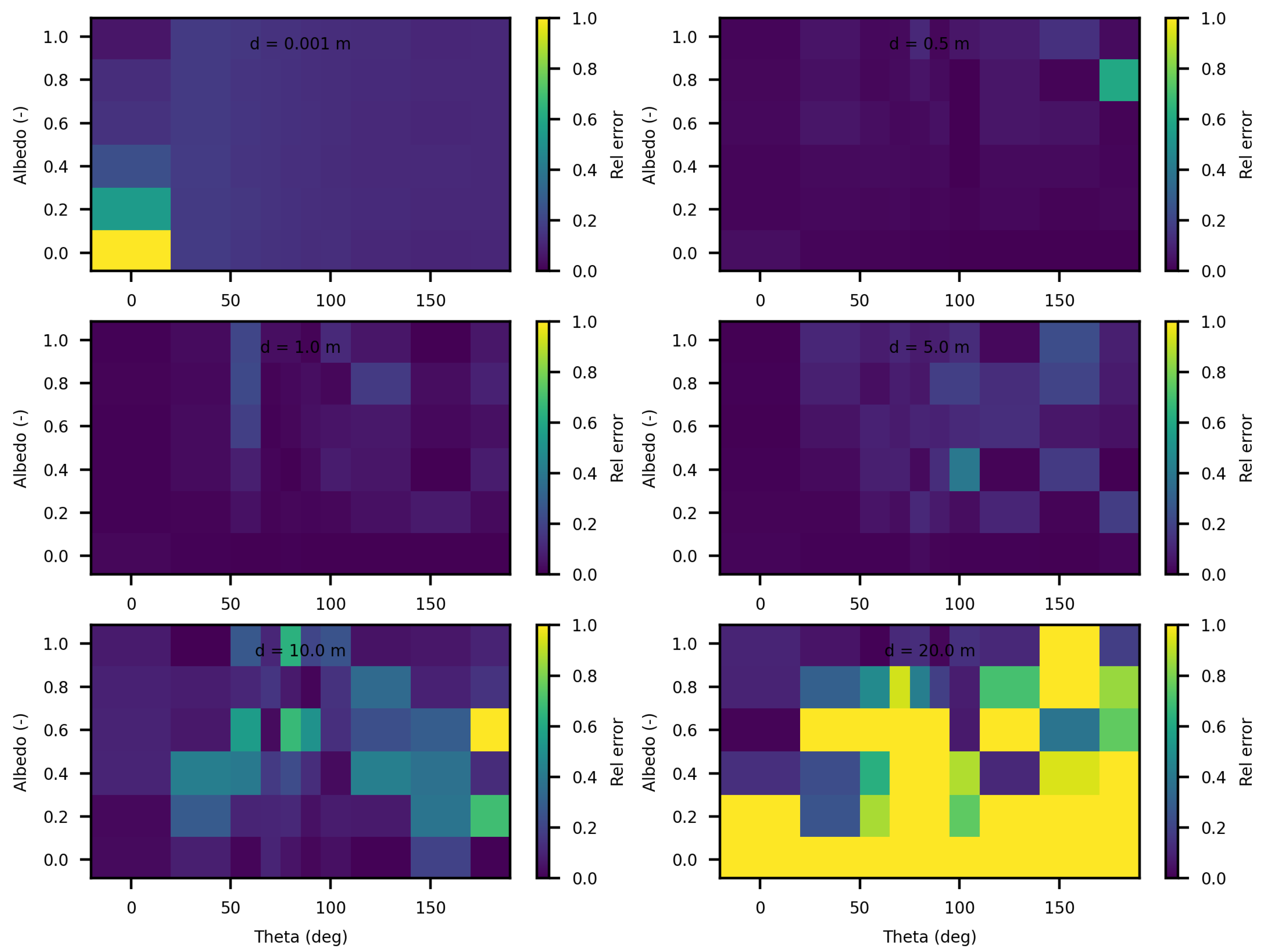
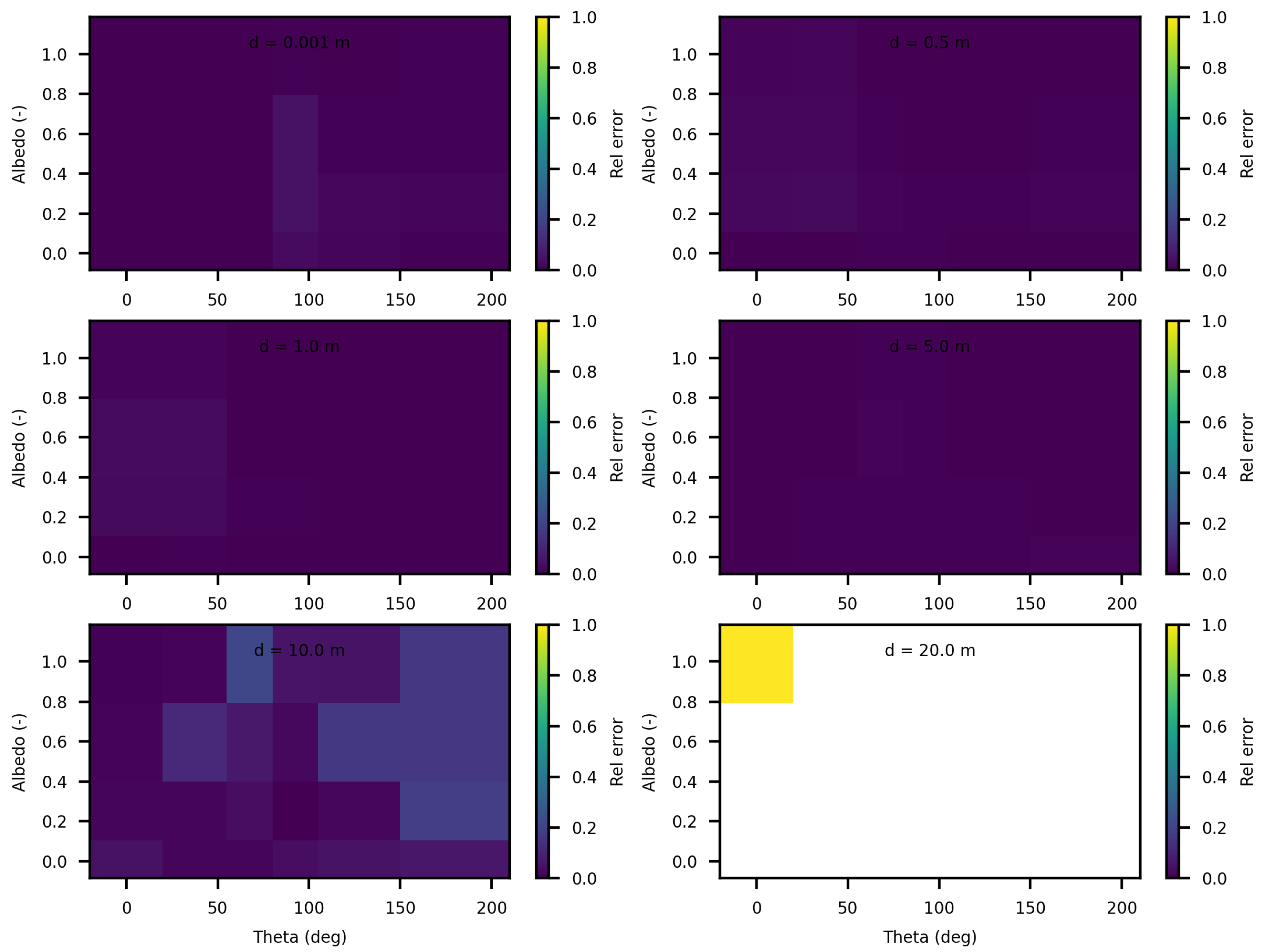
Figure 6.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of punctual light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 6.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of punctual light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
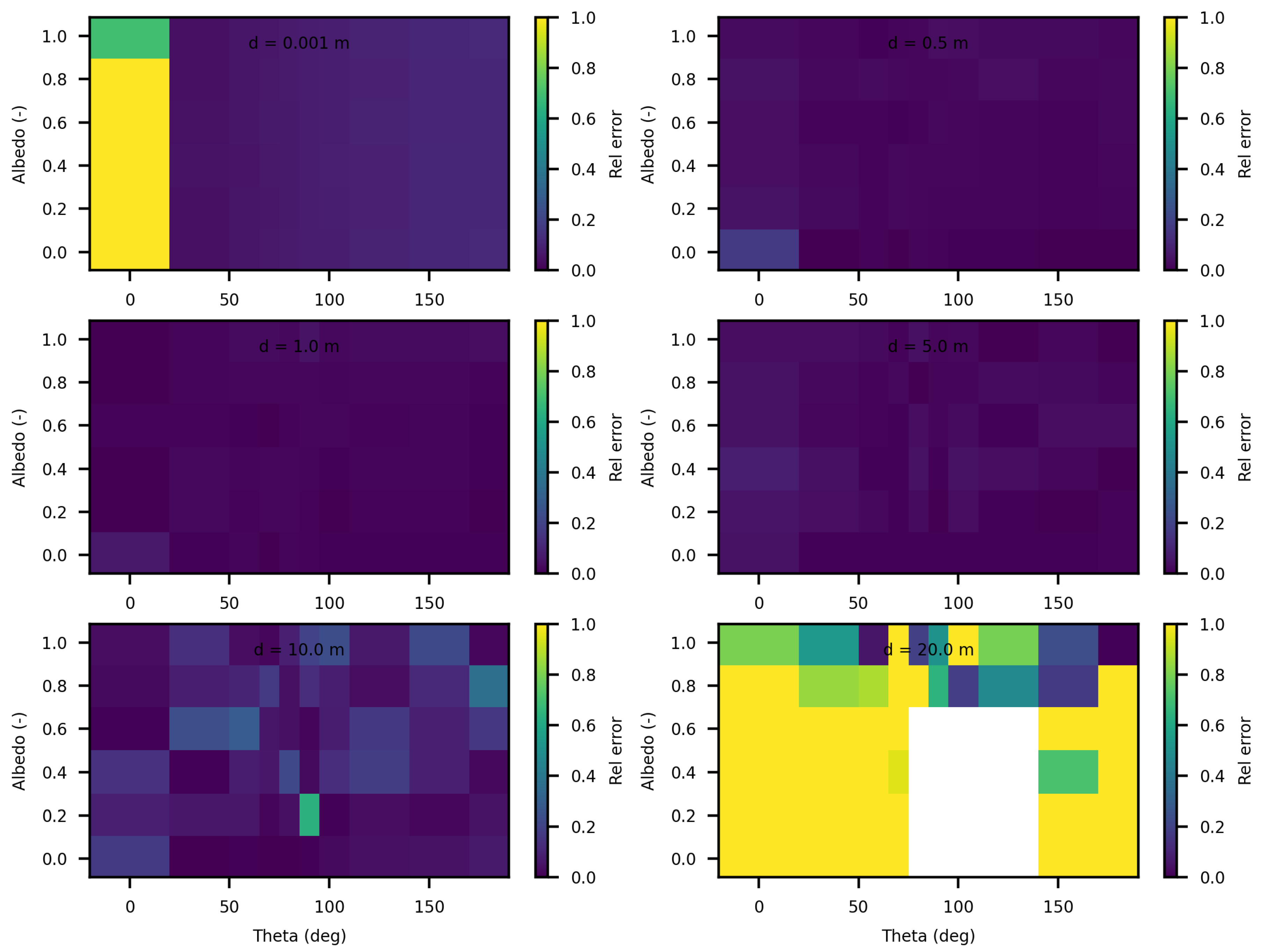
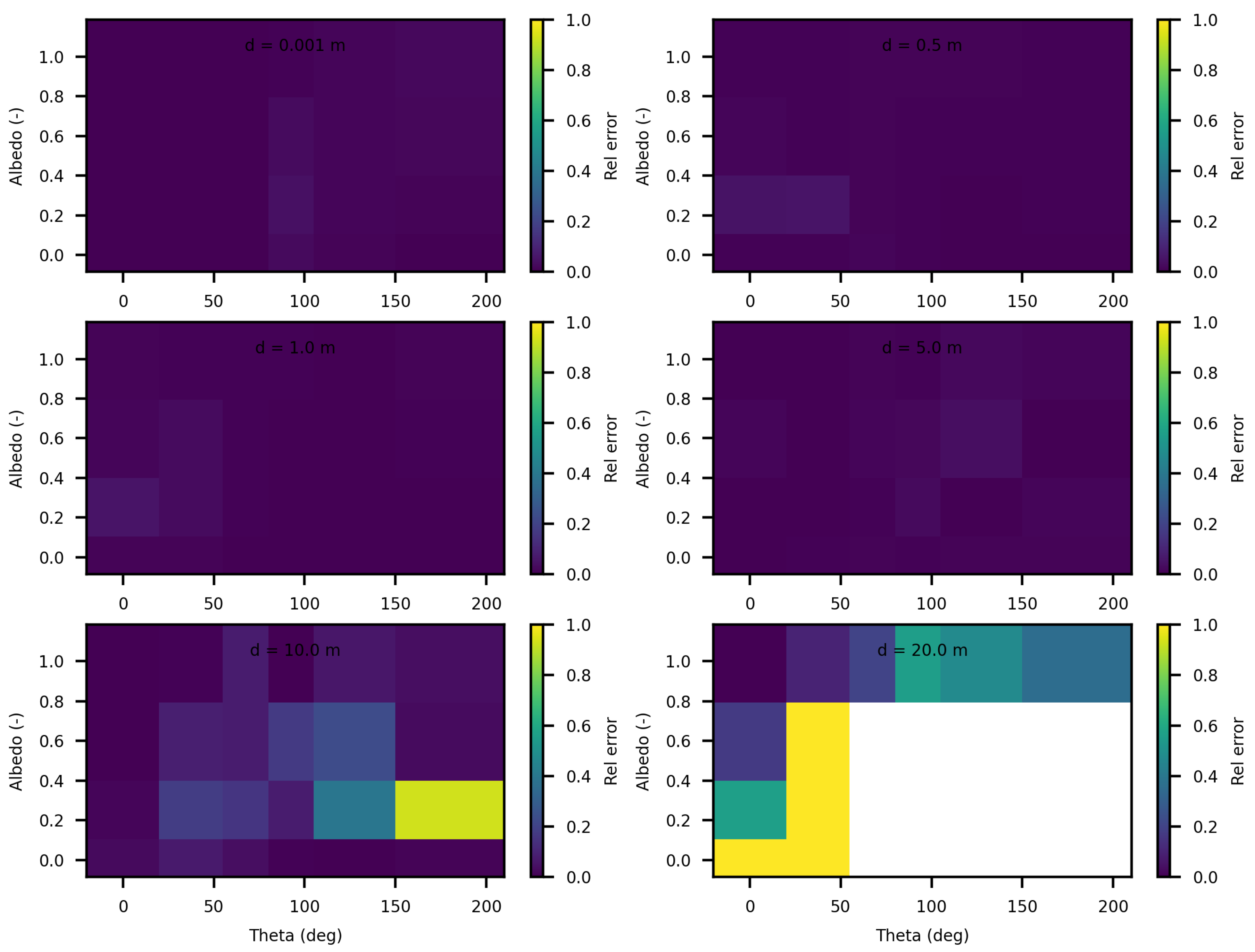
Figure 7.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.9, for the case of punctual light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 7.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.9, for the case of punctual light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
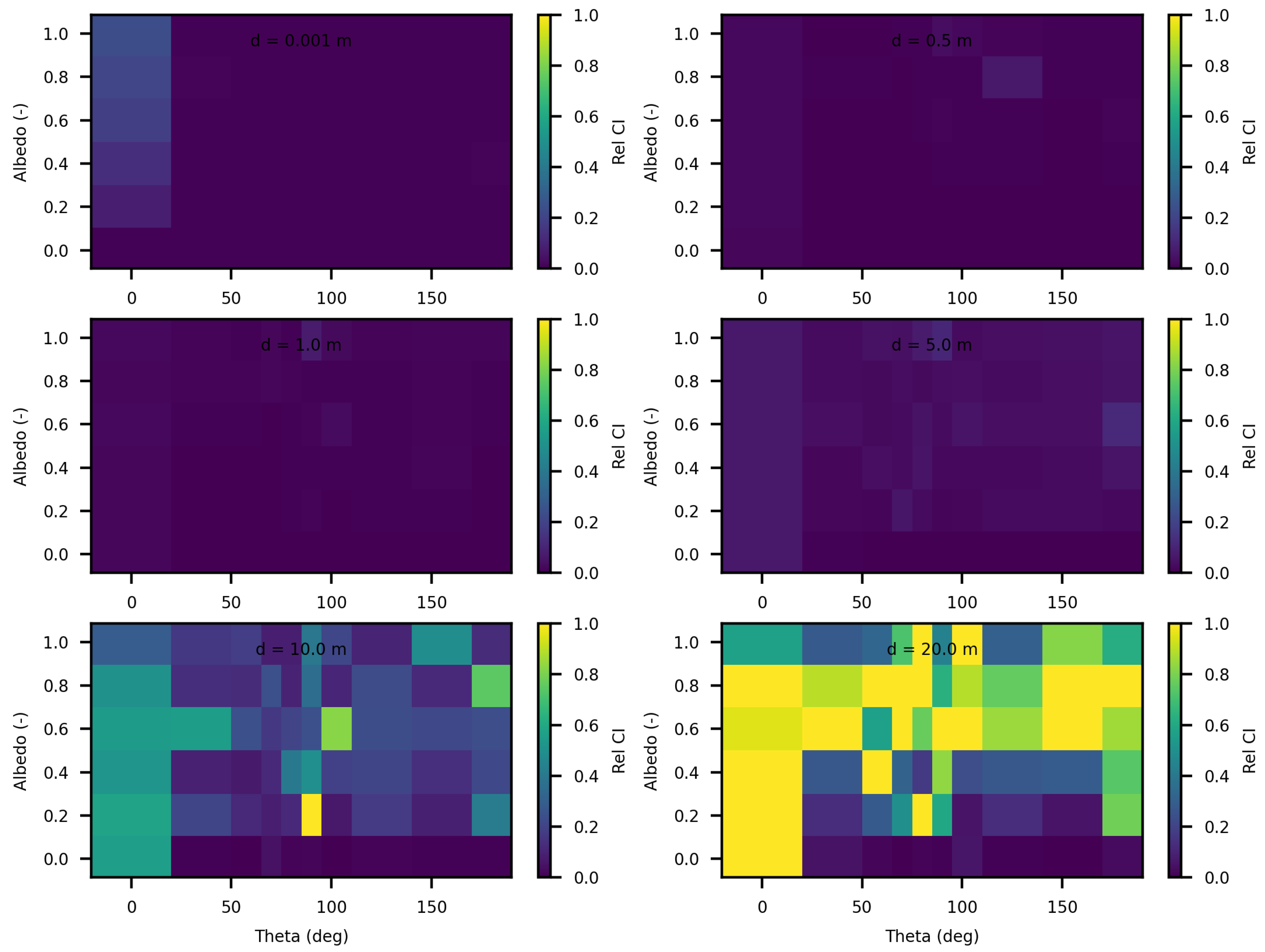
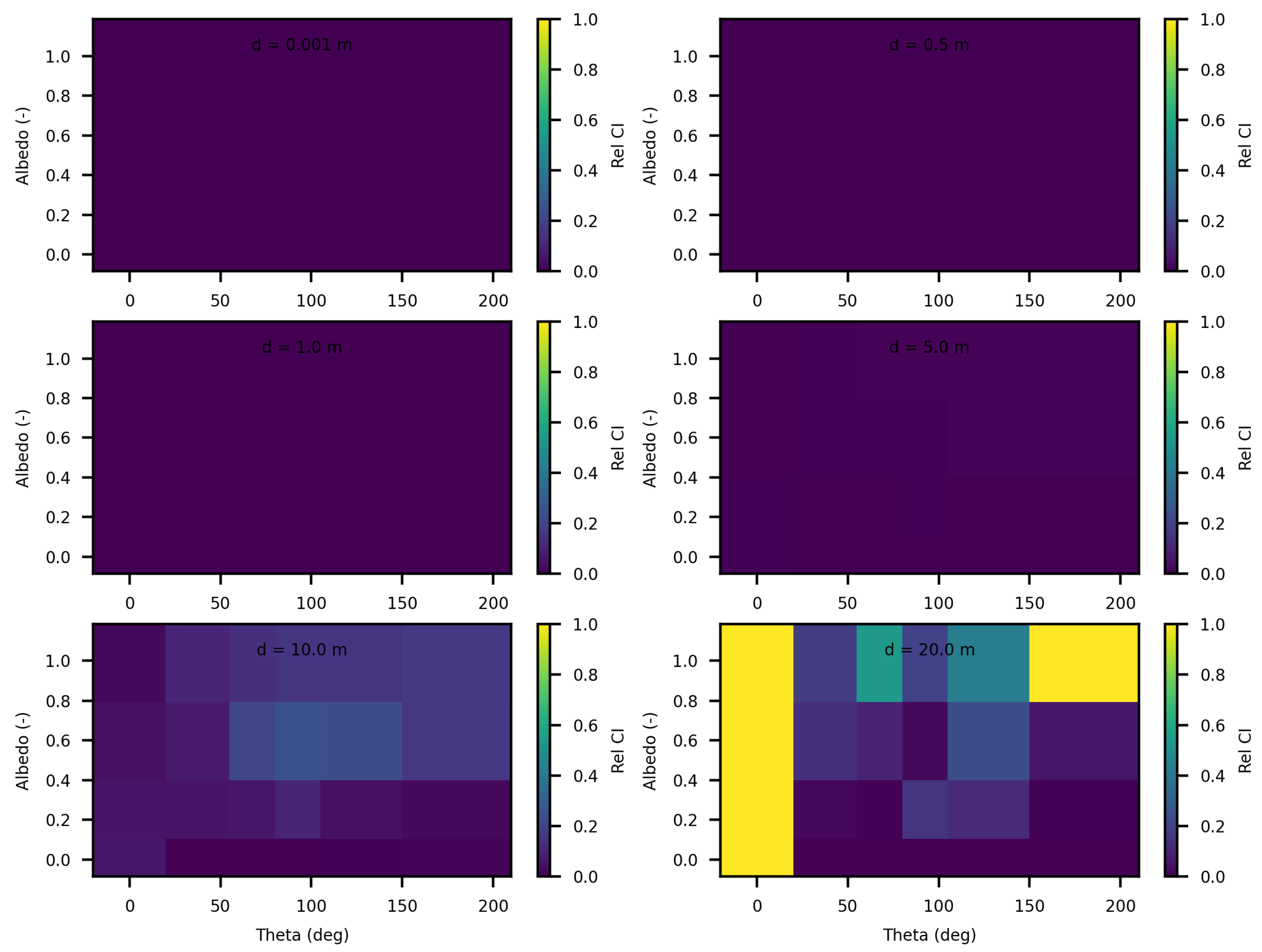
Figure 8.
SWEET relative 95% confidence interval for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of punctual light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 8.
SWEET relative 95% confidence interval for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of punctual light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
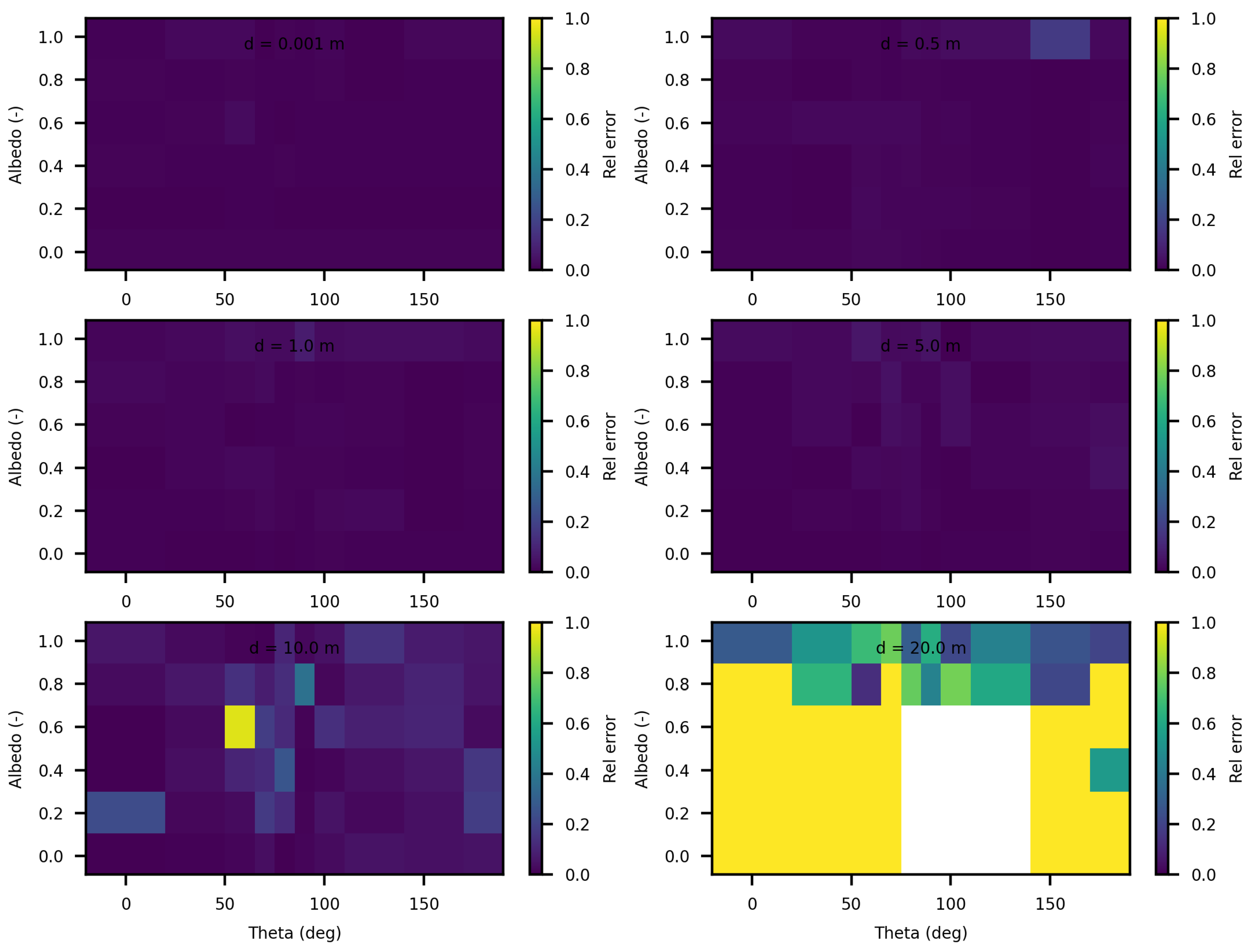
Figure 9.
SWEET relative 95% confidence interval for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.9, for the case of punctual light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 9.
SWEET relative 95% confidence interval for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.9, for the case of punctual light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 10.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of rectangular light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 10.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of rectangular light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 11.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.9, for the case of rectangular light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 11.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.9, for the case of rectangular light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 12.
SWEET relative 95% confidence interval for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of rectangular light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 12.
SWEET relative 95% confidence interval for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of rectangular light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 13.
SWEET relative 95% confidence interval for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.9, for the case of rectangular light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 13.
SWEET relative 95% confidence interval for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.9, for the case of rectangular light source. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 14.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of 2 point lights. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 14.
Comparing SWEET to Mitsuba Monte Carlo code for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of 2 point lights. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 15.
SWEET relative 95% confidence interval for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of 2 point lights. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
Figure 15.
SWEET relative 95% confidence interval for luminance for a set of distances and optical coeficients ( & ). Anisotropy phase parameter (g) equals to 0.0, for the case of 2 point lights. Albedo , where is taken equal to 1.0, each figure corresponds to a distance as in this matrix: m.
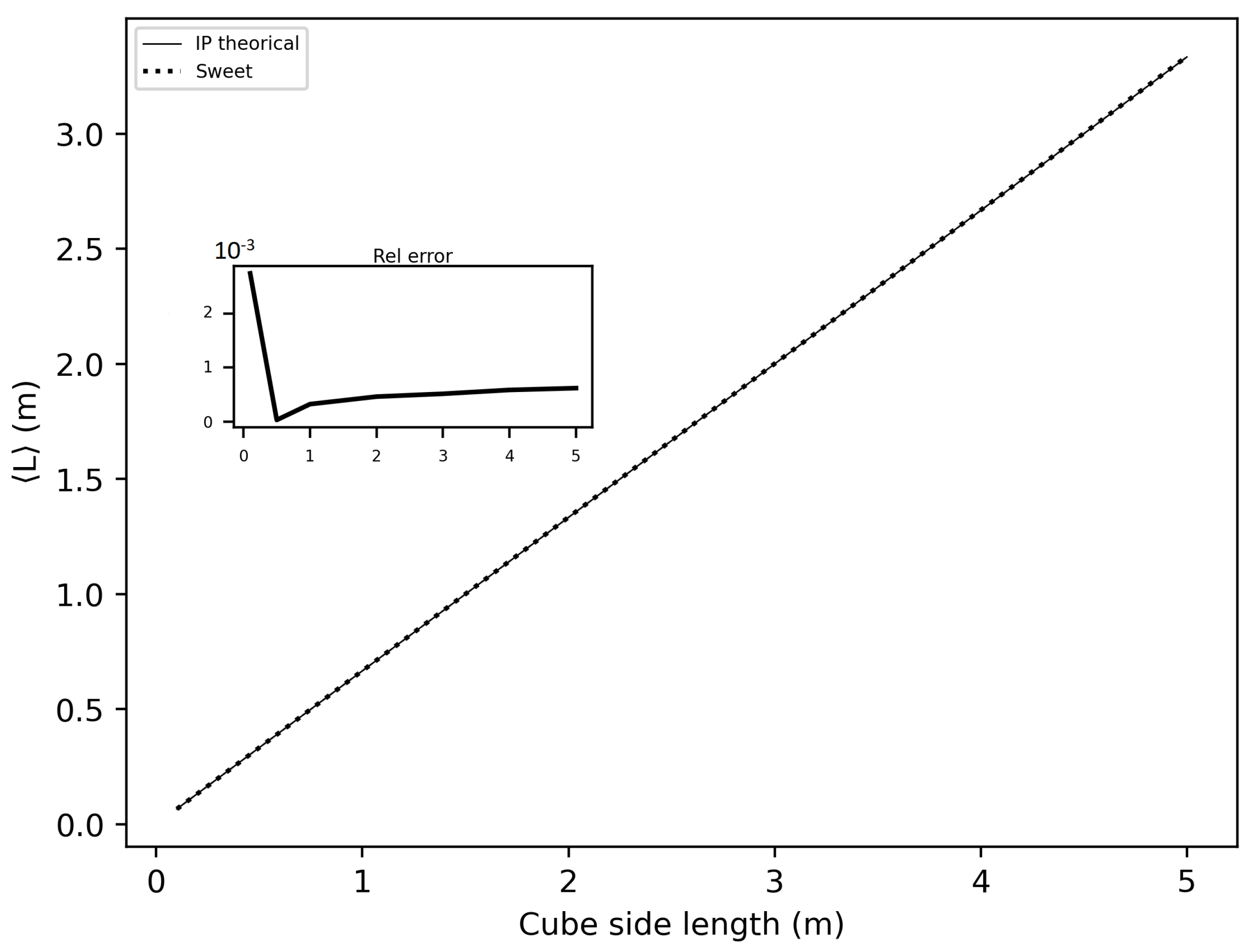
Figure 16.
Mean path length estimated with SWEET and theorically (using IP) for cubes with side lengths ranging from 10 cm to 5 m, for asymetry phase parameter: g = 0.0, optical parameters: = 1.0 m−1 and = 0.0 m−1.
Figure 16.
Mean path length estimated with SWEET and theorically (using IP) for cubes with side lengths ranging from 10 cm to 5 m, for asymetry phase parameter: g = 0.0, optical parameters: = 1.0 m−1 and = 0.0 m−1.
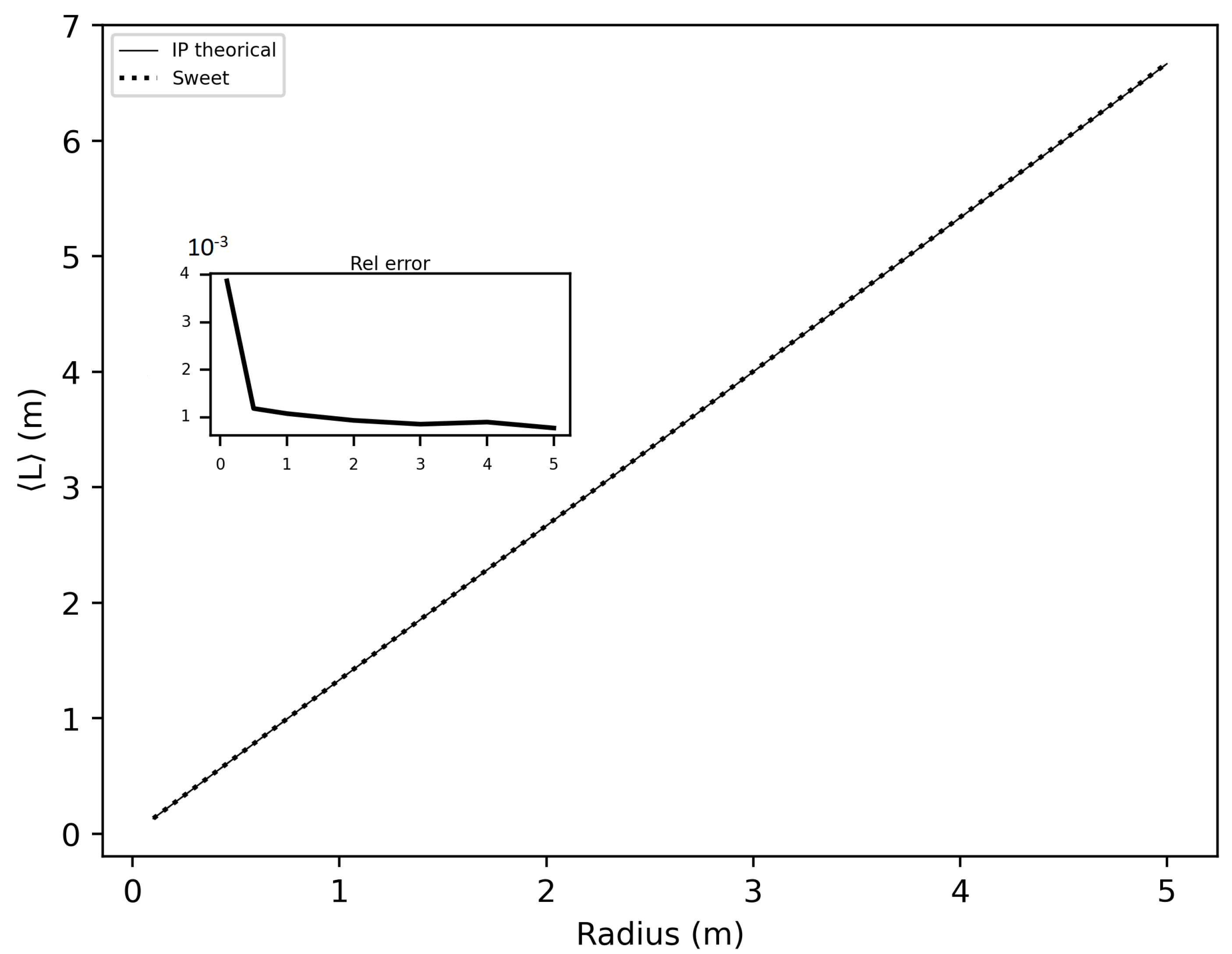
Figure 17.
Mean path length estimated with SWEET and theorically (using IP) for spheres with radii ranging from 10 cm to 5 m, for asymetry phase parameter: g = 0.0, optical parameters: = 1.0 m−1 and = 0.0 m−1.
Figure 17.
Mean path length estimated with SWEET and theorically (using IP) for spheres with radii ranging from 10 cm to 5 m, for asymetry phase parameter: g = 0.0, optical parameters: = 1.0 m−1 and = 0.0 m−1.
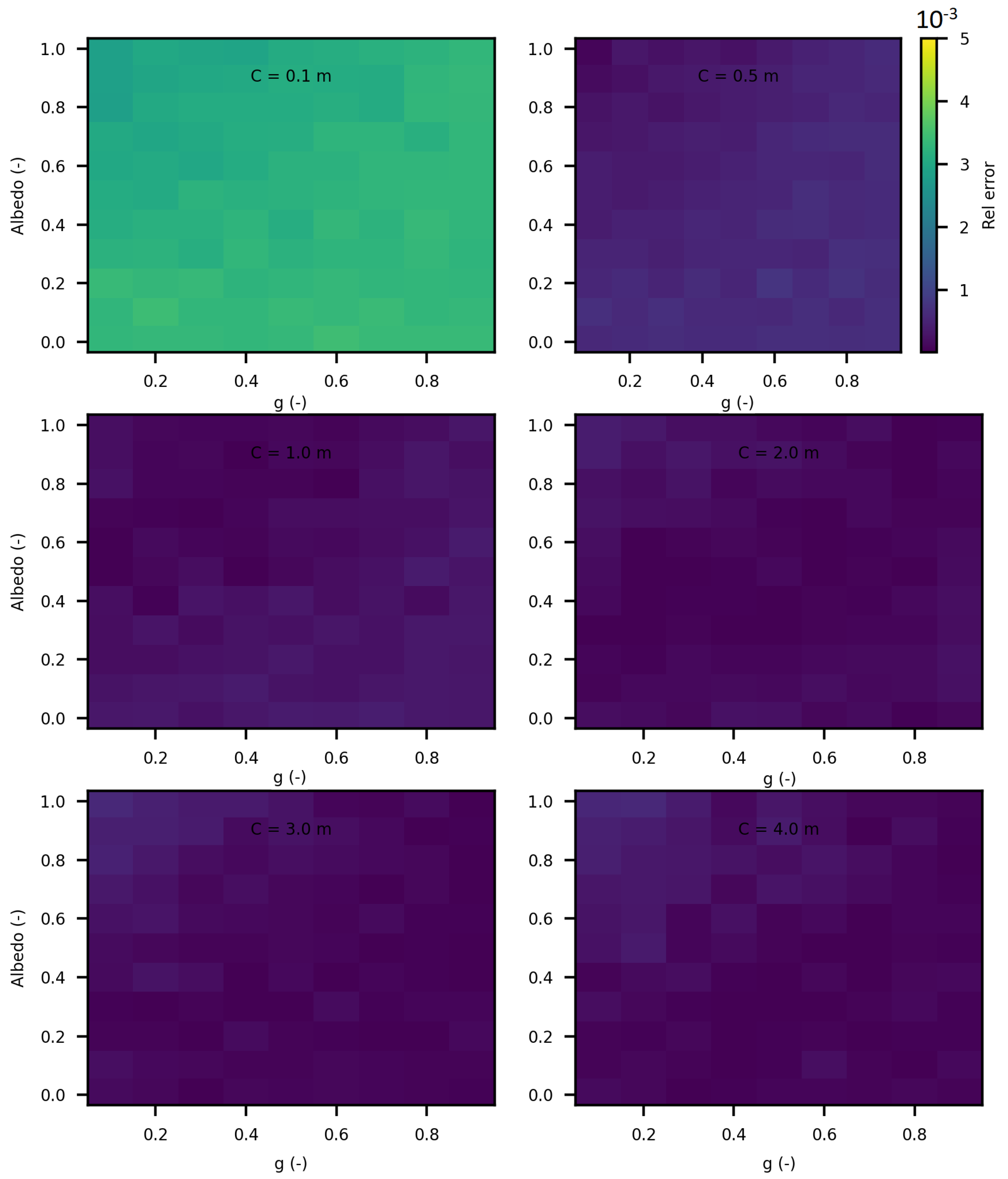
Figure 18.
Relative errors of invariance property (IP) estimated with SWEET and theorically for cubes with side lengths ranging from 10 cm to 4 m, for varying asymetry phase parameter g and optical parameters ( and ), each figure corresponds to a side length as in this matrix: m.
Figure 18.
Relative errors of invariance property (IP) estimated with SWEET and theorically for cubes with side lengths ranging from 10 cm to 4 m, for varying asymetry phase parameter g and optical parameters ( and ), each figure corresponds to a side length as in this matrix: m.
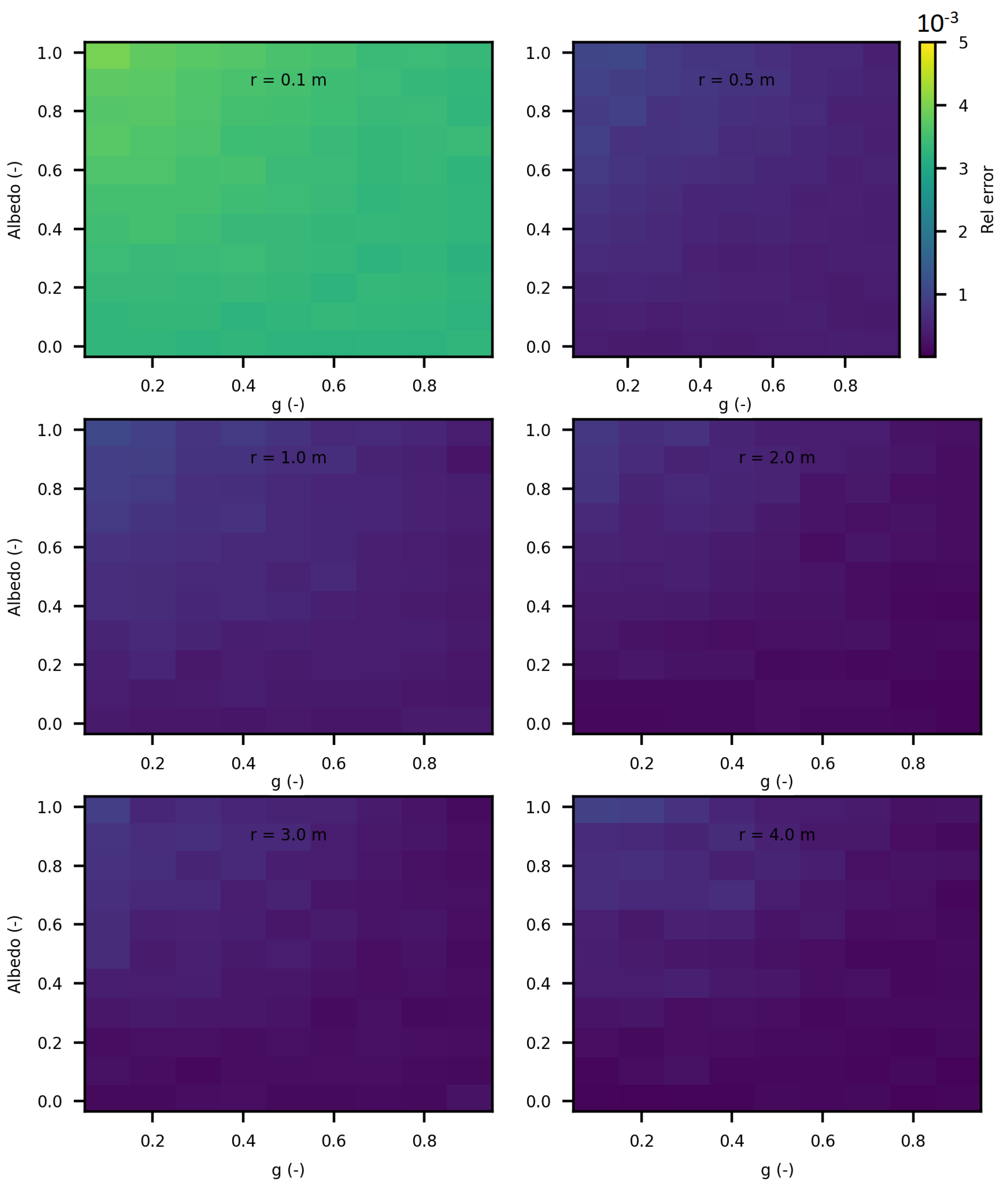
Figure 19.
Relative errors of invariance property (IP) estimated with SWEET and theorically for spheres with radii ranging from 10 cm to 4 m, for varying asymetry phase parameter g and optical parameters ( and ), each figure corresponds to a radius as in this matrix: m.
Figure 19.
Relative errors of invariance property (IP) estimated with SWEET and theorically for spheres with radii ranging from 10 cm to 4 m, for varying asymetry phase parameter g and optical parameters ( and ), each figure corresponds to a radius as in this matrix: m.
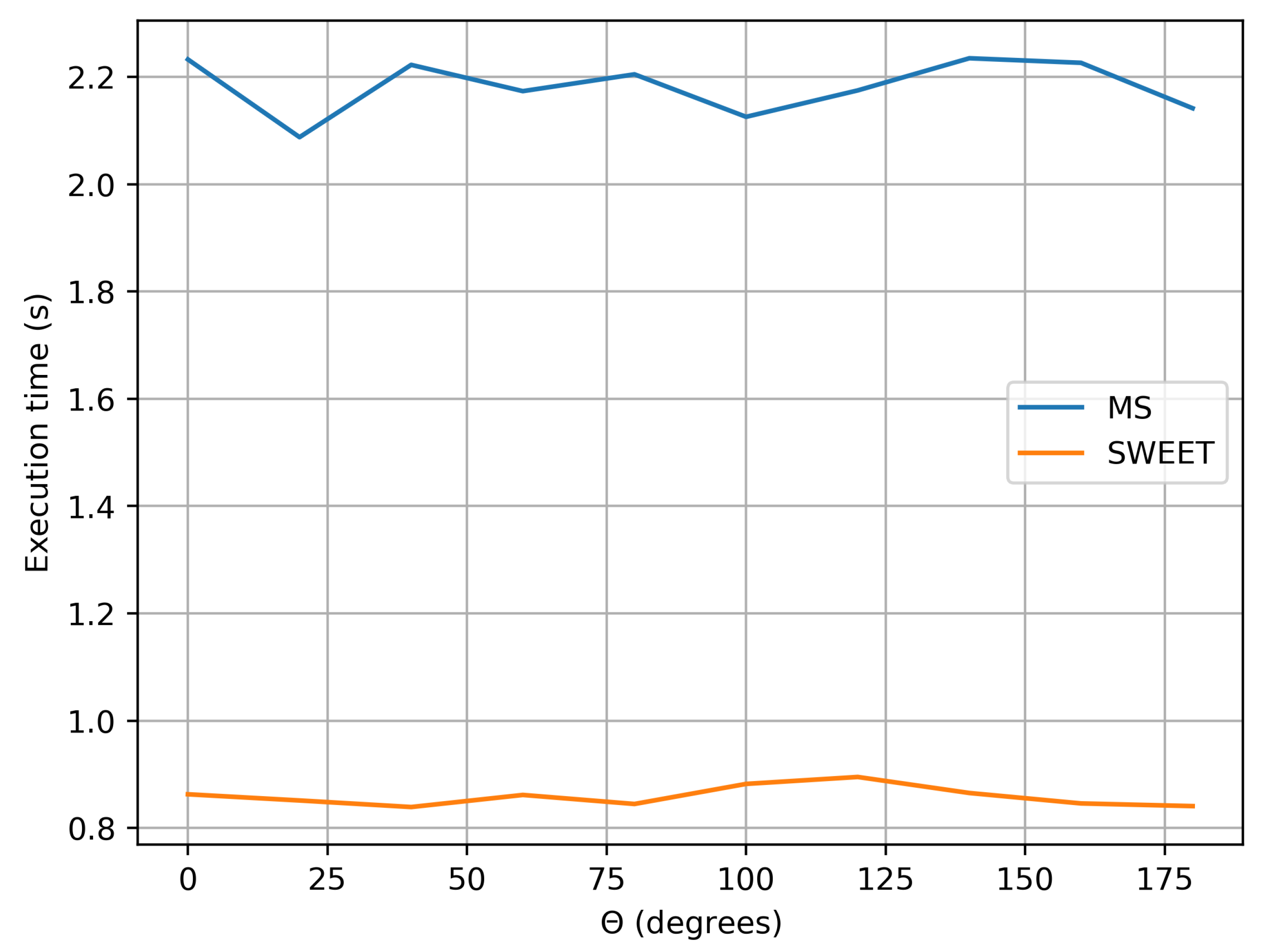
Figure 20.
Execution time for SWEET and MS done with photons in luminance computing, for asymetry phase parameter: g = 0.9, optical parameters: = 0.99 m−1 and = 0.01 m−1.
Figure 20.
Execution time for SWEET and MS done with photons in luminance computing, for asymetry phase parameter: g = 0.9, optical parameters: = 0.99 m−1 and = 0.01 m−1.
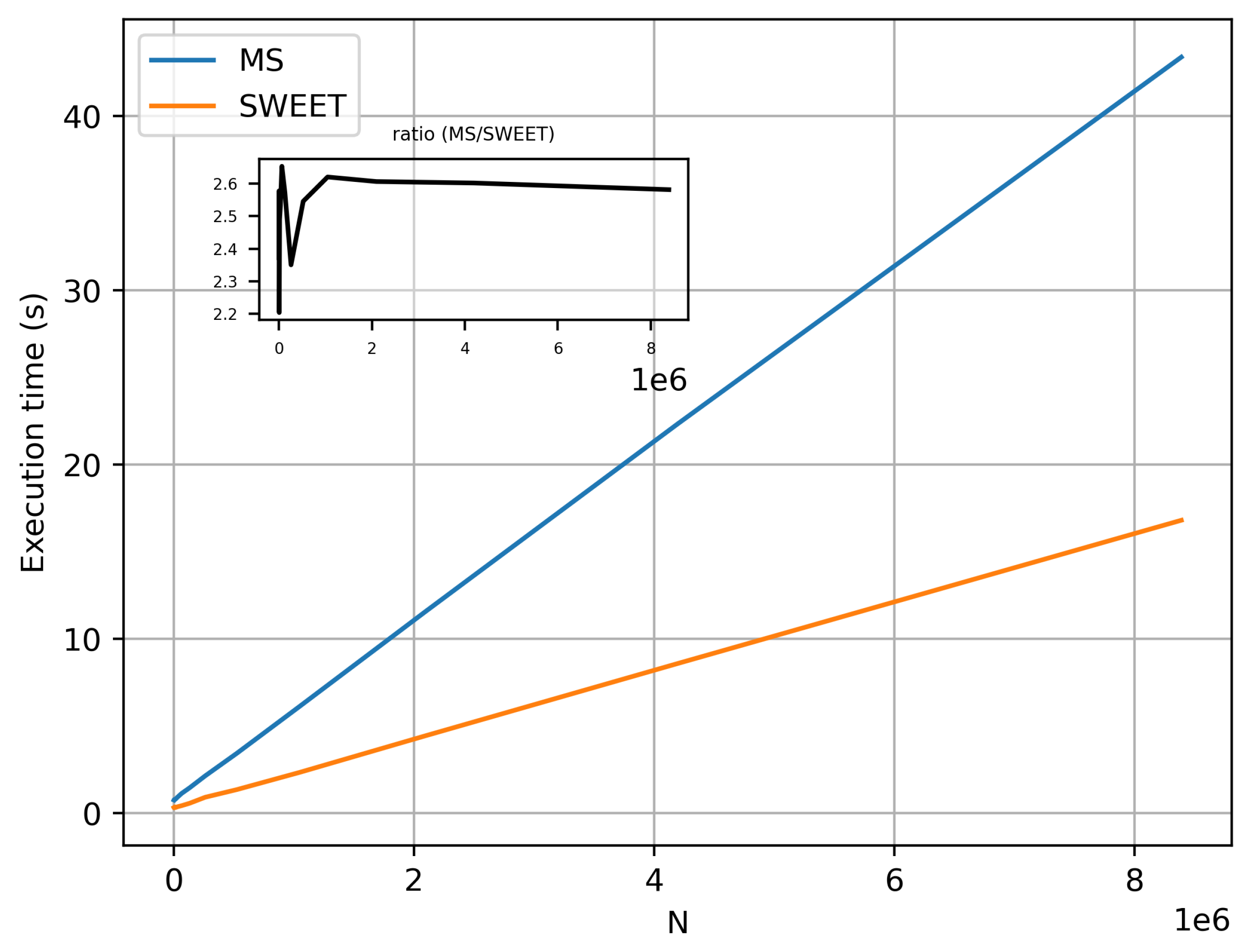
Figure 21.
Execution time variation with the photon count for SWEET and MS for a single luminance computing (), for asymetry phase parameter: g = 0.9, optical parameters: = 0.99 m−1 and = 0.01 m−1.
Figure 21.
Execution time variation with the photon count for SWEET and MS for a single luminance computing (), for asymetry phase parameter: g = 0.9, optical parameters: = 0.99 m−1 and = 0.01 m−1.
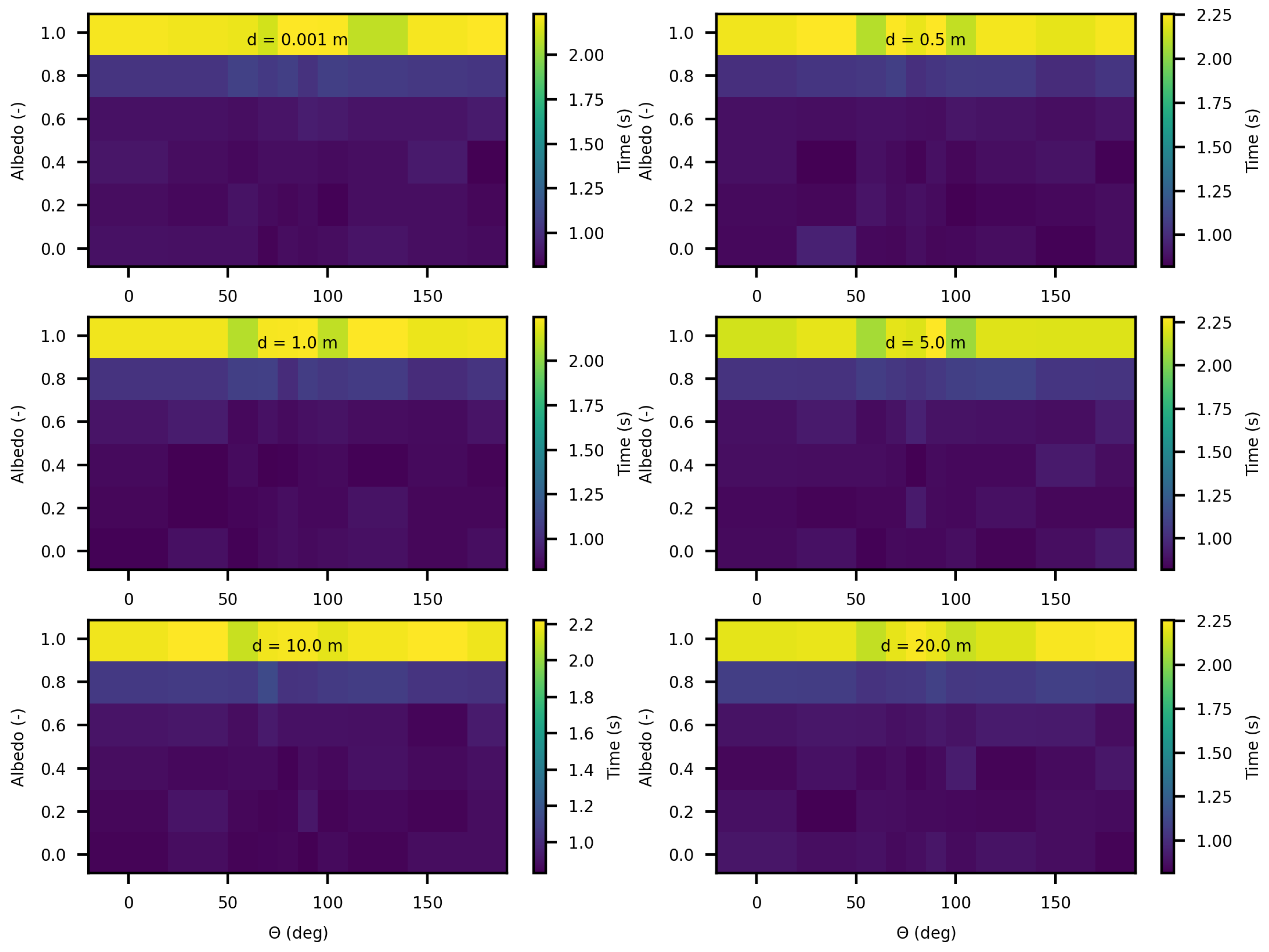
Figure 22.
Execution time variation for SWEET for luminance computing, done with photons for varying optical parameters, for asymetry phase parameter: g = 0.9, each figure corresponds to a distance as in this matrix: m.
Figure 22.
Execution time variation for SWEET for luminance computing, done with photons for varying optical parameters, for asymetry phase parameter: g = 0.9, each figure corresponds to a distance as in this matrix: m.
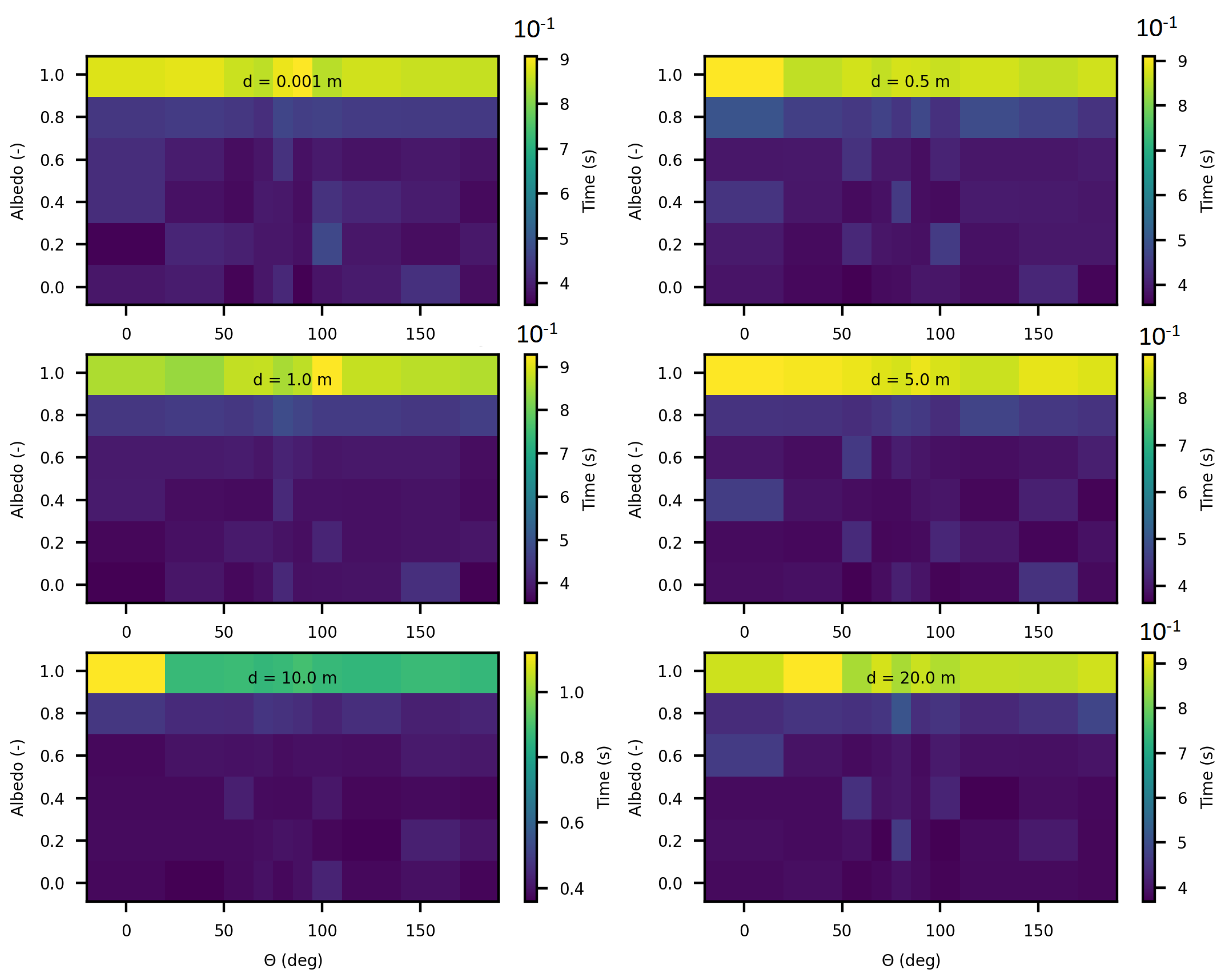
Figure 23.
Execution time variation for MS for luminance computing, done with photons for varying optical parameters, for asymetry phase parameter: g = 0.9, each figure corresponds to a distance as in this matrix: m.
Figure 23.
Execution time variation for MS for luminance computing, done with photons for varying optical parameters, for asymetry phase parameter: g = 0.9, each figure corresponds to a distance as in this matrix: m.
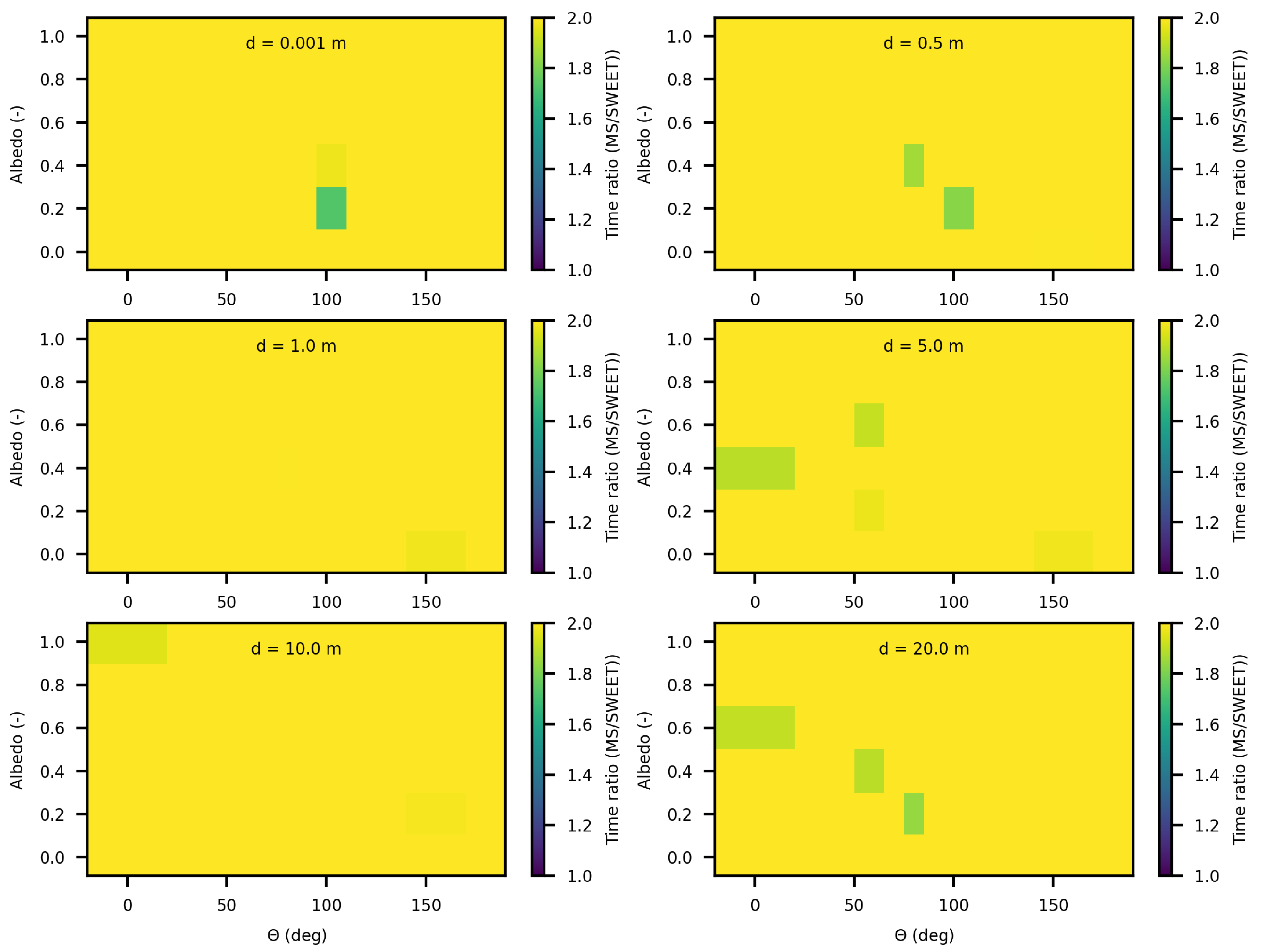
Figure 24.
Execution time ratio variation of MS to SWEET for luminance computing, done with photons for varying optical parameters, for asymetry phase parameter: g = 0.9, each figure corresponds to a distance as in this matrix: m.
Figure 24.
Execution time ratio variation of MS to SWEET for luminance computing, done with photons for varying optical parameters, for asymetry phase parameter: g = 0.9, each figure corresponds to a distance as in this matrix: m.
Table 1.
Optical thickness obtained for different limit cases in the automotive context, with the fog analysis.
Table 1.
Optical thickness obtained for different limit cases in the automotive context, with the fog analysis.
| | Light fog on Highway | Dense Fog on Road | Very Heavy Fog on Roa | PAVIN Fog and Rain Facility Maximum Capability |
|---|
| Vehicle speed (km/h) | 130 | 50 | 50 | |
| Minimal detection distance (m) | 108 | 42 | 42 | 50 |
| MOR (m) | 400 | 50 | 10 | 8 |
| Optical thickness | 0.8 | 2.5 | 12.5 | 18.8 |
Table 2.
Optical thickness obtained for different limit cases in the automotive context, with sensors analysis.
Table 2.
Optical thickness obtained for different limit cases in the automotive context, with sensors analysis.
| | | Camera vs. Sun | Camera vs. Headlamps | Cerema’s Sensors Capabilities |
|---|
| Sensor | Minimal radiance | 6.51 × 10−3 cd/m2 | 6.51 × 10−3 cd/m2 | 3.25 × 10−7 W/sr/cm2 |
| Optical aperture | 70° | 70° | 4° |
| Minimal irradiance | 5.00 × 10−2 Lm/m2 | 5.00 × 10−2 Lm/m2 | 1.43 × 10−7 W/cm2 |
| Source | Maximal Power | | 2.15 × 105 cd | 4.00 × 103 Lm |
| Surface | | 6.40 × 10−3 m2 | 1.30 × 103 cm2 |
| Maximal irradiance | 2.00 × 105 lux | | 4.52 × 10−3 W/cm2 |
| Maximal radiance | 6.37 × 104 cd/m2 | 3.36 × 107 cd/m2 | |
| Ratio sensor / source | 1.02 × 10−7 | 1.94 × 10−10 | 3.15 × 10−5 |
| Resultant optical thickness | 16.1 | 22.4 | 10.4 |
Table 3.
Summary of BSDF models in SWEET.
Table 3.
Summary of BSDF models in SWEET.
| Model | Common Name | Microfacet Model (If Applicable) |
|---|
| Diffuse | Lambertian | N/A |
| Smooth Dielectric | Glass, Water | N/A |
| Thin Dielectric | Soap Bubble, Thin Film | N/A |
| Rough Dielectric | Frosted Glass, Rough Plastic | Beckmann, GGX |
| Smooth Conductor | Polished Metal | N/A |
| Rough Conductor | Brushed Metal | Beckmann, GGX |
| Plastic | Glossy Plastic | N/A |
Table 4.
Comparing fluence with SWEET and Steven’s Monte Carlo code for m−1, g = and a set of distances, the mean values of SWEET and MC0 and the confidence intervals are shown and the relative differences, fluence values are in (W/m2), red-colored values are for relatively high discrepancies, - are for points where MC0 gives a null value.
Table 4.
Comparing fluence with SWEET and Steven’s Monte Carlo code for m−1, g = and a set of distances, the mean values of SWEET and MC0 and the confidence intervals are shown and the relative differences, fluence values are in (W/m2), red-colored values are for relatively high discrepancies, - are for points where MC0 gives a null value.
| Distance (m) | g = 0.0 | g = 0.9 |
|---|
|
Mean SWEET
|
Mean MC0
|
Inf SWEET
|
Sup SWEET
|
Rel Error
|
Mean SWEET
|
Mean MC0
|
Inf SWEET
|
Sup SWEET
|
Rel Error
|
|---|
| = 0.01 m−1 |
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | - | | | | | - |
| 30.0 | | | | | - | | | | | - |
| 40.0 | | | | | - | | | | | - |
| = 0.5 m−1 |
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | - | | | | | |
| 30.0 | | | | | - | | | | | - |
| 40.0 | | | | | - | | | | | - |
| = 0.99 m−1 |
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | | | | | | |
| 30.0 | | | | | | | | | | |
| 40.0 | | | | | | | | | | |
Table 5.
Comparing luminance with SWEET and MS for a point light source and for m−1, g = and a set of distances, the mean values of SWEET and MS and the confidence intervals are shown and the relative differences, luminance values are in (W/m2/sr), red-colored values are for relatively high discrepancies.
Table 5.
Comparing luminance with SWEET and MS for a point light source and for m−1, g = and a set of distances, the mean values of SWEET and MS and the confidence intervals are shown and the relative differences, luminance values are in (W/m2/sr), red-colored values are for relatively high discrepancies.
| Distance (m) | g = 0.0 | g = 0.9 |
|---|
|
Mean SWEET
|
Mean MS
|
Inf SWEET
|
Sup SWEET
|
Rel Error
|
Mean SWEET
|
Mean MS
|
Inf SWEET
|
Sup SWEET
|
Rel Error
|
|---|
| = 0.01 m−1 |
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | | | | | | |
| m−1 |
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | | | | | | |
| m−1 |
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | | | | | | |
Table 6.
Comparing luminance with SWEET and MS for a rectangular light source and for m−1, g = and a set of distances, the mean values of SWEET and MS and the confidence intervals are shown and the relative differences, luminance values are in (W/m2sr), red-colored values are for relatively high discrepancies, - are for points where MS gives null values.
Table 6.
Comparing luminance with SWEET and MS for a rectangular light source and for m−1, g = and a set of distances, the mean values of SWEET and MS and the confidence intervals are shown and the relative differences, luminance values are in (W/m2sr), red-colored values are for relatively high discrepancies, - are for points where MS gives null values.
| Distance (m) | g = 0.0 | g = 0.9 |
|---|
|
Mean SWEET
|
Mean MC0
|
Inf SWEET
|
Sup SWEET
|
Rel Error
|
Mean SWEET
|
Mean MS
|
Inf SWEET
|
Sup SWEET
|
Rel Error
|
|---|
| m−1 |
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | - | | | | | |
| m−1 |
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | - | | | | | |
| m−1 |
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | - | | | | | |
Table 7.
Comparison of luminance from SWEET and MS for a configuration with two point light sources and for m−1, g = and a set of distances, the mean values of SWEET and MS and the confidence intervals are shown and the relative differences, luminance values are in (W/m2/sr), red-colored values are for relatively high discrepancies.
Table 7.
Comparison of luminance from SWEET and MS for a configuration with two point light sources and for m−1, g = and a set of distances, the mean values of SWEET and MS and the confidence intervals are shown and the relative differences, luminance values are in (W/m2/sr), red-colored values are for relatively high discrepancies.
| Distance (m) | g = 0.0 | g = 0.9 |
|---|
|
Mean SWEET
|
Mean MC0
|
Inf SWEET
|
Sup SWEET
|
Rel Error
|
Mean SWEET
|
Mean MC0
|
Inf SWEET
|
Sup SWEET
|
Rel Error
|
|---|
|
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | | | | | | |
|
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | | | | | | |
|
| 0.001 | | | | | | | | | | |
| 0.5 | | | | | | | | | | |
| 1.0 | | | | | | | | | | |
| 5.0 | | | | | | | | | | |
| 10.0 | | | | | | | | | | |
| 20.0 | | | | | | | | | | |