Review of Multivalent Metal Ion Transport in Inorganic and Solid Polymer Electrolytes
Abstract
1. Introduction
2. Methods for Evaluating Ion Transport
3. Overview of Ion Transport Models
3.1. Solid Inorganic Ion Conductors
3.2. Solid Polymer Electrolytes
4. Ion Transport of Multivalent Cations in Solid Electrolytes
Solid Inorganic Electrolytes
5. Solid Polymer Electrolytes
6. Applications in Batteries
7. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, H.; Sheng, L.; Yasin, G.; Wang, L.; Xu, H.; He, X. Reviewing the current status and development of polymer electrolytes for solid-state lithium batteries. Energy Storage Mater. 2020, 33, 188–215. [Google Scholar] [CrossRef]
- Jia, M.; Zhao, N.; Huo, H.; Guo, X. Comprehensive Investigation into Garnet Electrolytes Toward Application-Oriented Solid Lithium Batteries. Electrochem. Energy Rev. 2020, 3, 656–689. [Google Scholar] [CrossRef]
- Randau, S.; Weber, D.A.; Kötz, O.; Koerver, R.; Braun, P.; Weber, A.; Ivers-Tiffée, E.; Adermann, T.; Kulisch, J.; Zeier, W.G.; et al. Benchmarking the performance of all-solid-state lithium batteries. Nat. Energy 2020, 5, 259–270. [Google Scholar] [CrossRef]
- Wanger, T.C. The Lithium future—Resources, recycling, and the environment. Conserv. Lett. 2011, 4, 202–206. [Google Scholar] [CrossRef]
- Watari, T.; Nansai, K.; Nakajima, K. Review of critical metal dynamics to 2050 for 48 elements. Resour. Conserv. Recycl. 2020, 155, 104669. [Google Scholar] [CrossRef]
- Agusdinata, D.B.; Liu, W.; Eakin, H.; Romero, H. Socio-environmental impacts of lithium mineral extraction: Towards a research agenda. Environ. Res. Lett. 2018, 13, 123001. [Google Scholar] [CrossRef]
- Li, Z.; Fuhr, O.; Fichtner, M.; Zhao-Karger, Z. Towards stable and efficient electrolytes for room-temperature rechargeable calcium batteries. Energy Environ. Sci. 2019, 12, 3496–3501. [Google Scholar] [CrossRef]
- Park, B.; Schaefer, J.L. Review—Polymer Electrolytes for Magnesium Batteries: Forging Away from Analogs of Lithium Polymer Electrolytes and Towards the Rechargeable Magnesium Metal Polymer Battery. J. Electrochem. Soc. 2020, 167, 070545. [Google Scholar] [CrossRef]
- Mainar, A.R.; Iruin, E.; Colmenares, L.C.; Kvasha, A.; de Meatza, I.; Bengoechea, M.; Leonet, O.; Boyano, I.; Zhang, Z.; Blazquez, J.A. An overview of progress in electrolytes for secondary zinc-air batteries and other storage systems based on zinc. J. Energy Storage 2018, 15, 304–328. [Google Scholar] [CrossRef]
- Hu, Z.; Zhang, H.; Wang, H.; Zhang, F.; Li, Q.; Li, H. Nonaqueous Aluminum Ion Batteries: Recent Progress and Prospects. ACS Mater. Lett. 2020, 2, 887–904. [Google Scholar] [CrossRef]
- Hwang, J.Y.; Myung, S.T.; Sun, Y.K. Sodium-ion batteries: Present and future. Chem. Soc. Rev. 2017, 46, 3529–3614. [Google Scholar] [CrossRef] [PubMed]
- Delmas, C. Sodium and Sodium-Ion Batteries: 50 Years of Research. Adv. Energy Mater. 2018, 8, 1703137. [Google Scholar] [CrossRef]
- Chayambuka, K.; Mulder, G.; Danilov, D.L.; Notten, P.H.L. Sodium-Ion Battery Materials and Electrochemical Properties Reviewed. Adv. Energy Mater. 2018, 8, 1800079. [Google Scholar] [CrossRef]
- Chen, L.; Fiore, M.; Wang, J.E.; Ruffo, R.; Kim, D.K.; Longoni, G. Readiness Level of Sodium-Ion Battery Technology: A Materials Review. Adv. Sustain. Syst. 2018, 2, 1700153. [Google Scholar] [CrossRef]
- Skundin, A.M.; Kulova, T.L.; Yaroslavtsev, A.B. Sodium-Ion Batteries (a Review). Russ. J. Electrochem. 2018, 54, 113–152. [Google Scholar] [CrossRef]
- Li, L.; Zheng, Y.; Zhang, S.; Yang, J.; Shao, Z.; Guo, Z. Recent progress on sodium ion batteries: Potential high-performance anodes. Energy Environ. Sci. 2018, 11, 2310–2340. [Google Scholar] [CrossRef]
- Vaalma, C.; Buchholz, D.; Weil, M.; Passerini, S. A cost and resource analysis of sodium-ion batteries. Nat. Rev. Mater. 2018, 3, 18013. [Google Scholar] [CrossRef]
- Chen, M.; Zhang, Y.; Xing, G.; Tang, Y. Building High Power Density of Sodium-Ion Batteries: Importance of Multidimensional Diffusion Pathways in Cathode Materials. Front. Chem. 2020, 8, 152. [Google Scholar] [CrossRef]
- Xu, J.; Dou, S.; Cui, X.; Liu, W.; Zhang, Z.; Deng, Y.; Hu, W.; Chen, Y. Potassium-based electrochemical energy storage devices: Development status and future prospect. Energy Storage Mater. 2021, 34, 85–106. [Google Scholar] [CrossRef]
- Komaba, S. Sodium-driven Rechargeable Batteries: An Effort towards Future Energy Storage. Chem. Lett. 2020, 49, 1507–1516. [Google Scholar] [CrossRef]
- Yao, Q.; Zhu, C. Advanced Post-Potassium-Ion Batteries as Emerging Potassium-Based Alternatives for Energy Storage. Adv. Funct. Mater. 2020, 30, 2005209. [Google Scholar] [CrossRef]
- Yi, Y.; Zhao, Y.; Sun, J. Promise and reality of practical potassium-based energy storage systems. Eng. Rep. 2020, 2, e12328. [Google Scholar] [CrossRef]
- Liu, Y.; Gao, C.; Dai, L.; Deng, Q.; Wang, L.; Luo, J.; Liu, S.; Hu, N. The Features and Progress of Electrolyte for Potassium Ion Batteries. Small 2020, 16, 2004096. [Google Scholar] [CrossRef] [PubMed]
- Desai, A.V.; Morris, R.E.; Armstrong, A.R. Advances in Organic Anode Materials for Na-/K-Ion Rechargeable Batteries. ChemSusChem 2020, 13, 4866–4884. [Google Scholar] [CrossRef] [PubMed]
- Qian, X.; Gu, N.; Cheng, Z.; Yang, X.; Wang, E.; Dong, S. Methods to study the ionic conductivity of polymeric electrolytes using ac impedance spectroscopy. J. Solid State Electrochem. 2001, 6, 8–15. [Google Scholar] [CrossRef]
- Irvine, J.T.S.; Sinclair, D.C.; West, A.R. Electroceramics: Characterization by Impedance Spectroscopy. Adv. Mater. 1990, 2, 132–138. [Google Scholar] [CrossRef]
- Zugmann, S.; Fleischmann, M.; Amereller, M.; Gschwind, R.M.; Wiemhöfer, H.D.; Gores, H.J. Measurement of transference numbers for lithium ion electrolytes via four different methods, a comparative study. Electrochim. Acta 2011, 56, 3926–3933. [Google Scholar] [CrossRef]
- Vest, R.W.; Tallan, N.M. High–Temperature Transference Number Determinations by Polarization Measurements. J. Appl. Phys. 1965, 36, 543–547. [Google Scholar] [CrossRef]
- Evans, J.; Vincent, C.A.; Bruce, P.G. Electrochemical measurement of transference numbers in polymer electrolytes. Polymer 1987, 28, 2324–2328. [Google Scholar] [CrossRef]
- Bruce, P.G.; Vincent, C.A. Polymer electrolytes. J. Chem. Soc. Faraday Trans. 1993, 89, 3187–3203. [Google Scholar] [CrossRef]
- Balsara, N.P.; Newman, J. Relationship between Steady-State Current in Symmetric Cells and Transference Number of Electrolytes Comprising Univalent and Multivalent Ions. J. Electrochem. Soc. 2015, 162, A2720–A2722. [Google Scholar] [CrossRef]
- Haile, S.M.; Staneff, G.; Ryu, K.H. Non-stoichiometry, grain boundary transport and chemical stability of proton conducting perovskites. J. Mater. Sci. 2001, 36, 1149–1160. [Google Scholar] [CrossRef]
- Fleig, J.; Maier, J. Finite-Element Calculations on the Impedance of Electroceramics with Highly Resistive Grain Boundaries: I, Laterally Inhomogeneous Grain Boundaries. J. Am. Ceram. Soc. 1999, 82, 3485–3493. [Google Scholar] [CrossRef]
- Valoen, L.O.; Reimers, J.N. Transport Properties of LiPF6–Based Li-Ion Battery Electrolytes. J. Electrochem. Soc. 2005, 152, A882. [Google Scholar] [CrossRef]
- Gering, K.L. Prediction of Electrolyte Conductivity: Results from a Generalized Molecular Model Based on Ion Solvation and a Chemical Physics Framework. Electrochim. Acta 2017, 225, 175–189. [Google Scholar] [CrossRef]
- Fong, K.D.; Self, J.; Diederichsen, K.M.; Wood, B.M.; McCloskey, B.D.; Persson, K.A. Ion Transport and the True Transference Number in Nonaqueous Polyelectrolyte Solutions for Lithium Ion Batteries. ACS Cent. Sci. 2019, 5, 1250–1260. [Google Scholar] [CrossRef]
- Aono, H.; Sugimoto, E.; Sadaoka, Y.; Imanaka, N.; ya Adachi, G. Ionic Conductivity of Solid Electrolytes Based on Lithium Titanium Phosphate. J. Electrochem. Soc. 1990, 137, 1023–1027. [Google Scholar] [CrossRef]
- Murugan, R.; Thangadurai, V.; Weppner, W. Fast Lithium Ion Conduction in Garnet-Type Li7La3Zr2O12. Angew. Chem. Int. Ed. 2007, 46, 7778–7781. [Google Scholar] [CrossRef]
- Kato, Y.; Hori, S.; Saito, T.; Suzuki, K.; Hirayama, M.; Mitsui, A.; Yonemura, M.; Iba, H.; Kanno, R. High-power all-solid-state batteries using sulfide superionic conductors. Nat. Energy 2016, 1, 16030. [Google Scholar] [CrossRef]
- Zhang, H.; Li, C.; Piszcz, M.; Coya, E.; Rojo, T.; Rodriguez-Martinez, L.M.; Armand, M.; Zhou, Z. Single lithium-ion conducting solid polymer electrolytes: Advances and perspectives. Chem. Soc. Rev. 2017, 46, 797–815. [Google Scholar] [CrossRef]
- Klein, R.J.; Welna, D.T.; Weikel, A.L.; Allcock, H.R.; Runt, J. Counterion Effects on Ion Mobility and Mobile Ion Concentration of Doped Polyphosphazene and Polyphosphazene Ionomers. Macromolecules 2007, 40, 3990–3995. [Google Scholar] [CrossRef]
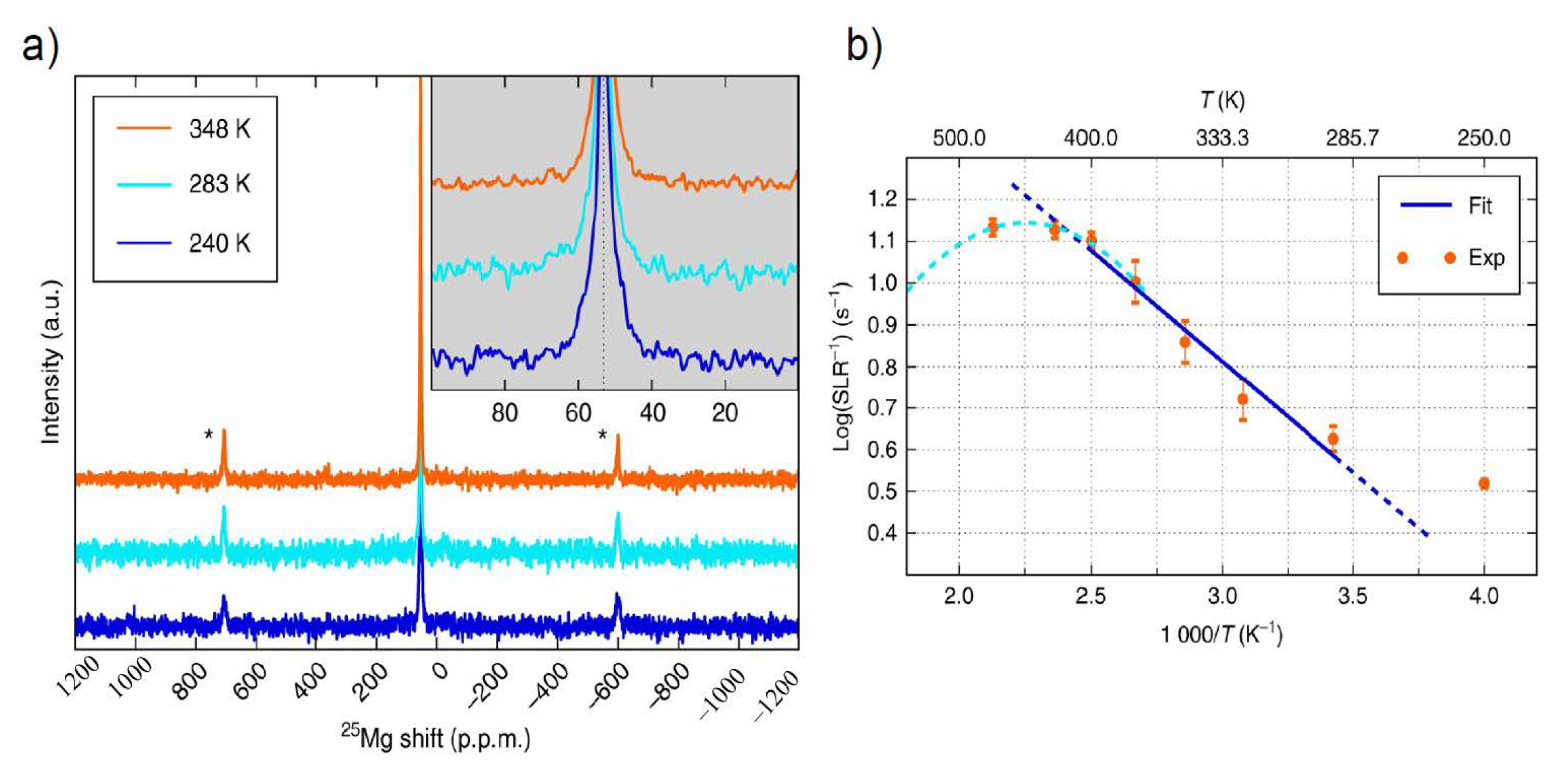
- Muñoz, S.; Greenbaum, S. Review of Recent Nuclear Magnetic Resonance Studies of Ion Transport in Polymer Electrolytes. Membranes 2018, 8, 120. [Google Scholar] [CrossRef] [PubMed]
- Berman, M.; Greenbaum, S. NMR Studies of Solvent-Free Ceramic Composite Polymer Electrolytes—A Brief Review. Membranes 2015, 5, 915–923. [Google Scholar] [CrossRef] [PubMed]
- Gainaru, C.; Stacy, E.W.; Bocharova, V.; Gobet, M.; Holt, A.P.; Saito, T.; Greenbaum, S.; Sokolov, A.P. Mechanism of Conductivity Relaxation in Liquid and Polymeric Electrolytes: Direct Link between Conductivity and Diffusivity. J. Phys. Chem. B 2016, 120, 11074–11083. [Google Scholar] [CrossRef]
- Krachkovskiy, S.; Trudeau, M.L.; Zaghib, K. Application of Magnetic Resonance Techniques to the In Situ Characterization of Li-Ion Batteries: A Review. Materials 2020, 13, 1694. [Google Scholar] [CrossRef]
- Morales, D.J.; Greenbaum, S. NMR Investigations of Crystalline and Glassy Solid Electrolytes for Lithium Batteries: A Brief Review. Int. J. Mol. Sci. 2020, 21, 3402. [Google Scholar] [CrossRef]
- Jadhav, A.L.; Xu, J.H.; Messinger, R.J. Quantitative Molecular-Level Understanding of Electrochemical Aluminum-Ion Intercalation into a Crystalline Battery Electrode. ACS Energy Lett. 2020, 5, 2842–2848. [Google Scholar] [CrossRef]
- Graham, T.R.; Han, K.S.; Dembowski, M.; Krzysko, A.J.; Zhang, X.; Hu, J.; Clark, S.B.; Clark, A.E.; Schenter, G.K.; Pearce, C.I.; et al. 27Al Pulsed Field Gradient, Diffusion—NMR Spectroscopy of Solvation Dynamics and Ion Pairing in Alkaline Aluminate Solutions. J. Phys. Chem. B 2018, 122, 10907–10912. [Google Scholar] [CrossRef]
- Sinnaeve, D. The Stejskal–Tanner equation generalized for any gradient shape—An overview of most pulse sequences measuring free diffusion. Concepts Magn. Reson. Part A 2012, 40, 39–65. [Google Scholar] [CrossRef]
- Volgmann, K.; Epp, V.; Langer, J.; Stanje, B.; Heine, J.; Nakhal, S.; Lerch, M.; Wilkening, M.; Heitjans, P. Solid-State NMR to Study Translational Li Ion Dynamics in Solids with Low-Dimensional Diffusion Pathways. Z. Phys. Chem. 2017, 231, 1215–1241. [Google Scholar] [CrossRef]
- Smiley, D.L.; Goward, G.R. Solid-state NMR studies of chemical exchange in ion conductors for alternative energy applications. Concepts Magn. Reson. Part A 2016, 45, e21419. [Google Scholar] [CrossRef]
- Messinger, R.J. (Invited) Molecular-Level Understanding of Ion Intercalation Mechanisms in Aluminum and Zinc Battery Electrodes Revealed By Solid-State NMR Spectroscopy. In Proceedings of the 235th ECS Meeting Abstracts, Dallas, TX, USA, 26–30 May 2019. [Google Scholar] [CrossRef]
- Bottke, P.; Freude, D.; Wilkening, M. Ultra slow Li Exchange Processes in Diamagnetic Li2ZrO3 As Monitored by EXSY NMR. J. Phys. Chem. C 2013, 117, 8114–8119. [Google Scholar] [CrossRef]
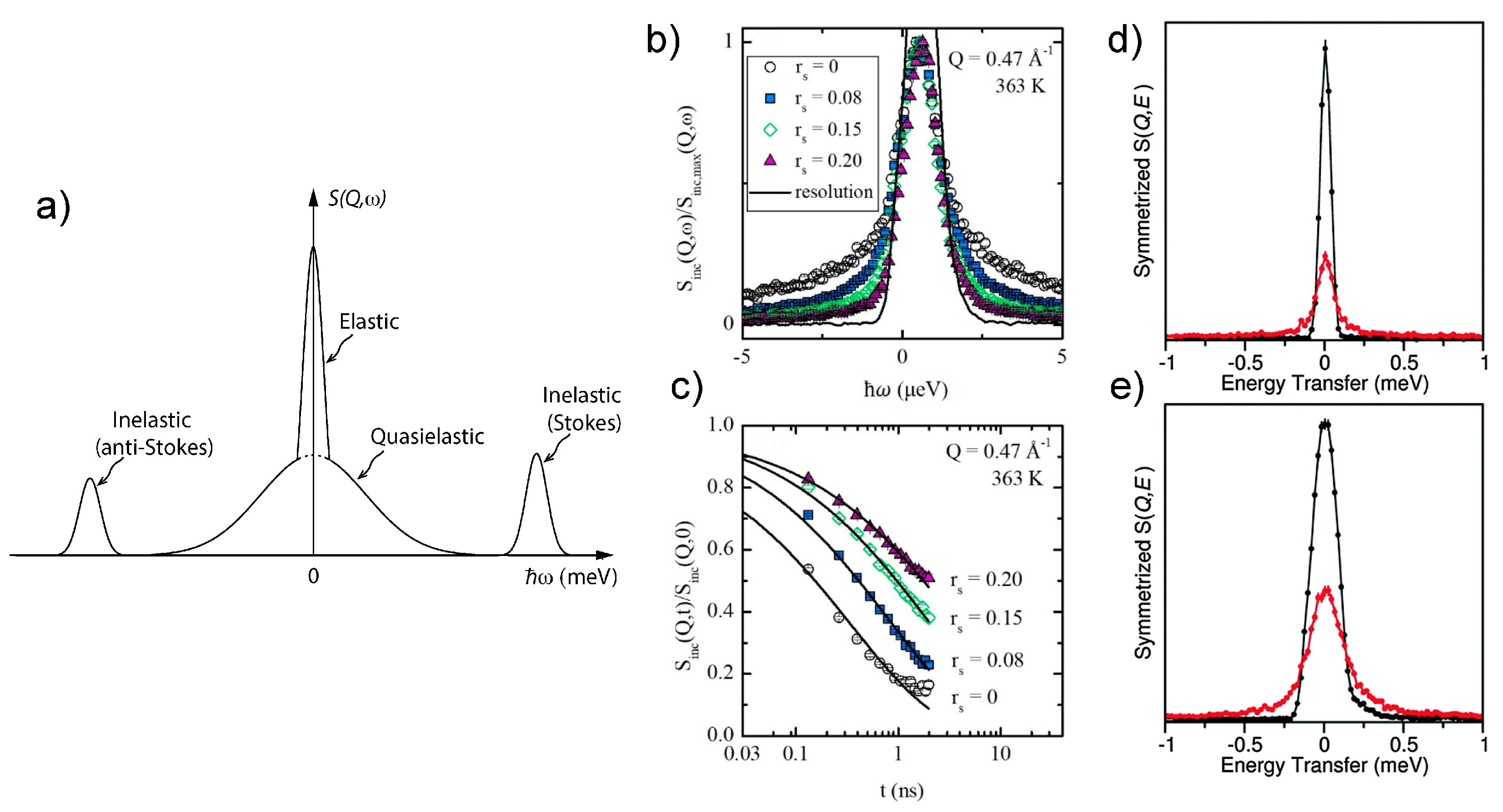
- Bée, M. Localized and long-range diffusion in condensed matter: State of the art of QENS studies and future prospects. Chem. Phys. 2003, 292, 121–141. [Google Scholar] [CrossRef]
- Karlsson, M. Proton dynamics in oxides: Insight into the mechanics of proton conduction from quasielastic neutron scattering. Phys. Chem. Chem. Phys. 2015, 17, 26–38. [Google Scholar] [CrossRef]
- Tang, W.S.; Unemoto, A.; Zhou, W.; Stavila, V.; Matsuo, M.; Wu, H.; Orimo, S.i.; Udovic, T.J. Unparalleled lithium and sodium superionic conduction in solid electrolytes with large monovalent cage-like anions. Energy Environ. Sci. 2015, 8, 3637–3645. [Google Scholar] [CrossRef]
- Dimitrievska, M.; Shea, P.; Kweon, K.E.; Bercx, M.; Varley, J.B.; Tang, W.S.; Skripov, A.V.; Stavila, V.; Udovic, T.J.; Wood, B.C. Carbon Incorporation and Anion Dynamics as Synergistic Drivers for Ultrafast Diffusion in Superionic LiCB11H12 and NaCB11H12. Adv. Energy Mater. 2018, 8, 1703422. [Google Scholar] [CrossRef]
- Duchêne, L.; Lunghammer, S.; Burankova, T.; Liao, W.C.; Embs, J.P.; Copéret, C.; Wilkening, H.M.R.; Remhof, A.; Hagemann, H.; Battaglia, C. Ionic Conduction Mechanism in the Na2(B12H12)0.5(B10H10)0.5 closo-Borate Solid-State Electrolyte: Interplay of Disorder and Ion–Ion Interactions. Chem. Mater. 2019, 31, 3449–3460. [Google Scholar] [CrossRef]
- Mao, G.; Perea, R.F.; Howells, W.S.; Price, D.L.; Saboungi, M.L. Relaxation in polymer electrolytes on the nanosecond timescale. Nature 2000, 405, 163–165. [Google Scholar] [CrossRef]
- Senses, E.; Tyagi, M.; Natarajan, B.; Narayanan, S.; Faraone, A. Chain dynamics and nanoparticle motion in attractive polymer nanocomposites subjected to large deformations. Soft Matter 2017, 13, 7922–7929. [Google Scholar] [CrossRef]
- Mongcopa, K.I.S.; Tyagi, M.; Mailoa, J.P.; Samsonidze, G.; Kozinsky, B.; Mullin, S.A.; Gribble, D.A.; Watanabe, H.; Balsara, N.P. Relationship between Segmental Dynamics Measured by Quasi-Elastic Neutron Scattering and Conductivity in Polymer Electrolytes. ACS Macro Lett. 2018, 7, 504–508. [Google Scholar] [CrossRef]
- Mongcopa, K.I.S.; Gribble, D.A.; Loo, W.S.; Tyagi, M.; Mullin, S.A.; Balsara, N.P. Segmental Dynamics Measured by Quasi-Elastic Neutron Scattering and Ion Transport in Chemically Distinct Polymer Electrolytes. Macromolecules 2020, 53, 2406–2411. [Google Scholar] [CrossRef]
- Lukichev, A. Physical meaning of the stretched exponential Kohlrausch function. Phys. Lett. A 2019, 383, 2983–2987. [Google Scholar] [CrossRef]
- Köhler, J.; Imanaka, N.; Adachi, G.Y. Multivalent Cationic Conduction in Crystalline Solids. Chem. Mater. 1998, 10, 3790–3812. [Google Scholar] [CrossRef]
- Schottky, W.; Wagner, C. Theory of ordered mixed phases, I. Z. Phys. Chem. B 1931, 11, 163–210. [Google Scholar]
- Frenkel, J. Über die Wärmebewegung in festen und flüssigen Körpern. Z. Phys. 1926, 35, 652–669. [Google Scholar] [CrossRef]
- Mehrer, H. Diffusion In Solids: Fundementals, Methods, Materials, Diffusion-Controlled Processes; Springer: Berlin/Heidelberg, Germany, 2007; Volume 1. [Google Scholar]
- He, X.; Zhu, Y.; Mo, Y. Origin of fast ion diffusion in super-ionic conductors. Nat. Commun. 2017, 8, 15893. [Google Scholar] [CrossRef]
- Whittingham, M.S.; Huggins, R.A. Transport Properties of Silver Beta Alumina. J. Electrochem. Soc. 1971, 118, 1. [Google Scholar] [CrossRef]
- Wang, J.C.; Gaffari, M.; Choi, S. On the ionic conduction in β-alumina: Potential energy curves and conduction mechanism. J. Chem. Phys. 1975, 63, 772–778. [Google Scholar] [CrossRef]
- Li, H.; Okamoto, N.L.; Hatakeyama, T.; Kumagai, Y.; Oba, F.; Ichitsubo, T. Fast Diffusion of Multivalent Ions Facilitated by Concerted Interactions in Dual-Ion Battery Systems. Adv. Energy Mater. 2018, 8, 1801475. [Google Scholar] [CrossRef]
- Jalem, R.; Yamamoto, Y.; Shiiba, H.; Nakayama, M.; Munakata, H.; Kasuga, T.; Kanamura, K. Concerted Migration Mechanism in the Li Ion Dynamics of Garnet-Type Li7La3Zr2O12. Chem. Mater. 2013, 25, 425–430. [Google Scholar] [CrossRef]
- Cuan, J.; Zhou, Y.; Zhou, T.; Ling, S.; Rui, K.; Guo, Z.; Liu, H.; Yu, X. Borohydride-Scaffolded Li/Na/Mg Fast Ionic Conductors for Promising Solid-State Electrolytes. Adv. Mater. 2019, 31, 1803533. [Google Scholar] [CrossRef] [PubMed]
- Zhao, W.; Yi, J.; He, P.; Zhou, H. Solid-State Electrolytes for Lithium-Ion Batteries: Fundamentals, Challenges and Perspectives. Electrochem. Energy Rev. 2019, 2, 574–605. [Google Scholar] [CrossRef]
- Chen, F.; Cheng, S.; Liu, J.B.; Li, S.; Ouyang, W.; Yuan, K.; Liu, B. Lithium superionic conduction in α-Li10P4N10: A promising inorganic solid electrolyte candidate. J. Power Sources 2020, 477, 228744. [Google Scholar] [CrossRef]
- Zou, Z.; Ma, N.; Wang, A.; Ran, Y.; Song, T.; Jiao, Y.; Liu, J.; Zhou, H.; Shi, W.; He, B.; et al. Relationships Between Na+ Distribution, Concerted Migration, and Diffusion Properties in Rhombohedral NASICON. Adv. Energy Mater. 2020, 10, 2001486. [Google Scholar] [CrossRef]
- Hu, P.; Zou, Z.; Sun, X.; Wang, D.; Ma, J.; Kong, Q.; Xiao, D.; Gu, L.; Zhou, X.; Zhao, J.; et al. Uncovering the Potential of M1-Site-Activated NASICON Cathodes for Zn-Ion Batteries. Adv. Mater. 2020, 32, 1907526. [Google Scholar] [CrossRef]
- Funke, K. Jump relaxation in solid electrolytes. Prog. Solid State Chem. 1993, 22, 111–195. [Google Scholar] [CrossRef]
- Kvist, A. Notizen: The Electrical Conductivity of Solid and Molten 6Li2SO4 and 7Li2SO4. Z. Naturforsch. A 1966, 21, 487. [Google Scholar] [CrossRef]
- Van Gool, W. Fast ion transport in solids, solid state batteries and devices. In Proceedings of the NATO-Sponsored Advanced Study Institute of Fast Ion Transport in Solids, Solid State Batteries and Devices, Belgirate, Italy, 5–15 September 1972. [Google Scholar]
- Wilmer, D.; Feldmann, H.; Lechner, R.E.; Combet, J. Correlated motion of anions and cations in fast cation conducting rotor phases. Solid State Ion. 2004, 175, 463–466. [Google Scholar] [CrossRef]
- Wilmer, D.; Meyer, H.W. Crystalline Cation Conductors with Rotational Anion Disorder: Results of Quasielastic Neutron Scattering Experiments on Orthophosphates. Z. Phys. Chem. 2009, 223, 1341–1357. [Google Scholar] [CrossRef]
- Burmakin, E.I.; Shekhtman, G.S. On ion transport mechanism in K+-conducting solid electrolytes based on K3PO4. Solid State Ion. 2014, 265, 46–48. [Google Scholar] [CrossRef]
- Smith, J.G.; Siegel, D.J. Low–temperature paddlewheel effect in glassy solid electrolytes. Nat. Commun. 2020, 11, 1483. [Google Scholar] [CrossRef] [PubMed]
- Zhou, L.; Assoud, A.; Shyamsunder, A.; Huq, A.; Zhang, Q.; Hartmann, P.; Kulisch, J.; Nazar, L.F. An Entropically Stabilized Fast-Ion Conductor: Li3.25[Si0.25P0.75]S4. Chem. Mater. 2019, 31, 7801–7811. [Google Scholar] [CrossRef]
- Secco, E.A. Electrical conductivity measurements to test for rotating sulfate ions in fast ion conductors. Phys. Status Solidi (A) 1985, 88, K75–K77. [Google Scholar] [CrossRef]
- Lundén, A. Evidence for and against the paddle-wheel mechanism of ion transport in superionic sulphate phases. Solid State Commun. 1988, 65, 1237–1240. [Google Scholar] [CrossRef]
- Lundén, A. Enhancement of cation mobility in some sulphate phases due to a paddle-wheel mechanism. Solid State Ion. 1988, 28–30, 163–167. [Google Scholar] [CrossRef]
- Lunde’n, A. Paddle-wheel versus percolation model, revisited. Solid State Ion. 1994, 68, 77–80. [Google Scholar] [CrossRef]
- Karlsson, L.; McGreevy, R.L. Mechanisms of ionic conduction in Li2SO4 and LiNaSO4: Paddle wheel or percolation? Solid State Ion. 1995, 76, 301–308. [Google Scholar] [CrossRef]
- Wilmer, D.; Funke, K.; Witschas, M.; Banhatti, R.D.; Jansen, M.; Korus, G.; Fitter, J.; Lechner, R.E. Anion reorientation in an ion conducting plastic crystal –coherent quasielastic neutron scattering from sodium ortho-phosphate. Phys. B Condens. Matter 1999, 266, 60–68. [Google Scholar] [CrossRef]
- Skripov, A.V.; Babanova, O.A.; Soloninin, A.V.; Stavila, V.; Verdal, N.; Udovic, T.J.; Rush, J.J. Nuclear Magnetic Resonance Study of Atomic Motion in A2B12H12 (A = Na, K, Rb, Cs): Anion Reorientations and Na+ Mobility. J. Phys. Chem. C 2013, 117, 25961–25968. [Google Scholar] [CrossRef]
- Verdal, N.; Udovic, T.J.; Stavila, V.; Tang, W.S.; Rush, J.J.; Skripov, A.V. Anion Reorientations in the Superionic Conducting Phase of Na2B12H12. J. Phys. Chem. C 2014, 118, 17483–17489. [Google Scholar] [CrossRef]
- Kweon, K.E.; Varley, J.B.; Shea, P.; Adelstein, N.; Mehta, P.; Heo, T.W.; Udovic, T.J.; Stavila, V.; Wood, B.C. Structural, Chemical, and Dynamical Frustration: Origins of Superionic Conductivity in closo-Borate Solid Electrolytes. Chem. Mater. 2017, 29, 9142–9153. [Google Scholar] [CrossRef]
- Heere, M.; Hansen, A.L.; Payandeh, S.; Aslan, N.; Gizer, G.; Sørby, M.H.; Hauback, B.C.; Pistidda, C.; Dornheim, M.; Lohstroh, W. Dynamics of porous and amorphous magnesium borohydride to understand solid state Mg-ion-conductors. Sci. Rep. 2020, 10, 9080. [Google Scholar] [CrossRef] [PubMed]
- Müller, W.; Jansen, M. (CN)ONa3, Kristallstruktur und Natriumionenleitfähigkeit. Z. Anorg. Und Allg. Chem. 1990, 591, 41–46. [Google Scholar] [CrossRef]
- Jansen, M.; Feldmann, C.; Müller, W. Über die quasi-binären Systeme NaNO2/Na2O und NaCN/Na2O. Phasendiagramme und Natrium-Ionenleitung in Na3O(NO2) und Na3O(CN). Z. Anorg. Und Allg. Chem. 1992, 611, 7–10. [Google Scholar] [CrossRef]
- Tripathi, S.; Mishra, K.M.; Tiwari, S.N. Electrical conduction of superionic conductors: Na2ZrO3. Emerg. Mater. Res. 2012, 1, 205–211. [Google Scholar] [CrossRef]
- Secco, E.A. Paddle wheel mechanism in lithium sulfates: Arguments in defense and evidence against. J. Solid State Chem. 1992, 96, 366–375. [Google Scholar] [CrossRef]
- Lundén, A. On the Paddle–Wheel Mechanism for Cation Conduction in Lithium Sulphate. Z. Naturforsch. A 1995, 50, 1067–1076. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, H.; Kaup, K.; Zhou, L.; Roy, P.N.; Nazar, L.F. Targeting Superionic Conductivity by Turning on Anion Rotation at Room Temperature in Fast Ion Conductors. Matter 2020, 2, 1667–1684. [Google Scholar] [CrossRef]
- Ratner, M.A.; Shriver, D.F. Ion transport in solvent-free polymers. Chem. Rev. 1988, 88, 109–124. [Google Scholar] [CrossRef]
- Angell, C. Perspective on the glass transition. J. Phys. Chem. Solids 1988, 49, 863–871. [Google Scholar] [CrossRef]
- Dyre, J.C. The random free-energy barrier model for ac conduction in disordered solids. J. Appl. Phys. 1988, 64, 2456–2468. [Google Scholar] [CrossRef]
- Maitra, A.; Heuer, A. Cation Transport in Polymer Electrolytes: A Microscopic Approach. Phys. Rev. Lett. 2007, 98, 227802. [Google Scholar] [CrossRef] [PubMed]
- Mao, G.; Saboungi, M.L.; Price, D.L.; Armand, M.; Mezei, F.; Pouget, S. α-Relaxation in PEO—LiTFSI Polymer Electrolytes. Macromolecules 2002, 35, 415–419. [Google Scholar] [CrossRef]
- Stacy, E.W.; Gainaru, C.P.; Gobet, M.; Wojnarowska, Z.; Bocharova, V.; Greenbaum, S.G.; Sokolov, A.P. Fundamental Limitations of Ionic Conductivity in Polymerized Ionic Liquids. Macromolecules 2018, 51, 8637–8645. [Google Scholar] [CrossRef]
- Tamman, G.; Hesse, W. Die Abhängigkeit der Viscosität von der Temperatur bie unterkühlten Flüssigkeiten. Z. Anorg. Allg. Chem. 1926, 156, 245–247. [Google Scholar] [CrossRef]
- Ferry, A. Ionic Interactions and Transport Properties in Methyl Terminated Poly(propylene glycol)(4000) Complexed with LiCF3SO3. J. Phys. Chem. B 1997, 101, 150–157. [Google Scholar] [CrossRef]
- Larsson, R.; Andersson, O. Properties of electrolytes under pressure: PPG400 and PPG4000 complexed with LiCF3SO3. Electrochim. Acta 2003, 48, 3481–3489. [Google Scholar] [CrossRef]
- Furtado, C.A.; Silva, G.G.; Machado, J.C.; Pimenta, M.A.; Silva, R.A. Study of Correlations between Microstructure and Conductivity in a Thermoplastic Polyurethane Electrolyte. J. Phys. Chem. B 1999, 103, 7102–7110. [Google Scholar] [CrossRef]
- Hou, W.H.; Chen, C.Y. Studies on comb-like polymer electrolyte with a nitrile group. Electrochim. Acta 2004, 49, 2105–2112. [Google Scholar] [CrossRef]
- Garcia-Colin, L.S.; del Castillo, L.F.; Goldstein, P. Theoretical basis for the Vogel-Fulcher-Tammann equation. Phys. Rev. B 1989, 40, 7040–7044. [Google Scholar] [CrossRef]
- Wheatle, B.K.; Lynd, N.A.; Ganesan, V. Effect of Polymer Polarity on Ion Transport: A Competition between Ion Aggregation and Polymer Segmental Dynamics. ACS Macro Lett. 2018, 7, 1149–1154. [Google Scholar] [CrossRef]
- Vincent, C.A. Ion transport in polymer electrolytes. Electrochim. Acta 1995, 40, 2035–2040. [Google Scholar] [CrossRef]
- Gadjourova, Z.; Andreev, Y.G.; Tunstall, D.P.; Bruce, P.G. Ionic conductivity in crystalline polymer electrolytes. Nature 2001, 412, 520–523. [Google Scholar] [CrossRef] [PubMed]
- Schauser, N.S.; Grzetic, D.J.; Tabassum, T.; Kliegle, G.A.; Le, M.L.; Susca, E.M.; Antoine, S.; Keller, T.J.; Delaney, K.T.; Han, S.; et al. The Role of Backbone Polarity on Aggregation and Conduction of Ions in Polymer Electrolytes. J. Am. Chem. Soc. 2020, 142, 7055–7065. [Google Scholar] [CrossRef] [PubMed]
- Yao, Y.F.Y.; Kummer, J.T. Ion exchange properties of and rates of ionic diffusion in beta-alumina. J. Inorg. Nucl. Chem. 1967, 29, 2453–2475. [Google Scholar] [CrossRef]
- Semkow, K.W.; Sammells, A.F. Ionic and Electronic Conductivity Measurements on Polycrystalline Calcium Conducting β″-Alumina. J. Electrochem. Soc. 1988, 135, 244–247. [Google Scholar] [CrossRef]
- Dorner, G.; Durakpasa, H.; Fafilek, G.; Breiter, M. Production and characterization of polycrystalline (Na, Ca)β″-alumina. Solid State Ion. 1992, 53-56, 553–558. [Google Scholar] [CrossRef]
- Farrington, G.; Dunn, B. Divalent β″-aluminas: High conductivity solid electrolytes for divalent cations. Solid State Ion. 1982, 7, 267–281. [Google Scholar] [CrossRef]
- Goodenough, J.; Hong, H.P.; Kafalas, J. Fast Na+–ion transport in skeleton structures. Mater. Res. Bull. 1976, 11, 203–220. [Google Scholar] [CrossRef]
- Ikeda, S.; Takahashi, M.; Ishikawa, J.; Ito, K. Solid electrolytes with multivalent cation conduction. 1. Conducting species in MgZrPO4 system. Solid State Ion. 1987, 23, 125–129. [Google Scholar] [CrossRef]
- Nomura, K.; Ikeda, S.; Ito, K.; Einaga, H. Framework Structure, Phase Transition, and Transport Properties in MIIZr4(PO4)6 Compounds (MII = Mg, Ca, Sr, Ba, Mn, Co, Ni, Zn, Cd, and Pb). Bull. Chem. Soc. Jpn. 1992, 65, 3221–3227. [Google Scholar] [CrossRef]
- Hosono, H.; Hayashi, K.; Kamiya, T.; Atou, T.; Susaki, T. New functionalities in abundant element oxides: Ubiquitous element strategy. Sci. Technol. Adv. Mater. 2011, 12, 034303. [Google Scholar] [CrossRef] [PubMed]
- Imanaka, N. Divalent Magnesium Ionic Conduction in Mg(1−2x)(Zr(1−x)Nbx)4P6O24(x = 0–0.4) Solid Solutions. Electrochem. Solid-State Lett. 1999, 3, 327. [Google Scholar] [CrossRef]
- Imanaka, N.; Okazaki, Y.; Adachi, G. Optimization of divalent magnesium ion conduction in phosphate based polycrystalline solid electrolytes. Ionics 2001, 7, 440–446. [Google Scholar] [CrossRef]
- Anuar, N.; Adnan, S.; Mohamed, N. Characterization of Mg00.5Zr2(PO4)3 for potential use as electrolyte in solid state magnesium batteries. Ceram. Int. 2014, 40, 13719–13727. [Google Scholar] [CrossRef]
- Tamura, S.; Yamane, M.; Hoshino, Y.; Imanaka, N. Highly conducting divalent Mg2+ cation solid electrolytes with well-ordered three-dimensional network structure. J. Solid State Chem. 2016, 235, 7–11. [Google Scholar] [CrossRef]
- Imanaka, N.; Itaya, M.; Adachi, G. First identification of tetravalent Hf4+ ion-conducting solid. Mater. Lett. 2002, 53, 1–5. [Google Scholar] [CrossRef]
- Lee, W.; Tamura, S.; Imanaka, N. Synthesis and characterization of divalent ion conductors with NASICON-type structures. J. Asian Ceram. Soc. 2019, 7, 221–227. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Egawa, T.; Tamura, S.; Imanaka, N.; Adachi, G.Y. Trivalent Al3+ Ion Conduction in Aluminum Tungstate Solid. Chem. Mater. 1997, 9, 1649–1654. [Google Scholar] [CrossRef]
- Imanaka, N.; Hasegawa, Y.; Yamaguchi, M.; Itaya, M.; Tamura, S.; Adachi, G.Y. Extraordinary High Trivalent Al3+ Ion Conduction in Solids. Chem. Mater. 2002, 14, 4481–4483. [Google Scholar] [CrossRef]
- Imanaka, N.; Hasegawa, Y.; Hasegawa, I. Novel trivalent cation conducting solids and their application. Ionics 2004, 10, 385–390. [Google Scholar] [CrossRef]
- Wang, J.; Sun, C.W.; Gong, Y.D.; Zhang, H.R.; Alonso, J.A.; Fernández-Díaz, M.T.; Wang, Z.L.; Goodenough, J.B. Imaging the diffusion pathway of Al3+ ion in NASICON-type (Al0.2Zr0.8)20/19 Nb(PO4)3as electrolyte for rechargeable solid-state Al batteries. Chin. Phys. B 2018, 27, 128201. [Google Scholar] [CrossRef]
- Kendall, K.R.; Navas, C.; Thomas, J.K.; zur Loye, H.C. Recent Developments in Oxide Ion Conductors: Aurivillius Phases. Chem. Mater. 1996, 8, 642–649. [Google Scholar] [CrossRef]
- Sinclair, D.C.; Watson, C.J.; Howie, R.A.; Skakle, J.M.; Coats, A.M.; Kirk, C.A.; Lachowski, E.E.; Marr, J. NaBi3V2O10: A new oxide ion conductor. J. Mater. Chem. 1998, 8, 281–282. [Google Scholar] [CrossRef]
- Porob, D.G.; Guru Row, T. Synthesis crystal structure and ionic conductivity of Ca0.5Bi3V2O10 and Sr0.5Bi3V2O10. J. Solid State Chem. 2004, 177, 4535–4541. [Google Scholar] [CrossRef]
- El Kharbachi, A.; Dematteis, E.M.; Shinzato, K.; Stevenson, S.C.; Bannenberg, L.J.; Heere, M.; Zlotea, C.; Szilágyi, P.Á.; Bonnet, J.P.; Grochala, W.; et al. Metal Hydrides and Related Materials. Energy Carriers for Novel Hydrogen and Electrochemical Storage. J. Phys. Chem. C 2020, 124, 7599–7607. [Google Scholar] [CrossRef]
- Rowberg, A.J.E.; Weston, L.; Van de Walle, C.G. Ion-Transport Engineering of Alkaline-Earth Hydrides for Hydride Electrolyte Applications. Chem. Mater. 2018, 30, 5878–5885. [Google Scholar] [CrossRef]
- Luo, X.; Aguey-Zinsou, K.F. Correlations between the ionic conductivity and cation size in complex borohydrides. Ionics 2020, 26, 5287–5291. [Google Scholar] [CrossRef]
- Unemoto, A.; Matsuo, M.; Orimo, S.I. Complex Hydrides for Electrochemical Energy Storage. Adv. Funct. Mater. 2014, 24, 2267–2279. [Google Scholar] [CrossRef]
- Lu, Z.; Ciucci, F. Metal Borohydrides as Electrolytes for Solid-State Li, Na, Mg, and Ca Batteries: A First-Principles Study. Chem. Mater. 2017, 29, 9308–9319. [Google Scholar] [CrossRef]
- Portier, J.M.R.J.; Levasseur, A.; Villeneuve, G.; Pouchard, M. Characteristic properties of new solid electrolytes. Mater. Res. Bull. 1978, 13, 1415–1423. [Google Scholar] [CrossRef]
- Matsuo, M.; Remhof, A.; Martelli, P.; Caputo, R.; Ernst, M.; Miura, Y.; Sato, T.; Oguchi, H.; Maekawa, H.; Takamura, H.; et al. Complex Hydrides with (BH4)– and (NH2)– Anions as New Lithium Fast–Ion Conductors. J. Am. Chem. Soc. 2009, 131, 16389–16391. [Google Scholar] [CrossRef] [PubMed]
- Mezaki, T.; Kuronuma, Y.; Oikawa, I.; Kamegawa, A.; Takamura, H. Li-Ion Conductivity and Phase Stability of Ca-Doped LiBH4 under High Pressure. Inorg. Chem. 2016, 55, 10484–10489. [Google Scholar] [CrossRef]
- Kim, S.; Kisu, K.; Takagi, S.; Oguchi, H.; Orimo, S.I. Complex Hydride Solid Electrolytes of the Li(CB9H10)–Li(CB11H12) Quasi-Binary System: Relationship between the Solid Solution and Phase Transition, and the Electrochemical Properties. ACS Appl. Energy Mater. 2020, 3, 4831–4839. [Google Scholar] [CrossRef]
- Zettl, R.; Gombotz, M.; Clarkson, D.; Greenbaum, S.G.; Ngene, P.; de Jongh, P.E.; Wilkening, H.M.R. Li-Ion Diffusion in Nanoconfined LiBH4-LiI/Al2O3: From 2D Bulk Transport to 3D Long-Range Interfacial Dynamics. ACS Appl. Mater. Interfaces 2020, 12, 38570–38583. [Google Scholar] [CrossRef] [PubMed]
- Schuth, F.; Bogdanović, B.; Felderhoff, M. Light metal hydrides and complex hydrides for hydrogen storage. Chem. Commun. 2004, 20, 2249–2258. [Google Scholar] [CrossRef]
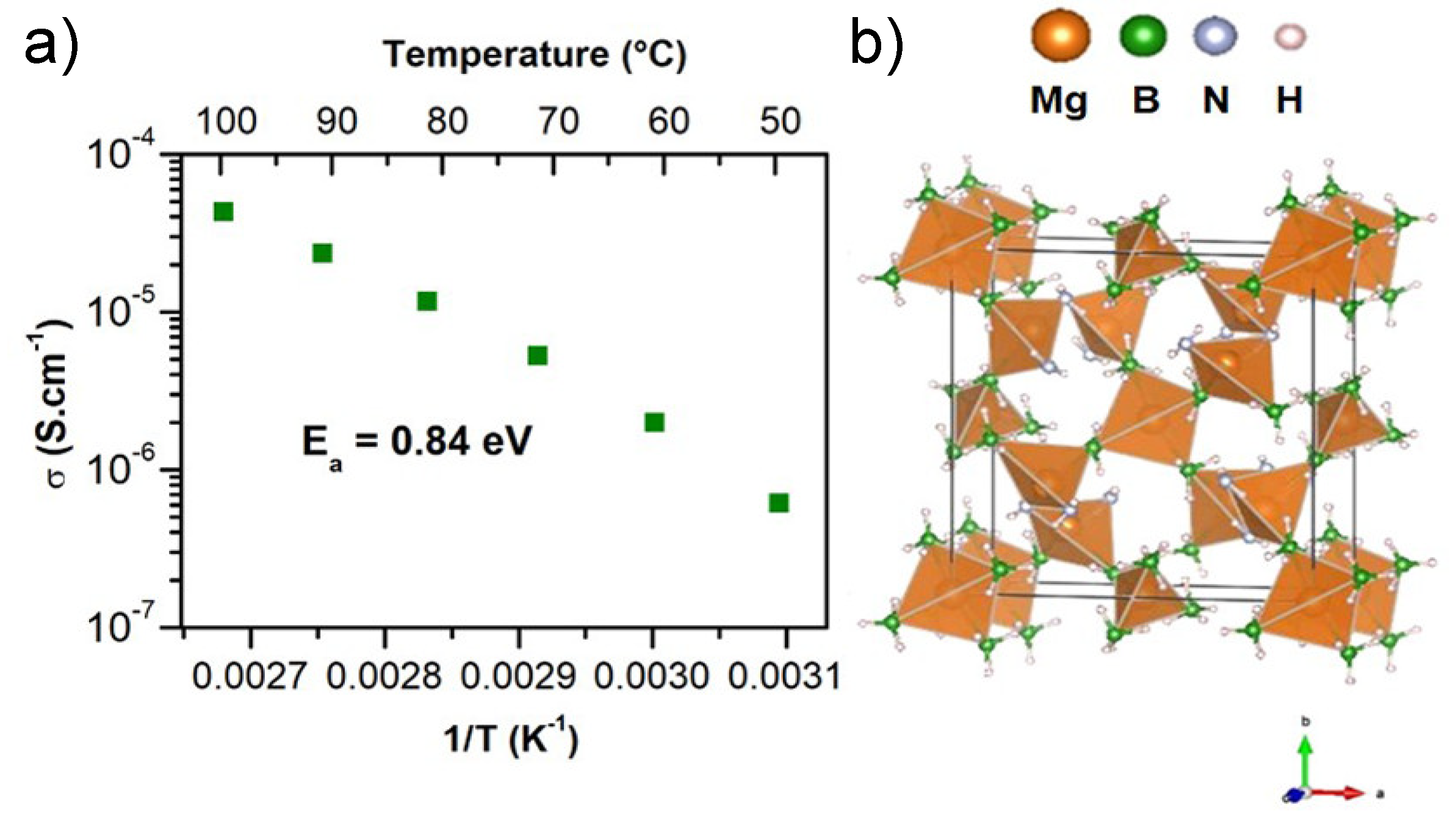
- Cerny, R.; Filinchuk, Y.; Hagemann, H.; Yvon, K. Magnesium Borohydride: Synthesis and Crystal Structure. Angew. Chem. Int. Ed. 2007, 46, 5765–5767. [Google Scholar] [CrossRef]
- Ikeshoji, T.; Tsuchida, E.; Takagi, S.; Matsuo, M.; Orimo, S.I. Magnesium ion dynamics in Mg(BH4)2(1−x)X2x (X = Cl or AlH4) from first-principles molecular dynamics simulations. RSC Adv. 2014, 4, 1366–1370. [Google Scholar] [CrossRef]
- Liu, H.; Ren, Z.; Zhang, X.; Hu, J.; Gao, M.; Pan, H.; Liu, Y. Incorporation of Ammonia Borane Groups in the Lithium Borohydride Structure Enables Ultrafast Lithium Ion Conductivity at Room Temperature for Solid-State Batteries. Chem. Mater. 2020, 32, 671–678. [Google Scholar] [CrossRef]
- Yan, Y.; Grinderslev, J.B.; Lee, Y.S.; JÞrgensen, M.; Cho, Y.W.; Černý, R.; Jensen, T.R. Ammonia-assisted fast Li-ion conductivity in a new hemiammine lithium borohydride, LiBH4·1/2NH3. Chem. Commun. 2020, 56, 3971–3974. [Google Scholar] [CrossRef]
- Ngene, P.; Lambregts, S.F.H.; Blanchard, D.; Vegge, T.; Sharma, M.; Hagemann, H.; de Jongh, P.E. The influence of silica surface groups on the Li–ion conductivity of LiBH4/SiO2 nanocomposites. Phys. Chem. Chem. Phys. 2019, 21, 22456–22466. [Google Scholar] [CrossRef] [PubMed]
- Roedern, E.; Kühnel, R.S.; Remhof, A.; Battaglia, C. Magnesium Ethylenediamine Borohydride as Solid-State Electrolyte for Magnesium Batteries. Sci. Rep. 2017, 7, 46189. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Chua, Y.S.; Wu, H.; Xiong, Z.; He, T.; Zhou, W.; Ju, X.; Yang, M.; Wu, G.; Chen, P. Synthesis, structures and dehydrogenation of magnesium borohydride–ethylenediamine composites. Int. J. Hydrog. Energy 2015, 40, 412–419. [Google Scholar] [CrossRef]
- Yan, Y.; Dononelli, W.; JÞrgensen, M.; Grinderslev, J.B.; Lee, Y.S.; Cho, Y.W.; Černý, R.; Hammer, B.; Jensen, T.R. The mechanism of Mg2+ conduction in ammine magnesium borohydride promoted by a neutral molecule. Phys. Chem. Chem. Phys. 2020, 22, 9204–9209. [Google Scholar] [CrossRef] [PubMed]
- Yan, Y.; Grinderslev, J.B.; Jorgensen, M.; Skov, L.N.; Skibsted, J.; Jensen, T.R. Ammine Magnesium Borohydride Nanocomposites for All-Solid-State Magnesium Batteries. ACS Appl. Energy Mater. 2020, 3, 9264–9270. [Google Scholar] [CrossRef]
- Higashi, S.; Miwa, K.; Aoki, M.; Takechi, K. A novel inorganic solid state ion conductor for rechargeable Mg batteries. Chem. Commun. 2014, 50, 1320–1322. [Google Scholar] [CrossRef] [PubMed]
- Le Ruyet, R.; Berthelot, R.; Salager, E.; Florian, P.; Fleutot, B.; Janot, R. Investigation of Mg(BH4)(NH2)-Based Composite Materials with Enhanced Mg2+ Ionic Conductivity. J. Phys. Chem. C 2019, 123, 10756–10763. [Google Scholar] [CrossRef]
- Le Ruyet, R.; Fleutot, B.; Berthelot, R.; Benabed, Y.; Hautier, G.; Filinchuk, Y.; Janot, R. Mg3(BH4)4(NH2)2 as Inorganic Solid Electrolyte with High Mg2+ Ionic Conductivity. ACS Appl. Energy Mater. 2020, 3, 6093–6097. [Google Scholar] [CrossRef]
- Momma, K.; Izumi, F. VESTA 3 for Three-Dimensional Visualization of Crystal, Volumetric and Morphology Data JAC. Available online: https://scripts.iucr.org/cgi-bin/paper?db5098 (accessed on 12 July 2020).
- Kisu, K.; Kim, S.; Inukai, M.; Oguchi, H.; Takagi, S.; Orimo, S.I. Magnesium Borohydride Ammonia Borane as a Magnesium Ionic Conductor. ACS Appl. Energy Mater. 2020, 3, 3174–3179. [Google Scholar] [CrossRef]
- Fang, H.; Jena, P. Li-rich antiperovskite superionic conductors based on cluster ions. Proc. Natl. Acad. Sci. USA 2017, 114, 11046–11051. [Google Scholar] [CrossRef]
- For Information Infrastructure, F.K.L.I. Available online: https://www.sciencedirect.com/topics/computer-science/information-infrastructure (accessed on 12 June 2020).
- ToposPro. Available online: https://topospro.com/ (accessed on 16 June 2020).
- Morkhova, E.A.; Kabanov, A.A.; Blatov, V.A. Modeling of Ionic Conductivity in Inorganic Compounds with Multivalent Cations. Russ. J. Electrochem. 2019, 55, 762–777. [Google Scholar] [CrossRef]
- Nestler, T.; Meutzner, F.; Kabanov, A.A.; Zschornak, M.; Leisegang, T.; Meyer, D.C. Combined Theoretical Approach for Identifying Battery Materials: Al3+ Mobility in Oxides. Chem. Mater. 2019, 31, 737–747. [Google Scholar] [CrossRef]
- Takeda, H.; Nakano, K.; Tanibata, N.; Nakayama, M. Novel Mg-ion conductive oxide of μ-cordierite Mg0.6Al1.2Si1.8O6. Sci. Technol. Adv. Mater. 2020, 21, 131–138. [Google Scholar] [CrossRef] [PubMed]
- Sai Gautam, G.; Canepa, P.; Urban, A.; Bo, S.H.; Ceder, G. Influence of Inversion on Mg Mobility and Electrochemistry in Spinels. Chem. Mater. 2017, 29, 7918–7930. [Google Scholar] [CrossRef]
- Liu, M.; Rong, Z.; Malik, R.; Canepa, P.; Jain, A.; Ceder, G.; Persson, K.A. Spinel compounds as multivalent battery cathodes: A systematic evaluation based on ab initio calculations. Energy Environ. Sci. 2015, 8, 964–974. [Google Scholar] [CrossRef]
- Canepa, P.; Bo, S.H.; Sai Gautam, G.; Key, B.; Richards, W.D.; Shi, T.; Tian, Y.; Wang, Y.; Li, J.; Ceder, G. High magnesium mobility in ternary spinel chalcogenides. Nat. Commun. 2017, 8, 1759. [Google Scholar] [CrossRef]
- Rong, Z.; Malik, R.; Canepa, P.; Sai Gautam, G.; Liu, M.; Jain, A.; Persson, K.; Ceder, G. Materials Design Rules for Multivalent Ion Mobility in Intercalation Structures. Chem. Mater. 2015, 27, 6016–6021. [Google Scholar] [CrossRef]
- Yang, L.L.; Huq, R.; Farrington, G.; Chiodelli, G. Preparation and properties of PEO complexes of divalent cation salts. Solid State Ion. 1986, 18–19, 291–294. [Google Scholar] [CrossRef]
- Yang, L.L.; McGhie, A.R.; Farrington, G.C. Ionic Conductivity in Complexes of Poly(ethylene oxide) and MgCl2. J. Electrochem. Soc. 1986, 133, 1380–1385. [Google Scholar] [CrossRef]
- Patrick, A.; Glasse, M.; Latham, R.; Linford, R. Novel solid state polymeric batteries. Solid State Ion. 1986, 18–19, 1063–1067. [Google Scholar] [CrossRef]
- Jaipal Reddy, M.; Chu, P.P. Ion pair formation and its effect in PEO:Mg solid polymer electrolyte system. J. Power Sources 2002, 109, 340–346. [Google Scholar] [CrossRef]
- Ramalingaiah, S.; SrinivasReddy, D.; Reddy, M.J.; Laxminarsaiah, E.; SubbaRao, U.V. Conductivity and discharge characteristic studies of novel polymer electrolyte based on PEO complexed with Mg(NO3)2 salt. Mater. Lett. 1996, 29. [Google Scholar] [CrossRef]
- Bakker, A.; Gejji, S.; Lindgren, J.; Hermansson, K.; Probst, M.M. Contact ion pair formation and ether oxygen coordination in the polymer electrolytes M[N(CF3SO2)2]2PEOn for M = Mg, Ca, Sr and Ba. Polymer 1995, 36, 4371–4378. [Google Scholar] [CrossRef]
- Wendsjo, Å.; Lindgren, J.; Thomas, J.; Farrington, G. The effect of temperature and concentration on the local environment in the system M(CF3SO3)2PEOn for M = Ni, Zn and Pb. Solid State Ion. 1992, 53–56, 1077–1082. [Google Scholar] [CrossRef]
- Anilkumar, K.; Jinisha, B.; Manoj, M.; Jayalekshmi, S. Poly(ethylene oxide) (PEO)–Poly(vinyl pyrrolidone) (PVP) blend polymer based solid electrolyte membranes for developing solid state magnesium ion cells. Eur. Polym. J. 2017, 89, 249–262. [Google Scholar] [CrossRef]
- Feng, H.; Feng, Z.; Shen, L. A High Resolution Solid-State n.m.r. and d.s.c. Study of Miscibility and Crystallization Behaviour of Poly(vinyl alcohol)poly(N–vinyl–2–pyrrolidone) Blends. Polymer 1993, 34, 2516–2519. [Google Scholar] [CrossRef]
- Rathika, R.; Suthanthiraraj, S.A. Ionic Interactions and Dielectric Relaxation of PEO/PVDF-Mg(CF3SO2)2N2) Blend Electrolytes for Magnesium Ion Rechargeable Batteries. Macromol. Res. 2016, 24, 422–428. [Google Scholar] [CrossRef]
- Rathika, R.; Padmaraj, O.; Suthanthiraraj, S.A. Electrical conductivity and dielectric relaxation behaviour of PEO/PVdF-based solid polymer blend electrolytes for zinc battery applications. Ionics 2018, 24, 243–255. [Google Scholar] [CrossRef]
- Yang, H.; Huq, R.; Farrington, G. Conductivity in PEO-based Zn(II) polymer electrolytes. Solid State Ion. 1990, 40–41, 663–665. [Google Scholar] [CrossRef]
- Jeong, S.K.; Jo, Y.K.; Jo, N.J. Decoupled ion conduction mechanism of poly(vinyl alcohol) based Mg-conducting solid polymer electrolyte. Electrochim. Acta 2006, 52, 1549–1555. [Google Scholar] [CrossRef]
- Nishio, Y.; Haratani, T.; Takahashi, T. Miscibility and orientation behavior of poly(vinyl alcohol)/poly(vinyl pyrrolidone) blends. J. Polym. Sci. Part B Polym. Phys. 1990, 28, 355–376. [Google Scholar] [CrossRef]
- Polu, A.R.; Kumar, R.; Rhee, H.W. Magnesium ion conducting solid polymer blend electrolyte based on biodegradable polymers and application in solid-state batteries. Ionics 2015, 21, 125–132. [Google Scholar] [CrossRef]
- Polu, A.R.; Kumar, R. Preparation and characterization of pva based solid polymer electrolytes for electrochemical cell applications. Chin. J. Polym. Sci. 2013, 31, 641–648. [Google Scholar] [CrossRef]
- Ramaswamy, M.; Malayandi, T.; Subramanian, S.; Srinivasalu, J.; Rangaswamy, M. Magnesium ion conducting polyvinyl alcohol–polyvinyl pyrrolidone-based blend polymer electrolyte. Ionics 2017, 23, 1771–1781. [Google Scholar] [CrossRef]
- Manjuladevi, R.; Thamilselvan, M.; Selvasekarapandian, S.; Mangalam, R.; Premalatha, M.; Monisha, S. Mg-ion conducting blend polymer electrolyte based on poly(vinyl alcohol)-poly (acrylonitrile) with magnesium perchlorate. Solid State Ion. 2017, 308, 90–100. [Google Scholar] [CrossRef]
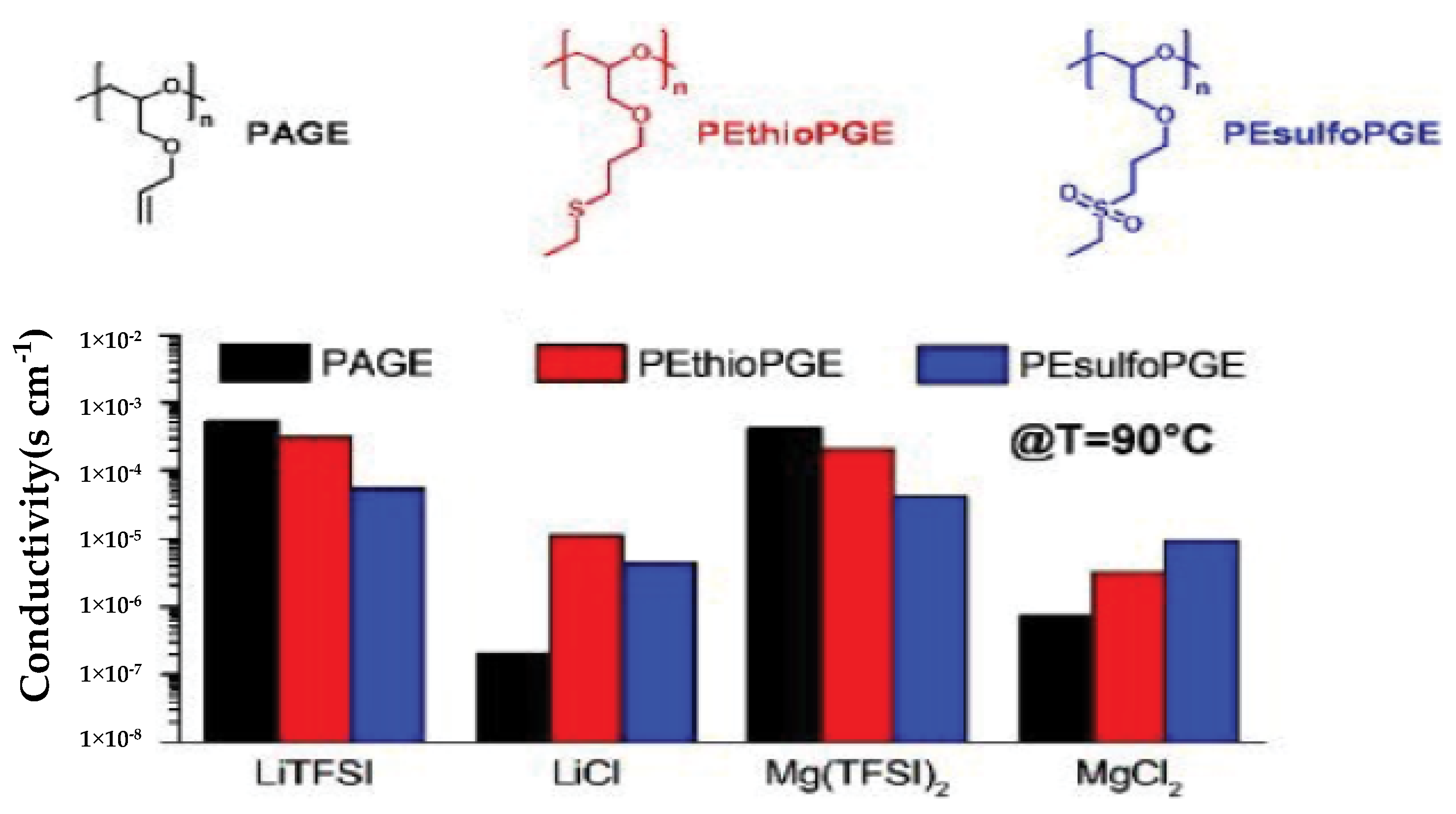
- Viviani, M.; Meereboer, N.L.; Saraswati, N.L.P.A.; Loos, K.; Portale, G. Lithium and magnesium polymeric electrolytes prepared using poly(glycidyl ether)-based polymers with short grafted chains. Polym. Chem. 2020, 11, 2070–2079. [Google Scholar] [CrossRef]
- Barteau, K.P.; Wolffs, M.; Lynd, N.A.; Fredrickson, G.H.; Kramer, E.J.; Hawker, C.J. Allyl Glycidyl Ether-Based Polymer Electrolytes for Room Temperature Lithium Batteries. Macromolecules 2013, 46, 8988–8994. [Google Scholar] [CrossRef]
- Wei, Z.; Chen, S.; Wang, J.; Wang, Z.; Zhang, Z.; Yao, X.; Deng, Y.; Xu, X. A large-size, bipolar-stacked and high-safety solid-state lithium battery with integrated electrolyte and cathode. J. Power Sources 2018, 394, 57–66. [Google Scholar] [CrossRef]
- Fu, G.; Dempsey, J.; Izaki, K.; Adachi, K.; Tsukahara, Y.; Kyu, T. Highly conductive solid polymer electrolyte membranes based on polyethylene glycol-bis-carbamate dimethacrylate networks. J. Power Sources 2017, 359, 441–449. [Google Scholar] [CrossRef]
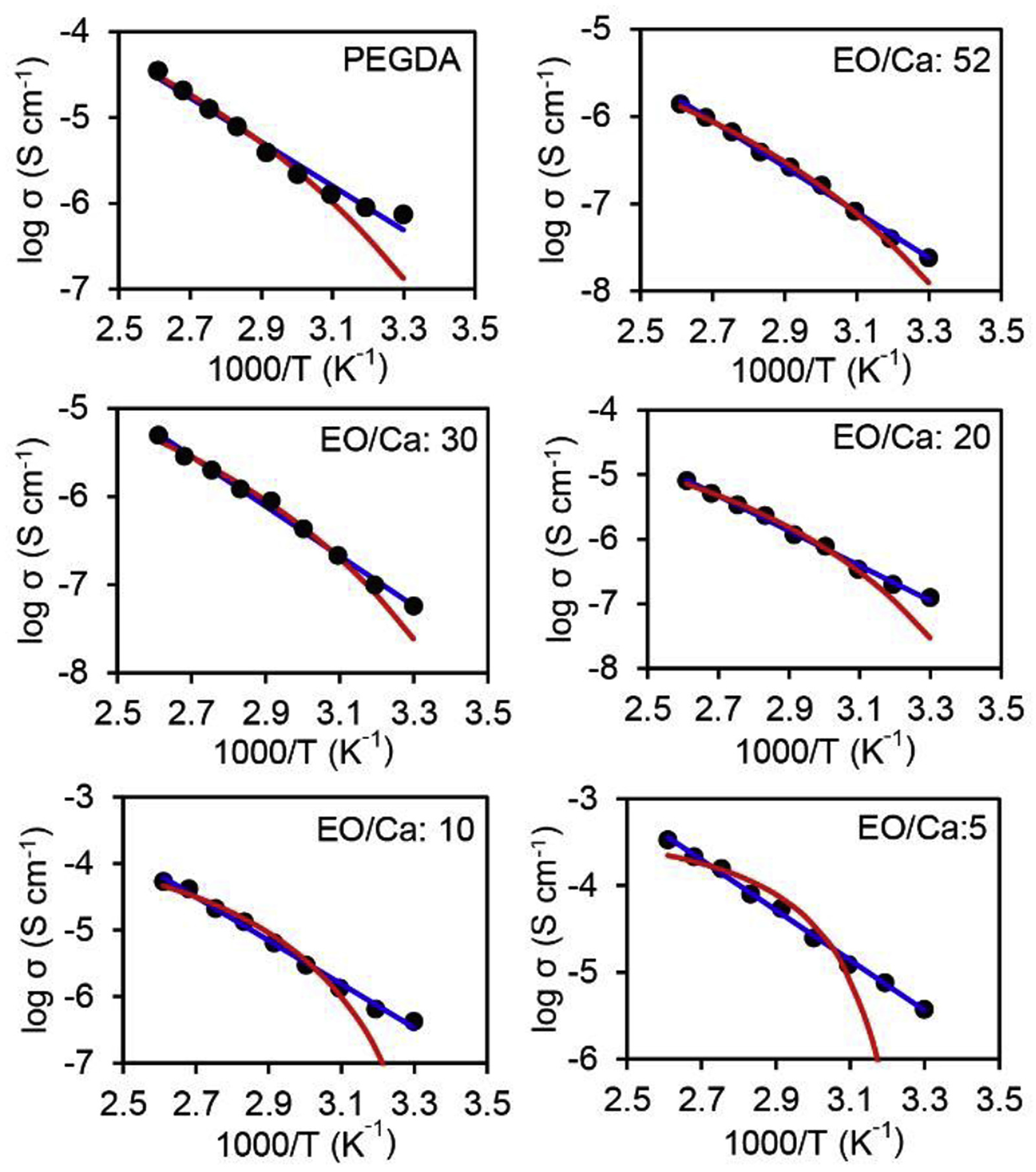
- Genier, F.S.; Burdin, C.V.; Biria, S.; Hosein, I.D. A novel calcium-ion solid polymer electrolyte based on crosslinked poly(ethylene glycol) diacrylate. J. Power Sources 2019, 414, 302–307. [Google Scholar] [CrossRef]
- Liu, J.; Khanam, Z.; Muchakayala, R.; Song, S. Fabrication and characterization of Zn-ion-conducting solid polymer electrolyte films based on PVdF-HFP/Zn(Tf)2 complex system. J. Mater. Sci. Mater. Electron. 2020, 31, 6160–6173. [Google Scholar] [CrossRef]
- Yao, T.; Genier, F.S.; Biria, S.; Hosein, I.D. A solid polymer electrolyte for aluminum ion conduction. Results Phys. 2018, 10, 529–531. [Google Scholar] [CrossRef]
- Xia, Y.; Fujieda, T.; Tatsumi, K.; Prosini, P.P.; Sakai, T. Thermal and electrochemical stability of cathode materials in solid polymer electrolyte. J. Power Sources 2001, 92, 234–243. [Google Scholar] [CrossRef]
- Tippens, J.; Miers, J.C.; Afshar, A.; Lewis, J.A.; Cortes, F.J.Q.; Qiao, H.; Marchese, T.S.; Di Leo, C.V.; Saldana, C.; McDowell, M.T. Visualizing Chemomechanical Degradation of a Solid-State Battery Electrolyte. ACS Energy Lett. 2019, 4, 1475–1483. [Google Scholar] [CrossRef]
- Kim, H.; Jeong, G.; Kim, Y.U.; Kim, J.H.; Park, C.M.; Sohn, H.J. Metallic anodes for next generation secondary batteries. Chem. Soc. Rev. 2013, 42, 9011–9034. [Google Scholar] [CrossRef]
- Canepa, P.; Sai Gautam, G.; Hannah, D.C.; Malik, R.; Liu, M.; Gallagher, K.G.; Persson, K.A.; Ceder, G. Odyssey of Multivalent Cathode Materials: Open Questions and Future Challenges. Chem. Rev. 2017, 117, 4287–4341. [Google Scholar] [CrossRef]
- Wu, S.; Zhang, F.; Tang, Y. A Novel Calcium-Ion Battery Based on Dual-Carbon Configuration with High Working Voltage and Long Cycling Life. Adv. Sci. 2018, 5, 1701082. [Google Scholar] [CrossRef]
- Aurbach, D.; Skaletsky, R.; Gofer, Y. The Electrochemical Behavior of Calcium Electrodes in a Few Organic Electrolytes. J. Electrochem. Soc. 1991, 138, 3536–3545. [Google Scholar] [CrossRef]
- Tchitchekova, D.S.; Monti, D.; Johansson, P.; Bardé, F.; Randon-Vitanova, A.; Palacín, M.R.; Ponrouch, A. On the Reliability of Half-Cell Tests for Monovalent (Li+, Na+) and Divalent (Mg2+, Ca2+) Cation Based Batteries. J. Electrochem. Soc. 2017, 164, A1384–A1392. [Google Scholar] [CrossRef]
- Gummow, R.J.; Vamvounis, G.; Kannan, M.B.; He, Y. Calcium-Ion Batteries: Current State-of-the-Art and Future Perspectives. Adv. Mater. 2018, 30, 1801702. [Google Scholar] [CrossRef]
- Juran, T.R.; Smeu, M. Hybrid density functional theory modeling of Ca, Zn, and Al ion batteries using the Chevrel phase Mo6S8 cathode. Phys. Chem. Chem. Phys. 2017, 19, 20684–20690. [Google Scholar] [CrossRef]
- Padigi, P.; Goncher, G.; Evans, D.; Solanki, R. Potassium barium hexacyanoferrate—A potential cathode material for rechargeable calcium ion batteries. J. Power Sources 2015, 273, 460–464. [Google Scholar] [CrossRef]
- Chao, D.; Zhu, C.R.; Song, M.; Liang, P.; Zhang, X.; Tiep, N.H.; Zhao, H.; Wang, J.; Wang, R.; Zhang, H.; et al. A High-Rate and Stable Quasi-Solid-State Zinc-Ion Battery with Novel 2D Layered Zinc Orthovanadate Array. Adv. Mater. 2018, 30, 1803181. [Google Scholar] [CrossRef]
- Lee, B.S.; Cui, S.; Xing, X.; Liu, H.; Yue, X.; Petrova, V.; Lim, H.D.; Chen, R.; Liu, P. Dendrite Suppression Membranes for Rechargeable Zinc Batteries. ACS Appl. Mater. Interfaces 2018, 10, 38928–38935. [Google Scholar] [CrossRef]
- Trudgeon, D.P.; Qiu, K.; Li, X.; Mallick, T.; Taiwo, O.O.; Chakrabarti, B.; Yufit, V.; Brandon, N.P.; Crevillen-Garcia, D.; Shah, A. Screening of effective electrolyte additives for zinc-based redox flow battery systems. J. Power Sources 2019, 412, 44–54. [Google Scholar] [CrossRef]
- Banik, S.J.; Akolkar, R. Suppressing Dendrite Growth during Zinc Electrodeposition by PEG-200 Additive. J. Electrochem. Soc. 2013, 160, D519–D523. [Google Scholar] [CrossRef]
- Adams, S. From bond valence maps to energy landscapes for mobile ions in ion-conducting solids. Solid State Ion. 2006, 177, 1625–1630. [Google Scholar] [CrossRef]
- Neiman, A.Y.; Pestereva, N.N.; Zhou, Y.; Nechaev, D.O.; Koteneva, E.A.; Vanec, K.; Higgins, B.; Volkova, N.; Korchuganova, I.G. The nature and the mechanism of ion transfer in tungstates Me2+{WO4} (Ca, Sr, Ba) and {WO4}3 (Al, Sc, In) according to the data acquired by the tubandt method. Russ. J. Electrochem. 2013, 49, 895–907. [Google Scholar] [CrossRef]
- Imanaka, N.; Tamura, S. Development of Multivalent Ion Conducting Solid Electrolytes. Bull. Chem. Soc. Jpn. 2011, 84, 353–362. [Google Scholar] [CrossRef]
- Lee, B.; Lee, H.; Yim, T.; Kim, J.; Lee, J.; Chung, K.; Cho, B.; Oh, S. Investigation on the structural evolutions during the insertion of aluminum ions into Mo6S8 Chevrel phase. J. Electrochem. Soc. 2016, 163, A1070–A1076. [Google Scholar] [CrossRef]
- Geng, L.; Lv, G.; Xing, X.; Guo, J. Reversible Electrochemical Intercalation of Aluminum in Mo6S8. Chem. Mater. 2015, 27, 4926–4929. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
O’Donnell, L.F.; Greenbaum, S.G. Review of Multivalent Metal Ion Transport in Inorganic and Solid Polymer Electrolytes. Batteries 2021, 7, 3. https://doi.org/10.3390/batteries7010003
O’Donnell LF, Greenbaum SG. Review of Multivalent Metal Ion Transport in Inorganic and Solid Polymer Electrolytes. Batteries. 2021; 7(1):3. https://doi.org/10.3390/batteries7010003
Chicago/Turabian StyleO’Donnell, Lauren F., and Steven G. Greenbaum. 2021. "Review of Multivalent Metal Ion Transport in Inorganic and Solid Polymer Electrolytes" Batteries 7, no. 1: 3. https://doi.org/10.3390/batteries7010003
APA StyleO’Donnell, L. F., & Greenbaum, S. G. (2021). Review of Multivalent Metal Ion Transport in Inorganic and Solid Polymer Electrolytes. Batteries, 7(1), 3. https://doi.org/10.3390/batteries7010003





