Corrections of Voltage Loss in Hydrogen-Oxygen Fuel Cells
Abstract
1. Introduction
2. Theoretical Analysis
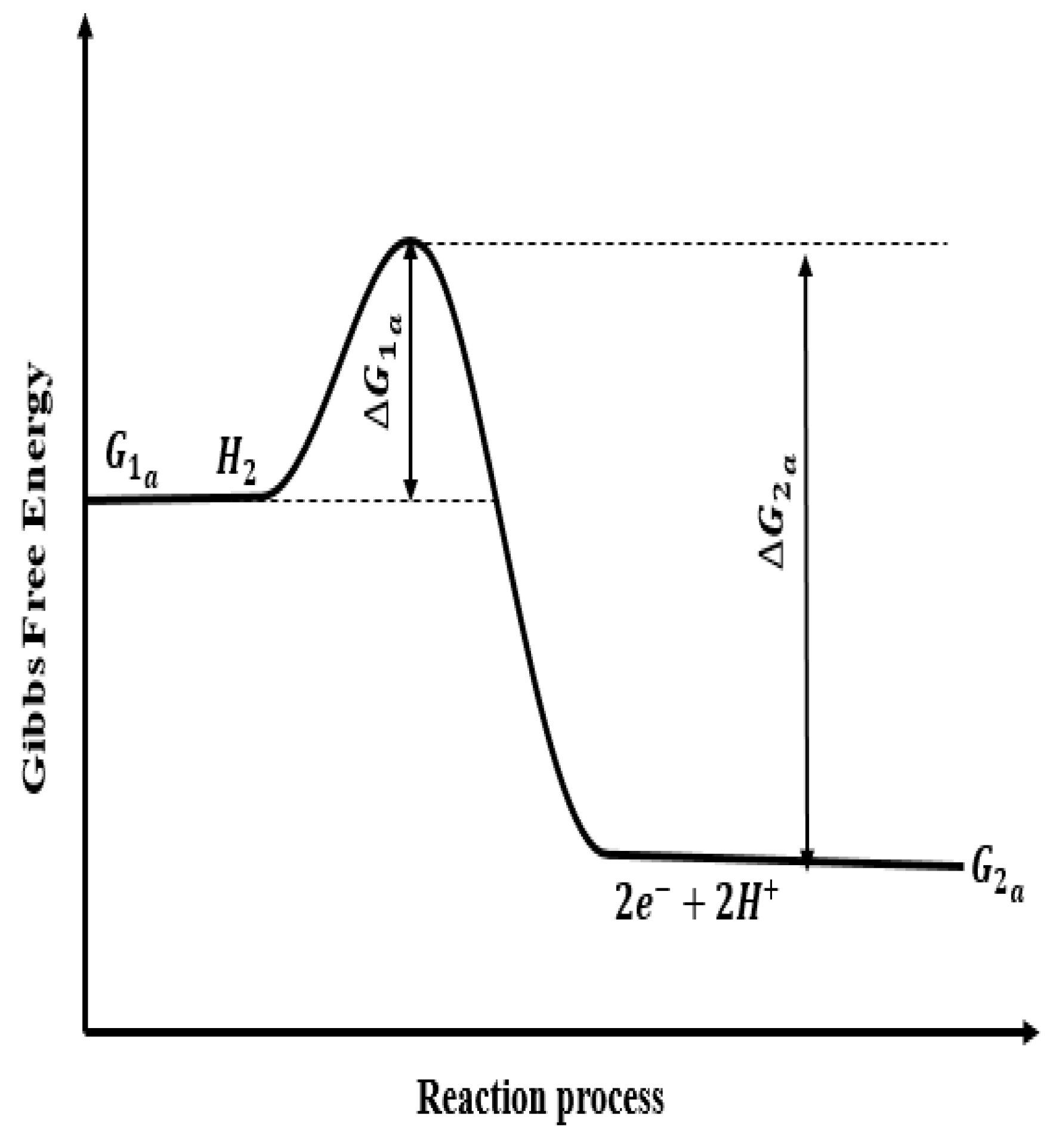
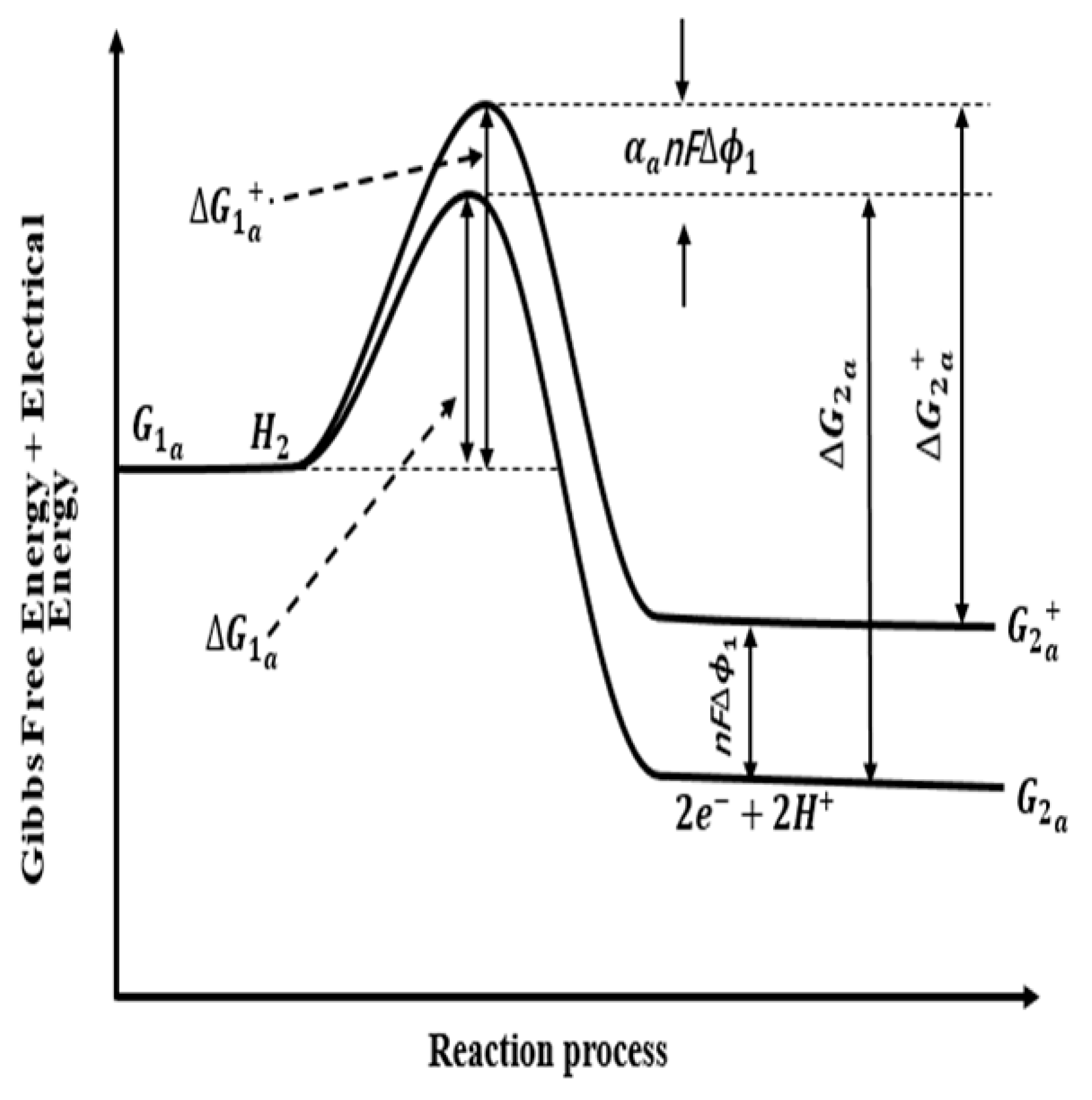
2.1. Galvani Potential
2.2. Cell Voltage Losses
3. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Derr, I.; Fetyan, A.; Schutjajew, K.; Roth, C. Electrochemical analysis of the performance loss in all vanadium redox flow batteries using different cut-off voltages. Electrochim. Acta 2017, 224, 9–16. [Google Scholar] [CrossRef]
- Miyashita, T. Loss of Gibbs Energy Using Sm-Doped Ceria Electrolytes in SOFCs Considering Local Equilibrium while Ion Hopping. Open Mater. Sci. J. 2009, 3, 47–49. [Google Scholar] [CrossRef]
- Chiodelli, G.; Malavasi, L. Electrochemical open circuit voltage (OCV) characterization of SOFC materials. Ionics 2013, 19, 1135–1144. [Google Scholar] [CrossRef]
- Miyashita, T. A Current-Independent Constant Anode Voltage Loss Using Sm-Doped Ceria Electrolytes in SOFCs. ECS Trans. 2014, 59, 53–61. [Google Scholar] [CrossRef]
- Barnhart, C.J.; Dale, M.; Brandt, A.R.; Benson, S.M. The energetic implications of curtailing versus storing solar- and wind-generated electricity. Energy Environ. Sci. 2013, 6, 2804–2810. [Google Scholar] [CrossRef]
- Calise, F.; Dentice d’Accadia, M.; Palombo, A.; Vanoli, L. Simulation and exergy analysis of a hybrid Solid Oxide Fuel Cell (SOFC)–Gas Turbine System. Energy 2006, 31, 3278–3299. [Google Scholar] [CrossRef]
- Fowler, M.W.; Mann, R.F.; Amphlett, J.C.; Peppley, B.A.; Roberge, P.R. Incorporation of voltage degradation into a generalised steady state electrochemical model for a PEM fuel cell. J. Power Sources 2002, 106, 274–283. [Google Scholar] [CrossRef]
- Rakhtala, S.M.; Ghaderi, R.; Ranjbar Noei, A. Proton exchange membrane fuel cell voltage-tracking using artificial neural networks. J. Zhejiang Univ. Sci. C 2011, 12, 338–344. [Google Scholar] [CrossRef]
- Kundu, S.; Fowler, M.; Simon, L.C.; Abouatallah, R. Reversible and irreversible degradation in fuel cells during Open Circuit Voltage durability testing. J. Power Sources 2008, 182, 254–258. [Google Scholar] [CrossRef]
- Ahmed, K.; Föger, K. Analysis of equilibrium and kinetic models of internal reforming on solid oxide fuel cell anodes: Effect on voltage, current and temperature distribution. J. Power Sources 2017, 343, 83–93. [Google Scholar] [CrossRef]
- Bove, R.; Lunghi, P.; Msammes, N. SOFC mathematic model for systems simulations. Part one: From a micro-detailed to macro-black-box model. Int. J. Hydrog. Energy 2005, 30, 181–187. [Google Scholar] [CrossRef]
- Ranjbar, F.; Chitsaz, A.; Mahmoudi, S.M.S.; Khalilarya, S.; Rosen, M.A. Energy and exergy assessments of a novel trigeneration system based on a solid oxide fuel cell. Energy Conv. Manag. 2014, 87, 318–327. [Google Scholar] [CrossRef]
- Arato, E.; Costa, P. Transport mechanisms and voltage losses in PEMFC membranes and at electrodes: A discussion of open-circuit irreversibility. J. Power Sources 2006, 159, 861–868. [Google Scholar] [CrossRef]
- Sharma, A.K.; Ahmed, K.; Birgersson, E. Nernst voltage losses in planar fuel cells caused by changes in chemical composition: Effects of operating parameters. Ionics 2018, 24, 2047–2054. [Google Scholar] [CrossRef]
- Winkler, W.; Williams, M.C. Basics for Fuel Cell Performance Loss Evaluation. ECS Trans. 2018, 83, 53–69. [Google Scholar] [CrossRef]
- Erilin, I.S.; Smorodova, O.V.; Baikov, I.R.; Kitaev, S.V. Experimental study of fuel cell properties based on current-voltage characteristic. J. Phys. Conf. Ser. 2018, 1111, 012070. [Google Scholar] [CrossRef]
- Gemmen, R.S.; Johnson, C.D. Effect of load transients on SOFC operation-current reversal on loss of load. J. Power Sources 2005, 144, 152–164. [Google Scholar] [CrossRef]
- Noren, D.A.; Hoffman, M.A. Clarifying the Butler–Volmer equation and related approximations for calculating activation losses in solid oxide fuel cell models. J. Power Sources 2005, 152, 175–181. [Google Scholar] [CrossRef]
- Luo, Y.Q.; Jiao, K.; Jia, B. Elucidating the constant power, current and voltage cold start modes of proton exchange membrane fuel cell. Int. J. Heat Mass Transf. 2014, 77, 489–500. [Google Scholar] [CrossRef]
- Au, S.F.; Peelen, W.H.A.; Standaert, F.R.A.M.; Hemmes, K.; Uchida, I. Verification of Analytical Fuel Cell Models by Performance Testing at a 110 cm2 Molten Carbonate Fuel Cell. J. Electrochem. Soc. 2001, 148, A1051. [Google Scholar] [CrossRef]
- Selimovic, A.; Palsson, J. Networked solid oxide fuel cell stacks combined with a gas turbine cycle. J. Power Sources 2002, 106, 76–82. [Google Scholar] [CrossRef]
- Reimer, U.; Lehnert, W.; Holade, Y.; Kokoh, B. Irreversible Losses in Fuel Cells. Fuel Cells and Hydrogen. 2018, pp. 15–40. Available online: https://doi.org/10.1016/B978-0-12-811459-9.00002-5. (accessed on 5 February 2020).
- Greene, E.S.; Chiu, W.K.S.; Medeiros, M.G. Mass transfer in graded microstructure solid oxide fuel cell electrodes. J. Power Sources 2006, 161, 225–231. [Google Scholar] [CrossRef]
- Yi, B.L. Fuel Cell—Principles, Technology, Apply, 1st ed.; Chemical Industry Press: Beijing, China, 2003; pp. 9–13. [Google Scholar]
- Ryan, O.; Suk-won, C.; Whitney, G.C.; Fritz, B.P. Chapter 3 Fuel Cell Reaction Kinetics, In Fuel Cell Fundamentals, 3rd ed.; John Wiley and Sons: Hoboken, NJ, USA, 2016; pp. 85–90. [Google Scholar]
- Colleen, S. PEM Fuel Cell Modeling and Simulation Using MATLAB, 1st ed.; Publishing House of Electronics Industry: Beijing, China, 2013; pp. 12–94. [Google Scholar]
- Reymond, F.; Fermin, D.; Lee, H.J.; Girault, H.H. Electrochemistry at liquid/liquid interfaces: Methodology and potential applications. Electrochim. Acta 2000, 45, 2647–2662. [Google Scholar] [CrossRef]
- Ryan, O.; Suk-won, C.; Whitney, G.C.; Fritz, B.P. Chapter 5 Fuel Cell Mass Transport, In Fuel Cell Fundamentals, 3rd ed.; John Wiley and Sons: Hoboken, NJ, USA, 2016; p. 169. [Google Scholar]
- Cha, Q.X. Introduction to Dynamics of Electrode Process, 3rd ed.; Science Press: Beijing, China, 2016; pp. 129–146. [Google Scholar]
- Bard, A.J.; Faulkner, L.R. Electrochemical Methods Fundamentals and Applications, 2nd ed.; Chemical Industry Press: Beijing, China, 2005; pp. 61–93. [Google Scholar]
- Samec, Z. Electrochemistry at the interface between two immiscible electrolyte solutions. Pure Appl. Chem. 2004, 76, 2147–2180. [Google Scholar] [CrossRef]
- Wang, L.S.; Li, Y. Fuel Cell, 2nd ed.; Metallurgical Industry Press: Beijing, China, 2005; pp. 29–30. [Google Scholar]
- Cao, D.X.; Wang, G.L.; Lyu, Y.Z. Fuel Cell System, 1st ed.; BEIHANG University Press: Beijing, China, 2009; pp. 46–48. [Google Scholar]
- Wei, W.C. Technology of Solid Oxide Fuel Cells, 1st ed.; Shanghai Jiaotong University Press: Shanghai, China, 2014; pp. 56–58. [Google Scholar]
- Lu, C.T.; Chiu, Y.W.; Li, M.J.; Hsueh, K.L.; Hung, J.S. Reduction of the Electrode Overpotential of the Oxygen Evolution Reaction by Electrode Surface Modification. Int. J. Electrochem. 2017, 2017, 1–7. [Google Scholar] [CrossRef]

| Parameter | Meaning |
|---|---|
| The anode current density of the forward electrode reaction, A∙cm−2 | |
| The anode current density of the reverse electrode reaction, A∙cm−2 | |
| Anode exchange current density, A∙cm−2 | |
| Cathode exchange current density, A∙cm−2 | |
| j | Net current density, A∙cm−2 |
| jL | The limiting current density, A∙cm−2 |
| Surface concentration of reactant of electrode reaction on the catalyst layer, mol∙cm−2 | |
| Surface concentration of reactant of electrode reaction on the anode catalyst layer, mol∙cm−2 | |
| Surface concentration of product of electrode reaction on the anode catalyst layer, mol∙cm−2 | |
| Surface concentration of reactant of electrode reaction on the cathode catalyst layer, mol∙cm−2 | |
| Surface concentration of product of electrode reaction on the cathode catalyst layer, mol∙cm−2 | |
| Surface concentration of reactant of electrode reaction at equilibrium potential on the anode catalyst layer, mol∙cm−2 | |
| Surface concentration of product of electrode reaction at equilibrium potential on the anode catalyst layer, mol∙cm−2 | |
| Surface concentration of reactant of electrode reaction at equilibrium potential on the cathode catalyst layer, mol∙cm−2 | |
| Surface concentration of product of electrode reaction at equilibrium potential on the cathode catalyst layer, mol∙cm−2 | |
| Concentration of reactant in the flow channel, mol∙cm−2 | |
| Concentration of reactant in the flow channel of the anode, mol∙cm−2 | |
| Concentration of reactant in the flow channel of the cathode, mol∙cm−2 | |
| E | Nernst voltage of hydrogen-oxygen fuel cell, V |
| E0 | Standard reversible voltage of hydrogen-oxygen fuel cell, V |
| E0 | Nernst voltage of hydrogen-oxygen fuel cell determined by the concentration of the reactive gas in the flow channel, V |
| E0* | Nernst voltage of hydrogen-oxygen fuel cell determined by the concentration of the reactive gas at equilibrium potential on the catalyst layer, V |
| ∆ϕ1 | Galvanic potential of the anode, V |
| ∆ϕ2 | Galvanic potential of the cathode, V |
| Concentration overpotential of the anode, V | |
| Concentration overpotential of the cathode, V | |
| n | Number of moles of electrons produced by 1 mol H2 |
| R | Ideal gas constant, 8.314472 J·mol−1·K−1 |
| F | Faraday constant, 96485.33289 C·mol−1 |
| T | The operating temperature of the fuel cell, K |
| f1 | The rate of decay from the activated state to the product, , k—Boltzmann constant; h—Planck constant. |
| αa | Transfer coefficient of anode electrode reaction |
| αc | Transfer coefficient of cathode electrode reaction |
| Activation loss of the anode, V | |
| Activation loss of the cathode, V | |
| δa | Thickness of anodic electrode (diffusion layer), cm |
| The effective reactant diffusivity within anodic electrode, cm2/s | |
| vohmic | Ohmic loss, V |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lyu, J.; Kudiiarov, V.; Lider, A. Corrections of Voltage Loss in Hydrogen-Oxygen Fuel Cells. Batteries 2020, 6, 9. https://doi.org/10.3390/batteries6010009
Lyu J, Kudiiarov V, Lider A. Corrections of Voltage Loss in Hydrogen-Oxygen Fuel Cells. Batteries. 2020; 6(1):9. https://doi.org/10.3390/batteries6010009
Chicago/Turabian StyleLyu, Jinzhe, Viktor Kudiiarov, and Andrey Lider. 2020. "Corrections of Voltage Loss in Hydrogen-Oxygen Fuel Cells" Batteries 6, no. 1: 9. https://doi.org/10.3390/batteries6010009
APA StyleLyu, J., Kudiiarov, V., & Lider, A. (2020). Corrections of Voltage Loss in Hydrogen-Oxygen Fuel Cells. Batteries, 6(1), 9. https://doi.org/10.3390/batteries6010009







