A Water-Based Fire-Extinguishing Agent of Lithium Iron Phosphate Battery Fire via an Analytic Hierarchy Process-Fuzzy TOPSIS Decision-Marking Method
Abstract
:1. Introduction
2. Methodology
3. Materials and Methods
3.1. Evaluation Index
3.1.1. Technical Index
3.1.2. Economic Index
3.1.3. Environmental Protection Index
3.1.4. Applicability Index
3.2. AHP
3.3. TOPSIS Method
3.4. AHP–Fuzzy TOPSIS Method
4. Results and Discussion
4.1. Determination of Evaluation Index Weights
4.2. Normalization of Fuzzy Decision Matrix
4.3. Determination of Optimal Scheme and the Worst Scheme
4.4. Calculation of the Distance Between Multiple Assessment Objects and the Best and Worst Object
4.5. Calculating the Proximity Between Each Assessment Scheme and the Best and Worst Object
5. Conclusions and Future Directions
- (1)
- The comprehensive performance index system of the fire-extinguishing agent was classified, including eleven second-level indexes, including fire extinguishing time, cooling effect, number of thermal runaway batteries, H2 concentration reduction, cost, GWP, ALT, NOAEL, boiling point, electric conductivity, and residual quantity. The mode is only suitable for assessing the water-based fire extinguishing for suppressing lithium iron phosphate battery fire.
- (2)
- The AHP was used to calculate the weights of the eleven evaluation indexes, and the consistency test was finished. The weight value of fire extinguishing time is 0.228, which is the largest among the 11 s-level indexes. Therefore, flame retardant should be considered a significant ingredient of fire-extinguishing agents used for LIBs. The weight value of the NOAEL index is 0.022, which is the smallest among these second-level indexes. The proposed method possesses the more correct and rational direction in determining the best fire-extinguishing agent.
- (3)
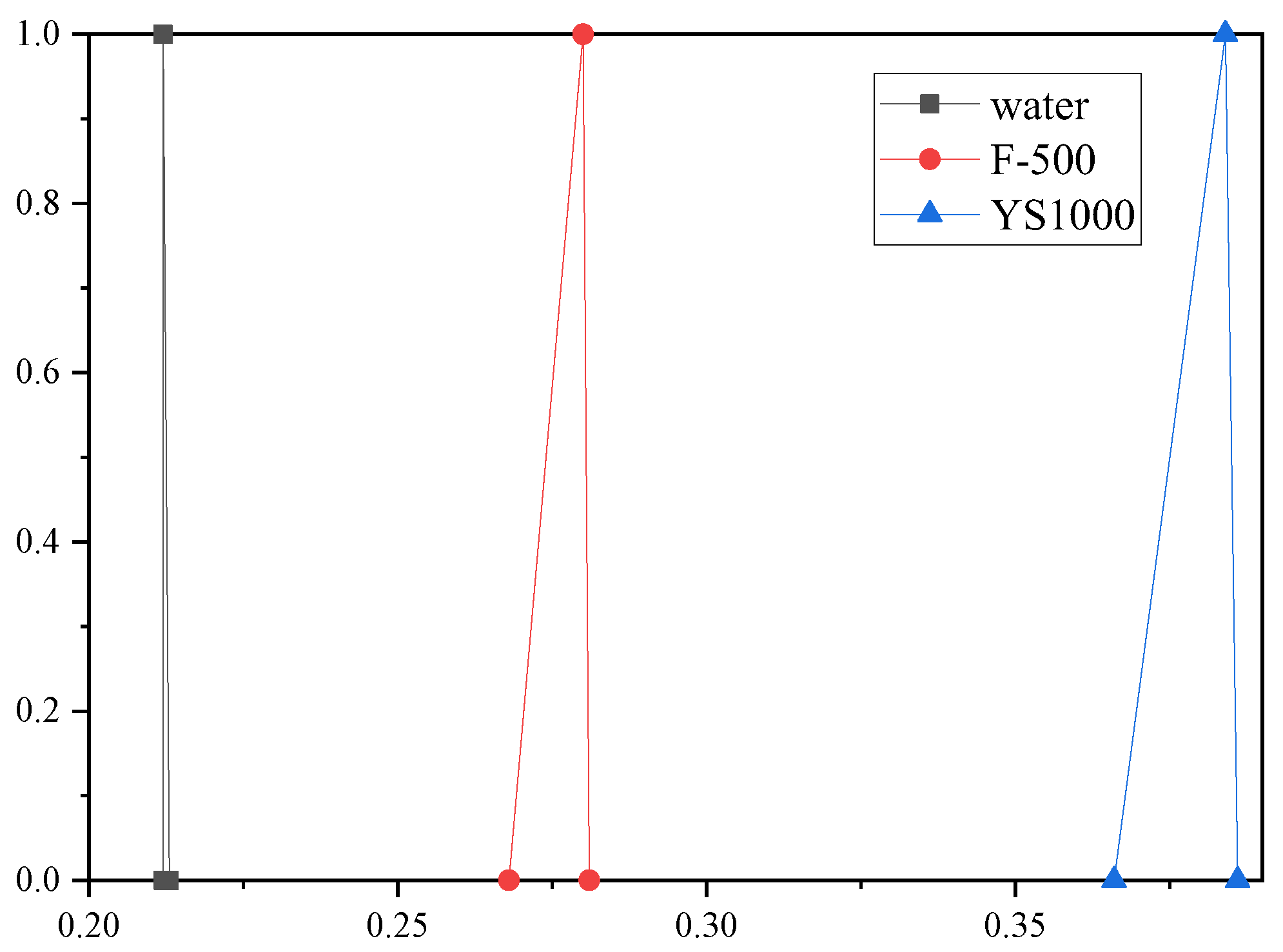
- The AHP–fuzzy TOPSIS calculated method was established, which is used to sort the comprehensive quality of the water-based fire-extinguishing agent used for LIBs fire selection accurately and objectively. The rank of comprehensive properties of fire-extinguishing agents was obtained as follows: YS1000 > F-500 > pure water. The calculated result is consistent with the tested result, which proved the feasibility of the approach. It provides an idea for the selection of other water-based fire-extinguishing agents used for lithium iron phosphate batteries.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhao, F.Q.; Bai, F.L.; Liu, X.L.; Liu, Z.W. A Review on Renewable Energy Transition under China’s Carbon Neutrality Target. Sustainability 2022, 14, 15006. [Google Scholar] [CrossRef]
- Baird, A.R.; Archibald, E.J.; Marr, K.C.; Ezekoye, O.A. Explosion hazards from lithium-ion battery vent gas. J. Power Sources 2020, 446, 13. [Google Scholar] [CrossRef]
- Lyon, R.E.; Walters, R.N. Energetics of lithium ion battery failure. J. Hazard. Mater. 2016, 318, 164–172. [Google Scholar] [CrossRef]
- Feng, X.N.; Lu, L.G.; Ouyang, M.G.; Li, J.Q.; He, X.M. A 3D thermal runaway propagation model for a large format lithium ion battery module. Energy 2016, 115, 194–208. [Google Scholar] [CrossRef]
- Ping, P.; Wang, Q.S.; Huang, P.F.; Li, K.; Sun, J.H.; Kong, D.P.; Chen, C.H. Study of the fire behavior of high-energy lithium-ion batteries with full-scale burning test. J. Power Sources 2015, 285, 80–89. [Google Scholar] [CrossRef]
- Chen, M.Y.; Liu, J.H.; He, Y.P.; Yuen, R.; Wang, J. Study of the fire hazards of lithium-ion batteries at different pressures. Appl. Therm. Eng. 2017, 125, 1061–1074. [Google Scholar] [CrossRef]
- Larsson, F.; Andersson, P.; Blomqvist, P.; Mellander, B.E. Toxic fluoride gas emissions from lithium-ion battery fires. Sci. Rep. 2017, 7, 13. [Google Scholar] [CrossRef]
- Sun, J.; Zhou, T.; Yang, K.; Wei, S.P.; Tang, N.; Dang, N.N.; Li, H.; Qiu, X.P.; Chen, L.Q. Toxicity, a serious concern of thermal runaway from commercial Li-ion battery. Nano Energy 2016, 27, 313–319. [Google Scholar] [CrossRef]
- Larsson, F.; Andersson, P.; Blomqvist, P.; Lorén, A.; Mellander, B.E. Characteristics of lithium-ion batteries during fire tests. J. Power Sources 2014, 271, 414–420. [Google Scholar] [CrossRef]
- Fu, Y.Y.; Lu, S.; Li, K.Y.; Liu, C.C.; Cheng, X.D.; Zhang, H.P. An experimental study on burning behaviors of 18650 lithium ion batteries using a cone calorimeter. J. Power Sources 2015, 273, 216–222. [Google Scholar] [CrossRef]
- Tao, C.F.; Ye, Q.P.; Wang, C.M.; Qian, Y.J.; Wang, C.F.; Zhou, T.T.; Tang, Z.G. An experimental investigation on the burning behaviors of lithium ion batteries after different immersion times. J. Clean. Prod. 2020, 242, 10. [Google Scholar] [CrossRef]
- Chen, M.Y.; Ouyang, D.X.; Weng, J.W.; Liu, J.H.; Wang, J. Environmental pressure effects on thermal runaway and fire behaviors of lithium-ion battery with different cathodes and state of charge. Process Saf. Environ. Prot. 2019, 130, 250–256. [Google Scholar] [CrossRef]
- Xu, J.J.; Guo, P.Y.; Duan, Q.L.; Yu, X.C.; Zhang, L.; Liu, Y.J.; Wang, Q.S. Experimental study of the effectiveness of three kinds of extinguishing agents on suppressing lithium-ion battery fires. Appl. Therm. Eng. 2020, 171, 9. [Google Scholar] [CrossRef]
- Zhao, J.; Xue, F.; Fu, Y.; Cheng, Y.; Yang, H.; Lu, S. A comparative study on the thermal runaway inhibition of 18650 lithium-ion batteries by different fire extinguishing agents. iScience 2021, 24, 102854. [Google Scholar] [CrossRef]
- Liu, Y.J.; Duan, Q.L.; Xu, J.J.; Li, H.; Sun, J.H.; Wang, Q.S. Experimental study on a novel safety strategy of lithium-ion battery integrating fire suppression and rapid cooling. J. Energy Storage 2020, 28, 9. [Google Scholar] [CrossRef]
- Zhang, L.; Li, Y.Q.; Duan, Q.L.; Chen, M.; Xu, J.J.; Zhao, C.P.; Sun, J.H.; Wang, Q.S. Experimental study on the synergistic effect of gas extinguishing agents and water mist on suppressing lithium-ion battery fires. J. Energy Storage 2020, 32, 10. [Google Scholar] [CrossRef]
- Jia, H.L.; Shen, H.D.; Xiang, H.J.; Li, D.H.; Zhai, R.P. Analysis of the fire-extinguishing effect and the weakening of flame intensification of nonionic liquid water mist. Combust. Sci. Technol. 2020, 192, 902–914. [Google Scholar]
- Joseph, P.; Nichols, E.; Noyozhilov, V. A comparative study of the effects of chemical additives on the suppression efficiency of water mist. Fire Saf. J. 2013, 58, 221–225. [Google Scholar] [CrossRef]
- Zhang, T.W.; Han, Z.Y.; Du, Z.M.; Zhang, Z.L.; Liu, K. Application of thermal mechanism to evaluate the effectiveness of the extinguishment of CH4/air cup-burner flame by water mist with additives. Int. J. Hydrogen Energy 2016, 41, 15078–15088. [Google Scholar] [CrossRef]
- Cao, X.Y.; Ren, J.J.; Zhou, Y.H.; Wang, Q.J.; Gao, X.L.; Bi, M.S. Suppression of methane/air explosion by ultrafine water mist containing sodium chloride additive. J. Hazard. Mater. 2015, 285, 311–318. [Google Scholar] [CrossRef]
- Yuan, S.; Chang, C.Y.; Yan, S.S.; Zhou, P.; Qian, X.M.; Yuan, M.Q.; Liu, K. A review of fire-extinguishing agent on suppressing lithium-ion batteries fire. J. Energy Chem. 2021, 62, 262–280. [Google Scholar] [CrossRef]
- Zhou, Y.X.; Bai, J.L.; Wang, Z.R.; Wang, J.L.; Bai, W. Inhibition of thermal runaway in lithium-ion batteries by fine water mist containing a low-conductivity compound additive. J. Clean. Prod. 2022, 340, 130841. [Google Scholar] [CrossRef]
- Yuan, S.; Chang, C.Y.; Zhang, J.Q.; Liu, Y.F.; Qian, X.M. Experimental investigation of a micelle encapsulator F-500 on suppressing lithium ion phosphate batteries fire and rapid cooling. J. Loss Prev. Process Ind. 2022, 79, 104816. [Google Scholar] [CrossRef]
- Yuan, S.; Chang, C.Y.; Zhou, Y.; Zhang, R.H.; Zhang, J.Q.; Liu, Y.F.; Qian, X.M. The extinguishment mechanisms of a micelle encapsulator F-500 on lithium-ion battery fires. J. Energy Storage 2022, 55, 105186. [Google Scholar] [CrossRef]
- Naziris, I.A.; Lagaros, N.D.; Papaioannou, K. Optimized fire protection of cultural heritage structures based on the analytic hierarchy process. J. Build. Eng. 2016, 8, 292–304. [Google Scholar] [CrossRef]
- Abrahamsen, E.B.; Milazzo, M.F.; Selvik, J.T.; Asche, F.; Abrahamsen, H.B.J.R.E.; Safety, S. Prioritising investments in safety measures in the chemical industry by using the Analytic Hierarchy Process. Reliab. Eng. Syst. Saf. 2020, 198, 106811. [Google Scholar] [CrossRef]
- Li, M.K.; Liu, Y.K.; Peng, M.J.; Xie, C.L.; Yang, L.Q. The decision making method of task arrangement based on analytic hierarchy process for nuclear safety in radiation field. Prog. Nucl. Energy 2016, 93, 318–326. [Google Scholar] [CrossRef]
- You, D.; Fan, Y.Y.; Zheng, F.B. Basin water environmental safety assessment based on fuzzy comprehensive evaluation method: A case study. Desalination Water Treat. 2018, 121, 316–322. [Google Scholar] [CrossRef]
- Wang, G.; Li, J.; Shen, L.; Ding, S.; Shi, Z.; Zuo, F. Towards efficient and accurate prediction of freeway accident severity using two-level fuzzy comprehensive evaluation. Heliyon 2024, 10, e36396. [Google Scholar] [CrossRef]
- Bortolan, G.; Degani, R. A review of some methods for ranking fuzzy subsets. Fuzzy Sets Syst. 1985, 15, 1–19. [Google Scholar] [CrossRef]
- Aiello, G.; Enea, M.; Galante, G.; La Scalia, G. Clean agent selection approached by fuzzy TOPSIS decision-making method. Fire Technol. 2009, 45, 405–418. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, H.; Qi, Z. An application of nonlinear fuzzy analytic hierarchy process in safety evaluation of coal mine. Saf. Sci. 2016, 86, 78–87. [Google Scholar] [CrossRef]
- Jin, L.; Liu, P.; Yao, W.; Wei, J.; Li, Z. Safety assessment of existing subgrade retaining wall based on a combined Weighting-TOPSIS evaluation method. Dev. Built Environ. 2024, 18, 100420. [Google Scholar] [CrossRef]
- Han, Y.; Cui, S.; Geng, Z.; Li, H.; Chu, C. Risk early warning and control of food safety based on an improved analytic hierarchy process integrating quality control analysis method. Food Control 2020, 108, 106824. [Google Scholar]
- Meng, X.; Jiang, L.; Duan, Q.; Wang, S.; Duan, P.; Wei, Z.; Zhang, L.; Jia, Z.; Jin, K.; Wang, Q. Experimental study on exploration of optimum extinguishing agent for 243 Ah lithium iron phosphate battery fires. Process Saf. Environ. 2023, 177, 138–151. [Google Scholar] [CrossRef]
- Wang, H.; Xu, H.; Zhang, Z.; Wang, Q.; Jin, C.; Wu, C.; Xu, C.; Hao, J.; Sun, L.; Du, Z. Fire and explosion characteristics of vent gas from lithium-ion batteries after thermal runaway: A comparative study. eTransportation 2022, 13, 100190. [Google Scholar] [CrossRef]
- Rohilla, M.; Saxena, A.; Dixit, P.K.; Mishra, G.K.; Narang, R. Aerosol forming compositions for fire fighting applications: A review. Fire Technol. 2019, 55, 2515–2545. [Google Scholar] [CrossRef]
- Li, X.; Zhou, Z.; Zhang, M.; Zhang, F.; Zhou, X. A liquid cooling technology based on fluorocarbons for lithium-ion battery thermal safety. J. Loss Prev. Process Ind. 2022, 78, 104818. [Google Scholar] [CrossRef]
- AlKheder, S.; Al Otaibi, H.; Al Baghli, Z.; Al Ajmi, S.; Alkhedher, M. Analytic hierarchy process (AHP) assessment of Kuwait mega construction projects’ complexity. Eng. Constr. Archit. Manag. 2023, 32, 1903–1946. [Google Scholar] [CrossRef]
- Pamučar, D.S.; Božanić, D.I.; Kurtov, D. Fuzzification of the Saaty’s scale and a presentation of the hybrid fuzzy AHP-TOPSIS model: An example of the selection of a Brigade Artillery Group firing position in a defensive operation. Mil. Tech. Cour. 2016, 64, 966–986. [Google Scholar] [CrossRef]
- Deng, X.; Li, J.; Zeng, H.; Chen, J.; Zhao, J. Research on computation methods of AHP wight vector and its applications (in Chinese). Math. Pract. Theory 2012, 42, 93–100. [Google Scholar]
- Yuan, S.; Wang, K.; Chang, C.; Zhang, J.; Chen, J.; Qian, X.M. Inhibition effect and extinguishment mechanisms of YS1000 microemulsion for lithium iron phosphate battery fires. J. Energy Storage 2024, 89, 111698. [Google Scholar] [CrossRef]
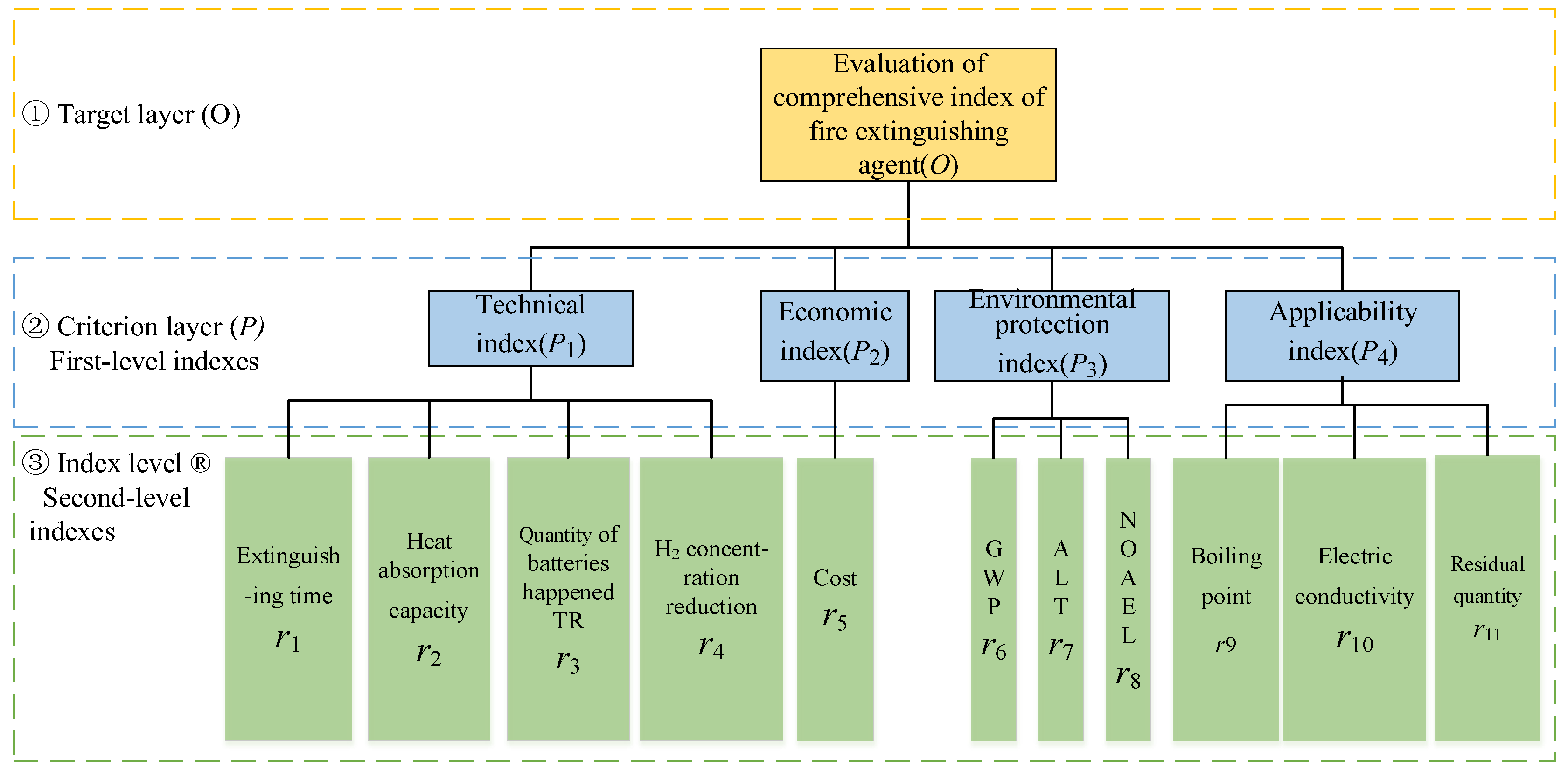

| No. | First-Level Indexes | Second-Level Indexes |
|---|---|---|
| 1 | Technical index | Extinguishing time (s) |
| 2 | Heat absorption capacity (kJ) | |
| 3 | Number of batteries happen to TR | |
| 4 | H2 concentration reduction (ppm) | |
| 5 | Economic index | Cost (RMB/L) |
| 6 | Environmental protection index | GWP |
| 7 | ALT | |
| 8 | NOAEL | |
| 9 | Applicability index | Boiling point at 1 atm (°C) |
| 10 | Electric conductivity (μs∙cm−1) | |
| 11 | Residual quantity |
| O-P | P1 | P2 | P3 | P4 |
|---|---|---|---|---|
| P1 | 1 | 5 | 5 | 3 |
| P2 | 1/5 | 1 | 1 | 1/2 |
| P3 | 1/5 | 1 | 1 | 1/2 |
| P4 | 1/3 | 2 | 2 | 1 |
| P1-r | r1 | r2 | r3 | r4 |
|---|---|---|---|---|
| r1 | 1 | 3 | 5 | 1 |
| r2 | 1/3 | 1 | 2 | 1/3 |
| r3 | 1/5 | 1/2 | 1 | 1/4 |
| r4 | 1 | 3 | 4 | 1 |
| P3-r | r6 | r7 | r8 |
|---|---|---|---|
| r6 | 1 | 2 | 3 |
| r7 | 1/2 | 1 | 1 |
| r8 | 1/3 | 1 | 1 |
| P4-r | r9 | r10 | r11 |
|---|---|---|---|
| r9 | 1 | 1/4 | 1 |
| r10 | 4 | 1 | 5 |
| r11 | 1 | 1/5 | 1 |
| Rank (n) | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
|---|---|---|---|---|---|---|---|---|---|---|
| Ri | 0.00 | 0.00 | 0.52 | 0.89 | 1.12 | 1.25 | 1.35 | 1.42 | 1.46 | 1.49 |
| P1-r | P3-r | P4-r | |
|---|---|---|---|
| 4.02 | 3.02 | 3.01 | |
| Ci | 0.0065 | 0.0091 | 0.0028 |
| Ri | 0.89 | 0.52 | 0.52 |
| CR | 0.0073 | 0.0176 | 0.0053 |
| P-r Sort | O-P Rank | Hierarchical Total Sort Weight | |||
|---|---|---|---|---|---|
| P1 = 0.571 | P2 = 0.110 | P3 = 0.110 | P4 = 0.210 | ||
| r1 | 0.40 | 0.228 | |||
| r2 | 0.14 | 0.080 | |||
| r3 | 0.08 | 0.046 | |||
| r4 | 0.38 | 0.217 | |||
| r5 | 1 | 0.110 | |||
| r6 | 0.55 | 0.061 | |||
| r7 | 0.24 | 0.026 | |||
| r8 | 0.20 | 0.022 | |||
| r9 | 0.16 | 0.034 | |||
| r10 | 0.69 | 0.145 | |||
| r11 | 0.15 | 0.033 | |||
| Qualitative Level | Description | Write Code | Grade Function |
|---|---|---|---|
| Level 1 | Extremely Disagree | ED | (0.00, 0.00, 0.20) |
| Level 2 | Very Disagree | VD | (0.10, 0.20, 0.30) |
| Level 3 | Disagree | D | (0.20, 0.30, 0.40) |
| Level 4 | Moderately Disagree | MD | (0.30, 0.40, 0.50) |
| Level 5 | Neutral | N | (0.40, 0.50, 0.60) |
| Level 6 | Moderately Agree | MA | (0.50, 0.60, 0.70) |
| Level 7 | Agree | A | (0.60, 0.70, 0.80) |
| Level 8 | Very Agree | VA | (0.70, 0.80, 0.90) |
| Level 9 | Extremely Agree | EA | (0.80, 1.00, 1.00) |
| Primary Index | Secondary Index | Water | 3%F-500 | YS1000 |
|---|---|---|---|---|
| Technical index | Extinguishing time (s) | 30 | 22 | 1 |
| Qc (kJ) | 43.7 | 118.2 | 74.6 | |
| Number of batteries happen to TR | 3 | 1 | 1.5 | |
| H2 concentration reduction (ppm) | 259 | 462 | 439 | |
| Economic index | Cost (YMB/L) | 0.004 | 150 | 16.6 |
| Environmental index | GWP | (0.00, 0.00, 0.20) | (0.00, 0.00, 0.20) | (0.00, 0.00, 0.20) |
| ALT | (0.00, 0.00, 0.20) | (0.00, 0.00, 0.20) | (0.00, 0.00, 0.20) | |
| NOAEL | (0.00, 0.00, 0.20) | (0.10, 0.20, 0.30) | (0.20, 0.30, 0.40) | |
| Applicable index | Boiling point (°C) | 100 | 120 | ≈100 |
| Electric conductivity (μs∙cm−1) | 4.48 | 416 | 26.9 | |
| Residual quantity | (0.00, 0.00, 0.20) | (0.20, 0.30, 0.40) | (0.20, 0.30, 0.40) |
| Denomination | r1 | r2 | r3 | r4 | r5 | r6 | r7 | r8 | r9 | r10 | r11 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Water | 0.000 | 0.000 | 0.000 | 0.000 | 1.000 | (0.00, 0.00, 0.20) | (0.00, 0.00, 0.20) | (0.00, 0.00, 0.20) | 1.000 | 1.000 | (0.00, 0.00, 0.20) |
| 3%F-500 | 0.276 | 1.000 | 1.000 | 1.000 | 0.000 | (0.00, 0.00, 0.20) | (0.00, 0.00, 0.20) | (0.10, 0.20, 0.30) | 0.000 | 0.000 | (0.20, 0.30, 0.40) |
| YS1000 | 1.000 | 0.415 | 0.750 | 0.887 | 0.889 | (0.00, 0.00, 0.20) | (0.00, 0.00, 0.20) | (0.20, 0.30, 0.40) | 0.000 | 0.946 | (0.20, 0.30, 0.40) |
| Denomination | r1 | r2 | r3 | r4 | r5 | r6 | r7 | r8 | r9 | r10 | r11 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Water | 0.000 | 0.000 | 0.000 | 0.000 | 0.110 | (0.000, 0.000, 0.012) | (0.000, 0.000, 0.005) | (0.000, 0.000, 0.004) | 0.034 | 0.145 | (0.000, 0.000, 0.007) |
| 3%F-500 | 0.063 | 0.080 | 0.046 | 0.217 | 0.000 | (0.000, 0.000, 0.012) | (0.000, 0.000, 0.005) | (0.002, 0.004, 0.007) | 0.000 | 0.000 | (0.007, 0.010, 0.013) |
| YS1000 | 0.228 | 0.033 | 0.035 | 0.192 | 0.098 | (0.000, 0.000, 0.012) | (0.000, 0.000, 0.005) | (0.004, 0.007, 0.008) | 0.000 | 0.137 | (0.007, 0.010, 0.013) |
| 0.228 | 0.228 | 0.228 | 0.228 | 0.228 | (0.228, 0.228, 0.228) | (0.228, 0.228, 0.228) | (0.228, 0.228, 0.228) | 0.228 | 0.228 | (0.228, 0.228, 0.228) | |
| 0.000 | 0.000 | 0.000 | 0.000 | 0.000 | (0.000, 0.000, 0.000) | (0.000, 0.000, 0.000) | (0.000, 0.000, 0.000) | 0.000 | 0.000 | (0.000, 0.000, 0.000) |
| Denomination | |||
|---|---|---|---|
| Water | (0.689, 0.689, 0.684) | (0.185, 0.185, 0.185) | (0.212, 0.212, 0.213) |
| 3%F-500 | (0.665, 0.626, 0.624) | (0.244, 0.244, 0.244) | (0.268, 0.280, 0.281) |
| YS1000 | (0.599, 0.554, 0.551) | (0.346, 0.346, 0.346) | (0.366, 0.384, 0.386) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, S.; Wang, K.; Tai, F.; Cheng, D.; Zhang, Q.; Cui, Y.; Qian, X.; Sun, C.; Liu, S.; Chen, X. A Water-Based Fire-Extinguishing Agent of Lithium Iron Phosphate Battery Fire via an Analytic Hierarchy Process-Fuzzy TOPSIS Decision-Marking Method. Batteries 2025, 11, 182. https://doi.org/10.3390/batteries11050182
Yuan S, Wang K, Tai F, Cheng D, Zhang Q, Cui Y, Qian X, Sun C, Liu S, Chen X. A Water-Based Fire-Extinguishing Agent of Lithium Iron Phosphate Battery Fire via an Analytic Hierarchy Process-Fuzzy TOPSIS Decision-Marking Method. Batteries. 2025; 11(5):182. https://doi.org/10.3390/batteries11050182
Chicago/Turabian StyleYuan, Shuai, Kuo Wang, Feng Tai, Donghao Cheng, Qi Zhang, Yujie Cui, Xinming Qian, Chunwen Sun, Song Liu, and Xin Chen. 2025. "A Water-Based Fire-Extinguishing Agent of Lithium Iron Phosphate Battery Fire via an Analytic Hierarchy Process-Fuzzy TOPSIS Decision-Marking Method" Batteries 11, no. 5: 182. https://doi.org/10.3390/batteries11050182
APA StyleYuan, S., Wang, K., Tai, F., Cheng, D., Zhang, Q., Cui, Y., Qian, X., Sun, C., Liu, S., & Chen, X. (2025). A Water-Based Fire-Extinguishing Agent of Lithium Iron Phosphate Battery Fire via an Analytic Hierarchy Process-Fuzzy TOPSIS Decision-Marking Method. Batteries, 11(5), 182. https://doi.org/10.3390/batteries11050182








