Experimental Analysis of Battery Cell Heating Through Electromagnetic Induction-Based Liquid System Considering Induction Power and Flow Rate Effects in Extreme-Cold Conditions
Abstract
1. Introduction
1.1. Background
1.2. Problems and Literature Review
1.3. Research Objectives
2. Materials and Methods
2.1. Experimental Setup
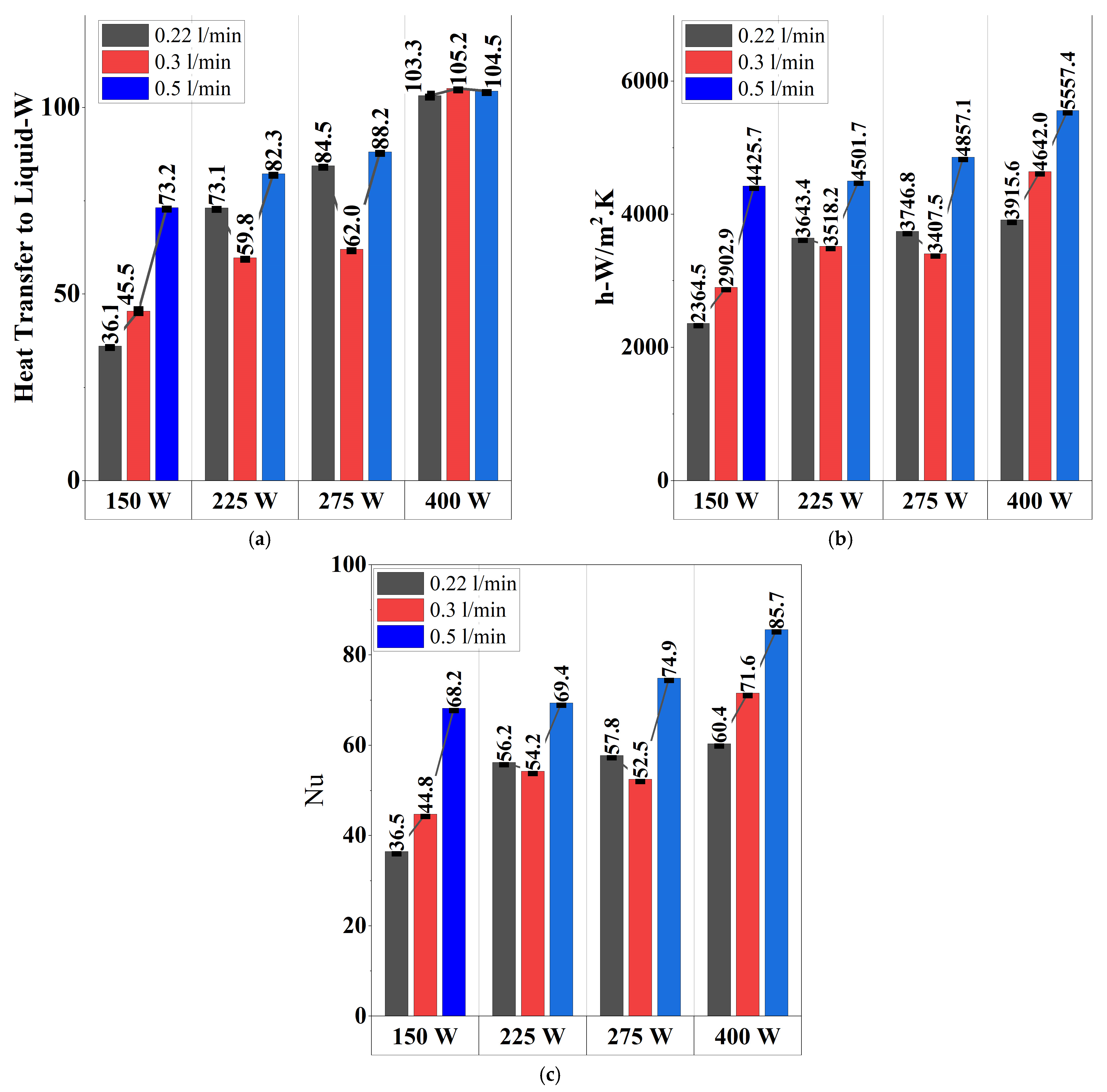
2.2. Heat Transfer Analysis and Dimensionless Parameters
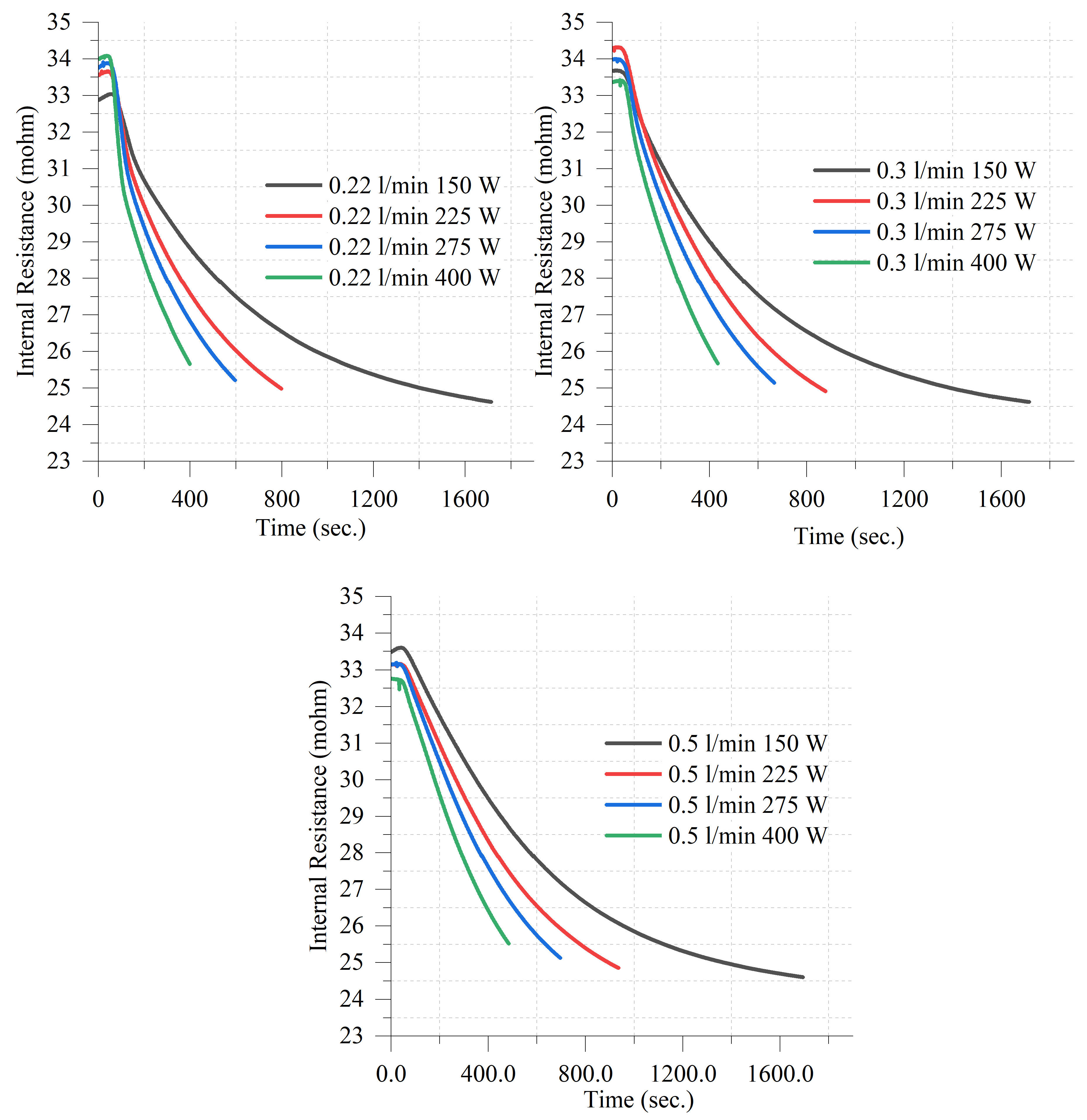
3. Results and Discussion
3.1. Evaluation of the Results at −15 °C Conditions
3.2. Evaluation of the Results at −5 °C Conditions
4. Conclusions
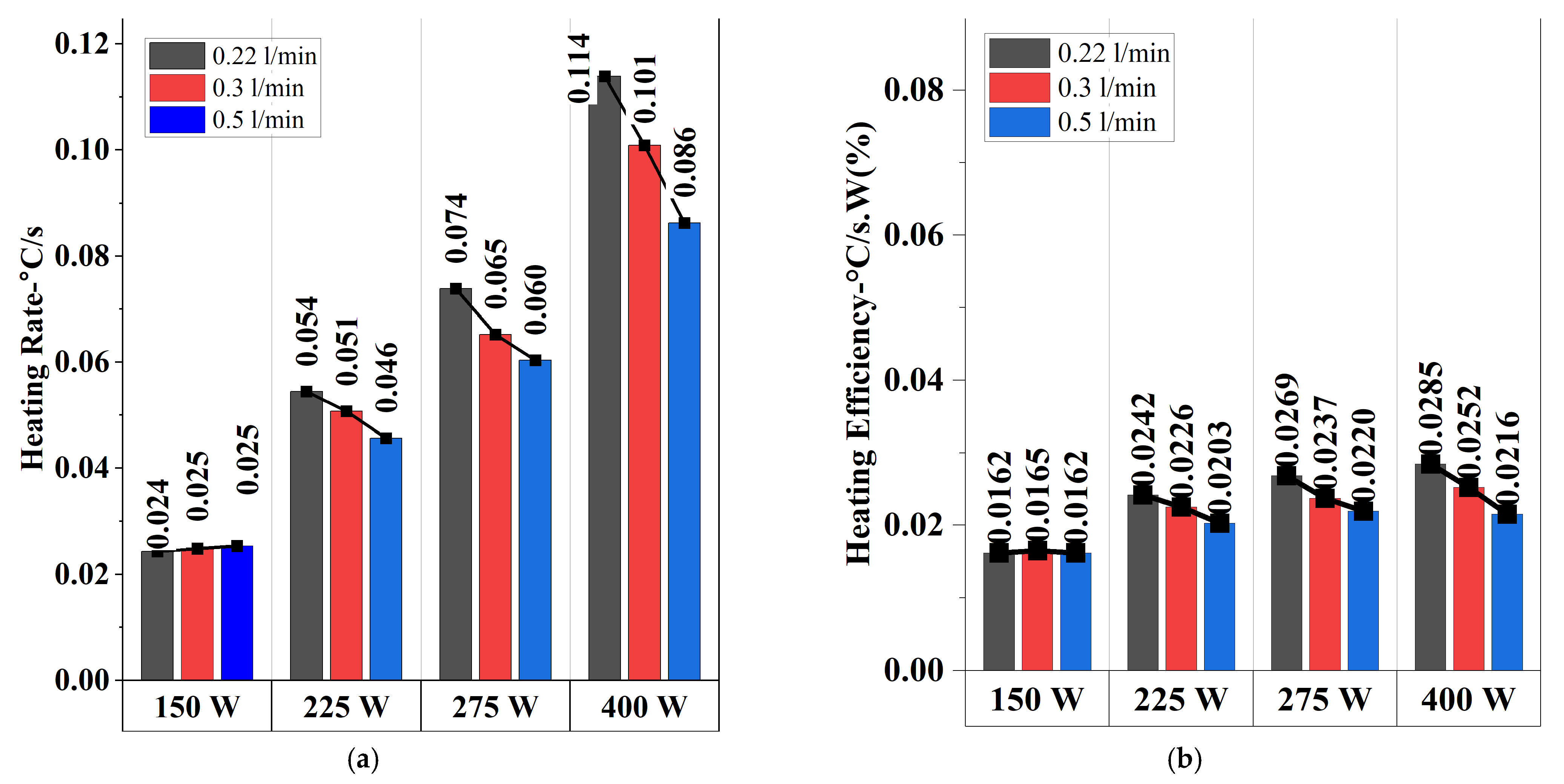
- Higher induction power resulted in faster heating, with 400 W providing the shortest heating durations across all conditions.
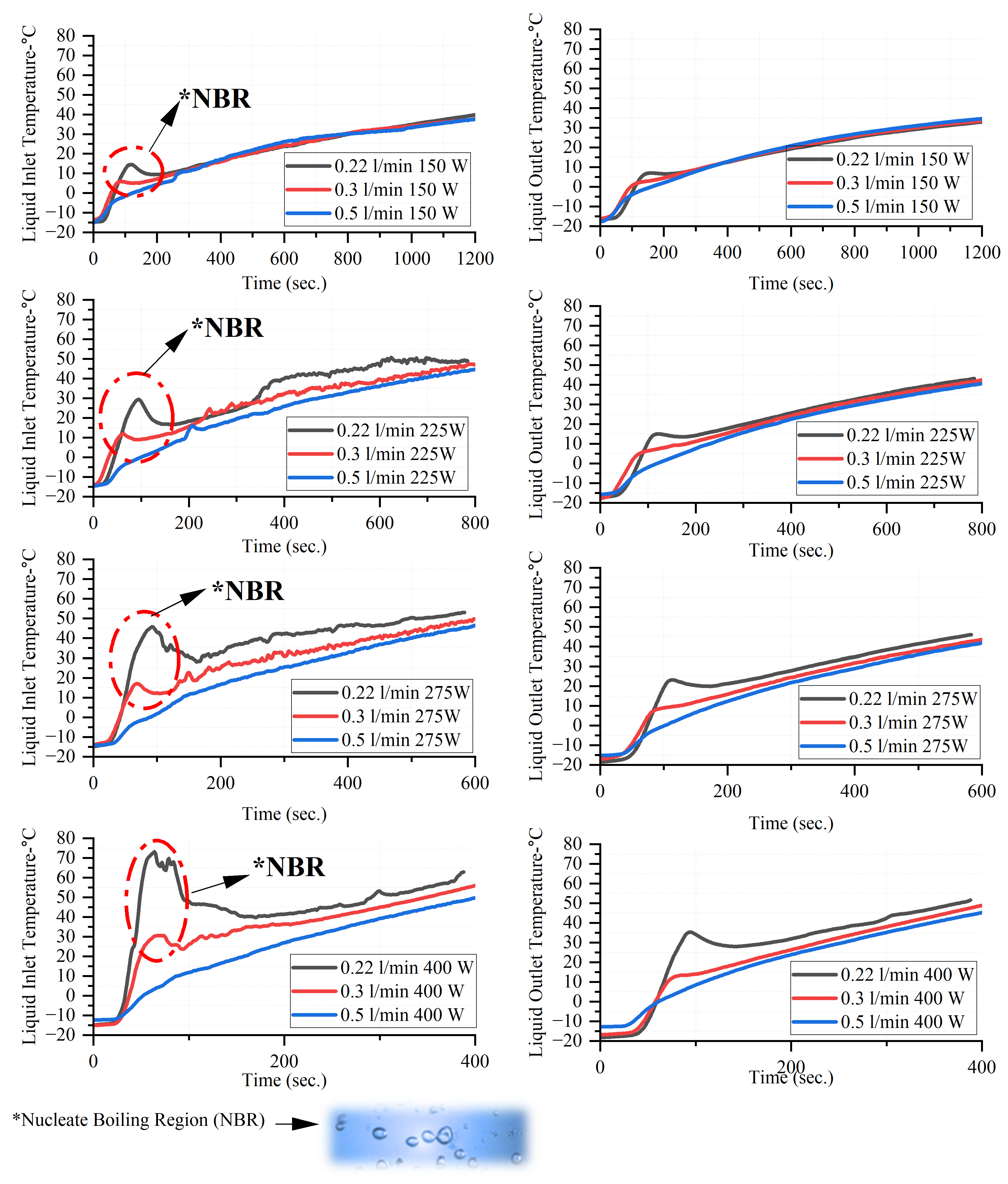
- The highest heating rates were observed at 0.22 L/min, where longer thermal interaction enhanced heat absorption. However, at higher power levels, localized nucleate boiling effects caused minor temperature fluctuations in this condition.
- A flow rate of 0.3 L/min provided the most stable and efficient heating, balancing rapid heat absorption and uniform temperature distribution while avoiding boiling-induced fluctuations.
- Higher flow rates (0.5 L/min) minimized temperature fluctuations but resulted in longer heating times due to increased convective heat dissipation.
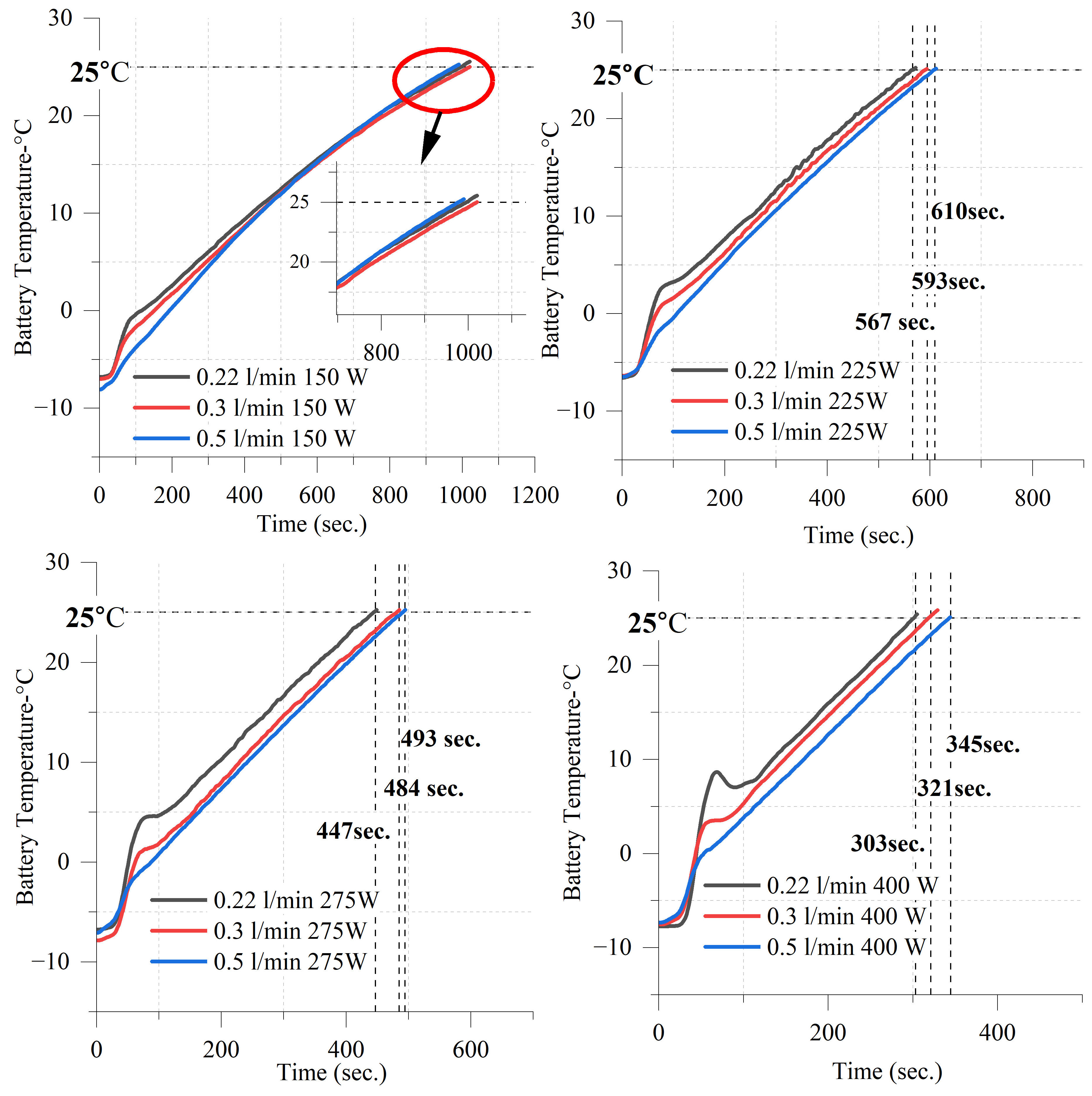
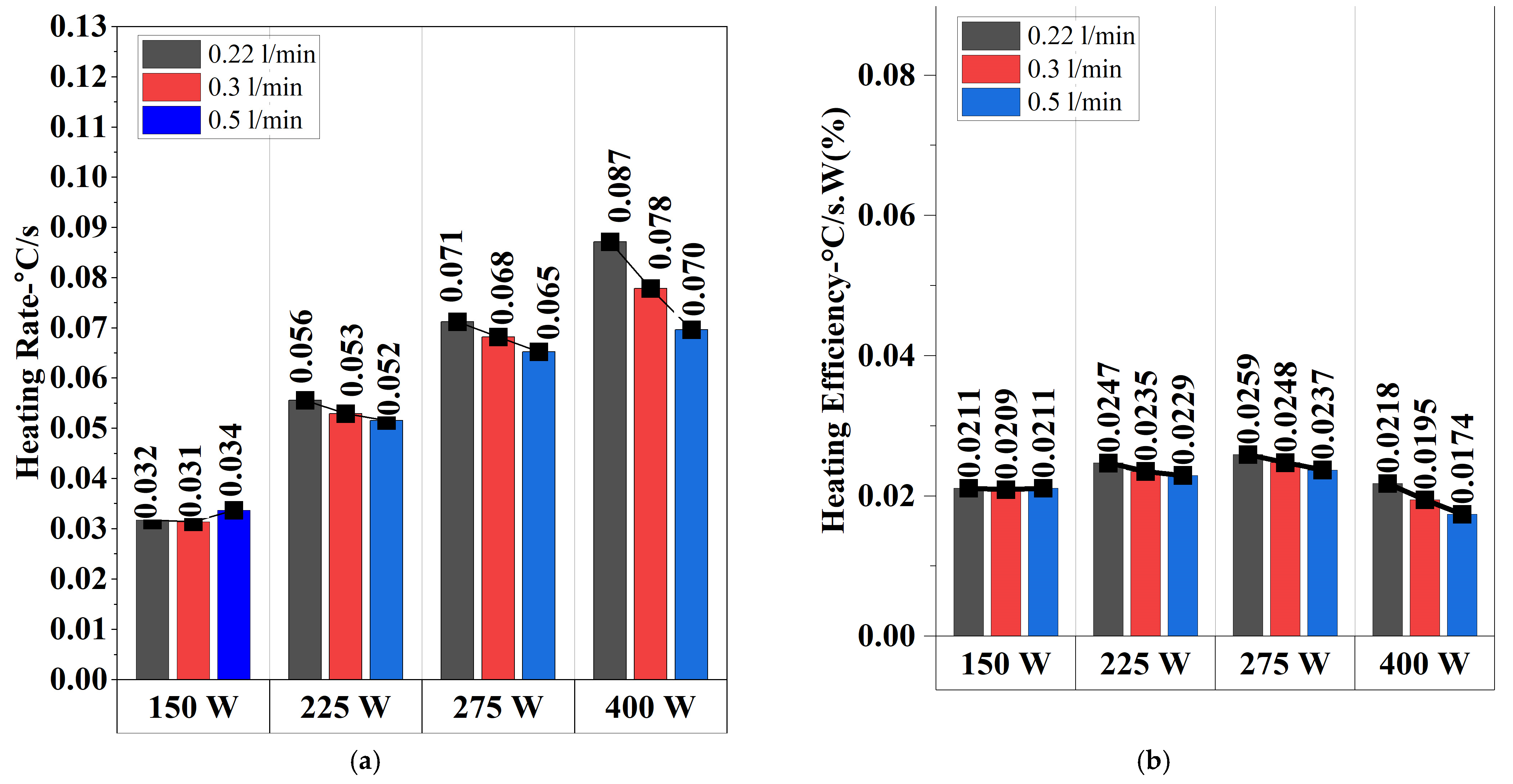
- At −5 °C, the fastest heating occurred at 400 W and 0.22 L/min, reaching 0 °C in 47 s (0.1064 °C/s) and 25 °C in 303 s (0.0990 °C/s).
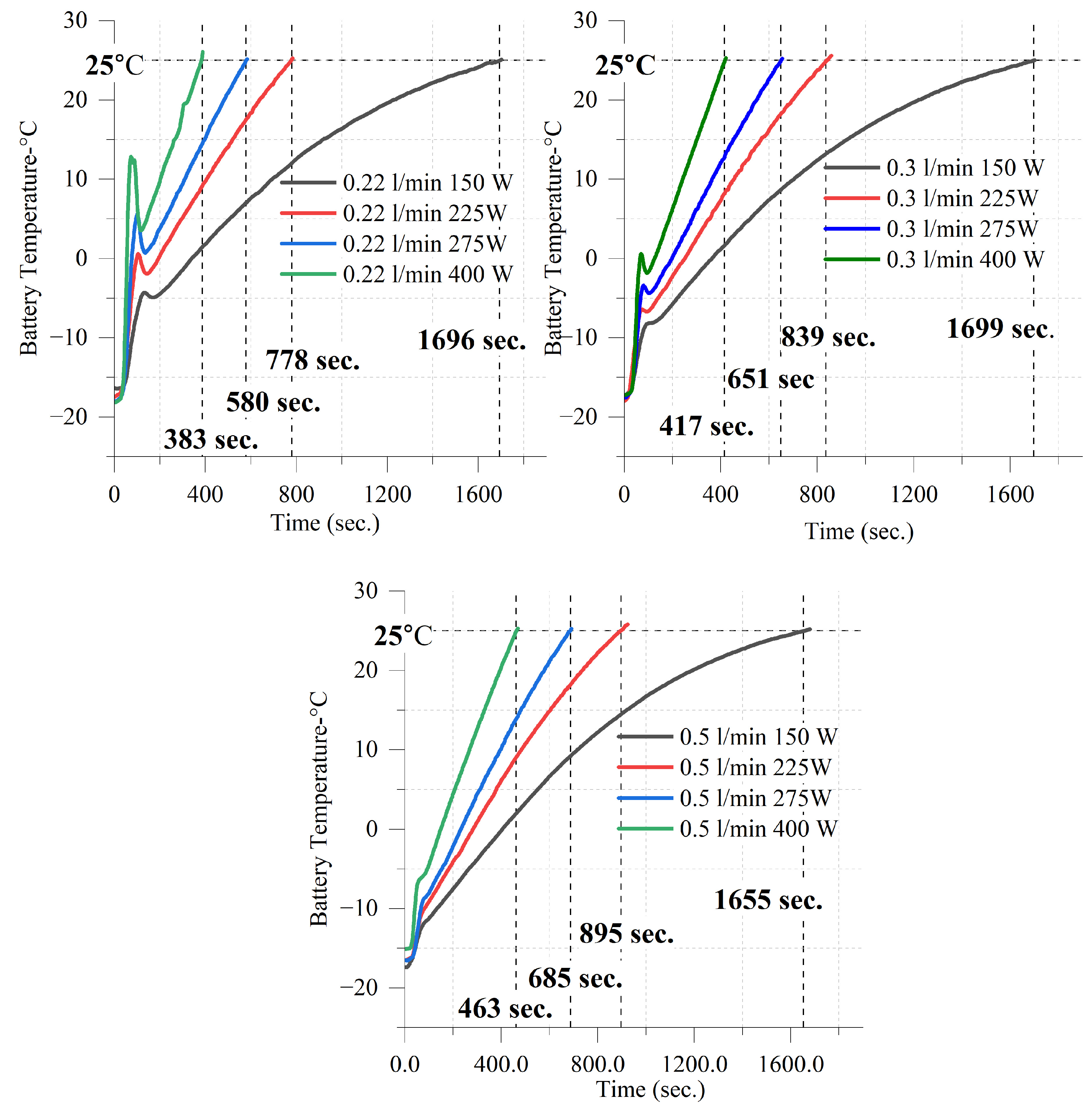
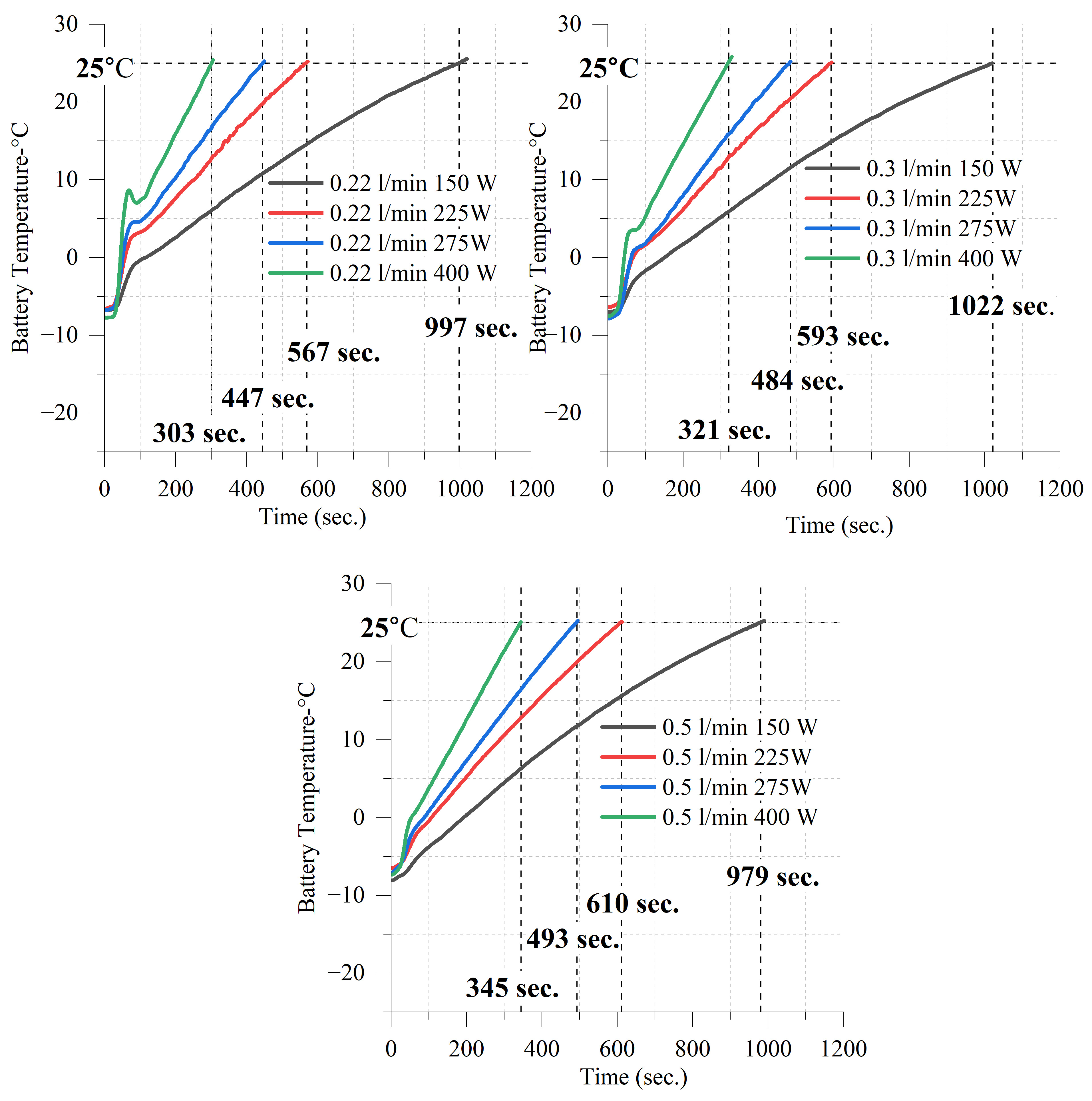
- At −15 °C, the fastest heating was also recorded at 400 W and 0.22 L/min, reaching 0 °C in 55 s (0.2727 °C/s) and 25 °C in 383 s (0.1044 °C/s).
- The slowest heating occurred at 150 W and 0.5 L/min, where at −5 °C, it required 194 s to reach 0 °C (0.0258 °C/s) and 979 s to reach 25 °C (0.0306 °C/s). Similarly, at −15 °C, 0.5 L/min at 150 W required 149 s to reach 0 °C (0.1007 °C/s) and 463 s to reach 25 °C (0.0864 °C/s).
- The heating rates declined as the battery temperature increased, demonstrating that thermal resistance rises at higher temperatures.
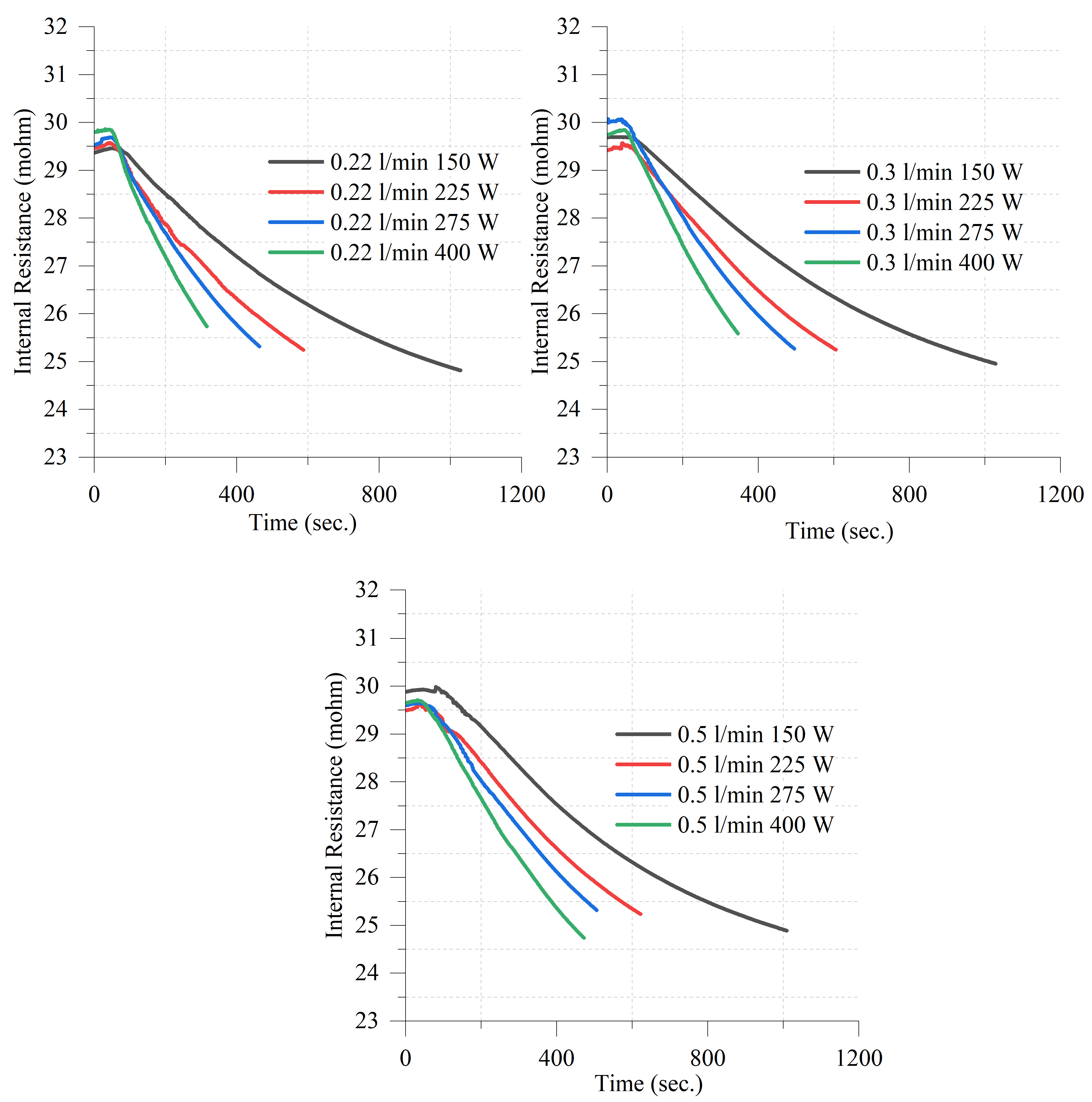
- Internal resistance decreased as the battery warmed up, validating the importance of preheating for improved battery performance in cold environments.
- When comparing −5 °C and −15 °C conditions, it was observed that although heating was more energy-efficient at −5 °C, the heating rate to 0 °C was higher at −15 °C due to the larger temperature gradient. For instance, at 400 W and 0.22 L/min, the heating rate to 0 °C was 0.2727 °C/s at −15 °C, whereas it was only 0.1064 °C/s at −5 °C. This suggests that at lower ambient temperatures, the battery heats up more rapidly in the early phase due to the greater temperature difference between the heating fluid and the battery. However, as the battery temperature rises, the rate of heating slows down, and energy efficiency becomes more favorable at −5 °C.
- Under −15 °C conditions, the fastest heating scenario (0.22 L/min, 400 W) reduced heating time by 344.26% compared to the slowest condition (0.3 L/min, 150 W), while the optimal scenario (0.3 L/min, 400 W) achieved a 308.09% improvement. However, the optimal case exhibited an 8.14% lower heating rate than the fastest condition, emphasizing a balance between rapid heating and thermal stability. In terms of heating efficiency, the optimal condition outperformed the lowest cases (0.22 L/min and 0.5 L/min, 150 W) by 55.56%, while the highest efficiency was observed at 0.22 L/min, 400 W, with a 75.93% improvement over the slowest conditions. Despite this, the optimal scenario’s efficiency remained 11.58% lower than the highest. The results indicate that while the fastest heating and highest efficiency conditions benefit from rapid energy transfer, they also introduce instability due to nucleate boiling. In contrast, the optimal condition ensures a more uniform and stable temperature distribution by mitigating nucleate boiling effects.
- Under −5 °C conditions, the fastest heating scenario (0.22 L/min, 400 W) achieved a 236.73% improvement in the heating rate compared to the slowest condition (0.3 L/min, 150 W), while the optimal scenario (0.3 L/min, 400 W) showed a 215.99% improvement. However, the heating rate in the optimal scenario was 6.16% lower than the fastest case, highlighting a balance between rapid heating and thermal stability due to nucleate boiling suppression. Although the highest heating efficiency was recorded at 275 W with a 0.22 L/min flow rate, exceeding the optimal condition’s efficiency by 24.71%, its heating rate was significantly lower. This suggests that despite achieving higher efficiency, the slower heating rate performance makes this condition less favorable for applications requiring rapid and uniform temperature increases, reinforcing the suitability of the optimal condition (400 W and 0.3 L/min) as a more practical choice.
- The optimal condition for effective heating was identified as 400 W and 0.3 L/min, ensuring both rapid heating and thermal stability without the fluctuations observed at 0.22 L/min.
- The experiments revealed that nucleate boiling occurs when induction power exceeds 275 W at a flow rate of 0.22 L/min, causing intermittent heat transfer disruptions due to vapor bubble formation. This phenomenon leads to local temperature fluctuations and reduced heat transfer efficiency. Such findings are particularly important for battery thermal management systems using induction heating as excessive power input with insufficient flow rates can cause a non-uniform temperature distribution. Therefore, future designs should consider optimizing flow rates based on the applied power level to maintain a stable heating performance without boiling-induced instabilities.
- The heating profile can be dynamically adjusted to optimize pre-heating performance and minimize the occurrence of nucleate boiling. By initially applying a lower power level and progressively increasing it as the bulk temperature rises, the system can maintain stable heat transfer characteristics. Implementing a feedback control mechanism that monitors real-time temperature variations will enable adaptive power regulation, reducing NBR effects and enhancing heating efficiency in extreme-cold conditions.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- IEA. Global EV Outlook 2023, License: CC BY 4.0. Available online: https://www.iea.org/reports/global-ev-outlook-2023 (accessed on 9 March 2025).
- Ortiz, Y.; Arévalo, P.; Peña, D.; Jurado, F. Recent Advances in Thermal Management Strategies for Lithium-Ion Batteries: A Comprehensive Review. Batteries 2024, 10, 83. [Google Scholar] [CrossRef]
- Li, W.; Zhou, Y.; Zhang, H.; Tang, X. A Review on Battery Thermal Management for New Energy Vehicles. Energies 2023, 16, 4845. [Google Scholar] [CrossRef]
- Wang, C.-Y.; Zhang, G.; Ge, S.; Xu, T.; Ji, Y.; Yang, X.-G.; Leng, Y. Lithium-Ion Battery Structure That Self-Heats at Low Temperatures. Nature 2016, 529, 515–518. [Google Scholar] [CrossRef]
- News in English. Available online: https://www.newsinenglish.no/2024/01/10/electric-bus-fleet-froze-in-the-cold (accessed on 5 January 2025).
- The Independent. Available online: https://www.independent.co.uk/tech/electric-car-snow-cold-weather-tesla-b2481002.html (accessed on 8 January 2025).
- Friesen, A.; Horsthemke, F.; Mönnighoff, X.; Brunklaus, G.; Krafft, R.; Börner, M.; Risthaus, T.; Winter, M.; Schappacher, F.M. Impact of Cycling at Low Temperatures on the Safety Behavior of 18650-Type Lithium Ion Cells: Combined Study of Mechanical and Thermal Abuse Testing Accompanied by Post-Mortem Analysis. J. Power Sources 2016, 334, 1–11. [Google Scholar] [CrossRef]
- Lin, H.-P.; Chua, D.; Salomon, M.; Shiao, H.-C.; Hendrickson, M.; Plichta, E.; Slane, S. Low-Temperature Behavior of Li-Ion Cells. Electrochem. Solid-State Lett. 2001, 4, A71–A73. [Google Scholar] [CrossRef]
- Nagasubramanian, G. Electrical Characteristics of 18650 Li-Ion Cells at Low Temperatures. J. Appl. Electrochem. 2001, 31, 99–104. [Google Scholar] [CrossRef]
- Ouyang, M.; Chu, Z.; Lu, L.; Li, J.; Han, X.; Feng, X.; Liu, G. Low Temperature Aging Mechanism Identification and Lithium Deposition in a Large Format Lithium Iron Phosphate Battery for Different Charge Profiles. J. Power Sources 2015, 286, 309–320. [Google Scholar] [CrossRef]
- Zhang, C.-W.; Xu, K.-J.; Li, L.-Y.; Yang, M.-Z.; Gao, H.-B.; Chen, S.-R. Study on a Battery Thermal Management System Based on a Thermoelectric Effect. Energies 2018, 11, 279. [Google Scholar] [CrossRef]
- Lyu, Y.; Siddique, A.R.M.; Majid, S.H.; Biglarbegian, M.; Gadsden, S.A.; Mahmud, S. Electric Vehicle Battery Thermal Management System with Thermoelectric Cooling. Energy Rep. 2019, 5, 822–827. [Google Scholar] [CrossRef]
- Lucía, O.; Maussion, P.; Dede, E.J.; Burdío, J.M. Induction Heating Technology and Its Applications: Past Developments, Current Technology, and Future Challenges. IEEE Trans. Ind. Electron. 2014, 61, 2509–2520. [Google Scholar] [CrossRef]
- Wu, X.; Chen, Z.; Wang, Z. Analysis of Low Temperature Preheating Effect Based on Battery Temperature-Rise Model. Energies 2017, 10, 1121. [Google Scholar] [CrossRef]
- Zhang, C.; Jin, X.; Li, J. PTC Self-Heating Experiments and Thermal Modeling of Lithium-Ion Battery Pack in Electric Vehicles. Energies 2017, 10, 572. [Google Scholar] [CrossRef]
- Zhu, T.; Min, H.; Yu, Y.; Zhao, Z.; Xu, T.; Chen, Y.; Li, X.; Zhang, C. An Optimized Energy Management Strategy for Preheating Vehicle-Mounted Li-Ion Batteries at Subzero Temperatures. Energies 2017, 10, 243. [Google Scholar] [CrossRef]
- Kamble, P. Battery Thermal Management System. Int. J. Eng. Res. 2020, 9, 216–220. [Google Scholar] [CrossRef]
- Ji, Y.; Wang, C.Y. Heating Strategies for Li-Ion Batteries Operated from Subzero Temperatures. Electrochim. Acta 2013, 107, 664–674. [Google Scholar] [CrossRef]
- Raza, W.; Ko, G.S.; Park, Y.C. Induction Heater Based Battery Thermal Management System for Electric Vehicles. Energies 2020, 13, 5711. [Google Scholar] [CrossRef]
- Wang, B.; Yan, M. Research on the Improvement of Lithium-Ion Battery Performance at Low Temperatures Based on Electromagnetic Induction Heating Technology. Energies 2023, 16, 7780. [Google Scholar] [CrossRef]
- Wang, L. Design of Electric Air-Heated Box for Batteries in Electric Vehicles. Chin. J. Power Sources 2013, 37, 1184–1187. [Google Scholar]
- Luo, M.; Song, J.; Ling, Z.; Zhang, Z.; Fang, X. Phase Change Material Coat for Battery Thermal Management with Integrated Rapid Heating and Cooling Functions from −40 °C to 50 °C. Mater. Today Energy 2021, 20, 100652. [Google Scholar] [CrossRef]
- Song, W.; Bai, F.; Chen, M.; Lin, S.; Feng, Z.; Li, Y. Thermal Management of Standby Battery for Outdoor Base Station Based on the Semiconductor Thermoelectric Device and Phase Change Materials. Appl. Therm. Eng. 2018, 137, 203–217. [Google Scholar] [CrossRef]
- Jaffal, H.M.; Mahmoud, N.S.; Imran, A.A.; Hasan, A. Performance Enhancement of a Novel Serpentine Channel Cooled Plate Used for Cooling of Li-Ion Battery Module. Int. J. Therm. Sci. 2023, 184, 107955. [Google Scholar] [CrossRef]
- Wang, J.; Lv, D.; Sha, H.; Lai, C.; Zeng, J.; Gao, T.; Yang, H.; Wu, H.; Jiang, Y. Numerical Investigation on the Thermal Performance of a Battery Pack by Adding Ribs in Cooling Channels. Energies 2024, 17, 4451. [Google Scholar] [CrossRef]

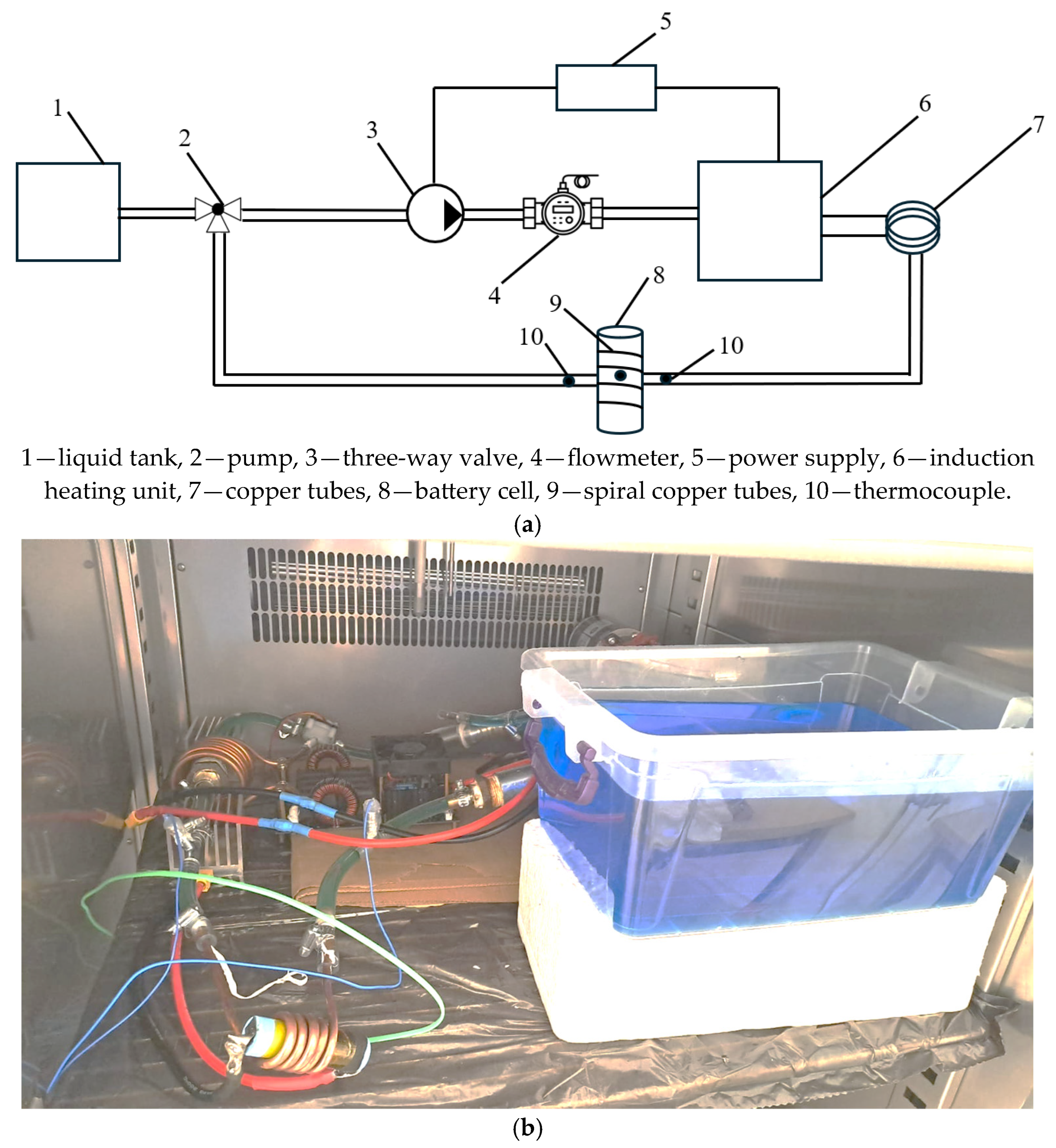



| Study | Method | Key Findings |
|---|---|---|
| Wu et al. [14] | Self-heating in Li-ion batteries | At 2C discharge, temp rose from −10 °C to 5 °C in 280 s, consuming <15% of capacity; at 1C, heating took >1080 s, consuming ~30%. |
| Zhang et al. [15] | External power and PTC self-heating | External power heating achieved 0.629 °C/min; PTC self-heating: 0.459 °C/min at SOC 100%, 0.270 °C/min at SOC 60%. |
| Zhu et al. [16] | PTC heaters integrated with liquid heating | Preheating needs to decrease due to self-heating during operation. |
| Kamble et al. [17] | Resistance heating reliability | Reliable across extreme temperatures (−50 °C to +50 °C). |
| Ji et al. [18] | Comparison of various heating strategies | Convective heating was fastest; mutual pulse heating minimized capacity loss; AC heating ensured uniform temperature distribution. |
| Raza et al. [19] | Induction heating system | Fast heating at −20 °C with 6 kW heater; max heat transfer rate 0.0661 °C/s. |
| Wang and Yan [20] | Induction heating system | Increased temp from −30 °C to +20 °C in <6 min; reduced internal resistance; doubled usable energy and power. |
| Lifang [21] | Electric air-heated box | 11 heating wires raised air temp from 20 °C to 90 °C in 8 min; heated battery pack from −15 °C to 0 °C in 21 min. |
| Luo et al. [22] | Hybrid Joule heating and PCM | Hybrid system maintained stable battery temp, improved safety. |
| Song et al. [23] | Thermoelectric + PCM system | Thermoelectric devices + PCM enhanced temp uniformity, extended battery life in cold climates. |
| Case | Ambient Temperature (°C) | Flow Rate (L/min) | Induction Heating Power (W) |
|---|---|---|---|
| 1 | −15.0 | 0.22 | 150.0 |
| 2 | −15.0 | 0.22 | 225.0 |
| 3 | −15.0 | 0.22 | 275.0 |
| 4 | −15.0 | 0.22 | 400.0 |
| 5 | −15.0 | 0.3 | 150.0 |
| 6 | −15.0 | 0.3 | 225.0 |
| 7 | −15.0 | 0.3 | 275.0 |
| 8 | −15.0 | 0.3 | 400.0 |
| 9 | −15.0 | 0.5 | 150.0 |
| 10 | −15.0 | 0.5 | 225.0 |
| 11 | −15.0 | 0.5 | 275.0 |
| 12 | −15.0 | 0.5 | 400.0 |
| 13 | −5.0 | 0.22 | 150.0 |
| 14 | −5.0 | 0.22 | 225.0 |
| 15 | −5.0 | 0.22 | 275.0 |
| 16 | −5.0 | 0.22 | 400.0 |
| 17 | −5.0 | 0.3 | 150.0 |
| 18 | −5.0 | 0.3 | 225.0 |
| 19 | −5.0 | 0.3 | 275.0 |
| 20 | −5.0 | 0.3 | 400.0 |
| 21 | −5.0 | 0.5 | 150.0 |
| 22 | −5.0 | 0.5 | 225.0 |
| 23 | −5.0 | 0.5 | 275.0 |
| 24 | −5.0 | 0.5 | 400.0 |
| Conditions | Battery Temperatures and Reaching Times (s) | Heating Rate to (°C/s) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| −10 °C | −5 °C | 0 °C | 5 °C | 10 °C | 15 °C | 20 °C | 25 °C | 0 °C | 25 °C | |
| 0.22 L/min—150 W | 78 | 116 | 339 | 507 | 693 | 916 | 1227 | 1696 | 0.0442 | 0.0236 |
| 0.3 L/min—150 W | 71 | 220 | 364 | 522 | 703 | 923 | 1220 | 1699 | 0.0412 | 0.0235 |
| 0.5 L/min—150 W | 133 | 265 | 404 | 550 | 716 | 920 | 1192 | 1655 | 0.0371 | 0.0242 |
| 0.22 L/min—225 W | 59 | 75 | 97 | 296 | 406 | 520 | 638 | 778 | 0.1546 | 0.0514 |
| 0.3 L/min—225 W | 48 | 140 | 251 | 351 | 454 | 566 | 698 | 839 | 0.0598 | 0.0477 |
| 0.5 L/min—225 W | 81 | 182 | 283 | 379 | 484 | 604 | 737 | 895 | 0.0530 | 0.0447 |
| 0.22 L/min—280 W | 58 | 66 | 76 | 95 | 309 | 397 | 482 | 580 | 0.1974 | 0.0690 |
| 0.3 L/min—280 W | 53 | 68 | 197 | 281 | 364 | 452 | 548 | 651 | 0.0761 | 0.0614 |
| 0.5 L/min—280 W | 67 | 153 | 232 | 312 | 397 | 481 | 578 | 685 | 0.0647 | 0.0584 |
| 0.22 L/min—400 W | 46 | 51 | 55 | 59 | 65 | 265 | 325 | 383 | 0.2727 | 0.1044 |
| 0.3 L/min—400 W | 46 | 53 | 66 | 186 | 242 | 301 | 360 | 417 | 0.2273 | 0.0959 |
| 0.5 L/min—400 W | 42 | 94 | 149 | 207 | 269 | 330 | 395 | 463 | 0.1007 | 0.0864 |
| Conditions | Battery Temperatures and Reaching Times (s) | Heating Rate to (°C/s) | ||||||
|---|---|---|---|---|---|---|---|---|
| 0 °C | 5 °C | 10 °C | 15 °C | 20 °C | 25 °C | 0 °C | 25 °C | |
| 0.22 L/min—150 W | 117 | 270 | 421 | 585 | 769 | 997 | 0.0427 | 0.0301 |
| 0.3 L/min—150 W | 152 | 296 | 441 | 598 | 785 | 1022 | 0.0329 | 0.0294 |
| 0.5 L/min—150 W | 194 | 314 | 444 | 594 | 766 | 979 | 0.0258 | 0.0306 |
| 0.22 L/min—225 W | 60 | 149 | 254 | 341 | 452 | 567 | 0.0833 | 0.0529 |
| 0.3 L/min—225 W | 69 | 178 | 271 | 368 | 474 | 593 | 0.0725 | 0.0506 |
| 0.5 L/min—225 W | 109 | 198 | 290 | 390 | 496 | 610 | 0.0459 | 0.0492 |
| 0.22 L/min—280 W | 53 | 111 | 197 | 276 | 358 | 447 | 0.0943 | 0.0671 |
| 0.3 L/min—280 W | 64 | 157 | 231 | 308 | 390 | 484 | 0.0781 | 0.0620 |
| 0.5 L/min—280 W | 90 | 166 | 244 | 323 | 406 | 493 | 0.0556 | 0.0609 |
| 0.22 L/min—400 W | 47 | 55 | 137 | 192 | 249 | 303 | 0.1064 | 0.0990 |
| 0.3 L/min—400 W | 46 | 99 | 151 | 207 | 263 | 321 | 0.1087 | 0.0929 |
| 0.5 L/min—400 W | 56 | 116 | 174 | 229 | 286 | 345 | 0.0893 | 0.0870 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaleli, A.; Sungur, B. Experimental Analysis of Battery Cell Heating Through Electromagnetic Induction-Based Liquid System Considering Induction Power and Flow Rate Effects in Extreme-Cold Conditions. Batteries 2025, 11, 105. https://doi.org/10.3390/batteries11030105
Kaleli A, Sungur B. Experimental Analysis of Battery Cell Heating Through Electromagnetic Induction-Based Liquid System Considering Induction Power and Flow Rate Effects in Extreme-Cold Conditions. Batteries. 2025; 11(3):105. https://doi.org/10.3390/batteries11030105
Chicago/Turabian StyleKaleli, Alirıza, and Bilal Sungur. 2025. "Experimental Analysis of Battery Cell Heating Through Electromagnetic Induction-Based Liquid System Considering Induction Power and Flow Rate Effects in Extreme-Cold Conditions" Batteries 11, no. 3: 105. https://doi.org/10.3390/batteries11030105
APA StyleKaleli, A., & Sungur, B. (2025). Experimental Analysis of Battery Cell Heating Through Electromagnetic Induction-Based Liquid System Considering Induction Power and Flow Rate Effects in Extreme-Cold Conditions. Batteries, 11(3), 105. https://doi.org/10.3390/batteries11030105






