Numerical Investigation of the Thermal Performance of Air-Cooling System for a Lithium-Ion Battery Module Combined with Epoxy Resin Boards
Abstract
1. Introduction
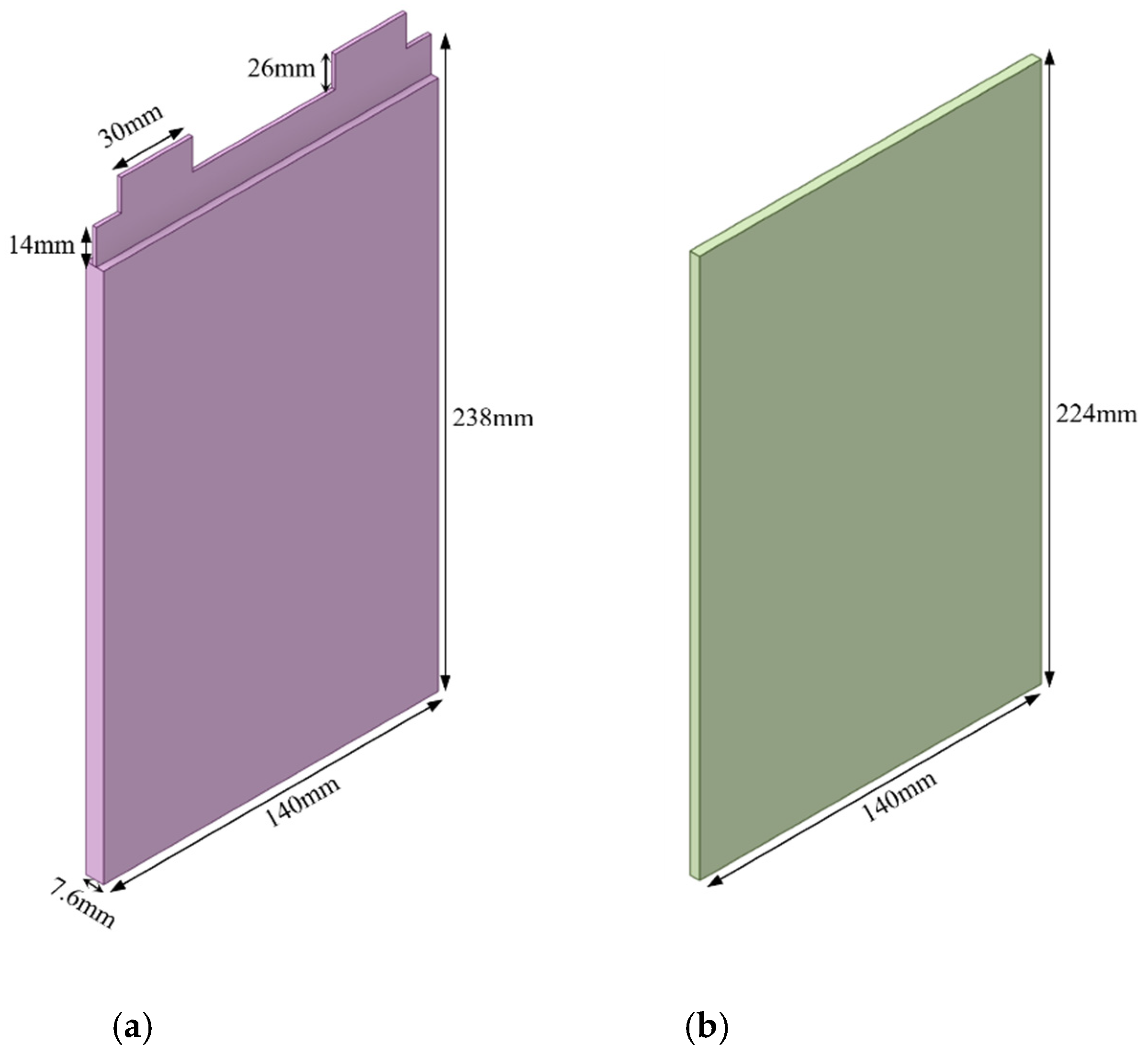
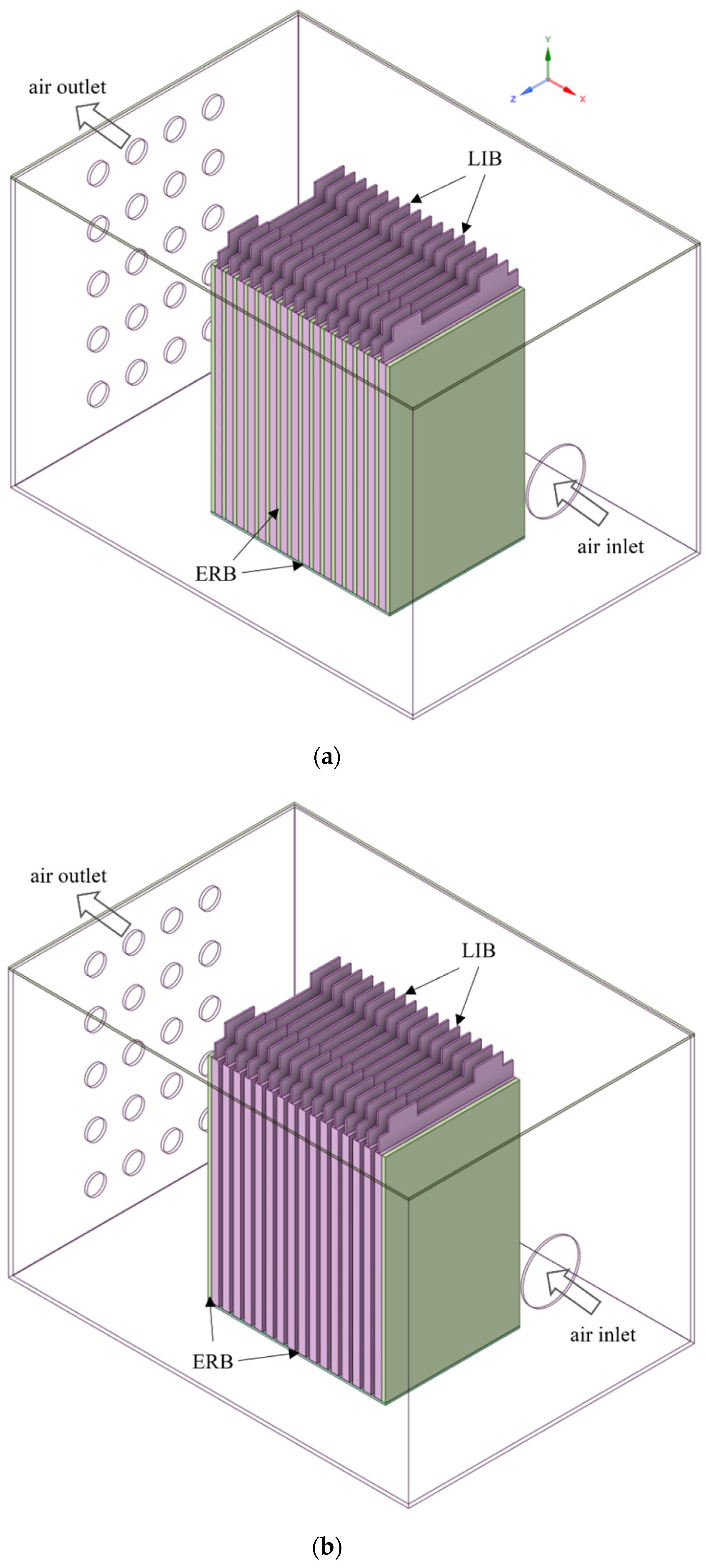
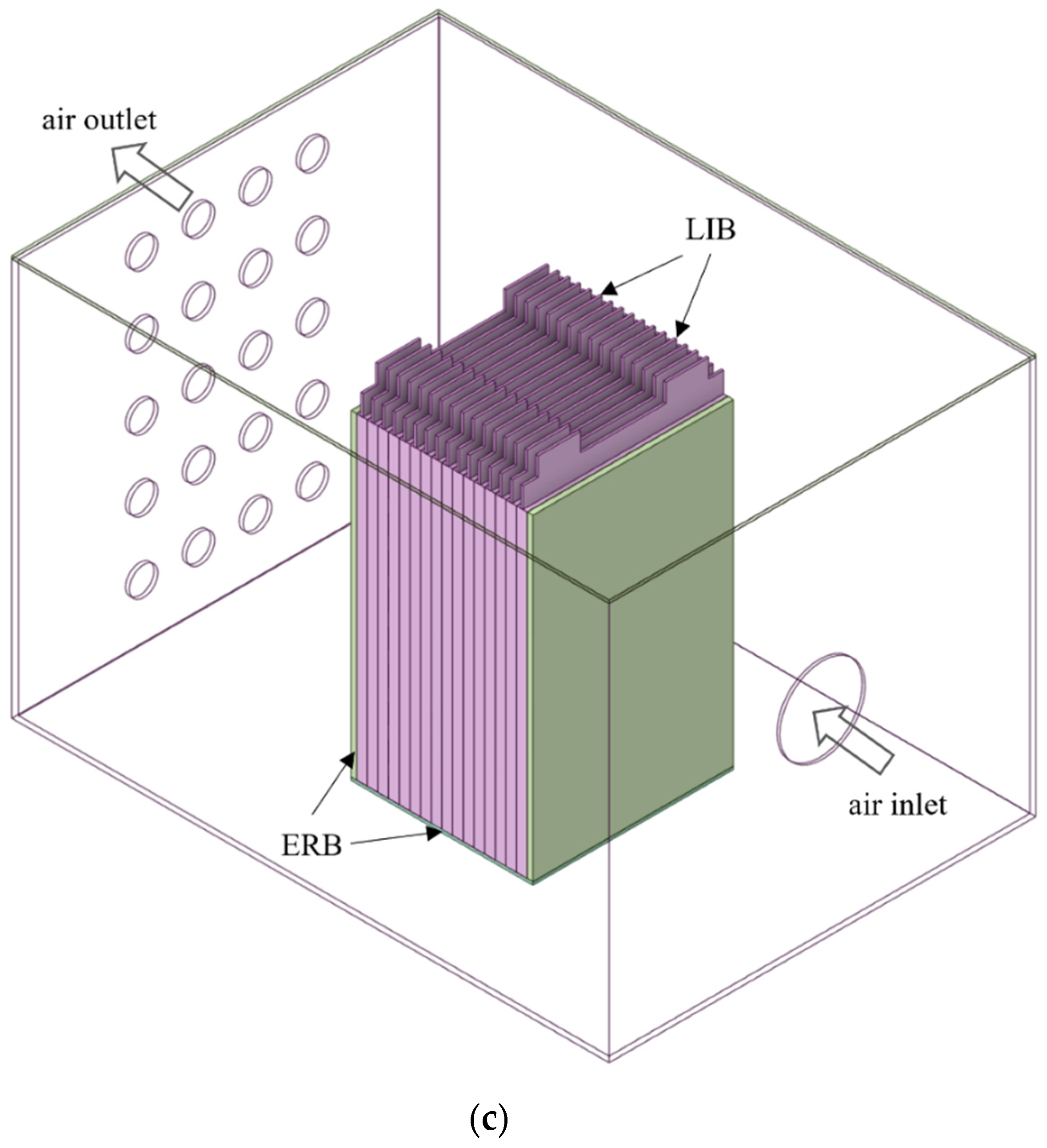
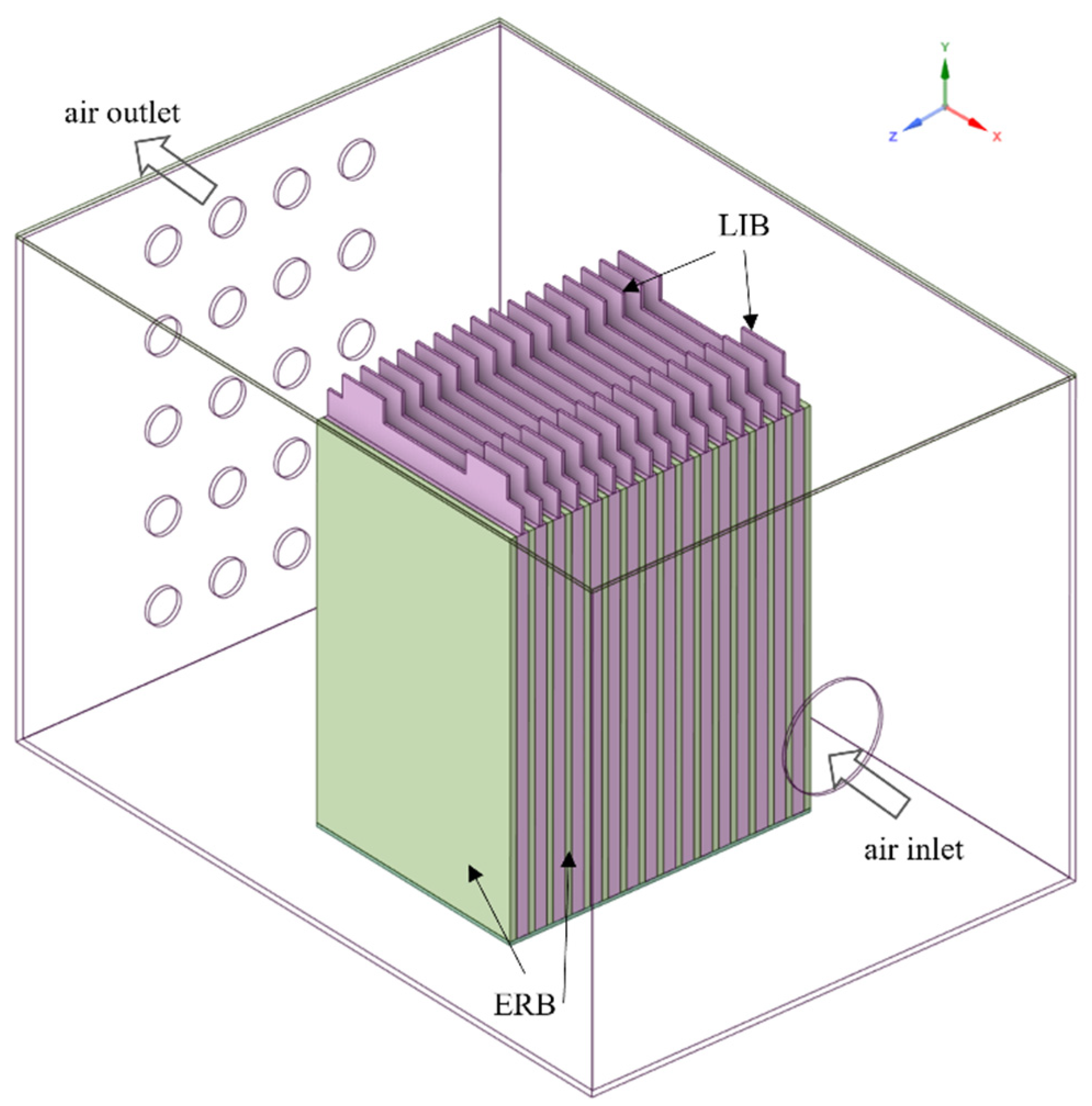
2. Model Description
3. Numerical Description
3.1. Model Assumptions and Governing Equations
3.2. Heat Generation of Battery
3.3. Boundary and Initial Conditions
3.4. Numerical Strategy and Model Validation
4. Results and Discussion
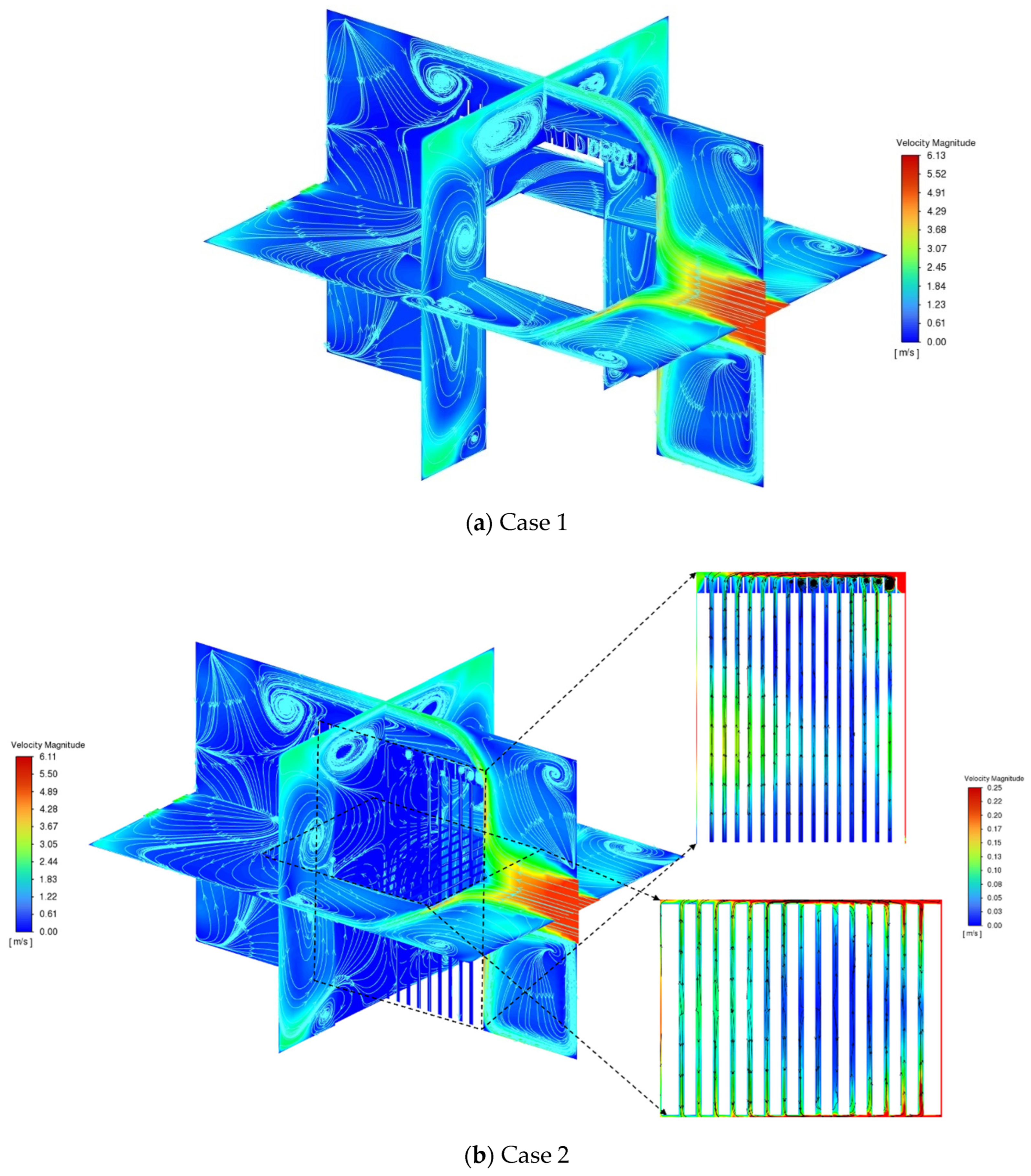
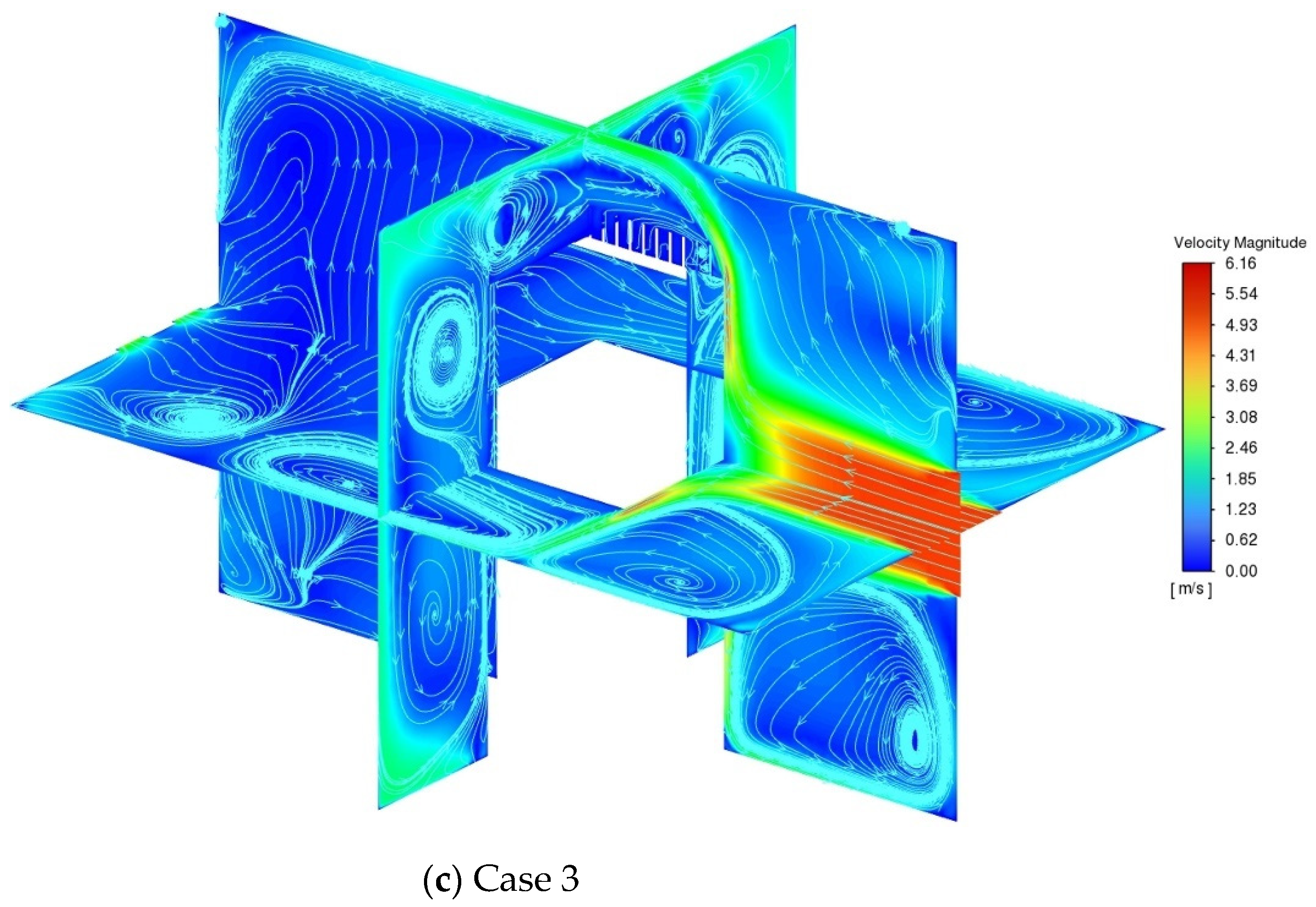
4.1. Streamlines and Velocity Contours
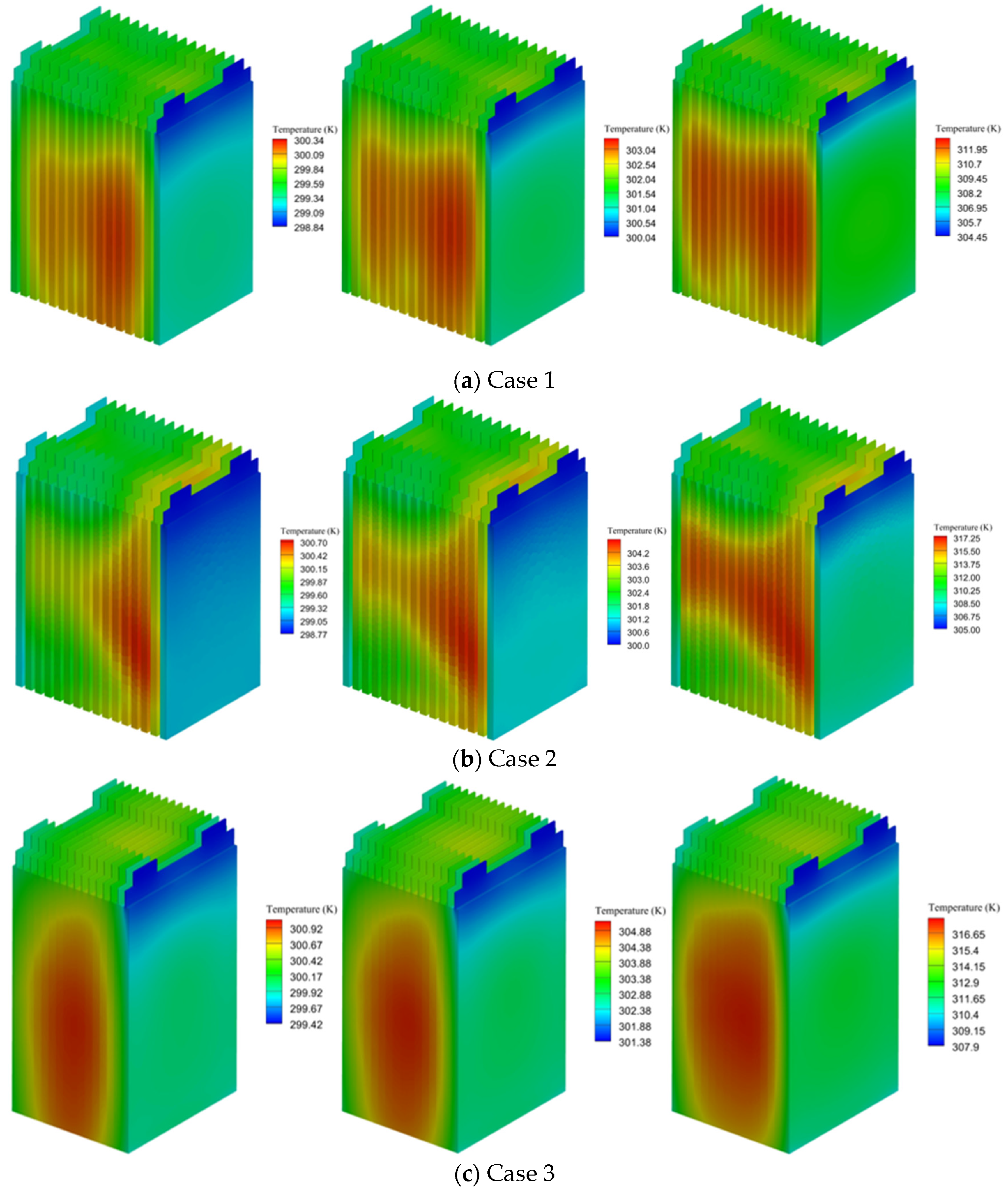
4.2. Temperature Contours
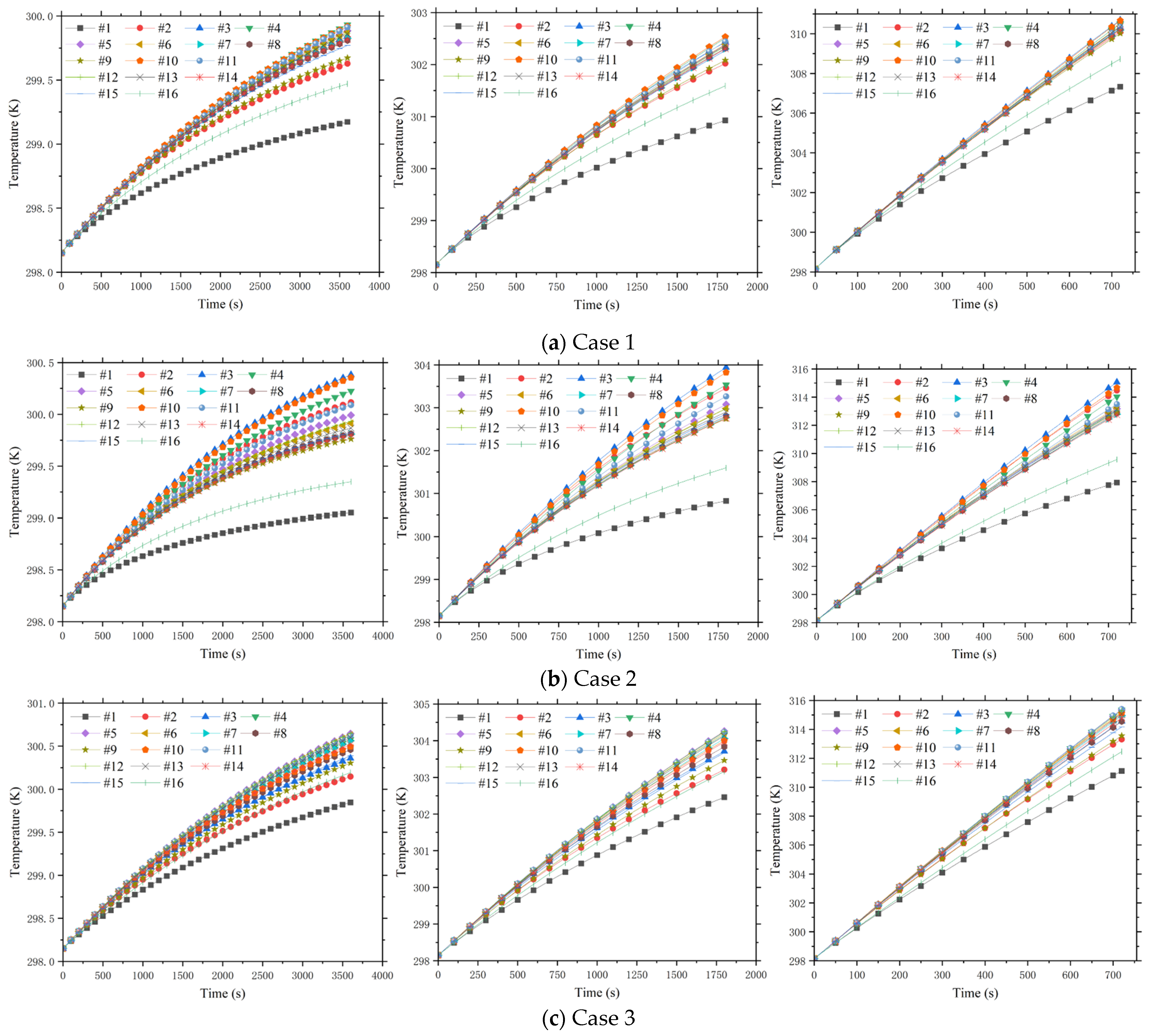
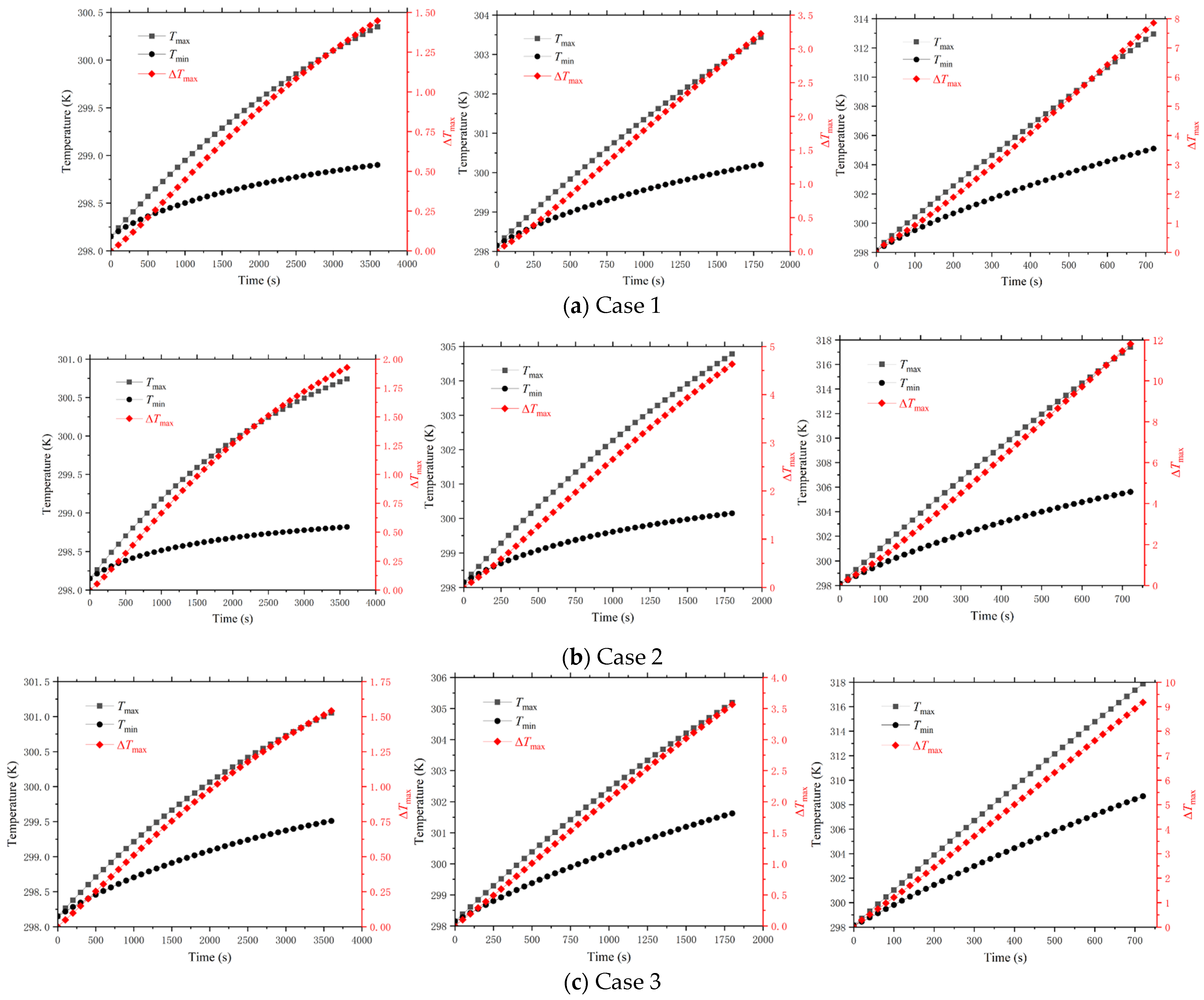
4.3. Temperature Characterization of Single Battery
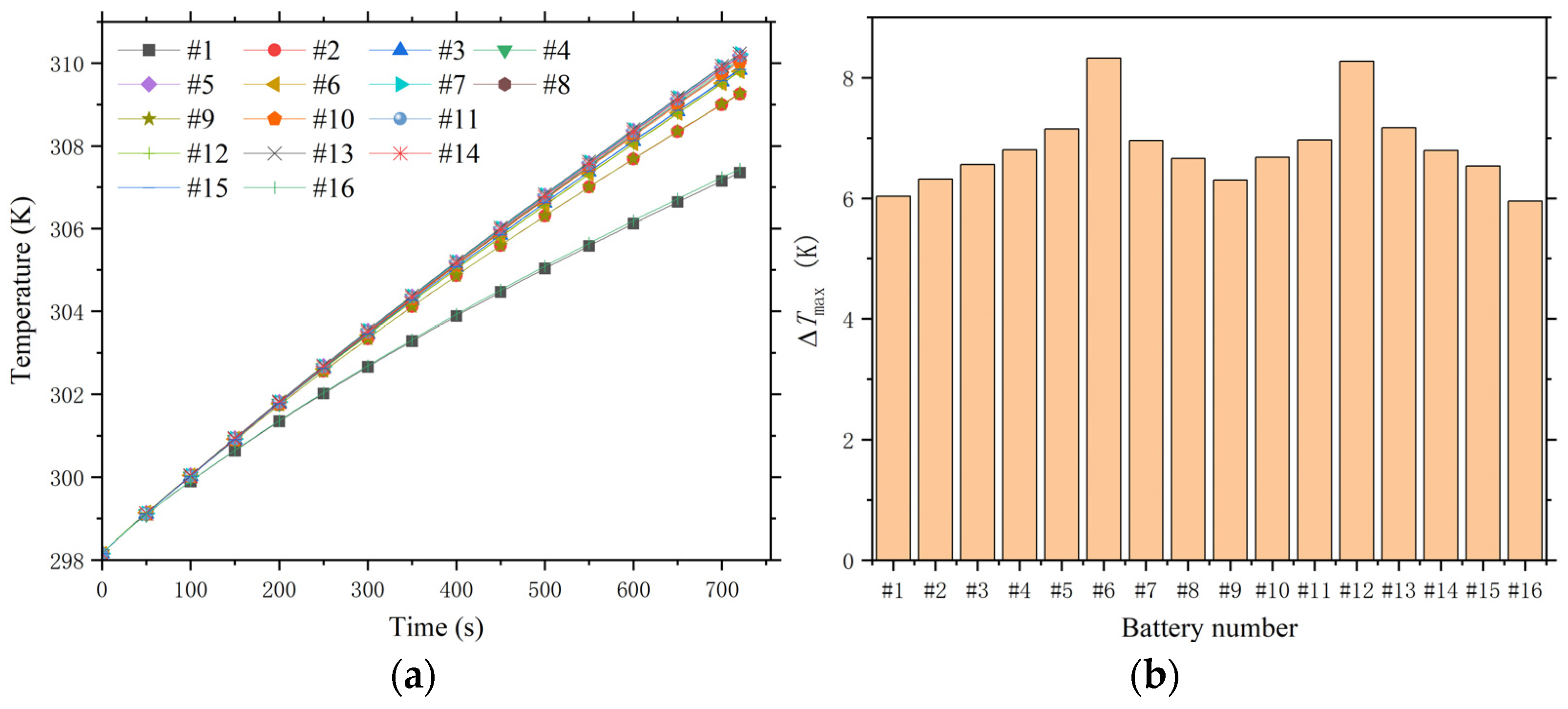
4.4. Temperature Characterization of Battery Module
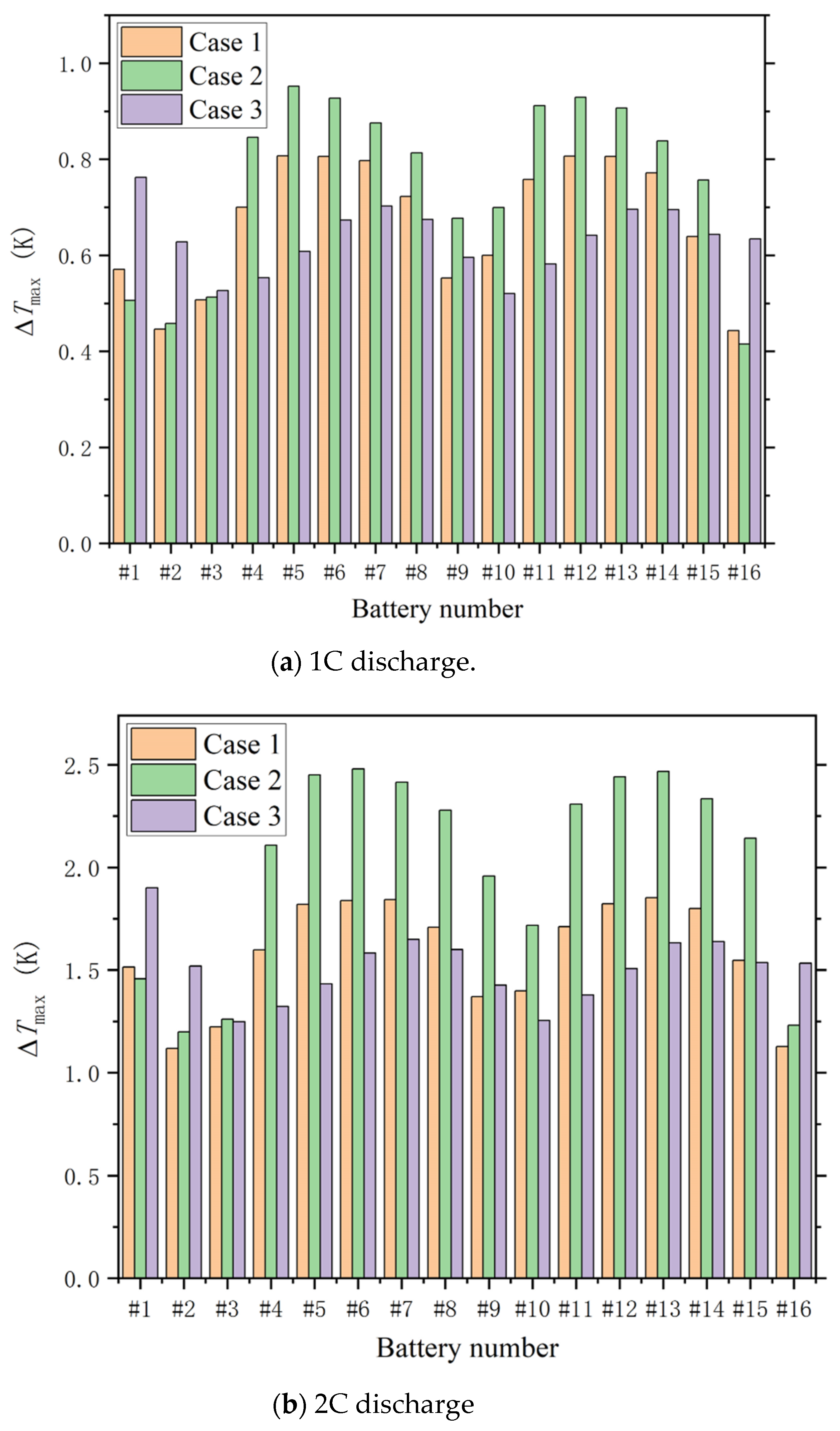
4.5. Influence of Battery Arrangement
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| cp | specific heat capacity, J kg−1 K−1 |
| parameters of the k-ε model | |
| I | current, A |
| k | turbulent kinetic energy, m2 s−2 |
| p | pressure, pa |
| Pk | turbulent kinetic energy generation result of mean velocity, W m−3 |
| q | volumetric heat generation rate, W m−3 |
| R | resistance, Ω |
| T | temperature, K |
| t | time, s |
| ui | velocity component, m s−1 |
| velocity vector, m s−1 | |
| xi | coordinate component, m |
| Greek symbols | |
| gradient operator | |
| turbulent kinetic energy dissipation, m2 s−3 | |
| thermal conductivity, W m−1 K−1 | |
| dynamic viscosity, Pa s | |
| turbulent dynamic viscosity, Pa s | |
| density, kg m−3 | |
| k-ε model parameters | |
| Subscripts | |
| a | air |
| b | battery |
| e | ERB |
| Acronyms | |
| BTMS | battery thermal management system |
| PCM | phase change material |
| ERB | epoxy resin board |
| CFD | computational fluid dynamics |
| FVM | finite volume method |
References
- Houache, M.S.E.; Yim, C.H.; Karkar, Z.; Abu-Lebdeh, Y. On the current and future outlook of battery chemistries for electric vehicles—Mini review. Batteries 2022, 8, 70. [Google Scholar] [CrossRef]
- Martínez-Sánchez, R.; Molina-García, A.; Ramallo-González, A.P. Regeneration of Hybrid and Electric Vehicle Batteries: State-of-the-Art Review, Current Challenges, and Future Perspectives. Batteries 2024, 10, 101. [Google Scholar] [CrossRef]
- Ajanovic, A.; Haas, R. Electric vehicles: Solution or new problem? Environ. Dev. Sustain. 2018, 20, 7–22. [Google Scholar] [CrossRef]
- Väyrynen, A.; Salminen, J. Lithium-ion battery production. J. Chem. Thermodyn. 2012, 46, 80–85. [Google Scholar] [CrossRef]
- Saw, L.H.; Ye, Y.; Tay, A.A.; Chong, W.T.; Kuan, S.H.; Yew, M.C. Computational fluid dynamic and thermal analysis of Lithium-ion battery pack with air cooling. Appl. Energy 2016, 177, 783–792. [Google Scholar] [CrossRef]
- Dan, D.; Yao, C.; Zhang, Y.; Zhang, H.; Zeng, Z.; Xu, X. Dynamic thermal behavior of micro heat pipe array-air cooling battery thermal management system based on thermal network model. Appl. Therm. Eng. 2019, 162, 114183. [Google Scholar] [CrossRef]
- Mahamud, R.; Park, C. Reciprocating air flow for Li-ion battery thermal management to improve temperature uniformity. J. Power Sources 2011, 196, 5685–5696. [Google Scholar] [CrossRef]
- Zhong, G.; Zhang, G.; Yang, X.; Li, X.; Wang, Z.; Yang, C.; Yang, C.; Gao, G. Researches of composite phase change material cooling/resistance wire preheating coupling system of a designed 18650-type battery module. Appl. Therm. Eng. 2017, 127, 176–183. [Google Scholar] [CrossRef]
- Mali, V.; Saxena, R.; Kumar, K.; Kalam, A.; Tripathi, B. Review on battery thermal management systems for energy-efficient electric vehicles. Renew. Sustain. Energy Rev. 2021, 151, 111611. [Google Scholar] [CrossRef]
- Wang, M.; Teng, S.; Xi, H.; Li, Y. Cooling performance optimization of air-cooled battery thermal management system. Appl. Therm. Eng. 2021, 195, 117242. [Google Scholar] [CrossRef]
- Yin, B.; Zuo, S.; Xu, Y.; Chen, S. Performance of liquid cooling battery thermal management system in vibration environment. J. Energy Storage 2022, 53, 105232. [Google Scholar] [CrossRef]
- Peng, P.; Wang, Y.; Jiang, F. Numerical study of PCM thermal behavior of a novel PCM-heat pipe combined system for Li-ion battery thermal management. Appl. Therm. Eng. 2022, 209, 118293. [Google Scholar] [CrossRef]
- Yang, T.; Su, S.; Xin, Q.; Zeng, J.; Zhang, H.; Zeng, X.; Xiao, J. Thermal Management of Lithium-Ion Batteries Based on Honeycomb-Structured Liquid Cooling and Phase Change Materials. Batteries 2023, 9, 287. [Google Scholar] [CrossRef]
- Guo, Z.; Wang, Y.; Zhao, S.; Zhao, T.; Ni, M. Modeling and optimization of liquid-based battery thermal management system considering battery electrochemical characteristics. J. Energy Storage 2023, 70, 108028. [Google Scholar] [CrossRef]
- Xu, C.; Ma, C.; Souri, M.; Moztarzadeh, H.; Nasr Esfahani, M.; Jabbari, M.; Hosseinzadeh, E. Numerical Investigation of Thermal Management of a Large Format Pouch Battery Using Combination of CPCM and Liquid Cooling. Batteries 2024, 10, 113. [Google Scholar] [CrossRef]
- Chen, K.; Song, M.; Wei, W.; Wang, S. Design of the structure of battery pack in parallel air-cooled battery thermal management system for cooling efficiency improvement. Int. J. Heat Mass Transf. 2019, 132, 309–321. [Google Scholar] [CrossRef]
- Ma, R.; Ren, Y.; Wu, Z.; Xie, S.; Chen, K.; Wu, W. Optimization of an air-cooled battery module with novel cooling channels based on silica cooling plates. Appl. Therm. Eng. 2022, 213, 118650. [Google Scholar] [CrossRef]
- Feng, Z.; Zhao, J.; Guo, C.; Panchal, S.; Xu, Y.; Yuan, J.; Fraser, R.; Fowler, M. Optimization of the cooling performance of symmetric battery thermal management systems at high discharge rates. Energy Fuels 2023, 37, 7990–8004. [Google Scholar] [CrossRef]
- Yu, D.; Huang, W.; Wan, X.; Fan, S.; Sun, T. Optimization of simultaneous utilization of air and water flow in a hybrid cooling system for thermal management of a lithium-ion battery pack. Renew. Energy 2024, 225, 120248. [Google Scholar] [CrossRef]
- Chen, K.; Song, M.; Wei, W.; Wang, S. Structure optimization of parallel air-cooled battery thermal management system. Int. J. Heat Mass Transf. 2017, 111, 943–952. [Google Scholar] [CrossRef]
- E, J.; Yue, M.; Chen, J.; Zhu, H.; Deng, Y.; Zhu, Y.; Zhang, F.; Wen, M.; Zhang, B.; Kang, S. Effects of the different air cooling strategies on cooling performance of a lithium-ion battery module with baffle. Appl. Therm. Eng. 2018, 144, 231–241. [Google Scholar] [CrossRef]
- Li, A.; Yuen AC, Y.; Wang, W.; Weng, J.; Yeoh, G.H. Numerical investigation on the thermal management of lithium-ion battery system and cooling effect optimization. Appl. Therm. Eng. 2022, 215, 118966. [Google Scholar] [CrossRef]
- Jin, F.L.; Li, X.; Park, S. Synthesis and application of epoxy resins: A review. J. Ind. Eng. Chem. 2015, 29, 1–11. [Google Scholar] [CrossRef]
- Kim, Y.M.; Kim, S.; Lee, J.Y.; Park, Y.K. Pyrolysis reaction pathways of waste epoxy-printed circuit board. Environ. Eng. Sci. 2013, 30, 706–712. [Google Scholar] [CrossRef]
- Jia, Y.S.; Shi, R.F.; Jian, X. Development of epoxy resin and its application in electrical and electronic materials. J. Funct. Mater. 2020, 51, 40–45. [Google Scholar]
- Levchik, S.; Piotrowski, A.; Weil, E.; Yao, Q. New developments in flame retardancy of epoxy resins. Polym. Degrad. Stab. 2005, 88, 57–62. [Google Scholar] [CrossRef]
- Chen, C.; Niu, H.; Li, Z.; Li, L.; Mo, S.; Huang, X. Thermal runaway propagation mitigation of lithium-ion battery by epoxy resin board. Energy Storage Sci. Technol. 2019, 8, 532. (In Chinese) [Google Scholar]
- Launder, B.E. DB Spalding Mathematical Models of Turbulence; Academic Press: London, UK, 1972. [Google Scholar]
- Panchal, S.; Dincer, I.; Agelin-Chaab, M.; Fraser, R.; Fowler, M. Experimental and simulated temperature variations in a LiFePO4-20 Ah battery during discharge process. Appl. Energy 2016, 180, 504–515. [Google Scholar] [CrossRef]
- Chen, D.; Jiang, J.; Kim, G.H.; Yang, C.; Pesaran, A. Comparison of different cooling methods for lithium ion battery cells. Appl. Therm. Eng. 2016, 94, 846–854. [Google Scholar] [CrossRef]
- Luo, W.; He, F.; Huang, Q.; Li, X.; Zhang, G.; Zhong, Z. Experimental investigation on thermal performance of silica cooling plate-aluminate thermal plate-coupled forced convection-based pouch battery thermal management system. Int. J. Energy Res. 2019, 43, 7604–7613. [Google Scholar] [CrossRef]




| Parameters | Air [10] | Battery * | ERB * |
|---|---|---|---|
| Density (kg m−3) | 1.165 | 2169 | 1200 |
| Specific heat (J kg−1 K−1) | 1005 | 1060 | 1300 |
| Thermal conductivity (W m−1 K−1) | 0.0267 | 1.06 (kx), 16 (ky), 16 (kz) | 0.2 |
| Dynamic viscosity (kg m−1 s−1) | 1.86 × 10−5 | - | - |
| Internal resistance (Ω) | - | 0.001 | - |
| Case | Number of Grids (million) | The Maximum Temperature of Battery Surface (K) |
|---|---|---|
| 1 | 3.74 | 313.12 |
| 2 | 5.3 | 313.24 |
| 3 | 6.17 | 313.26 |
| 4 | 8.49 | 313.26 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, D.; Peng, P.; Wang, Y.; Qiu, Y.; Wu, W.; Jiang, F. Numerical Investigation of the Thermal Performance of Air-Cooling System for a Lithium-Ion Battery Module Combined with Epoxy Resin Boards. Batteries 2024, 10, 318. https://doi.org/10.3390/batteries10090318
Lin D, Peng P, Wang Y, Qiu Y, Wu W, Jiang F. Numerical Investigation of the Thermal Performance of Air-Cooling System for a Lithium-Ion Battery Module Combined with Epoxy Resin Boards. Batteries. 2024; 10(9):318. https://doi.org/10.3390/batteries10090318
Chicago/Turabian StyleLin, Da, Peng Peng, Yiwei Wang, Yishu Qiu, Wanyi Wu, and Fangming Jiang. 2024. "Numerical Investigation of the Thermal Performance of Air-Cooling System for a Lithium-Ion Battery Module Combined with Epoxy Resin Boards" Batteries 10, no. 9: 318. https://doi.org/10.3390/batteries10090318
APA StyleLin, D., Peng, P., Wang, Y., Qiu, Y., Wu, W., & Jiang, F. (2024). Numerical Investigation of the Thermal Performance of Air-Cooling System for a Lithium-Ion Battery Module Combined with Epoxy Resin Boards. Batteries, 10(9), 318. https://doi.org/10.3390/batteries10090318







