Next Article in Journal
New Dmit-Based Organic Magnetic Conductors (PO-CONH-C2H4N(CH3)3)[M(dmit)2]2 (M = Ni, Pd) Including an Organic Cation Derived from a 2,2,5,5-Tetramethyl-3-pyrrolin-1-oxyl (PO) Radical
Previous Article in Journal
Antiferromagnetic Insulating Ground State of Molecular π-d System Λ-(BETS)2FeCl4 (BETS = Bis(ethylenedithio)tetraselenafulvalene): A Theoretical and Experimental Review
Previous Article in Special Issue
Recent Advances in Organic Radicals and Their Magnetism
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Magnetochemistry
Volume 3
Issue 1
10.3390/magnetochemistry3010012
magnetochemistry-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Perspective

Molecular Spins in the Context of Quantum Technologies

by
Alberto Ghirri
1,
Andrea Candini
1 and
Marco Affronte
1,2,*
1
CNR Institute of Nanosciences, S3, via G. Campi 213A, 41125 Modena, Italy
2
Dipartimento di Scienze Fisiche, Informatiche e Matematiche, Università di Modena e Reggio Emilia, via G. Campi 213A, 41125 Modena, Italy
*
Author to whom correspondence should be addressed.
Magnetochemistry 2017, 3(1), 12; https://doi.org/10.3390/magnetochemistry3010012
Submission received: 13 January 2017 / Accepted: 13 February 2017 / Published: 25 February 2017
(This article belongs to the Special Issue Molecules in Quantum Information)
Browse Figures
Versions Notes

Abstract

:
Molecular spins have shown interesting quantum features, which make them potential candidates for the implementation of quantum information processing. New challenges related to possible applications in the broader class of quantum technologies are currently under discussion. Here, we revisit some key features trying to learn something from experiences in related fields.

1. Introduction

Quantum computing (QC) is one of the quantum technologies (QT) [1] that aims at exploiting genuine quantum features of systems and devices. QC was envisaged in the early 1980s by Richard Feynman and other pioneers and, since then, other possible applications of quantum systems became feasible thanks to technological progress. Among QTs, quantum communications, sensing, cryptography, and metrology are now attracting much interest. In addition to the discreteness of energy levels, the possibility to create and maintain superposition of states and quantum correlation (entanglement) are considered two of the main features of quantum systems that do not exist in the classical world. Quantum systems, such as photons, cold atoms, spin impurities in solids, and semiconducting and superconducting devices have been intensively studied in the last decades, several applications based on these systems are in rapid development, and some of these have already appeared in the market.
Different spin centers in the solid state have been—and currently are—intensively studied for potential applications: spin impurities in Si have been studied and proposed for qubit encoding [2]; Nitrogen vacancy (NV) centers, as well as other color centers in diamond or SiC, are now attracting much interest for their potentialities as atomic scale sensors, besides the possibility to use them as hardware for QC [3]. In spite of different preparation technologies, experiments (e.g., manipulation and read out of spin) and modeling (e.g., spin dynamics, sources of decoherence, spin entanglement, etc.) all of these spin systems obviously share many analogies, thus we can probably learn something by comparing the properties of molecular spins to those of other spin systems. This is intended to be the main stream of this article. We shall focus on three main trends in the field: coherent manipulation of spin ensembles; molecular quantum spintronics; and the perspective to embed (molecular) spins in quantum circuits.

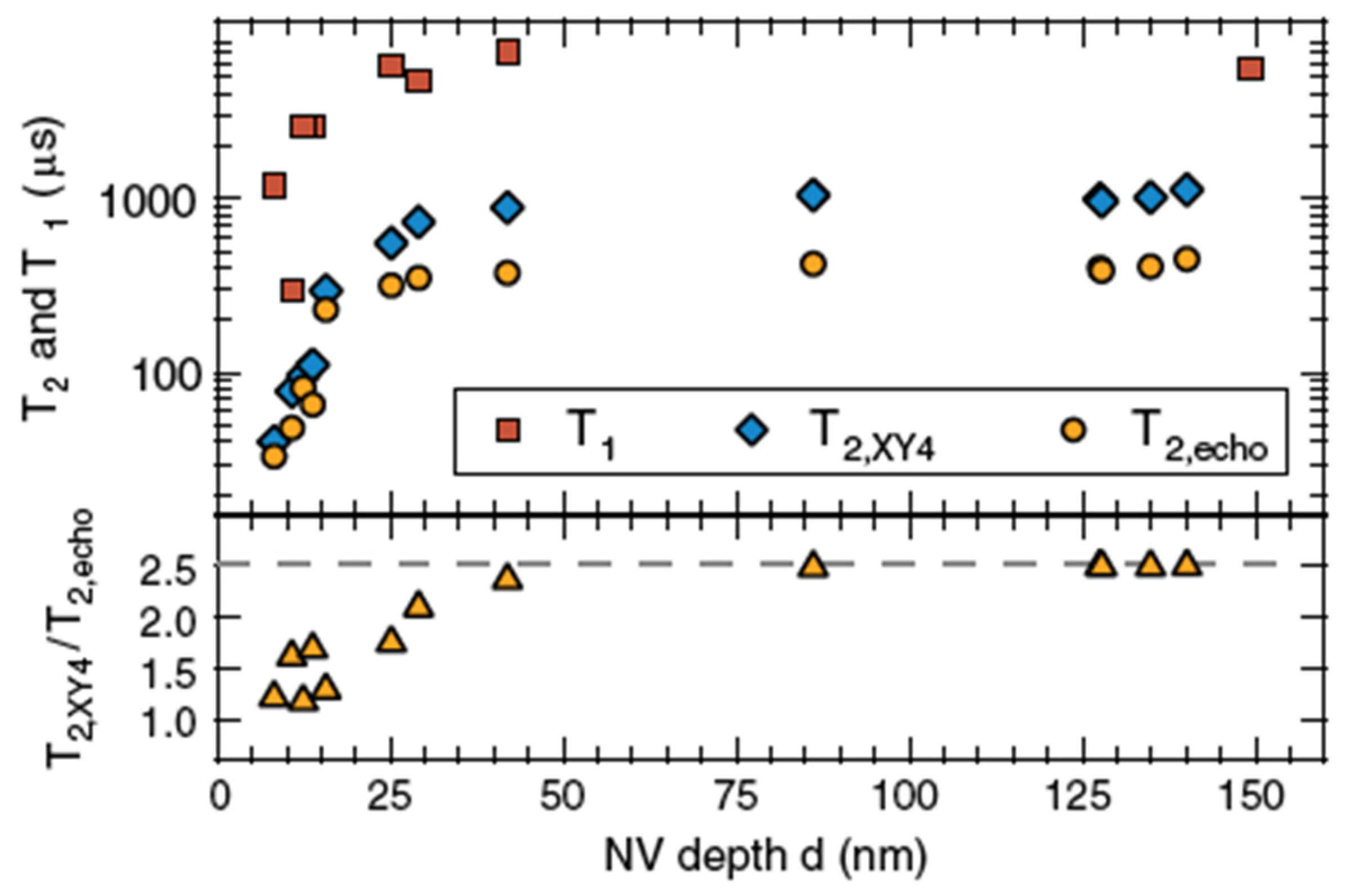
2. Coherent Spin Dynamics of Spin Ensembles

Quantum effects in molecular spin systems have been explored in the last two decades. The first milestone was the discovery of quantum tunneling of the magnetization in the 1990s that demonstrated that molecular spins are real systems on which quantum effects can be controlled and studied in laboratory conditions [4]. The next challenge was to control the dynamics of molecular spins. Molecules with low spin ground states (ideally S = 1/2), well isolated by excited states, are prototypical cases for this type of study [5], and long-lived coherent oscillations between two spin states is a target experiment that can be performed by pulsed electron spin resonance (ESR) techniques. The understanding and control of decoherence mechanisms are of fundamental importance for the dynamics of spins in the solid state environment. Thus, in the last decade, there has been an intense activity in searching molecular spin systems with improved performances, more specifically with the longer relaxation (T1) and dephasing (T2) times, as discussed in previous reviews [6,7] and themed issues of specialized journals and book series [8,9,10]. Inter-molecular interactions are, in general, detrimental, thus working with spin ensembles diluted in non-magnetic matrix is mandatory. In this way, coherent spin oscillations were soon observed in low-spin Cr7Ni rings [11] and V15 [12] at liquid He temperature.
Most of the solid state hardware for quantum technology works at cryogenic temperatures, yet this limits all of them to niche applications and increasing the working temperature—and reducing the use of magnetic field—is certainly appealing in view of widely spread applications. Working at 2 K instead of 20 mK is a gain of two orders of magnitude in temperature and this allows us to avoid the use of dilution refrigerators. Working at 20 K will allow a gain of another order of magnitude and, for instance, the use of closed cycle refrigerators that can be developed even on one chip. At 80 K we may use liquid nitrogen, and room temperature operation will open to much broader class of applications. A first breakthrough along this line was obtained with the report of persistence of long T2 up to 100 K in commercial CuPc derivative [13]. This result was followed by rational design of mononuclear derivatives for which T2 was further improved [14,15,16]. The relevant figure of merit here is the ratio between the manipulation time and the spin coherence time. Since, for typical pulsed ESR setups, the time to manipulate an electron spin is about 10 ns, both T1 and T2 need to be much longer in order to observe coherent spin oscillations and, possibly to perform some error corrections. As a matter of facts, Rabi oscillations at room temperature have been recently reported in VOPc [17]. These results represent an important milestone for molecular magnetism.
In [13] one can find a direct comparison of relevant coherence time measured at different temperatures in different spin systems. Yet care should be taken in making direct comparisons since data should be taken in similar conditions and experiments. One relevant point is the dependence of decoherence times of spin defects on the depth of impurity in the solid. For instance, it is well documented that T1 and T2 decrease for shallow impurities in both Si and diamond and they dramatically drop when spin is closer than 5 nm to the surface. For instance, Figure 1 (from [18]) shows that both T1 and T2 drop below 100 μs for NV positioned less than 10 nm from the diamond surface. Interestingly, the coordination of the spin center is not altered but the shallow defects were demonstrated to be sensitive to both the magnetic and the electric [19] noise on the diamond surface. This should draw our attention to control the environment of our spin: if impurities are diluted in a nuclear free environment, magnetic noise can be drastically reduced. The same attention should be taken to reduce (electrical) noise produced by local vibrations. From this point view, the seminal work reported in [20] demonstrated that molecular engineering can be a powerful tool to control the closest environment of the spin. On the other hand, if we need to expose spins to an external environment, such as a biological system or an electronic circuit, the ligand shell may, in some ways, protect or, at least, define the closest surrounding around the sensitive spin.
In view of possible applications for which spins need to be embedded in external environment, the next challenge seems to be the consolidation of performances with an overall (chemical, structural) robustness of the molecules in different working conditions. To this end, tests on isolated molecules on surfaces or under different stimuli or thermal cycling need to be performed. Important achievements have done by studying isolated molecules on surfaces by different techniques, such as STM or X-ray spectroscopies. Not all, but few molecules, were robust enough to substantially maintain their (static) magnetic features when dispersed. However subtle effects may occur when the environment change and these need to be carefully checked case by case [21]. We expect that different molecules can be designed for specific ambient/applications, thus, for instance, molecules with external organic ligand and specific linkers will be more suitable for biological applications; molecules that can be sublimed and with linkers designed to graphitic or CMOS compatible sustrate will better work embedded in electronic circuits; while molecules with robust periphery (e.g., oxygen ligand) can be possible applied in ambient (air) conditions.
Quantum computation may require the implementation of quantum gates [22]. A universal scheme for computation that, in principle, solves a broad class of computational problems, can be reduced to basic gates using one or two qubits. For spins, a one-qubit gate corresponds to rotation along two orthogonal directions and, therefore, relevant tests are observation of Rabi oscillations in pulsed ESR experiments, as previously discussed. Several experiments on mononuclear molecular spin centers have been performed as also reviewed in one article of this issue [23].
Next challenge is to perform conditioned quantum operations involving more than one spin center. In general terms, we need to demonstrate that the dynamics of one (target) spin is conditioned by the state of the second (control) spin [22]. This may require the two spins to be distinguishable either spatially or spectroscopically and a typical sequence for two-qubit gate encoding encompasses the initialization of the system, the rotation of the target spin under different conditions of the control spin qubit and, finally, the read-out of the system state. According to the specific pulse sequence and rotation, a number of two-qubit gates can be performed, similarly classical two-bit gates, such as controlled-NOT. These conditional spin dynamics are possible by exploiting quantum correlation (entanglement) of two or more spin centers and, since this is a genuine quantum property, quantum gates are essentially different from classical gates.
Spin correlation (entanglement) is intrinsic property of system states that needs to be properly quantified by using suitable experimental quantities or mathematical functions [24]. Spin entanglement can be obtained within a single molecule or at supramolecular level (i.e., between molecules) by controlling spin topology and magnetic interactions. Along this line, dimers of molecular spins with weak permanent magnetic coupling could be designed and synthesized [25] and sizable spin entanglement was demonstrated [26]. One issue of this approach is related to the fact that, during the gate operation, the coupling between the two qubits needs to be switched on and off in order to allow independent rotation of the two spin qubits. In other words, switchable links would be required. This problem can be bypassed by engineering the molecular states in such a way to make use of auxiliary states [27], as also described in the realistic case of antiferromagnetic rings [28]. An alternative way to entangle two spins is to simply position two spin centers at a fixed distance (few nm) and exploit dipolar (i.e., through space) interaction, as has been done for spin defects in diamond [29] and Si. The drawback of using dipolar interaction is, however, its persistent character and its strong dependence on both distance and orientation of the two spin centers. These make such an approach simply not scalable for defects. Perhaps this problem is less critical for molecular assemblies. A further alternative way to entangle two spin centers far apart is, however, the use of flying qubits (photons) as demonstrated in a recent experiment in which entanglement between two NV centers 25 nm distant from one another was achieved by engineering the pulse sequence and using a dynamic decoupling technique [30].
Proposals for the implementation of two-qubit gates with specific molecular spins systems have appeared and preliminary experiments have been reported using binuclear lanthanides [31], radicals [32], and antiferromagnetic rings [33]. Moreover, an intense search of a suitable bi-nuclear molecular system is currently under way and many potential candidates are ready to be tested. In terms of experiments, this would require the use of multi-frequency spectrometers that allow distinguishing the two qubits or to activate switchable interaction. Commercial instrumentation, such as that for pulsed electron-electron double resonance (PELDOR), is well developed but, in most of the cases, it needs be adapted to working conditions (frequency, temperature, magnetic field) of molecular qubits. At present, dedicated instrumentation is accessible only to few laboratories worldwide whist the implementation of two-qubit gates with molecular spin ensembles would require more flexibility/tunability on both molecular qubits and instrumentation. Moreover, in spite of the progress achieved in using pulsed ESR techniques to molecular electron spins [34], much work still remains to be done to encompass heterogeneities of both molecular features and applied field and, more in general, to efficiently decouple spin to the environment. For instance, very interesting results have been obtained in the optimization of pulse sequences for dynamical decoupling [35]. In view of using multi-frequency pulse spectrometer, it is worth reminding that magnetic molecules generally possess both electron and nuclear spins and probably the best approach will be to combine the long coherence time of nuclear spins with the faster manipulation and read out of electronic spins. For this electron nuclear double resonance (ENDOR) technique offers several interesting solutions as discussed in [36].
Final general remarks should be offered concerning the use of spin ensembles. The main criticism, well known in the QC community for NMR, is that working with ensembles makes the systems not—easily—scalable. In other words, the resources, (e.g., spins) required for solving problems and for the relative error correction, scale fast with increasing numbers of data, thus making this type of architectures not suitable for a universal use. Within a more pragmatic perspective, we may focus our interest on quantum simulators [37], i.e., small quantum computers that solve specific problems which would require huge amount of classical computing resources, or even intractable by classical computers. In practice this requires the use of molecular derivatives comprising small, but well defined, spin clusters whose dynamics may solve a complex problem or emulate the behavior of system of interest in other fields (for instance, a chemical reaction or a problem in solid state physics, see also review article in this issue [38]). This topic is still largely unexplored and deserves joint effort from chemists and physicists.

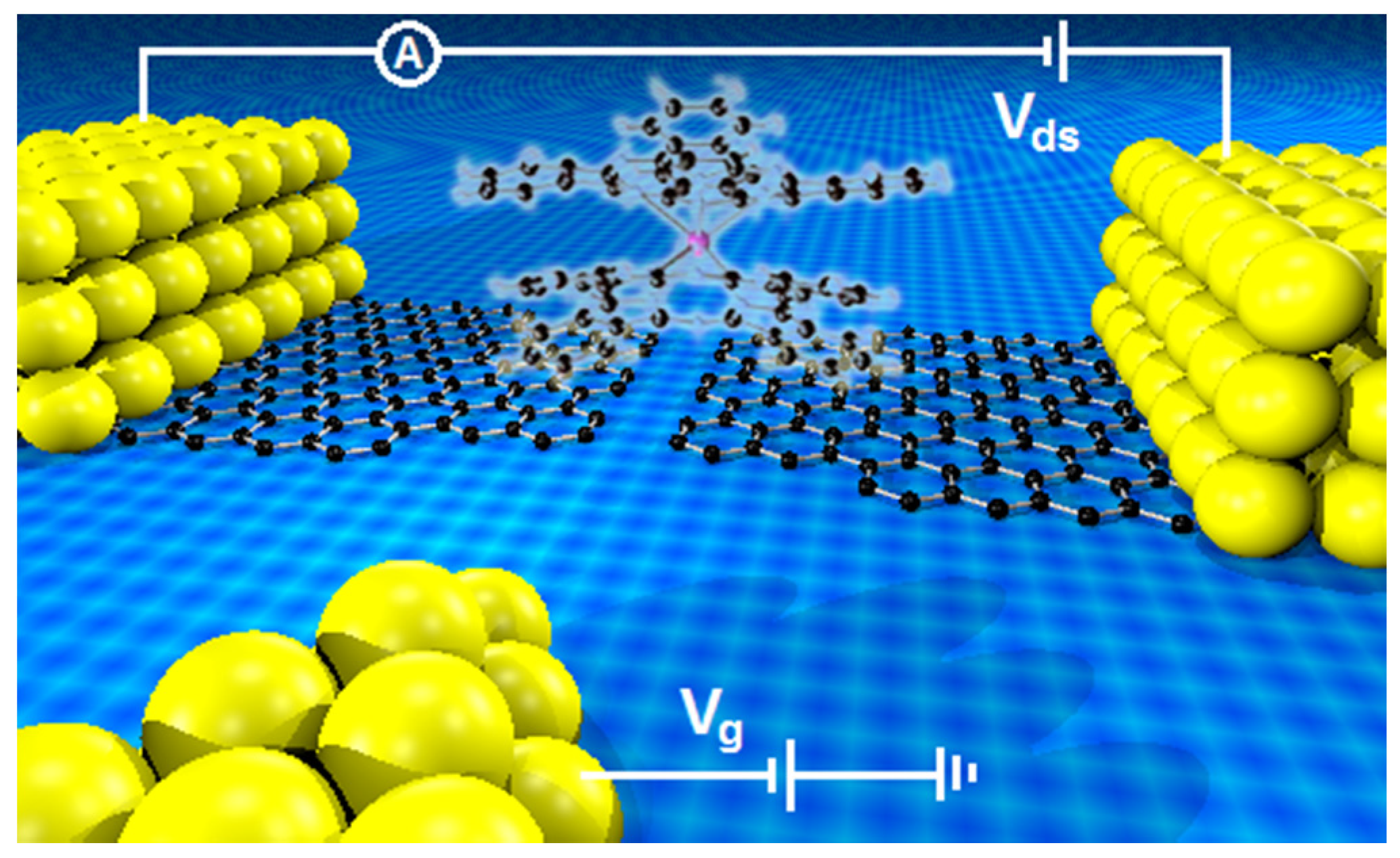
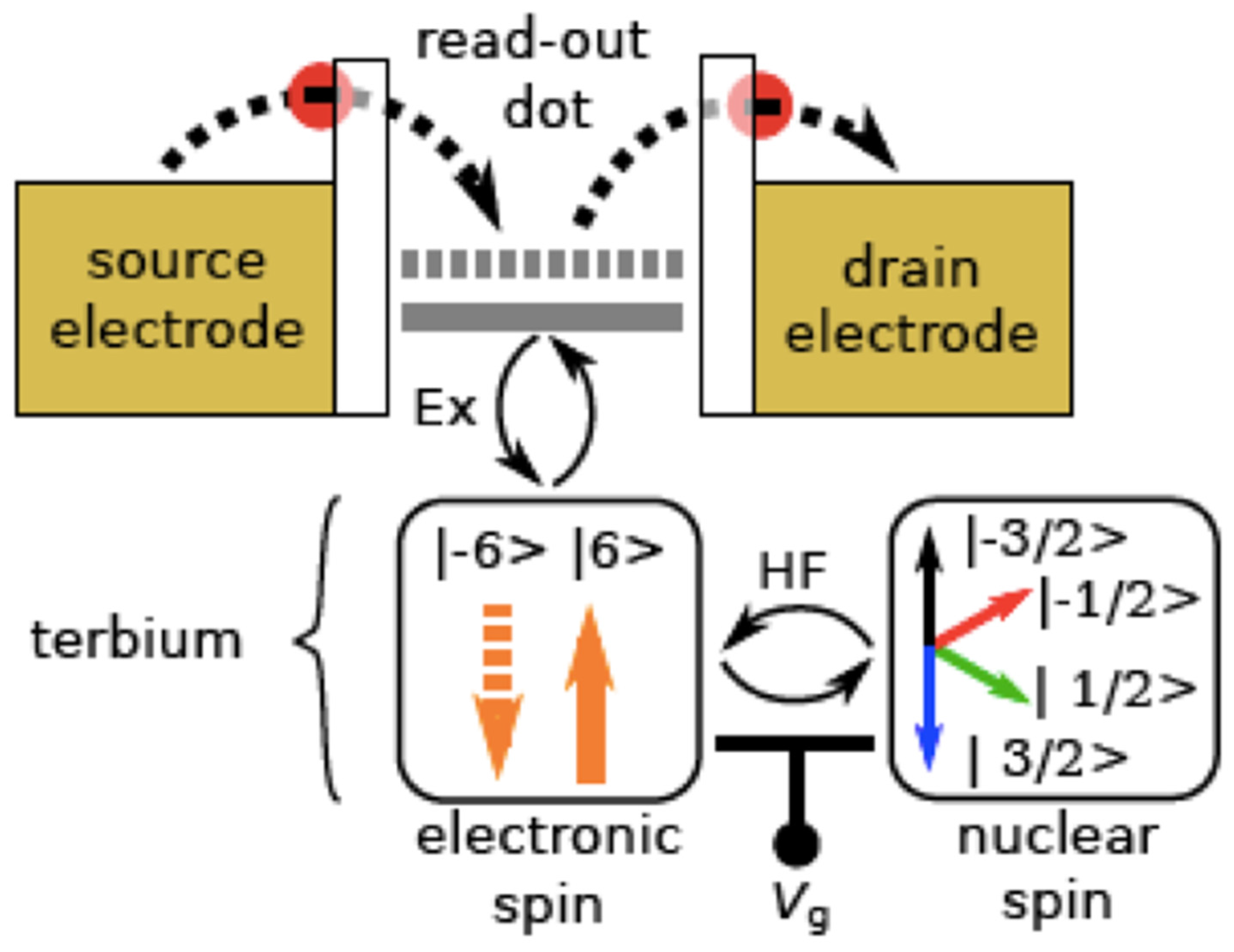
3. Molecular Quantum Spintronics

The addressing, manipulation, and read out of single molecular objects constitute the next great conceptual and technological challenge. If we can avoid some of the drawbacks found in using spin ensembles, here we have to find efficient ways to detect tiny magnetic signals and to individually manipulate spins by protecting fragile quantum states from the environment at the same time.
Different approaches have been tested to detect tiny magnetic signals: one milestone was the development of nano-SQUID made with carbon nanotube [39]. Despite the magnetic flux sensitivity of these devices being able to achieve the quantum limit, the main limitation of these types of magnetometers is constituted by the magnetic coupling—through space—of the molecule with the sensor. Direct single molecule detection by charge current seems more appealing, at least for spintronic devices, and it may benefit from the progresses achieved in close fields such as molecular electronics, scanning microscopy, and single-electron semiconducting devices.
The use of scanning probe microscopes, more specifically tunneling tips, holds much promise for their potentialities to localize, move and read out single magnetic atoms and molecules [40]. With respect to bare magnetic atoms, the presence of an organic ligand seems to delocalize the magnetic features of the molecule [41] and data interpretation is still under debate. Recently interesting experiments on the manipulation and read out of molecular spin (TbPc2) by radio frequency [42] and single magnetic atom on a surface by pulsed microwave sequences have been reported [43].
At the same time, the development of tunnel junctions that may host a single molecule (Figure 2) have been independently reported by different groups. Magnetic features of individual molecules have been observed and reported for Fe4 [44,45], TbPc2 [46], and organic radicals [47]. An open question here is how the charge current from the leads perturbs the magnetic state of the molecule. Changes of valence (and spin) state of the magnetic core are indeed expected. Since each type of molecule behaves in different way, this issue needs to be evaluated case by case. An alternative read out scheme comprises a quantum dot whose conductivity is affected by the spin state of the magnetic center that is in close proximity and coupled with the device. This scheme is analogous to the spin dependent tunneling that is used to read out spin qubits also in semiconductors, but here the quantum dot can be made by carbon nanotube [48], graphene nano-constrictions [49], or even the organic radical present in the ligand of the molecule, as demonstrated in the case of a TbPc2 molecular spin transistor [50]. In this scheme, the charge channel and the spin center are two separate bodies, coupled by exchange interaction (see Figure 3).
The experiments reported by the Grenoble team went a step ahead demonstrating the possibility to read out and manipulate the nuclear spin state. The nuclear spin of Tb (I = 3/2) is indeed coupled to the electron magnetic moment (ground state J = 6, mJ = ±6) by the hyperfine interaction (Figure 3) giving rise to hybrid electron-nuclear states whose level anti-crossing (LAC) are quite visible at low magnetic fields. The nuclear states can be identified by sweeping the magnetic field and comparing the position of the LACs with those measured on bulk samples. Note that, since measurements are performed on a single qubit, the process must be repeated several times in order to obtain significant statistics.
By exploiting the long coherence time of nuclear spin (coherence time exceeding 60 μs) and the hyperfine electric Stark effect, the same team has then demonstrated that it is possible to manipulate the nuclear spin of a single molecule. More specifically, nuclear spin trajectories [52] and Ramsey fringes have been observed [53]. These results compare well with similar experiments reported for spin impurity (P donor) in Si [54] and witness a tangible contribution of molecular magnetism to QT. At the same time, we expect that the next steps along this research line will move in parallel with similar devices based on spin impurities in semiconductors [55] or NV centers in diamond [56], that is, the implementation of two-qubit gates or multi-qubit algorithms.
Working devices need to be reliable and protocols for quantum error correction need to be developed. The problem of error correction is well known in computer science and some schemes are discussed in textbooks [22]. Briefly, it may occur that during a quantum operation a qubit accidently flips, invalidating the whole process. To mitigate and correct these errors we may encode the qubit in more than one (typically three) processor and then use the majority rule: if the probability of an accidental flip is relatively low, two qubits over three remain in the correct state while one contains an error. In this case the majority (two over three) determine the correct state while the third one can be corrected. Further methods to correct different type of quantum errors have been reported in the literature for other spin systems, in particular those tested for NV centers [57].
The previous discussion leads us to another technological issue: soon or later we would need to have arrays of similar devices working at the same time in order to guarantee the scalability of our computing machine. Although this aspect is less discussed in literature, the rate of success R in the device fabrication process is relatively low (often <10%). These numbers are typical for tunnel junctions in molecular electronics, although they related to the specific fabrication process and certainly need—and can—be improved in future by exploiting novel bottom-up fabrication methods. It should be noted, however, that the fabrication of CMOS-compatible quantum devices below 10 nm by top-down (lithographic) techniques are also affected by low yields. In view of applications, it is worth fixing some benchmarks that may assess the reliability of the fabrication process. As an example, let us suppose we intend to test a quantum error correction protocol. As mentioned above, we’d need at least three identical devices and in this case the probability to get all of these working at the same time will drop at R3. Thus, we would need to fabricate 103 devices to get at least one working machine to test quantum error correction code!
Concerning multi-qubit devices, a key experiment would be the implementation of Grover’s algorithm within the ground state multiplet [58]. The Grover’s algorithm efficiently solves a specific problem by performing a search of an item within a set of data. The sub-levels of the ground multiplet inequivalently spaced in energy constitute an excellent playground to test this scheme since each of them can be addressed separately by microwave pulses. Note that, in this scheme, spin entanglement is not required, so the scheme can be implemented within a single molecule. As a proof of concept, few sub-levels can be considered, but it is worth mentioning that the implementation is hardly scalable by using a single molecule. It is nevertheless worth to highlight here that, so far, the Grover’s algorithm has been implemented with trapped ions, photons, and superconducting qubits but, for the best of our knowledge, not with other solid state platforms, such as impurities in Si.

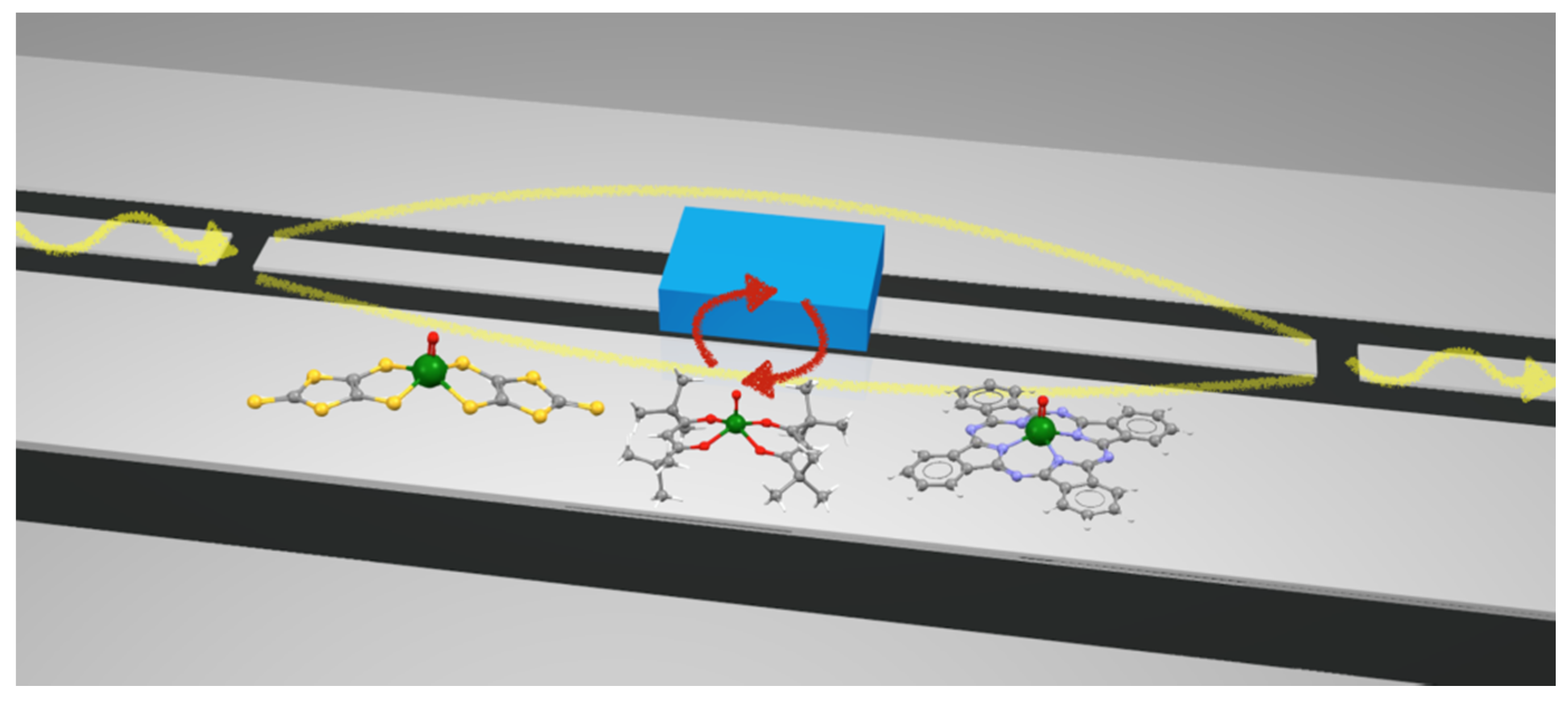
4. Molecular Spins in Hybrid Quantum Architectures

The ability to manipulate and read out an arbitrary spin state in a molecule is certainly a pre-requisite to be a good candidate for quantum information processing. Yet, other features are required in view of the wider exploitation of molecular spins for quantum technologies. One of these is the possibility to exchange quantum information between solid state registers (spins) and flying qubits (photons). Molecules offer a broad spectrum of frequencies for an efficient coupling with photons: nuclear spins are active at radio frequencies (MHz) while the pattern of magnetic energy levels of electron spins fully covers the microwave (MW) range (GHz). The main challenge is to coherently couple the spin with photons, that is, the match in energy should occur along with the transfer of phase information. This implies that the spin manipulation should be fast enough to overcome the decoherence mechanisms of both the spin and photon systems. Key experiments in this context make use of microwave resonant cavities with high quality factor in which long-lived photons trapped in the cavity couple with spins (Figure 4). First tests are typically performed in the continuous wave operation mode, although the final goal is to obtain hybrid devices in which quantum information is exchanged through MW pulse sequences. Coherent spin-photon states are obtained in the so-called high coperativity regime for which the spin-photon coupling is stronger than each decoherence rate of spins and photons [7]. Again, only molecular spins with the longest coherence time are suitable to pass this test. Spin can couple with the magnetic component of radiation B1 through their dipole moment gμBS, yet this interaction is in general very weak and we need to develop strategies to enhance it. Molecular engineering may allow the enhancement of both the Landé g-factor and the total magnetic moment of the ground multiplet beyond ordinary values obtained for single atoms. Another strategy to strengthen the spin-photon coupling is to use spin ensembles. It has been demonstrated in fact that the spin-photon coupling can be enhanced by a factor of √N—with N being the total number of spins—by using collective modes [7]. Although the general problem of coupling photons with a two-level system (e.g., atoms, molecules with electric dipoles, etc.) is well documented in the literature; only recently experimental investigations focused on spin systems have been reported. The high coperativity regime was achieved by using coplanar superconducting resonators and NV centers [59] or Er spin impurities in inorganic matrix [60] at mK temperature. We are currently using high Tc superconducting planar resonators [61] that show excellent performances at finite temperature (up to 50 K, at least) and in strong magnetic fields and, with these, we can achieve a strong coupling regime with 2,2-diphenyl-1-picrylhydrazyl (DPPH) [59] and (3,5-Dichloro-4-pyridyl)bis(2,4,6-trichlorophenyl)methyl (PyBTM) [62] organic radicals at the temperature of liquid helium, and even above. It is worth noting that, in these latter cases, the strong coupling regime was obtained by using concentrate samples that present sharp resonance lines due to exchange narrowing. As concerns mononuclear molecular metal-spin centers, preliminary results on diluted crystals show that we get close—but not yet fully in—to the high cooperativity regime [63]. For comparison, a snapshot summary of these preliminary results is plotted in Figure 5. It should be emphasized, however, that data reported in Figure 5 depend only in part on the intrinsic features of the spin centers. Other parameters, such as temperature, number of spins, and cavity characteristics, influence both the spin-photon coupling and the spin resonance linewidth. Thus, for instance, in Figure 4 different values are reported for same derivative at different temperatures or spin concentrations. Results on Cu(nmt)2 crystals are quite encouraging: if we extrapolate trend at low temperature (open symbols) we can safely expect that the strong coupling regime can be achieved below 1 K.
An alternative approach is to locally enhance the intensity of MW zero-point current fluctuations. It has been proposed indeed that nano-structured superconducting strip lines may allow the achievement of the strong coupling regime even with a single (molecular) spin, and this can be used for scalable architectures [64]. Experiments are currently testing different solutions since the detection of tiny signals requires extraordinary sensitivity. By using Josephson junctions amplification, the detection of a small ensemble of about 103 spins has been reported and this is, at present, the best performance of EPR nano-detection [65]. The use of electric field component to manipulate spins is a further attractive alternative and several mechanisms have been proposed [66] and they are currently under investigation [67].
Achieving strong coupling regime with microwave photons allows the integration of molecular spins in hybrid quantum devices. Superconducting circuits are normally used as a bus to transfer quantum information between different quantum memories and registers. In a recent experiment, we have demonstrated that we can couple two or more distinguished spin ensembles through resonant microwaves photons by spectroscopic measurements [62]. Next steps along this line could be the integration of molecular spins in more complex superconducting circuits with the encoding of sequences of MW pulses. This will eventually allow the performance of quantum algorithms [68] or simply to transfer qubits from fast quantum registers to memories. Along this line, pulse MW sequences have been used to efficiently transfer qubits in hybrid superconducting circuits with NV centers [69]. Again, we may learn a significant amount from fields close to molecular magnetism!

Acknowledgments

This work has been partially supported by the Italian Ministry of University and Research through the PRIN project QCNaMos N. 2015HYFSRT, by the European Community through the FET-Proactive Project MoQuaS by contract no. 610449 and by the US AFOSR/AOARD program, contract 134031 FA2386-13-1-4029.

Author Contributions

All authors contributed to write this article.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Quantum technology. Available online: https://en.wikipedia.org/wiki/Quantum_technology (accessed on 24 February 2017).
  2. Dutt, M.V.; Childress, L.; Jiang, L.; Togan, E.; Maze, J.; Jelezko, F.; Zibrov, A.S.; Hemmer, P.R.; Lukin, M.D. Quantum Register Based on Individual Electronic and Nuclear Spin Qubits in Diamond. Science 2007, 316, 1312–1316. [Google Scholar] [CrossRef] [PubMed]
  3. Morton, J.J.; McCamey, D.R.; Eriksson, M.A.; Lyon, S.A. Embracing the quantum limit in silicon computing. Nature 2011, 479, 345–353. [Google Scholar] [CrossRef] [PubMed]
  4. Gatteschi, D.; Sessoli, R.; Villain, J. Molecular Nanomagnets; Oxford University Press: Oxford, UK, 2006. [Google Scholar]
  5. Troiani, F.; Ghirri, A.; Affronte, M.; Carretta, S.; Santini, P.; Amoretti, G.; Piligkos, S.; Timco, G.; Winpenny, R.E.P. Molecular Engineering of antiferromagnetic rings for quantum computation. Phys. Rev. Lett. 2005, 94, 207208. [Google Scholar] [CrossRef] [PubMed]
  6. Troiani, F.; Affronte, M. Molecular Spins for Quantum Information Technologies. Chem. Soc. Rev. 2011, 40, 3119–3129. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  7. Ghirri, A.; Troiani, F.; Affronte, M. Quantum Computation with Molecular Nanomagnets: achivements, challenges and new trends. In Molecular Nanomagnets and Related Phenomena—Structure and Bonding; Gao, S., Ed.; Springer: Berlin/Heidelberg, Germany, 2014; Volume 164, pp. 383–430. [Google Scholar]
  8. J. Mater. Chem. 2009, whole issue 19. Available online: http://pubs.rsc.org/en/journals/journalissues/jm?e=1#!issueid=jm019019&type=archive&issnprint=0959-9428 (accessed on 24 February 2017).
  9. Bartolomé, j.; Luis, F.; Fernandez, J.F. Molecular magnets Physics and Applications; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
  10. Coronado, E.; Yamashita, M. Molecular Spintronics: The role of Coordination Chemistry. Dalton Trans. 2016, 45, 16553–16555. [Google Scholar] [CrossRef] [PubMed]
  11. Ardavan, A.; Rival, O.; Morton, J.J.L.; Blundell, S.J.; Tyryshkin, A.M.; Timco, G.A.; Winpenny, R.E.P. Will Spin-Relaxation Times in Molecular Magnets Permit Quantum Information Processing? Phys. Rev. Lett. 2007, 98, 057201. [Google Scholar] [CrossRef] [PubMed]
  12. Bertaina, S.; Gambarelli, S.; Mitra, T.; Tsukerblat, B.; Müller, A.; Barbara, B. Quantum oscillations in a molecular magnet. Nature 2008, 453, 203–206. [Google Scholar] [CrossRef] [PubMed]
  13. Warner, M.; Din, S.; Tupitsyn, I.S.; Morley, G.W.; Marshall Stoneham, A.; Gardener, J.A.; Wu, Z.; Fisher, A.J.; Heutz, S.; Kay, C.W.M.; et al. Potential for spin-based information processing in a thin-film molecular semiconductor. Nature 2013, 503, 504–508. [Google Scholar] [CrossRef] [PubMed]
  14. Bader, K.; Dengler, D.; Lenz, S.; Endeward, B.; Jiang, S.-D.; Neugebauer, P.; van Slageren, J. Room temperature quantum coherence in a potential molecular qubit. Nat. Commun. 2014, 5, 5304. [Google Scholar] [CrossRef] [PubMed]
  15. Graham, M.J.; Zadrozny, J.M.; Shiddiq, M.; Anderson, J.S.; Fataftah, M.S.; Hill, S.; Freedman, D.E. Influence of Electronic Spin and Spin-Orbit Coupling on Decoherence in Mononuclear Transition Metal Complexes. J. Am. Chem. Soc. 2014, 136, 7623–7626. [Google Scholar] [CrossRef] [PubMed]
  16. Zadrozny, J.M.; Niklas, J.; Poluektov, O.G.; Freedman, D.E. Multiple Quantum Coherences from Hyperfine Transitions in a Vanadium(IV) Complex. J. Am. Chem. Soc. 2014, 136, 15841–15844. [Google Scholar] [CrossRef] [PubMed]
  17. Atzori, M.; Tesi, L.; Morra, E.; Chiesa, M.; Sorace, L.; Sessoli, R. Room Temperature Quantum Coherence and Rabi Oscillations in Vanadyl Phthalocyanine: Toward Multifunctional Molecular Spin Qubits. J. Am. Chem. Soc. 2016, 138, 2154–2157. [Google Scholar] [CrossRef] [PubMed]
  18. Myers, B.A.; Das, A.; Dartiailh, M.C.; Ohno, K.; Awschalom, D.D.; Bleszynski Jayich, A.C. Probing Surface Noise with Depth-Calibrated Spins in Diamond. Phys. Rev. Lett. 2014, 113, 027602. [Google Scholar] [CrossRef] [PubMed]
  19. Kim, M.; Mamin, H.J.; Sherwood, M.H.; Ohno, K.; Awschalom, D.D.; Rugar, D. Decoherence of Near-Surface Nitrogen-Vacancy Centers Due to Electric Field Noise. Phys. Rev. Lett. 2015, 115, 087602. [Google Scholar] [CrossRef] [PubMed]
  20. Wedge, C.J.; Timco, G.A.; Spielberg, E.T.; George, R.E.; Tuna, F.; Rigby, S.; McInnes, E.J.L.; Winpenny, R.E.P.; Blundell, S.J.; Ardavan, A. Chemical Engineering of Molecular Qubits. Phys. Rev. Lett. 2012, 108, 107204. [Google Scholar] [CrossRef] [PubMed]
  21. Cornia, A.; Taham, D.; Affronte, M. Thin Layers of Molecular Nanomagnets Chapter in Book Molecular Magnetic Materials. Concepts and Applications; Sieklucka, B., Pinkowicz, D., Eds.; Wiley: Hoboken, NJ, USA, 2016. [Google Scholar]
  22. Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
  23. Ding, Y.-S.; Deng, Y.-F.; Zheng, Y.-Z. The Rise of Single-Ion Magnets as Spin Qubits. Magnetochemistry 2016, 2, 40. [Google Scholar] [CrossRef]
  24. Troiani, F.; Bellini, V.; Candini, A.; Lorusso, G.; Affronte, M. Spin Entanglement in supramolecular structures. Nanotechnology 2010, 21, 274009. [Google Scholar] [CrossRef] [PubMed]
  25. Timco, G.A.; Carretta, S.; Troiani, F.; Tuna, F.; Pritchard, R.J.; McInnes, E.J.L.; Ghirri, A.; Candini, A.; Santini, P.; Amoretti, G.; et al. Engineering coupling between molecular spin qubits by coordination chemistry. Nat. Nanotechnol. 2009, 4, 173–178. [Google Scholar] [CrossRef] [PubMed]
  26. Candini, A.; Lorusso, G.; Troiani, F.; Ghirri, A.; Carretta, S.; Santini, P.; Amoretti, G.; Muryn, C.; Tuna, F.; Timco, G.; et al. Entanglement in supramolecular spin systems of two weakly coupled antiferromagnetic rings (purple Cr7Ni). Phys. Rev. Lett. 2010, 104, 037203. [Google Scholar] [CrossRef] [PubMed]
  27. Troiani, F.; Affronte, M.; Carretta, S.; Santini, P.; Amoretti, G. Proposal for Quantum-gate in permanently coupled AF spin rings, without need of local fields. Phys. Rev. Lett. 2005, 94, 190501. [Google Scholar] [CrossRef] [PubMed]
  28. Chiesa, A.; Whitehead, G.F.S.; Carretta, S.; Carthy, L.; Timco, G.A.; Teat, S.J.; Amoretti, G.; Pavarini, E.; Winpenny, R.E.P.; Santini, P. Molecular nanomagnets with switchable coupling for quantum simulation. Sci. Rep. 2014, 4, 7423. [Google Scholar] [CrossRef] [PubMed]
  29. Toyli, D.M.; Weis, C.D.; Fuchs, G.D.; Schenkel, T.; Awschalom, D.D. Chip-scale nanofabrication of single spins and spin arrays in diamond. Nano Lett. 2010, 10, 3168–3172. [Google Scholar] [CrossRef] [PubMed]
  30. Dolde, F.; Bergholm, V.; Wang, Y.; Jakobi, I.; Naydenov, B.; Pezzagna, S.; Meijer, J.; Jelezko, F.; Neumann, P.; Schulte-Herbruggen, T.; et al. High-fidelity spin entanglement using optimal control. Nat. Commun. 2014, 5, 3371. [Google Scholar] [CrossRef] [PubMed]
  31. Aguila, D.; Barrios, L.A.; Velasco, V.; Roubeau, O.; Repollés, A.; Alonso, P.J.; Sesé, J.; Teat, S.J.; Luis, F.; Aromí, G. Heterodimetallic [LnLn′] Lanthanide Complexes: Toward a Chemical Design of Two-Qubit Molecular Spin Quantum Gates. J. Am. Chem. Soc. 2014, 136, 14215–14222. [Google Scholar] [CrossRef] [PubMed]
  32. Nakazawa, S.; Nishida, S.; Ise, T.; Yoshino, T.; Mori, N.; Rahimi, R.D.; Sato, K.; Morita, Y.; Toyota, K.; Shiomi, D.; et al. A Synthetic Two-Spin Quantum Bit: G-Engineered Exchange-Coupled Biradical Designed for Controlled-NOT Gate Operations. Angew. Chem. Int. Ed. 2012, 51, 9860–9864. [Google Scholar] [CrossRef] [PubMed]
  33. Ferrando-Soria, J.; Pineda, E.M.; Chiesa, A.; Fernandez, A.; Magee, S.A.; Carretta, S.; Santini, P.; Vitorica-Yrezabal, I.J.; Tuna, F.; Timco, G.A.; et al. A modular design of molecular qubits to implement universal quantum gates. Nat. Commun. 2016, 7, 11377. [Google Scholar] [CrossRef] [PubMed]
  34. Baker, M.L.; Blundell, S.J.; Domingo, N.; Hill, S. Spectroscopy Methods for Molecular Nanomagnets. In Molecular Nanomagnets and Related Phenomena; Gao, S., Ed.; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
  35. Du, J.; Rong, X.; Zhao, N.; Wang, Y.; Yang, J.; Liu, J.R.B. Preserving electron spin coherence in solids by optimal dynamical decoupling. Nature 2009, 461, 1265–1268. [Google Scholar] [CrossRef] [PubMed]
  36. Sato, K.; Nakazawa, S.; Rahimi, R.; Ise, T.; Nishida, S.; Yoshino, T.; Mori, N.; Toyota, K.; Shiomi, D.; Yakiyama, Y.; et al. Molecular electron-spin quantum computers and quantum information processing: pulse-based electron magnetic resonance spin technology applied to matter spin-qubits. Mater. Chem. 2009, 19, 3739–3754. [Google Scholar] [CrossRef]
  37. Santini, P.; Carretta, S.; Troiani, F.; Amoretti, G. Molecular Nanomagnets as Quantum Simulators. Phys. Rev. Lett. 2011, 107, 230502. [Google Scholar] [CrossRef] [PubMed]
  38. Chiesa, A.; Santini, P.; Carretta, S. Supramolecular Complexes for Quantum Simulation. Magnetochemistry 2016, 2, 37. [Google Scholar] [CrossRef]
  39. Cleuziou, J.-P.; Wernsdorfer, W.; Bouchiat, V.; Ondarcuhu, T.; Monthioux, M. Carbon nanotube superconducting quantum interference device. Nat. Nanotechnol. 2006, 1, 53–59. [Google Scholar] [CrossRef] [PubMed]
  40. Brede, J.; Chilian, B.; Khajetoorians, A.A.; Wiebe, J.; Wiesendanger, R. Atomic-Scale Spintronics. In Handbook of Spintronics; Xu, Y., Awschalom, D., Nitta, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
  41. Komeda, T.; Isshiki, H.; Liu, J.; Zhang, Y.-F.; Lorente, N.; Katoh, K.; Breedlove, B.K.; Yamashita, M. Observation and electric current control of a local spin in a single-molecule magnet. Nat. Commun. 2011, 2, 217. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  42. Müllegger, S.; Tebi, S.; Das, A.K.; Schöfberger, W.; Faschinger, F.; Koch, R. Radio Frequency Scanning Tunneling Spectroscopy for Single-Molecule Spin Resonance. Phys. Rev. Lett. 2014, 113, 133001. [Google Scholar] [CrossRef] [PubMed]
  43. Baumann, S.; Paul, W.; Choi, T.; Lutz, K.P.; Ardavan, A.; Heinrich, A.J. Electron paramagnetic resonance of individual atoms on a surface. Science 2015, 350, 417. [Google Scholar] [CrossRef] [PubMed]
  44. Zyazin, A.S.; van den Berg, J.W.G.; Osorio, E.A.; van der Zant, H.S.J.; Konstantinidis, N.P.; Leijnse, M.; Wegewijs, M.R.; May, F.; Hofstetter, W.; Danieli, C.; et al. Electric Field Controlled Magnetic Anisotropy in a Single Molecule. Nano Lett. 2010, 10, 3307–3311. [Google Scholar] [CrossRef] [PubMed]
  45. Burgess, J.A.J.; Malavolti, L.; Lanzilotto, V.; Mannini, M.; Yan, S.; Ninova, S.; Totti, F.; Rolf-Pissarczyk, S.; Cornia, A.; Sessoli, R.; et al. Magnetic fingerprint of individual Fe4 molecular magnets under compression by a scanning tunnelling microscope. Nat. Commun. 2015, 6, 8216. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  46. Vincent, R.; Klyatskaya, S.; Ruben, M.; Wernsdorfer, W.; Balestro, F. Electronic read-out of a single nuclear spin using a molecular spin transistor. Nature 2012, 488, 357–360. [Google Scholar] [CrossRef] [PubMed]
  47. Gaudenzi, R.; Burzurí, E.; Reta, D.; de P.R. Moreira, I.; Bromley, S.T.; Rovira, C.; Veciana, J.; van der Zant, H.S.J. Exchange Coupling Inversion in a High-Spin Organic Triradical Molecule. Nano Lett. 2016, 16, 2066–2071. [Google Scholar] [CrossRef] [PubMed]
  48. Urdampilleta, M.; Klyatskaya, S.; Cleuziou, J.P.; Ruben, M.; Wernsdorfer, W. Supramolecular spin valves. Nat. Mater. 2011, 10, 502–506. [Google Scholar] [CrossRef] [PubMed]
  49. Candini, A.; Klyatskaya, S.; Ruben, M.; Wernsdorfer, W.; Affronte, M. Graphene Spintronic Devices with Molecular Nanomagnets. Nano Lett. 2011, 11, 2634–2639. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  50. Urdampilleta, M.; Klayatskaya, S.; Ruben, M.; Wernsdorfer, W. Magnetic Interaction between a Radical Spin and a Single-Molecule Magnet in a Molecular Spin-Valve. ACS Nano 2015, 9, 4458–4464. [Google Scholar] [CrossRef] [PubMed]
  51. Lumetti, S.; Candini, A.; Godfrin, C.; Balestro, F.; Wernsdorfer, W.; Klyatskaya, S.; Ruben, M.; Affronte, M. Single-molecule devices with graphene electrodes. Dalton Trans. 2016, 45, 16570–16574. [Google Scholar] [CrossRef] [PubMed]
  52. Thiele, S.; Vincent, R.; Holzmann, M.; Klyatskaya, S.; Ruben, M.; Balestro, F.; Wernsdorfer, W. Electrical Readout of Individual Nuclear Spin Trajectories in a Single-Molecule Magnet Spin Transistor. Phys. Rev. Lett. 2013, 111, 037203. [Google Scholar] [CrossRef] [PubMed]
  53. Thiele, S.; Balestro, F.; Ballou, R.; Klyatskaya, S.; Ruben, M.; Wernsdorfer, W. Electrically driven nuclear spin resonance in single-molecule magnets. Science 2014, 344, 1135–1138. [Google Scholar] [CrossRef] [PubMed]
  54. Pla, J.J.; Tan, K.Y.; Dehollain, J.P.; Lim, W.H.; Morton, J.J.L.; Jamieson, D.N.; Dzurak, A.S.; Morello, A. A single-atom electron spin qubit in silicon. Nature 2012, 489, 541–545. [Google Scholar] [CrossRef] [PubMed]
  55. Veldhorst, M.; Yang, C.H.; Hwang, J.C.C.; Huang, W.; Dehollain, J.P.; Muhonen, J.T.; Simmons, S.; Laucht, A.; Hudson, F.E.; Itoh, K.M.; et al. A two-qubit logic gate in silicon. Nature 2015, 526, 410–414. [Google Scholar] [CrossRef] [PubMed]
  56. Jelezko, F.; Gaebel, T.; Popa, I.; Domhan, M.; Gruber, A.; Wrachtrup, J. Observation of Coherent Oscillation of a Single Nuclear Spin and Realization of a Two-Qubit Conditional Quantum Gate. Phys. Rev. Lett. 2004, 93, 130501. [Google Scholar] [CrossRef] [PubMed]
  57. Waldherr, G.; Wang, Y.; Zaiser, S.; Jamali, M.; Schulte-Herbrüggen, T.; Abe, H.; Ohshima, T.; Isoya, J.; Du, J.F.; Neumann, P.; et al. Quantum error correction in a solid-state hybrid spin register. Nature 2014, 506, 204–207. [Google Scholar] [CrossRef] [PubMed]
  58. Leuenberger, M.N.; Loss, D. Quantum Computing in Molecular Nanomagnets. Nature 2001, 410, 789–793. [Google Scholar] [CrossRef] [PubMed]
  59. Kubo, Y.; Ong, F.R.; Bertet, P.; Vion, D.; Jacques, V.; Zheng, D.; Dreau, A.; Roch, J.F.; Auffeves, A.; Jelezko, F.; et al. Strong Coupling of a Spin Ensemble to a Superconducting Resonator. Phys. Rev. Lett. 2010, 105, 140502. [Google Scholar] [CrossRef] [PubMed]
  60. Probst, S.; Rotzinger, H.; Wunsch, S.; Jung, P.; Jerger, M.; Siegel, M.; Ustinov, A.V.; Bushev, P.A. Anisotropic Rare-Earth Spin Ensemble Strongly Coupled to a Superconducting Resonator. Phys. Rev. Lett. 2013, 110, 157001. [Google Scholar] [CrossRef] [PubMed]
  61. Ghirri, A.; Bonizzoni, C.; Gerace, D.; Sanna, S.; Cassinese, A.; Affronte, M. YBCO microwave resonators for strong collective coupling with spin ensemble. Appl. Phys. Lett. 2015, 106, 184101. [Google Scholar] [CrossRef] [Green Version]
  62. Ghirri, A.; Bonizzoni, C.; Troiani, F.; Buccheri, N.; Beverina, L.; Cassinese, A.; Affronte, M. Coherently coupling distinct spin ensembles through a high-Tc superconducting resonator. Phys. Rev. A 2016, 93, 063855. [Google Scholar] [CrossRef]
  63. Bonizzoni, C.; Ghirri, A.; Bader, K.; van Slageren, J.; Perfetti, M.; Sorace, L.; Lan, Y.; Fuhr, O.; Ruben, M.; Affronte, M. Coupling molecular spin centers to microwave planar resonators: towards integration of molecular qubits in quantum circuits. Dalton Trans. 2016, 45, 16596–16603. [Google Scholar] [CrossRef] [PubMed]
  64. Jenkins, M.D.; Zueco, D.; Roubeau, O.; Aromí, G.; Majer, J.; Luis, F. A scalable architecture for quantum computation with molecular nanomagnets. Dalton Trans. 2016, 45, 16682–16693. [Google Scholar] [CrossRef] [PubMed]
  65. Bienfait, A.; Pla, J.J.; Kubo, Y.; Stern, M.; Zhou, X.; Lo, C.C.; Weis, C.D.; Schenkel, T.; Thewalt, M.L.W.; Vion, D.; et al. Reaching the quantum limit of sensitivity in electron spin resonance. Nat. Nanotechnol. 2015, 11, 253–257. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  66. Trif, M.; Troiani, F.; Stepanenko, D.; Loss, D. Spin-Electric Coupling in Molecular Magnets. Phys. Rev. Lett. 2008, 101, 217201. [Google Scholar] [CrossRef] [PubMed]
  67. George, R.E.; Edwards, J.P.; Ardavan, A. Coherent Spin Control by Electrical Manipulation of the Magnetic Anisotropy. Phys. Rev. Lett. 2013, 110, 027601. [Google Scholar] [CrossRef] [PubMed]
  68. Carretta, S.; Chiesa, A.; Troiani, F.; Gerace, D.; Amoretti, G.; Santini, P. Quantum Information Processing with Hybrid Spin-Photon Qubit Encoding. Phys. Rev. Lett. 2013, 111, 110501. [Google Scholar] [CrossRef] [PubMed]
  69. Grezes, C.; Julsgaard, B.; Kubo, Y.; Stern, M.; Umeda, T.; Isoya, J.; Sumiya, H.; Abe, H.; Onoda, S.; Ohshima, T.; et al. Multimode Storage and Retrieval of Microwave Fields in a Spin Ensemble. Phys. Rev. X 2014, 4, 021049. [Google Scholar] [CrossRef]
Magnetochemistry 03 00012 g001 550
Figure 1. Coherence time T2 and relaxation time T1 as a function of the NV depth in diamond. The plot shows strong suppression of coherence for shallow NV centers. The lower panel shows T2;XY4 = T2echo ratio that is reduced as well with decreased depth, also indicating that dynamical decoupling with N = 4 pulses is less efficient for shallower NVs (reproduced from [18] with permission from the American Physical Society).
Figure 1. Coherence time T2 and relaxation time T1 as a function of the NV depth in diamond. The plot shows strong suppression of coherence for shallow NV centers. The lower panel shows T2;XY4 = T2echo ratio that is reduced as well with decreased depth, also indicating that dynamical decoupling with N = 4 pulses is less efficient for shallower NVs (reproduced from [18] with permission from the American Physical Society).
Magnetochemistry 03 00012 g001
Magnetochemistry 03 00012 g002 550
Figure 2. Molecular spin transistor made by graphene electrodes and a TbPc2 molecule. In this case graphene-based electrodes are used to contact the TbPc2 molecule (reproduced from [51] with permission from the Consiglio Nazionale delle Ricerche (CNR) and The Royal Society of Chemistry).
Figure 2. Molecular spin transistor made by graphene electrodes and a TbPc2 molecule. In this case graphene-based electrodes are used to contact the TbPc2 molecule (reproduced from [51] with permission from the Consiglio Nazionale delle Ricerche (CNR) and The Royal Society of Chemistry).
Magnetochemistry 03 00012 g002
Magnetochemistry 03 00012 g003 550
Figure 3. Scheme of a functioning for molecular spin transistor with separate quantum dot (Pc radical) in which charge can tunnel from electrodes, the electron magnetic moment J = 6 and nuclear spin (I = 3/2) of the Tb3+ ion (reproduced from [54] with permission from The American Association for the Advancement of Science).
Figure 3. Scheme of a functioning for molecular spin transistor with separate quantum dot (Pc radical) in which charge can tunnel from electrodes, the electron magnetic moment J = 6 and nuclear spin (I = 3/2) of the Tb3+ ion (reproduced from [54] with permission from The American Association for the Advancement of Science).
Magnetochemistry 03 00012 g003
Magnetochemistry 03 00012 g004 550
Figure 4. Artistic view of molecular spin coupled to planar superconducting resonators.
Figure 4. Artistic view of molecular spin coupled to planar superconducting resonators.
Magnetochemistry 03 00012 g004
Magnetochemistry 03 00012 g005 550
Figure 5. Coupling strength against spin linewidth parameters as measured in different molecular spins systems. Results can be compared with those obtained with NV centers and Er spin defects in YSiO or YAlO. The parameters of DPPH and PyBTM organic radicals are taken from [62,63], respectively. Data taken at different temperatures are indicated by the black arrows and by symbols of different sizes that range from 2 K (larger symbols) to 50 K (smaller symbols). Empty symbols display the parameters extrapolated to 0.3 K. The dashed line represent the threshold above which high cooperative regime is achieved. Dashed rectangles show the typical working ranges used for NV centers (blue) and Er spin centers (green) coupled to superconducting Nb planar resonators at mK temperature region. (Reproduced from [63] with permission from the Consiglio Nazionale delle Ricerche (CNR) and The Royal Society of Chemistry.)
Figure 5. Coupling strength against spin linewidth parameters as measured in different molecular spins systems. Results can be compared with those obtained with NV centers and Er spin defects in YSiO or YAlO. The parameters of DPPH and PyBTM organic radicals are taken from [62,63], respectively. Data taken at different temperatures are indicated by the black arrows and by symbols of different sizes that range from 2 K (larger symbols) to 50 K (smaller symbols). Empty symbols display the parameters extrapolated to 0.3 K. The dashed line represent the threshold above which high cooperative regime is achieved. Dashed rectangles show the typical working ranges used for NV centers (blue) and Er spin centers (green) coupled to superconducting Nb planar resonators at mK temperature region. (Reproduced from [63] with permission from the Consiglio Nazionale delle Ricerche (CNR) and The Royal Society of Chemistry.)
Magnetochemistry 03 00012 g005

Share and Cite

MDPI and ACS Style

Ghirri, A.; Candini, A.; Affronte, M. Molecular Spins in the Context of Quantum Technologies. Magnetochemistry 2017, 3, 12. https://doi.org/10.3390/magnetochemistry3010012

AMA Style

Ghirri A, Candini A, Affronte M. Molecular Spins in the Context of Quantum Technologies. Magnetochemistry. 2017; 3(1):12. https://doi.org/10.3390/magnetochemistry3010012

Chicago/Turabian Style

Ghirri, Alberto, Andrea Candini, and Marco Affronte. 2017. "Molecular Spins in the Context of Quantum Technologies" Magnetochemistry 3, no. 1: 12. https://doi.org/10.3390/magnetochemistry3010012

APA Style

Ghirri, A., Candini, A., & Affronte, M. (2017). Molecular Spins in the Context of Quantum Technologies. Magnetochemistry, 3(1), 12. https://doi.org/10.3390/magnetochemistry3010012

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop